Abstract
The human proximal tibiofibular joint (PTFJ) and its relationship to overall knee joint mechanics have been largely unexplored. This study describes force/displacement data from experiments done on four human cadaveric knee specimens and general conclusions obtained with the help of a statistical modeling technique. Specimens were rigidly affixed at the tibia to a force plate and the femur was attached to a custom made device allowing for manual load application. Motion of the fibular head was tracked relative to the tibial plateau by means of reflective markers and a high speed digital camera synchronized with the force plate data stream. Each specimen was subjected to a range of loading conditions and a quadratic regression model was created and then used to predict the specimen's response to standardized loading conditions and compare these across specimens. Statistical analysis was performed with a three-factor analysis of variance with repeated measures.
Proximal tibiofibular joint motion was largest in the anterior-posterior direction with translations of 1–3 mm observed during a range of physiological loading conditions. The applied internal-external rotation moment had a significant effect on proximal tibiofibular joint translation (P < 0.05). Effects of varus-valgus loading and flexion angle were seen in some specimens. This study demonstrates that substantial proximal tibiofibular joint motion can occur in physiologic loading states. Preservation of proximal tibiofibular joint function, and anatomical variations which affect this function, may need to be considered when designing surgical procedures for the knee joint.
Keywords: fibula, human biomechanics, tibiofibular joint
Introduction
The proximal tibiofibular joint (PTFJ) is a synovial membrane-lined, hyaline cartilage articulation. The joint capsule is comprised of a thick anterior capsule, the anterior proximal tibiofibular ligament, and a thinner posterior capsule, the posterior proximal tibiofibular ligament. The PTFJ is the site of attachment of numerous structures which help stabilize the tibiofemoral joint. These include the fibular collateral ligament (FCL), the capsular arm of the short head of the biceps femoris, the fabellofibular ligament, the popliteofibular ligament and the popliteus muscle (Terry & LaPrade, 1996). In addition, the biceps femoris tendon and the popliteus muscle insert onto the styloid process of the proximal fibula.
Previous studies have focused on the characterization of the morphology of the PTFJ (Ogden, 1974; Eichenblat & Nathan 1983; Bozkurt et al. 2003; de Seze et al. 2005). Various shapes of the PTFJ have been characterized. The most frequently encountered types were the planar, trochoid and double trochoid shapes which account for 33%, 30% and 23% respectively (Eichenblat & Nathan, 1983). Two arbitrary variants of the PTFJ with regards to joint inclination have been characterized. One variant is horizontal and has less than 20° of joint inclination relative to the horizontal plane. The surface area of the horizontal variant is planar, circular and has an average area of around 26 mm2. In contrast, the oblique variant has an inclination of greater than 20° and has an average surface area of roughly 17 mm2 (Eichenblat & Nathan, 1983).
There is a paucity of literature concerning the biomechanical functions of the PTFJ. The tibia and fibula move relative to one another at the PTFJ with coupled motion through the interosseus membrane and the distal tibiofibular syndesmosis (Ogden, 1974; Eichenblat & Nathan, 1983; Bozkurt et al. 2003; de Seze et al. 2005). The proximal fibula is known to externally rotate at the proximal tibiofibular joint during ankle dorsiflexion (Ogden, 1974; Eichenblat & Nathan, 1983; Bozkurt et al. 2003; de Seze et al. 2005). One of the functions of the PTFJ is believed to be the dissipation of torsional stresses applied at the ankle joint (Lambert, 1971). Other literature exists which supports the premise that the proximal fibula mainly bears a tensional force rather than a compressive one (Preuschoft, 1972). Studies have also observed increased rotation of the fibula at the PTFJ in horizontal variants versus the oblique variants (Ogden, 1974). In addition to rotational movement of the fibula relative to the tibia, the fibular head moves in the anterior-posterior plane as a function of knee flexion (Ogden, 1974; Andersen, 1985). As the knee flexes, the proximal fibula moves anteriorly with relative relaxation of the fibular collateral ligament and the biceps femoris while with knee extension, these structures become taut and pull the fibula posteriorly (Ogden, 1974; Andersen, 1985).
A recent study has shown high relative loads on the FCL with varus and external tibial rotation, and on the popliteus tendon and popliteofibular ligament with external rotation depending on the knee flexion angle (LaPrade et al. 2004). We hypothesize that these same loading conditions could cause motion in the PTFJ. Due to the nature of the syndesmosis, non-physiologic motion in this joint may contribute to the development of posterolateral knee pain. Here we present a descriptive study showing the displacement of the PTFJ under varying loading conditions and a technique utilizing a quadratic regression model enabling us to simulate other loading conditions.
Methods
Data collection
Four non-paired, fresh-frozen, male cadaveric knee specimens all between the ages of 70–85 years were tested with the knee joint fully intact. The specimens were thawed to room temperature and the foot was removed so that the leg could be attached to the fixator. A custom fixator was designed so that the distal fibular connections to the tibia and talus and interosseous membrane could remain undisturbed during dissection and subsequent testing. The tibia was mounted vertically to a custom designed fixator with three bicortical cannulated surgical titanium bone screws and then fixed to a six degree of freedom force/torque sensor (SI-2500-400, ATI Industrial Automation, Apex, NC, USA) mounted rigidly to the floor. A custom designed device was then attached rigidly to the femur allowing for attachment of a ‘handle bar’ device by which the experimenter could manually apply forces and torques to the knee joint (Fig. 1). In addition, the femoral jig was equipped with adjustable attachment points to facilitate the application of compressive loads via weights. These attachment points were aligned as closely as possible to the knee joint center in order to minimize their contribution to other loading conditions. Load cell data were sampled at 10 Hz and converted to a tibial coordinate system to represent forces and torques at the knee joint center (Blankevoort et al. 1988). Two reflective markers were mounted on pins driven into the tibial plateau and the head of the fibula at the proximal tibiofibular joint. Motion was recorded by a video camera (model CL-C3, DALSA Corp., Waterloo, Ontario, Canada) and Epix FrameGrabber software (EPIX Inc., Buffalo Grove, IL, USA) at 10 frames per second. Using custom software and the image analysis toolbox from MATLAB (Mathworks Inc., Natick, MA, USA), the 10 Hz video data was manually digitized and transformed into a data set consisting of displacements of the fibular head relative to the tibial plateau, in both the anterior-posterior and proximo-distal directions. Load cell data was synchronized to the video data by tapping the specimen with a rubber hammer at the start of each trial, which allowed synchronization within 0.1 s.
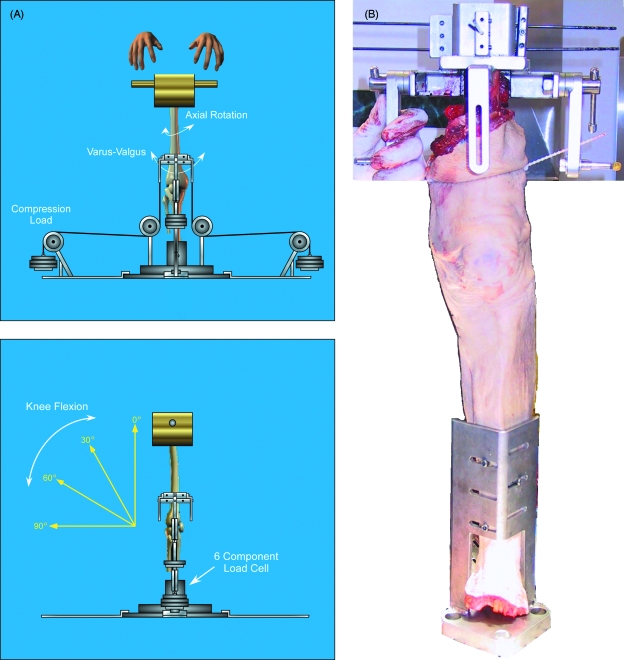
Fig. 1.
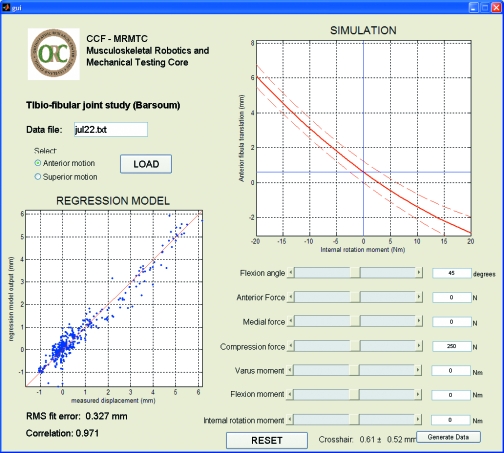
(A)Schematic representation of our experimental apparatus, showing a frontal view (top) and lateral view (bottom) of a right knee joint. Mechanical loads are applied to the femur through a combination of weights and manipulation. Three dimensional forces and moments are recorded continuously by a load cell at the tibia fixture and converted by software into a tibial reference frame with its origin at the knee joint center. (B) Detail of the fixator for attachment of the tibia to the load cell. Skin and soft tissue between tibia and fibula remained intact.
The knee joint was then manually subjected to continuously varying loading conditions at four successive flexion angles: 0, 30, 60 and 90°, as established by a manual goniometer. Varying loads were applied manually to a ‘handlebar’ on the femur, in combination with weights to generate a baseline of compressive loading (Mizuno et al. 2004). At each flexion angle, the specimen was slowly moved for 10–15 s, including phases of internal rotation, external rotation, varus, and valgus. In each direction, load was slowly increased until a distinct endpoint of the range of motion was felt. The load cell data was transformed in real time to varus-valgus and internal-external rotation moments and visualized on the computer screen. This allowed the experimenter to ensure that these had reached a magnitude of well over 10 Nm. Because of the manual load application, it was not possible to apply well controlled loading conditions, but sufficient data was collected to allow regression modeling and interpolate the raw data to specific controlled loading states.
After testing and data analysis, it was decided to not include the 90° data sets in our analysis. This decision was made due to the fact that there was significant instability in the joints at this flexion angle during the loading conditions applied, and the subsequent joint subluxations and reductions were deemed to be deleterious to both the ligament material properties and the data set. Since the 90° data were collected last in each specimen, this did not affect the other results.
Data reduction
Loads applied to the knee joint were not controlled, and this required a data reduction step in order to interpolate the data and obtain the dependent variables (PTFJ translation) at specific loading conditions. Data from each specimen were entered into custom MATLAB software (Fig. 2) to obtain a second order regression model for PTFJ motion as a function of the tibiofemoral joint loading state:
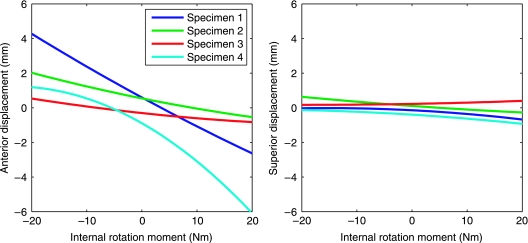
Fig. 2.
A representative output screen from one specimen's regression modeling. The MATLAB program performed the regression modeling and allowed interactive exploration of the relationships between joint motion and any of the seven independent variables. The software also allowed sampling of the regression model at specific loading conditions in order to compare joint mechanics across specimens.
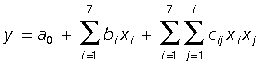 |
where y is the dependent variable (anterior-posterior or inferior-superior PTFJ motion) and x1–7 are the independent variables: flexion angle, 3-D tibiofemoral force vector, and 3-D tibiofemoral torque vector. The validity of these regression models, for each specimen and each dependent variable, was quantified via the root-mean-square (RMS) fit error and the correlation coefficient between measured and modeled response.
After a regression model was created for each specimen, it was used to predict PTFJ motion in 18 specific physiological loading conditions: combinations of varus and internal tibial rotation torques (10, 0 and –10 Nm), combined with 250 N compression, at 15° and 45° flexion. The torque values are representative of gait (Bellchamber & van den Bogert, 2000; Moisio et al. 2003) while the flexion angles represent typical values for the stance phase of gait (15°) and stair climbing (45°). The compressive loading represents partial weightbearing.
Statistical analysis
Means and standard deviations were calculated across specimens for each loading condition for descriptive purposes. Analysis of variance with repeated measures was used to test for main effects and two-factor interactions of varus-valgus moment (3 levels: –10, 0, 10 Nm), internal-external rotation moment (3 levels: –10, 0, 10 Nm), and flexion angle (2 levels: 15, 45°), with specimen as a random factor. Statistical significance was defined as P < 0.05.
Results
In all specimens, the quadratic regression model was able to fit the measured data well. The RMS fit error ranged from 0.11–0.35 mm, and the correlations of the models ranged from 0.785–0.971 (Table 1).
Table 1.
Results of model fitting for each specimen
| Anterior translation | Superior translation | |||
|---|---|---|---|---|
| RMS fit error (mm) | Correlation coefficient | RMS fit error (mm) | Correlation coefficient | |
| Specimen 1 | 0.325 | 0.952 | 0.173 | 0.785 |
| Specimen 2 | 0.315 | 0.975 | 0.108 | 0.925 |
| Specimen 3 | 0.348 | 0.967 | 0.12 | 0.882 |
| Specimen 4 | 0.173 | 0.941 | 0.104 | 0.876 |
Substantial tibiofibular joint motion was observed in all specimens, mainly along the anterior-posterior axis. Translation was mainly influenced by internal-external rotation torque. The fibula translated anteriorly when the tibia was externally rotated, and posteriorly when the tibia was internally rotated (Fig. 3).
Fig. 3.
Translational motion in the proximal tibiofibular joint as a function of internal and external rotation torques at 30° of knee flexion and with a 250 N compressive load.
Descriptive statistics for PTFJ translation in the 18 specific combined loading conditions are shown in Table 2. The greatest motion was seen in combination loading of varus and external tibial rotation at all flexion angles. For anterior-posterior PTFJ motion, the effect of internal-external rotation was statistically significant (P = 0.016), and there were significant specimen interactions with internal-external rotation (P < 0.0001) and flexion (P = 0.003) which suggests that there are substantial differences between specimens. For inferior-superior PTFJ motion, there were no significant main effects, but there were significant specimen interactions with flexion (P = 0.0007), varus (P = 0.014), and internal rotation (P = 0.004), indicating that these loading variables had effects that were consistent within each specimen, but different between specimens.
Table 2.
Translational motion in the proximal tibiofibular joint at specific loading conditions, mean and standard deviation (N = 4) in mm. Each loading condition also included 250 N of compressive loading. Positive torques indicate varus and internal rotation of tibia relative to femur, negative torques indicate valgus and external rotation
| Anterior translation (mm) in PTFJ at 15° knee flexion | |||
|---|---|---|---|
| 10 Nm external rotation | Neutral | 10 Nm internal rotation | |
| 10 Nm valgus | 1.11 ± 0.75 | –0.15 ± 0.58 | –1.43 ± 1.25 |
| Neutral | 1.25 ± 0.59 | 0.16 ± 0.58 | –0.96 ± 1.26 |
| 10 Nm varus | 1.51 ± 0.66 | 0.58 ± 0.71 | –0.38 ± 1.40 |
| Anterior translation (mm) in PTFJ at 45° knee flexion | |||
| 10 Nm external rotation | Neutral | 10 Nm internal rotation | |
| 10 Nm valgus | 0.85 ± 1.54 | –0.38 ± 0.73 | –1.63 ± 1.60 |
| Neutral | 0.90 ± 1.55 | –0.17 ± 0.96 | –1.25 ± 1.69 |
| 10 Nm varus | 1.07 ± 1.65 | 0.17 ± 1.32 | –0.76 ± 2.02 |
| Superior translation (mm) in PTFJ at 15° knee flexion | |||
| 10 Nm external rotation | Neutral | 10 Nm internal rotation | |
| 10 Nm valgus | 0.18 ± 0.16 | 0.05 ± 0.03 | –0.11 ± 0.10 |
| Neutral | 0.12 ± 0.14 | –0.01 ± 0.13 | –0.17 ± 0.18 |
| 10 Nm varus | 0.12 ± 0.17 | 0.00 ± 0.29 | –0.15 ± 0.47 |
| Superior translation (mm) in PTFJ at 45° knee flexion | |||
| 10 Nm external rotation | Neutral | 10 Nm internal rotation | |
| 10 Nm valgus | –0.05 ± 0.32 | –0.16 ± 0.26 | –0.31 ± 0.34 |
| neutral | 0.00 ± 0.33 | –0.11 ± 0.33 | –0.24 ± 0.52 |
| 10 Nm varus | 0.12 ± 0.34 | 0.02 ± 0.43 | –0.12 ± 0.77 |
Discussion
Anterior and posterior translation of the fibular head relative to the tibia was consistently seen when external and internal rotation moments were applied to the tibia. This can be explained from the functional anatomy of the joint. The fibular collateral ligament (FCL) transmits force to the head of the fibula. In external tibia rotation, this tensile force vector is oriented anteriorly, causing anterior motion of the head of the fibula, and vice versa for internal rotation. LaPrade et al. (2004) quantified the force in the FCL and found it to be largest in external rotation and varus loading, suggesting that varus load should have an effect on PTFJ motion as well. Our results (Table 2) suggest larger anterior displacement with varus load, but not a larger posterior displacement. Consequently there was no significant interaction between varus-valgus and rotational loads.
In pure varus loading, there was substantial PTFJ motion, both in anterior-posterior and proximo-distal direction. However, not all specimens moved in the same direction, as shown by the large standard deviation between specimens, relative to the mean (Table 2). The statistical analysis confirmed this by showing highly significant interaction between varus-valgus load and specimen. We suspect that in a pure varus loading condition, the motion of the PTFJ is sensitive to joint geometry, specifically the orientation of the articular surface of the PTFJ. When the surface lies anterior, varus load would cause an anterior translation of the fibular head, and vice versa. Quantification of articular surface orientation should be considered in future studies.
The study included only four specimens and we made no attempt to classify their anatomy according to Eichenblatt & Nathan (1983). Based on our finding of significant interactions between loading and specimen, we suspect that the number of specimens is far too small to represent the relevant anatomical variations in the general population. The effects of those loading factors that had interactions with specimen can not be generalized to a broader population. Future studies may attempt to identify subgroups in which these effects are consistent. However, we are confident that the effect of internal-external rotation, which was significant without interactions and also makes sense mechanically, has general validity.
In this study we considered only a specific set of 18 loading conditions, which may not include certain clinically relevant conditions. Patients with osteoarthritis often have substantially larger varus moments at the knee during gait (Mundermann et al. 2004). The levels of 10 Nm that we used are typical for cadaveric protocols for knee joint mechanics (Kanamori et al. 2002) because they are well below the levels that can cause injury to ligaments or cartilage. In order to prevent the risk of failure of the specimen fixation, it was not possible to consistently apply compressive loads that represent full weightbearing. It was decided to avoid the risk of extrapolating the regression model to load levels not seen during the experiment, and therefore all statistical analysis was performed at 250 N compression. Future studies need to examine full weightbearing conditions, when geometry of articular surfaces and menisci has a larger effect on tibiofemoral kinematics. No attempt was made to simulate the effect of the quadriceps and other muscles. This would be equivalent to an increase in compressive load, and adding an anterior or posterior component to the applied force. Such questions could be explored in future studies.
These findings have potential clinical relevance. The proximal tibiofibular joint is an articular joint located in the posterolateral corner of the knee that serves as the attachment for numerous tendons and ligaments integral for knee structure and function. Pain in this corner of the knee can be a particularly daunting opponent for both the patient and the clinician as its origins are often difficult to pinpoint. Our study has shown that the proximal tibiofibular joint may need to be considered as a possible source of pain. Translational motion of 1–3 mm was observed during torques and forces that correspond to physiologic motions such as gait and stair climbing. Considering the small size of the joint (less than 10 mm), such translations may correspond to substantial soft tissue strain. Knee joint dysfunction, either because of inherent mechanical instability, traumatic or surgical changes or muscular insufficiency could cause dysfunction in this joint and, subsequently, clinical symptoms. It has been proposed that the fibula can serve as a channel for dissipation of torsional stresses in the ankle (Lambert, 1971), and we propose that this could also be the case for excessive stresses in the knee joint itself. If the knee were to become unbalanced due to ligament injury, or if the PTFJ were to become compromised, this ‘shock absorber’ action could, in turn, be compromised, leaving this function to active stabilizers, including the biceps femoris.
The PTFJ is a joint that has largely been ignored for its contribution to knee joint mechanics. Our study has shown that there is significant motion in this joint during forces and torques consistent with physiologic motion. More studies are needed to explore function and dysfunction of this joint and its possible role in posterolateral knee pain.
Acknowledgments
We acknowledge Stryker Corporation and the U.S. National Institutes of Health (1P30AR050953) for financial support. J.G. Scott was supported in part by NIH T32 Training Grant AR050959.
References
- Andersen K. Dislocation of the superior tibiofibular joint. Injury. 1985;16:494–498. doi: 10.1016/0020-1383(85)90180-9. [DOI] [PubMed] [Google Scholar]
- Bellchamber TL, van den Bogert AJ. Contributions of proximal and distal moments to axial tibial rotation during walking and running. J Biomech. 2000;33(11):1397–1403. doi: 10.1016/s0021-9290(00)00113-5. [DOI] [PubMed] [Google Scholar]
- Blankevoort L, Huiskes R, de Lange A. The envelope of passive knee joint motion. J Biomech. 1988;21(9):705–720. doi: 10.1016/0021-9290(88)90280-1. [DOI] [PubMed] [Google Scholar]
- Bozkurt M, Yilmaz E, Atlihan D, Tekdemir I, Havitcioglu H, Gunal I. The proximal tibiofibular joint: an anatomic study. Clin Orthop Relat Res. 2003;406:136–140. doi: 10.1097/01.blo.0000030167.56585.2f. [DOI] [PubMed] [Google Scholar]
- de Seze MP, Rezzouk J, de Seze M. Anterior innervation of the proximal tibiofibular joint. Surg Radiol Anat. 2005;27(1):30–32. doi: 10.1007/s00276-004-0284-5. [DOI] [PubMed] [Google Scholar]
- Eichenblat M, Nathan H. The proximal tibio fibular joint. An anatomical study with clinical and pathological considerations. Inter Orthop. 1983;7:31–39. doi: 10.1007/BF00267557. [DOI] [PubMed] [Google Scholar]
- Kanamori A, Zeminski J, Rudy TW, Li G, Fu FH, Woo SL. The effect of axial tibial torque on the function of the anterior cruciate ligament: a biomechanical study of a simulated pivot shift test. Arthroscopy: The Journal of Arthroscopic & Related Surgery: Official Publication of the Arthroscopy Association of North America and the International Arthroscopy Association. 2002;18(4):394–398. doi: 10.1053/jars.2002.30638. [DOI] [PubMed] [Google Scholar]
- Lambert KL. The weight-bearing function of the fibula. A strain gauge study. J Bone Joint Surg. 1971;53(3):507–513. American Volume. [PubMed] [Google Scholar]
- LaPrade RF, Tso A, Wentorf FA. Force measurements on the fibular collateral ligament, popliteofibular ligament, and popliteus tendon to applied loads. Am J Sports Med. 2004;32(7):1695–1701. doi: 10.1177/0363546503262694. [DOI] [PubMed] [Google Scholar]
- Mizuno K, Andrish JT, Su A, van den Bogert AJ, McLean SG. In vitro estimation of ACL loads linked to hazardous sporting postures. Orthopaedic Research Society 50th Annual Meeting. paper 240.
- Moisio KC, Sumner DR, Shott S, Hurwitz DE. Normalization of joint moments during gait: a comparison of two techniques. J Biomech. 2003;36:599–603. doi: 10.1016/s0021-9290(02)00433-5. [DOI] [PubMed] [Google Scholar]
- Mundermann A, Dyrby CO, Hurwitz DE, Sharma L, Andriacchi TP. Potential strategies to reduce medial compartment loading in patients with knee osteoarthritis of varying severity: reduced walking speed. Arth Rheum. 2004;50:1172–1178. doi: 10.1002/art.20132. [DOI] [PubMed] [Google Scholar]
- Ogden JA. The anatomy and function of the proximal tibiofibular joint. Clin Orthop Relat Res. 1974;101(101):186–191. [PubMed] [Google Scholar]
- Preuschoft H. Mechanical stress on the fibula of primates. Gegenbaurs morphologisches Jahrbuch. 1972;117(2):211–216. [PubMed] [Google Scholar]
- Terry GC, LaPrade RF. The posterlateral aspect of the knee. Anatomy and surgical approach. Am J Sports Med. 1996;24:732–739. doi: 10.1177/036354659602400606. [DOI] [PubMed] [Google Scholar]