Comparing bird and human soaring strategies

Abstract

Gliding saves much energy, and to make large distances using only this form of flight represents a great challenge for both birds and people. The solution is to make use of the so-called thermals, which are localized, warmer regions in the atmosphere moving upwards with a speed exceeding the descent rate of bird and plane. Whereas birds use this technique mainly for foraging, humans do it as a sporting activity. Thermalling involves efficient optimization including the skilful localization of thermals, trying to guess the most favorable route, estimating the best descending rate, etc. In this study, we address the question whether there are any analogies between the solutions birds and humans find to handle the above task. High-resolution track logs were taken from thermalling falcons and paraglider pilots to determine the essential parameters of the flight patterns. We find that there are relevant common features in the ways birds and humans use thermals. In particular, falcons seem to reproduce the MacCready formula widely used by gliders to calculate the best slope to take before an upcoming thermal.

During long-term gliding, birds and people make use of the so-called thermals, which are spatially and temporally localized parts of the atmosphere typically moving upwards with a speed in the range of 1–5 m/s. After locating it, a glider remains within a thermal by circling until the desired height is attained. Then, a more or less straight advancing, but sinking, phase follows until the next thermal is reached. Paraglider pilots use watching the birds thermalling nearby for finding the next thermal, and sometimes the birds seem to follow the glider (Fig. 1A). Learning about previously unavailable details of this fascinating process can lead us to a better understanding of the main features of flight trajectories and optimization tactics. To locate the best route to a distant point, at least in the case of human gliders who typically use specific devices assisting in making the best decisions, is a complex mental process involving both calculations and intuition. We consider thermalling as one of the scarce examples when an intellectually driven activity of humans is apparently so closely related to the actual behavior of an animal. Several interesting questions emerge: Does the obvious size difference result in a different flight pattern and speed? Are the common tricks the same or are there alternative successful tactics?

Fig. 1.

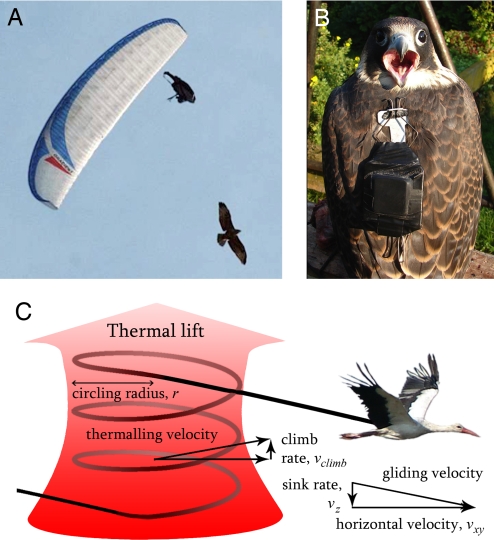
Photos of the observed flyers and the tracking device. (A) A paragliding pilot and a bird of prey thermalling together. (B) Peregrine falcon with the GPS device on its back. (C) Schematic picture of the thermalling and gliding parts of the flights with the notations indicated.

Because collecting data on the soaring flight of birds is a rather difficult task, several techniques have been used for this purpose. A powered sailplane with a camera and ornithodolite techniques were used to determine the polar curves and the circling radius of various birds (1, 2). Gliding of four different bird species was investigated by radar during their migration (3, 4). An altimeter with a satellite transmitter was used in similar studies on the American White pelicans in Nevada (5). A further project demonstrated that the Magnificent frigate bird is thermalling continuously, day and night (6). Since MacCready published his theory about soaring flight optimization (7), sailplane pilots have tried to adjust their gliding speed to the expected thermal climb rate according to their own polar curve p(v_xy) (vertical speed versus the horizontal one, v_xy, during gliding). In this context, the migration flight of Marsh harriers was studied (8). Polar curves of several bird species were measured in wind tunnel studies on trained birds (9). The miniaturization of GPS devices enabled their usage in bird flight research (Fig. 1B). A miniaturized GPS device was used to investigate the navigation strategy of Homing pigeons (10, 11). Very recently, simulations were used to calculate the flight efficiency of the huge volant bird Argentavis magnificiens from the upper Miocene (12), and wind tunnel experiments and modeling were carried out to understand how swifts use morphing wings to control their glide performance (13).

We investigated primarily the soaring flight of the Peregrine falcon (Falco peregrinus), but we also obtained a smaller set of data for the White stork (Ciconia ciconia) to see whether there is a qualitative difference between the two species. Peregrine falcons use the thermals during foraging to soar up to a suitable height from where they can stoop for the prey, and they are able to migrate ≈190 km/day with this soaring technique (14). White storks make great use of thermals during their 10,000-km-long annual migration from the breeding area to the wintering quarters. We assume that both birds and humans are trying to maximize their cross-country speed, although for different reasons. It is beneficial for migrating birds to get to their wintering (breeding) grounds spending a migration period as short as possible (during migration, there is a relatively smaller food supply, and the migrating birds are more vulnerable), whereas in the case of birds of prey, scanning a larger area for a shorter time period is more advantageous. The detailed data we have about humans have been collected from paraglider and hang glider contests where the pilots are aiming at the highest speed possible to win the competition. The trajectories were obtained with the help of an ultra light GPS device providing the three dimensional position data with a high spatial (1 m) and temporal (1 s) resolution (see Materials and Methods), except for the hang gliders for which positions at every 5 seconds could be downloaded from flight contest home pages. Thus, smaller scale details of the flight trajectories could not be obtained for the hang gliders.

Results and Discussion

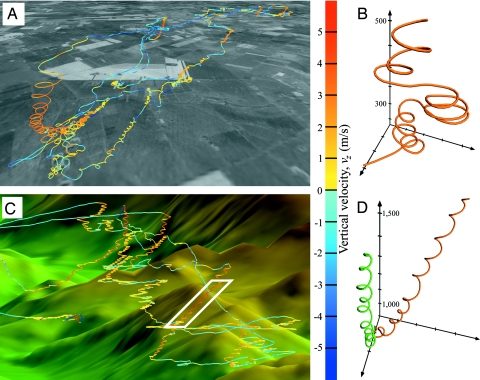
To characterize the thermalling part of the flights (two examples are visualized in Fig. 2 A and C; see also supporting information (SI) Movies 1–4), first their drift due to wind had to be eliminated (Fig. 2D). The horizontal (perpendicular to gravity) circling radius distribution of these wind-removed trajectories was calculated. The most frequent circling radius data were given as the mode value of an Inverse Gaussian function fitted on the circling radius distribution function, where the data of thermalling in clockwise (−) and counterclockwise (+) directions were calculated separately. Table 1 shows the results of the analysis. The circling radius distribution functions indicate that the typical radius values of the soaring flight of the two bird species (falcon and stork) and the paragliders are in the range of 20–26 m, i.e., do not deviate significantly. The same closeness is true for the average velocities, indicating that it is rather the parameters of the representative polar curves (being very similar in the present case) than other features of the flyers that influence the flight patterns of birds and paragliders. Our findings concerning birds are in agreement with the observations and argument by Pennycuick (2), i.e., the mean circling radius is linearly proportional to wing loading. The same proportionality, however, does not hold for the paragliders (see SI Appendix for details); thus, despite the different values of their wing loadings, paraglider pilots and the birds considered fly in roughly the same part of the thermals.

Fig. 2.

Visualizations of the track logs of a falcon and a paraglider. (A) Trajectory (track log) of a single flight of the falcon with the background being a black and white satellite map of the region. Coloring indicates the values of the vertical velocity component; red corresponds to climbing (mostly within thermals), blue to sinking (gliding parts). (B) An interesting feature of the falcon's thermalling flight is that from time to time it changes the direction of circling. Marks on the axes indicate 50-m distances. (C) Trajectory of a paraglider pilot with the local relief. (D) Compensating for the wind: the red trajectory corresponds to the original data (in the white box in C), whereas the same trajectory is shown in green after the effect of the wind has been eliminated. Marks on the axes indicate 100-m distances.

Table 1.

Soaring flight data determined for the Peregrine falcon, White stork, and paraglider

Flyer	r+, m	r–, m	vcircling, m/s	vclimb, m/s	vxy, m/s	vz, m/s	AB,°	T, s
Falcon	20.2	22.0	10.0	1.4	13.3	−1.9	26	15.4
Stork	22.3	21.1	9.5	0.9	12.1	−1.1	23	15.5
Paraglider	24.9	26.0	8.7	1.4	10.9	−1.3	17	20.1

r₊ and r_–, thermal circling radius in counterclockwise and clockwise directions, respectively; v_circling, average circling velocity, horizontal velocity component during thermalling; v_climb, average climb rate, vertical velocity during thermalling; v_xy, average horizontal velocity during gliding; v_z, average vertical velocity during gliding; sinking velocity, sink rate; AB, angle of bank; T, circling period time.

To check whether the falcon and the paragliders follow the predictions of the MacCready theory, the knowledge of the corresponding polar curves is needed. We used polar curves published by the manufacturer (if it was available) or measured by pilots. The falcon polar curve was obtained from the data points measured in wind tunnel by Tucker (9). In addition, for the falcon, we determined the “effective” polar curve as fitted to the measured average sinking and horizontal velocities of the nonthermalling parts of the flights (gray curves). Intermittent periods of flapping flight (embedded into ordinary gliding) of the falcon are the likely reason for the larger spread (as compared with para/hang glider data) of the measured values around the polar curve. We fitted the polar data by f(x) = a/x + b x³ + cx⁻³, with a = −4.1, b = −0.00056, and c = −100. For the “effective” polar curve, we used a parabola for simplicity, f(x) = ax² + bx + c, with a = −0.014, b = 0.20 and c = −1.58. When determining the polar curve from actual flight data, one also has to take into account rising and sinking air masses during the gliding periods, resulting in a higher scattering of the data (because the falcon was thermalling over flat regions with no soaring flight over wind-blown ridges, no systematic errors were expected to occur). The polar curves of the paragliders and hang gliders were fitted by f(x) = ax² + bx + c, with a = −0.015, b = 0.16 and c = −1.2 and a = −0.0095, b = 0.20, c = −1.7, respectively. The resulting root mean squares of the residuals were in the range of 0.02–0.05.

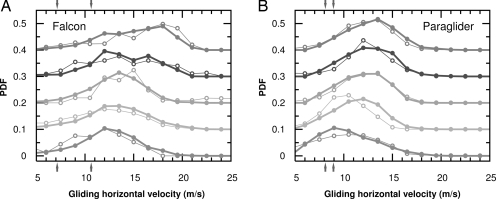
Next, we estimated how closely the falcons, the hang glider and the paraglider pilots follow the optimal thermalling strategy (i.e., the best sinking speed to choose for optimal overall horizontal velocity) as given by the MacCready theory (in principle there could be various strategies used by individual flyers but the only scientifically well documented strategy is this theory and its variants). Knowing the functional form of the polar curve the optimal values can be calculated for various climb rates v_climb from v_climb = p(v_xy) − v_xy dp(v_xy)/dv_xy (see Materials and Methods). In Fig. 3, we show a comparison of the actual and the predicted (by the MacCready theory) velocity distributions for the falcon and the paragliders. The predicted values were obtained by feeding the climbing rate distribution into the MacCready formula and calculating the corresponding horizontal velocity distribution from it. A perfect agreement between the observed velocities and the predicted ones would correspond to birds and people applying the theory to a 100% degree. We chose to quantify the agreement by calculating the mean square deviation of the actual and predicted distributions of the gliding velocities v_xy (where the predicted distributions are obtained by inserting the measured climbing rates into the MacCready expression and calculating the corresponding horizontal velocity distribution from it). Then, this deviation was compared with an average deviation that would have been observed, if the birds and the paragliders had used an incorrect value (randomly selected from other flights) for the climb rate in the preceding thermal when “calculating” the best theoretical value for the horizontal speed. The conclusion of this calculation is that, for both the Peregrine falcon and the paragliders, the actual deviations are significantly different from those obtained for the randomized case. In particular, the Student's t test gives the values t_falcon = 2.36 and t_paraglider = 2.80 for the comparison of the actual and the randomized data for the falcon's and the paragliders' flights, respectively. The corresponding t values for a 2.5% level of significance are smaller, t_{2.5, falcon} = 2.179 and t_{2.5, paraglider} = 2.131 (see SI Appendix).

Fig. 3.

Comparison of the actual and the predicted (by the MacCready theory) speeds. This figure shows two distributions of the gliding horizontal velocities during the top 5 days concerning thermalling conditions for the falcon (A) and two paragliders (B) who are among the few best performing contestants. The data are shifted upward by a value 0.1 for different days to improve the visualization. The filled and open symbols denote the circling radius distribution (PDF) of the predicted and the actual values, respectively. The predicted values were obtained by feeding the climbing rate distribution into the MacCready formula and calculating the corresponding horizontal velocity distribution from it. A perfect agreement between the observed velocities and the predicted ones would correspond to birds and people applying the theory to a 100% degree. The left and the right arrows on the x axis show the horizontal velocity value corresponding to the minimum sink and the best glide ratio, respectively.

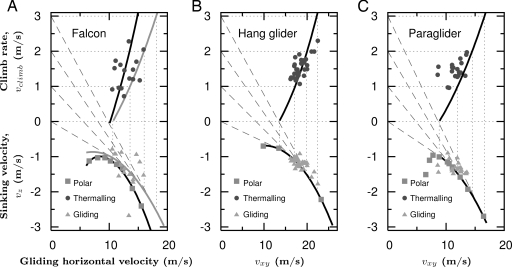
Fig. 4 shows the polar curve, the calculated optimal soaring strategy curve and the measured, flight averaged data points for falcon, a rigid hang glider and for two paraglider pilots (Fig. 4 A, B, and C, respectively) showing a reasonable agreement between theory and observations. The original MacCready theory did not take into account several factors that could influence the optimal choice for the gliding speed. These factors include changing of the air density as a function of height or the fact that the various thermals even within a single flight are quite different as concerning their lifting potential (15). We estimate that the corresponding accumulated error involved is in the range of 10–15%, and conclude that a perturbation of this order does not change our conclusion. In fact, a range of thermalling conditions is useful for checking the statistically relevant applicability of the MacCready theory.

Fig. 4.

Soaring strategy plots of flyers. Squares denote the known points of the polar curve (solid line). The solid line in the upper part represents the calculated optimal soaring strategy curve (see text). The dashed lines show tangent lines to the polar curve from points corresponding to different climb rates represented on the y axis. The dotted lines indicate the horizontal gliding speed values at the tangent point of the polar curve for the given dashed lines, and the corresponding climb rates. Circles denote the measured average thermal climb rate for the given measured average gliding horizontal velocity (one circle represents one flight). Measured average gliding horizontal velocity as a function of sinking speed of each gliding flight is indicated by triangles. (A) Peregrine falcon. The gray curves show the effective polar curve (due to flapping flight parts) and the corresponding optimal strategy (see text). (B) The flights of the hang glider pilot reaching third place. (C) Two paraglider pilots reaching first and second place of OLC.

Thus, we found that the leading paraglider pilots taking part in world contests and the falcon follow a similar flight pattern and a soaring strategy close to the optimal one as predicted by the theory. Paraglider pilots apply somewhat slower horizontal gliding speed as the optimal (points are more scattered to the left of the optimal curve). This can be interpreted by taking into account that the paragliders' glide ratio is worse than that of hang gliders, so they chose a lower speed to minimize the risk of not reaching the next thermal before landing. In addition, paragliders have a lower stability at higher speeds, so in some situations, pilots do not apply the maximum speed for safety reasons. The similarity of the paragliders' and the falcon's flight strategies could be demonstrated by considering the effective polar curve we introduced to take into account the occasional flapping flight periods of the falcon.

As for our original question, the result seems to be a draw. All of the parameters we determined were nearly the same for both humans and birds. Thus, as it happens, evolving flight strategies of birds and human calculations lead to virtually the same outcome.

Materials and Methods

The GPS Device.

The GPS device we developed was based on a Fastrax (Vantaa, Finland) product. It was capable of logging 24,500 log points (latitude, longitude, and altitude coordinates and time) and had the size of 4.5 × 6.2 cm, weighing only 34 g. This logging function could be restricted to the time when the bird was moving. The spatial and temporal resolution of the device was 1 m and 1 s with an error of 0.1 m and 20 ns, respectively. At evenings we detached the GPS device from the birds and downloaded the log files from the GPS to a PC. With the above setting, the memory was sufficient for storing the flight data of a whole day. We constructed four GPS units with the same features. Teflon-treated ribbons from Marshall Radio Telemetry (North Salt Lake City, UT), commercially used for similar purposes were used to fix them on the birds.

Collecting Data About Flights of Tame Birds.

In the Peregrine falcon project, we cooperated with a professional falconer breeding falcons. His breeding technique is to let the young birds fly free from the time they first start flying until they become almost totally self-supporting, which takes ≈1 month. During this time, the birds come home for food and sleep, and learn to hunt for themselves. Meanwhile, they become masters of soaring. The falcon chicks were hand-raised, so they were tame, enabling the falconer to change the GPS unit on the bird's back. For safety reasons, we also used a radio device (Micro transmitter by Marshall Radio Telemetry). This project took place in July 2006, in the Southeast part of Hungary near to the town of Békéscsaba. We collected the data for 3 weeks continuously. Fortunately, the weather was good during this period, with high thermal activity almost every day.

Under the White stork project, we worked in cooperation with the Hortobágy National Park and one of its institutions, the Bird Repatriation Station. Two ≈2-week-old storks that had fallen out of their nest and an ≈1-month-old stork were involved in the project. One of us (Z.Á.) moved with the birds to the Repatriation Park for 1.5 months. We fitted the three tame birds with ribbons after they had reached the adult size. From the time they were fitted with the ribbon, we put the GPS on them every day so they could get used to it. We collected soaring flight data for 2 weeks before our storks joined the migrating group. Unfortunately, the two younger storks started thermalling later than we had expected, and very soon they joined a migrating group. Therefore, the most of the flying tracks come from the older stork.

Collecting Flight Data from Pilots.

Paraglider, hang glider, and sailplane pilots record their flight track with GPS during competitions, or just for themselves to be able to overview the flight track later. These track logs are rarely available with 1-s resolution (pilots usually set their GPS devices to 3- to 5-s logging). To collect 1-s time resolution human flight track logs, we had arranged that a couple of paraglider pilots flew in competitions with the GPS devices developed by us. Besides, several paraglider pilots who had recorded 1-s time resolution tracks on their own sent us their data upon our request. For some part of our research, we did not need 1-s resolution track logs (e.g., research related to MacCready theory). Here, we used track logs downloaded from the Internet. The main source was the Online Contest (OLC) site, to which pilots from every part of the Earth upload their track logs.

Evaluation of the Data.

The geodetic coordinates provided by the GPS were converted into x, y, and z coordinates using the Flat Earth model. The Cubic B-Spline method was used for fitting curves onto the points obtained with 1-s sampling rate. The thermalling and gliding parts were separated by using information about the curvature and the vertical velocity parameters. The statistics was calculated from 43,000, 3,700, and 180,000 positional data (separated in time by 1 s); 1,460, 140, and 1,430 circles in thermals; 109, 16, and 114 separate thermals for the Peregrine falcon, the White stork and the paraglider pilots, respectively.

To determine the circling radius during thermalling, the effect of the wind had to be eliminated. It is difficult to estimate the change of the wind force from the ground to the top of the thermal (1,500–3,000 m) and along the horizontal route that the birds and paragliders fly (10–100 km). Therefore, we calculated the local wind velocity from the drifting of the thermal parts of the track log. Both horizontal components of the velocity during thermalling were calculated. For each component, the local maximum (minimum) places were determined. These data were smoothed by a Gaussian filter (σ = 1) and a cubic B-spline function was fitted, providing the local maximum (minimum) velocity function. We obtained the horizontal wind velocity component as a function of time by averaging the local maximum and minimum velocity functions. The wind velocity component functions were subtracted from the corresponding components of the horizontal speed of the original track. More details about the evaluation procedures are given in SI Appendix.

Application of the MacCready Theory.

In this theory the relation between the horizontal and the corresponding sinking speeds (the so-called gliding polar curve, p(x), characteristic for the given gliding object) is used. It is supposed that the climbing rate of the next thermal is known by the flyer, and no geographical effects are taken into account. We apply the following interpretation of the MacCready theory. The goal of the gliders is to make a given distance L_AB (using both thermalling and gliding and not loosing height in average) during a time as short as possible. Thus, they intend to minimize the quantity (time) L_AB [1/v_xy − v_z/(v_xy v_climb)], where v_xy, v_z = p(v_xy) are the gliding horizontal and vertical velocities, and v_climb denotes the climbing rate in the thermals (see Fig. 1 and SI Appendix Fig. 2). The optimal strategy is determined from equalling the derivative of this expression to zero, and in this way obtaining a relationship between the optimal v_xy and v_climb. This leads to the expression

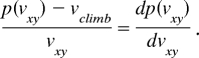This equation is equivalent to the statement that the optimal v_xy can be obtained by drawing a line from the point v_climb (along the vertical axis) tangent to the p(v_xy) polar curve and reading the corresponding v_xy value.