Thermal substitution and aerobic efficiency: measuring and predicting effects of heat balance on endotherm diving energetics

Abstract

For diving endotherms, modelling costs of locomotion as a function of prey dispersion requires estimates of the costs of diving to different depths. One approach is to estimate the physical costs of locomotion (P_mech) with biomechanical models and to convert those estimates to chemical energy needs by an aerobic efficiency (η=P_mech/Vo₂) based on oxygen consumption (Vo₂) in captive animals. Variations in η with temperature depend partly on thermal substitution, whereby heat from the inefficiency of exercising muscles or the heat increment of feeding (HIF) can substitute for thermogenesis. However, measurements of substitution have ranged from lack of detection to nearly complete use of exercise heat or HIF. This inconsistency may reflect (i) problems in methods of calculating substitution, (ii) confounding mechanisms of thermoregulatory control, or (iii) varying conditions that affect heat balance and allow substitution to be expressed. At present, understanding of how heat generation is regulated, and how heat is transported among tissues during exercise, digestion, thermal challenge and breath holding, is inadequate for predicting substitution and aerobic efficiencies without direct measurements for conditions of interest. Confirming that work rates during exercise are generally conserved, and identifying temperatures at those work rates below which shivering begins, may allow better prediction of aerobic efficiencies for ecological models.

1. Introduction

Estimating the food requirements of animals, and how foraging profitability changes with prey dispersion, are important to understanding constraints on where the animals can live and how much viable habitat is available (Lovvorn & Gillingham 1996; Luo et al. 2001; Fryxell et al. 2004). For diving endotherms, modelling costs of locomotion as a function of prey dispersion requires estimates of the costs of diving to different depths for varying durations. Heat loss to water is often substantial; however, much heat can be produced by the inefficiency of exercising muscles and the processing of food, thereby reducing the need for shivering thermogenesis. This energy savings, termed thermal substitution, varies with water temperature, exercise level, and the type, amount and timing of food eaten; it is also quite difficult to quantify in actively diving animals. As a result, and for reasons not well understood, measurements of thermal substitution have varied widely with experimental conditions. Despite its ecological importance, past studies of substitution have yielded little ability to predict its magnitude under different circumstances and its effects on the overall efficiency of energy use during foraging. In light of these needs, this paper reviews current concepts, methods and results regarding heat balance, thermal substitution and aerobic efficiency in diving endotherms. In regard to modelling dive costs, a central question will be, ‘Can we estimate the amount of shivering thermogenesis required to maintain body temperature, after accounting for substitution of heat from exercise and digestion?’

2. Alternative methods for measuring dive costs

In animals that normally dive to depths of less than a few metres, total dive costs (including thermoregulation) can be measured directly on captive animals in dive tanks via respirometry (e.g. MacArthur & Krause 1989; Kruuk et al. 1994; de Leeuw 1996; references in Kaseloo & Lovvorn 2005). The buoyancy of air in the respiratory system, and both the buoyancy and insulative value of the air layer in fur or plumage, decrease dramatically as the air is compressed with increasing depth, especially in the top 5–10 m (Lovvorn & Jones 1991; Wilson et al. 1992). Owing to changes in work against buoyancy with depth, and increased heat loss as air layers are more compressed, measurements in shallow tanks or for animals swimming only horizontally may not capture patterns or total costs of diving to deeper depths (cf. Croll & McLaren 1993 versus Lovvorn et al. 2004; Culik et al. 1994 versus Sato et al. 2002; Schmid et al. 1995 versus Enstipp et al. 2001 and Watanuki et al. 2005). A number of aquatic endotherms dive to depths of tens to even hundreds of metres (Schreer & Kovacs 1997), and respirometry on free-ranging animals is seldom possible except when individuals can be restricted to surfacing in a small chamber (Williams et al. 2004). Doubly labelled water measurements can be made on animals in the field (Nagy et al. 1984; Butler et al. 2004), but integrate costs of all activities (including non-locomotor costs) over periods of approximately a day, and are specific to the conditions during measurements; thus, they provide limited ability to predict dive costs for different patterns of prey dispersion.

Heart-rate microloggers have much promise for estimating variable dive costs in the field (Butler et al. 2004). However, heart-rate data must be calibrated to measurements of energy expenditure during exercise by captive animals, often for very different dive depths, temperatures, fluid media (air versus water) or even types of locomotion (treadmill walking versus diving; Hawkins et al. 2000; Froget et al. 2004; Guillemette et al. 2004). In extrapolating such calibrations to deep breath-hold dives, studies are needed of how correlations between heart rate and energy use are affected by diving responses of bradycardia, selective vasoconstriction and anaerobic metabolism (Williams et al. 1991; Kooyman et al. 1992; Bevan et al. 1997; Enstipp et al. 2001). Correlations between heart rate and oxygen consumption (Vo₂) are not the same for exercise versus thermoregulation (Froget et al. 2002), and heart rate may not change with increased Vo₂ due to the heat increment of feeding (HIF; McPhee et al. 2003). Also, heart-rate loggers on free-ranging animals must be retrieved for downloading data, which can prevent their use during non-breeding periods when some species may be difficult or impossible to recapture.

3. Biomechanics, aerobic efficiencies and substitution

Yet another approach for estimating dive costs is to calculate the physical power required for locomotion based on biomechanical models. Such models can account for varying dive depths, swim speeds, propulsive modes (e.g. wings versus feet) and durations of different dive phases (descent, activities at the bottom and ascent; Lovvorn et al. 1991, 1999, 2004; Lovvorn & Liggins 2002). These mechanical costs are then converted to chemical energy (food) requirements by an aerobic efficiency η, defined as mechanical power output divided by aerobic power input (Lovvorn 1994; Lovvorn & Gillingham 1996). This method has also been widely used in models of aerial flight (Pennycuick 1989). Calculation of η requires measuring Vo₂ and estimating mechanical power output (P_mech) during exercise in captive animals (Kaseloo & Lovvorn 2005) so that

$$\eta =\frac{P_{\text{mech}}}{V{\text{o}}_2}.$$ (3.1)

Values of η subsume a number of component efficiencies: (i) the efficiency of oxygen delivery to muscles by the respiratory and circulatory systems, (ii) the efficiency of muscles in doing contractile work, (iii) the efficiency of contractile work in moving fluid as opposed to accelerating and deforming tissues in the propulsive limbs, and (iv) the Froude efficiency or the fraction of work done to move fluid that yields useful thrust as opposed to being dissipated in the water as heat (Daniel 1991). Although heat dissipated in moving fluid (item iv) is ultimately derived from Vo₂, it does not increase the heat content of the animal as do items i–iii; thus, internal heat generation is not a direct or simple function of overall aerobic efficiency.

Since the deepest dive tanks available are only approximately 10 m deep (see Enstipp et al. 2001), and control of temperature in these tanks is limited, conditions for determining η often differ from those in the field. It is generally believed that η stays relatively constant over the range of work rates exhibited by free-ranging animals, or conversely that the animals regulate their work levels to stay within limited ranges of power output and efficiency (Pennycuick 1991; Lovvorn et al. 1999; Lovvorn 2001; but see Lichtwark & Wilson 2005). Once identified, such ranges might be useful in predicting aerobic power output and efficiency for varied combinations of buoyant resistance and swim speed (see Banister & Jackson 1967; Lovvorn et al. 2004). However, because η depends on aerobic power measured as Vo₂ (equation (3.1)), η will change if more energy is required for thermoregulation at colder temperatures. The amount of additional thermogenesis by shivering that is needed at a lower temperature depends on how much heat is already being generated by exercise. If Q_shiv is the ‘residual thermogenesis’, or the cost of shivering to generate heat above that supplied by substitution, then the value of η at the colder temperature (η_cold) could be expressed as

$${\eta }_{\text{cold}}=\frac{P_{\text{mech}}}{V{o}_{2‐\text{sub}}+Q_{\text{shiv}}},$$ (3.2)

where Vo_2-sub is Vo₂ during exercise within the range of substitution.

For field applications, we would like to estimate η_cold without measuring Vo₂ under conditions of temperature, buoyancy and compression of insulative air layers that are often infeasible to duplicate in dive-tank studies. By equation (3.2), calculating η_cold requires estimating residual thermogenesis Q_shiv at a given temperature and exercise level. If suitable methods are available (see §8), Q_shiv might be calculated from estimates of heat lost and the amount of heat generated that could replace heat lost. Alternatively, if direct measurements or estimates of heat balance are not possible, respirometry might yield values of Q_shiv. As temperature decreases during a given level of exercise, there may be a threshold in temperature below which substitution of heat from exercise is exceeded and shivering begins, as revealed by increases in Vo₂ (McArdle et al. 1984). By this threshold concept, heat from digestion (HIF) would be expected to lower the temperature for onset of residual thermogenesis. In animals with an insulative air layer in fur or plumage, compression of the air layer at depth would raise the temperature threshold. In later sections, methods of estimating heat balance, and the potential for identifying thresholds of thermal substitution with respirometry, will be discussed in more detail.

4. Why are measurements of substitution inconsistent?

Despite its intuitive importance, experimental results regarding thermal substitution have been variable. HIF, also known as specific dynamic action, is the heat produced by the digestion, absorption or processing of food. For substitution of HIF, findings have ranged from lack of detection through partial or even complete use of HIF for thermoregulation (references in Appendix A; see Rosen & Trites 2003). For heat from exercise, estimated substitution has ranged from none to apparently complete (Appendix B), although the validity of specific estimates is questionable (see §5). Despite a number of studies on birds, substitution of exercise heat in mammals has seldom been measured directly (Appendix B), but rather inferred based on patterns of body temperature (Costa & Kooyman 1982; Williams 1986; MacArthur 1989). For large marine mammals, exercise heat may often exceed heat loss (Williams et al. 1999, 2004) so that aerobic efficiency changes little due to varying substitution; however, even in large mammals such as dolphins, this balance may depend on body size (McGinnis et al. 1972). In birds and smaller mammals for which variations in substitution can be important, what factors account for the inconsistent patterns?

Studies of thermal substitution of the heat increment of feeding (HIF) in endotherms, based on energy consumption.

species	substitution	reference
birds
mallard (Anas platyrhynchos)	present	Kaseloo & Lovvorn (2003)
kestrel (Falco tinnunculus)	present	Masman et al. (1989)
Japanese quail (Coturnix coturnix)	present	Marjoniemi (2000)
Arctic tern (Sterna paradisaea)	none	Klaassen et al. (1989)
pigeon (Columba livia)	present	Rashotte et al. (1999)
tawny owl (Strix aluco)	present	Bech & Præsteng (2004)
house wren (Troglodytes aedon)	present	Chappell et al. (1997)
mammals
short -tailed shrew (Blarina brevicauda)	present	Hindle et al. (2003)
star-nosed mole (Condylura cristata)	none	Campbell et al. (2000)
golden hamster (Mesocricetus auratus)	present	Šimek (1975)
muskrat (Ondatra zibethicus)	present	MacArthur & Campbell (1994)
Steller sea lion (Eumetopias jubatus)	none	Rosen & Trites (2003)
white-tailed deer (Odocoileus virginianus)	present	Jensen et al. (1999)

Studies of thermal substitution of heat produced by exercise in endotherms, based on energy consumption.

species	substitution	reference
birds
tufted duck (Aythya fuligula)	present	Bevan & Butler (1992a)
lesser scaup (Aythya affinis)	present	Kaseloo & Lovvorn (2005)
Japanese quail (Coturnix coturnix)	present	Nomoto et al. (1983)
Gambel's quail (Callipepla gambelii)	present	Zerba & Walsberg (1992)
knot (Calidris canutus)	present	Bruinzeel & Piersma (1998)
ruby-throated hummingbird (Archilochus colubris)	present	Chai et al. (1998)
verdin (Auriparus flaviceps)	present	Webster & Weathers (1990)
dipper (Cinclus cinclus)	none	Bryant et al. (1985)
white-crowned sparrow (Zonotrichia leucophrys)	present	Ketterson & King (1977) and Paladino & King (1984)
dark-eyed junco (Junco hyemalis) and yellow-eyed junco (J. phaeonotus)	present	Weathers & Sullivan (1993)
house finch (Carpodacus mexicanus)	present	Zerba et al. (1999)
common chaffinch (Fringilla coelebs)	present	Pohl (1969)
common redpoll (Carduelis flammea)	present	Pohl & West (1973)
mammals
white rat (Rattus norvegicus)	present	Hart & Jansky (1963)
Australian water rat (Hydromys chrysogaster)	present	Dawson & Fanning (1981)

I will explore three aspects that might contribute to variable results from studies of thermal substitution in smaller endotherms: (i) problems in methods of calculating substitution, (ii) confounding mechanisms of thermoregulatory control, and (iii) varying conditions that affect heat balance and allow substitution to be expressed.

5. Methods for calculating thermal substitution

A major problem in studying thermal substitution is that it must usually be measured indirectly by methods with definite shortcomings. For substitution of HIF, the usual approach is to measure resting metabolic rate (RMR) via oxygen consumption in fasted animals at thermoneutral and sub-thermoneutral temperatures, and then to make the same measurements in fed animals (references in Appendix A). If the increase in Vo₂ from thermoneutral to sub-thermoneutral conditions is less in fed animals, then the difference is attributed to substitution of HIF which reduces the need for shivering at the cold temperature in the fed animals. Any differences in heat storage between fasted and fed animals must be accounted for, which is usually done by implanting a deep-body temperature sensor in the abdomen. However, due to regional heterothermy (see §8), measurements of heat storage based on sensors in the abdomen may be inadequate for detecting low levels of substitution of HIF.

Owing to differences in intermediary metabolism, foods with high protein content tend to have high HIF, whereas foods containing mainly lipid or carbohydrate have lower HIF (Blaxter 1989). The HIF usually increases as food intake exceeds that needed for maintenance metabolism, with the excess being used in anabolic processes which have lower efficiency (greater heat production) than catabolic processes (Blaxter 1989; Robbins 1993). Since the potential for substitution depends strongly on the magnitude of HIF, the above measurement approach works well for species that eat high-protein foods in large meals (e.g. Costa & Kooyman 1984; Markussen et al. 1994; Hawkins et al. 1997). However, for species that normally eat low-protein foods in smaller intermittent meals, peaks of HIF and thermal substitution tend to be low relative to the typically high variance in Vo₂ (Kaseloo & Lovvorn 2003, 2005). Thus, the substitution of HIF may be occurring, but the signal-to-noise ratio might be low for certain diets and consumption patterns.

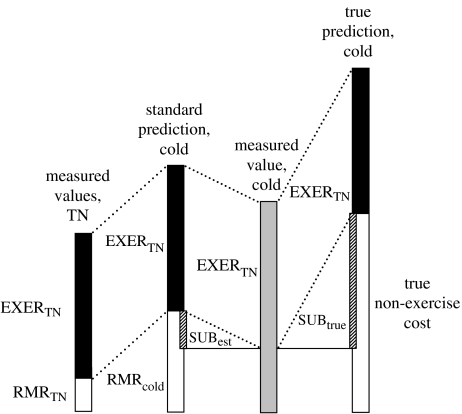
For measuring substitution of heat from exercise (figure 1), Vo₂ is measured at rest (RMR) and during exercise at thermoneutral temperature, and again under sub-thermoneutral conditions of either cold temperature or high convection (wind). The cost of exercise alone is estimated by subtracting RMR from total Vo₂ during exercise under thermoneutral conditions (Stainsby et al. 1980). By the standard method, the sum of RMR under cold conditions (RMR_cold) plus the cost of exercise alone under thermoneutral conditions (EXER_TN) is used to estimate the total cost of exercise under cold conditions (‘standard prediction, cold’ in figure 1). The latter estimate is then compared to the actual measurement of total cost during exercise under the cold conditions (‘measured value, cold’ in figure 1). Any decrease in the measured value compared to the standard prediction of total cost is attributed to substitution (cross-hatched area on second bar in figure 1; references in Appendix B). Substitution is presumed to have lowered that part of RMR_cold that was due to shivering.

Figure 1.

Graphical depiction of the standard method of calculating thermal substitution of exercise heat, and demonstration of how this standard method can underestimate substitution. For explanation refer to §5 of text. RMR_TN, resting metabolic rate at thermoneutral temperature; EXER_TN, cost of exercise above RMR at thermoneutral temperature; RMR_cold, RMR at cold temperature; SUB_est, substitution of exercise heat as estimated by standard method; SUB_true, true substitution of exercise heat.

Although similar problems apply to terrestrial animals, this measurement approach has particular shortcomings when applied to diving birds (cf. Bevan & Butler 1992a; de Leeuw et al. 1998; Kaseloo & Lovvorn 2005). The standard method assumes that the thermoregulation cost of a bird only partly submerged while resting on the water surface at the cold temperature (the conditions for RMR_cold, figure 1) is the same as for a bird completely submerged and experiencing high convection while swimming underwater. However, heat loss to water is increased several fold by complete submergence as opposed to floating on the surface (de Vries & van Eerden 1995; Banta et al. 2004), and convection during active underwater swimming can enhance this effect exponentially (Boutilier et al. 1977; Fish 1983). Consequently, the ‘True non-exercise cost’ (see fourth bar in figure 1) of a bird swimming underwater at the cold temperature is much larger than RMR_cold. This difference means that the sum of EXER_TN and RMR_cold (standard prediction, cold) greatly underestimates the sum of EXER_TN and the true non-exercise cost while moving underwater at the cold temperature (‘true prediction, cold’, figure 1). Thus, the standard estimate of reduction in cost due to substitution, SUB_est, is much smaller than the true reduction in cost, SUB_true (compare cross-hatched areas in second and fourth bars in figure 1).

Owing to this methodological shortcoming, Vo₂ comparisons do not always indicate when and how much substitution is occurring, and will often underestimate the magnitude of energy savings. If there is no increase at all in the total cost of diving between thermoneutral and colder temperatures, it can be assumed that costs of thermoregulation are either negligible or entirely met by substitution and incur no additional cost over maintenance and locomotion. However, we have no measure of thermogenic costs for a non-exercising bird that is submerged and experiencing high convection. Consequently, if the measured value of total dive cost is higher at the cold temperature than at thermoneutrality, it does not mean that less than 100% of exercise heat was used for substitution, as the increase in Vo₂ might have been much greater if substitution had not occurred. In other words, we cannot know the total amount or fraction of exercise heat that is used for substitution, without knowing the total heat loss (and shivering cost to replace that loss) from a submerged, inactive bird experiencing convection at the cold temperature.

6. Mechanisms of thermoregulatory control

Potentially important in predicting patterns of substitution are the strategies and priorities of thermoregulatory control. If substitution is less than complete, a possible reason is that deep-body sensors that detect increases in internal heat do not trigger decreases in shivering, because those sensors are overridden by sensors in the skin which are more sensitive to cooler peripheral temperatures (cf. Bullard & Rapp 1970; Jessen 1990; Østnes & Bech 1997, 1998). To my knowledge, no studies of thermal substitution relative to temperatures of different body regions have been done, so the viability of this explanation is unknown. More will be said about control of thermogenesis by peripheral versus deep-body sensors in §8g.

Thermoregulatory control strategies unique to breath-hold diving in cold water may confound studies of substitution, by causing dramatic regional changes in body temperature and lags in thermogenic responses. In some diving birds, the temperature of inactive tissues can drop substantially during long dives, in extreme cases by 10°C or more. This phenomenon has been interpreted as active downregulation of temperature via vasoconstriction, to decrease the oxygen demand of those tissues (Bevan et al. 1997; Handrich et al. 1997). By assuming a Q₁₀ of 3 and an average temperature drop of 2.4°C for the entire body over a dive bout, Bevan et al. (2002) estimated for gentoo penguins (Pygoscelis papua) that the maximum duration of a dive fuelled by aerobic metabolism could be extended by 38 s, and that all dives they recorded for free-ranging penguins would be within that limit. The question of whether temperatures in peripheral body tissues are actively reduced or simply allowed to fall passively was considered irrelevant, as cooling would decrease metabolic rate regardless of cause (Bevan et al. 2002).

However, for modelling costs of thermoregulation as they affect aerobic efficiency during dives, understanding whether changes in tissue temperature are regulated or passive is critical. For example, if cooling is only passive, patterns of heat balance and distribution might differ among animals of different body size, which have different work rates and heat generation relative to body volume and surface area, and might stay submerged for shorter periods at shallower depths with less compression of the insulative air layer. In very large birds, measurements of temperature at a range of body sites in freely diving emperor penguins (Aptenodytes forsteri) did not indicate metabolic suppression of the abdominal organs as a means of decreasing oxygen demand during dives (Ponganis et al. 2003). High temperatures were maintained in the body core, while there were decreases in forelimb, hindlimb, anterior abdomen, subcutaneous and sub-feather temperatures. These patterns indicated preservation of core temperature and cooling of peripheral tissues by either vasoconstriction, decreased insulation of the compressed air layer in feathers, or convection of heat to the water.

Regarding these mechanisms, the extent and importance of voluntary peripheral vasoconstriction has not been directly demonstrated in freely diving birds. In unrestrained redhead ducks (Aythya americana), diving to shallow depths with access to the water surface, the heart rate, arterial blood pressure and blood flow distribution were the same as during surface swimming without breath holding (Stephenson & Jones 1992); similar results were obtained for rhinoceros auklets (Cerorhinca monocerata; Stephenson et al. 1992). Dive depths and durations in these experiments were quite small, and both diving ducks and auklets showed marked cardiovascular responses when prevented from surfacing. Thus, although diving birds are certainly capable of such responses, it is unclear how much free-ranging birds use peripheral vasoconstriction during deeper dives.

Lower tissue temperature also decreases the temperature gradient that drives heat loss to water, especially while insulative air layers are compressed by hydrostatic pressure. Consequently, even without regulated vasoconstriction, smaller endotherms such as birds may let the temperature of certain tissues fall passively during dives and later restore that temperature upon resurfacing. This delayed response would lower thermogenic costs due to more favourable temperature gradients and thicker insulative air layers at the surface (MacArthur 1989; Wilson & Grémillet 1996). Moreover, shivering at the surface could be done by large locomotor muscles that were unavailable for shivering during exercise (Hong & Nadel 1979; Nomoto & Nomoto-Kozawa 1985; Rautenberg 1989; Hohtola et al. 1998; Kvadsheim et al. 2005; see §8e below).

In short, the degree to which cooling is passive versus actively regulated in different body regions during dives, and the magnitude of energy savings due to reduced heat gradients versus temperature-induced metabolic depression, are poorly known (Hochachka 1988; Culik et al. 1996; Boyd 2000; Ponganis et al. 2001; Kvadsheim et al. 2005). However, from the standpoint of thermal substitution, it is perhaps important that the time course and consistency of thermoregulatory patterns might differ between these two mechanisms. For example, vasoconstriction to regulate cooling for metabolic depression might occur rather quickly and consistently among taxa, whereas passive cooling might vary substantially among species of different body size and thermal inertia (e.g. large penguins versus small alcids). If passive cooling is an important mechanism for reduced body temperature, high variability among body sizes and insulative types (air versus blubber) might confound attempts to identify common conditions for expression of substitution in diving endotherms.

7. Heat balance and expression of thermal substitution

Another possible reason for varying assessments of the importance of substitution is that experiments may not create conditions of heat balance that allow substitution to be expressed. In addition to potential effects of control mechanisms mentioned in §8, the onset and magnitude of thermal substitution may depend on several factors: (i) heat loss must be high enough to create opportunity for substitution, (ii) heat generated by exercise or digestion must be great enough to offset heat loss and thereby reduce the need for shivering, (iii) meal size or protein content must be high enough to produce appreciable HIF, and (iv) heat from either exercise or digestion may satisfy thermogenic demands and thereby reduce need for the other (Kaseloo & Lovvorn 2006).

For heat from digestion, some experiments that seem to meet these criteria have not detected substitution (see Rosen & Trites 2003), perhaps owing to deficiencies in measurement methods or confounding mechanisms of thermoregulatory control (see §§5–6). Distinguishing these other factors from effects of overall heat balance requires estimates of heat generation versus heat loss under varying conditions. Section 8 reviews methods that have been used to estimate heat balance in diving endotherms, and their potential value in identifying patterns of substitution.

8. Approaches to measuring heat generation and loss

(a) Direct calorimetry

Total heat loss from body parts such as the feet of diving birds can be determined from changes in water temperature in a small water bath (Kilgore & Schmidt-Nielsen 1975; Midtgård 1980). Heat flux across unfeathered legs and feet may be an important component of overall heat balance. However, measurements for limbs immobilized in a small water bath generally do not reflect the high convection experienced by propulsors during swimming, or perhaps the circulatory patterns in active limbs. Consequently, resulting values may yield erroneous estimates of heat loss during locomotion.

(b) Heat flux across intact or excised integument

Two related approaches to estimating heat loss of diving endotherms involve (i) measuring heat flux across fat layers, skin, and fur or feathers in either living animals or excised tissue (Øritsland 1970; Frisch et al. 1974; Kooyman et al. 1976; review in Dunkin et al. 2005) or (ii) placing heat-flux sensors on the integument surface of captive or free-ranging animals (Kasting et al. 1989; Noren et al. 1999; Willis & Horning 2005). For animals with an insulative air layer in fur or plumage, a major challenge in such methods is maintaining the air layer in a natural state, without the constant cleaning, preening and ptilomotion exhibited by live birds and some marine mammals (Nolet & Kruuk 1989).

Heat-flux measurements on animals without insulative air layers can be relatively straightforward, but establishing the proper context for applying a few measurements to the animal's entire surface area is not. Depending on the intensity of the ‘dive response’, breath-hold diving can result in peripheral vasoconstriction and selective perfusion of different tissues including organs, muscles, fat and skin (Fish 1979; Stephenson & Jones 1992). As a result, integument (fat and skin) that overlies active locomotor muscles may experience different internal boundary conditions for heat flux than integument that overlies organs or muscles that are selectively ischaemic during a dive (e.g. pectoral muscles in a foot-propelled diver; Cooper et al. 1959; Luecke et al. 1975; Bevan & Butler 1992b; Østnes & Bech 1998). Although the conductivity of subcutaneous fat may decrease due to peripheral vasoconstriction during dives, during resting the conductivity of living blubber in seals submerged in cold water exceeded that of dead blubber by about 50% (Hart & Irving 1959). Perfusion and vasoconstriction in some tissues may cycle over time to avoid adverse effects, and blood from cooled extremities may be periodically flushed back to the body core and vice versa; thus, temporal variations may be important to heat flux at a given site (Johansen & Millard 1973; Hong & Nadel 1979; Johansen & Bech 1983; Cherepanova et al. 1993; Østnes & Bech 1998; but see Davis & Kanatous 1999). Moreover, both the thickness of subcutaneous fat, and the type and thickness of fur or plumage, can vary widely among body areas (Øritsland 1970; Evans & Moen 1975; Beck & Smith 1995), and different parts of the body surface can experience very different convective regimes during oscillatory stroking at different speeds (Liu et al. 1996; Wolfgang et al. 1999). For body surfaces exposed to the air after dives, thermal imaging can be used to assess regional variations of surface temperature and resulting heat flux (Hill et al. 1980; Williams et al. 1999). However, such methods cannot be used underwater, when factors affecting surface temperature and heat flux in swimming animals are quite different.

Consequently, before heat-flux measurements for only a few locations on the body can be used to estimate total heat loss, it should be shown that heat flux in those few locations is representative and over what portions of the body they are representative. Nevertheless, it is possible that some of these issues can be neglected in deriving useful values, or at least in assessing whether more detailed studies are justified. For birds and mammals with insulative air layers, such analyses will require data on the thickness of the air layer over different areas of the body, especially in species which depress their feather layer just before diving (cf. Grémillet et al. 1998). To date, no study has adequately evaluated the assumptions required to translate heat flux at selected locations into estimates of total heat loss from an actively diving animal.

(c) Heat loss estimated from physical and theoretical models

Given the difficulty of extrapolating a few heat-flux measurements to the entire body, a number of researchers have used taxidermic mounts stretched over heated metal casts to estimate overall heat balance in a range of terrestrial microclimates. Results have been mixed, often with unacceptably large errors (Walsberg & Wolf 1996; Dzialowski 2005). In water, such physical models have the same problems as heat-flux sensors in duplicating the insulative air layers that are so carefully maintained by live birds and some mammals. This approach has been little used for aquatic endotherms (Williams 1986) and not for diving birds or large diving mammals.

For endotherms that lack an insulative air layer in fur or plumage, total heat loss has also been estimated by theoretical models of heat flux through internal tissue layers and across the interface between integument and water (Luecke et al. 1975; Watts et al. 1993; Boily 1995; Hind & Gurney 1997). Estimates from such models have seldom been validated by empirical measurements, especially for actively swimming animals (for an exception, see Hind & Gurney 1997). In one case, all input parameters were measured, and estimates of total heat loss were compared with Vo₂ of inactive seals submerged in cold water. Large errors appeared to result from the fact that the blubber envelope and body core (mainly muscles and internal organs) were not concentrically positioned cylinders as they were in the model; heat flux was overestimated unless input data were based on direct morphometric measurements of the core (Kvadsheim et al. 1997). If one further considers the dive responses of bradycardia and peripheral vasoconstriction, the error in these models is potentially large and currently unassessed. Despite recent advances for horizontal swimming (Hind & Gurney 1997), such models need substantial validation and refinement before being used routinely to estimate thermal substitution or additional thermogenesis required in deep-diving animals.

(d) Respiratory heat loss

Evaporative heat loss in expired air may also be important to the heat balance of diving endotherms. When resting, both penguins and seals can decrease heat loss substantially by counter-current exchange of water and heat in nasal sinuses (Murrish 1973; Huntley et al. 1984). At low temperatures in air, several bird species have also altered their ventilation rates while increasing oxygen extraction rates to decrease respiratory heat loss (review in Johansen & Bech 1983; Brent et al. 1984; Stahel & Nicol 1988). Perhaps due to a combination of such mechanisms, respiratory heat loss in an inactive harp seal (Phoca groenlandica) submerged in cold water was only 1.8% of total metabolic heat production (Gallivan & Ronald 1979). Data for birds resting in air suggest that values may be higher in birds (Murrish 1973; Baudinette et al. 1986). Respiratory heat loss increases during exercise and can vary appreciably with ambient temperature and seasonality of insulation (Folkow & Mercer 1986). Effects of these factors on heat loss in air expired after exercise during breath-hold dives have not been investigated. Lacking such studies, it is not possible at present to make reliable predictions of respiratory heat loss in actively diving endotherms.

(e) Activity and heat generation by muscle

If total heat generation and loss are so difficult to quantify, might the onset and costs of residual thermogenesis (equation (3.2)) be determined from the activity of selected muscle groups? For shivering, groups of skeletal muscles appear to be recruited sequentially depending on muscle size and function. For example, in pigeons (Columba livia) and house finches (Carpodacus mexicanus) with large pectoral muscles for flight and only small leg muscles, pectoral muscles were the first to start shivering as ambient temperature declined during resting, with much lower temperature thresholds for shivering in leg muscles (Nomoto & Nomoto-Kozawa 1985; Carey et al. 1989). In domestic chickens (Gallus domesticus), which seldom fly and have much larger leg muscles, shivering started first in the leg muscles and began in pectoral muscles only at lower temperatures (Aulie & Tøien 1988). However, these patterns were for inactive birds, and exercise is believed to prevent shivering in active skeletal muscles while shivering can continue in muscles not being used for locomotion (Hong & Nadel 1979; Nomoto & Nomoto-Kozawa 1985; Rautenberg 1989; Hohtola et al. 1998). Thus, the first and primary muscles to be used for shivering in a resting animal would probably not be used for shivering during normal locomotion, when other muscles would be recruited instead. If alternative muscle groups important to shivering during exercise could be identified, their activity could perhaps be monitored in free-ranging animals to indicate the onset of residual thermogenesis. However, in diving animals, much of thermogenesis probably occurs after resurfacing (Wilson & Grémillet 1996) when large locomotor muscles may be available for shivering.

In terms of generating heat, the efficiency of producing mechanical power from chemical substrates by vertebrate skeletal muscle is approximately 25% (Taylor 1980). However, the efficiency of muscle contraction (and therefore heat produced) can vary appreciably with the mix of fibre types, muscle temperature, contraction speed and load (Holmer & Bergh 1974; Goldspink 1981; Pennycuick 1991). Substantial research would be needed to develop reliable estimates of total heat generation based on the activity of selected muscles.

(f) Heat increment of feeding

Although aspects such as peristalsis and enhanced blood flow play a role, HIF appears to result mostly from intermediary metabolism (Blaxter 1989). Heat increments for carbohydrate, fat and protein have been measured for a variety of endotherms at intake rates both above and below maintenance (Blaxter 1989), and conversion efficiencies for carbohydrate and fat are well understood and predictable (Schulz 1978; McDonald et al. 1981; Livesey 1984). However, carbohydrates and lipids have very low HIF compared with proteins, and HIF from protein metabolism varies appreciably with a range of factors including amino acid content of the food and amino acid needs of the animal (Krebs 1964; Buttery & Boorman 1976; Kielanowski 1976). Larger meals yield more HIF, and HIF usually increases as energy balance becomes more positive and more nutrients are used in anabolic rather than catabolic processes (Blaxter 1989; Robbins 1993). For birds that eat bivalves, crushing of shells in the gizzard might contribute to HIF (de Leeuw et al. 1998), but this effect was minimal when directly investigated (Piersma et al. 2003). Heating of foods obtained from cold water may also be a large fraction of HIF (Wilson & Culik 1991), although results have been mixed regarding the importance of heating food versus metabolic processing (Hawkins et al. 1997). Given all the factors affecting HIF of high-protein foods obtained from cold water, determining effects of HIF will in most cases require direct measurements with captive animals, using typical foods and meal sizes consumed on natural schedules. Simultaneous substitution of HIF and exercise heat has seldom been examined (Poehlman & Horton 1989; Kaseloo & Lovvorn 2006), but such studies are needed to understand patterns in actively foraging animals.

(g) Temperatures linked to thermogenic control

For deploying thermistors and microloggers on free-ranging animals, it is desirable to identify a few key temperature measurements that would integrate effects of heat generation and heat loss, and thereby indicate the degree of thermogenesis needed to restore body temperature after dives. For endotherms in air, much effort has been made to quantify the increase in whole-body Vo₂ resulting from change in temperature of various tissues (in W kg⁻¹ °C⁻¹; Simon et al. 1986; Jessen 1990). A shortcoming of many of these studies was that the temperatures of particular tissues (skin, spinal cord, hypothalamus, lower gut, etc.) were manipulated independently while others were held constant. This approach eliminated important feedbacks from other sensors, and elicited responses quite different (and often inappropriate) from those under normal conditions of environmental cooling (Simon et al. 1986; Mercer & Simon 1987). These earlier manipulative studies indicated that deep-body sensors were more important than sensors in the skin for controlling thermogenesis, although it was recognized that the overall metabolic response reflected inputs from multiple temperature sensors from the skin, spinal cord, skeletal muscle and elsewhere throughout the body (Simon et al. 1986; Kuhnen & Jessen 1988; Jessen 1990).

In more recent studies under conditions of natural cold exposure, total metabolism (Vo₂) in adult pigeons and ducklings responded to short-term changes in skin temperature well before changes in deep-body temperature occurred (Østnes & Bech 1997, 1998). In humans whose body temperature had dropped during submersion in cold water, shivering stopped as skin temperatures levelled off during rewarming, but well before deep-body temperatures had increased to normal values (Bullard & Rapp 1970). These results suggest that at the time-scale of one or a series of dives, skin temperature should be a better predictor of shivering thermogenesis. In birds, however, there can be large variations in skin temperature among areas of the body, such as bare skin versus feathered skin that either does or does not overlie active skeletal muscle; these areas in turn have differing influence on thermogenic responses (Necker 1977; Østnes & Bech 1997, 1998). Moreover, metabolic response to changes in skin temperature can have a dynamic component, where the magnitude of change in Vo₂ (W kg⁻¹ °C⁻¹) depends on the rate of change in skin temperature (Kuhnen & Jessen 1988). Study of correlations between Vo₂ and the magnitude and rates of change in skin temperature might identify a few key sites for measurements in the field. However, such research has not been done in diving endotherms.

9. Is thermal substitution predictable?

The above review suggests that our understanding of how heat generation is regulated, and how heat is transported among tissues during exercise, digestion and thermal challenge, is inadequate to predict the transition between thermal substitution and residual thermogenesis, and thus effects of thermoregulation on aerobic efficiencies (equation (3.2)). The situation is especially complicated in breath-hold divers. These animals may slow their heart rate and restrict blood flow to certain tissues while underwater and then re-perfuse these cooled tissues between dives, perhaps causing rapid reversals among tissues in relative temperature and metabolic activity. In the foreseeable future, it appears that estimates of aerobic efficiencies for modelling dive costs will continue to depend on Vo₂ in captive animals under as relevant conditions as possible. Measurements should be made for endotherms swimming over the range of usual speeds for typical dive durations, at ambient field temperatures, eating realistic meals at usual intervals (Kaseloo & Lovvorn 2006). For shallow divers, realistic dive depths should be used to the extent possible (de Leeuw 1996; Enstipp et al. 2001). For deeper divers, work is needed to verify key assumptions that allow extrapolation of measurements in shallow tanks to deeper dives.

10. Extrapolation of aerobic efficiencies

(a) Preferred work rates and aerobic efficiencies

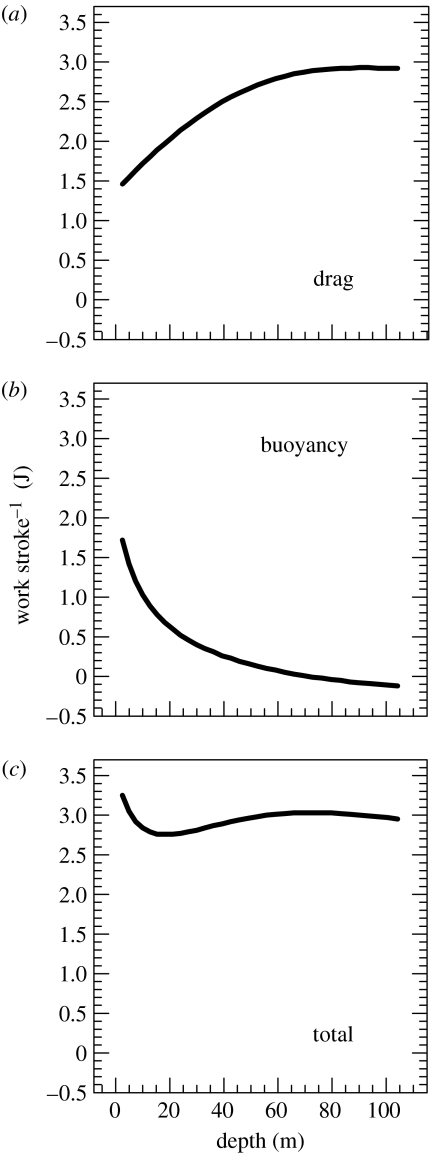
For deep-diving animals, given our dependence on measuring Vo₂ and aerobic efficiencies in shallow tanks, what principles might aid in extrapolating such data to the field? Recent work suggests that diving birds regulate their swim speeds and stroke rates to maintain relatively constant work per stroke (Lovvorn et al. 2004; Watanuki et al. 2005). As the buoyancy of air spaces declined with increasing compression during descent, wing-propelled Brünnich's guillemots (Uria lomvia) gradually increased their speed and associated drag to maintain relatively constant work per stroke, as estimated from biomechanical models (figure 2). Unlike the guillemots, foot-propelled European shags (Phalacrocorax aristotelis) decreased their stroke frequencies as buoyancy decreased during descent; however, they too appeared to maintain relatively constant work per stroke, as indicated by heave acceleration perpendicular to the direction of motion (Watanuki et al. 2005). Based on work with isolated muscles and respirometry of humans peddling ergometers at different speeds and resistance (Hill 1950; Gibbs & Gibson 1972; Gaesser & Brooks 1975; Goldspink 1981; di Prampero 1989), it has long been inferred that muscles are adapted to function most efficiently over certain ranges of contraction speeds and loads (Pennycuick 1991; Lovvorn et al. 1999; Lovvorn 2001). Animals should avoid exercising outside these ranges owing to rapid declines in efficiency. Such constraints might be especially important for breath-hold divers, for which efficient use of oxygen is critical.

Figure 2.

Estimated work per stroke against (a) drag, (b) buoyancy, and (c) both combined by a Brünnich's guillemot descending to 105 m, based on time-depth and accelerometer data from a micrologger deployed on a free-ranging guillemot, estimated volumes of air in the respiratory system and plumage, and drag measurements for a frozen specimen at different speeds (Lovvorn et al. 2004).

It is also apparent, however, that the locomotor systems of animals can accommodate quite variable demands. For example, guillemots (Uria spp.) stroke their wings at approximately 8.7 Hz during flight in air, but at 1.9–2.8 Hz when swimming underwater in a medium 800 times denser than air (Pennycuick 1987). Mechanisms for accommodating such different conditions, and the consequences for exercise efficiency, are an active area of research (Boggs & Dial 1993; Biewener et al. 1998; Sokoloff et al. 1998; Biewener & Gillis 1999). Although there may be clear optimal conditions for achieving maximum power and efficiency, muscle can potentially achieve high levels of both over a range of conditions (Lichtwark & Wilson 2005). Low temperature reduces the efficiency of muscle contraction in the short term (Holmer & Bergh 1974; Swoap et al. 1993); and in the long term, functional and biochemical adaptations of muscles to chronic shivering may reduce their efficiency for exercise (Schaeffer et al. 2005). Thus, optimal work rates and efficiencies may change with water temperature. Moreover, given the potential for substituting the heat of inefficiency for thermoregulation, lower efficiencies at higher contraction speeds or greater loads might have reduced impacts on total costs at cold temperatures (Hind & Gurney 1997; Chai et al. 1998).

Nevertheless, a number of studies have shown that endotherms often choose to swim at certain speeds (Ponganis et al. 1990; Culik et al. 1991; Williams et al. 1993; Schmid et al. 1995; Allers & Culik 1997; Bethge et al. 1997; Pfeiffer & Culik 1998; Lovvorn et al. 2004; Watanuki et al. 2005). Work rates at these voluntary speeds presumably correspond to maximum aerobic efficiencies. If diving endotherms do seek a narrow range of efficiencies, measurements on captive animals might identify associated work rates for different body sizes and locomotor modes. These efficiencies might then be extrapolated to similar work rates estimated from biomechanical models for different conditions. For example, the aerobic efficiency at a given work rate during horizontal swimming, when there is higher speed against drag but little work against buoyancy, might be extrapolated to the same total work rate during descent at lower speed but with higher work against buoyancy (Banister & Jackson 1967).

(b) Caveats to extrapolation

Even if animals of comparable size tend to limit themselves to similar ranges of work rates, the same aerobic efficiencies cannot be assumed among animals with different swimming modes or types of insulation (Fish 1993, 1996). In analogous experiments (table 1), great cormorants (Phalacrocorax carbo) weighed 10% less and had RMR in air 18% lower than Adélie penguins (Pygoscelis adeliae). However, in water, RMR of the cormorants was 68% higher at 13°C than for the penguins at 4°C, and total costs of underwater swimming were 135% higher in the cormorants (table 1). Cormorants have partially wettable plumage that allows higher heat loss to water (Wilson & Grémillet 1996), and they also swim by foot propulsion which is generally less efficient than wing propulsion (Fish 1996; Lovvorn & Liggins 2002). Thus, the striking difference in swimming cost apparently resulted from both greater heat loss and lower propulsive efficiency in cormorants, which would lower their overall aerobic efficiency relative to penguins (see also Enstipp et al. 2005 regarding dive costs in cormorants and penguins). These studies and others show that aerobic efficiencies may differ appreciably between locomotor modes, even within the same species (Williams 1989). The overall significance of different locomotor efficiencies depends partly on thermal substitution, as more heat produced by less efficient locomotion may allow greater reductions in shivering (Hind & Gurney 1997; Chai et al. 1998). At present, we have no way to predict these interactions and must rely on empirical measurements at relevant temperatures.

Table 1.

Comparison of the metabolic costs of Adélie penguins and great cormorants resting (RMR) in air and water, and swimming horizontally underwater in a shallow tank.

	Adélie penguina	great cormorantb	percent difference
body mass (kg)	2.56	2.31	−10
RMR in air (W kg−1)	3.8	3.1	−18
water temperature (°C)	4	13
RMR on water (W kg−1)	8.4	14.1	+68
underwater swimming (W kg−1)	15.6	36.7	+135
optimum speed (m s−1)	2.2	1.9	−14

^aCulik et al. (1994).

^bSchmid et al. (1995).

A further limitation to extrapolating aerobic efficiencies (η, see §3) among locomotor modes is that values of η (mechanical power output/aerobic power input) are specific to the models used to estimate mechanical work. If a different model is used that differs in estimated mechanical cost, the new estimate must be divided by the original aerobic cost measurement to generate a new value of η that is specific to the different model (equation (3.1)). Although timely ecological applications may require use of standard values, practitioners should always be aware that values of η depend strongly on how well different models for the same or different swimming modes estimate mechanical work (see table 3 in Stephenson et al. 1989). For example, if one model accounts for 80% of the mechanical work while another accounts for only 70%, the calculated aerobic efficiency in the former case would be appreciably higher, despite using the same measurement of aerobic cost (Vo₂).

(c) Cost of transport

To avoid uncertainties in the relative adequacy of mechanical work estimates (see §10b), it has been suggested that minimum cost of transport (COT_min) is a more standardized variable for prediction of swimming costs (Fish 1996, 2000). Cost of transport (J kg⁻¹ m⁻¹) is defined as the metabolic energy required to transport a unit mass a unit distance, and does not require biomechanical models for prediction. Animals are expected to select speeds that minimize COT.

Some species do choose to swim at speeds near their COT_min (Culik et al. 1991; Williams et al. 1993; Bethge et al. 1997). However, birds and mammals in fluids often travel slower than the ‘optimum’ speed (Schmid et al. 1995; Allers & Culik 1997; Fish et al. 1997; Pennycuick 1997; Pfeiffer & Culik 1998; Luna-Jorquera & Culik 2000). In some cases, COT does not change much between the slower speed and the apparent optimum; for example, in the study of great cormorants described in §10b (Schmid et al. 1995), COT_min was unchanged over speeds of 1.5–2.1 m s⁻¹. However, power output increased by 23% over the same range and the birds preferred to swim at 1.5 m s⁻¹. If the ability of muscles to generate power is not limiting, and COT does not change over this range of speeds, the birds should swim at 2.1 m s⁻¹ because they cover the same distance at the same cost in less time. However, for steady cruising, the higher power at faster speeds might not be sustainable. The fact that the birds do not swim at the higher speed suggests a limit to acceptable inefficiency or power output, so that exercise becomes too inefficient or unsustainable if the work against drag increases above a threshold that occurs around 1.5 m s⁻¹. These findings caution against using allometric or other broad patterns of COT_min to predict the swimming speed of a given species under particular conditions, without knowing aerobic efficiencies at different speeds (see also Pennycuick 1997).

11. Future prospects

Acquiring captive animals, obtaining adequate facilities and equipment, and conducting respirometry are costly and time-consuming. Consequently, for estimating foraging profitability and the extent of viable habitat, better ability to predict aerobic efficiencies under a range of conditions is very important to modelling based on biomechanical estimates. Advances are being made in understanding and estimating the mechanical costs of different swimming modes (Skrovan et al. 1999; Lovvorn & Liggins 2002; Johansson 2003). Improved electronic devices for free-ranging animals are providing more and better data (Sato et al. 2002; Watanuki et al. 2005), which can be used in developing mechanical models applicable to animals that cannot be recaptured to retrieve loggers (Lovvorn et al. 2004). At present, however, there are few known relationships for predicting aerobic efficiencies needed to convert those mechanical estimates to food requirements, and costly empirical measurements on captive animals seem essential. Moving towards predictive capability will require developing ways to measure and estimate thermal substitution, and its effects on aerobic efficiencies at depths not possible in dive tanks.

To help focus future efforts based on respirometry, I propose the following approach to predicting aerobic efficiencies for varying dive depths and water temperatures:

1. Assess the need to consider thermoregulation costs by seeking combinations of exercise levels and temperatures below which substitution can no longer replace lost heat and shivering begins.

2. At temperatures below the limits to substitution, assume that thermoregulation costs are additive to those of exercise. In other words, in calculating η_cold, aerobic power would include the cost of exercise within the range of substitution, plus any shivering (equation (3.2)).

3. Extrapolate the resulting aerobic efficiencies to varying depths and temperatures by assuming that work rates and aerobic power are conserved. In other words, work against buoyancy is included as part of the total work rate, and residual shivering depends on temperature independent of that total work rate.

By this construct, identifying the threshold temperature for onset of residual thermogenesis during exercise (equation (3.2)) would be akin to identifying the lower critical temperature of the thermoneutral zone for resting animals. That there is often a clear transition between substitution and shivering during exercise is yet to be determined (see McArdle et al. 1984); however, the lower critical temperature in a resting animal is usually quite recognizable, and similar principles may apply. Moreover, this construct does not yet deal with effects on heat loss of the compression of insulative air layers with depth, a topic we know very little about (see Grémillet et al. 1998). However, if the above approach can serve as a testable framework, it may facilitate progress towards merging biomechanics and physiology to determine energetic constraints on viable foraging conditions.