Auditory-Nerve Rate Responses are Inconsistent with Common Hypotheses for the Neural Correlates of Loudness Recruitment

Abstract

A number of perceptual phenomena related to normal and impaired level coding can be accounted for by the degree of compression in the basilar-membrane (BM) magnitude response. However, the narrow dynamic ranges of auditory-nerve (AN) fibers complicate these arguments. Because the AN serves as an information bottleneck, an improved understanding of the neural coding of level may clarify some of the limitations of current hearing aids. Here three hypotheses for the neural correlate of loudness recruitment were evaluated based on AN responses from normal-hearing cats and from cats with a noise-induced hearing loss (NIHL). Auditory-nerve fiber rate-level functions for tones were analyzed to test the following hypotheses:

• Loudness recruitment results from steeper AN rate functions after impairment. This hypothesis was not supported; AN rate functions were not steeper than normal following NIHL, despite steeper estimated BM responses based on the AN data.

• Loudness is based on the total AN discharge count, and recruitment results from an abnormally rapid spread of excitation after impairment. Whereas abnormal spread of excitation can be observed, steeper growth of total AN rate is not seen over the range of sound levels where recruitment is observed in human listeners.

• Loudness of a narrowband stimulus is based on AN responses in a narrow BF region, and recruitment results from compression of the AN-fiber threshold distribution after impairment. This hypothesis was not supported because there was no evidence that impaired AN threshold distributions were compressed and the growth of AN activity summed across BFs near the stimulus frequency was shallower than normal.

Overall, these results suggest that loudness recruitment cannot be accounted for based on summed AN rate responses and may depend on neural mechanisms involved in the central representation of intensity.

Introduction

A common characteristic of listeners with sensorineural hearing loss (SNHL) is reduced dynamic range and loudness recruitment. Recruitment means a faster than normal growth of loudness between the elevated threshold and high sound levels, where loudness typically returns to normal values. Hearing aids must overcome this reduced dynamic range; soft sounds require amplification to become audible, whereas loud sounds must not be amplified to avoid painfully loud sounds. An improved understanding of the neural correlates of recruitment might allow better design of amplitude compression in hearing aids.

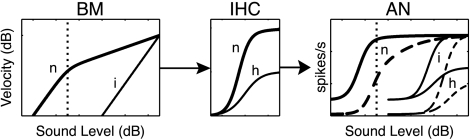
The growth of loudness with sound level in human listeners corresponds well to the growth of basilar-membrane (BM) motion in experimental animals, in both normal ears and impaired ears with loss of outer hair cell (OHC) function (Ruggero and Rich 1991; Moore 1995; Ruggero et al. 1997; Schlauch et al. 1998; Buus and Florentine 2002; Fridberger et al. 2002). This result suggests that recruitment is caused by steepened BM response growth in impaired ears. Steeper BM responses must be conveyed to the brain through the auditory nerve (AN), so this model seems to require steeper AN rate functions (discharge rate versus SPL) in at least some fibers following SNHL (e.g., Harrison 1981; Pickles 1988; Moore 1991, 1995; Schroder et al. 1994). However, AN rate functions in impaired ears are only steeper in limited conditions (e.g., for vowel stimuli or at very high levels) and are generally shallower than normal for tones, the stimuli typically used for loudness testing (Heinz and Young 2004). Figure 1 summarizes the current understanding of this problem.

Fig. 1.

The factors underlying the steepness of auditory-nerve (AN) rate versus level functions, taken from Heinz and Young (2004). The first box shows basilar membrane (BM) velocity plotted versus sound level in normal (n) and impaired (i) ears (Ruggero and Rich 1991). The differences are due to loss of cochlear amplification and compression due to outer hair cell (OHC) damage. The central box shows a plot of inner hair cell (IHC) response versus BM motion for normal ears and for those with IHC damage (h). The third box shows AN rate functions in normal and impaired ears. The solid lines are for low threshold (high spontaneous rate, SR) fibers, and the dashed lines are for high threshold (low and medium SR) fibers. Cases with OHC impairment only (i) show steeper rate functions in the low SR fibers because of the change in slope of the BM response. Cases with OHC and IHC impairment (h) show a reduced slope because of the reduced output of IHCs.

Loudness is often assumed to be directly related to the total AN discharge count (e.g., Wever 1949; Goldstein 1974; Moore 1995), although the exact relationship remains unclear (Pickles 1983; Relkin and Doucet 1997). Thus factors associated with summing activity across AN fibers could be important in producing recruitment. It has been hypothesized that abnormally rapid spread of excitation because of reduced frequency selectivity could underlie recruitment in impaired ears, an explanation that does not require steeper rate functions (e.g., Kiang et al. 1970; Evans 1975). However, when the spread of excitation is restricted, e.g., with flanking noise bands or for tones adjacent to a high-frequency hearing loss, the growth of loudness in impaired ears is generally unaltered (Moore et al. 1985; Hellman and Meiselman 1986; Zeng and Turner 1991). A second hypothesis for recruitment, which works when loudness is encoded in a narrow best frequency (BF) region near the stimulus frequency, is that recruitment results from a compressed distribution of AN thresholds in impaired ears (Moore et al. 1985; Zeng and Turner 1991). Presumably the compressed distribution could result if SNHL were to raise thresholds more among low-threshold (high spontaneous rate, SR) fibers than among high-threshold fibers. The result would be a more rapid recruitment of fibers as stimulus level increases.

Here we show that the rate functions of AN fibers in cats with SNHL are inconsistent with the hypothesized neural correlates of loudness recruitment. This result is primarily because of the fact that inner hair cell (IHC) damage appears to decrease the slope of AN activity growth more than OHC damage increases it (Heinz and Young 2004). This finding suggests that the at-BF magnitude response on the BM, which can account for a wide variety of perceptual phenomena including recruitment (Moore 1995; Moore and Oxenham 1998), is likely to have a complex neural representation.

Methods

The single-unit data used in this study represent a subset of the data described in a previous paper (Heinz and Young 2004), in which the effects of noise-induced hearing loss (NIHL) on the rate functions of AN fibers were studied for a range of stimuli, including BF tones, 1- and 2-kHz tones, broadband noise, and a speech token. The acoustic-trauma paradigm, the recording procedures, the stimuli, and the analyses are described in the previous report and are only briefly summarized here. All animal care and use procedures were approved by the Johns Hopkins Animal Care and Use Committee (protocol #CA99M255).

Acoustic trauma and electrophysiology

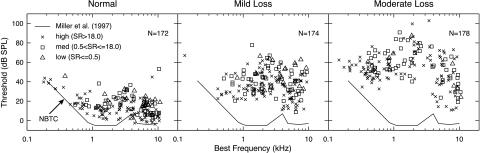
Auditory-nerve recordings were made from healthy adult cats that were free from external- or middle-ear pathology and were typically 3.5 kg in weight. Data were collected from both normal-hearing cats and from cats with a NIHL. The acoustic trauma was a 50-Hz-wide noise band centered at 2 kHz, presented at 103–108 dB SPL for 4 h, while the cats were anesthetized with xylazine/ketamine (similar to previous studies by Liberman and Dodds 1984b and Miller et al. 1997). Cats were allowed to recover for at least 30 days after the noise exposure to eliminate temporary threshold shifts. Glass microelectrodes were used to make extracellular recordings from single AN fibers in pentobarbital-anesthetized cats. The noise exposure produced elevated thresholds for BFs from 0.5 to 4.0 kHz and broadened tuning, as measured by Q10 values (the ratio of BF to bandwidth 10 dB above threshold). Figure 2 shows tuning curve threshold distributions in normal animals and in two groups of exposed animals. Each noise-exposed animal was assigned to either the mild- or moderate-loss group according to its threshold shift, with mild losses being threshold shifts of 25–30 dB near 2 kHz and moderate losses being threshold shifts of 45–50 dB near 2 kHz. The exposure was the same in all animals; the difference in NIHL is presumably because of differing susceptibility to the exposure (Maison and Liberman 2000). In each population, data were combined across cats; there were eight normal animals, six with mild losses, and four with moderate losses. Within the mild pool, best thresholds varied across cats by less than 10 dB in BF regions with a good representation of fibers; within the moderate pool, the variation was less than 20 dB.

Fig. 2.

Threshold distributions for AN fibers in three populations. Thresholds from tuning curves are plotted versus BF for all AN fibers for which 2-kHz tone rate functions were collected. Tuning curve thresholds were defined as the minimum level necessary to induce an increase of 20 spikes/s in response to a 50-ms tone. The line (NBTC) shows minimum thresholds in normal animals from our supplier. Normal animals are unexposed; mild and moderate populations received the noise exposure described in the text. Tuning curve widths (Q10s) are shown in the original paper (Heinz and Young 2004); they were depressed at BFs with large threshold shifts, especially in the moderate loss group.

Stimuli

Broadband noise bursts were used to search for fibers; isolated AN fibers were initially characterized using an automated tuning-curve algorithm, from which threshold, BF, and Q10 were estimated. Rate functions were measured in 1-dB steps, ranging from about 20 dB below threshold up to 80–90 dB SPL for normal-hearing cats and up to 100–120 dB SPL for hearing-impaired cats. Responses were measured for a variety of stimuli using 200-ms stimuli and a 1000-ms repetition period. Because several stimuli were used in these experiments, generally it was only possible to collect one to three repetitions of the rate function for each stimulus. Stimulus-driven rate was measured during a 200-ms window beginning 10 ms after the stimulus onset to account for acoustic delay and latency. Spontaneous rate was estimated from the final 600 ms of silence during presentation of the lowest 20 levels of the 2-kHz tone. Fibers were characterized into one of three SR groups: low (SR ≤ 0.5 spikes/s), medium (0.5 < SR ≤ 18), and high (SR > 18), as described by Liberman (1978).

Analysis

Slopes of rate functions

Slopes of rate functions were estimated by fitting a simple one- or two-line model, depending on whether sloping saturation was present (for the method, see Heinz and Young 2004). The low-level slope represents the response growth between threshold and the saturation point or knee in functions with sloping saturation; the high-level slope, when fit, represents the response growth above the knee of rate functions with sloping saturation. Data on high-level slopes are not shown here. Many fewer fibers have such slopes in impaired ears, compared with normal ears, and high-level slopes in impaired ears are not steeper than normal for tone stimuli (Heinz and Young 2004).

Estimating basilar-membrane slope

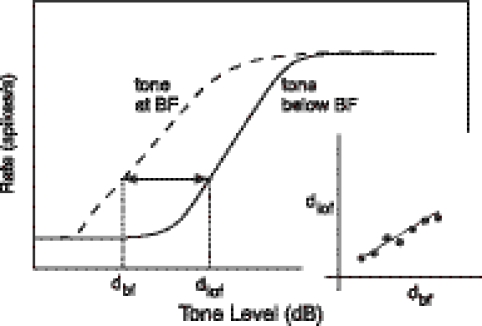
The relative slopes of rate functions to BF tones and tones in the tail of the tuning curve, i.e., well below BF, were used to provide an estimate of the slope of BM response growth at BF as a function of level (Yates et al. 1990). The method assumes that BM responses to tones well below BF are linear (Ruggero et al. 1997), and that all other nonlinearities contributing to AN responses are the same at and below BF. As illustrated in Figure 3, a series of sound level pairs (d_bf, d_lof) are found such that the discharge rate to a BF tone at level d_bf is equal to the rate to a tone well below BF at level d_lof. These levels are plotted against each other as in the figure inset. The slope of the resulting function is the estimate of the slope of BM response growth at BF.

Fig. 3.

The method of estimating relative BM slopes from Yates et al. (1990). The main plot shows hypothetical rate functions for a BF tone and a tone below BF. The dotted lines show extraction of sound levels d_bf and d_lof at which rates are equal. (d_bf, d_lof) pairs from a series of rates across the dynamic range of the fiber are plotted in the inset for relative slope determination (line).

For the relative slope calculation, responses to 1-kHz tones were used as the below-BF tone and fibers with BFs between 2.3 and 6 kHz were included in the analysis (1 kHz was the lowest frequency for which we have data for a large number of fibers). The range of BFs chosen is a compromise between having the below-BF tone frequency be sufficiently low to give a linear response, using a BF region with significant threshold shift, and using a wide enough range of BFs to give robust slope estimates. Over this range of BFs, there is not a significant change in relative slope with BF.

Rate functions were smoothed with a 15-dB triangular window before deriving (d_bf, d_lof) pairs. Basilar-membrane response slope (dB/dB) was estimated from plots of d_bfversusd_lof by fitting a regression line over a 9-dB window, shifted in 1-dB steps across level. The result is a series of estimates of slope at various sound levels. The slope estimates were smoothed with an 11-dB triangular window and then averaged across fibers, giving mean slopes and standard errors. Average slopes were obtained for the normal hearing and for both impaired populations. Although different AN fiber groups generally contribute to the BM slope estimates at different levels (i.e., high-SR, low-threshold fibers at low levels and low-SR, high-threshold fibers at higher levels), the self-normalization of each AN fiber response allows a contiguous estimate of BM slopes across a wide dynamic range.

A composite BM input–output function was then derived for each population by integrating the average slope across tone level and assuming the BM responses were equal in all three populations at 95 dB SPL. The choice of 95 dB SPL is ad hoc; it does not affect the basic conclusions from this analysis and is generally consistent with BM data (Ruggero et al. 1997; Fridberger et al. 2002) and psychophysical loudness studies (Miskolczy-Fodor 1960; Moore 1995). In both cases, responses at 90–100 dB SPL are roughly independent of hearing loss.

Summing auditory-nerve rates across fibers within a population

Summed population responses were estimated by combining measured AN fiber rates across populations of fibers (e.g., across all BFs, Fig. 8, or within a narrow BF region centered on the tone frequency, Fig. 10). Driven rate, computed by subtracting SR, was used to exclude spontaneous activity from the average. The combination of AN rates was performed in a way that minimizes the potential effects of uneven sampling across BFs and SR groups. Average driven rate per fiber was calculated separately within each of 13 BF channels, with one channel centered on 2 kHz and adjacent channels spaced in 0.4-octave steps to fill the BF range. A BF-channel width of 0.4 octave was chosen as an approximation to critical bandwidths in cat (Pickles 1979). Within each channel, average driven rate per fiber was calculated separately for high-SR and for low/medium-SR fibers. An overall average rate in each channel was then computed based on the SR-group proportions observed in the normal-hearing population (60% high SR, 40% low/medium SR) and in the impaired populations (50% high SR, 50% low/medium SR) (Heinz and Young 2004, consistent with Liberman and Dodds 1984a). This calculation was carried out separately in each channel as a function of level, followed by smoothing across level (5-dB triangular window) and frequency (three-channel triangular window).

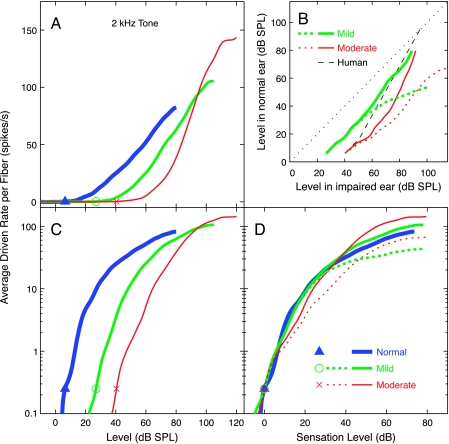
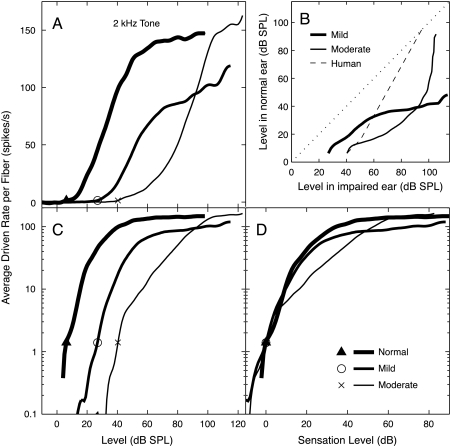
Fig. 8.

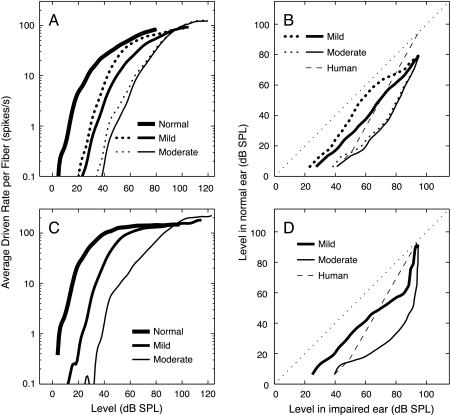
Average driven discharge rate summated across the entire population shown in Figure 4 (BFs of 0.25–9.2 kHz) is plotted versus level. Rate is expressed as average rate per fiber, minus SR, weighted as described in Methods. (A) Average rate plotted on a linear ordinate for the three populations. (B) Rate matches between normal and impaired populations are plotted as the sound levels at which rate in the normal ear equals rate in the impaired ear. The dashed black line shows the typical behavior of monaurally impaired human observers in a binaural loudness balance (Moore 2004). Recruitment is indicated by a steeper growth of the rate matches relative to the diagonal (1 dB/dB, dotted black line), which is only evident at high levels in the AN data. (C, D) Average rate versus level, with rate plotted on a logarithmic ordinate, as loudness is usually plotted. The abscissae are stimulus level (C) or sensation level (D). The stimulus was a 2-kHz tone in all cases. Thresholds (indicated by symbols) were determined by the level at which average rate per fiber reached 0.25 spikes/s. This criterion was chosen so that thresholds for the population average matched the thresholds for the 2-kHz BF channel (which were based on 1.4 spikes/s, see Fig. 10). The dotted red and green curves in B and D represent the summed activity from the impaired populations excluding the three highest BF channels (BFs > 4 kHz).

Fig. 10.

Average driven rate summated over a 0.4-octave region centered on the 2-kHz tone (normal, 7 fibers; mild, 26 fibers; moderate, 20 fibers). Plots show average rate per fiber plotted against the level of the tone. As in Figure 8, rate is plotted on linear (A) and logarithmic (C, D) ordinates, and level is plotted as dB SPL (A, C) or as dB re threshold (D). The three populations are defined in the legend. (B) Rate balances between the normal and impaired populations, computed as in Figure 8. Thresholds (indicated by symbols) were determined by the level at which average rate per fiber reached 1.4 spikes/s. This criterion produced threshold values that were within 1 dB of the minimum AN-fiber threshold in the 2-kHz channel for each population.

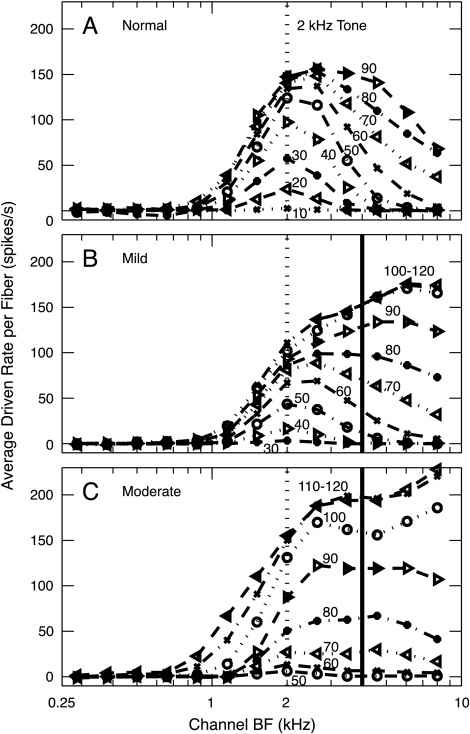
Figure 4 shows the computed distribution of average driven rate per fiber in response to a 2-kHz tone across the set of 0.4-octave channels (fiber BFs from 0.25 to 9.2 kHz) for a wide range of levels. An asymmetric activity pattern is observed in each population, meaning that the spread of activity is more pronounced for BFs above the tone frequency than below, especially in the impaired populations. However, in looking at these data, remember that best AN fiber thresholds return to near normal for BFs above 4–5 kHz (Fig. 2). In a true high-frequency hearing loss, those high-BF fibers (to the right of the solid vertical lines in Fig. 4B and C) probably would not be present.

Fig. 4.

Average driven rate (rate minus SR) per fiber as a function of level in 0.4-octave BF channels. The stimulus was a 2-kHz tone (dotted vertical line), and the sound level (in dB SPL) is the parameter on the curves. Results are shown for the normal, mildly impaired, and moderately impaired populations. The solid vertical lines show the BF channels at which best thresholds return to near normal for higher BFs. Note that data were not typically collected from AN fibers in the normal population at levels above about 90 dB SPL.

For the entire-population estimate, average rate was then combined, taking the spiral-ganglion cell density along the BM into account (Keithley and Schreiber 1987). In some conditions, the contribution of the three highest BF channels was evaluated by repeating the calculations with the responses in these channels set to zero (e.g., dotted curves in Figs. 7 and 8).

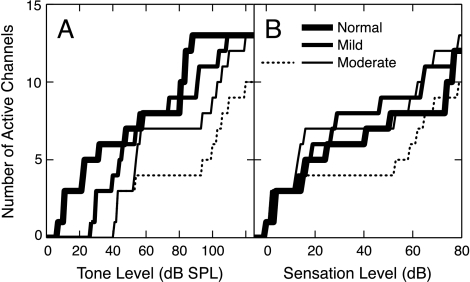
Fig. 7.

The spread of excitation versus sound level. The number of 0.4-octave channels having an average driven rate of at least 1.4 spikes/s/fiber is plotted as a function of (A) tone level and (B) sensation level (dB re threshold in the 2-kHz BF channel). The stimulus is the 2-kHz tone. Populations are identified in the legend. Solid lines: all channels shown in Fig. 4 are included. Dotted line: the three channels with BFs above 4 kHz are excluded from the moderate population.

Results

The results in this paper are computed from responses to 2-kHz tones, BF tones, and 1-kHz tones (Heinz and Young 2004). The 2-kHz results are shown because loudness data are usually taken with tonal stimuli, and this frequency is the center of the noise exposure used to produce acoustic trauma; results similar to those reported here were obtained for 1-kHz tones.

Estimates of basilar membrane slope

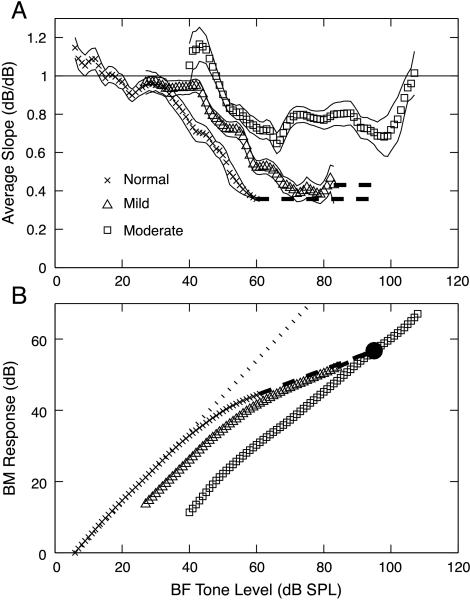
Data on BM motion suggest a direct correlate of loudness recruitment in that the growth of BM velocity with sound level is steeper with SNHL (Ruggero and Rich 1991; Fridberger et al. 2002). Psychophysical masking data in normal and impaired human observers lead to the same conclusion (Oxenham and Plack 1997). Figure 5 shows that the same behavior is observed for BM slope estimates from AN fibers, using the method of Yates et al. (1990).

Fig. 5.

Estimated BM slopes and input–output functions derived from AN rate functions. (A) Slope estimates averaged across three populations of fibers, defined in the legend. Estimates were smoothed as described in Methods. Thin lines are plotted one standard error away from the slope estimates. (B) Estimated BM input–output functions computed by integrating the slopes in A. The dotted line shows 1 dB/dB response growth. The dashed lines show extrapolations of the slope estimates. The constants of integration were chosen to make the functions equal at 95 dB SPL (filled circle).

Slope ratios are shown in Figure 5A for the normal, mild, and moderate populations. In each case, slopes are near 1 dB/dB at low sound levels, which is expected because BM responses are approximately linear near threshold. The slope ratios are less than 1, consistent with compression at BF, for higher sound levels. The impaired slopes flatten out at values near 0.4 (mild loss) and 0.8 (moderate loss) dB/dB. The slopes from normal ears did not reach saturation at the highest level for which BM slope estimates could be made (limited by AN rate saturation to BF tones); they have a slope of 0.36 dB/dB at the last data point. This value matches the BM slope of 0.33 used by Sachs et al. (1989) to model cat AN fiber rate functions, although it is larger than estimates of BM compression from direct measurements in chinchilla (0.2, Ruggero et al. 1997) and from masking experiments in humans (0.2, Oxenham and Plack 1997; Nelson et al. 2001; Plack and O’Hanlon 2003). At very high levels, the relative slope returns to 1 in the moderately impaired population, also consistent with BM data (Ruggero et al. 1997).

To show how BM input–output functions might look, the slopes in Figure 5A were integrated across level to estimate BM response functions (Fig. 5B). These functions were extrapolated for the normal and mildly impaired populations by fixing the slopes at their highest-level values, as shown by the thick dashed lines. The BM response functions were assumed to be equal at 95 dB SPL. In the moderate-loss case, the maximum compression (i.e., lowest slope) is only 0.7–0.8 dB/dB, and the BM input–output function is shifted to the right by >20 dB. In the mild-loss case, the compression is close to the normal case except that the onset of compression is shifted to a higher sound level, similar to recent psychophysical estimates of BM input–output functions in listeners with mild hearing losses (Plack et al. 2004).

Slopes of response growth in individual auditory-nerve fibers

The results in Figure 5 are consistent with models of recruitment that postulate a steeper suprathreshold growth of peripheral response with sound level. However, AN rate functions are not consistently steeper after NIHL (Heinz and Young 2004); they reflect changes in the physiology of the inner hair cells as well as the basilar membrane, so that they do not behave as predicted from Figure 5A. Across a variety of stimuli, impaired slopes are steeper only for vowels or at high sound levels (>80 dB SPL). At high sound levels, there is an apparent change in the mode of stimulation of AN fibers in the cat, the so-called component 1/component 2 (C1/C2) transition (Liberman and Kiang 1984). Rate functions at high sound levels, corresponding to C2 responses, are very steep (Heinz and Young 2004).

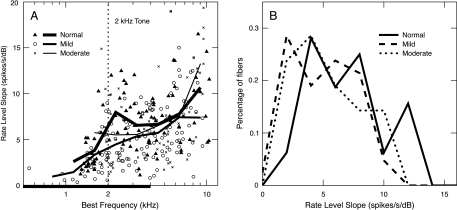
Most important for loudness data, slopes are not steeper for tones. Figure 6A shows the BF dependence of low-level slopes in response to 2-kHz tones for the three populations. For tones, slopes are expected to be shallower for frequencies at and above BF, compared with frequencies below BF (Sachs and Abbas 1974). This effect is observed in the drop-off of the slopes at BFs below 2 kHz for normal and mildly impaired fibers. For BFs between 2 and 4 kHz, impairment produced shallower slopes in response to the 2-kHz tone, as illustrated by the distributions of slopes in Figure 6B. Across this BF range, the slopes from the mild-loss population were significantly less than normal (p < 0.01). Slopes from the moderate loss population were reduced, but not significantly so (p > 0.05). Steeper slopes were observed for BFs above 7 kHz in the moderately impaired population, which is also not statistically significant (p > 0.05). These slopes were computed from responses at high sound levels (>80 dB SPL), where many responses are likely C2. Such high levels are not relevant to recruitment phenomena in moderate hearing losses, which depend on slopes at 50–80 dB SPL.

Fig. 6.

(A) Low-level slopes of rate functions in response to 2-kHz tones are plotted versus BF for the three populations, identified in the legend. Points are the results for individual fibers, and the solid lines are moving averages based on an octave-wide triangular window advanced in half-octave steps. All SR groups were included in the averages, and a minimum of three fibers was required within each window. The range of significant threshold shift in the impaired populations is shown by the heavy line on the abscissa. (B) Distributions of low-level slopes are shown for each population. Only AN fibers with BFs within the elevated threshold region and above the tone frequency (i.e., 2–4 kHz) were included. BFs below 2 kHz were excluded because of the normal frequency dependence in slope for fibers with BFs below the tone frequency (see text).

The finding that rate-level slopes are not steeper after impairment contradicts the results in Figure 5, which suggested steeper response growth for the BM in impaired ears. The difference is that relative slopes were estimated in Figure 5, but absolute slopes are shown in Figure 6. Presumably, the latter are less steep in impaired ears because of IHC damage (as discussed in Fig. 1), which would make both the BF and 1-kHz tone responses shallower (Heinz and Young 2004).

Spread of excitation and summed activity in the whole auditory-nerve population

If it is accepted that rate functions for individual AN fibers are not steeper than normal after impairment, then recruitment must result from other factors that produce steeper activity growth. Often, such factors are assumed to involve abnormally rapid spread of excitation in populations of fibers because of broadened tuning (e.g., Kiang et al. 1970; Evans 1975).

Figure 7 shows the spread of activity as stimulus level increases for the three AN-fiber populations. Fiber responses were averaged in channels of 0.4 octave according to BF, and the number of active BF channels is plotted as a function of stimulus level (Fig. 7A) and sensation level (SL, defined for the AN data as dB re threshold in the 2-kHz BF channel) (Fig. 7B). A channel was considered “active” when its average rate per fiber was more than 1.4 spikes/s. This criterion produced a threshold in the 2-kHz channel that was within 1 dB of the minimum threshold of all AN fibers in that channel for each population. The spread of excitation within the two impaired populations is only slightly more rapid than normal. The number of active channels in the moderate-loss population grows more quickly than normal between 10 and 15 dB SL, whereas the growth in the mild-loss population is more rapid between 20 and 30 dB SL. Although this effect is qualitatively like recruitment, it is unlikely to be a general explanation for recruitment. This effect is primarily because of the high-BF channels (i.e., above 4 kHz, see Fig. 4), which include near-normal AN fiber thresholds (Fig. 2) that are not likely to be present in a true high-frequency hearing loss. When these high BF channels are excluded (dotted lines in Fig. 7), recruitment is not observed until much higher levels.

Figure 8 shows AN activity summed across all BF channels. Figure 8A plots driven rate on a linear scale, similar to typical AN-fiber rate functions, whereas Figure 8C and D plots rate on a logarithmic scale, similar to typical loudness functions. Figure 8D shows rate as a function of sensation level (SL) to allow comparison of the slopes in different populations. Summed rate for each population is shown only up to the levels for which data were available in all BF channels. Figure 8D shows that slopes are not steeper in the impaired ears than in the normal ears, except at high levels. In the moderate population, the slope is initially shallower, then is steeper beginning about 20 dB SL. The steepening in this case again results largely from high BF fibers with near normal thresholds. Excluding the responses from the three BF channels above 4 kHz produces a shallower response growth at levels above about 20 dB SL in the impaired populations (dotted red and green curves). At higher levels (>40 dB SPL or 80 dB SPL), the increased steepness reflects C2 responses in the impaired fibers.

To compare the results more directly with recruitment in human ears, the results of binaural loudness balances were simulated by plotting the levels necessary to produce equal average rates in normal and impaired ears. This calculation simulates interaural loudness balances in persons with unilateral impairment (e.g., Moore et al. 1985; Zeng and Turner 1991; Moore 2004), under the assumption that equal average rates would give equal loudness. Figure 8B shows the results of these matches for the data in Figure 8A. The dashed black line shows the typical behavior of human subjects with recruitment, as defined by Moore (2004). Such matches show a slope near 1 within 4–10 dB of threshold, then an increased slope up to the point of equal levels (assumed here to be 95 dB SPL). For levels within 30 dB of threshold, the AN rate data show a slope near or below 1 for both impaired populations. Significantly steeper slopes are not observed at levels below 60 dB SPL for the mild-loss population and at levels below 75 dB SPL for the moderate-loss population. Again, the steeper slopes are not seen if fibers from the three highest-BF channels are removed (dashed lines).

Threshold distributions and summed activity in a channel near 2 kHz

Current psychophysical evidence suggests that loudness growth for tones depends on responses over a narrow range of BFs near the stimulus frequency (Moore et al. 1985; Hellman and Meiselman 1986; Zeng and Turner 1991). One effect that could lead to recruitment in AN activity is compression of the threshold distribution in impaired ears (Moore et al. 1985; Zeng and Turner 1991). Figure 9 shows that AN fiber threshold distributions are not compressed in our data. Thresholds were analyzed in a way that avoids three effects that could artificially increase the variance of thresholds in impaired ears (i.e., effects that could obscure the occurrence of threshold compression). First, thresholds were analyzed within individual cats, rather than across the whole population. This approach prevents inclusion of the variance in hearing loss from one impaired cat to the next. Second, data collected after the occurrence of a threshold shift in a given impaired experiment, which sometimes occurred (Heinz and Young 2004), were excluded. Finally, only BFs between 1 and 3 kHz were considered because the hearing losses in this region were roughly constant across BF.

Fig. 9.

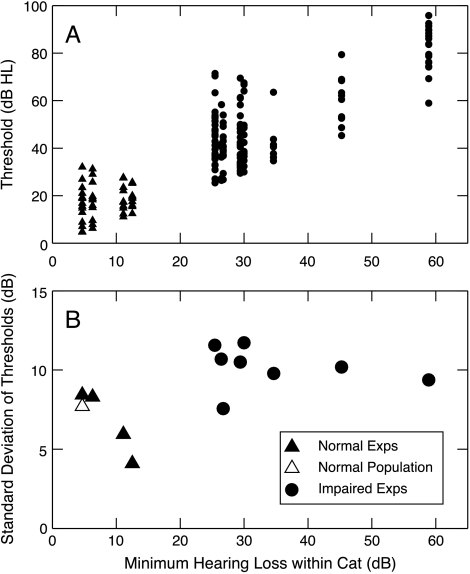
The effect of impairment on AN fiber threshold distributions. (A) Tuning curve thresholds for BFs from 1 to 3 kHz are plotted as a function of minimum threshold for normal and impaired ears. These are all the cases in which there were five or more fibers in this BF range. Thresholds are given in dB HL, i.e., relative to the best thresholds in normal cats from our supplier (NBTC, Miller et al. 1997). Data from both mild and moderate impairments are shown. (B) Standard deviations of the threshold distributions in A are plotted as a function of minimum threshold. Filled symbols: individual experiments. Open triangle: entire normal-hearing population.

Figure 9A shows thresholds of single fibers plotted against the threshold shift at the same BF, defined as the lowest fiber threshold within the analysis band relative to the lowest threshold observed in normal cats from our supplier (NBTC in Fig. 2). The standard deviations (STDs) of these threshold distributions (Fig. 8B) are actually larger in the hearing-impaired cats (mean STD across cats, 10.2 dB) than in the normal-hearing cats (mean STD across cats, 6.7 dB; STD of thresholds across all normal cats, 7.7 dB). Thus the present data show no evidence for the assumptions used in a model of recruitment based on a compressed threshold distribution (Zeng and Turner 1991).

Figure 10 shows the growth of AN activity summed across a single 0.4-octave channel centered on 2 kHz. As in Figure 8, the results for the three populations are compared in various ways. Again, rates do not grow more rapidly with level within 30 dB of threshold (Fig. 10D) and, in fact, grow more slowly than normal for the moderate-loss group over this level range. The corresponding rate matches are shown in Figure 10B. For both populations, the slopes of the rate matches are less than or equal to 1 for sound levels up to 90 dB SPL. The only evidence for steeper growth is at very high levels in cases of moderate impairment, again because of C2 responses. Thus models of loudness based on summing across a narrow range of BFs are not supported by our data.

Discussion

Rate growth in the auditory nerve is not consistent with loudness recruitment

We have assumed, in this paper, a direct relationship between loudness and summed discharge rate across some population of AN fibers. This is the most common assumption for the neural correlate of loudness and underlies most loudness theories. For example, such a relationship is implicit in psychophysical models of loudness that are based on summation of excitation patterns across an array of auditory filters (Zwicker and Scharf 1965; Moore and Glasberg 1997, 2004).

Previously, a direct relationship between rate and loudness has been challenged on various grounds. Pickles (1983) compared AN responses to perceptual loudness summation (Zwicker et al. 1957) and showed that total AN activity inferred from single fiber recordings did not show the behavior of loudness growth with changes in stimulus bandwidth. Relkin and Doucet (1997) measured the peristimulus compound action potential from the AN, which provides a direct measure of total AN activity. They found that the growth of total AN activity in response to tones was significantly shallower (on a log–log scale) than the growth of loudness, and that this discrepancy increased as frequency increased above 1 kHz. The frequency dependence of total AN activity growth is inconsistent with the frequency-independent growth of loudness above 1 kHz (Scharf 1978), and thus Relkin and Doucet concluded that loudness is not simply proportional to total AN activity.

The present results also imply that loudness is not directly related to summed discharge rate in the AN. In our case, we can add that changes in the slope of AN summed activity after impairment do not correspond to changes in the slope of loudness growth after impairment. When the whole AN population was considered, the more rapid spread of excitation because of broadened tuning (Fig. 7) was not a significant enough factor to produce steeper than normal growth at levels within about 30 dB of threshold (Fig. 8), in contrast to human loudness growth (Buus and Florentine 2002; Moore 2004). This was true even for the moderate-loss population in which almost all fibers with BFs between 1.5 and 4 kHz had broadened tuning. When only BFs near the tone frequency were considered, summed AN growth was shallower than normal (Fig. 10), contradicting hypotheses based on a change in the threshold distribution of AN fibers as a recruitment mechanism (Moore et al. 1985; Zeng and Turner 1991).

As discussed in Figure 1, there are two factors that regulate the steepness of AN rate functions. First, damage to OHCs steepens rate functions, in fibers of sufficiently high thresholds, because of the loss of BM compression. This effect has been demonstrated using ototoxic antibiotic poisoning (Evans 1975; Schmiedt and Zwislocki 1980; Harrison 1981). Second, damage to IHCs decreases the slope of rate functions (Heinz and Young 2004), presumably because of degradation of transducer function (Liberman and Kiang 1984; Sewell 1984b). In ears with a mixed hair cell loss, the net change in the slope of rate growth will depend on the relative size of these effects. In our acoustically traumatized animals, the factors decreasing rate-function slopes seem to be dominant (see also Kiang et al. 1970; Salvi et al. 1983). It seems likely that a mixed hair cell loss, as produced by acoustic trauma, is more typical of the hearing loss suffered by hearing-aid users than a pure OHC loss, as discussed below. Thus we conclude that our data are inconsistent with current theories of recruitment that postulate a peripheral explanation for the phenomenon, in which a change in the properties of the BM or of AN fibers leads to a steeper growth of the activity conveyed to the brain by the AN. Even when the plausible assumption is made that the central nervous system could adjust its gain after impairment to normalize total activity at high sound levels, all conditions except one (mild loss, excluding high BFs) are inconsistent with recruitment (Fig. 11).

Fig. 11.

Simulation of a central gain change after impairment that normalizes total AN activity at high sound levels. The summed AN data from Figures 8 and 10 were recomputed by normalizing the impaired population responses to match the saturated normal response at 95 dB SPL (solid lines). The dotted lines show the same calculation when the three highest BF channels are deleted. (A, B) The renormalized summed responses for the entire-population calculations from Figure 8, with the same conventions used as in Figure 8C and B. (C, D) The renormalized summed responses for the 2-kHz BF channel calculations from Figure 10. The mild-loss case without high BFs (thicker dotted line in A and B) provides an example of how recruitment-like effects can be produced by a change in central gain to overcome reduced peripheral activity. However, in all other cases, the renormalization does not alleviate the discrepancy between summed AN activity and recruitment.

Problems of sampling auditory-nerve fibers

One uncertainty that must be considered regarding this conclusion is that, in any AN single-fiber study, there are problems of inadequate sampling. The method of constructing average rate plots (Figs. 4, 8, and 10) was designed to minimize the effects of uneven sampling along the BF axis and among the SR groups. However, a problem of inadequate sampling of high-threshold fibers remains. The distribution of thresholds of AN fibers has a long tail extending toward high sound levels, to an extent that depends on the prior sound-exposure history of the animal (Kiang et al. 1976; Liberman 1978). Our threshold distributions (Fig. 9) probably undersample these fibers. This is likely to be particularly true in the normal population, which may account for the smaller standard deviations of normal thresholds in Figure 9B. However, it is unlikely that a more complete sampling of these few fibers would change the conclusions regarding the role of threshold distributions for recruitment because our data suggest that the dominant effect is the reduction in rate function slopes because of IHC damage. Our conclusions are based primarily on sound levels within 35 dB of threshold (Figs. 8 and 10), whereas the high-threshold fibers would only be expected to influence the slope of summed AN rate at higher levels.

Recruitment and the nature of the hearing loss

A significant uncertainty is the possibility of differences in the status of IHCs between our preparations and the impaired listeners that have been studied. Based on histological analysis of preparations given the same trauma by Liberman and Dodds (1984a,b) and Liberman and Kiang (1984), the lesion in the ears studied here is expected to consist of mixed damage to IHCs and OHCs. Examination of tuning curves is consistent with that prediction (Heinz and Young 2004). The hearing loss can be divided between IHC and OHC components using a model of the cochlea (Bruce et al. 2003); the OHC loss is determined by the degree of widening of the tuning curve, and the remaining loss is attributed to IHC damage. With this method, losses like those in Figure 2 yield about equal IHC and OHC damage, i.e., 10–30 dB of threshold shift accounted for by each. This contrasts with estimates in human observers, for whom most of the loss (70–90%) is usually attributed to OHC damage, on the basis of auditory filter widths and a model of loudness growth (Moore and Glasberg 1997, 2004; Moore et al. 1999a). Inner hair cells are thought to affect recruitment because loudness matching functions, analogous to Figures 8B and 10B, can show incomplete recruitment (loudness in the impaired ear never achieves equality with the normal ear) and also show reduced slopes in cases of partial or complete (dead zones) IHC damage. However, the slopes of published loudness balance functions still typically exceed 1 dB/dB at levels slightly above threshold, even in cases of incomplete recruitment (e.g., Miskolczy-Fodor 1960; Zeng and Turner 1991; Moore and Glasberg 1997; Moore 2004; however, see Stillman et al. 1993). By contrast, neural rate balance functions have slopes less than or equal to 1 up to 35 dB above threshold (Figs. 8B and 10B). An important question raised by our results is whether cats with the hearing losses we have studied show recruitment. This is an experimental question that is currently under study.

It is possible that AN rate responses could account for recruitment in cases of isolated OHC loss, e.g., in kanamycin-treated ears, although this remains unproven. Even if this were to be demonstrated, such a result would not diminish the importance of our conclusions. The present results suggest that AN rate responses do not account for recruitment whenever there is dysfunction in the IHC transduction process, even in cases with significant OHC damage. A reduction in the maximum IHC transduction current is likely to occur in cases of noise-induced hearing loss because of stereocilia damage (Liberman and Kiang 1984), as well as in cases of age-related hearing loss (presbycusis) because of reduced endocochlear potential (EP) associated with dysfunction of the stria vascularis (Sewell 1984a,b; Schuknecht 1993; Schmiedt et al. 2002). Given the prevalence of these two etiologies and recruitment in people with SNHL, our results provide a significant constraint on peripherally based theories of recruitment.

Alternative hypotheses for loudness recruitment

The BM input–output function appears to be an important factor in many aspects of auditory perception in normal and impaired listeners (Moore 1995; Moore and Oxenham 1998; Moore et al. 1999b), particularly related to loudness recruitment (Schlauch et al. 1998; Buus and Florentine 2002). The correlation of recruitment with other perceptual properties thought to be related to cochlear nonlinearity has led to the assumption that recruitment is primarily associated with OHC damage (Moore et al. 1999b). The present results raise the essential question of how to explain the correlation between loudness growth and the BM input–output function, given that the absolute growth of AN fiber discharge rate is not directly related to BM response growth, especially in the presence of IHC damage.

One possibility is that recruitment results from changes in BM response properties that are different from, but strongly correlated with, the loss of compression (Moore et al. 1999b). Two well-studied possibilities are the presumably related changes in the width of tuning and in the nonlinear phase behavior of BM and AN responses (Anderson et al. 1971; Liberman and Dodds 1984b; Ruggero and Rich 1991; Miller et al. 1997; Ruggero et al. 1997; Fridberger et al. 2002; Heinz et al. 2005). In addition to its effects on spread of excitation in the AN, changes in the width of tuning of AN fibers will change the degree of synchrony of AN fibers of different BFs. That is, broader tuning curves mean that fibers of different BFs will respond more similarly in response to the envelopes of complex stimuli because they now have more frequency components in common within their tuning curves (Miller et al. 1997). For narrowband stimuli, such as tones, broader tuning curves also produce an increase in across-BF correlation because of the associated shallower phase transitions near BF (Carney 1994). Such across-BF synchrony could, in turn, lead to a broadening of the specific tonotopic connections in central nuclei because of synchrony-driven synaptic plasticity (e.g., Snyder et al. 1990; Rajan et al. 1993; Schwaber et al. 1993; see also Thai-Van et al. 2003). This effect would lead to a more rapid spread of excitation in central neurons, an effect that would occur independently of spread of excitation in peripheral neurons and would thus be immune to the peripherally based arguments against spread of excitation as a mechanism for recruitment (e.g., Hellman 1978; Moore et al. 1985; Hellman and Meiselman 1986; Zeng and Turner 1991).

The second phenomenon is the nonlinear phase behavior of AN fiber (Anderson et al. 1971) and BM (Ruggero et al. 1997) responses, which provides a strong intensity cue at low to mid frequencies (Carney 1994; Heinz et al. 2001; Colburn et al. 2003). This nonlinear temporal response property is reduced after NIHL (Heinz et al. 2005), in that neurons show less variation in their phase response to a tone as level varies. Like the synchrony argument in the previous paragraph, the nonlinear phase effect depends on neural mechanisms that are sensitive to the synchrony of discharges in spike trains from AN fibers with different BFs. An across-BF correlation mechanism could encode the BM magnitude response independent of IHC damage and thus could theoretically produce recruitment effects related specifically to OHC damage. Hypotheses such as these two can be evaluated by studying cochlear nucleus neurons, especially after SNHL.

Ultimately, of course, recruitment must be found in the properties of central auditory neurons. There are good reasons to think that the actual changes that produce recruitment are changes in the input–output functions or hyperexcitability of central neurons, secondary to peripheral impairment. Acoustic trauma, cochlear ablation, or hair-cell degeneration cause evoked potentials recorded in central auditory structures to show faster amplitude growth with sound level and/or larger maximum values, often in the absence of similar changes in the cochlear action potential (Saunders et al. 1972; Popelar et al. 1987; Salvi et al. 1990; Syka et al. 1994; Szczepaniak and Møller 1996). Similar results have been obtained in mouse mutants that are deaf from birth (Bock et al. 1982) or that lack OHCs (Sterbing and Schrott-Fischer 2002). Neurons in the CN and inferior colliculus show larger excitatory synaptic currents in deaf compared with control preparations (Oleskevich and Walmsley 2002; Vale and Sanes 2002), providing a mechanism by which the postulated central recruitment could occur. Reduction in central inhibition could also contribute (e.g., Bledsoe et al. 1995; Wang et al. 1996; Willott et al. 1997; Vale and Sanes 2002). It is possible that the changes that occur centrally after impairment (e.g., changes in synaptic gain and/or connection strength) are an adjustment to overcome the general reduction in AN activity following impairment (Liberman and Dodds 1984a). This adjustment could produce recruitment effects by overcompensating for high-level sounds (e.g., the mild-loss case without high BFs in Fig. 11B), or it could simply allow recruitment-like effects related to the cross-BF properties of cochlear nonlinearity to be observed.