Intensive Intervention for Students with Mathematics Disabilities: Seven Principles of Effective Practice

Abstract

The focus of this article is intervention for third - grade students with serious mathematics deficits at third grade. In third grade, such deficits are clearly established, and identification of mathematics disabilities typically begins. We provide background information on two aspects of mathematical cognition that present major challenges for students in the primary grades: number combinations and story problems. We then focus on seven principles of effective intervention. First, we describe a validated, intensive remedial intervention for number combinations and another for story problems. Then, we use these interventions to illustrate the first six principles for designing intensive tutoring protocols for students with mathematics disabilities. Next, using the same validated interventions, we report the percentage of students whose learning outcomes were inadequate despite the overall efficacy of the interventions and explain how ongoing progress monitoring represents a seventh, and perhaps the most essential, principle of intensive intervention. We conclude by identifying issues and directions for future research in the primary and later grades.

Approximately 5-9% of the school-age population may be identified with mathematics disability (e.g., Badian, 1983; Gross-Tsur, Manor, & Shalev, 1996). Although this estimate is similar to the prevalence of reading disability, less systematic study has been directed at mathematics disability (Rasanen & Ahonen, 1995), even though poor mathematics skills are associated with life-long difficulties in school and in the workplace. For example, mathematics competence accounts for variance in employment, income, and work productivity even after intelligence and reading have been explained (Rivera-Batiz, 1992).

Some research illustrates how prevention activities at preschool (e.g., Clements & Sarama, 2007), kindergarten (e.g., Griffin, Case, & Siegler, 1994), or first grade (e.g., Fuchs, Fuchs, Yazdian, & Powell, 2002) can substantially improve math performance. For example, at the beginning of first grade, Fuchs and colleagues (2005) identified 169 students in 41 classrooms as being at risk for math difficulties based on their low initial performance. These children were randomly assigned to a control group or to receive small-group tutoring three times per week for 16 weeks. Results showed that math development across first grade was significantly and substantially superior for the tutored group than for the control group on computation, concepts, applications, and story problems. In addition, the incidence of students with mathematics disability was substantially reduced at the end of first grade, and this reduction in mathematics disability remained in the spring of second grade, one year after tutoring ended (Compton, Fuchs, & Fuchs, 2006).

Nevertheless, despite the efficacy of tutoring, students were not universally responsive. A subset of the tutored students, approximately 3-6% of the school population (depending on the measure and cut-point for the severity of mathematics performance used), manifested severe mathematics deficits. As this example illustrates, the need for intensive remedial intervention persists even when prevention services are generally effective.

In this article, we focus on serious mathematics deficits at third grade. In third grade, serious mathematics deficits are clearly established, and identification of mathematics disabilities typically begins (Fletcher, Lyon, Fuchs, & Barnes, 2007). We provide background information on two aspects of mathematical cognition that present major challenges for students in the primary grades: number combinations and story problems. We describe validated, intensive interventions for number combinations and another for story problems and use these interventions to illustrate six principles for designing interventions for students with mathematics disabilities. Next, using the same validated interventions, we report the percentage of students whose learning outcomes were inadequate despite the overall efficacy of the interventions and we how ongoing progress monitoring therefore represents a seventh and perhaps the most essential principle for intensive intervention. We conclude by identifying issues and directions for future research in the primary and later grades.

Number Combinations and Story Problems

Number combinations refer to problems with single-digit operands, which can be solved by counting or committing to long-term memory for automatic retrieval. Widespread agreement exists that number combination skill is essential for all children to acquire. The National Research Council (Kilpatrick, Swafford, & Findell, 2001), for example, identified number combination skill as a key component of math proficiency. Moreover, research shows that number combination skill is a significant path to procedural computation and story-problem performance (Fuchs et al., 2006).

To answer number combination problems, typical children gradually develop procedural efficiency in counting. First they count the two sets (e.g., 2+3) in their entirety (i.e., 1, 2, 3, 4, 5); then they count from the first number (i.e., 2, 3, 4, 5); and eventually they count from the larger number (i.e., 3, 4, 5). Also, as their conceptual knowledge about number becomes more sophisticated, children develop additional back-up strategies for deriving answers (e.g., decomposition: [2+2] = 4 + 1 = 5). As increasingly efficient counting and back-up strategies help children consistently and quickly pair problems with correct answers in working memory, associations become established in long-term memory, and children gradually favor memory-based retrieval of answers, although most continue to use a mix of strategies over time.

Students with mathematics disabilities, on the other hand, manifest greater difficulty with counting (e.g., Geary, Bow-Thomas, & Yao, 1992; Geary, Hoard, Byrd-Craven, Nugent, & Numtee, in press). They also persist with immature back-up strategies. Not surprisingly, therefore, they fail to make the shift to memory-based retrieval of answers (Fleishner, Garnett, & Shepherd, l982; Geary, Widaman, Little, & Cormier, l987; Goldman, Pellegrino, & Mertz, l988). When children with mathematics disabilities do retrieve answers from memory, they commit more errors and manifest unsystematic retrieval speeds compared to younger, typically developing counterparts (e.g., Geary et al., in press; Geary, Brown, & Samaranayake, l991; Gross-Tsur et al., l996; Ostad, l997). In fact, number combinations constitute a signature deficit of students with mathematics disabilities (e.g., Fleishner et al., 1982; Geary et al., 1987; Goldman et al., 1988).

Conventionally, number combinations are incorporated into the curriculum at kindergarten through second grade, although most general education programs do not allocate systematic attention to developing strategies for fluent number combination performance. Nevertheless, most typically developing students are well on their way toward automatic retrieval of number combinations as their major solution strategy by the beginning of third grade. Therefore, when students still manifest deficiencies involving number combinations at the beginning of third grade, a pressing need exists for remediation.

In contrast to number combinations and other aspects of calculation skill, story problems incorporate linguistic information that requires children to construct a problem model. Whereas a calculation problem is already set up for solution, a story problem requires students to use text to identify missing information, construct the number sentence, and derive the calculation problem for finding the missing information. This transparent difference would seem to alter the nature of the task and, in fact, some research suggests that computation and math problem solving may be distinct aspects of mathematical cognition (Fuchs et al., in press).

Four large-scale studies have addressed indirectly the distinction between computation and problem solving by examining the cognitive correlates of these domains of mathematical cognition in large representative samples.

In a study of 353 first through third graders, Swanson and Beebe-Frankenberger (2004) identified working memory as an ability that contributed to strong performance across both areas of mathematical cognition, but some unique cognitive abilities were also important, including phonological processing for computation and fluid intelligence as well as short-term memory for story problems.

In the second study, Swanson (2006) followed these students' development of calculation and problem-solving skill over one year. He identified predictors of computation (inhibition or controlled attention, vocabulary knowledge, visual-spatial working memory) that differed from problem solving (working memory's executive system; i.e., listening span, backward digit span, and digit/sentence span).

The third study involved a sample of 312 third graders. Here Fuchs et al. (2006) examined the concurrent cognitive correlates of computation versus story problems, this time controlling for the role of arithmetic skill within story problems. Teacher ratings of inattentive behavior emerged as a correlate common to both domains of math, but the remaining abilities differed: for computation, phonological decoding and processing speed; and for story problems, nonverbal problem solving, concept formation, sight word efficiency, and language.

Finally, the fourth study (Fuchs et al., 2005) used beginning-of-the-year cognitive abilities to predict the development of skill across the year among 272 first graders. Results again suggested some common and some unique patterns of cognitive abilities. The common predictors were working memory and ratings of attentive behavior. The unique predictors were phonological processing for computation and nonverbal problem solving for simple word problems.

Across these studies, some findings recur; others are idiosyncratic. But together, results indicate that some abilities underlying these aspects of mathematical cognition are unique. This provides the basis for hypothesizing that the cognitive dimensions underlying difficulty in each of these domains may also be distinct.

More recently, Fuchs et al. (in press) assessed this question directly. Using a sample of 924 third graders from 89 classrooms, we (a) assessed the students on computation and problem solving; (b) classified them as having difficulty with computation, problem solving, both domains, or neither domain; and (c) measured them on nine cognitive dimensions. Difficulty occurred across the two math domains with the same prevalence as difficulty with a single domain, and specific difficulty with calculation occurred as frequently as specific difficulty with story problems. Moreover, specific computational difficulty was associated with a strength in language and weaknesses in attentive behavior and processing speed. By contrast, problem-solving difficulty was associated with poverty and deficient language.

These findings lend support to the hypothesis that computation and problem solving represent distinct domains of mathematical cognition in students at the lower ranges of performance, who might be identified with mathematics learning disabilities in the schools. Together, therefore, these lines of research suggest that practitioners need to consider computational skill and problem-solving skill separately in diagnosing and instructing students with learning disabilities.

Number Combination Deficits in Third Graders: A Validated Intervention

Math Flash

The number combinations intervention we researched is referred to as Math Flash because number combinations “flash” during the computerized practice activity. The Math Flash protocol relies on scripts (a) to clarify for tutors how to frame precise, effective explanations; and (b) to provide tutors a concrete model for how to implement the lessons. Tutors study scripts; they do not read them. Each lesson lasts 20-25 minutes, and the Math Flash standard protocol runs 16 weeks, with three sessions per week.

Math Flash addresses the 200 number combinations with addends and subtrahends from 0 to 9. Math facts are introduced in a deliberate order. For the first two lessons, tutors address facts of + 1 and - 1, using manipulatives and the number line, teaching the commutative property of addition, and emphasizing that this property does not apply to subtraction. In the next two lessons, facts of + 0 and - 0 are introduced, again using manipulatives and the number line. In lessons five and six, + 1, - 1, + 0, and - 0 math facts are reviewed.

In lesson seven, students begin learning doubles that run from 0 through 6 (i.e., 0 + 0, 1 + 1, 2 + 2, 3 + 3, 4 + 4, 5 + 5, 6 + 6, 0 - 0, 2 - 1, 4 - 2, 6 - 3, 8 - 4, 10 - 5, 12 - 6). Students work on these doubles using manipulatives and rehearsing doubles chants. At this point in Math Flash, mastery criteria are introduced, and students spend a minimum of one day on each lesson topic (so they do not waste time on facts they already know) and a maximum of four days on each lesson topic (to avoid students getting “stuck” on a topic and losing content coverage). Mastery is assessed in each lesson during computerized practice (see below). After doubles, students learn facts with + 2 and - 2. Manipulatives and the number line practice are used again.

Next, students are taught to use two strategies for answering a math fact. They are taught that if they “just know” the math fact, they “pull it out of your head.” However, if they do not know an answer immediately, they count up. Counting-up strategies for addition and subtraction are taught using the number line and their fingers. To count up addition problems, students start with the bigger number and count up the smaller number on their fingers. The answer is the last number spoken.

For subtraction counting-up, new math vocabulary is introduced. The minus number is the number directly after the minus sign. The number you start with is the first number in the equation. To count up subtraction problems, students start with the minus number and count up to the number you start with. The answer is the number of fingers used to count up. From this point on, during every subsequent lesson, students are reminded to “Know It or Count Up.”

Because students are now equipped with two strategies for answering math facts, the tutor introduces additional sets of number combinations, beginning with the 5 set. This includes all addition problems equaling 5 and all subtraction problems with 5 as the minuend: 0 + 5, 1 + 4, 2 + 3, 3 + 2, 4 + 1, 5 + 0, 5 - 0, 5 - 1, 5 - 2, 5 - 3, 5 - 4, 5 - 5. After mastery of the 5 set, students progress to the 6 set, then the 7 set, and so on: 8, 9, 10, 11, 12, 13, 14, 15, 16, and 17-18. The tutor works with the student on each set for a maximum of four days. Between the 12 set and the 13 set, students work on doubles of 7 through 10 (i.e., 7 + 7, 8 + 8, 9 + 9, 10 + 10, 14 - 7, 16 - 8, 18 - 9, 20 -10). If a student masters all sets before session 48, he/she reviews for the remaining Math Flash sessions.

Each of the 48 Math Flash daily lessons comprises five activities: flash card warm-up, conceptual and strategic instruction, lesson-specific flash card practice, computerized practice with mastery assessment, and paper-pencil review. In addition, throughout every lesson, a systematic reinforcement program is used to motivate good attention, hard work, and accurate work.

With flash card warm-up, tutors show flash cards, one at a time, for 2 minutes. The cards are a representative sample of the pool of the 200 number combinations addressed in Math Flash. Flash cards answered correctly are placed in a “correct” pile. When students answer incorrectly, the tutor instructs them to “count up.” Students count up to produce the correct answer, but the card is placed in the “incorrect” pile. At the end of 2 minutes, the flash cards answered correctly are counted, and the student graphs this number on his/her flash card graph.

During conceptual and strategic instruction, tutors introduce or review concepts and strategies. Throughout, tutors emphasize the two strategies for deriving answers –“Know It or Count Up”– provide practice in counting up, and require students to explain how to count up addition and subtraction problems. Tutors then work with students on the day's number combinations set (e.g., +1 and -1, doubles 0-6, combinations of 12) using the number line and manipulatives.

After the tutor-led lesson, tutors conduct lesson-specific flash card practice for 1 minute. Lesson-specific flash cards consist of the math facts that are the focus of the day's lesson. (For example, if a lesson focuses on the 5 set, lesson-specific flash cards are facts with addends that sum to 5 and with minuends of 5.) Correctly answered flash cards are placed in the “correct” pile. When students answer incorrectly, tutors require them to “count up.” The cards for which an incorrect answer is given are placed in the “incorrect” pile. After 1 minute, the lesson-specific flash cards answered correctly are counted, but the score is not graphed. On the second, third, and fourth days of a given lesson topic, students get a chance to beat their lesson-specific flash card score. Tutors remind students what their score on the first minute was and encourage them to answer more cards during the upcoming minute. Scoring and feedback are the same as in the first minute. Tutors praise students when they beat their score.

For the next 7.5 minutes, students complete computerized practice to build fluency with number combinations and to assess their mastery of the day's number combination set. Math facts presented on the computer game include 10 lesson-specific facts and 5 review facts.

The game goes like this. A math fact flashes on the screen for 1.3 seconds. Students rehearse the fact (e.g., 3 + 2 = 5) while it briefly appears; when the fact disappears, students retype the entire fact (e.g., addends and answer). If the answer is correct, the student hears applause and earns a point. If it is incorrect, the student has another chance to enter the problem correctly. The computer game ends after the student has answered each of the 10 lesson-specific facts correctly twice or after 7.5 minutes. The student then receives feedback on his/her mastery of correctly answered number combinations for that day's session.

Mastery on the lesson-specific number combinations set is assessed automatically as the student completes the computer game. Immediately after the game ends, the computer provides a report to the tutor. If the student answers each of the 10 lesson-specific facts correctly twice before 7.5 minutes elapses, mastered appears on the screen. If not, review appears on the screen. The tutor takes note of the student's mastery status, moving students onto the next number combinations set when mastery occurs, or after four days on a given set.

Finally, students complete a paper-and-pencil review. The student has 1 minute to complete 15 lesson-specific facts on one side of a paper and then has another minute to complete 15 review facts on the other side of the paper. At the end of 2 minutes, tutors circle correct answers and write the score at the top of the paper. Students take home these papers each day.

A systematic reinforcement program is also incorporated. Tutors award gold stars following each component of the tutoring session, with the option to withhold stars for inattention or poor effort. At the end of a session, each gold star is placed on a “Star Chart.” Sixteen stars lead to a picture of a treasure box and, when this goal is reached, the student chooses a small prize from a real treasure box. The student keeps the Star Chart, and a new chart is provided during the next lesson.

Evaluation of Math Flash

During the 2006-2007 school year, Fuchs et al. (2007) randomly assigned 133 students in the Nashville Metropolitan Public Schools and in the Houston Independent School District who experienced substantial difficulty with computation and story problems (a) to receive the Math Flash standard intervention protocol, as just described; (b) to receive the Pirate Math standard intervention protocol addressing story problems, as described next; or (c) to continue in school without any additional math tutoring.

Half of the students in each intervention condition had math difficulties without reading difficulties; the other half experienced concurrent difficulty with math and reading. Each session was audiotaped. A representative sample of lessons was coded for fidelity against the tutoring scripts: fidelity was strong for both interventions. Students were pre- and posttested on measures of fluency with number combinations and on measures of problem solving. Math Flash and Pirate Math students (which incorporated instruction and practice on counting-up strategies, but less drill and practice and less conceptual instruction on number combinations) improved on number combinations fluency significantly more than students in the control group. The effect size comparing Math Flash to the control group was large: 0.85 standard deviation units. The effect size comparing Pirate Math to the control group was somewhat smaller but also substantial: 0.72. The difference in effect sizes may reflect less practice and less conceptual instruction on number combinations in Pirate Math.

Problem-Solving Deficits in Third Graders: A Validated Remediation

Pirate Math

The story-problem intervention is referred to as Pirate Math because posters and materials rely on a pirate theme. Again, scripts are studied, not read. Each lesson lasts 25-30 minutes, and the Pirate Math standard protocol runs 16 weeks, with three sessions per week. The 48 lessons are divided into four units. An introductory unit addresses skills foundational to story-problem instruction. Specifically, tutors (a) teach students the counting-up strategy for solving addition and subtraction number combinations; (b) review double-digit addition and subtraction; (c) teach students to solve for “X” in any position in simple algebraic equations (i.e., a + b = c; x - y = z); and (d) teach students to check their story-problem work.

The remaining three units focus on solving story problems while incorporating and reviewing the foundational skills taught in the introductory unit. Each unit introduces one story-problem type and, after the first problem-type unit, subsequent units provide systematic, mixed cumulative review that includes previously taught problem types. The problem types are Total (two or more amounts being combined), Difference (two amounts being compared), and Change (initial amounts that increase or decrease). In the Total unit, the first of the three problem types taught, tutors teach students to RUN through a problem: a three-step strategy prompting students to Read the problem, Underline the question, and Name the problem type. Students use the RUN strategy across all three problem types.

Next, for each problem type, students are taught to identify and circle relevant information. For example, for Total problems, students circle the item being combined and the numerical values representing that item, and then label the circled numerical values as “P1” (i.e., for part one), “P2” (i.e., for part two), and “T” (i.e., for the combined total). Students mark the missing information with an “X” and construct an algebraic equation representing the underlying mathematical structure of the problem type. For Total problems, the algebraic equation takes the form of “P1 + P2 = T.” The “X” can appear in any of the three variable positions. Students are taught to solve for X, to provide a word label for the answer, and to check the reasonableness and accuracy of work. The strategy for Difference problems and Change problems follows similar steps but uses variables and equations specific to those problem types. For Difference problems, students are taught to look for the bigger amount (labeled “B”), the smaller amount (labeled “s”), and the difference between amounts (labeled “D”), and to use the algebraic equation “B - s = D.” For Change problems, students are taught to locate the starting amount (labeled “St”), the changed amount (labeled “C”), and the ending amount (labeled “E”); the algebraic equation for Change problems is “St +/- C = E” (+/- depends on whether the change is an increase or decrease in amount).

Across problem types, explicit instruction to identify transfer features occurs in four ways. First, students are taught that because not all numerical values in story problems are relevant for finding solutions, they should identify and cross out irrelevant information. Second, students are taught to recognize and solve problems with the missing information in the first or second position. Third, students learn to apply the problem-solving strategies to problems that involve addition and subtraction with double-digit numbers, including those requiring regrouping. Finally, students are taught to find relevant information for solving problems in pictographs, bar charts, and pictures. Across the three problem-type units, previously taught problem types are included for review and practice.

Following the introductory unit (six lessons), each Pirate Math daily lesson comprises four activities: flash card warm-up, conceptual and strategic instruction, problem-type flash card practice, and paper-pencil review. Also, throughout every lesson, a systematic reinforcement program is used to motivate good attention, hard work, and accurate work.

The first activity, flash card warm-up, is identical to the flash card warm-up used for Math Flash. The second activity, conceptual and strategic instruction, lasts 15-20 minutes. Tutors provide scaffolded instruction in solving the three types of story problems, along with instruction on identifying and integrating transfer features, using role-playing, manipulatives, instructional posters, modeling, and guided practice. In each lesson, students solve three problems, with decreasing amounts of support from the tutor.

The third activity is sorting word problems. Tutors read aloud flash cards; one word problem is printed on each card. The student listens to the problem, identifies the word-problem type, and places the card on a mat with four boxes labeled “Total,” “Difference,” “Change,” or “?.” Students do not solve problems. They simply sort the problems into problem type. To discourage students from associating a particular cover story with a problem type, the cards use similar cover stories but with varied numbers, actions, and placement of missing information. After 2 minutes, the tutor notes the number of correctly sorted cards and provides corrective feedback for up to three errors.

For the last activity, paper-and-pencil review, students work independently. They have 2 minutes to complete 10 addition and subtraction number combinations and four addition and subtraction double-digit computation items, two of which require regrouping. Then, students have 2 minutes to complete one story problem on the back of the paper. Tutors provide corrective feedback and note the number of correct problems on the top of sheet. Students take home the paper-and-pencil review sheets. The Pirate Math reinforcement program is similar to the one used for Math Flash.

Evaluation of Pirate Math

In the efficacy study described earlier for Math Flash (Fuchs et al., 2007), one of the three conditions was Pirate Math. In that study, story-problem performance improved significantly more for Pirate Math than for both Math Flash and the control group. The effect size comparing Pirate Math to Math Flash was large (0.72). The effect size comparing Pirate Math to the control group was somewhat larger but in the same range (0.89).

The First Six Principles of Effective Intervention for Students with Mathematics Disabilities

The Math Flash and Pirate Math interventions illustrate six principles of effective intervention for students with mathematics disabilities (see Table 1). The first is instructional explicitness. Typically developing students profit from the general education mathematics program, which relies, at least in part, on a constructivist, inductive instructional style. Students who accrue serious mathematics deficits, however, fail to profit from those programs in a way that produces understanding of the structure, meaning, and operational requirements of mathematics.

Table 1. Seven Principles of Effective Intervention for Students with Mathematics Disabilities.

Instructional explicitness

Instructional design to minimize the learning challenge

Strong conceptual basis

Drill and practice

Cumulative review

Motivators to help students regulate their attention and behavior to work hard

Ongoing progress monitoring

A meta-analysis of 58 math studies (Kroesbergen & Van Luit, 2003) revealed that students with math disability benefit more from explicit instruction than from discovery-oriented methods. Therefore, effective intervention for students with math disability requires an explicit, didactic form of instruction, in which the teacher directly shares the information the child needs to learn.

Explicitness is not sufficient, however. A second and often overlooked principle of effective intensive mathematics intervention is instructional design to minimize the learning challenge. The goal is to anticipate and eliminate misunderstandings with precise explanations and the use of carefully sequenced and integrated instruction so that the achievement gap can be closed as early as possible. This may be especially important for mathematics, which involves many branches and strands that may be distinct, each with its own conceptual and procedural demands. So, given the ever-changing and multiple demands of the mathematics curriculum, instructional efficiency is critical, creating the need for the tutor, or the program on which the tutor relies, to minimize the learning challenges for the student.

Careful instructional design is illustrated in Math Flash in terms of sequencing, where the introduction of number combination sets is ordered to capitalize on the knowledge the student possesses, while maximizing the student's rate of acquisition and sense of accomplishment. So, for example, we introduce + 1/ - 1 first for three reasons: It corresponds well to a basic number line concept that is easy to teach, if not already mastered; it eases counting demands; and it results in the student mastering 29 of the 200 number combinations in the first week of the program. We introduce + 0/- 0 next for three reasons: Although conceptually demanding, it builds well on + 1/- 1; it presents no counting demands; and it efficiently creates mastery of another large group of number combinations. In a related way, foundational concepts are presented only as they become instrumental to learning, and strategies are taught only as they become broadly applicable. So, for example, “Know It or Count Up” is introduced (a) after enough “Know It” combinations (+ 1/- 1, + 0,- 0, easy doubles) have been addressed to make the “Know It” strategy salient for the student and (b) after enough number combinations for which counting-up is an efficient strategy have been introduced.

Instructional design that minimizes the learning challenge is also illustrated in Pirate Math. Take three examples. Pirate Math begins by teaching a set of foundational skills the student can apply across the entire program: counting up for number combinations, two-digit calculations, solving algebraic equations, and checking work. Once story-problem instruction begins, these foundational skills can be taught as intact instructional targets and then applied efficiently across subsequent units.

A second example is that Pirate Math purposefully conceptualizes, organizes, and teaches students to recognize problem types that pertain broadly to the kinds of problems found in the general education curriculum and in high-stakes tests. That way, novel story problems are not random events, each requiring the student to create a solution strategy. Rather, the student recognizes novel problems as familiar, using schemas for problem types the program teaches, and thereby deciphers when to apply which set of solution rules they have learned.

A third example is that Pirate Math conceptualizes transfer within the same problem-type structure, so that irrelevant information, finding missing information in any of the three slots of an equation, and finding relevant information within charts/graphs recur predictably and efficiently across problem-type instructional units.

The third principle of effective intensive mathematics intervention is the requirement that instruction provide a strong conceptual basis for the procedures that are taught. Special education is already strong in emphasizing drill and practice, a critical and fourth principle of effective practice. However, it has sometimes neglected the conceptual foundation of mathematics, and such neglect can result in confusion, learning gaps, and a failure to maintain and integrate previously mastered content. Math Flash illustrates the need for conceptual instruction as it relies strongly on manipulatives and the number line to introduce and review each number combination set. Pirate Math illustrates the need for conceptual foundation with its use of role-playing for each problem type.

Drill and practice, the fourth principle of effective intensive mathematics intervention, is also evident in our sample interventions: in Math Flash, with the use of flash cards, computerized practice, and daily review; in Pirate Math, with practice in sorting problems into problem types, the mixing of problem types within the daily lesson (once at least two problem types have been introduced), and daily review.

We note that this practice is rich in cumulative review, a fifth principle of effective intensive mathematics intervention. This is reflected in Math Flash's flash card warm-up activity, computerized practice, and paper-pencil review. It is also incorporated in Pirate Math's continual reliance on the foundational skills taught in the introductory unit, the use of mixed problem types within conceptual instruction, sorting practice, and paper-pencil review.

Finally, intensive mathematics interventions need to incorporate motivators to help students regulate their attention and behavior and to work hard. Students with learning disabilities often display attention, motivation, and self-regulation difficulties, which may adversely affect their behavior and learning (Fuchs et al., 2005, 2006; Montague, 2007). By the time students enter intensive intervention, they have experienced repeated failure, causing many to avoid the emotional stress associated with mathematics. As a result, they no longer try to learn for fear of failing. For this reason, intensive intervention must incorporate systematic self-regulation and motivators, and for many students, tangible reinforcers are required. Math Flash illustrates this principle (a) using the “beat your score” flash card activity, where goal-directed behavior is required; (b) when students graph scores they achieve; and (c) in the use of stars as rewards. Pirate Math incorporates similar activities to help students regulate attention and behavior and to work hard.

Valid Is Not the Same as Universally Effective: Progress Monitoring as the Most Essential Principle of Intensive Intervention

As summarized in this article (see Fuchs et al., 2007, for a full report), Math Flash and Pirate Math are demonstrably efficacious, resulting in statistically significant and practically important effects on number combinations fluency (i.e., Math Flash and Pirate Math, each compared to a control group) and on problem solving (i.e., Pirate Math, compared both to a control group and to the Math Flash group). Yet, no instructional method, even those validated using randomized control studies, works for all students.

We use Math Flash and Pirate Math to illustrate this point. Although Math Flash resulted in statistically significantly better improvement in number combinations fluency compared to the control group, with an effect size of 0.88, 3 of 21 Math Flash students failed to realize improvement that exceeded the 30th percentile among the 66 study participants. Moreover, although the effects for Pirate Math were statistically significant (effect sizes of 0.82 and 0.97), the improvement/outcome scores of 8 of 22 students did not exceed the 30th percentile – this time, using a comparison group of 159 students whose math performance was typically developing and who received conventional classroom problem-solving instruction over the course of third grade.

When a standard protocol of validated instruction proves ineffective for a given student, this is unexpected because research has shown that the intervention works for the majority of students. Therefore, when unexpected unresponsiveness occurs, we assume the student has individual needs that are unusual or special, such that he or she requires an individually tailored instructional program.

Because schools must assume that validated intervention protocols will work for most, but not all, students, schools need to monitor the effects of interventions on individual children's learning. That way, children who do not respond adequately can be identified promptly, and the teacher can adjust the intervention to develop an individually tailored instructional program that does work for the student. This leads us to propose a seventh and most essential element of intensive remedial programming: ongoing progress monitoring. Progress monitoring is used to determine whether a validated treatment protocol is, in fact, effective for a given student. When progress monitoring reveals that a student is failing to respond as expected to a validated intervention protocol, progress monitoring is then used for a second purpose: to formulate an individually tailored instructional program that is in fact effective for that student.

Curriculum-Based Measurement

In this section, we describe curriculum-based measurement (CBM), the form of progress monitoring for which the preponderance of research has been conducted. CBM differs from most forms of classroom assessment in two major ways (Fuchs & Deno, 1991). First, it is standardized so that the behaviors to be measured and the procedures for measuring them are prescribed, with documented reliability and validity. Second, CBM's testing methods and content remain constant, with equivalent weekly tests that span much, if not all, of the school year. (The primary reason for long-term consistency is so that progress can be monitored systematically and coherently over time.)

To illustrate how CBM is used, let's say Roberto, a hypothetical student, developed sizeable math deficits over the course of first and second grade, despite strong general education programming and despite small-group tutoring implemented during the spring semester of second grade. At the beginning of third grade, Roberto is identified for remedial intervention. Mrs. Hayes, the special education teacher, sets Roberto's mathematics goal for year-end performance as competent second-grade performance (which is transparently connected to the third-grade mathematics curriculum, but includes some easier problem types that create the platform for learning the harder, third-grade problems).

Relying on established methods, Mrs. Hayes creates or identifies enough CBM tests to assess Roberto's performance each week across the school year. Each of these tests systematically samples the second-grade mathematics curriculum in the same way. Each week, Mrs. Hayes administers one computation test in exactly the same way; Roberto has 3 minutes to complete as many problems as he can. The score is the number of correct digits written in answers. Each week, Mrs. Hayes also administers one concepts/applications test in exactly the same way. Roberto has 6 minutes to complete as many problems as he can; the score is the number of correct points written in answers.

Each progress-monitoring test collected across the school year is of equivalent difficulty, and the score on each week's CBM test is an indicator of mathematics competence at second grade. At the beginning of the year, we expect Roberto's performance to be low. As Mrs. Hayes teaches and Roberto learns the second-grade curriculum, we expect Roberto's score to gradually increase. Because each progress-monitoring test collected across the school year is of equivalent difficulty, each week's computation scores can be graphed and directly compared to each other. Also, each week's concepts/application scores can be graphed and compared to each other. Moreover, on each graph (computation; concepts/applications), a slope can be calculated on the series of scores. This slope quantifies Roberto's rate of mathematics improvement in terms of the weekly increase in score. In addition, because each week's assessment samples the annual curriculum in the same way, Mrs. Hayes can derive a systematic analysis of which skills Roberto has and has not mastered at any point in time, and Mrs. Hayes can look across time at a given skill to determine whether Roberto is retaining mastery.

A large body of work indicates that CBM progress monitoring enhances teachers' capacity to plan mathematics programs and to effect stronger mathematics achievement among students with serious learning problems (Fuchs & Fuchs, 1998). To inform instructional planning, teachers rely on the CBM graphed scores. Once the teacher sets the year-end goal, she draws the desired score on the graph at the date corresponding to the end of the year. The teacher then draws a straight line connecting the student's beginning-of-the-year score with the year-end goal. This line is called the goal line. It represents the approximate rate of weekly improvement (or slope) we hope a student will achieve. When a student's trend line (i.e., the slope through the student's actual scores) is steeper than the goal line, the teacher increases the goal for the student's year-end performance. On the other hand, when a student's trend line is flatter than the goal line, the teacher relies on her knowledge about the student along with her CBM analysis of the student's skills, derived from the CBM data, to revise the instructional program in an attempt to boost the weekly rate of student learning.

Research shows that with CBM decision rules, teachers design more varied instructional programs that are more responsive to individual needs (Fuchs, Fuchs, & Hamlett, 1989b), that incorporate more ambitious student goals (Fuchs, Fuchs, & Hamlett, 1989a), and that result in stronger end-of-year scores on commercial, standardized reading tests (e.g., Fuchs, Fuchs, Hamlett, & Stecker, 1991).

Let's return to Roberto and Mrs. Hayes to illustrate how a teacher uses CBM in these ways to monitor the effectiveness of a validated treatment protocol and, when that protocol proves ineffective for an individual student, how a teacher uses CBM to inductively develop an individually tailored instructional program. When Mrs. Hayes assumed responsibility for Roberto's math remediation program, she decided to use the validated protocol Pirate Math. This entailed tutoring for 30 minutes per session three times per week. As Mrs. Hayes began to implement this validated protocol, she also began to administer second-grade CBM tests once each week for computation and once each week for concepts/applications.

After the first three weeks, Mrs. Hayes calculated the median of the first three computation test scores (5) and the median of the first three concepts/application test scores (3). These represented Roberto's baseline or beginning-of-the-year scores. Using CBM guidelines for goal setting, Mrs. Hayes decided that her year-end goal for Roberto would seek a weekly increase of .5 digits for computation and a weekly increase of .6 points for concepts/applications. So 25 weeks later, at the end of the school year, Roberto would score 18 digits correct on second-grade CBM computation and 18 points correct on second-grade CBM concepts/applications.

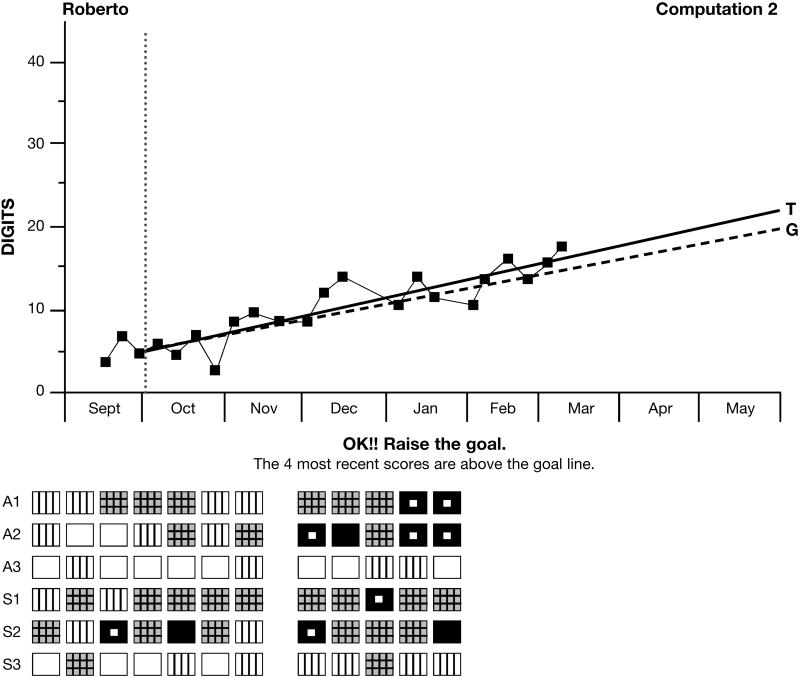
Mrs. Hayes drew these scores onto Roberto's graphs, one for computation and the other for concepts/applications, and then connected Roberto's baseline scores with the year-end goal to show the goal lines, or desired weekly rates of improvement (see Figure 1 for computation; see Figure 2 for concepts/applications). Ten weeks later, Mrs. Hayes then drew lines of best fit through Roberto's actual CBM scores and compared these trend lines to the goal lines. The CBM data showed that Pirate Math, with its focus on the counting-up strategy for number combinations and with its foundational focus and ongoing review of double-digit addition and subtraction, was producing strong growth for Roberto. As shown in Figure 1, Roberto's actual rate of improvement (solid diagonal line) was steeper than the goal line (broken diagonal line).

Figure 1.

Roberto's progress on CBM computation.

Note. Each dot represents Roberto's score on one weekly test. G is the year-end goal, and the diagonal line connecting Roberto's baseline score to G is the goal line, representing where Roberto needs to be any given week to realize the year-end goal. The solid line (T) below the graph shows Roberto's actual rate of progress since the goal was set. The grid below the Roberto's skills profile. Each box represents Roberto's mastery status on one skill for each half-month interval (time correponds to the time label on the graph). An empty box signifies that Roberto did not attempt that skill during that half-month interval; a striped box indicates that Roberto tried that skill during that half-month interval but his performance was substantially below mastery; a checkered box indicates that Roberto tried that skill during that half-month interval and his performance revealed partial mastery; a black box with a dot indicates that Roberto tried that skill during that half-month interval and his performance revealed probable mastery; a black box indicates that Roberto tried that skill during that half-month interval and his performance revealed mastery. So, for A1 (simple addition), Roberto began the year attempting the skill, but his performance did not indicate mastery; in the second half of October, Roberto's performance improved to suggest partial mastery, but after the Thanksgiving break, his performance deteriorated to beginning-of-the-year levels; after the winter break, Roberto's performance improved again to suggest partial mastery, and in the second half of February, Roberto's performance suggested partial mastery. The column of boxes for the second half of September indicates that fewer skills were mastered, compared to the column of boxes for the first half of March. This skills profile corresponds to the increasing graphed scores.

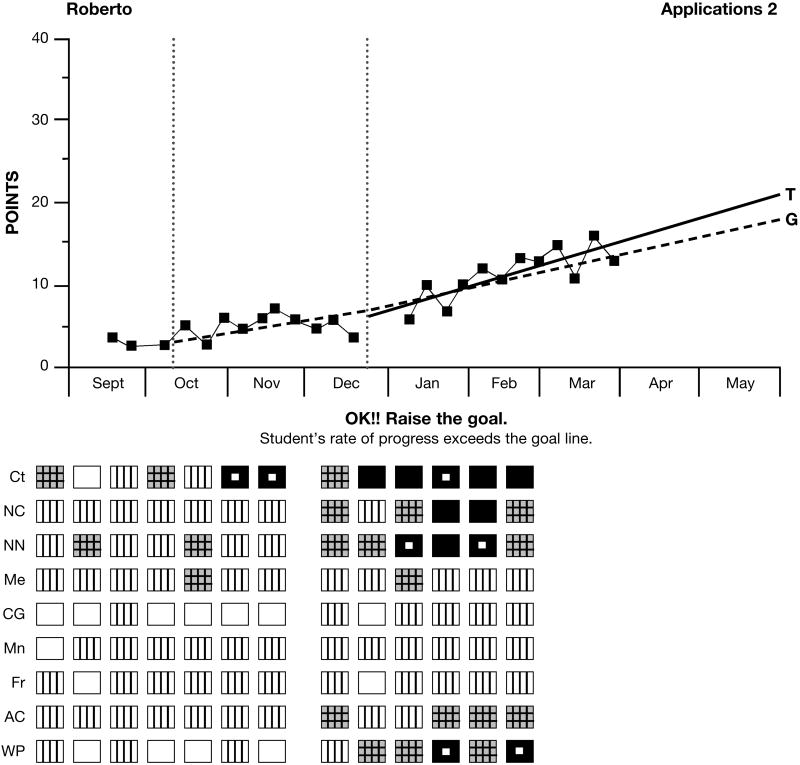
Figure 2.

Roberto's progress on CBM concepts and applications.

Note. This figure is read the same way as Figure 1. On concepts and applications, Roberto's trend line prior to the winter break was less steep than the goal line, at which time the teacher introduced a revision to the instructional program (signified by the second dotted line). With that revision to the instructional program, Roberto's rate of progress accelerated, signified by the trend line, which, by the end of March, is steeper than the goal line. This suggested that, at the end of March, the goal needs to be increased.

By contrast, Roberto was proving insufficiently unresponsive to Pirate Math's word-problem instruction. As shown in Figure 2, Roberto's actual rate of improvement was dramatically less steep than the goal line (broken diagonal line), indicating that he was growing slower than hoped and was unlikely to achieve his year-end goal. Therefore, Mrs. Hayes modified the Pirate Math standard protocol. She considered Roberto's performance during tutor sessions and reviewed Roberto's performance on the CBM concepts/applications story problems. She determined that Roberto was having difficulty differentiating problem types when irrelevant information was included in problems and when the missing information in story problems occurred anywhere but in the final position in the number sentence. Based on this analysis, Mrs. Hayes decided (a) to add instruction on mixed problem types, (b) to lengthen the problem-type sorting activity, and (c) to add instructional time on irrelevant information and deriving number sentences when the missing information is in the first or second slot of the equation. This revision in the intervention protocol is signified on Roberto's CBM concepts/applications graph with the dotted vertical line. As she implemented this revision in the intervention protocol, Mrs. Hayes continued to monitor Roberto's responsiveness using weekly CBM concepts/applications assessments. As indicated in Figure 2, Roberto's learning, as shown in the new trend line, improved and was now steeper than the goal line Mrs. Hayes had set for Roberto. It is in this formative, inductive, and recursive way that teachers use CBM to derive individual instructional programs that are effective for individual students and increase the probability of improved student outcomes.

Implications for Practice

In this article, we have argued for the importance of using seven principles in designing effective intensive interventions for students with mathematics disabilities: instructional explicitness, instructional design to minimize learning challenges, conceptual foundation, drill and practice, cumulative review, systematic motivation to promote self-regulation and encourage students to work hard, and ongoing progress monitoring to quantify response and formulate individually tailored programs as needed. We noted that two of these principles, instructional design to minimize learning challenges and attention to the conceptual foundation of the mathematics, are often overlooked. We also emphasized that the last principle, ongoing progress monitoring to quantify response and formulate individually tailored programs, may be the most essential principle of intensive intervention.

Issues and Directions for Future Research in the Primary and Later Grades

Although we focused on third grade here, specifically with respect to deficits with number combinations and story problems, similar instructional principles recur in other programs of research (e.g., Allsop, 1997; Jitendra, DiPipi, & Perron-Jones, 2002; Macinni, Mulcahy, & Wilson, 2007; Miller & Hudson, 2007; Montague, 2007) conducted at higher grades and on other aspects of the mathematics curriculum. However, additional work is required. Major aspects of the mathematics curriculum have received inadequate attention. For example, as school systems ratchet up high-school graduation requirements, interventions (see, e.g., Allsop, 1997) need to be expanded to ensure mastery of the algebra curriculum. In a related way, as the demands of a technology-rich environment continue to grow, additional attention is needed to address everyday mathematics problem solving, which requires flexible use of computational skills combined with knowledge about how to find relevant information within real-life situations.

Given the scope of intervention development still needed to ensure intensive intervention in mathematics, a second major issue that warrants the field's attention is professional development. The question is how to prepare new and practicing special educators to master and integrate the principles of effective practice so they (a) can design their own intensive interventions to address the skill deficits their students manifest and (b) have the knowledge to formatively develop effective program revisions when students do not respond as expected to intensive intervention protocols.

Unfortunately, special education training programs in universities and special education professional development opportunities within school districts focus disproportionately on reading intervention, with less attention to mathematics. Given the scope of the mathematics curriculum, along with many teachers' discomfort with their own mathematics skills, coursework and additional texts must be developed to assist university professors and school districts in preparing their trainees and teachers to understand and effectively employ principles of effective intensive mathematics intervention.

Finally, and in a related way, although a sense of urgency for improving student outcomes has been effected for reading, the same is not true for mathematics. The majority of students with learning disabilities suffer large deficits in both domains, but time is allocated disproportionately to reading intervention (Rasanen & Ahonen, 1995). If mathematics difficulties are to be addressed effectively, a similar sense of urgency needs to be mustered to (a) permit adequate opportunity for intensive intervention in mathematics and (b) open doors for researchers to develop and validate intensive tutoring protocols.