ACCURACY AND PRECISION OF A METHOD TO STUDY KINEMATICS OF THE TEMPOROMANDIBULAR JOINT: COMBINATION OF MOTION DATA AND CT IMAGING

Abstract

The purpose of the study was to test the precision and accuracy of a method used to track selected landmarks during motion of the temporomandibular joint (TMJ). A precision phantom device was constructed and relative motions between two rigid bodies on the phantom device were measured using optoelectronic (OE) and electromagnetic (EM) motion tracking devices. The motion recordings were also combined with a 3D CT image for each type of motion tracking system (EM+CT and OE+CT) to mimic methods used in previous studies. In the OE and EM data collections, specific landmarks on the rigid bodies were determined using digitization. In the EM+CT and OE+CT data sets, the landmark locations were obtained from the CT images. 3D linear distances and 3D curvilinear path distances were calculated for the points. The accuracy and precision for all 4 methods were evaluated (EM, OE, EM+CT and OE+CT). In addition, results were compared with and without the CT imaging (EM vs. EM+CT, OE vs. OE+CT). All systems overestimated the actual 3D curvilinear path lengths. All systems also underestimated the actual rotation values. The accuracy of all methods was within 0.5 mm for 3D curvilinear path calculations, 0.05 mm for 3D linear distance calculations, and 0.2° for rotation calculations. In addition, Bland-Altman plots for each configuration of the systems suggest that measurements obtained from either system are repeatable and comparable.

Introduction

Recently, methods combining three-dimensional (3D) imaging and motion tracking data (both optoelectric and electromagnetic) were introduced to study TMJ kinematics. Researchers have specifically examined the motion of the mandibular condyles and the front incisors as landmarks during jaw opening and closing. However, it is difficult to obtain the correct anatomical locations of the condylar points of interest using kinematic methods alone because of their location approximately 2 cm subcutaneously. Treating the mandibular bone and lower teeth as a rigid body, motion tracking sensors can be attached directly to the teeth to assess TMJ motion. Then, by combining 3D imaging with motion tracking data, it is possible to track any condylar point during jaw motion. (Baltali et al., 2007; Gallo, 2005) However, factors such as the data collection environment, setup of coordinate systems, and the combination of the motion data with 3D imaging affect the accuracy and precision of the resulting data. (Wagner et al., 2002)

The aim of this study was to use a calibration device to quantify the accuracy and precision of a clinical method used to study TMJ motion. Both optoelectric (OE) and electromagnetic (EM) kinematic tracking devices were used in the study. In the OE and EM data collections, specific landmarks were determined using digitization. In the EM+CT and OE+CT data sets, the landmark locations were obtained from the CT images.

Methods

Experimental Setup

A calibration device consisting of a series of precision slides controlled by micrometers (0.01 mm translation & 0.02 degree rotation accuracy) was constructed to produce relative translation (along 3 orthogonal axes) and rotation (about a single axis) between two rigid bodies (Figure 1). Two plastic blocks were rigidly secured to the calibration device using screws such that no motion occurred between the blocks and calibration device. Then, optoelectric (OE, Optotrak Certus far-focus optoelectronic tracking system, Northern Digital Inc., Waterloo, Canada, reported accuracy of 0.15mm in translation) or electromagnetic (EM, Fastrak electromagnetic tracking system, Polhemus, Inc., Colchester, VT, reported accuracy of 0.71mm) sensors were attached to the blocks with double-sided adhesive tape. The same data collection software (The MotionMonitor, Innovative Sports Training, Inc., Chicago, IL) was used to record the 3D movements of the block representing the mandible relative to the block representing the maxilla for both tracking systems.

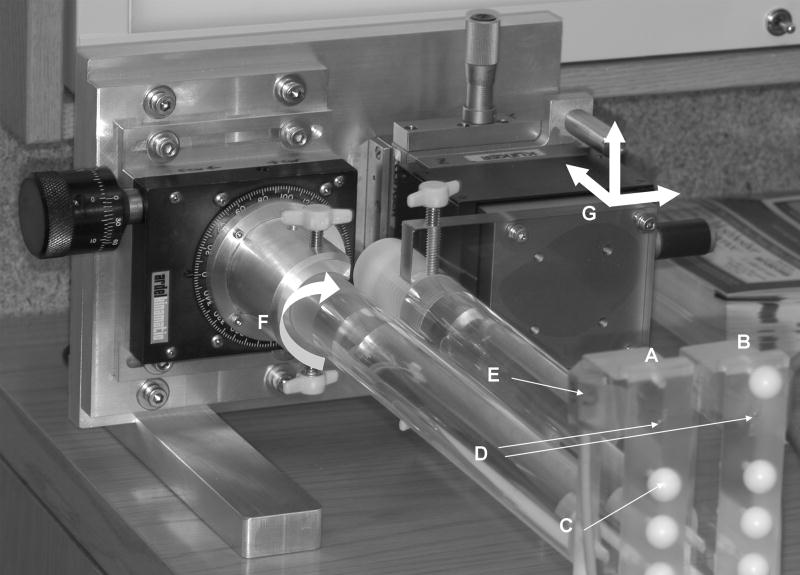
Figure 1.

Two plastic blocks (A, B) were constructed to represent the upper and lower jaw, and plastic spheres (C) 12 mm in diameter were attached to the blocks to represent the mandibular condyles. Three 0.8 mm diameter metal beads (D) were embedded in each block for identification and digitization of landmark points in three dimensions, during motion data collection and subsequent CT imaging. Plastic blocks were rigidly fixed to the calibration device and the sensors (E) were attached to the blocks via adhesive tape. The device provided for rotation about a single axis (F) and translation along three orthogonal axes (G).

Data Collection

Kinematic testing was performed in the same manner for each motion system and was identical to the method previously described. (Baltali et al., 2007) 11 trials of translation movement (1, 2, 5 and 10 mm along the x and y axes, and 1, 2, 5 mm along the z axis) and 4 trials of rotation movement (5, 10, 20 and 30 degrees) were collected on 2 separate days. These values are accepted as the gold standard for the study. In each movement, the block representing the mandible started and ended in the zero position and was moved manually at approximately 1 mm or degree/second. EM data was collected at 30 Hz and OE data was collected at 100 Hz. Translation and rotation data of the moving block were output in the defined local coordinate system.

To create the EM+CT and OE+CT datasets, a CT image of the calibration device with the Plexiglas blocks in the zero position was obtained with a 64-slice MDCT system (Sensation 64, Siemens, Forchheim, Germany). Scanning was done in a spiral mode using 1-second rotation times, with 10-0.6 collimation with z-flying focal spot, 0.8 pitch, 120 kV, and 425 effective mAs (mAs per pitch). One set of overlapped axial images (slice width, 1 mm; increment, 0.5 mm) were reconstructed with the sharp H70 kernel. A 3D model of the Plexiglas blocks were obtained from the CT images with a 0.5 mm cubic voxel. The accuracy of landmark selection is directly related to the voxel size in the CT image. In the present study, a cubic voxel size of 0.5 mm was used. In the worst-case scenario, error in detecting landmarks would be approximately 0.866 mm (the diagonal of a voxel).

Data Analysis

A fourth order zero-phase-shift Butterworth filter with a cut-off frequency of 1 Hz was used for both data sets (the EM sampled at 30 hz and the OE sampled at 100 hz). As such, the filter coefficients differed for the two data sets. CT images were reconstructed using Analyze software (BIR, Mayo Clinic, Rochester, MN). The three metal markers on the block representing the mandible and the volumetric centroid of the Plexiglas spheres representing the condyles were chosen as landmarks to be analyzed. The 3D locations of the metal makers and landmarks were recorded in the CT coordinate system.

Custom written MATLAB (Mathworks, Natick, MA) programs were used to calculate the corresponding position of the CT landmarks in the kinematic data coordinate system. A transformation matrix was generated (Challis, 1995) between the 3 mandibular metal markers identified in the CT coordinate system and the same metal markers previously identified in the kinematic coordinate system:

$${\overrightarrow{\mathit{p}}}_{\mathit{MM}}=\underset{\sim }{\mathit{R}}·{\overrightarrow{\mathit{p}}}_{\mathit{CT}}+\overrightarrow{\mathit{v}}$$

where p⃗_CT are the x, y, z coordinates of the landmarks in the CT coordinate system, p⃗_MM are the x, y z coordinates of the same landmarks in the kinematic coordinate system, and $\underset{\sim }{\mathit{R}}$ and v⃗ are the rotation matrix and translation vector, respectively, between the two coordinate systems. This transformation was applied to the landmark data to determine their 3D location in the kinematic coordinate system.

Opening portions of the movements were selected from the time series data for the trial (Figure 2). The motion of digitized points (EM and OE) and landmarks defined with CT reconstructions (EM+CT and OE+CT) were evaluated. The 3D linear distance (LD) was defined as the distance between the start and end position of the landmark. The 3D curvilinear path (CP) was defined as the length of the path traveled by a landmark between the start and end position. The total rigid body rotation was defined as the total rotation between the start and end position as determined using helical axes decomposition of the calculated transformation matrix. (Veldpaus et al., 1988)

Figure 2.

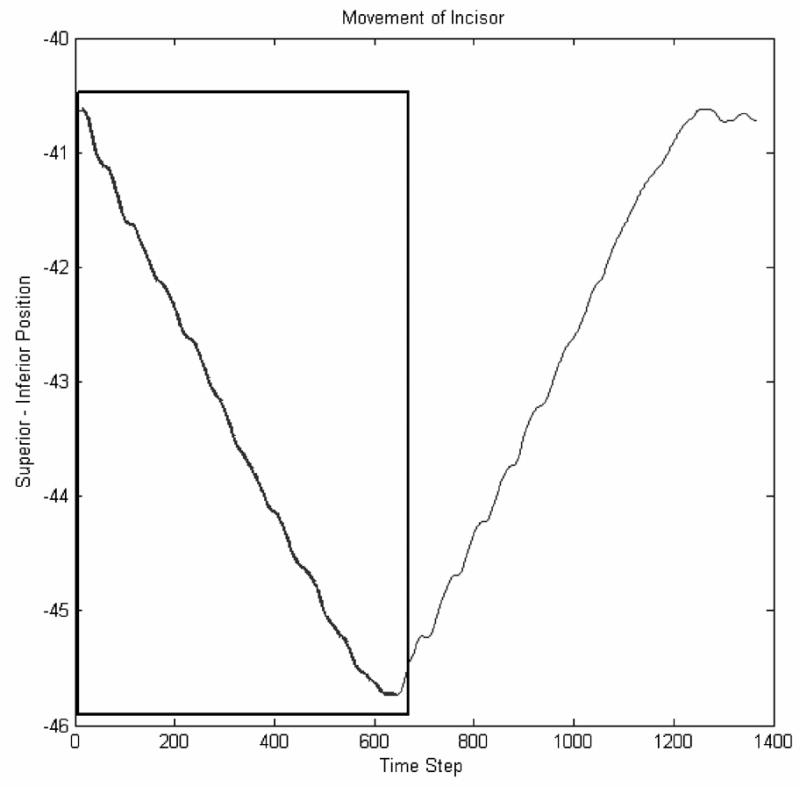
Motion representing the opening portions (zero to maximum) of each movement was selected form the data series.

The accuracy of the measurements (measured value vs. actual value) was evaluated for LD, CP and rotation values with two-tailed Student’s t-tests (p<0.05). The day-to-day precision of the EM, OE, EM+CT and OE+CT methods, as well as the comparison of methods (EM vs. EM+CT and OE vs. OE+CT) and systems (electromagnetic (EM and EM+CT) vs. optoelectric (OE and OE+CT) was assessed using Bland-Altman plots (Bland et al., 1986) for the variables of LD, CP and rotation.

Results

Accuracy

Mean differences in the LD and CP calculations from gold standard values were less than 0.05 mm and 0.40 mm, respectively. Both systems underestimated the rotational values, with OE being slightly less than EM. The rotational error was less than 0.20 degrees for both systems (Table 1).

Table 1.

Rotational and Translational Accuracy of Electromagnetic and Optoelectric Systems. Data are reported as (actual value − calculated value)

	System Alone		System + CT		Rotation
	LD	CP	LD	CP
Electromagnetic
Mean	0.046	0.390	0.045	0.4775	−0.196
S.D.	0.071	0.271	0.062	0.3151	0.227
Optoelectric
Mean	0.022	0.1159	0.033	0.350	−0.172
S.D.	0.021	0.0549	0.074	0.181	0.050

Precision

There were significant differences in precision between CP calculations. However, all measured differences were less than the measured accuracy of the systems. (Table 2).

Table 2.

Bland-Altman test values for precision measurements. All measured differences were less than the measured accuracy of the systems.

	Output Bland-Altman
	Mean	S.D.	95 % confidence		Mean−2S.D.	Mean+2S.D.
Day 1 vs Day 2
EM_LD	−0.009	0.065	−0.053	0.034	−0.139	0.121
EM CP	−0.158	0.192	−0.287	−0.029	−0.542	0.226
EMCT LD	0.008	0.071	−0.040	0.055	−0.135	0.150
EMCT CP	−0.133	0.170	−0.247	−0.018	−0.473	0.208
EM ROT	−0.031	0.018	−0.060	−0.002	−0.068	0.005
OE LD	0.003	0.026	−0.015	0.02	−0.049	0.054
OE CP	0.003	0.037	−0.022	0.028	−0.072	0.078
OECT LD	−0.032	0.102	−0.100	0.037	−0.236	0.172
OECT CP	−0.008	0.101	−0.076	0.059	−0.210	0.193
OE ROT	−0.039	0.063	−0.139	0.061	−0.164	0.087
System vs System+CT
EM LD	0.001	0.023	−0.009	0.011	−0.046	0.048
EM CP	−0.088	0.067	−0.117	−0.058	−0.222	0.047
OE LD	−0.011	0.078	−0.045	0.024	−0.167	0.145
OE CP	−0.234	0.134	−0.294	−0.175	−0.503	0.034
EM vs OE
LD	0.024	0.059	−0.002	0.050	−0.095	0.143
CP	0.274	0.232	0.171	0.377	−0.190	0.738
CT LD	0.012	0.102	−0.033	0.057	−0.192	0.216
CT CP	0.127	0.188	0.044	0.211	−0.249	0.504
Rotation	−0.066	0.057	−0.114	−0.018	−0.181	0.048

Discussion

In this study we used a calibration device to evaluate and compare the accuracy and precision of a method to measure the kinematics of the TMJ when using two different motion tracking devices alone and when coupled with 3D CT images.

Accuracy of the systems (EM, OE, OE+CT and EM+CT) were calculated as the average difference between gold standard and measured values. All methods had a tendency to overestimate the CP values. The CP values were expected to be equal to the LD values since all translation tests were recorded along a single axis. Although noise of the overall data acquisition system was reduced with appropriate data filtering techniques, significant differences between the actual motions and calculated motions were still present. The nature of the CP calculations (point-by-point distance summation) as well as noise of the acquisition system, which may not be completely removed by filtering, may have resulted in the elevated CP values. Thus the mean calculated accuracy of the systems was less then 0.05 mm for LD values and 0.40 mm for CP values.

Precision of the systems were measured in a systematic pattern. Day to day precision was tested for each method. Then each method was tested with and without adding the 3D CT image. Finally, the electromagnetic and optoelectric systems were compared with each other. Calculated differences for all precision measurements were less than the calculated accuracy of the systems.

Tracking the mandibular condyle using motion recordings may provide an optimal method for kinematic analysis of the TMJ. However, locating the mandibular condyle accurately for kinematic studies of the TMJ motion is still a challenge. When studying patients with osteoarthritic degenerative changes that dramatically affect the condylar shape, utilizing 3D imaging will enable the researchers to choose several landmarks. However, combining 3D images with kinematic data recordings may affect the overall accuracy and precision of the data collection method. In this study we found no significant difference in the accuracy and precision of the two different motion tracking devices alone and when coupled with 3D CT images.

In conclusion, one can avoid use of a CT image in the clinic when landmarks can be directly located. In addition, in cases where it is hard to directly digitze the landmark of interest, one can combine a 3D CT with the motion tracking data without losing accuracy. In addition our findings suggest that researchers and clinicians may select either an optoelectric or electromagnetic tracking device without sacrificing precision or accuracy.