Computing the stability diagram of the Trp-cage miniprotein

Abstract

We report molecular dynamics simulations of the equilibrium folding/unfolding thermodynamics of an all-atom model of the Trp-cage miniprotein in explicit solvent. Simulations are used to sample the folding/unfolding free energy difference and its derivatives along 2 isochores. We model the ΔG_u(P,T) landscape using the simulation data and propose a stablility diagram model for Trp-cage. We find the proposed diagram to exhibit features similar to globular proteins with increasing hydrostatic pressure destabilizing the native fold. The observed energy differences ΔE_u are roughly linearly temperature-dependent and approach ΔE_u = 0 with decreasing temperature, suggesting that the system approached the region of cold denaturation. In the low-temperature denatured state, the native helical secondary structure elements are largely preserved, whereas the protein conformation changes to an “open-clamp” configuration. A tighter packing of water around nonpolar sites, accompanied by an increasing solvent-accessible surface area of the unfolded ensemble, seems to stabilize the unfolded state at elevated pressures.

The stability of natively folded proteins in solution is determined by the competition of many effects that reach a balance near physiological conditions. As a consequence, the stability of a protein can be affected in many different ways (1–5). High-temperature denaturation is mostly accompanied by a dramatic loss of protein secondary structure (6). However, elevated pressures (2, 3), changing pH (2), and cosolvents such as salts (7) and osmolytes (4) also affect the stability of the native state, often destabilizing in character but under certain conditions also significantly stabilizing (4). In addition, many globular proteins are also destabilized at low (subzero) temperatures, a process known as “cold denaturation” (8). Cold denaturation is experimentally accomplished with the help of elevated pressures (2), leading to a characteristic tongue-shaped P,T-stability diagram, found for many globular proteins (9–15). Hydrophobic forces play a key role in the protein folding process (16–18), but it is the balance of hydrophilic and hydrophobic forces that determines the conformational equilibrium. The notion that proteins under native conditions are only “marginally stable” (7) seems to be an important requirement for their ability to explore different conformational substates (19) and hence for protein function. The application of high hydrostatic pressure (20) has been shown to be able shift the equilibrium of conformational states (21, 22), promoting denaturation (23) but also altering the native state (24) and modifying protein–protein interaction (20). Pressure effects on protein structure appear to be determined mostly by changing the balance between hydrophilic and hydrophobic interactions (25–27).

Model peptides and proteins have long been sought as templates for understanding protein structure and function. The Trp-cage miniprotein (28) is a relatively well-characterized system, designed to shed light on protein folding pathways and understanding stability of globular proteins. Trp-cage is a 20-residue protein (28), exhibiting a cooperatively folded tertiary structure (29). Its structure has been determined by NMR [Protein Data Bank (PDB) ID code 1L2Y], and its melting behavior has been characterized using CD (28, 30, 31), changes in chemical shifts, fluorescence quenching (30), fluorescence correlation (32), and UV-resonance Raman spectroscopy (33). The small size and the rapid kinetics of Trp-cage have made it an attractive target for computer simulation studies using implicit (34–40) and explicit solvent models (41–44). The specific virtue of an explicit water solvent is that it can model the different contributions stabilizing both the native and the unfolded state, such as hydrophobic and hydrophilic hydration effects, better than implicit solvent models. Of particular importance is the correct balance of enthalpic and entropic solvation effects and their temperature and pressure dependence. However, due to the large computational burden, the folding/unfolding equilibrium of even small biomolecules still represents a major computational challenge. Here, we present a previously undescribed unbiased computation of the P,T-stability diagram of an atomic detail model of a computer simulated protein starting from an unfolded initial structure. The P,T-stability diagram has been calculated for small peptides (45, 46) and an RNA oligomer (47). Our simulations demonstrate that the Trp-cage, although small in size, exhibits essential thermodynamic features also found in globular proteins (5, 12, 31, 48). Replica-exchange molecular dynamics simulations are performed on the Trp-cage protein. To study the effect of volume changes on protein hydration, we study the folding/unfolding thermodynamics on 2 different isochores.

Results and Discussion

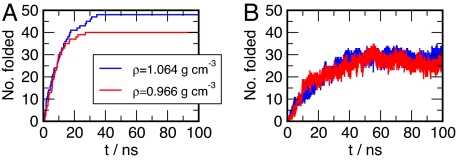
Replica exchange simulations at 2 different densities are used to compute the folding/unfolding equilibrium thermodynamics of the Trp-cage. Time series of the number of replicas that have reached the folded state at least once during the simulation [number of folded replicas (NFR)] and the total number of folded replicas (rmsd ≤0.22 nm) are shown in Fig. 1. A single exponential fit of the NFR gives folding times of 8.5 and 10.5 ns in the replica ensemble for the 0.966 and 1.064 gcm⁻³ isochores, respectively. The number of folded states for both isochores have reached steady states after ≈40 ns. Consequently, the final 60 ns of the simulation runs are used for analysis.

Fig. 1.

Convergence of the REMD simulations. (A) Time history of the number of replicas that have folded (rmsd ≤ 0.22 nm) at least once in the simulation (NFR). (B) Number of replicas sampling the folded state as a function of time. The total number of replicas sampling the 0.966 gcm⁻³ and 1.064 gcm⁻³ isochores is 40 and 48, respectively. After 40 ns all replicas have reached folded state at least once.

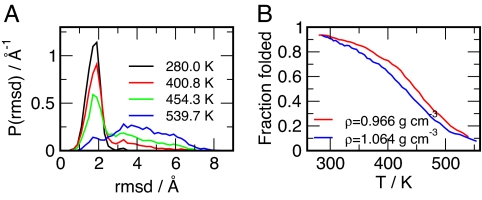
We use the rmsd from the NMR structure (backbone atoms of frame 1 of PDB ID code 1L2Y) as a measure to distinguish between folded and unfolded configurations. The rmsd distributions shown in Fig. 2A exhibit a narrow peak, representing the folded configurations, centered at ≈0.18 nm. With increasing temperatures, the narrow peak diminishes at the expense of the appearance of a broad distribution of rmsds between 0.3 and 0.8 nm, representing unfolded configurations. The system apparently shows a 2-state folding behavior, as proposed for Trp-cage from fluorescence quenching data (30), but recently has been taken into question according to an extensive fluorescence correlation spectroscopy study (32).

Fig. 2.

Folding/unfolding equilibrium of trp-cage. (A) Backbone atom rmsd distributions obtained from the 0.966 gcm−³-isochore for 4 selected temperatures. (B) Fraction of folded configurations (rmsd ≤ 0.22 nm) as a function of temperature for both studied isochores.

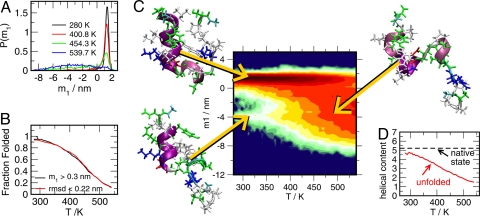
The fraction of folded states with rmsd ≤0.22 nm as a function of temperature has a quasisigmoidal shape, indicating transition temperatures of 445 and 425 K for 0.966 and 1.064 gcm⁻³, respectively. Alternatively, we have also determined the equilibrium of folded and unfolded states using principal component analysis (PCA) based the entire replica exchange molecular dynamics (REMD) ensemble of configurations. The largest eigenmode m₁ describes a clamp-like opening of the cage, as suggested by the representatative low-temperature configurations shown in Fig. 3. A quantitative analysis based on the projection of the configurational density on the largest PCA-eigenmode m₁ is given in Fig. 3 A–C. Similar to the rmsd distributions given in Fig. 2A, the projection of density of states on m₁ is represented by a large narrow peak at low temperatures, which is decreasing at the expense of a broad distribution at higher temperatures. Employing a threshold of m₁>0.3 nm to separate the “folded” basin from the “unfolded” configurations, we obtain a similar temperature dependence of the fraction of folded stated as due to the rmsd distributions, as shown in Fig. 3B.

Fig. 3.

Structural aspects related to the folding/unfolding of Trp-cage. (A–C) Principal component analysis (70) of the entire REMD configurational ensemble obtained for the 0.966 gcm⁻³ isochore: (A) Probability distributions of the largest eigenmode m₁ for 4 selected temperatures. (B) Comparison of the temperature dependence of fraction of folded states as obtained from the rmsd and m₁ distributions. (C) Free energy landscape of Trp-cage (in units of kT) projected on the m₁ eigenmode. Representative configurations of Trp-cage for selected states are indicated. (D) Helical content (defined as in ref. 71) of unfolded Trp-cage as a function of temperature. The helical content of the native state (PDB ID code 1L2Y) is given as a reference.

We would like to emphasize that our simulations differ in certain aspects from the simulations of Trp-cage reported recently by Juraszek and Bolhuis (43). Their extensive simulation study using the OPLS-AA forcefield model with explicit simple point charge model (SPC) water suggests the existence of 2 different folding pathways: One in which the helix forms first (i), and 1 in which a contact between Trp-6 and a polyproline forms before the helix (ii). The latter pathway is found to be ≈4 times more likely in their study. It is not unlikely, however, that the formation of a Trp-6-polyproline contact could represent a kinetic bottleneck, hampering the formation of the helices and therefore reaching the fully folded state. In our simulations, pathway i is observed almost exclusively, with the cage opening and the helix melting having roughly the same transition temperature (44). The restriction to path i apparently allows the molecule to reach the folded state (and the folding/unfolding equilibrium) more quickly, also suggesting that the ff94-model has a less rugged, more funnel-like free energy landscape, which is enabling our REMD simulations to equilibrate within a 40 ns time-window.

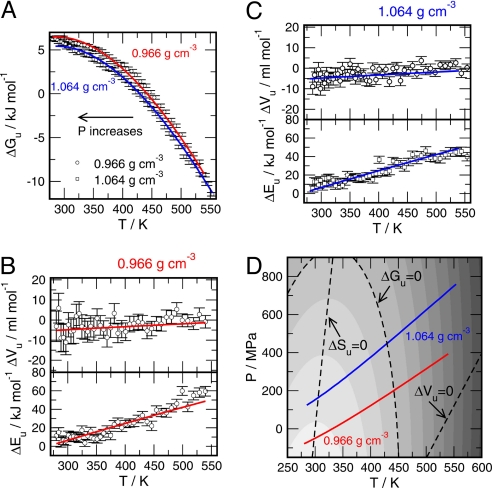
Because we can distinguish folded and unfolded ensembles, we can calculate the free energy of unfolding according to ΔG_u(T,P) = G_unfolded − G_folded = −RT ln [(1−x_folded)/x_folded], where x_folded is the fraction of folded states obtained from the REMD at each T, and P is the average pressure, <P>_V. In a similar fashion we can determine the energy and volume changes associated with unfolding according to ΔE_u = E_unfolded − E_folded and ΔV_u = V_unfolded − V_folded. All 3 properties (ΔG_u, ΔE_u, and ΔV_u) were sampled for the states along the 2 isochores and are shown in Fig. 4 A–C. ΔE_u is directly available via the average potential energies obtained for the folded and unfolded ensembles sampled by the REMD simulation. ΔV_u is not directly available due to the application of constant volume conditions during the REMD simulation. However, the free energy change associated with volume changes becomes evident by observing slightly differing average pressures for the folded and unfolded ensembles following VΔP≈ PΔV. To determine the associated volume changes directly and not rely on the observed pressure differences only, we performed a posteriori additional short (80 ps) constant-pressure simulation runs at the average pressures obtained for each of the statepoints. The simulations were performed under isobar isothermal conditions using a Nosé–Hoover (49, 50) thermostat and a Rahman–Parrinello barostat (51, 52) with coupling times of τ_T = 0.5 ps and τ_P = 1.0 ps, using a molecular dynamics timestep of Δt = 2.0 fs. Exactly 200 NPT simulations were conducted for each statepoint: 100 simulations representing the folded and 100, the unfolded ensemble. The starting configurations for these simulations (in total: 17,600 simulation runs) were sampled randomly from the set of REMD configurations obtained from the final 60 ns. The length of the simulations was chosen such that a volume relaxation was feasible, whereas the configuration of the protein stayed in sufficiently close proximity to the initial configuration. The calculated ΔE_u obtained from the NPT simulations are within the errorbars of ΔE_u obtained from REMD, and the observed volume changes were found to be consistent with the calculated pressure difference for the subensembles. The data shown Fig. 4 depict the data according the NPT simulations.

Fig. 4.

Averages of the difference upon unfolding of the (A) free energy, (B and C) total energy, and specific volume, calculated for the 2 indicated isochores. (D) Free energy surface ΔG_u(P,T) obtained by fitting to a Hawley-type model the free energy and its derivatives calculated for the 2 isochores. Different grayscale colors indicate changes of ΔG_u of 2 kJ mol⁻¹.

Having traced the free energy and 2 of its derivatives along the 2 isochores, both of them effectively crossing a large portion of the experimentally accessible P- and T-ranges, we are able to construct the stability diagram of the Trp-cage in terms of ΔG_u as a function of temperature and pressure by fitting the calculated data to a Hawley-type (9, 12, 13) free energy surface ΔG_u (P,T) = Δβ/2 (P−P₀)² + Δα(P−P₀)(T−T₀) − ΔC_P [T(ln T/T₀ −1) + T₀] + ΔV₀ (P−P₀) − ΔS₀ (T−T₀) + ΔG₀. Here, Δβ, Δα, and ΔC_P are the changes in compressibility, expansivity, and heat capacity, and ΔV₀, ΔS₀, and ΔG₀ are the unfolding volume, entropy, and free energy at the reference state (T₀ = 331 K, P₀ = 0 MPa), correspondingly. All coefficients in this expansion are assumed to be constant with temperature and pressure. The fitted values are given in Table 1. First, we would like to emphasize that the independently calculated isochores shown in Fig. 4A directly demonstrate the destabilizing effect of an increasing hydrostatic pressure. In addition, the fitted free energy landscape ΔG_u(P,T), shown in Fig. 4D, indicates that simulated Trp-cage is very likely to exhibit a ellipse shaped stability diagram, similar to globular proteins. The condition for an elliptical phase diagram, Δα² > ΔC_P Δβ/T₀, is satisfied here with a positive ΔC_p and a negative Δβ (12). The Trp-cage is found to be quite stable with respect to changes in temperature and pressure, as the high unfolding temperatures (≈450 K at normal pressure) and pressures (≈>800 MPa at ambient temperatures) suggest. Moreover, the transition ranges are found to be very broad, which is apparently due to the small size of the molecule and associated with small energy and volume changes. Fig. 4A strongly suggests a parabolic shape of the unfolding free energy vs. temperature curve for Trp-cage, which is further supported by temperature dependence of the corresponding ΔE_u-isochores, both increasing almost linearly and qualitatively resembling very much the curves reported by Makhatadze and Privalov for myoglobin (5). In addition, the calculated heat capacity of 0.2 ± 0.05 kJ K⁻¹mol⁻¹ is found to be close to the experimental value of 0.3± 0.1 kJ K⁻¹·mol⁻¹ reported recently by Streicher and Makhatadze (31). From the Hawley model we can extrapolate the free energy to 25°C and 1 atm (0.1 MPa) and get ΔG_u = 6.2 ± 0.5 kJ mol⁻¹, ΔH_u = 36.0 ± 5 kJ mol⁻¹ (at T_m = 448 K). These values can be compared with the measured ΔG_u = 3.2 ± 5 kJ mol⁻¹, and ΔH_u = 56.0 ± 2 kJ mol⁻¹ (at T_m = 317 K).

Table 1.

Thermodynamics parameters describing the Hawley-type (9, 12) free energy surface ΔG_u(P,T) for simulated Trp-cage shown in Fig. 4

ΔG0	5.9 ± 0.5 kJ mol−1
ΔV0	−4.3 ± 1.0 ml·mol−1
ΔS0	1.9 ± 0.5 × 10−2 kJ mol−1·K−1
ΔCp	2.0 ± 0.5 × 10−1 kJ mol−1 K−1
Δα	2.2 ± 2.0 × 10−2 ml· mol−1 K−1
Δβ	−4.0 ± 6.0 × 10−6 kJ mol−1 MPa−2

The chosen reference state is at T⁰ = 331 K, and P⁰ = 0 MPa.

The following aspects of the calculated diagram seem to be noteworthy. First, the observed variation of the unfolding energy ΔE_u over the entire temperature interval is much larger (a factor of ≈4) than the variation of the free energy ΔG_u, leading to a similar kind of enthalpy/entropy compensation behavior as observed in globular proteins (12). In addition, for the lowest temperatures, ΔE_u approaches 0, indicating that at ambient temperature conditions the Trp-cage protein can unfold with only little expense of energy, which is another significant thermodynamic signature of globular proteins in the native state. Moreover, the almost constant heat capacity, as indicated by the linear T-behavior of ΔE_u, and ΔE_u≈ 0 at low T, strongly suggests that the lowest temperatures of the 2 isochores are in close proximity to the ΔS_u = 0-line (also drawn in Fig. 4D for the fitted data), where the Trp-cage enters the cold denaturation regime (8). As suggested by Fig. 3D, the observed small energy differences ΔE_u at low temperatures go along with the preservation of the secondary structure elements of the native fold. With decreasing temperatures, the helical content of the unfolded Trp-cage approaches the value for native state.

The details of the P-T stability diagram presented here depend on the chosen forcefield. The ff94 force field is known to exaggerate α- helix stability. The thermodynamic stability diagram for Trp-cage has been calculated only for the ff94 forcefield (44) and showed that the fraction of the states occupying the folded state is correct for low T but failed to describe the stability for higher temperatures. The high propensity to form α-helices may be partly resposible for the enhanced stability of the protein at high temperature. Our calculations show the same effect and we expected that the stability with pressure will also be exaggerated and that the Trp-cage will unfold (at low temperatures) at pressures below the 800 MPa predicted here. However, the general features of the stability diagram (like cold denaturation) are similar to the features found for globular proteins. Further calculations of the P-T stability diagram for other force fields and water models may be needed to better understand the forcefield dependence of the folding free energy, energy and volume changes.

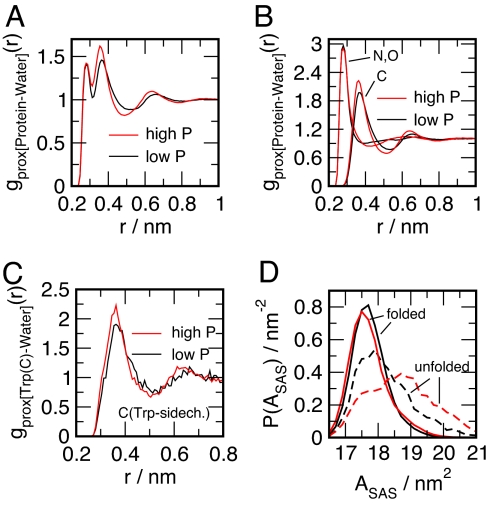
To elucidate the effect of pressure on the stability of the native fold, we inspect the water density in close proximity to the protein. We use a procedure for calculating protein-water “proximal pair correlation functions” g_prox(r), similar to the 1 used by Ashbaugh and Paulaitis (53, 54), suggested earlier by others (55, 56). As reference sites we use heavy atoms of the protein (C, N, O) divided in “polar” (O, N) and “nonpolar” (C) groups. The normalization volume s^α(r)dr is defined by volume elements with a shortest distance to any atom belonging to 1 of those groups of atoms, taken out of the set of atoms containing all protein heavy atoms. Additivity of the partial surface area s^α(r) leads to the relation [s^α(r)+s^β(r)] g_total^prox(r) = s^α(r) g_α^prox(r)+s^β(r)g_β^prox(r), where s^α represents the surface area of a subgroup of heavy atoms as a function of distance to the protein r. Fig. 5A shows the proximal radial distribution function of all heavy atoms of the entire protein at low and high pressures. The given g_prox(r) exhibit a typical 2-peak feature, which has been reported to be according to the hydration of polar and nonpolar atoms (56, 57), which is also clearly demonstrated for the Trp-cage in Fig. 5A. Note that the proportion between the 2 peaks is markedly different from for proteins reported hitherto (56, 57), with the nonpolar peak being significantly more dominant in the Trp-cage case. This suggests that the surface of larger size proteins is on average significantly more polar, which, however, might be also a consequence of the small size of the molecule. When comparing the low and high density isochores, it is observed that the water packing (density) around the nonpolar sites changes significantly more than the packing around the polar sites, as revealed by the increase of the second peak, and by the individual component proximal radial distribution functions shown in Fig. 5 A and B. A similar trend is observed for the packing of water around the C atoms of the Trp-sidechain in the unfolded state given in Fig. 5C, where the Trp-sidechain has been previously shown to be significantly exposed to the water solvent (44). A tighter packing of water around nonpolar, or in particular hydrophobic atoms, might explain the increasing surface area observed for the unfolded configurations at elevated pressures shown in Fig. 5D. A tighter water packing efficiency around unpolar atoms might hence also be responsible for shifting the configurational equilibrium toward the unfolded state. Our results are in agreement with the description of hydrophobic hydration as “soft” and polar hydration as “hard” in response to high pressures (58).

Fig. 5.

Water packing around Trp-cage. (A–C) Proximal radial distribution functions g_prox(r) between water's center of mass and the heavy atoms of the Trp-cage molecule. All data shown refers to “low temperature configurations” with T<320 K (A) g_prox(r) including both, “polar” (O and N) and nonpolar “C” atoms. (B) separate g_prox(r) for “polar” (O and N) and nonpolar “C” atoms. (C) g_prox(r) for nonpolar “C” atoms of the Trp-sidechain, calculated for the “unfolded” configurations. (D) Solvent accessible surface area distributions (72) calculated for folded and unfolded configurations of Trp-cage for T<320 K.

In addition to the observed differences in water packing around polar and nonpolar atoms, there might be additional factors affecting the stability of the folded state. The formation of an Asp-9/Arg-16 salt-bridge has been proposed to significantly stabilize the folded state (28, 41). Indeed, the folded conformation of ff94-model Trp-cage has been shown to largely exhibit an Asp-9/Arg-16 contact (44). Comparing low- and high- pressure configurations, we observe that the tendency to form a Asp-9/Arg-16 contact in the folded ensemble (averaging over states with T ≤ 320 K) drops from 86% to 73% with increasing pressure, possibly destabilizing the folded state. The enhanced stability of open salt bridge conformations is very likely related to the increasing ionic solvation strength of water depending on the almost linearly increasing dielectric constant due to an increasing dipole density (59). However, on a less coarse grained level, the importance of a changing local hydration of side chains and backbone cannot be ruled out, and should be further investigated.

Conclusion

We have used the REMD enhanced sampling approach to study the stability diagram of the Trp-cage miniprotein by calculating 2 isochores, scanning effectively a large portion of the experimentally accessible P,T-range.

With the help of an approximate description of the free energy of unfolding ΔG_u(P,T) in terms of the Hawley theory, employing sampled free energy, energy and volume differences, we are able to generate a stability diagram for Trp-cage. With increasing pressure, as with increasing temperature the native state is found to be destabilized, leading to a typical ellipsoidal (tongue-shaped) protein stability diagram, as found for many globular proteins. The energy difference between folded and unfolded conformations is found to approach zero energy difference at the lowest temperatures, which is accompanied by a conservation of helical secondary structure in the unfolded ensemble. With increasing pressure we observe a tighter packing of water around nonpolar atoms, accompanied by an increase of the solvent accessible surface area of the unfolded ensemble, and destabilized Asp-9/Arg-16 salt-bridge in the folded ensemble.

We would like to emphasize that with a melting temperature being approximately 140 K too high on a quantitative level, the presented computer models are still far from being completely satisfactory. In addition, the used ff94-forcefield has the known tendency to overemphasize the stability of helices, which is in our case apparently leading to an overall dominance of a folding pathway with an initial α-helical segment being formed first. However, this results in a less rugged free energy surface compared with that of the OPLS-AA model, finally allowing our REMD simulations to converge in the presented 100 ns time window.

We have demonstrated that, with present existing computer simulation techniques, it is possible to obtain reasonable rigorous thermodynamic data for the temperature- and pressure-induced folding/unfolding of proteins based on atomic detail models. Together with the constantly increasing computing power, experimental P,T data might thus be used to significantly improve current forcefield models in the future.

Simulation Methods.

We use REMD simulations (60) to study the unbiased equilibrium folding of the Trp-cage miniprotein, using the Amber (ff94) (61) forcefield and TIP3P water (62). We perform calculations of 2 isochores at densities of 0.966 gcm−³ and 1.064 gcm⁻³, effectively scanning the (P,T)-plane in a range between −100 and 800 MPa and 280 K and 280 K 550 K using 40 and 48 replicas. The densities were chosen to obtain average pressures of 0.1 MPa (1 bar) and 200 MPa at 330 K for the low- and high-density simulations, respectively. Simulations extending over 100 ns per replica provide a total of 8.8 μs worth of trajectory data. The entire simulation of the 2 isochores represents ≈10⁵ CPU hours (2.2 GHz AMD Opteron) on the Linux clusters at Rensselaer Polytechnic Institute and Technische Universität Dortmund.

The Trp-cage sequence (Ac-NLYIQWLKDGGPSSGRPPPS-NME) is generated in an initially all-PPII conformation by the LEAP program distributed with AMBER 6.0. The N and C termini were capped with methyl groups, and the constructed model peptide consists of 313 atoms. The LEAP-generated structure is slightly compacted during a short 25-ps simulation in the gas phase at 300 K. This structure is solvated in a cubic box 2,637 by TIP3P (62) water molecules, and the system is equilibrated during a 100-ps constant pressure simulation at 330 K and 1 bar. The final structure obtained from this simulation is placed in cubic boxes of lengths 4.40562 nm and 4.20562 nm, and is used as a starting configuration for the constant volume REMD simulations for the 0.966, and 1.064 gcm⁻³ isochores. The peptide is found to be completely unfolded with a (C, N, O) backbone rmsd of from the first NMR structure of 6.0 Å. Moreover it lacks any regular secondary structure elements; in particular, it has none of the helical structure elements that are present in the native state. REMD (60) has been used to study the thermodynamics of the Trp-cage protein starting from this initial structure. REMD is an enhanced sampling technique based on the parallel tempering Monte Carlo method (63–65), where multiple copies (or replicas) of identical systems are simulated in parallel at different temperatures. Periodically, state-exchange moves are attempted, where 2 neighboring replicas exchange their thermodynamic state (their temperature). The acceptance rule for each state-exchange moves between 2 neighboring states i and j is chosen to be

where β = 1/k_BT and U(r⃗_i^N) represents the configurational energy of the system in state i. The state-exchange acceptance probability P_acc has been shown to obey the detailed balance condition for an extended ensemble of canonical states (65).

Our simulations employ 40 and 48 replicas for distribution over a temperature range from 280.0 K to 539.7 K. The temperature spacing between each of the replicas was chosen such that the energy distributions overlap sufficiently, and state exchange attempts are (on average) accepted with a 20% probability. To initially set up the temperature spacings, energy distributions were obtained from a preceding series of noncoupled short (0.5 ns) constant volume MD simulations at similar density. Simulations are run at ρ = 0.966 gcm⁻³: 280.0, 284.1, 288.2, 292.4, 296.7, 301.1, 305.6, 310.2, 314.9, 319.7, 324.6, 329.6, 334.7, 340.0, 345.4, 351.0, 356.6, 362.5, 368.4, 374.6, 380.9, 387.3, 394.0, 400.8, 407.8, 415.1, 422.5, 430.1, 438.0, 446.0, 454.3, 462.8, 471.6, 480.6, 489.8, 499.3, 509.0, 519.0, 529.2, and 539.7 K, and at ρ = 1.064 gcm⁻³: 285.0, 288.3, 291.7, 295.1, 298.5, 302.1, 305.7, 309.3, 313.0, 316.8, 320.7, 324.6, 328.7, 332.8, 337.0, 341.2, 345.6, 350.1, 354.6, 359.3, 364.1, 368.9, 373.9, 379.1, 384.3, 389.7, 395.2, 400.8, 406.6, 412.6, 418.7, 425.0, 431.4, 438.0, 444.8, 451.8, 459.0, 466.5, 474.1, 481.9, 490.0, 498.3, 506.9, 515.8, 524.9, 534.3, 544.0, and 553.9 K.

State exchange attempts were undertaken with a probability of 0.05, leading to an average time of ≈1.6 ps for each replica between 2 state exchanges. The time step used in the MD steps is 2 fs, and a Nosé–Hoover (49, 50) thermostat is used with a time coupling of τ_T = 0.5 ps. Solvent constraints were solved using the SETTLE procedure (66), whereas the SHAKE method was used to constrain the solute bond lengths (67). The simulations were carried out with the GROMACS (68) simulation program, modified by us to allow for state-swapping moves. The electrostatic interactions were treated by smooth-particle mesh Ewald summation (69) using a 36 × 36 × 36 grid with fourth-order charge interpolation and a real-space cutoff of 0.9 nm. The Ewald convergence factor α was set to 3.38 nm⁻¹ (corresponding to a relative accuracy of the Ewald sum of 10⁻⁵). Appropriate Lennard–Jones long-range correction for energy and pressure were taken into account.