Skip to main content
PMC home page
Diabetes Care logoLink to Diabetes Care
. 2008 Dec;31(12):2265–2267. doi: 10.2337/dc08-0958

Is the New Mayo Clinic Quadratic Equation Useful for the Estimation of Glomerular Filtration Rate in Type 2 Diabetic Patients?

Néstor Fontseré 1, Jordi Bonal 2, Isabel Salinas 3, Manel Ramírez de Arellano 1, Jose Rios 4, Ferran Torres 4, Anna Sanmartí 3, Ramón Romero 2
PMCID: PMC2584175  PMID: 18835955

Abstract

OBJECTIVE—To test the Mayo Clinic Quadratic (MCQ) equation against isotopic glomerular filtration rate, compared with the Modification of Diet in Renal Disease (MDRD) and the Cockcroft-Gault formulas, in type 2 diabetes.

RESEARCH DESIGN AND METHODS—Based on values obtained with iothalamate, 118 type 2 diabetic patients were divided into three groups according to renal function: hyperfiltration (26), normal function (56), or chronic kidney disease (CKD) stages 3–4 (36). ANOVA, the Bland-Altman procedure, and Lins coefficient (Rc) were performed to study accuracy.

RESULTS—In the hyperfiltration and normal function groups, all prediction equations significantly underestimated the value obtained with isotopic glomerular filtration rate (P < 0.05). In the CKD group, all equations also presented significant differences with the isotopic method. However, MDRD had a bias of −5.3 (Rc 0.452), Cockcroft-Gault formula −0.2 (Rc 0.471), and the MCQ −4.5 (Rc 0.526).

CONCLUSIONS—The MCQ and prediction equations proved inaccurate (excessive underestimation) in type 2 diabetic patients with hyperfiltration or normal renal function. With regard to CKD, the results obtained provided no evidence of superiority of the MCQ over the MDRD or the Cockcroft-Gault formula.

According to current epidemiologic data, type 2 diabetes is considered one of the most frequent causes of end-stage chronic renal disease and inclusion in renal substitution programs (1,2). In a previous study, our group evaluated the accuracy of different prediction equations for the ambulatory follow-up of a cohort of type 2 diabetic patients (3). From the results obtained, it can be concluded that the application of these equations is inadequate in situations of normal renal function and hyperfiltration. Recently, the Mayo Clinic group has developed a new Mayo Clinic Quadratic (MCQ) equation based on the results of both healthy subjects (n = 580), who had an iothalamate clearance test specifically for kidney donor evaluation, and patients with chronic kidney disease (CKD) (n = 320) (4). However, only 13% of 320 patients with CKD were diabetic, and the validity of the MCQ for patients outside the Mayo Clinic has been questioned (5).

The aim of the present study was to test the MCQ against isotopic glomerular filtration rate (GFR), compared with the recommended MDRD and Cockcroft-Gault formulas, in type 2 diabetic patients with a wide range of GFR (15–209 ml/min per 1.73 m2)—particularly in those with hyperfiltration or normal renal function.

RESEARCH DESIGN AND METHODS

The study was conducted in 118 Caucasian type 2 diabetic patients (63 women; mean ± SD age 57.2 ± 9.7 years and A1C 7.3 ± 2%). According to the values obtained with isotopic GFR, patients were divided into three study subgroups: hyperfiltration (GFR >140 ml/min per 1.73 m2, 26 patients); normal renal function (GFR 90–140 ml/min per 1.73 m2, 56 patients); and CKD stages 3–4 (GFR 15–59 ml/min per 1.73 m2, 36 patients).

Isotopic GFR was measured by a single-shot clearance technique using an intravenous injection of 30–50 μCi 125I-iothalamate corrected for body surface area of 1.73 m2 (6). In each study subgroup, the isotopic GFR was compared with those of the following prediction equations: MDRD (7), Cockcroft-Gault (8), and MCQ (4). The equation for MCQ calculation is as follows:

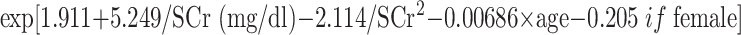graphic file with name M1.gif

where exp is exponent and SCr is serum creatinine. All serum creatinine measurements were performed in the same laboratory and determined by the Jaffé alkaline picrate method (normal range 0.6–1.5 mg/dl), calibrated using the SETpoint Calibrator T13-1291 (Bayer, Barcelona, Spain).

Statistical analysis

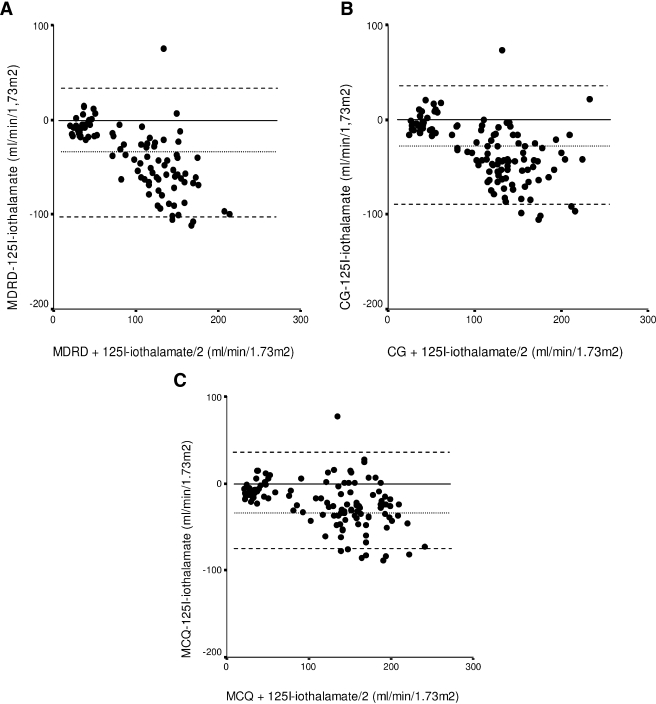
ANOVA was used to determine significant differences (P < 0.05 with Bonferroni adjusted for three contrast tests). The Bland-Altman procedure (9,10) (Fig. 1) and Lins coefficient (Rc) (11) were used to study accuracy. SAS software (version 9.1; SAS Institute, Cary, NC) was used for statistical analysis.

Fig. 1.

Fig. 1

Open in a new tab

RESULTS

Mean 125I-iothalamate GFR was 96.3 ± 50.9 ml/min per 1.73 m2. In the hyperfiltration group (aged 49.6 ± 8.5 years [range 31–62]; 13 women), mean isotopic GFR was 159.5 ± 18.8 ml/min per 1.73 m2 and SCr 79.3 ± 21.7 μmol/l. In this group, the prediction equations that included MCQ were inaccurate compared with isotopic GFR and differed statistically and significantly (P < 0.05). Bias obtained with the MDRD was −83.1 ml/min per 1.73 m2 (Rc 0.034), with the Cockcroft-Gault formula −62.0 ml/min per 1.73 m2 (Rc 0.015) and the MCQ equation −50.9 ml/min per 1.73 m2 (Rc 0.045).

In the normal renal function group (56 ± 8.2 years [range 31–69]; 37 women), mean isotopic GFR was 115.6 ± 14.1 ml/min per 1.73 m2 and SCr 88.7 ± 14.8 μmol/l. In this group, all prediction equations and MCQ were inaccurate compared with the isotopic GFR and differed statistically and significantly (P < 0.05). Bias obtained with the MDRD was −46.5 ml/min per 1.73 m2 (Rc 0.025), with the Cockcroft-Gault formula −41.4 ml/min per 1.73 m2 (Rc 0.013) and the MCQ equation −23.2 ml/min per 1.73 m2 (Rc 0.040).

In the CKD stages 3–4 group (64.1 ± 8.0 years [range 45–84]; 13 women), mean isotopic GFR was 31.2 ± 10.8 ml/min per 1.73 m2 and SCr 249.0 ± 91.5 μmol/l. In this group, the prediction equations and MCQ also presented significant differences compared with isotopic GFR (P < 0.05). However, bias obtained with the MDRD was −5.3 ml/min per 1.73 m2 (Rc 0.452), with the Cockcroft-Gault formula −0.2 ml/min per 1.73 m2 (Rc 0.471) and the MCQ equation −4.5 ml/min per 1.73 m2 (Rc 0.526).

CONCLUSIONS

According to our results, the application of these equations is inadequate in situations of hyperfiltration and normal renal function. In the CKD stages 3–4 group, the results obtained presented no evidence of superiority of the MCQ equation over the MDRD equation and Cockcroft-Gault formula. The validity of GFR predictive equations must be verified in the diabetic population, as both equations (MDRD and Cockcroft-Gault) were developed from the results of nondiabetic subjects with CKD.

Despite yielding statistically significant differences, in hyperfiltration and normal renal function situations, MCQ presents a mean ± SD closer to those of the isotopic method and lower bias and CIs in the estimation of renal function. In this respect, a recent study (12) concluded that the MDRD equation results in considerably higher rates of estimated GFR for CKD classes 2 and 3 compared with the MCQ equation, whereas MDRD and MCQ were comparable in CKD classes 4 and 5. In patients with normal Scr, the MDRD equation underestimated the iothalamate GFR; thus, its limitation in clinical practice may give rise to a misclassification of renal function stage. In contrast to Rigalleau et al. (13), we believe the MCQ equation offers no advantage over conventional prediction equations because it excessively underestimates GFR (high bias and low precision).

As in previous studies (14,3), we showed the hyperfiltration situation, with a greater slope for GFR compared with normal filters, to be a marker of poor evolution and worse renal function deterioration in type 2 diabetic patients. In these situations that limit the use of prediction equations, new markers of renal function are required. In this respect, one recent study (15) recommended the use of serum cystatin C (100/cystatin C, expressed as milligrams per liter) to diagnose early renal function decline and develop interventions for protecting renal function while it is normal or even elevated.

In conclusion, our results showed the MCQ and conventional prediction equations to be inaccurate (excessive underestimation) in type 2 diabetic patients with hyperfiltration or normal renal function. In CKD stages 3–4, the results obtained presented no evidence of superiority of the MCQ equation over the MDRD equation or Cockcroft-Gault formula.

Published ahead of print at http://care.diabetesjournals.org on 3 October 2008.

The costs of publication of this article were defrayed in part by the payment of page charges. This article must therefore be hereby marked “advertisement” in accordance with 18 U.S.C. Section 1734 solely to indicate this fact.

References

  • 1.United States Renal Data System: Incidence and prevalence of ESRD. In US Renal Data System 2003 Annual Data Report. Bethesda, MD, National Institutes of Health, National Institutes of Diabetes and Digestive and Kidney Diseases, 2003, p. 47–60
  • 2.Department of Public Health: Catalonia registry [article online], 2004. Barcelona, Spain. Available from http://www.10.gencat.net/catsalut/ocatt/pdfs/Info_renal _2004_cat.pdf. Accessed 10 April 2008 [in Spanish]
  • 3.Fontseré N, Salinas I, Bonal J, Bayés B, Riba J, Torres F, Rios J, Sanmartí A, Romero R: Are prediction equations for glomerular filtration rate useful for the long-term monitoring of type 2 diabetic patients? Nephrol Dial Transplant 21:2152–2158, 2006 [DOI] [PubMed] [Google Scholar]
  • 4.Rule AD, Larson TS, Bergstralh EJ, Slezak JM, Jacobsen SJ, Cosio FG: Using serum creatinine to estimate glomerular filtration rate: accuracy in good health and in chronic kidney disease. Ann Intern Med 141:929–937, 2004 [DOI] [PubMed] [Google Scholar]
  • 5.Froissart MC, Rossert J, Houillier P: The new Mayo Clinic equation for estimating glomerular filtration rate. Ann Intern Med 142:679, 2005 [DOI] [PubMed] [Google Scholar]
  • 6.K/DOQI clinical practice guidelines for chronic kidney disease: evaluation, classification, and stratification. Part 5: evaluation of laboratory measurements for clinical assessment of kidney disease. Am J Kidney Dis 39(Suppl. 1):S76–S110, 2002 [PubMed] [Google Scholar]
  • 7.Levey AS, Bosch JP, Lewis JB, Greene T, Rogers N, Roth D, the Modification of Diet in Renal Disease Study Group: A more accurate method to estimate glomerular filtration rate from serum creatinine: a new prediction equation. Ann Intern Med 130:461–470, 1999 [DOI] [PubMed] [Google Scholar]
  • 8.Cockcroft DW, Gault MH: Prediction of creatinine clearance from serum creatinine. Nephron 16:31–41, 1976 [DOI] [PubMed] [Google Scholar]
  • 9.Bland JM, Altman DG: Statistical methods for assessing agreement between two methods of clinical measurement. Lancet 1:307–310, 1986 [PubMed] [Google Scholar]
  • 10.Bland JM, Altman DG: Measuring agreement in method comparison studies. Stat Methods Med Res 8:135–160, 1999 [DOI] [PubMed] [Google Scholar]
  • 11.Lin LI: A concordance correlation coefficient to evaluate reproducibility. Biometrics 45:255–268, 1989 [PubMed] [Google Scholar]
  • 12.Marsik C, Endler G, Gulesserian T, Wagner OF, Sunder-Plassmann G: Classification of chronic kidney disease by estimated glomerular filtration rate. Eur J Clin Invest 38:253–259, 2008 [DOI] [PubMed] [Google Scholar]
  • 13.Rigalleau V, Lasseur C, Raffaitin C, Perlemoine C, Barthe N, Chauveau P, Combe C, Gin H: The Mayo Clinic quadratic equation improves the prediction of glomerular filtration rate in diabetic subjects. Nephrol Dial Transplant 22:813–818, 2007 [DOI] [PubMed] [Google Scholar]
  • 14.Jones SL, Wiseman MJ, Viberti GC: Glomerular hyperfiltration as a risk factor for diabetic nephropathy: five-year report of a prospective study. Diabetologia 34:59–60, 1991 [DOI] [PubMed] [Google Scholar]
  • 15.Perkins BA, Ficociello LH, Ostrander BE, Silva KH, Weinberg J, Warram JH, Krolewski AS: Microalbuminuria and the risk for early progressive renal function decline in type 1 diabetes. J Am Soc Nephrol 18:1353–1361, 2007 [DOI] [PubMed] [Google Scholar]

Articles from Diabetes Care are provided here courtesy of American Diabetes Association

RESOURCES