Abstract
Variation between individuals is an essential component of natural selection and evolutionary change, but it is only recently that the consequences of persistent differences between individuals on population dynamics have been considered. In particular, few authors have addressed whether interactions exist between individual quality and environmental variation. In part, this is due to the difficulties of collecting sufficient data, but also the challenge of defining individual quality. Using a long-established study population of red deer, Cervus elaphus, inhabiting the North Block of the Isle of Rum, and three quality measures, this paper investigates how differences in maternal quality affect variation in birth body mass and date, as population density varies, and how this differs depending on the sex of the offspring and the maternal quality measure used. Significant interactions between maternal quality, measured as a hind's total contribution to population growth, and population density are reported for birth mass, but only for male calves. Analyses using dominance or age at primiparity to define maternal quality showed no significant interactions with population density, highlighting the difficulties of defining a consistent measure of individual quality.
Keywords: individual heterogeneity, individual quality, environmental variation, population density, Cervus elaphus
1. Introduction
There is increasing evidence that individual differences in performance can have important consequences for population dynamics (Vaupel et al. 1979; Berube et al. 1999; Benton & Beckerman 2005; Metcalf & Pavard 2007; Pelletier et al. 2007). Individual differences may arise for various reasons: for example, differences in resource acquisition (Van Noordwijk & De Jong 1986), variation in digestion efficiencies (Gross et al. 1995) and development (Lummaa & Clutton-Brock 2002). Where such individual differences in performance are consistent throughout life, they can be considered as differences in individual quality, and population biologists have consequently become interested in defining and quantifying this concept (Cam et al. 2002; Van de Pol & Verhulst 2006; Lewis et al. 2006; Metcalf & Pavard 2007).
Many studies examining the effects of variation in individual quality have focused on maternal effects that influence offspring traits (Clutton-Brock et al. 1984, 1987b; Beckerman et al. 2002; Mousseau & Fox 1998; Lummaa 2003). These are of particular interest where offspring quality at birth influences both maternal and offspring lifetime reproductive success and so can lead to a large variance in inclusive maternal fitness (Clutton-Brock 1998; Hewison & Gaillard 1999; Kruuk et al. 1999b; Loison et al. 2004), and where selection pressures acting through maternal versus offspring fitness may be antagonistic, as for example, for offspring birth mass in Soay sheep (Wilson et al. 2005). The effects of environmental variation on traits such as birth mass have also been widely demonstrated (for example in red deer, Albon et al. 1983; Clutton-Brock et al. 1987a; Coulson et al. 2003), but although studies have been able to show that heterogeneity exists among individuals in their response to such environmental effects, the majority of this work focuses on differences in the strength of environmental effects among different stage, age and sex classes (for example, in a plant species, Calathea ovandensis, Horvitz & Schemske 1995; experiments on soil mites, Benton & Beckerman 2005; red deer, Clutton-Brock et al. 1982; and Soay sheep, Coulson et al. 2001). By contrast, there has been comparatively little work explicitly investigating how persistent individual differences in quality interact with environmental variation to influence offspring quality. For example, if the effects of increasing competition are felt more strongly by individuals that are already at a competitive disadvantage (Rubenstein 1981), we would expect individuals of lower quality to be more influenced by increases in population density than high-quality individuals.
The objective of this study is to examine how differences in individual quality in red deer hinds affect the extent of density-dependent effects on offspring birth traits. Although density-dependent effects have been recorded in many populations (for example, Jorgenson et al. 1997; Bennetts et al. 2000; Kruger 2005; Stewart et al. 2005), effects in the red deer population of the North Block of the Isle of Rum, Scotland are particularly well characterized thanks to long-term monitoring of life histories. Previous work has shown that age at primiparity, birth date and inter-birth interval increased with population density, while female fecundity and calf over-winter survival declined (Clutton-Brock et al. 1982; Kruuk et al. 1999a; Clutton-Brock & Coulson 2002).
In addition, earlier work has investigated how interactions between individual quality and density influence individual traits. Twenty years ago, Clutton-Brock et al. (1987b) reported a significant interaction between population density and maternal dominance rank on calf survival through the second year of life, such that at high densities offspring born to dominant individuals were no more likely to survive than those born to more subordinate females, but in contrast, at low densities, yearling survival was positively correlated with maternal dominance. The authors suggested that at high densities, scramble competition between female groups increased relative to contest competition within groups, so that there was no longer a dominance advantage. Additional evidence for interaction between individual heterogeneity and density on plasticity in calving date has been reported by Nussey et al. (2005). Among those females that experienced high population density in the year of birth, only a few individuals, suggested to be those in the best condition, were able to calve early following favourable weather conditions. By contrast, among deer, those had experienced low population density conditions during their year of birth, all females were able to respond to favourable conditions by calving early. Overall, however, few authors have considered whether permanent differences between individuals affect their response to changes in population density in this or other species.
Exploring the role of persistent individual quality in wild populations requires long-term detailed datasets of marked and monitored animals, because even a high-quality individual may not appear to be of high quality at some stages (Cam et al. 2004). Even where such data are available, assigning a persistent measure of quality to an individual is not trivial. For example, per generational measures such as lifetime reproductive success, though widely agreed to be more appropriate than measures calculated at a single life-history stage as a measure of permanent heterogeneity (Endler 1986; Stearns 1992; Brommer et al. 2002; Moyes et al. submitted), have been criticized for their inability to correct for temporal environmental and ecological fluctuations (Coulson et al. 2006). Contribution to population growth is one, more dynamic alternative. In general, however, the measure of quality that should be selected is currently unclear, and ecologists are yet to agree on how best to characterize individuals.
In this study, we investigate how maternal quality interacts with population density to explain variation in the offspring traits of birth mass and birth date. Maternal quality is characterized by three measures of performance: total lifetime contribution to population growth, age at primiparity and dominance (see Moyes et al. (submitted) for further discussion of these measures). For each maternal quality measure, we test the prediction that females of different quality respond differently to variation in population density.
2. Material and methods
(a) Study population
Data were collected from the red deer population living in the North Block of the Isle of Rum, off the west coast of Scotland, where research has continued since the termination of the annual cull of 14% in 1972 (Clutton-Brock 1985; Coulson et al. 1997). Numbers rose rapidly to approximately 300, stabilizing in the early 1980s (Clutton-Brock & Coulson 2002). In this population, females can be sexually mature at 2.3 years and give birth to their first offspring at the age of 3 years, although at high density many females delay their first reproductive attempt until they are aged 4 years (Clutton-Brock et al. 1989).
All individuals can be recognized, either from individual idiosyncrasies or artificial marks (Clutton-Brock et al. 1982). Censuses take place five times a month in most months of the year to record the identity, location and activity of each animal seen (Clutton-Brock et al. 1982). In addition, during the calving season (approx. 20 May–30 June), daily observations are taken to identify calving date for each female and monitor calf neonatal survival (Clutton-Brock et al. 1982). Most calves born in the study area are caught, sexed and weighed within hours of birth, and the rest are sexed through observation as soon as possible afterwards (Clutton-Brock et al. 1982). In addition, the date of death for the majority of animals is identified within a week (Clutton-Brock et al. 1982, 1987b). Life histories are known for over 95% of the animals in the study area, including date of birth and maternity (Clutton-Brock et al. 1982).
For the purpose of analyses, only female deer born after 1974, when regular censuses of the study area began, and before 1987 were included. This excludes cohorts in which some individuals are still alive. Complete life histories were required as calculating total contribution to population growth requires information about lifetime performance.
The following variables were used in the analyses.
(i) Female reproductive status
Females were classified according to their reproductive status in the previous year.
First breeders. Those who had not previously been pregnant.
True yelds. Those who had reproduced before, but not in the previous year.
Summer yelds. Those who had reproduced in the previous year but had lost the calf before the end of September.
Winter yelds. Those who had bred in the previous year but had lost the calf between October and May.
Milk hinds. Those who had successfully raised a calf in the previous year. (Clutton-Brock et al. 1982)
(ii) Residual offspring birth mass
Variation in the age at which new born calves were weighed (in hours) was accounted for by linearly regressing mass at capture against age at capture in hours and subtracting the predicted mass at the appropriate time from the actual capture mass. Each individual's residual from this line is considered its residual birth mass. The regression equation used in these analyses is as follows (R2=0.295):
Offspring born to hinds included in this dataset which could not be caught and weighed (8.6% of calves) were excluded from the analysis.
(iii) Offspring birth date
Estimated birth date of the offspring expressed as number of days since 1 January.
(iv) Measures of maternal quality
Three measures of maternal quality were used in the analyses: lifetime contribution to population growth (Σpt(i)), age at primiparity and dominance. Various other measures of quality, for example, longevity or lifetime reproductive success, have been used in previous studies in this and other populations (Espie et al. 2004; Blackmer et al. 2005; Moyes et al. 2006). However, lifetime performance measures are frequently highly correlated (Moyes et al. submitted). We found high correlation between Σpt(i), lifetime reproductive success and longevity and, for reasons of brevity, do not report analyses using the latter metrics here. By contrast, age at primiparity and dominance correlate less well with lifetime performance measures (Moyes et al. submitted) and are therefore included as alternative maternal quality measures. In particular, using dominance as a measure of maternal quality when considering performance is helpful, for it removes the circularity of employing performance-based quality measures in such studies.
(v) Lifetime contribution to population growth
Coulson et al. (2006) developed an approach that allows an individual's contribution to population growth over a time step, pt(i), to be estimated from life-history and population data. Here, the pt(i) for each year of a female's life is calculated and then summed to give a lifetime measure, Σpt(i), for each female.
where st(i) is a binary variable denoting whether individual i survives from 1 year, t, to the next (for the purposes of this calculation year is defined as from 15 May in year t to 14 May in year t+1); ft(i) is the number of offspring produced by individual i in year t which survive to the beginning of year t+1; is mean survival at time t; is mean fecundity at time t; and Nt is the number of females in the population aged 1 year or older in year t.
Thus, pt(i) can be positive or negative, with a negative value indicating that an individual performed worse than the population mean.
(vi) Age at primiparity
The age at which the female first gave birth to a calf, fitted as a three-level factor: 3, 4 or 5 years.
(vii) Dominance
Dominance was calculated using David's score (DS), which provides a measure of individual successes in dominance interactions, from which a rank order can be derived (David 1987, 1988; Gammell et al. 2003). This measure can be used to calculate dominance ranks for individuals in a group based on the outcomes of their antagonistic interactions with other group members, while taking relative strengths of opponents and repeated interactions between group members into account (Gammell et al. 2003).
DS is calculated as
where w is sum of i's Pij values, where Pij is the proportion of wins by individual i in his interactions with another individual j, i.e. the number of times that i defeats j (αij) divided by the total number of interactions between i and j (nij), so that Pij=αij/nij; w2 is summed w values (weighted by appropriate Pij values) of those individuals with which i interacted; l is sum of i's Pji values; and l2 is summed l values (weighted by appropriate Pij) of those individuals with which i interacted.
Dominance scores for each hind were based on all social interactions recorded in their lifetime, rather than on a per year basis. Data on the dominance interactions for this population were available between the years of 1974 and 1995, and therefore all, or at least 9 years, of a hind's lifetime dominance interactions were captured by this dataset.
(viii) Year
The year in which the calf was born was included as a continuous covariate to account for change in offspring traits over time (Coulson et al. 2003).
(ix) Population density
For each year, the total number of females older than 1 year seen in more than 10% of censuses conducted between January and May was used as a measure of the population density, following Coulson et al. (2003). A 10% cut-off was used to exclude transients while ensuring that all resident individuals were included in the population (Coulson et al. 2004).
(x) Climatic variables
Climatic variables previously identified as significant in explaining offspring birth mass and birth date in the population were used: average temperature through March and April (Albon et al. 1987; Sims et al. 2007) and precipitation between September and December (Coulson et al. 2003), respectively.
(b) Statistical analysis
The aim of the statistical analysis was to analyse offspring traits (birth mass and birth date) as a function of the interaction between population density and maternal quality. To do this, linear mixed effects models were fitted using the restricted maximum likelihood method. Linear mixed effects models were used to account for pseudoreplication arising from multiple data observations from each female. The use of the ‘lme’ function from the library ‘nlme’ in the statistical package ‘R’ v. 2.6.0 (R Development Core Team 2007) allowed models with nested factors for the random effects (mother's identity) to be used.
Offspring birth date and birth mass were log transformed, a method chosen by graphical analysis of histograms of the raw and transformed data. Once models were fitted, leverage of a given data point xi was calculated as
where n is the number of points in the dataset and is the mean value of x, so that the denominator is the corrected sum of squares for x. A point was considered highly influential if
where p is the number of parameters in the model (Crawley 2002).
These highly influential points were then considered as outliers and removed: this reduced the dataset by one and two points for male and female offspring data, respectively. No data points were removed from the models of offspring birth dates. Results reported below are for the datasets without outliers.
Aside from measures of maternal quality, explanatory variables for both offspring traits were selected based on the results reported by Coulson et al. (2003) for both offspring birth date and offspring birth mass. The full models of birth mass contained mother's identity as a random factor, and mother's reproductive status, mother's age (quadratic), average March to April temperature, offspring birth date, population size of adult females (the measure of population density) and three measures of maternal quality as fixed effects. Offspring sex was omitted as the sexes were analysed separately (see below). The initial model of birth date contained mother's identity as a random factor and mother's reproductive status, mother's age (quadratic), total precipitation between September and December during gestation, offspring birth mass, year (as a continuous variable), population density and the three measures of maternal quality as fixed effects. In both models, interaction terms were fitted between population density and maternal quality measures.
To determine the significance of these interactions, terms were progressively removed until no further simplification was possible or all interactions had fallen out of the model. Model selection was based on the results of ANOVAs (F tests) and Akaike's information criterion (AIC), which compares the goodness of fit of models, while penalising an increase in the number of parameters (Crawley 2002; Burnham & Anderson 2004). Where there is a very small difference in AIC between models (less than 2), it is argued that the models are equivalent and the model with the smallest number of parameters should be used (Burnham & Anderson 2004).
Previous analyses of offspring traits in this population have suggested differences between sexes in their response to changes in population density (Kruuk et al. 1999a; Clutton-Brock & Coulson 2002). To explore how interactions between female quality and density were affected by differential costs or investment strategies when bearing male versus female offspring, the analyses were carried out separately for each sex.
3. Results
(a) Birth mass
(i) Male offspring
Maternal identity explained 33% of the variance in male offspring birth mass in both the full and simplified models. After model simplification, only one of the three measures of maternal quality showed a significant interaction with density in explaining male calf birth mass: lifetime contribution to population growth (Σpt(i)). This interaction effect and the main effects of Σpt(i) and population density on male offspring birth mass in this model are reported in table 1a. The AIC values for (i) the full model without interaction terms, (ii) the full model and (iii) the most simplified model are given in supplementary material for all four models of offspring birth traits.
Table 1.
Results from linear mixed effects models of offspring birth traits. Each sub-table gives the main effects and interaction terms for all maternal fitness measures and population density effects remaining in the models after simplification. Results are for models of (a) male offspring birth mass (346 calves born to 131 mothers), (b) female offspring birth mass (328 calves born to 127 mothers), (c) male offspring birth date (346 calves born to 131 mothers) and (d) female offspring birth date (328 calves born to 127 mothers). Both birth mass models (a) and (b) still retain significant main effects of maternal reproductive status, maternal age (quadratic), average March to April temperature and birth date, but these are not shown here for brevity. Similarly, both birth date models (c) and (d) still retain significant main effects of maternal reproductive status, maternal age (quadratic), September to December rain, year and birth mass. All p-values are for a two-tailed test of the null hypothesis that the value of the fixed effect is equal to zero (Crawley 2002).
| value | s.e. | residual d.f. | t-value | p-value | |
|---|---|---|---|---|---|
| (a) simplified birth mass model (male calves) | |||||
| no. of adult females | −0.0003 | 0.0003 | 206 | −1.0143 | 0.3116 |
| Σpt(i) | −3.6335 | 2.6294 | 135 | −1.3818 | 0.1693 |
| no. of adult females: Σpt(i) | 0.0388 | 0.0161 | 206 | 2.5075 | 0.0169 |
| (b) simplified birth mass model (female calves) | |||||
| no. of adult females | −0.0001 | 0.0002 | 196 | −0.2609 | 0.7945 |
| Σpt(i) | 2.3016 | 0.5243 | 130 | 4.3903 | 0.0000 |
| (c) simplified birth date model (male calves) | |||||
| no. of adult females | 0.0000 | 0.0000 | 206 | 0.5148 | 0.6072 |
| Σpt(i) | −0.4225 | 0.1484 | 135 | −2.8463 | 0.0051 |
| (d) Simplified birth date model (female calves) | |||||
| no. of adult females | 0.0001 | 0.0001 | 191 | 0.9675 | 0.3345 |
| Σpt(i) | −0.1055 | 0.1624 | 123 | −0.6497 | 0.5171 |
| age at primiparity (4) | 0.0028 | 0.0053 | 123 | 0.5382 | 0.5914 |
| age at primiparity (5) | 0.0173 | 0.0075 | 123 | 2.2997 | 0.0232 |
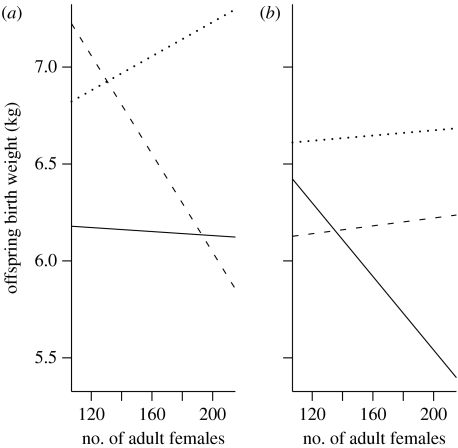
The interaction is displayed graphically in figure 1a. Here ‘low quality’ refers to mothers with Σpt(i) less than or equal to −0.005 (n=97), medium quality refers to females with a Σpt(i) greater than −0.005 but less than 0.005 (n=105), i.e. centred around the mean value, and high quality refers to females with a total Σpt(i) greater than or equal to 0.005 (n=151). High-quality females gave birth to male offspring of slightly higher mass at high density. Very low-quality females gave birth to slightly lighter male offspring at high densities than at low densities. The strongest effect, however, is shown by medium-quality mothers that had much lighter offspring at high population densities compared with low population densities. Overall, at low density, there was less difference in the mass of male offspring born to different quality females than at high density.
Figure 1.
Plots showing the interaction between density and lifetime contribution to population growth, Σpt(i), in explaining offspring birth mass for (a) male offspring (interaction significant, see table 1a) and (b) female offspring (interaction not significant, see table 1b). See text for definitions of high, medium and low quality. Solid line, low total pt(i); dashed line, medium total pt(i); dotted line, high total pt(i).
(ii) Female offspring
Table 1b gives the main effects of population density and the remaining maternal quality measure (Σpt(i)), after model simplification until all interaction terms had fallen out. Maternal identity explained 38% of the variance in the full and simplified models.
None of the three measures of maternal quality showed a significant interaction with density in explaining female calf birth mass. Lifetime contribution to population growth (Σpt(i)) remained in the minimal adequate model as a main effect, but maternal dominance and age at primiparity had no effect on female calf birth mass. There was also no significant effect of population density. Therefore, only female quality, as measured by Σpt(i), had a significant effect on female calf mass.
Low-quality females (defined as above, n=70) producing female offspring gave birth to lighter offspring at high densities than at low densities (figure 1b). This is similar to the trend seen for medium-quality (n=105) mothers producing male offspring. By contrast, both high- (n=162) and medium-quality females produced slightly heavier female offspring at high population density than at low density. In general, although the effects of density on female birth mass are dependent on maternal quality, the effects are smaller than for male birth mass, and this is reflected in the non-significance of the interaction term in the analysis.
(b) Birth date
There were no significant interactions between population density and maternal quality in explaining offspring birth date for either offspring sex. Population density as a main effect also had no significant influence on the birth date of either male or female calves. Indeed, only female quality, as measured by (Σpt(i)), had a significant effect on male calf birth date. Maternal identity explained 17% of the variation between offspring in the full and simplified models for male calves, and 16% in the full and simplified models for female calves. Effect sizes of population density and remaining measures of maternal quality in the simplified models (until all interaction terms had fallen out) are reported in table 1c,d for male and female calves, respectively.
4. Discussion
These results show that the association between population density and birth mass of male, but not female, offspring is dependent on maternal quality, when measured as lifetime Σpt(i). However, no such interactions were found for birth date. In addition, no interactions between maternal quality and density in explaining variation in offspring birth mass or birth date were found with maternal age at primiparity or dominance.
(a) Birth mass
In the following, ‘low quality’ refers to mothers with Σpt(i) less than or equal to −0.005, medium quality refers to females with a Σpt(i) greater than −0.005 but less than 0.005 and high quality refers to females with a total Σpt(i) greater than or equal to 0.005.
Results presented here suggest the birth mass of male offspring born to medium-, rather than low- or high-, quality mothers may be more affected by high population densities when food is presumably limiting. This is consistent with a hypothesis that females that are of lower quality than others in the population may consistently be in poorer physiological condition at the start of gestation and so allocate less to offspring when experiencing reduced food availability, perhaps controlling partition of energy to the calf so as to maintain their own condition. By contrast, high-quality mothers may experience no trade-off between maintaining their own condition and allocation to their offspring. Festa-Bianchet et al. (1998) showed a higher cost of reproduction for lighter ewes at high population density. These results could also be explained by competition mechanisms. Lower quality mothers may be less successful in contest competitions, whereby dominant individuals prevent subordinates from feeding at the best sites, at all population densities. When the population is food limited at high density, lower quality mothers may struggle to feed and be in particularly poor physiological condition throughout gestation compared with high-quality mothers. However, the concept of increased effects of contest competition at high population densities is in contrast to the hypothesis put forward by Clutton-Brock et al. (1987b), who suggested decreasing relative importance of contest competition with population density and increasing importance of scramble competition, as neighbouring feeding parties begin to overlap in home ranges. Given this, and the lack of interaction found between dominance and density, competition explanations seem irrelevant, unless lower quality females associate in feeding groups that perform badly at scramble competition, perhaps through possession of smaller home ranges. Knowledge of female physiological condition at conception and during gestation would be useful to disentangle these explanations.
Compared with medium-quality mothers, low-quality mothers showed only a very slight decrease in male offspring birth mass as population density increased. However, they also had notably lighter calves than higher quality mothers at low density, i.e. in favourable conditions. This trend may reflect a lack of plasticity in low-quality mothers, so that they are not able to respond to favourable low-density conditions by allocating greater resources to male offspring. Such trends have been previously shown in the population: Nussey et al. (2005) showed that females who had early experience of high population density on average showed no plasticity for calving date, in contrast to females who had early experience of low population density. In addition, the effects of density on birth mass of calves born to low-quality females may be an artefact of reproductive filtering. Given that not all females in the population breed every year, we would expect low-quality females not to breed when under nutritional stress, or to suffer higher foetal mortality. Therefore, any low-quality females who do breed in such conditions are presumably in better condition on average and so would be predicted to have relatively heavier offspring than if all low-quality females bred.
It is less clear why the birth mass of males born to high-quality females should increase with population density, as opposed to remaining constant. It is possible that females that can afford to do so allocate more to male offspring at higher densities, in an attempt to counteract effects on lifetime fitness that arise as a result of being born at high population density (for example, reduced physiological condition, Nussey et al. 2005). Further work examining interactions between density and downstream maternal effects would be useful to elucidate such effects.
The analyses show strong differences in the interaction between maternal quality and population density in explaining male versus female calf birth mass. This supports the theory that in polygynous species high-quality mothers should allocate more to sons than to daughters, which was originally suggested by Trivers & Willard (1973) as an additional comment to their work proposing male sex ratio bias in such mothers. In strongly polygynous species, males have greater variance in lifetime reproductive success than females, so small increases in parental investment in male offspring can lead to larger fitness returns than an equivalent investment in female offspring (but see Hewison & Gaillard (1999) and Hewison et al. (2005), for conditions under which this can be reversed). For example, Kruuk et al. (1999b) showed that male, but not female, lifetime reproductive success in this population of red deer was associated with birth mass. Similarly, Loison et al. (2004) found that maternal quality accounts for more variance in male offspring body mass than female body mass in red deer. Male-biased maternal care has been found in a number of ungulates (Cassinello 1996; Berube et al. 1996; Birgersson et al. 1998), and Kühl et al. (2007) report a consistent bias towards allocation to male calves in saiga antelope, suggesting mothers are able to preferentially target resources in utero. In our study, when giving birth to females, even mothers that could afford to do so (i.e. high-quality mothers) did not appear to allocate significantly more to female offspring as density increased, in contrast to increased allocation seen in male calves produced by high-quality mothers. However, the difference in the response of medium-quality females to increasing population density for different sex calves also suggests a greater cost of producing a male calf. In contrast to female calves, male offspring of medium-quality females are much lighter at high density. That the reverse is true for low-quality mothers may again be an artefact of the reproductive filtering as discussed previously.
(b) Offspring birth date
Females of higher quality gave birth earlier than females of lower quality at all densities, consistent with Coulson et al. (2003) who found that there was significant directional selection for earlier birth date in the population. Previous studies have shown that calves born earlier have a higher probability of first year survival, presumably due to increased lactation period or growth time before winter (Clutton-Brock et al. 1982). Additionally, birth date has been recorded to get later with rising density in the population (Clutton-Brock et al. 1987a; Coulson et al. 2003). Although there is no significant effect of density on birth date reported here, the same trend is found (with the non-significance due to the use of a smaller dataset than those in previous studies). This was independent of female quality. This effect is presumably because conception date increases with density (Clutton-Brock et al. 1982), although gestation length may also be important as found in the Soay sheep population (Forchhammer et al. 2001).
Maternal identity explained a much lower percentage of the variance in offspring birth date than offspring birth mass, i.e. maternal individual heterogeneity was much smaller for this trait. Although modification of gestation length is possible in this species (Garcia et al. 2006), selection for earlier calving date may be stabilized by the costs to offspring pre-natal growth. This is supported by the smaller downstream effect of birth date on lifetime reproductive success compared with birth mass (Kruuk et al. 1999b). Additionally, calving date is not considered a wholly maternal trait, as gestation length is controlled by both mother and offspring (Clutton-Brock et al. 1987b; Nussey et al. 2005). Further work should address the role of gestation length versus conception date in determining calving date in this population.
(c) Fitness measures
Although this study demonstrates that there is a significant association between maternal quality, measured as a lifetime contribution to population growth, and density-dependent changes on offspring birth mass, the results were not repeated for age at primiparity and dominance. This may reflect variation in the root causes of heterogeneity in each of these measures: for example, age at primiparity is expected to be strongly determined by conditions in the early part of life, such as density and weather in the year of birth (see Forchhammer et al. 2001), while dominance is probably determined by the quality of habitat accessed and home range throughout life (see Clutton-Brock et al. 1982). Lifetime measures of fitness have, in contrast, been shown to be determined by both early environment (Kruuk et al. 1999b) and the strength of a hind's selection for Agrostis/Festuca grassland during her lifespan (McLoughlin et al. 2006).
In general, although the best definition of quality is probably individual fitness, fitness is not a simple quantity to define or quantify (Metz et al. 1992; Stearns 1992; McGraw & Caswell 1996; Murray 1997; Benton & Grant 2000; Brommer et al. 2004). Various studies have shown that different proxies of fitness are appropriate in different contexts (for example, Metz et al. 1992; Mylius & Diekmann 1995; Brommer et al. 2004), and that they may not always be highly correlated (Moyes et al. submitted). The variation in the behaviour of different quality measures presented here supports this complexity.
The results presented here have important implications for our understanding of the role of individual variation and density-dependent effects in both this deer population and in the wider field. For example, previous studies have reported that birth mass in red deer is not greatly affected by the additive effects of population density (Clutton-Brock et al. 1982), whereas our results suggest that population density can influence individual birth mass, at least that of males. Although the population dynamical consequences of such an interaction are unknown, it is clear that different quality individuals are affected by changes in population density in contrasting ways.
Acknowledgments
We would like to thank Scottish Natural Heritage for permission to work on Rum, their staff for local support, Fiona Guinness, Ali Morris, Sean Morris and many other fieldworkers on the Rum deer project, Tom Ezard for statistical advice and Kelly Moyes, Dan Nussey, Jean-Michel Gaillard and two anonymous reviewers for their helpful comments and discussion. The long-term data collection on Rum is funded by the Natural Environment Research Council.
Supplementary Material
AIC values from the linear mixed effects models of offspring birth traits reported in table 1. Each sub-table gives the AIC value for (i) the model containing all three measures of female quality and population density but no interactions, (ii) the model containing all three measures of female quality and their interactions with density (the full model), and (iii) the minimum adequate model for which the effect sizes are reported in table 1. Results are for models of (a) male offspring birth mass, (b) female offspring birth mass, (c) male offspring birth date and (d) female offspring birth date, as above.
References
- Albon S.D, Guinness F.E, Clutton-Brock T.H. The influence of climatic variation on the birth weights of red deer (Cervus elaphus) J. Zool. 1983;200:295–298. [Google Scholar]
- Albon S.D, Clutton-Brock T.H, Guinness F.E. Early development and population dynamics in red deer. II. Density independent effects and cohort variation. J. Anim. Ecol. 1987;56:69–81. doi:10.2307/4800 [Google Scholar]
- Beckerman A, Benton T.G, Ranta E, Kaitala V, Lundberg P. Population dynamic consequences of delayed life-history effects. Trends Ecol. Evol. 2002;17:263–269. doi:10.1016/S0169-5347(02)02469-2 [Google Scholar]
- Bennetts R.E, Fasola M, Hafner H, Kayser Y. Influence of environmental and density-dependent factors on reproduction of Little Egrets. Auk. 2000;117:634–639. doi:10.1642/0004-8038(2000)117[0634:IOEADD]2.0.CO;2 [Google Scholar]
- Benton, T. G. & Beckerman, A. P. 2005 Population dynamics in a noisy world: lessons from a mite experimental system. In Advances in ecological research, vol. 37. Population dynamics and laboratory ecology (eds H. Caswell & R. Dasharnais), pp. 143–181. London, UK: Academic Press Ltd.
- Benton T.G, Grant A. Evolutionary fitness in ecology: comparing measures of fitness in stochastic, density-dependent environments. Evol. Ecol. Res. 2000;2:769–789. [Google Scholar]
- Berube C.H, Festa Bianchet M, Jorgenson J.T. Reproductive costs of sons and daughters in Rocky Mountain bighorn sheep. Behav. Ecol. 1996;7:60–68. doi:10.1093/beheco/7.1.60 [Google Scholar]
- Berube C.H, Festa-Bianchet M, Jorgenson J.T. Individual differences, longevity, and reproductive senescence in bighorn ewes. Ecology. 1999;80:2555–2565. doi:10.2307/177240 [Google Scholar]
- Blackmer A.L, Mauck R.A, Ackerman J.T, Huntington C.E, Nevitt G.A, Williams J.B. Exploring individual quality: basal metabolic rate and reproductive performance in storm-petrels. Behav. Ecol. 2005;16:906–913. doi:10.1093/beheco/ari069 [Google Scholar]
- Birgersson B, Tillbom M, Ekvall K. Male-biased investment in fallow deer: an experimental study. Anim. Behav. 1998;56:301–307. doi: 10.1006/anbe.1998.0783. doi:10.1006/anbe.1998.0783 [DOI] [PubMed] [Google Scholar]
- Brommer J.E, Merila J, Kokko H. Reproductive timing and individual fitness. Ecol. Lett. 2002;5:802–810. doi:10.1046/j.1461-0248.2002.00369.x [Google Scholar]
- Brommer J.E, Gustafsson L, Pietiainen H, Merila J. Single-generation estimates of individual fitness as proxies for long-term genetic contribution. Am. Nat. 2004;163:505–517. doi: 10.1086/382547. doi:10.1086/382547 [DOI] [PubMed] [Google Scholar]
- Burnham K.P, Anderson D.R. Multimodel inference: understanding AIC and BIC in model selection. Soc. Methods Res. 2004;33:261–304. doi:10.1177/0049124104268644 [Google Scholar]
- Cam E, Link W.A, Cooch E.G, Monnat J.Y, Danchin E. Individual covariation in life-history traits: seeing the trees despite the forest. Am. Nat. 2002;159:96–105. doi: 10.1086/324126. doi:10.1086/324126 [DOI] [PubMed] [Google Scholar]
- Cam E, Monnat J.Y, Royle J.A. Dispersal and individual quality in a long lived species. Oikos. 2004;106:386–398. doi:10.1111/j.0030-1299.2003.13097.x [Google Scholar]
- Cassinello J. High-ranking females bias their investment in favour of male calves in captive Ammotragus lervia. Behav. Ecol. Sociobiol. 1996;38:417–424. doi:10.1007/s002650050259 [Google Scholar]
- Clutton-Brock T.H. Reproductive success in red deer. Sci. Am. 1985;252:86. [Google Scholar]
- Clutton-Brock T.H. Introduction: studying reproductive costs. Oikos. 1998;83:421–423. doi:10.2307/3546669 [Google Scholar]
- Clutton-Brock T.H, Coulson T. Comparative ungulate dynamics: the devil is in the detail. Phil. Trans. R. Soc. B. 2002;357:1285–1298. doi: 10.1098/rstb.2002.1128. doi:10.1098/rstb.2002.1128 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Clutton-Brock T.H, Guinness F.E, Albon S.D. University of Chicago Press; Chicago, IL: 1982. Red deer: behaviour and ecology of two sexes. [Google Scholar]
- Clutton-Brock T.H, Albon S.D, Guinness F.E. Maternal dominance, breeding success and birth sex-ratios in red deer. Nature. 1984;308:358–360. doi:10.1038/308358a0 [Google Scholar]
- Clutton-Brock T.H, Major M, Albon S.D, Guinness F.E. Early development and population dynamics in red deer. I. Density-dependent effects on juvenile survival. J. Anim. Ecol. 1987a;56:53–67. doi:10.2307/4799 [Google Scholar]
- Clutton-Brock T.H, Albon S.D, Guinness F.E. Interactions between population-density and maternal characteristics affecting fecundity and juvenile survival in red deer. J. Anim. Ecol. 1987b;56:857–871. doi:10.2307/4953 [Google Scholar]
- Clutton-Brock T.H, Albon S.D, Guinness F.E. Fitness costs of gestation and lactation in wild mammals. Nature. 1989;337:260–262. doi: 10.1038/337260a0. doi:10.1038/337260a0 [DOI] [PubMed] [Google Scholar]
- Crawley M.J. Wiley; Chichester, UK: 2002. Statistical computing: an introduction to data analysis using S-plus. [Google Scholar]
- Coulson T, Albon S, Guinness F, Pemberton J, Clutton-Brock T. Population substructure, local density, and calf winter survival in red deer (Cervus elaphus) Ecology. 1997;78:852–863. doi:10.2307/2266064 [Google Scholar]
- Coulson T, Catchpole E.A, Albon S.D, Morgan B.J.T, Pemberton J.M, Clutton-Brock T.H, Crawley M.J, Grenfell B.T. Age, sex, density, winter weather, and population crashes in Soay sheep. Science. 2001;292:1528–1531. doi: 10.1126/science.292.5521.1528. doi:10.1126/science.292.5521.1528 [DOI] [PubMed] [Google Scholar]
- Coulson T, Kruuk L.E.B, Tavecchia G, Pemberton J.M, Clutton-Brock T.H. Estimating selection on neonatal traits in red deer using elasticity path analysis. Evolution. 2003;57:2879–2892. doi: 10.1111/j.0014-3820.2003.tb01528.x. doi:10.1554/03-126 [DOI] [PubMed] [Google Scholar]
- Coulson T, Guinness F, Pemberton J, Clutton-Brock T. The demographic consequences of releasing a population of red deer from culling. Ecology. 2004;85:411–422. doi:10.1890/03-0009 [Google Scholar]
- Coulson T, Benton T.G, Lundberg P, Dall S.R.X, Kendall B.E, Gaillard J.-M. Estimating individual contributions to population growth: evolutionary fitness in ecological time. Proc. R. Soc. B. 2006;273:547–555. doi: 10.1098/rspb.2005.3357. doi:10.1098/rspb.2005.3357 [DOI] [PMC free article] [PubMed] [Google Scholar]
- David H.A. Ranking from unbalanced paired-comparison data. Biometrika. 1987;74:432–436. doi:10.1093/biomet/74.2.432 [Google Scholar]
- David H.A. An extreme null distribution approach to the problem of paired comparisons. Commun. Stat. Theory Methods. 1988;17:4005–4009. doi:10.1080/03610928808829852 [Google Scholar]
- Endler J.A. Princeton University Press; Princeton, NJ: 1986. Natural selection in the wild. [Google Scholar]
- Espie R.H.M, James P.C, Oliphant L.W, Warkentin I.G, Lieske D.J. Influence of nest-site and individual quality on breeding performance in merlins Falco columbarius. Ibis. 2004;146:623–631. doi:10.1111/j.1474-919x.2004.00294.x [Google Scholar]
- Festa-Bianchet M, Gaillard J.M, Jorgenson J.T. Mass- and density-dependent reproductive success and reproductive costs in a capital breeder. Am. Nat. 1998;152:367–379. doi: 10.1086/286175. doi:10.1086/286175 [DOI] [PubMed] [Google Scholar]
- Forchhammer M.C, Clutton-Brock T.H, Lindstrom J, Albon S.D. Climate and population density induce long-term cohort variation in a northern ungulate. J. Anim. Ecol. 2001;70:721–729. doi:10.1046/j.0021-8790.2001.00532.x [Google Scholar]
- Gammell M.P, De Vries H, Jennings D.J, Carlin C.M, Hayden T.J. David's score: a more appropriate dominance ranking method than Clutton-Brock's index. Anim. Behav. 2003;66:601–605. doi:10.1006/anbe.2003.2226 [Google Scholar]
- Garcia A.J, Landete-Castillejos T, Carrion D, Gaspar-Lopez E, Gallego L. Compensatory extension of gestation length with advance of conception in red deer (Cervus elaphus) J. Exp. Zool. Part A-Comp. Exp. Biol. A. 2006;305:55–61. doi: 10.1002/jez.a.244. doi:10.1002/jez.a.244 [DOI] [PubMed] [Google Scholar]
- Gross J.E, Demment M.W, Alkon P.U, Kotzman M. Feeding and chewing behaviours of nubian ibex—compensation for sex-related differences in body-size. Funct. Ecol. 1995;9:385–393. doi:10.2307/2390001 [Google Scholar]
- Hewison A.J.M, Gaillard J.M. Successful sons or advantaged daughters? The Trivers–Willard model and sex-biased maternal investment in ungulates. Trends Ecol. Evol. 1999;14:229–234. doi: 10.1016/s0169-5347(99)01592-x. doi:10.1016/S0169-5347(99)01592-X [DOI] [PubMed] [Google Scholar]
- Hewison A.J.M, Gaillard J.M, Kjellander P, Toigo C, Liberg O, Delorme D. Big mothers invest more in daughters: reversed sex allocation in a weakly polygynous mammal. Ecol. Lett. 2005;8:430–437. doi:10.1111/j.1461-0248.2005.00743.x [Google Scholar]
- Horvitz C.C, Schemske D.W. Spatiotemporal variation in demographic transitions of a tropical understory herb: projection matrix analysis. Ecol. Monogr. 1995;65:155–192. doi:10.2307/2937136 [Google Scholar]
- Jorgenson J.T, Festa-Bianchet M, Gaillard J.M, Wishart W.D. Effects of age, sex, disease, and density on survival of bighorn sheep. Ecology. 1997;78:1019–1032. doi:10.2307/2265855 [Google Scholar]
- Kruger O. Age at first breeding and fitness in goshawk Accipiter gentilis. J. Anim. Ecol. 2005;74:266–273. doi:10.1111/j.1365-2656.2005.00920.x [Google Scholar]
- Kruuk L.E.B, Clutton-Brock T.H, Albon S.D, Pemberton J.P, Guinness F.E. Population density affects sex ratio variation in red deer. Nature. 1999a;399:459–461. doi: 10.1038/20917. doi:10.1038/20917 [DOI] [PubMed] [Google Scholar]
- Kruuk L.E.B, Clutton-Brock T.H, Rose K.E, Guinness F.E. Early determinants of lifetime reproductive success differ between the sexes in red deer. Proc. R. Soc. B. 1999b;266:1655–1661. doi: 10.1098/rspb.1999.0828. doi:10.1098/rspb.1999.0828 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Kühl A, Mysterud A, Erdnenov G.I, Lushchekina A.A, Grachev I.A, Bekenov A.B, Milner-Gulland E.J. The ‘big spenders’ of the steppe: sex-specific maternal allocation and twinning in the saiga antelope. Proc. R. Soc. B. 2007;274:1293–1299. doi: 10.1098/rspb.2007.0038. doi:10.1098/rspb.2007.0038 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Lewis S, Wanless S, Elston D.A, Schultz M.D, Mackley E, Du Toit M, Underhill J.G, Harris M.P. Determinants of quality in a long-lived colonial species. J. Anim. Ecol. 2006;75:1304–1312. doi: 10.1111/j.1365-2656.2006.01152.x. doi:10.1111/j.1365-2656.2006.01152.x [DOI] [PubMed] [Google Scholar]
- Loison A, Solberg E.J, Yoccoz N.G, Langvatn R. Sex differences in the interplay of cohort and mother quality on body mass of red deer calves. Ecology. 2004;85:1992–2002. doi:10.1890/03-0600 [Google Scholar]
- Lummaa V. Early developmental conditions and reproductive success in humans: downstream effects of prenatal famine, birth weight, and timing of birth. Am. J. Hum. Biol. 2003;15:370–379. doi: 10.1002/ajhb.10155. doi:10.1002/ajhb.10155 [DOI] [PubMed] [Google Scholar]
- Lummaa V, Clutton-Brock T. Early development, survival and reproduction in humans. Trends Ecol. Evol. 2002;17:141–147. doi:10.1016/S0169-5347(01)02414-4 [Google Scholar]
- Metcalf C.J.E, Pavard S. Why evolutionary biologists should be demographers. Trends Ecol. Evol. 2007;22:205–212. doi: 10.1016/j.tree.2006.12.001. doi:10.1016/j.tree.2006.12.001 [DOI] [PubMed] [Google Scholar]
- Metz J.A.J, Nisbet R.M, Geritz S.A.H. How should we define fitness for general ecological scenarios. Trends Ecol. Evol. 1992;7:198–202. doi: 10.1016/0169-5347(92)90073-K. doi:10.1016/0169-5347(92)90073-K [DOI] [PubMed] [Google Scholar]
- McGraw J.B, Caswell H. Estimation of individual fitness from life-history data. Am. Nat. 1996;147:47–64. doi:10.1086/285839 [Google Scholar]
- McLoughlin P.D, Boyce M.S, Coulson T, Clutton-Brock T. Lifetime reproductive success and density-dependent, multi-variable resource selection. Proc. R. Soc. B. 2006;273:1449–1454. doi: 10.1098/rspb.2006.3486. doi:10.1098/rspb.2006.3486 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Mousseau T.A, Fox C.W. The adaptive significance of maternal effects. Trends Ecol. Evol. 1998;13:403–407. doi: 10.1016/s0169-5347(98)01472-4. doi:10.1016/S0169-5347(98)01472-4 [DOI] [PubMed] [Google Scholar]
- Moyes K, Coulson T, Morgan B.J.T, Donald A, Morris S.J, Clutton-Brock T.H. Cumulative reproduction and survival costs in female red deer. Oikos. 2006;115:241–252. doi:10.1111/j.2006.0030-1299.15200.x [Google Scholar]
- Moyes, K., Morgan, B. J. T., Donald, A., Morris, S. J. Clutton-Brock, T. H. & Coulson, T. Submitted. Exploring individual quality in a wild population of red deer. [DOI] [PubMed]
- Murray B.G. Population dynamics of evolutionary change: demographic parameters as indicators of fitness. Theor. Popul. Biol. 1997;51:180–184. doi: 10.1006/tpbi.1997.1313. doi:10.1006/tpbi.1997.1313 [DOI] [PubMed] [Google Scholar]
- Mylius S.D, Diekmann O. On evolutionarily stable life histories, optimization and the need to be specific about density dependence. Oikos. 1995;74:218–224. doi:10.2307/3545651 [Google Scholar]
- Nussey D.H, Clutton-Brock T.H, Elston D.A, Albon S.D, Kruuk L.E.B. Phenotypic plasticity in a maternal trait in red deer. J. Anim. Ecol. 2005;74:387–396. doi:10.1111/j.1365-2656.2005.00941.x [Google Scholar]
- Pelletier F, Reale D, Garant D, Coltman D.W, Festa-Bianchet M. Selection on heritable seasonal phenotypic plasticity of body mass. Evolution. 2007;61:1969–1979. doi: 10.1111/j.1558-5646.2007.00160.x. doi:10.1111/j.1558-5646.2007.00160.x [DOI] [PubMed] [Google Scholar]
- R Development Core Team. R Foundation for Statistical Computing; Vienna, Austria: 2007. R: a language and environment for statistical computing.http://www.R-project.org [Google Scholar]
- Rubenstein D.I. Individual variation and competition in the Everglades pygmy sunfish. J. Anim. Ecol. 1981;50:337–350. doi:10.2307/4059 [Google Scholar]
- Sims, M., Elston, D., Larkham, A., Nussey, D. H. & Albon, S. D. 2007 Identifying when weather influences life-history traits of grazing herbivores. J. Anim. Ecol.76, 761–770. [DOI] [PubMed]
- Stearns S.C. Oxford University Press; Oxford, UK: 1992. The evolution of life histories. [Google Scholar]
- Stewart K.M, Bowyer R.T, Dick B.L, Johnson B.K, Kie J.G. Density-dependent effects on physical condition and reproduction in North American elk: an experimental test. Oecologia. 2005;143:85–93. doi: 10.1007/s00442-004-1785-y. doi:10.1007/s00442-004-1785-y [DOI] [PubMed] [Google Scholar]
- Trivers R.L, Willard D.E. Natural-selection of parental ability to vary sex-ratio of offspring. Science. 1973;179:90–92. doi: 10.1126/science.179.4068.90. doi:10.1126/science.179.4068.90 [DOI] [PubMed] [Google Scholar]
- Van de Pol M, Verhulst S. Age-dependent traits: a new statistical model to separate within- and between-individual effects. Am. Nat. 2006;167:766–773. doi: 10.1086/503331. doi:10.1086/503331 [DOI] [PubMed] [Google Scholar]
- Van Noordwijk A.J, De Jong G. Acquisition and allocation of resources: their influence on variation in life-history tactics. Am. Nat. 1986;128:137–142. doi:10.1086/284547 [Google Scholar]
- Vaupel J.W, Manton K.G, Stallard E. Impact of heterogeneity in individual frailty on the dynamics of mortality. Demography. 1979;16:439–454. doi:10.2307/2061224 [PubMed] [Google Scholar]
- Wilson A.J, Pilkington J.G, Pemberton J.M, Coltman D.W, Overall A.D.J, Byrne K, Kruuk L.E.B. Selection on mothers and offspring: whose phenotype is it and does it matter? Evolution. 2005;59:451–463. doi:10.1554/04-480 [PubMed] [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.
Supplementary Materials
AIC values from the linear mixed effects models of offspring birth traits reported in table 1. Each sub-table gives the AIC value for (i) the model containing all three measures of female quality and population density but no interactions, (ii) the model containing all three measures of female quality and their interactions with density (the full model), and (iii) the minimum adequate model for which the effect sizes are reported in table 1. Results are for models of (a) male offspring birth mass, (b) female offspring birth mass, (c) male offspring birth date and (d) female offspring birth date, as above.