Aphid aerial density profiles are consistent with turbulent advection amplifying flight behaviours: abandoning the epithet ‘passive’

Abstract

Seminal field studies led by C. G. Johnson in the 1940s and 1950s showed that aphid aerial density diminishes with height above the ground such that the linear regression coefficient, b, of log density on log height provides a single-parameter characterization of the vertical density profile. This coefficient decreases with increasing atmospheric stability, ranging from −0.27 for a fully convective boundary layer to −2.01 for a stable boundary layer. We combined a well-established Lagrangian stochastic model of atmospheric dispersal with simple models of aphid behaviour in order to account for the range of aerial density profiles. We show that these density distributions are consistent with the aphids producing just enough lift to become neutrally buoyant when they are in updraughts and ceasing to produce lift when they are in downdraughts. This active flight behaviour in a weak flier is thus distinctly different from the aerial dispersal of seeds and wingless arthropods, which is passive once these organisms have launched into the air. The novel findings from the model indicate that the epithet ‘passive’ often applied to the windborne migration of small winged insects is misleading and should be abandoned. The implications for the distances traversed by migrating aphids under various boundary-layer conditions are outlined.

1. Introduction

Except in the Earth's coldest regions (and seasons), there is an enormous daily flux of terrestrial arthropods through the atmosphere. One immediately thinks of winged insects as comprising this aerial ‘bioflow’ (Johnson 1969; Isard & Gage 2001; Chapman et al. 2004), but large numbers of small wingless arthropods also have a migratory phase in which they take to the air and are transported by the wind. Wingless arthropods engage in specialized behaviours to get themselves airborne, e.g. the pre-ballooning behaviours of spiders and other airborne migrants that use silk draglines (Bell et al. 2005), or the rearing up body stance of aerially dispersed mites (Acari; Johnson & Croft 1976; Smitley & Kennedy 1985; Frost 1997) and scale insect (Coccoidea) ‘crawlers’ (Washburn & Washburn 1984). So the take-off or launch phase is largely under the control of the individual, and even small animals usually enter the airstream as part of an active behavioural process, rather than being carried away inadvertently or accidentally. Even some plants and fungi have ballistic systems that are adapted to launch their wind-dispersed seed or spores only under particular weather conditions (Pedgley 1982), and these can be considered analogous to the take-off behaviours of animals (Dingle 1996). Consequently, the terms ‘passive’ and ‘active’ to categorize the dispersing organism can only really be applied after it has become airborne.

There will be a spectrum of passivity with, at one extreme, minute wingless arthropods such as the mites and scale insect crawlers mentioned above, which, like pollen grains, spores and seeds, are not able to control their movements in the air. Even here there is the possibility that a wingless arthropod is able to change its fall speed by, say, extending or drawing in its legs (Washburn & Washburn 1984; Jung & Croft 2001). Also, there has been speculation that spiders may be able to influence the time spent aloft by paying out or drawing in their silken lines. For example, nearing the end of their sorties, wolf spiders have been observed to accumulate a ‘…small white flossy ball…’ as if they were drawing in silk to control drag and therefore the timing of their descent (McCook 1877). Next in the spectrum of passivity, come weakly flying insects that are largely at the mercy of the wind, but are able to exert a degree of control over whether they remain in the air by choosing to beat their wings, keep them extended without beating, or to close them (Thomas et al. 1977). Lastly, there are large insects, bats and birds with relatively good control over their movements. Atmospheric motions can still have a strong effect as shown by the concentrations of insects that appear as line echoes, cellular patterns, etc. on entomological radars (e.g. Schaefer 1976; Drake & Farrow 1988). In this paper, we develop a model that accounts for the vertical distribution of migrating aphids (Order Homoptera: Family Aphididae) in the atmosphere. The model indicates that aphids and possibly other small winged insects, although weakly flying, are clearly different from ballooning spiders and lepidopteran larvae that use secreted strands of silk (draglines) as a kind of parachute for passive dispersal on the wind (Bell et al. 2005), and from the passive dispersal of windborne seeds (Nathan et al. 2005).

In the late 1940s and 1950s, C. G. Johnson and his collaborators undertook a series of detailed studies of the airborne migration of aphids over flat arable land in southern England, using a variety of sampling methods including traps attached to a balloon tethering cable (Johnson 1969). These studies were exceptional in that they employed simultaneous sampling, at frequent intervals, over most of the flight heights (not just near the ground) and there was no doubt about the identity of the insects being sampled. One of the key findings was that, above a zone approximately 10 m deep close to the ground, the density of aphids diminishes with height such that the linear regression coefficient, b, of log density on log height provides a single-parameter characterization of the vertical density profile (Johnson & Penman 1951; Johnson 1957). This empirical relationship was later found to fit the density profiles of a variety of other small insects (Johnson 1969; Taylor 1974; and see figure 1). The regression is inversely correlated with the mean lapse rate, an indicator of atmospheric stability.

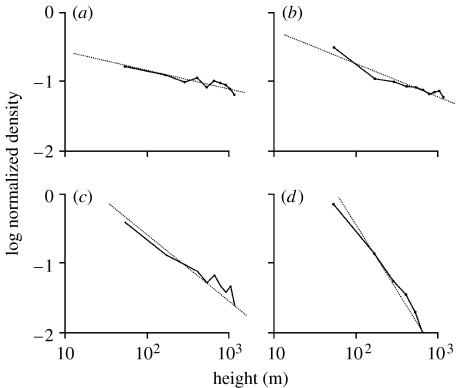
Figure 1.

Examples of hourly profiles of log aphid density on log height for 8 August 1955 (adapted from Johnson 1957). The trapping heights were 3, 6, 15, 76 and 305 m above ground. The density of aphids (numbers per 10⁶ m³ of air) diminishes with height such that the linear regression coefficient, b, of log density on log height provides a single-parameter characterization of the vertical density profile. The dashed lines are least-squares fits to the simulation data above 10 m with linear regression, b. The coefficient b has the following values: (a) b=−0.61 at 08.00 (r²=0.93), (b) b=−0.72 at 10.00, (c) b=−0.74 at 12.00, (d) b=−1.24 at 14.00, (e) b=−1.32 at 16.00, and (f) b=−1.88 at 18.00 (r²=0.98). The atmosphere was unstable up to approximately 15.00, the surface temperate at the monitoring site (Cardington, UK) increasing from 15°C at 09.00 to 20.6°C at 14.00. At approximately 15.00 however, a steady cooling of the atmosphere began (Johnson 1957).

Johnson & Penman (1951) proposed that this density/height relationship stems from a balance between turbulent transport and a ‘mean clearance rate’ (the rate at which aphids return to the earth). Underlying this is the notion that once airborne, the net effect of upward and downward transport of aphids made it seem as if they ‘obey the laws of turbulent diffusion applicable to inert particles’ (Johnson 1969). Kennedy & Fosbrooke (1973), keen to emphasize the behavioural component of aphid migration, suggested that the migrants' lift was unlikely to be constant, and that atmospheric turbulence amplifies rather than nullifies the aphids' own movements. That is, aphids fly upwards in updraughts and fly downwards or free-fall in downdraughts. Recently Geerts & Miao (2005), using the data from an airborne Doppler radar, have proposed, somewhat counter-intuitively, that small insects (less than 10 mg) actively oppose being taken up in convective plumes, and furthermore that this opposition increases with updraught strength. However, some of the insect targets observed by Geerts and Miao seem to have been quite a bit larger than common migrant aphids that weigh approximately 0.5 mg. With the advent of Lagrangian stochastic (LS) models, it has now become possible to test these different possibilities in numerical simulations. By taking explicit account of flow inhomogeneities and non-Gaussian velocity statistics, these models can predict accurately the dispersion within the atmospheric boundary layer (Thomson 1989).

Here, such a ‘mechanistic’ model is used together with simplified models of aphid flight behaviour to predict the densities of migratory aphids in atmospheric boundary layers with stabilities ranging from almost purely convective (no wind shear) to strongly stable (Pasquill 1974). We show that observed densities are consistent with the aphids producing just enough lift to counterbalance gravity and become neutrally buoyant when they are in updraughts and with their ceasing to produce lift when they are in downdraughts. This finding supports the suggestion made by Kennedy & Fosbrooke (1973) and has important ramifications for the modelling and prediction of the migration of aphids under different atmospheric conditions.

2. Simulation model

Underlying the modelling approach are four apparently realistic assumptions: (i) passive advection by the wind makes the dominant contribution to aphid dispersal in the downwind direction, (ii) that vertical movements arise from the combined effects of passive advection by the wind and the aphid's own movements, (iii) aphids at an early stage of their migration are not responding to visual cues that can initiate landing, and (iv) aphids arriving at the ground either refrain from landing or, more realistically (Kennedy & Fosbrooke 1973), land and take-off again. Assumptions (iii) and (iv) are necessary for the maintenance of airborne counts and the establishment of equilibrium aerial density profiles that can be compared with the observations of Johnson & Penman (1951) and Johnson (1957, 1969).

Passive dispersal within complex non-Gaussian, turbulent flows such as the atmospheric boundary layer is best predicted by LS models because other approaches such as diffusion models or similarity scaling are either inappropriate or invalid (Thomson 1989). LS models for the evolution of the velocity (u) and position (x) of a passive body in atmospheric turbulence take the general form,

$$\begin{array}{c}\text{d}{u}_i=a_i(\mathit{x},\mathit{u},t)\text{d}t+\sqrt{C_0ϵ}\text{d}{\xi }_i,\\ \text{d}\mathit{x}=\mathit{u}\text{d}t,\end{array}$$ (2.1)

where bold-italic quantities denote the vectors; the subscripts denote the Cartesian components; C₀=5 is the Kolmogorov's Lagrangian velocity structure constant; t is the time; and ϵ is the mean rate of dissipation of turbulent kinetic energy divided by the density of air (Thomson 1989). The quantities dξ are increments of a Wiener (white noise) process and have mean zero and variance equal to the time increment, dt. The model is, by construction, compatible with Kolmogorov similarity theory, a scaling widely observed in atmospheric flows. The function a_i(x, u, t) can be constrained, but in general not uniquely determined by invoking Thomson's (1989) well-mixed condition. Mathematically, this requires that the function a_i(x, u, t) be a solution of the Fokker–Planck equation (Thomson 1989). The well-mixed condition currently constitutes the most rigorously correct theoretical framework for the formulation of LS models such as equation (2.1). It guarantees that the velocity statistics of simulated dispersing bodies are compatible with prescribed Eulerian (fixed point) velocity statistics that characterize the turbulent flow and which are used as model inputs.

By invoking the well-mixed condition, Rotach et al. (1996) formulated a two-dimensional LS model for the simulation of passive body dispersal in atmospheric boundary layers over flat terrain with stabilities ranging from ideally neutral to fully convective. This approach is more economical than a full three-dimensional model and is appropriate when, as in the current application, we are predicting dispersal in airflows whose statistical properties are homogeneous in the crosswind (y) direction. The correlation between turbulent fluctuations in velocity in both the horizontal, x, and vertical, z, directions due to the presence of coherent flow structures is accounted for, as is the skewness of the turbulent velocity distribution. Model predictions are in good agreement with the data collected in laboratory-scale experiments and in the field (Willis & Deardorff 1976; Gryning & Lyck 1984). A detailed description of this now well-established LS model, its numerical implementation and the accompanying parametrization of required meteorological inputs can be found in Rotach et al. (1996). Accompanying flow parametrizations for stable boundary layers can be found in Wilson (2000). This wholesale adoption of standard parametrizations precludes any possibility of tuning the model to obtain ‘desirable’ aphid density profiles, and provides a reliable and well-understood platform for examining candidate active behaviours in aphids. Here we adopt the model (2.1) for simulating aphid dispersal in an atmospheric boundary layer. To do this, we simply modify (2.1) so that the increments in position become,

$$\frac{\text{d}x}{\text{d}t}=u\text{and}\frac{\text{d}z}{\text{d}t}=w+w_{\text{A}},$$ (2.2)

where (u, w) are the horizontal and velocity components of the air velocity at the position (x, z) of the aphid and w_A is the velocity of the aphid due to upward flight and settlement under gravity. Drag, which causes a delayed response to changes in air velocity, is ignored because the durations of these accelerations are short in comparison with the turbulent integral time scales characterizing changes in air velocity. Model predictions of aphid density profiles obtained using equations (2.1) and (2.2) do not differ significantly from those obtained using a more elaborate approach that takes explicit account of the effects of inertia (Boehm & Aylor 2005).

The model was used to examine how flight behaviours affect the predictions of insect density profiles in atmospheric boundary layers with stabilities ranging from purely convective to strongly stable. Several plausible active flight behaviours, together with a passive response by the aphids, were assessed for consistency with the observed density profiles (Johnson & Penman 1951; Johnson 1957).

In the numerical simulations, aphids are released from a height of 100 m (this height value is not a critical parameter because equilibrium aerial density profiles that form after 60 min are not influenced by initial conditions), and their subsequent trajectories within a boundary layer having a height 1000 m are then simulated by numerically integrating equations (2.1) and (2.2). The time step of integration was

$$\text{d}t=0.01\text{min}\left(T,\frac{{\sigma }_{\text{u}}}{\left|a\right|},\frac{{\sigma }_{\text{w}}}{\left|a\right|},\frac{{\sigma }_{\text{u}}}{\left|w\frac{\text{d}{\sigma }_{\text{u}}}{\text{d}z}\right|}\frac{{\sigma }_{\text{w}}}{\left|w\frac{\text{d}{\sigma }_{\text{w}}}{\text{d}z}\right|},\frac{ϵ}{\left|w\frac{\text{d}ϵ}{\text{d}z}\right|}\right),$$ (2.3)

where $T=2{\sigma }_{\text{w}}^2/C_0ϵ$ is a key turbulence de-correlation time scale and where ${\sigma }_{\text{u}}^2$ and ${\sigma }_{\text{w}}^2$ are the mean-square variations in the streamwise and vertical components of wind velocity. This choice for the time step of integration guarantees that spatial variations in the values of all model inputs that occur along the trajectory of a simulated aphid are accurately resolved. This in turn ensures that the numerical implementation of the stochastic model (2.1) satisfies the well-mixed condition. Time steps are typically 1 s long. Equilibrium (time-independent) profiles of aphid density are formed after aphids have been dispersing for 60 or more minutes. This time span is less than the typical duration during which many migratory aphids (e.g. autumn migrants of Rhopalosiphum padi: Nottingham et al. 1991) are unresponsive to visual cues that solicit landing behaviours. Predicted density profiles were obtained using 10 000 aphids and did not change significantly when 100 000 aphids were used. Model predictions of the density profiles are not sensitively dependent upon the boundary-layer height and do not change significantly when the fall speed is increased or decreased by a factor of 2.

3. Results and discussion

We begin by showing that observed aphid density profiles (Johnson & Penman 1951; Johnson 1957) can be reproduced in the numerical simulations if aphids produce just enough lift to become neutrally buoyant when they are in updraughts and cease producing lift when they are in downdraughts, i.e. w_A=0 when w>0 m s⁻¹ and w_A=−w_fall when w≤0 m s⁻¹. Model predictions do not change significantly if the aphids are assumed to fly upwards at an airspeed of 0.2 m s⁻¹ when flying in updraughts. Such migratory climb rates are close to the maximum rates that have been observed in laboratory flight chambers (David & Hardie 1988; Nottingham et al. 1991). Here, following Thomas et al. (1977) who reported that in the absence of wingbeating, the fall speeds of Aphis fabae are approximately 0.8 m s⁻¹ (with wings extended) to 1.8 m s⁻¹ (with wings closed), we have taken the fall speed of aphids to be w_fall=1 m s⁻¹.

Predictions of the density of aphids in atmospheric boundary layers with stabilities ranging from almost purely convective to strongly stable are shown in figure 2. In accordance with the observations (Johnson & Penman 1951; Johnson 1957), the model predicts that aphid densities diminish with height such that the linear regression coefficient, b, of log density on log height is an approximate expression of the vertical density profile. The model also predicts that the regression coefficient decreases with increasing atmospheric stability, ranging from −0.25 for a fully convective boundary layer to −2.0 for a stable boundary layer. This is in strikingly close agreement with the observations of Johnson (1957) who reported that the regression coefficient ranged from b=−0.27 (in summer) to −2.01 (in late autumn), and with the near-ground observations (less than 32 m) of Taylor (1974) who reported that airborne counts of aphids in June were characterized by b=−0.425. The dependency of aerial density upon atmospheric stability stems directly from the mixing efficiency of the atmosphere. In convective boundary layers, rising and sinking plumes of air promote large-scale mixing and as a result aphids soon become distributed throughout the boundary layer. In stable boundary layers, aphids are mostly confined to fly just a few tens of metres above the ground (Johnson 1969) or are confined by stable or neutral layers aloft (Isard et al. 1990). If the aphids were passively advected by turbulence, then the model predicts that aphids cannot remain aloft within a stable boundary layer but instead will fall out with a speed close to their fall speed in still air. This prediction is consistent with the scarcity of ‘ballooning’ spiders in stable nocturnal boundary layers (Glick 1939).

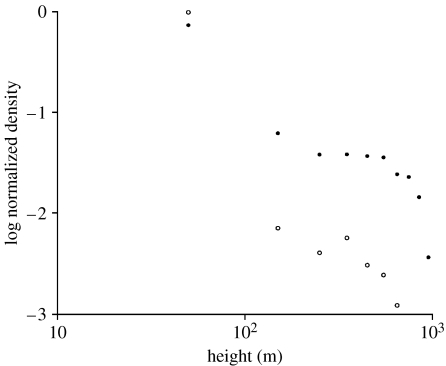
Figure 2.

Predictions of the normalized densities of airborne aphids in (a) a convective boundary layer with wind shear (u_*=0.3 m s⁻¹ and w_*=2.0 m s⁻¹), (b) a boundary layer with intermediate stability (u_*=0.4 m s⁻¹ and w_*=1.0 m s⁻¹), (c) a boundary layer with neutral stability (u_*=0.8 m s⁻¹ and w_*=0.5 m s⁻¹) and (d) a stable boundary layer (u_*=0.5 m s⁻¹ and w_*=0 m s⁻¹). The simulation data are indicated by the symbols and the solid lines are added to guide the eye. The dashed lines are least-squares fits to the simulation data with linear regression (a) b=−0.25, (b) b=−0.5, (c) b=−1.0 and (d) b=−2.0. The friction velocity u_* is just the square root of the surface stress divided by the density of the air. The convective velocity scale w_*=(−H_*Z)^1/3, and consequently w_*=1 m s⁻¹ corresponds to a small surface heat flux, H_*, of approximately 30 J m⁻² s⁻¹ (buoyancy flux −10³ m² s⁻³).

The close correspondence between observed and predicted aphid densities suggests that atmospheric turbulence serves to amplify rather than ‘dampen’ the aphid's own movements, as suggested by Kennedy & Fosbrooke (1973). Our finding is entirely novel and indicates that the description ‘passive’ frequently applied to the windborne migration of small winged insects is misleading and should be abandoned.

Height–density profiles extending to high altitudes are also available for the day-flying Oscinella frit (Diptera: Chloropidae; Johnson et al. 1962; Johnson 1969) and these may adopt aerial migration behaviours that are similar to those of aphids. Estimates for frit fly regression coefficients can be extracted from plots of observed mean hourly density profiles of late summer catches that are presented in Johnson et al. (1962). These estimates range from a b value of approximately−0.37 for midday profiles to approximately −2.3 for late afternoon, and are consistent with the model predictions.

We carried out simulations on two other possible active behavioural scenarios but could not produce the observed density profiles, and this null result provides further support for flight amplifying behaviours in aphids and in frit flies during updraughts. These simulations embraced the possibility that (i) aphids are continuously generating lift that just counterbalances their fall speed and (ii) aphids take off at random times, ascend continually for a time and then cease flapping unless they come into close proximity with the ground whereupon they either land or repeat the cycle. In the former case, the aphids are effectively neutrally buoyant and so behave as marked fluid particles, whose equilibrium (well-mixed) density does not vary with height (Thomson 1989). In the latter case, predicted aerial densities are at variance with observations (Johnson & Penman 1951; Johnson 1957) because the logarithm of density does not diminish linearly with the logarithm of height (figure 3 and caption). We also found that observed aphid density profiles are not reproduced by state-of-the-art models of spider ballooning (Reynolds et al. 2007) or seed dispersal (Nathan et al. 2002, 2005) in which organisms are initially taken aloft in updraughts and are then carried along ‘passively’ by turbulent air currents while gradually settling under the action of gravity. The dispersal of seeds is therefore quite distinct from that of aphids—the latter can not only control when they take off and, to a degree, where they land, but they (as we have shown) can influence their distribution in the air. The present contribution is thus concerned with bridging the division between the research into the passive dispersal of spores, seeds and wingless arthropods and that into the dispersal of large insects, bats and birds that have a much higher degree of control over their movements.

Figure 3.

Predictions of the normalized densities of airborne aphids in a convective boundary layer with wind shear (u_*=0.3 m s⁻¹ and w_*=2.0 m s⁻¹) (filled circles) and boundary layer with intermediate stability (u_*=0.4 m s⁻¹ and w_*=1.0 m s⁻¹) (open circles). Aphids are assumed to take off at random times, ascend continually to an altitude of 100 m and then cease flapping unless they come within 10 m of the ground whereupon they ascend once again to an altitude of 100 m and the cycle repeats. The aphids have a fall speed of −1 m s⁻¹ in still air and most aphids complete several cycles within 1 hour. This model plainly does not fit the log density/log height relationship found in the observed aphid density profiles.

This advancement still leaves the major challenge of determining the balance between atmospheric physics and migration behaviour in determining the regression coefficient, b, for other migratory insects. Taylor's (1974) accurately measured profiles of insect densities of various taxa (albeit only up to a height of 32 m) are particularly interesting in this respect. Taylor (1974) found that above the ‘flight boundary layer’ (a layer of air in which the insect's self-propelled flight speed exceeds the wind speed), small night-flying insects generally have regressions with large negative coefficients (indicating relatively more of the population at lower altitudes); next come various crepuscular species and then mainly day-flying insects. These observations are broadly consistent with the aphid dispersal model which predicts that the regression (i.e. the value of b) decreases with increasing atmospheric stability, although there will no doubt be exceptions to these trends. Taylor (1974) also found that the regression coefficients for Homoptera (mainly aphids) were plainly different from other major taxa such as Diptera and Coleoptera measured over the same period. This clearly indicates that flight behaviours are specific to species or small groups of species.

We now turn to the distances moved by insects under various conditions of the daytime boundary layer. After 1 hour of actively responding to air currents, aphids aloft within a convective boundary layer (u_*=0.3 m s⁻¹ and w_*=2.0 m s⁻¹) are predicted to have travelled approximately 6 km downwind while those aloft within a boundary layer of neutral stability (u_*=0.8 m s⁻¹ and w_*=0.5 m s⁻¹) are predicted to have travelled approximately 30 km. Once they cease flying, most aphids are predicted to fall out of the atmosphere with a speed close to their fall speed in still air (figure 4). It is important to note, however, that fallout is something of a lottery because their dispersal is determined both by the mean flow (leading to a mean displacement of the population) and the turbulence (leading to dispersal). According to the model, some non-flying aphids can remain airborne for exceedingly long times as a result of being intermittently transported upwards over hundreds of metres through a boundary layer, carried along by occasionally occurring turbulent flow structures. For instance, most aphids that cease flying when at a height of 100 m are predicted to descend through a convective boundary layer with a speed close to their fall speed in still air (1 m s⁻¹) and reach the ground in approximately 100 s. Despite this, 16 per cent of the aphids are predicted to remain airborne for more than 1000 s, and 0.1 per cent of the aphids are predicted to be still airborne even after 10 000 s (approx. 3 hours; figure 4).

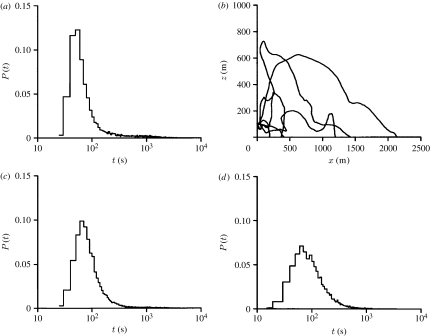
Figure 4.

Predicted distribution of fallout times, P(t), for aphids that cease flying when at a height of 100 m and within (a) a convective boundary layer with wind shear (u_*=0.3 m s⁻¹ and w_*=2.0 m s⁻¹), (c) a boundary layer of intermediate stability (u_*=0.4 m s⁻¹ and w_*=1.0 m s⁻¹) and (d) a boundary layer of neutral stability (u_*=0.8 m s⁻¹ and w_*=0.5 m s⁻¹). The mean fallout times are (a) $\overline{t}=467\text{s}$, (c) $\overline{t}=302\text{s}$ and (d) $\overline{t}=202\text{s}$; the mean distances travelled downwind are (a) $\overline{x}=0.8\text{km}$, (c) $\overline{x}=1.1\text{km}$ and (d) $\overline{x}=1.7\text{km}$ and the maximum distance travelled are (a) x_max = 15.7 km, (c) x_max = 25.9 km and (d) x_max=38.5 km. (b) Example of predicted trajectories of passively advected non-flying aphids in a convective boundary layer with wind shear.

The results of the numerical simulations may have important ramifications for the prediction and understanding of outbreaks of aphid infestations or of aphid-vectored plant diseases (Reynolds et al. 2006), and warrants further investigation. The model could, for instance, be used to relate the numbers of airborne aphids caught by traps on different days, under different atmospheric conditions, to aphid densities available to colonize crops at local and regional scales.