Economics, cultural transmission, and the dynamics of the sex ratio at birth in China

Abstract

In rural China, the ratio of newborn boys to newborn girls [sex ratio at birth (SRB)] has been rising for several decades, to values significantly above its biological norm. This trend has a number of alarming societal consequences, and has attracted the attention of scholars and politicians. The root of the problem lies in a 2,500-year-old culture of son preference. This culture is intricately linked with the economic reality of each couple's life, so that there are financial and psychological repercussions to parents who have no sons. To bring greater clarity and understanding to this issue, we present a quantitative framework that describes the interaction between economics and cultural transmission. We start with an explicit mechanism by which economic incentives can change cultural beliefs of a given individual, and go on to include a mechanism of cultural inheritance from generation to generation. We then show how economic conditions can affect the dynamics of cultural change in an entire society, and may lead to a decrease in the country's sex ratio at birth.

In most human populations, sex ratio at birth (SRB) (the ratio of newborn boys per newborn girls) is close to 1.05. SRB may vary with the number of children per birth, paternal age (1), and even season (2). Today, however, the most significant deviation of SRB from its biological value is due to a combination of socioeconomic factors in a number of countries of South and East Asia, including India and the People's Republic of China (3–6). The chief factor responsible for this deviation is a strong preference for sons (7–10). Although part of this preference may be due to economic necessity, for instance in rural areas where the need for manual labor is great, it is also traceable to deeply rooted practices and cultural beliefs (11). Examples are the continuation of the family lineage, restriction of certain funeral practices to sons of the deceased, and the widespread practice of virilocal marriage (in which a bride goes to live in her husband's household) (12, 13). Due to this preference for sons, many couples in South and East Asia face a difficult choice: either to risk having no sons and lose face in front of their friends and family, or to take deliberate steps to ensure that at least one of their children is male. Many couples make the second choice by means of sex-selective abortions and infanticide, thus contributing to the abnormally high ratio of male to female children in their countries (14–16).

In China, the problem has been exacerbated by two additional factors: availability of ultrasound technology that allows the parents to determine the sex of a fetus (although this technology is illegal for sex determination, its use for this purpose is widespread) (17, 18), and governmental policies that have significantly reduced fertility (10, 19, 20). These factors contribute to an increasing SRB, and make modification of the culture of son preference all of the more important (21–24). China's government has been working to solve this problem in several ways: for instance, by discouraging the illegal use of ultrasound technologies, by providing financial incentives to couples that give birth to girls, or by directly facilitating cultural change away from son preference (12, 22). Here, we aim to model the relationship between cultural transmission and economic incentives to suggest conditions under which each of the above methods might be effective.

In models of transmission of son preference, a certain percentage of women choose to pursue sex selection to ensure that at least one of their children is male (25, 26). In the language of Li and colleagues (26), these women have cultural value π. The remaining women do not carry out sex selection (they have cultural value π₀). Thus, π and π₀ correspond to the behavior of women with respect to sex selection.

Li et al. (26) suggest that if the transmission of π₀ from parents to children or via the media is sufficiently strong, the extent of son preference might eventually decrease. If this occurred, then the strongly male-biased sex ratio in some Asian countries would tend back to normal. However, the model of Li and colleagues (26) does not incorporate the difference in economic value between sons and daughters, and thus does not address how fiscal incentives might affect the dynamics of cultural change.

Explicit economic values assigned to daughters and sons form the basis of the framework developed by Bhattacharjya et al. (27). However, this framework does not allow for cultural change that can alter these values. Rather, it describes the state of economic equilibrium to which the system tends under static conditions.

In this article, we propose a quantitative framework for the influence of financial incentives on cultural transmission of son preference. We discuss the application of this model to socioeconomic dynamics of sex ratio in China, and the conditions under which it reduces to a purely economic model (27).

Results

Cultural and Economic Value: σ and m.

As son preference can be traced to both cultural beliefs and economic necessity, we partition the value of a child into two separate components: economic and cultural. Economic value is that which is readily translatable into money. It includes the value of the child's manual labor over his or her lifetime, the support of parents in their old age, and any governmental subsidies the parents would receive toward raising the child.

Cultural value, in contrast, cannot be immediately converted into money and is dependent on the parents' cultural beliefs. It includes the value of continuing the family's surname in future generations, and the value of knowing that the family's ancestors will be remembered for years to come. We assume that a mother can translate the cultural value of her child into the currency of economic value and that shortly after its birth she accurately assesses the child's total value—cultural and economic combined.

We use σ and m to denote cultural and economic value, respectively, and measure the two types of value in the same monetary units. We also assume that cultural value (σ) is entirely marital—in other words, it is realized only if the child finds himself or herself a spouse. Conversely, economic value (m) is assumed to be completely non-marital, i.e., not contingent on his or her marital success. So far, all of the examples of economic and cultural value we have given fit these criteria. For a discussion of marital and non-marital value, please see SI Appendix, section A.

Let the cultural value of a married son be σ_s. Because cultural value is completely marital, for an unmarried son σ_s = 0. We assume that the ratio of males to females at birth (r) is >1, and equal to the male/female ratio at marriageable age. In other words, we ignore sex-differential mortality before marriage. We also assume that all marriages are monogamous, and that the marriage market is panmictic, so that every woman finds herself a husband, but a number of men do not find themselves wives. In this environment, the probability that a son gets married is 1/r. It follows that the expected amount of cultural value a son brings to his household is σ_s/r. This amount would be different if we had assumed a non-panmictic marriage market, but that would not change any of our qualitative results (SI Appendix, section A).

We assume that for mothers who do not follow the sex selection strategy, the cultural value of married sons is equal to that of married daughters: σ_s = σ_d = σ₀. For those who do follow the sex selection strategy, σ_s = σ₁, where σ₁ > σ_d = σ₀. The probability that a daughter gets married in a panmictic marriage market is equal to 1. Consequently, the average amount of cultural value she will bring to her household is equal to σ₀.

The expected difference between the cultural values of sons and daughters to their mothers is Δσ, where

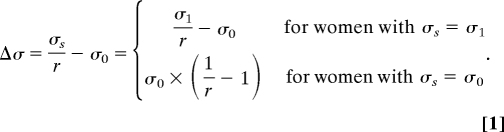Note that σ₁ and σ₀ are constants, but the proportion of women with σ_s = σ₁ can vary in time. For women with σ_s = σ₀, Δσ ≤ 0, and approaches zero as r approaches 1. This follows basic intuition: when r = 1 (i.e., sons and daughters are equally likely to marry), and a given woman believes that the cultural value of a married son is equal to that of a married daughter, we expect her difference in cultural value between sons and daughters to be zero. For women with σ_s = σ₁, the expected difference in cultural value can be either positive or negative, depending on the values of σ₁ and r.

The economic value of sons, m_s, does not depend on cultural beliefs of individuals. Instead, m_s is determined by society-wide economic factors. As a result, its value is homogeneous in the society at any given time. Thus, we can define Δm—a society-wide difference between the economic value of sons and that of daughters:

where m_d is the economic value of daughters. Because we assume that economic value is entirely non-marital, neither m_s nor m_d need to be discounted by the sex ratio.

Finally, we define total value difference (TVD) as the sum of Δσ and Δm:

Prejudice Toward Son Preference: ρ.

A mother's behavior with respect to sex selection is correlated with her σ_s—the cultural value she places on sons. We now introduce ρ—a separate, inherited marker of prejudice toward son preference. Unlike σ_s, ρ does not determine the mother's behavior directly.

After the birth of her children, a mother assesses the economic reality in the surrounding society, combines that with her own cultural beliefs, and evaluates the TVD between a son and a daughter (Eq. 3). If TVD is greater than some threshold, she forms prejudice toward son preference (ρ = 1). If it is less than that threshold, her prejudice will be against son preference (ρ = 0). We normalize Δm in such a way that this threshold value is zero:

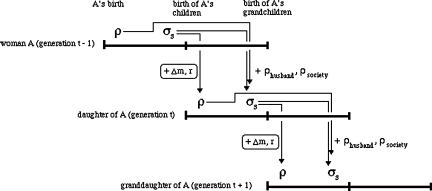
Every woman holds values for σ_s and ρ, and every man—a value for ρ. Fig. 1 depicts the full inheritance scheme for σ_s and ρ. We can use it to trace one path in the inheritance process through 3 generations, starting with σ_s of woman A. The σ_s of A is translated to her daughter's ρ via TVD during the early stage of the daughter's life, when much of her learning is intuitive and direct (28). During a later, conceptual learning stage, the σ_s that the daughter of Alearned from her mother is modified according to her own ρ, her husband's ρ, and the societal ρ (i.e., prevalence of ρ = 1 in the entire parental generation). She then transfers this modified σ_s to her own daughter–the grandmother of A.

Fig. 1.

Inheritance scheme for σ_s and ρ. Soon after the children in generation t are born, their mothers form a prejudice (ρ) from a combination of their cultural beliefs (their σ_s values) and the socioeconomic environment (Δm and r). The mothers then transfer the value of ρ to their children while the children are still young. Later, when the children are older, their mothers teach them their cultural beliefs explicitly. In the process, these beliefs will sometimes be modified in line with the mother's and father's ρ.

Translation from σ_s to ρ.

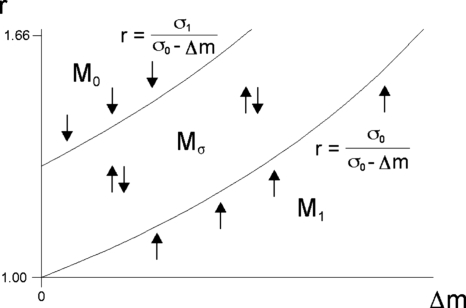
TVD can take on one of two possible values: one for σ_s = σ₁, and one for σ_s = σ₀ (see equations 1 and 3). Consequently, there are three possible modes of translation from σ_s to ρ via Eqs. 3 and 4. When r, the sex ratio, is close to 1, both possible values of TVD are greater than zero, so that all children receive ρ = 1 (Eqs. 3 and 4). We call this mode M₁. When r is large, many men cannot find wives, so that both values of TVD are less than zero, and all children receive ρ = 0. This is mode M₀. Finally, in mode M_σ, TVD ≥0 for mothers with σ_s = σ₁ and TVD <0 for those with σ_s = σ₀, so that the cultural belief of a mother is fully correlated with the prejudice value inherited by her child.

To obtain equations for the boundaries between the three modes of translation, we let TVD = 0 with σ_s = σ₀ and σ_s = σ₁:

and

Values of r less than the right hand side of Eq. 5 correspond to mode M₁, values of r between the lines in equations 5 and 6 correspond to M_σ, and those greater than the right hand side of Eq. 6 to M₀ (Fig. 2).

Fig. 2.

Summary of model dynamics. In mode M₁, all children receive ρ = 1, regardless of their mothers' cultural beliefs. Thus, in M₁, sex ratio r always increases. In mode M₀, all children receive ρ = 0, and r always decreases. In M_σ, a child's ρ follows his/her mother's cultural belief σ_s. In this mode, direction and rate of change in r is determined by the effect of parental and societal intuitive preferences (ρ) on the transmission of cultural belief (σ_s), i.e., parameters C₀₁, C₁₀, V₀₁ and V₁₀.

Dynamic Variables.

We study the dynamics of change in r for a given value of Δm. To do so, we define variables x₁₀, x₀₁, x₀₀, x₁₁, y₀, y₁, and z₁. The first 6 variables keep track of the proportions of women and men with different combinations of cultural beliefs (σ_s = σ₀ or σ_s = σ₁) and prejudice values (ρ = 0 or ρ = 1) (see Table 1). z₁ is the overall proportion of people that have ρ = 1:

Table 1.

Temporal variables^a that keep track of individuals with different combinations of σ_s and ρ

Variable	Gender	ρ	σs
x11	♀	1	σ1
x10		1	σ0
x01		0	σ1
x00		0	σ0
y1	♂	1	—
y0		0	—
z1	Both	1	—

^aSee text for variable definitions.

Cultural Conversion.

A mother's σ_s can be modified to follow with her own, her husband's, and the societal ρ during transmission to her daughter (Fig. 1). The probability of such conversion is determined by parameters C₀₁, C₁₀, V₀₁ and V₁₀. V₀₁ and V₁₀ come into play in families where the prejudice of both parents is in conflict with the mother's behavior. For instance, in a family where ρ = 1 for both parents, and the mother's σ_s = σ₀, the σ_s she transfers to her daughter will be modified to σ₁ with probability V₀₁. Conversely, in a family where both parents hold ρ = 0 and the mother holds σ_s = σ₁, the probability that her daughter's σ_s will be σ₀ is V₁₀. Parameters C₀₁ and C₁₀ are relevant when prejudice values of the two parents are at odds with each other (i.e., one parent holds ρ = 1 and the other ρ = 0), so that the probability of cultural conversion is determined by the prevailing societal prejudice (z₁). Specifically, if the mother's σ_s is equal to σ₀, the probability that it will change to σ₁ is C₀₁ × (1 − z₁). Conversely, if the mother's σ_s is σ₁, the probability of change in σ_s as it is transmitted from mother to daughter is C₁₀ × z₁. Definitions of C₀₁, C₁₀, V₀₁ and V₁₀ are summarized in Table 2.

Table 2.

Parameters that determine how often a mother's cultural belief σ_s is modified by her own, her husband's, and the societal prejudice ρ

Parameter	Conditions for conversion		Multiplying factor (due to societal ρ)	Result of conversion (daughter's σs)
	Maternal σs	Parental ρ
C10	σ1	{1, 0}	(1 − z1)	σ0
V10	σ1	{0, 0}	1	σ0
C01	σ0	{1, 0}	z1	σ1
V01	σ0	{1, 1}	1	σ1

See text for parameter definitions. Parental ρ = {1, 0} refers both to situations where father's ρ = 1 and mother's ρ = 0, and those where the two values are reversed. Note that parameter subscripts refer to the direction of change in σ_s, not the state of parental ρ.

Recursion Equations in M_σ.

Suppose the proportion of women with σ_s = σ₁ and ρ = 1 is x₁₁^t at time t, and the proportion of men with ρ = 1 is y₁^t. Then the proportion of marriages in which the bride has σ_s = σ₁ and ρ = 1, and the groom has ρ = 1, is the product x₁₁^t × y₁^t. We follow a similar procedure to write down the proportions of the other 7 possible combinations of male and female traits in marriages at time t (Table 3, column 4). We then calculate the proportions of male and female progeny with different combinations of σ_s and ρ for each marriage type using the definitions of parameters C₀₁, C₁₀, V₀₁ and V₁₀ (Table 3, columns 5–10). Finally, we obtain x₁₁ at time t + 1 by multiplying the probabilities of the 8 possible marriage types (Table 3, column 4) by the corresponding probabilities that a female child will have ρ = 1 and σ_s = σ₁ (Table 3, column 5), and adding up the resulting products. We obtain x₁₀, x₀₁ and y₁ via a similar process, by cross-multiplying the probabilities of marriage types (Table 3, column 4) with the corresponding probabilities of offspring types (Table 3, columns 6, 7 and 9, respectively):

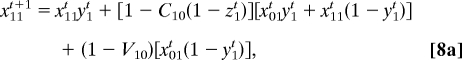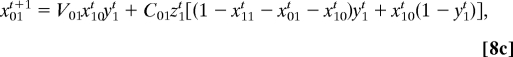z^t₁ in Eq. 8 can be expressed in terms of other variables (for the derivation, see SI Appendix, section B):

Given a number of simplifying assumptions, r at time t + 1 can be expressed as a function of x₁₁ and x₀₁ at time t (SI Appendix, section B):

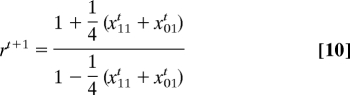Together, equations 8 through 10 define the behavior of the system in mode M_σ, and can be numerically iterated for different sets of parameters C₀₁, C₁₀, V₁₀ and V₀₁. When the parameters are such that the probability of conversion to σ₀ is relatively high and the probability of conversion away from σ₀ is relatively low (in other words, C₁₀ and V₁₀ are large, whereas C₀₁ and V₀₁ are small (see Fig. S1), r tends to increase with time. However, if the former probability is decreased and the latter is increased, r can decrease instead (Fig. S2).

Table 3.

Marriage table in mode M_σ

♀		♂	Probability of marriage type at time period t	Frequencies of trait combinations at time period t + 1
				Among daughters				Among sons
ρ	σs	ρ		ρ = 1, σs = σ1 (x11)	ρ = 1, σs = σ0 (x10)	ρ = 0, σs = σ1 (x01)	ρ = 0, σs = σ0 (x00)	ρ = 1 (y1)	ρ = 0 (y0)
1	σ1	1	(1 − x11 − x01 − x10) y1	1	0	0	0	1	0
1	σ0	1	x11 (1 − y1)	0	0	V01	1 − V01	0	1
0	σ1	1	x10 (1 − y1)	1 − C10 (1−z1)	C10 (1 − z1)	0	0	1	0
0	σ0	1	x01 (1 − y1)	0	0	C01z1	1 − C01z1	0	1
1	σ1	0	(1 − x11 − x01 − x10) × (1 − y1)	1 − C10 (1−z1)	C10 (1 − z1)	0	0	1	0
1	σ0	0	(1 − x11 − x01 − x10) × (1 − y1)	0	0	C01z1	1 − C01z1	0	1
0	σ1	0	(1 − x11 − x01 − x10) × (1 − y1)	1 − V10	V10	0	0	1	0
0	σ0	0	(1 − x11 − x01 − x10) × (1 − y1)

Recall that in mode M₀ (Fig. 2), r is so high that everyone has intuitive preference ρ = 0. Thus, we do not expect any conversion to σ_s = σ₁. In addition, if the rate of conversion to σ₀ is non-zero, we expect the proportion of women with σ_s = σ₁, and sex ratio r, to decrease. Accordingly, either the society will reenter mode M_σ, or r will reach 1 (see Fig. S2). Similarly, if the society finds itself in mode M₁, it will tend toward increasing values of r. In this case, either the society will reach mode M_σ, or r will reach the maximum value of 5/3. For a quantitative treatment of these processes, see SI Appendix, section C.

Discussion

Reducing to a Model of Economic Equilibrium.

Bhattacharjya et al. (27) constructed a purely economic model that predicts the equilibrium sex selection potency (i.e., the proportion of women who have behavior π) and SRB (i.e., r), given a set of values attributed to married and unmarried sons and daughters (27). These authors distinguish between the value v_m of a married son, the value v_u of an unmarried son, and the value v_d of a daughter. In our model, these values are equal to m_s + σ_s, m_s, and m_d + σ_d, respectively. Bhattacharjya's model (27) does not allow for the possibility of cultural variation and transmission. In terms of our model, this means that σ_s = σ₀ for all women. Thus, we can rewrite v_m, v_u and v_d as m_s + σ₀, m_s and m_d + σ₀. As a result, cultural transmission mode M_σ in Fig. 2 collapses to a single curve, and the society tends to an equilibrium SRB defined by this line (Fig. S3).

In (27), the equilibrium sex selection potency p* is defined as the equilibrium proportion of women with behavior π, and

where T is the total fertility rate and y is the probability that a woman is in a sex selection situation. In our model, T = 2, and y is the probability that a woman's first child is a girl, i.e., 1/2. By substituting 2 for T, 1/2 for y, m_s + σ₀ for v_m, m_s for v_u and m_d + σ₀ for v_d (see above) in Eq. 11, we obtain

When the width of mode M_σ is zero (Fig. S3), the boundary between modes M₀ and M₁ is defined by Eq. 6:

where r* is the equilibrium SRB to which the system will tend.

Also, from Eq. 10, we can deduce that

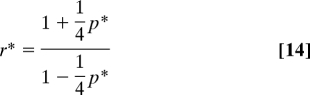under equilibrium conditions. The inverse of Eq. 14 is

By substituting Eq. 13 into Eq. 15, we obtain

which is the same as the equilibrium potency derived in (27) (Eqs. 11 and 12).

Resemblance to a Model of Cultural Transmission.

When the society is in mode M_σ, our model closely resembles a model of cultural transmission described in ref. 26. In both models, the total fertility is equal to 2, and the biological sex ratio at birth is assumed to be 1:1. Unlike Li et al. (26), however, we include the possibility of conversion from behavior π₀ to behavior π, and do not allow cultural transmission due to mass media. Our cultural conversion parameters V₁₀ and C₁₀ are similar to the parameters that determine the rates of vertical and oblique transmission of Li et al. (26).

Interpreting Societal Observations in Terms of the Model.

We now present a handful of socioeconomic observations related to sex ratio at birth in China, and interpret them in the context of our model.

Recent studies on the patterns of marriage and son preference in China have focused on a pair of counties in Shaanxi province: Sanyuan and Lueyang (26, 29, 30).

Sanyuan is a densely populated county where large family clans have a strong influence on village life, and villages maintain a strict patrilineal family system. Lueyang, however, is sparsely populated, and the patrilineal family system is much more relaxed. SRB in Lueyang between 1990 and 1996 was at 105.0, below the national average and close to the biological norm. At the same time, in Sanyuan SRB was at 117.2, much higher than in Lueyang and above the national average.

One possible reason for this discrepancy is that in Lueyang, the perceived cultural value of sons does not vary as much as it does in Sanyuan (i.e., σ₁ − σ₀ is smaller, see Fig. S4). As a result, the boundary between M₀ and M_σ may be farther to the right, which may push SRB to lower values. Additionally, SRB in Lueyang may be lower due to a lower value of Δm (Fig. S5).

Widely available ultrasound technologies that can determine the sex of a fetus exacerbate China's sex ratio problem (10, 17).

In response, the Chinese government has instituted punishment for the use of ultrasound to exercise sex selection. To explore this phenomenon in terms of our model we can define an arbitrary threshold value difference C in Eq. 4:

C is the cost of sex-selection. When ultrasound technologies are made available, C is decreased. When the use of these technologies is made punishable by law, C is increased, the boundaries of cultural transmission mode M_σ shift to the right, and the value of SRB is pushed downwards (Fig. S6).

Between the years 2000 and 2003, a number of Chinese and international agencies participated in a program entitled “Chaohu Experimental Zone Improving Girl-Child Survival Environment.”

Its purpose was to improve the chances of survival for girls in Chaohu city of Anhui province via a number of reproductive-health training and social-development activities. As a result of this program, SRB in Chaohu decreased from >125 in 1999 to 114 in 2002 (22). Its greatest effect was likely to raise the awareness of the total cost and benefits of sons, daughters, and of following the strategy of sex selection—thus making the purely economic model (Fig. S3) appropriate for the women who gave birth between 2000 and 2003.

It also could have decreased SRB by reducing Δm (e.g., via the enhancement of the social security system), by shifting the boundaries between M₀ and M₁ in the direction of increasing Δm (e.g., via punishing those who commit sex-selective abortions and infanticide (Fig. S6), or via a combination of these factors.

Conclusion

Our findings help conceptualize the socioeconomic processes that can alter the sex ratio in today's China, and hence have implications on the future governmental policies directed to bring the requisite societal change. We show that policy makers wishing to reduce female selective abortion or infanticide have two levers. One is economic incentives that alter Δm and the other is cultural changes operating on Δσ. It seems reasonable that the framework developed here can be modified to become more appropriate to the situation in India, where the dowry custom is prevalent in many areas and where the sex ratio is also extremely male-biased. Future work will build stronger connections between theoretical models of economics and cultural transmission and real-life observations.

Materials and Methods

Simulations of this article's dynamic model were performed using Mathematica software (31). The resulting plots were annotated using Graphic Converter X (32). SI Appendix, section A contains a brief discussion of the model behavior under a localized marriage market. SI Appendix, section B contains a derivation of SRB and the proportion of people with prejudice toward son preference in terms of other dynamic variables. SI Appendix, section C contains a derivation of the recursion equations under dynamic modes M₀ and M₁. Figs. S1–S6 provide additional insight into the behavior of the theoretical model.