Modeling Napping, Post-Lunch Dip, and Other Variations in Human Sleep Propensity

Abstract

Study Objectives:

To model sleep propensity (SP) as a continuous variable across 24 hours and to model the post-noon nap zone, or post-lunch dip in performance, and the early evening trough in SP.

Methods:

The present model is a variant of the 2-process model with 2 major modifications. (1) The circadian threshold process was replaced by sleep drive R, derived from REM sleep propensity, which shows a strong circadian modulation. (2) The model is based on a multiplicative interaction between the 2 input variables S and R. The model parameters S and R were estimated from experimental data. Thus, SP is modeled by multiplicative interaction of 2 sleep drives, S and R, the former of homeostatic, the latter of circadian nature. In short: SP = S × R.

Results:

Under the condition of normal phase and duration of nighttime sleep, SP across 24 hours displays 4 characteristics, (a) a major peak at nighttime, (b) a secondary increase, which peaks post-noon, (c) a first local minimum at sleep offset in the morning, and (d) a second local minimum in the early evening hours. Model simulations with either delayed or advanced sleep times suggest that the magnitude of the post-noon nap zone depends on the phase of the major sleep period within 24 hours. While the nap zone is attenuated or disappears when night sleep is delayed, SP increases during daytime when night sleep is advanced. In all conditions, the evening local minimum of SP remained stable.

Conclusions:

SP can be modeled as a continuous variable, based on the multiplicative interaction of 2 basic sleep drives. The model predictions are in agreement with known variations of SP across 24 hours.

Citation:

Bes F; Jobert M; Schulz H. Modeling napping, post-lunch dip, and other variations in human sleep propensity. SLEEP 2009;32(3):392-398.

HUMAN SLEEP PROPENSITY (SP) CAN BE DEFINED AS THE PROBABILITY TO FALL OR TO REMAIN ASLEEP AT A GIVEN POINT IN TIME. SP VARIES SYSTEMATICALLY within 24 hours with one major peak, which is normally associated with a long and consolidated sleep phase, typically placed at nighttime and coinciding with low values of deep body temperature. In addition, SP shows a secondary, but minor increase half-way between 2 subsequent major sleep phases.¹ Finally, SP displays 2 local minima within 24 hours, one in the late morning hours, about 4 to 8 hours after the body temperature minimum,^2–4 and another in the evening hours,^4–10 which has been called the forbidden zone of sleep by Lavie¹¹ and the wake maintenance zone by Strogatz.¹²

Evidence for a secondary increase of SP post noon, also called nap zone,¹³ stems from studies with free-running sleep-wake cycles,¹ ultrashort sleep-waking schedules,^9,11 constant routine protocols,¹⁴ under constant darkness for 72 hours,¹⁵ from other studies under entrained conditions,¹⁶ and from measuring unintentional sleep episodes.¹⁷ Findings from experimental studies were also supported by field surveys on napping.¹⁸ Although napping is the most obvious indicator for a secondary increase in SP, additional evidence comes from studies showing a post-lunch dip in performance tests under conditions of normal night sleep^19–21 or after sleep deprivation.²² In addition, performance decrements became evident at this time of the day in real life situations as industrial performance errors¹⁷ and traffic accidents.^17,23,24 However, Åkerstedt et al.²⁵ have suggested that the post-noon increase of traffic accidents may disappear when corrected for traffic density at different times of the day. Finally, sleep latencies in the multiple sleep latency test (MSLT) were shortest in the early afternoon.^26–28 There is experimental evidence, suggesting that the “post-lunch dip” in performance measures is not a consequence of meal ingestion,^1,21,28–30 but rather represents an independent variation in alertness. Broughton¹³ has proposed that the afternoon nap zone is an expression of a circasemidian rhythmic component. This assumption fits with earlier observations by Lack and Lushington.¹⁴

In the following, we will show that fluctuations in SP, as expressed by the afternoon nap zone, can be modeled by assuming a straightforward multiplicative interaction between 2 sleep drives. This view has been preliminarily described by us in congress proceedings.^31,32 Our approach is based on the widely accepted assumption that sleep and its timing is to a large extent determined by the interaction of 2 processes, one homeostatic and one circadian in nature.^33,34

Since the circadian process C and the homeostatic process S in the 2-process model interact via a threshold, the output of the model is either sleep or wakefulness, but not a continuous function, such as SP. The modified 2-process model,³⁴ which operates with 2 thresholds, a higher one for sleep onset and a lower one for sleep offset, allows to generate short sleep episodes or naps in addition to the major sleep phase by lowering the upper threshold.³⁴ Nevertheless, small fluctuations in SP are not intended as an output variable of that model. The 2-process model has been expanded to also predict human waking alertness and sleepiness, by quantifying the “distance” between S and the upper or lower threshold of process C.^34,35 A simulation of the time course of sleepiness across the day thus showed, after wake onset, a decrease to a plateau level, followed by a gradual increase until sleep onset,³⁵ while it did not show evidence of a post-noon nap zone, or a wake-maintenance zone in the early evening hours.

While our approach takes over the concept of 2 major processes regulating sleep, we propose several modifications. First, in our model approach we have replaced process C by a different circadian variable, called R. It is considered as a sleep drive and defined as the probability to enter REM sleep, which follows a circadian distribution across 24 hours.^36,37 We assume that the intensity of R can be estimated from REM sleep latency. Thus, in our approach SP is conceived as a continuous variable, which results from the interaction of 2 sleep drives.

The circadian modulation of R means that the probability to enter the REM sleep state fluctuates systematically within 24 hours, roughly inverse to the time course of deep body temperature.³⁸ On the other hand, S is identical with the one of the 2-process model. It represents a wake dependent growth function which dissipates during sleep and as such is of homeostatic nature. The intensity of S can be estimated from parameters of EEG slow wave activity (SWA).

As a second modification of the original 2-process model we propose a multiplicative interaction of the 2 sleep drives S and R. They may either magnify or dampen each other at a given time. When Achermann and Borbély discussed the mode of interaction between processes C and S in the 2-process model, they concluded that “The data are consistent with an additive interaction, although nonlinear (e.g., multiplicative) interactions cannot be excluded.”³⁵ More recently, several researchers have suggested to replace the additive interaction for S and C by a “non-additive” interaction.^4,10,39–41 However, none of the authors was explicit in specifying the meaning of the expression “non-additive.”

We assume that the interaction between the 2 drives (S and R) is indeed non-additive and can be formally expressed by multiplying the strength of the 2 at a given time. The rationale for this came from a mathematical point of view. By trying out modes of interaction (addition, subtraction, division, multiplication) between the 2 functions, we found that only a multiplicative interaction was able to simulate the known features in the time course of SP. Other modes fell short in showing a realistic time course for SP. Furthermore, in compliance with Dijk and Czeisler,⁸ who assumed equal contribution of sleep homeostasis and circadian rhythm to sleep consolidation, the strength of both sleep drives is supposed to be of comparable magnitude. For practical reasons we depict them on an arbitrary scale, running from 0 to 1 under conditions of 8 hours sleep and 16 hours wakefulness. SP is thus conceptualized as a probability, being the product of S and R (so, SP = S × R). In the following we will show that typical fluctuations of SP, as observed in experimental and field studies (see above), can be adequately modeled by a multiplicative interaction of the 2 sleep drives, S and R.

It should be mentioned that a multiplicative interaction was first proposed by Webb⁴² in his 3-factor model with sleep demand, circadian tendencies, and behavioral responding. He assumed a multiplicative interaction between sleep demand and circadian tendencies. However, in a later publication Webb⁴³ revised the concept and proposed an additive mode of interaction, reasoning that there is sufficient agreement between predictions of an additive model and published sleep onset data from the MSLT.

METHODS

The parameters of the constituent sleep drives S and R were estimated from a nap study, designed to assess the diurnal variation of S and R between 08:00 and 24:00,³⁷ while the time course of S and R from midnight to 08:00 was estimated from polygraphic sleep data, in accordance with data from the literature.³⁵

The nap study was performed with 12 subjects living under normal environmental conditions. The subjects napped once per day, starting their nap at systematic various times. The time span between 08:00 and 24:00 was covered by 9 naps, timed at 2-h intervals. The order of the naps was systematically varied within and across subjects. For each subject, the time between successive nap recordings was at least 3 days.

Since during normal sleep the first REM sleep episode follows SWS, the estimation of REM sleep parameters such as REM latency, may be biased by the temporal sequence of sleep states. To reduce the mutual dependence of the occurrence of SWS and REM sleep, a double-nap technique was used, i.e., the nap was divided into 2 adjacent parts, A and B, which were separated by a 10-min break. In part A of the double-nap, sleep was recorded for 30 min after sleep onset with the goal of measuring SWA (power density from 0.5-4 Hz) in the EEG. After a 10-min standardized break, during which the subject was out of bed and performed 2 short cognitive tests, part B of the double-nap started. This part of the double-nap was continued until one NREM-REM cycle was completed, or it was ended after 120 minutes if REM sleep had not occurred. The aim of part B was to measure REM sleep parameters, more specifically REM latency. For further details of the experimental setup and the results we refer to Bes et al.³⁷

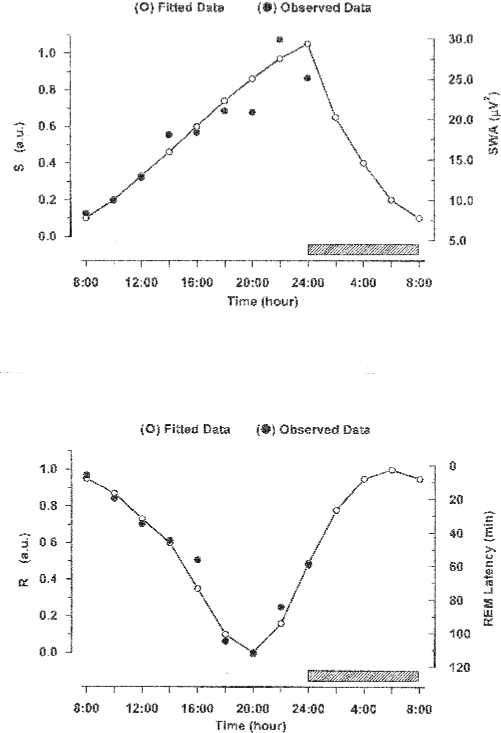
Figure 1 shows the observational data for SWA and REM sleep latency and the fitted curves which were used to depict the time courses of S and R. Curve fitting to the SWA data points between 08:00 and 24:00 was done while assuming a saturating, exponentially increasing function. For the time segment from 24:00 to 08:00, an exponential decrease of SWA was assumed in agreement with published data in the literature.³⁵ SWA shows a steady increase across the observational time, increasing 2- to 3-fold from the first to the last nap. This result complies with the notion that S can be represented as a wake-dependent growth curve.⁴⁴

Figure 1.

Observed data between 08:00 and 24:00 (from³⁷), and fitted curves representing the time courses of the homeostatic sleep drive S and circadian sleep drive R. For the time segment from 24:00 to 08:00, where no own observational data were available, we refer to Achermann and orbely.³⁵

Upper insert: Mean observed SWA values (filled circles) and fitted function S (open circles, connected by a line). S increases during waking (from 08:00 to 24:00) and is assumed to decrease during sleep (from 24:00 to 08:00, indicated by the hatched bar). The scale for the observed SWA values is given on the right side. The scale for the model function S on the left side is relative, running in arbitrary units (a.u.) from 0 to 1, where 0 indicates a low and 1 a high intensity for sleep drive S.

Lower insert: Mean observed REM latencies (filled circles) and fitted function R (open circles, connected by a line). R has a slightly skewed circadian profile with a minimum around 20:00 and a maximum around 06:00. The scale for the observed REM latencies is given on the right side. It runs from top to bottom. The scale for the model function R on the left side is relative, running in arbitrary units from 0 to 1, where 0 indicates a low and 1 a high intensity for sleep drive R.

Curve fitting to the measured data points of REM latency, the target parameter to estimate sleep drive R, was done between 08:00 and 24:00 with a 3-point moving average of the trimean.⁴⁵ In the area between 24:00 and 08:00, where no observational data were available, a sinusoidal function was applied.

REM latency increased continuously between 08:00 and 20:00 and decreased thereafter. A circadian variation of REM sleep has been consistently reported by different authors.^4,5,36,38 Likewise, in our data the percentage of sleep onset REM episodes (SOREM, defined with a latency < 25 min) decreased from 83% at 08:00 to 0% between 20:00 and 22:00.³⁷ Furthermore, in a former sleep interruption study, the amount of SOREMs after the resumption of sleep increased from 0% at 02:30 to 45% at 05:30.⁴⁶

The time course of SP was computed by multiplying the values of S and R at each point in time under the additional assumption of 8 hours of sleep, starting at midnight. To further explore the dependence of SP on the phase of the sleep period, simulations with systematic phase delays and phase advances of the sleep period were performed. From these model computations, predictions were derived for experimental testing.

RESULTS

SP in the Unshifted Sleep-Wake Cycle

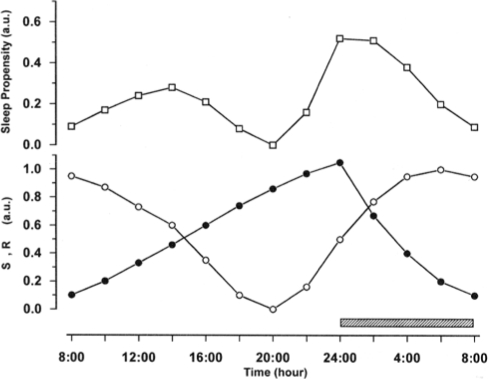
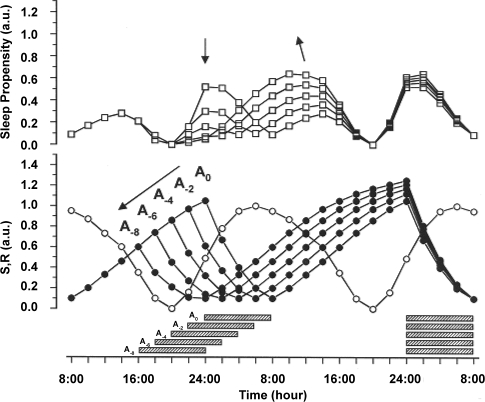
In the unshifted condition the SP curve shows 2 minima and 2 maxima (Figure 2). The first minimum is located at the end of night sleep, at 08:00, and the second minimum at approximately 20:00. Between these 2 minima, SP increases slightly and reaches its highest value in the early afternoon, at 14:00. The second minimum of SP is followed by a much steeper increase of SP which ends with sleep onset at midnight. Thereafter SP decreases steadily until the end of sleep. The curve thus depicts 2 main aspects of the daily variation in SP. In the early afternoon it shows an increase in SP, corresponding to the nap zone or post-lunch dip, and later, the curve reaches a local minimum at 20:00, corresponding to the wake maintenance zone. Multiplying the 2 sleep drives S and R always results in a double-peaked SP curve. The nighttime peak of SP is reached when S is high and R is increasing, while the smaller post-noon peak of SP appears when R is still high and S is increasing. This suggests that the magnitude of the secondary increase of SP may depend on the phase relation of the time courses of S and R, and thus can be manipulated by phase shifting sleep time.

Figure 2.

The time courses for the homeostatic sleep drive S (filled circles) and the circadian sleep drive R (open circles) are represented in the lower panel. The sleep propensity function (SP, open squares, upper panel) was computed by multiplying the values of S and R at each point in time. An 8-h sleep period (indicated by the hatched bar) is assumed to take place between 24:00 and 08:00. During this time, S decays from a high initial value to a low level at the end of sleep.

SP When Sleep Is Delayed

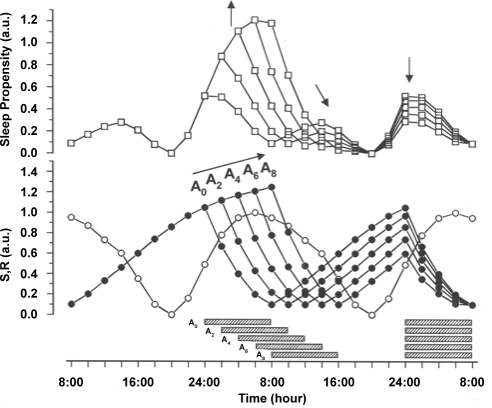
Simulated sleep phase delays are accompanied by systematic changes in SP. Increasing sleep delay from 2 to 8 hours (A₂ to A₈ in Figure 3) results in a continuous increase of the main (nighttime) peak of SP, compared to the unshifted condition (A₀ in Figure 3). As a direct consequence of shifting sleep time, the SP main peak is phase delayed correspondingly. When sleep is delayed, the magnitude of the secondary peak of SP first decreases and finally disappears completely with larger shifts. Independent of these changes, the local minimum of SP before the next sleep period remains time-locked to the evening phase position.

Figure 3.

Simulations of phase delay shifts in 2-h increments between the original phase position (A₀) and an 8-h phase delay (A₈) of sleep onset. The shifts are supposed to be independent from each other, i.e., separated long enough in time not to affect the phase of the circadian rhythm. S (filled circles) and R (open circles) are represented in the lower panel, the resulting SP functions (open squares) in the upper panel. The directions of the shift and corresponding changes in the SP function are indicated by arrows. In all simulations an 8-h sleep episode (indicated by horizontal hatched bars) begins at a given point A_n. The effects of the delay shift on SP are described in the text.

The attenuation of the secondary peak of the SP function strongly suggests that the phenomenon of a post-noon nap zone is dependent on the phase of the nighttime sleep period.

SP When Sleep Is Advanced

Advancing sleep time has even more pronounced effects on SP since it causes a decoupling of sleep time from the phase of maximum SP. A phase advance of only a few hours results in a dramatic reduction of SP prior to and during sleep. At the same time, the post-sleep SP increases correspondingly (Figure 4). The secondary SP peak, which is located in the early afternoon in the unshifted sleep condition (Figure 2), advances into the morning hours. Interestingly, with a sleep phase advance of 2 to 4 hours, the primary and secondary peaks in SP are of comparable magnitude. In contrast to these changes, the phase position of the local minimum between 18:00 and 20:00 remains unaltered.

Figure 4.

Simulations of phase advance shifts in 2-h increments between the original phase position (A₀) and an 8-h phase advance (A_-8) of sleep onset. As in Figure 3, the shifts are supposed to be independent from each other. S (filled circles) and R (open circles) are represented in the lower panel, the resulting SP functions (open squares) in the upper panel. The direction of the shifts and corresponding changes in the SP function are indicated by arrows. In all simulations an 8-h sleep episode begins at a given point A_n (indicated by horizontal hatched bars). The effects of the advance shifts on SP are described in the text.

In summary, the model computations suggest that the phase as well as the magnitude of the secondary increase of SP during daytime depend on the temporal position of the preceding sleep period. In contrast, the phase of the evening local minimum of SP seems to be independent from these phase shifts, at least as long as the placement of the subsequent sleep period is fixed.

DISCUSSION

A straightforward multiplication of the levels of sleep drive S, which is homeostatically regulated, and sleep drive R, which displays a circadian variation, appears to be sufficient to model major aspects of the time course of SP across 24 hours. Under conditions of normal nighttime sleep placement, the output of the present model during the sleep period (24:00 to 08:00) is essentially the same as that of the 2-process model, which is based on an additive interaction between processes S and R. However, during the wake period (08:00 to 20:00) the multiplicative interaction of sleep drives S and R in our model results inevitably in an additional smaller increase of SP during daytime, flanked by 2 local minima. If either S or R reaches its lowest value, S × R will also be low. This is the case in the morning, when S is at its minimum, and in the evening, when R is at its minimum. Between these 2 minima S and R have somewhat higher values and therefore the product S × R rises, resulting in a secondary peak of SP.

The choice of the scales for R and S, in particular whether the functions R and S really approach zero or not, naturally influences the time course of the resulting SP. For S we have chosen a minimum value that slightly deviates from zero because the estimation parameter for S, i.e., the power in the delta frequency band of the EEG, never attains zero. Even at minimum levels there is always some remaining power in the delta band.

For R, there was no obvious reason to choose a minimum that deviates from zero. We decided to use REM latency as the best estimation parameter for sleep drive R, and we had to invert the scale to keep its polarity (maximum drive up, minimum drive down) in line with the one of drive S. The zero level for R thus corresponds with the limit of 120 minutes for REM latency, enforced from the experimental design that we used to quantify the parameters for S and R.³⁷ This implies some arbitrariness in the choice of the origin for R. If the minimum of R deviates from zero, this would certainly have consequences for the time course of the resulting SP function.

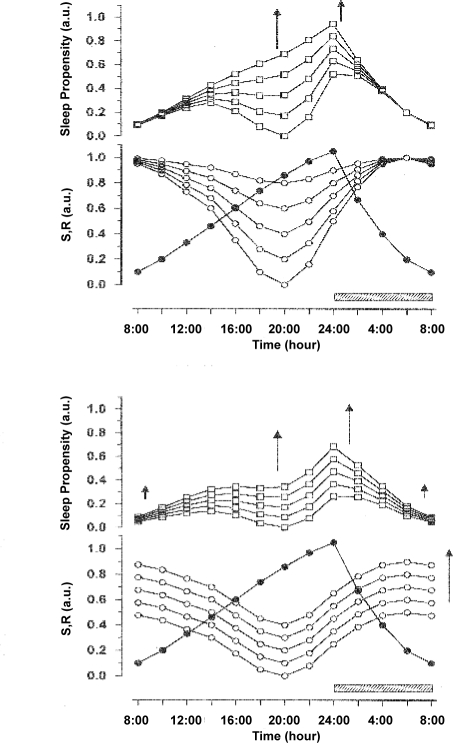
To demonstrate the expected effects, R was shifted upwards in successive steps. The 2 relevant types of shift are depicted in Figure 5. In both situations, the evening minimum in SP becomes less pronounced, while the nighttime maximum increases further.

Figure 5.

Consequences on the time course of SP if the minimum of R deviates from zero. To clearly demonstrate the effects, R was shifted upwards in successive steps, relative to S.

Upper insert: Reducing the amplitude of R in successive steps while keeping the maximum of R at 1.0 cause the nadir of R to shift upwards. The main effect on SP is that the evening minimum gets less pronounced and tends to disappear when the amplitude of R shrinks.

Lower insert: The R curve as a whole is shifted upwards (linear translation). R is not allowed to exceed the value of 1.0, as values >1 would correspond to negative REM-latencies and thus would turn meaningless. To avoid this situation, the amplitude of R has been reduced to enable upward shift. The main effect on SP of the upward shift of R is again a reduction of the evening minimum, though to lesser extent than in the upper insert.

Nevertheless the model shows quite some robustness, as with small to moderate deviations of the R-nadir from zero, all characteristic features of SP remain preserved. Only with large deviations from zero does the evening minimum disappear. Thus, we conclude that the arbitrariness in the choice of the origin for R does not substantially affect the general outcome of SP.

In fact, a slight shift of R upwards, just 0.1 or 0.2 scaling points above zero, may be more realistic and even give the opportunity to fine tune the time course of SP, as the effects on the 2 local minima turn out differently: the second minimum becomes less pronounced than the first (Figure 5). This is consistent with published data about variations in sleepiness across the nycthemeron.^53,54 Also, we have simulated prolonged waking periods (for preliminary results see³²) and SP would always drop to zero at times where R is zero, even under conditions of a very long period of sleep deprivation. This would be unrealistic and thus indeed indicates that we should never set R to actually attain zero.

The post-noon secondary peak of SP is in agreement with experimental data, which show shorter sleep latencies in the early afternoon,^26–28 a secondary (circasemidian) increase in slow wave sleep,¹⁵ an increased tendency to nap at this circadian time, either under normal entrained conditions^16,17 or under freerunning conditions,¹ and finally, a decrease in speed and quality of performance at this time.^{16,17,19–22},47 Since the model operates only with 2 sleep drives, and makes no additional assumptions, the secondary increase in SP is better explained as a circasemidian phenomenon¹³ than as an aftereffect of eating lunch. As early as 1975, Broughton referred to the observation that SP tends to display 2 peaks within 24 hours⁴⁸ and proposed the concept of a circasemidian endogenous biorhythm,^13,49 which was modeled by this author in a different way.¹³ However, our data suggest that it is not necessary to postulate an endogenous biorhythm to explain the secondary increase in SP. The present model rather suggests that this phenomenon is the consequence of a specific phase relationship between the assumed sleep drives S and R. The simulations with delaying or advancing the major sleep period demonstrate that the phenomenon is variable, changing its magnitude and phase in response to the phase, and probably also to the duration and degree of fragmentation, of the preceding sleep period.⁵⁰

Concerning interindividual differences in napping,^14,16 or in the presentation of a secondary peak in slow wave sleep between 2 major sleep episodes,¹⁵ our model would predict that morning type subjects will experience a more pronounced post-lunch dip in performance than evening types. The underlying assumption is that morning types have an advanced sleep period (Figure 4) in comparison to evening types (Figure 3). This model prediction fits quite well with experimental observations from one study, showing a post-lunch dip (between noon and 14:00) selectively in performance measures of morning types, but not in that of evening types.⁵¹ Differences between morning and evening types were also observed for self-rated alertness in a large sample of university students by Smith et al.⁵² As expected, the time course of alertness differed clearly between morning and evening types. Morning type subjects had an early increase in alertness which peaked before noon, followed by a decrease in alertness until 16:00. Thereafter alertness increased again until 20:00, followed by a steep decrease in alertness. In contrast to this, evening type subjects had a delayed but steady increase of alertness until the late evening, with only a small halt, but no decrease of alertness in the afternoon hours. Finally, the time course of alertness of intermediate type subjects took a middle position with an increase until noon and a plateau phase until 20:00, followed by a decrease in alertness thereafter.

With this line of reasoning we suppose a strong (negative) correlation between alertness and SP. However, we do not assume that alertness and SP are simply antonymes. Alertness is more strongly dependent on task demands, situational factors, and the behavioral repertoire of a person than SP. The measurement of alertness differs substantially from that of SP, and thus, in the present context, one has to acknowledge methodological differences between the 2 concepts.

While many studies support the concept of 2 daily peaks in sleepiness, studies using forced desynchrony protocols generally did not find a secondary peak of SP, likely because of the systematic shifts of the major sleep phase under these conditions.^4,8,10

In addition to an increased SP half-way between 2 major sleep periods, the S × R model predicts 2 local minima of SP, one in the morning, following sleep, the other in the evening, preceding night sleep. The morning minimum of SP in our model is obviously caused by the low level of S at the end of sleep. The minimum of SP occurs in spite of the relatively high level of R at that time (Figure 2). Interestingly, Dijk and Czeisler remarked, when discussing their results on sleep initiation, consolidation and sleep duration: “Since the circadian drive for wakefulness does not rise steeply until 4-8 hr after the minimum of core-body temperature rhythm, spontaneous awakening at the habitual wake-time under entrained conditions must occur primarily because of prior sleep (…..) and is unlikely to be related to a strong circadian wake-up signal or morning wake maintenance zone (according to Strogatz and Kronauer, 1987).”⁴ If we consider their circadian drive for wakefulness to correspond to the inverse of sleep drive R, which is not unlikely, our model simulations are in agreement with their conclusion. The attractive consequence of a multiplicative interaction of S and R is that SP will be low when only one of the 2 constituent drives has a low intensity. Simulations like those presented in Figures 3 and 4 show that the post-sleep minimum of SP is quite variable and clearly depends on the phase of the major sleep period in 24 hours. While, under conditions of normal night sleep, SP reaches a local minimum at the end of sleep, this minimum shifts and broadens with increasingly later sleep times, to finally merge with the evening minimum (Figure 3). On the other hand, if sleep is advanced, the post-sleep minimum of SP disappears, due a pronounced increase of SP.

The evening minimum of SP, corresponding to the wake maintenance zone,¹² or forbidden zone of sleep,¹¹ turned out to be a robust phenomenon, which appeared in the simulations not only under normal night sleep conditions but also equally under conditions of phase delay or advance shifts of the main sleep period. The only condition that might predict a diminution of the wake maintenance zone would be an amplitude reduction of sleep drive R (Figure 5).

Finally, it remains to justify the choice of R as the second variable (with S) to model SP. There are other variables, (for instance, deep body temperature) that are traditionally used to represent circadian rhythmicity of the system. In contrast to body temperature, which can be measured continuously, REM sleep has the disadvantage of being a “covered” variable, which can be measured only while a subject is sleeping (although, admittedly, such a disadvantage also applies for the measurement of S). Nevertheless we preferred R, because REM sleep represents, beside slow wave activity (S), the second major constituent of sleep and as such, it would be very appealing to recognize its direct contribution to SP.

DISCLOSURE STATEMENT

This was not an industry supported study. The authors have indicated no financial conflicts of interest.