Transverse Relaxation and Magnetization Transfer in Skeletal Muscle: Effect of pH

Abstract

Exercise increases the intracellular T₂ (T_2,i) of contracting muscles. The mechanism(s) for the T_2,i increase have not been fully described, and may include increased intracellular free water and acidification. These changes may alter chemical exchange processes between intracellular free water and proteins. In this study, the hypotheses were tested that 1) pH changes T_2,i by affecting the rate of magnetization transfer (MT) between free intracellular water and intracellular proteins and 2) the magnitude of the T_2,i effect depends on acquisition mode (localized or non-localized) and echo spacing. Frog gastrocnemius muscles were excised and their intracellular pH was either kept at physiological pH (7.0) or modified to model exercising muscle (pH 6.5). The intracellular transverse relaxation rate (R_2,i =1/T_2,i) always decreased in the acidic muscles, but the changes were greater when measured using more rapid refocusing rates. The MT rate from the macromolecular proton pool to the free water proton pool, its reverse rate, and the spin-lattice relaxation rate of water decreased in acidic muscles. It is concluded that intracellular acidification alters the R_2,i of muscle water in a refocusing rate-dependent manner and that the R_2,i changes are correlated with changes in the MT rate between macromolecules and free intracellular water.

INTRODUCTION

The proton T₂ of water increases in exercising muscles by up to 30% (1,2). This robust change in T₂ in response to a normal physiological perturbation has allowed exercising muscle and models of exercising muscle to be outstanding experimental paradigms for studying the biophysical basis of transverse relaxation. Moreover, measuring T₂ or T₂-weighted image signal intensity may provide information concerning the metabolic and hemodynamic responses of muscle to exercise (3,4).

Several hypotheses regarding the mechanism of the T₂ increase have been proposed. Several researchers have shown that increases in intracellular muscle water content may be the most important determinant of exercise-induced T₂ changes (5–7). During exercise, intracellular water accumulation may result from osmotically (7,8) and/or hydrostatically driven fluid shifts. For a system containing free intracellular water and macromolecular protons exchanging rapidly by way of a third pool of interfacial water, such fluid shifts will increase the apparent T₂ of the free intracellular water by changing the relative populations and/or exchange rates between the pools (9,10). Support for the hypothesis that osmotically induced intracellular water accumulation increases T₂ during exercise comes from 1) greater levels of metabolite accumulation, volume change, and T₂ change in predominantly glycolytic muscles than in predominantly oxidative muscles (6); 2) greater levels of T₂ and relative metabolite accumulation (and, presumably, volume change) in exercised fresh water than marine invertebrates (8); and 3) the direct relationship between T₂ and intracellular volume in frog sartorius muscles during osmotic shock experiments (7).

In addition, intense exercise decreases the intracellular pH (pH_i) in healthy subjects. Support for a pH effect on T₂ comes from the inverse relationship between pH_i and T₂ following exercise (11) and during direct manipulations of pH in isolated muscles (7,12); we proposed that pH affects the T₂ by modulating chemical exchange pathways between macromolecules and free water (7). However, during incremental arm ergometer exercise, pH_i changes lag T₂ changes and following exercise, pH_i recovers more rapidly than T₂ (13). Also, Meyer et al. have shown that there is no additional effect of pH_i on the in vivo T₂ changes measured during imaging experiments (8). These discrepancies concerning the role of pH_i changes in the T₂ change of exercise may be due to differences in the T₂ measurement techniques employed. Because the sensitivity of T₂ to chemical exchange effects depends on the refocusing rate (14), the rapid refocusing pulses used the Damon et al. study may have been sensitive to a pH_i effect on T₂, while the slower refocusing rates using the imaging studies of Meyer et al. may not have been.

Therefore, in order to understand better the mechanism of the increased T₂ in exercising muscle and more specifically the effect of pH_i on muscle T₂, the T₂ of ex vivo frog muscle was measured at two different pH_i values using non-localized and localized Carr-Purcell Meiboom-Gill (CPMG) pulse sequences with different echo spacing. A second reason for the study was to relate pH_i-induced T₂ changes with changes in the magnetization transfer (MT) rate between the immobile macromolecular proton pool and the free water proton pool. pH_i values of 7.0 and 6.5 were chosen to model resting and exercise conditions, respectively. Frog muscle was used because it is a valid model for many aspects of mammalian muscle physiology and because it is more robust ex vivo than mammalian tissue. The results of the study show that intracellular acidification increases the intracellular T₂ (T_2,i) without changes in muscle density, and that the magnitude of the effect depends on echo spacing. These T_2,i changes are accompanied by changes in the MT rate between the macromolecular and free intracellular water proton pools and in the longitudinal relaxation rate (R₁) of water.

METHODS

Tissue Preparation

These procedures were approved by the Institutional Animal Care and Use Committee at Vanderbilt University Medical Center. Rana pipiens were purchased from Carolina Biological Supply (Burlington, NC), anesthetized in a solution of Fenquil 1 g/L for 15–20 min, and then decapitated and doubly pithed. Pairs of gastrocnemius muscles (<0.5 cm (diameter, d) × ~4.5 cm (length, l) were removed and the tendons of origin and insertion were tied with nylon sutures. The muscles were bathed in Ringer’s solution and oxygenated in an ice bath for 1 hour and 45 minutes. The Ringer’s solution contained (in mM) 115 NaCl, 2.0 KCl, 2.5 CaCl₂, 2.15 Na₂HPO₄, and 0.85 NaH₂PO₄ (pH 7.0 at 20 °C). A modified Ringer’s solution was used to acidify the muscles (15) and it contained (in mM) 75 NaCl, 40 NH₄Cl, 2.0 KCl, 2.5 CaCl₂, 2.15 Na₂HPO₄, and 0.85 NaH₂PO₄ (pH 7.0 at 20 °C). The muscles were refrigerated in either the Ringer’s solution or modified Ringer’s solution at 4 °C for ~12 hours. The muscle stored in modified Ringer’s solution was rinsed several times in Ringer’s solution for at least 3 hours prior to experiment, acidifying the muscle (15). The muscle stored in Ringer’s solution was maintained in its preceding condition during the experiment.

The muscle was placed inside a 12 mm (d) × 7 cm (l) NMR tube (Wilmad, Buena, NJ) with open ends. The tendon sutures were held in place by the tube’s end caps, ensuring that the muscle remained securely in place. This inner tube was placed in an outer tubular holder (15 mm (d) × 8 cm (l)), also containing Ringer’s solution. Holes in the end caps of the inner tube and in the top portion of the outer tube allowed air bubbles to escape, improving B₀ homogeneity. The muscle tube assembly was placed in a Chemagnetics (Fort Collins, CO) 40 mm inner diameter millipede coil, parallel to the long axis of the RF coil, and the entire assembly was parallel to B₀.

Experiments were performed at room temperature. Temperature measurements were made by placing a non-magnetic thermocouple probe in the sample holder adjacent to the muscle and were used to verify the thermal stability of the sample and to determine the negative log of the equilibrium acid constant of carnosine (pK_a,c) for the calculation of pH_i, as described below.

MR Data Acquisition

General

All MR experiments were made over a three-hour period using a 120 mm, horizontal bore 4.7 T superconducting magnet (Magnex Scientific, Oxfordshire, UK) with a Varian Inova console (Varian, Inc., Palo Alto, CA). Prior to all studies, global shimming was done using a single pulse-acquire sequence until the water linewidth was less than 20 Hz. For measurements requiring slice selection, localized shimming was performed using a PRESS sequence on 10 × 10 × 30 mm voxel until the water linewidth was less than 9 Hz.

Total Muscle Volume

Muscle volume was measured by using a single spin-echo multi-slice (SEMS) sequence. The experimental parameters included a 4 ms Gaussian pulse, echo time (TE)/repetition time (TR) = 30/2000 ms, 128 × 128 matrix size, 46 – 50 slices, 1 slice-interleaved acquisition with thickness = 1 mm and no gap, number of excitations (N_EX) = 1, and field of view (FOV) = 18 mm × 18 mm.

¹H MR Spectroscopy

At the middle and conclusion of the MR experiments, ¹H water suppressed spectra were obtained using a PRESS sequence modified with MEGA (16) water saturation. The experimental parameters included a 2 ms sinc excitation pulse with 8 ms Gaussian saturation pulse, TE/TR = 28/3000 ms, N_EX = 64 – 128 and voxel size = 7 mm × 7 mm × 20 mm.

Localized T₂

Transverse relaxation decay data were obtained using a single-slice, multi-echo CPMG sequence. The experimental parameters included a 1 ms sinc excitation pulse followed by 300 μs duration, 90°-180°-90° composite refocusing pulses. For all studies, TR = 3s; for the 8 ms echo spacing, the number of echoes (N_E) = 50, and for the 30 ms echo spacing, N_E = 30. The FOV was 16 mm × 16 mm with a 2 mm slice thickness, matrix = 64 × 64 matrix, and N_EX = 4. The power was calibrated for a 300 μs, 90°-180°-90° composite pulse with maximum signal intensity profile attained with a 4 mm slice and FOV of 10 mm × 10 mm that included as much of the muscle sample and as little of the Ringer’s solution as possible.

Non-localized T₂

Transverse relaxation data were obtained by using a CPMG sequence having a 37.5 – 40 μs hard excitation pulse and refocusing pulses of 75 – 80 μs. For the echo time spacing of 2 ms, N_E = 4096 echoes and for the echo time spacing of 8 ms, N_E = 2048. The TR was 5 s with N_EX = 16.

Quantitative MT (qMT)

The MT imaging data were acquired with a selective inversion recovery preparation (a 1 ms hard inversion (180°) pulse) followed by a variable inversion time (TI) and then a fast spin echo readout (a 500 μs Gaussian (90°) pulse with a train of eight 180° Gaussian refocusing pulses separated by 10 ms) (17). Twenty-five TI values were used, with 21 points logarithmically spaced between 3.5 ms and 150 ms with additional times of 300 ms, 1 s, 2 s and 8 s. Other parameters included a predelay (PD) = 3 s, N_EX = 4, 64 × 64 matrix, FOV of 18 mm × 18 mm, and 1 mm thick slice.

Data Analysis

Muscle mass, volume, and density

Intracellular acidification brought about by transient exposure of muscle cells to NH₄Cl activates Na⁺-H⁺ exchange as the principal pH_i regulatory mechanism (18), which in turn may lead to a regulatory volume increase. Because the density of muscle under control conditions is greater than that of water, intracellular water accumulation would be reflected in a decrease in muscle density. Therefore, the mass of the muscles (m_m) was measured at the end of NMR experiments and the muscle volume (V_m) was calculated from the SEMS data by defining a region of interest (ROI) around the muscle in each slice. The volume of each ROI was computed from the FOV, slice thickness, and matrix size and used to calculate V_m. Muscle density was calculated as ρ_m = m_m/V_m.

Intracellular pH

Time domain data were processed in Matlab v. 7.0 (The Mathworks, Inc. Natick MA) using 0.2 Hz exponential line broadening, baseline correction, and Fourier transformation. Chemical shifts were referenced to creatine –R–NH₂ at 3.02 ppm (19). The C-2 carnosine proton peak was fitted to a Lorentzian function and the chemical shift of the maximum peak height (δ_c) was used to calculate pH_i, using

$$pH=p{K}_{a,c}+log\left(\frac{{\delta }_a-{\delta }_c}{{\delta }_c-{\delta }_b}\right)$$ [1]

where δ_a is the acidic limiting chemical shift (8.58 ppm) and δ_b is the basic limiting chemical shift (7.66 ppm) (19). The pK_a,c was calculated from the information provided in reference (19) and the temperature.

T₂

A multi-component T₂ model and a non-negative least squares (NNLS) algorithm (20) were used. The value for the regularizer term, μ, was ~10/SNR (μ = 0.01 and 0.05 for the nonlocalized and localized data, respectively). The non-localized transverse relaxation decay data were fitted to 128 T₂ values logarithmically spaced between the first TE (2 or 8 ms) and 5 s. The localized transverse relaxation decay data were obtained by specifying an ROI around the muscle borders (ROI size range 460 – 745 pixels). These data were fitted to 250 T₂ values logarithmically spaced between 8 or 30 ms and 1 s. To characterize the information in the T₂ spectra simply, regions of short and long biological T₂ values, assumed to represent intracellular and extracellular water, were defined. The intracellular T₂ (T_2,i) regions were defined as: 15 – 52 ms (non-localized, TE spacing = 2 ms); 15 – 70 ms (non-localized, TE spacing = 8 ms); 15 – 57 ms (control, localized, TE spacing = 8 ms); 15 – 45 ms (acidic, localized, TE spacing = 8 ms); 30 – 45 ms (localized, TE spacing = 30 ms). The extracellular T₂ (T_2,e) regions were defined as the remaining values less than 400 ms. The rationale for the thresholds distinguishing T_2,i and T_2,e is illustrated in the Results section. The 400 ms upper value for T_2,e was set on the basis of T₂ spectra from phantom studies in which a vial containing a short T₂ species (~75 ms) placed within Ringer’s solution (T₂ ~2.1 s). The T₂ spectra contained an extra peak at ~617 ms when T₂ was measured using non-localized acquisitions, but not with localized acquisitions (results not shown). This 400 ms threshold is approximately two times greater than the largest value of T_2,e that has been reported in the literature. Within each region, the average T₂ value (weighted by relative signal intensity) was calculated and the transverse relaxation rates were calculated as R₂ ≡ 1/T₂. All echoes were used in the T₂ analysis since pilot studies revealed similar results regardless of whether all echoes, even echoes-only or odd echoes-only were used.

Intracellular volume fraction

The intracellular and extracellular water contents were assumed to relate directly to the areas under the curve in the T_2,i and T_2,e spectral regions. The intracellular water fraction, F_i, was calculated as 100% × the ratio of the area of intracellular region to the total area under the curve.

qMT Analysis

The analysis described in reference (17) was applied to the selective inversion recovery data. This analysis assumes that the sample can be described as containing a macromolecular pool with population fraction p_m and a free water pool with population fraction p_f. The data were fit on a pixel-by-pixel basis using Matlab’s built-in Nelder-Mead Simplex search algorithm to a bi-exponential function of TI as

$$\frac{M_f(TI)}{M_{f\infty }}=b_f^{+}exp(-R_1^{+}\ast TI)+b_f^{-}exp(-R_1^{-}\ast TI)+1,$$ [2]

where M_f(TI) is the longitudinal magnetization at time TI, M_f∞ is the equilibrium magnetization, $R_I^{+}$ and $R_I^{-}$ are the slow and fast recovery rates, respectively, and $b_f^{+}$ and $b_f^{-}$ are the corresponding amplitudes (17). The MT rate from the macromolecular pool to the free water pool (k_mf) and the pool size ratio (p_m/p_f) were determined by using Eqs. 4 and 10 of reference (17); the reverse rate (k_fm) was calculated as k_fm=(p_m/p_f)·k_mf.

Statistics

Descriptive statistics include the mean and standard deviation (SD). Paired, two-tailed Student’s t-tests were performed to compare the mean values of pH_i, ρ_M, R₂, k_mf, and $R_1^{-}$ in the control and acidic muscles. A one-way analysis of variance was used to compare the mean values of the R_2,i difference between control and acidic muscle (ΔR_2,i) and for the different echo time spacings and acquisition type. The hypotheses that k_mf is linearly related to [H⁺] and pH_i were tested by calculating linear correlations between pH_i and k_mf and between [H⁺] and k_mf, separately for the acidic muscles, control muscles, and all muscles. Because k_fm is calculated from p_m/p_f and k_mf and is therefore not an independent measurement, statistics for this variable were not calculated. A p value <0.05 was considered statistically significant.

Results

Intracellular pH

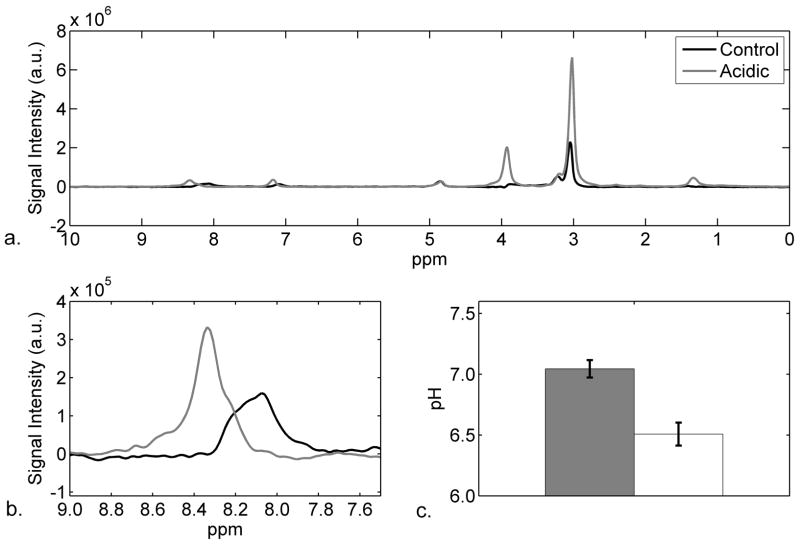
Figure 1a shows typical ¹H NMR water-suppressed spectra for the control (black line) and acidic (gray line) muscles. Figure 1b shows the carnosine C-2 proton resonance in a zoomed region (7.5 – 9 ppm) of the spectra in Figure 1a. The chemical shift of the C-2 proton is 8.10 ppm for the control muscle (pH_i 7.03) and shifts to 8.30 ppm for the acidic muscle (pH_i 6.58). Figure 1c shows the mean and SD for the pH_i of all muscles. The control muscles had a mean pH_i of 7.04 ± 0.07 whereas the acidic muscles had a mean pH_i of 6.51 ± 0.09 (P < 0.001). During the course of the MR experiments, the pH_i did not vary more than 0.05 pH units.

Figure 1.

a) Representative ¹H NMR water suppressed spectra of control (black line) and acidic (gray line) muscles at pH_i 7.03 and 6.58, respectively. b) Zoomed-in region of Panel a, showing the shift of the C-2 carnosine resonance between the control (black line) and acidic (gray line) muscles. For panels a and b, the differences in peak heights reflect receiver gain differences only. c) Bar graph of pH_i of muscles (n=7) showing mean and standard deviation of control (pH 7.04 ± 0.07) and acidic (pH 6.51 ± 0.09) muscles (P < 0.001).

Mass, Volume, Density, Temperature

The masses of the control and acidic muscles were 1.63 ± 0.20 and 1.68 ± 0.23 g, respectively (P = 0.019). The average volumes for the control and acidic muscles were 1.32 ± 0.15 cm³ and 1.34 ± 0.16 cm³, respectively (P = 0.033). The density for the control muscles was 1.23 ± 0.02 g/cm³ and for the acidic muscles was 1.25 ± 0.03 g/cm³ (P = 0.22). The muscle temperature did not vary more than 0.5° within an individual experiment and for all experiments ranged between 20 – 23 °C.

Transverse Relaxation Rates and Derived Measures

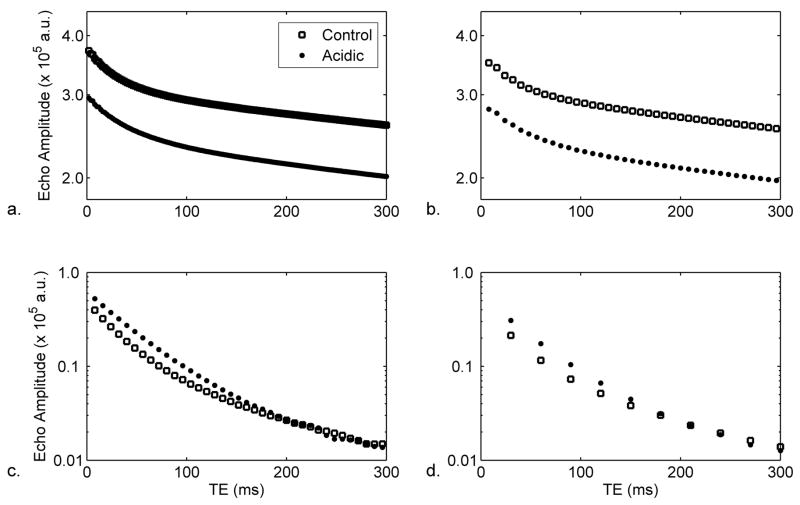
Semi-log plots illustrating transverse magnetization decay data for TE<300 ms are shown for non-localized experiments with echo spacing times of 2 ms and 8 ms in Figures 2a and 2b, respectively. Representative T₂ decay data for localized experiments with echo spacings of 8 ms and 30 ms are shown in Figures 2c and 2d, respectively. In all four plots, the decay is non-monoexponential.

Figure 2.

Representative semi-log plots of transverse magnetization decay data for a) 2 ms echo spacing of non-localized experiments; b) 8 ms echo spacing of non-localized experiments; c) 8 ms echo spacing of localized experiments; and d) 30 ms echo spacing of localized experiments. As indicated in the legend to Panel a, the data are shown for control and acidic conditions. For all plots, the TE region from 0–300 ms is shown, in order to highlight the transverse relaxation of the muscle water; note that non-localized acquisitions include contributions from Ringer’s solution. Also, note that the differences in the Y intercepts result from differences in receiver gain settings and do not imply proton density differences between the conditions.

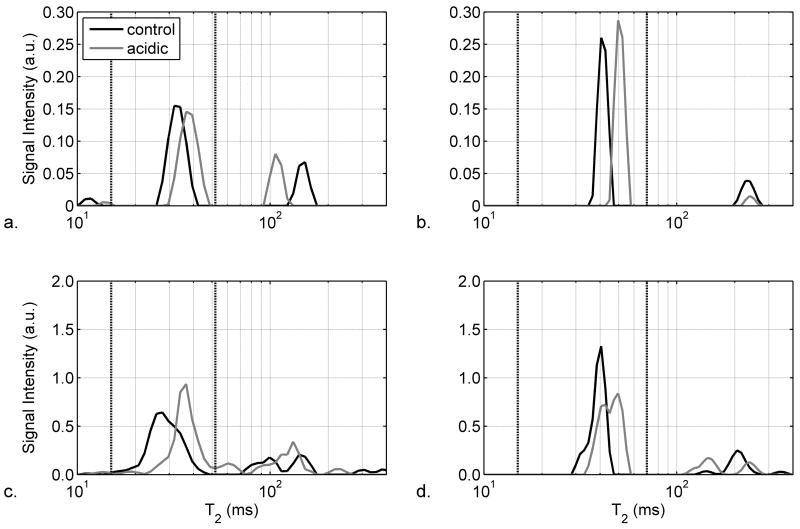
Representative non-localized T₂ spectra from a control muscle (black line) and the paired acidic muscle (gray line) are shown as a semilog plot in Figures 3a and 3b for echo spacing times of 2 ms and 8 ms, respectively. There is a peak representing T_2,i at ~30 ms and a peak representing T_2,e at ~125 ms. Other peaks that appeared but are not shown include a third apparent T₂ component between 400 ms and 1 s and a large peak with a T₂ of ~2 s that resulted from the Ringer’s solution. The summed T₂ spectra are shown in Figures 3c and 3d for the experiments performed at 2 ms and 8 ms, respectively. The vertical lines indicate the boundaries of the T_2,i region.

Figure 3.

Semi-log plots of T₂ spectra from representative (Panels a and b) and summed (Panels c and d; n=7) non-localized acquisitions with echo spacings of 2 ms (Panels a and c) and 8 ms (Panels b and d). As indicated in the legend to Panel a, the data are shown for control muscles (black lines) and acidic muscles (gray lines). In order to highlight the muscle region of the T₂ spectrum, the region from 10–400 ms is shown. The vertical dashed lines indicate the boundaries of the intracellular T₂ component, as discussed in the text.

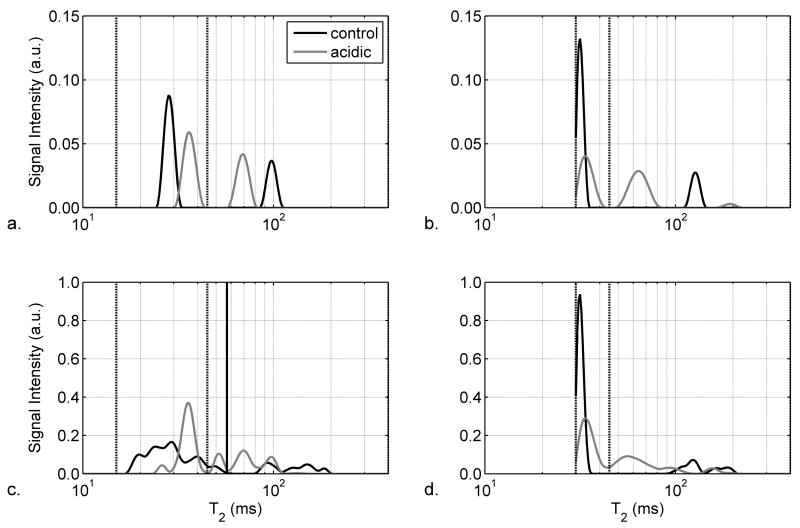
Representative T₂ spectra from a control muscle (black line) and the paired acidic muscle (gray line) are shown in Figures 4a and 4b for localized acquisitions using echo time spacings of 8 ms and 30 ms, respectively. The two peaks represent the T_2,i component at ~30 ms and the T_2,e component at 80 to 150 ms. The summed T₂ spectra measured with echo spacing times of 8 and 30 ms are shown in Figures 4c and 4d, respectively. The vertical lines indicate the T_2,i region. For both echo spacings, the summed T₂ spectrum of the acidic muscles show that the T_2,i increases and the T_2,e decreases as compared to the control muscles.

Figure 4.

Semi-log plots of T₂ spectra from representative (Panels a and b) and summed (Panels c and d; n=7) non-localized acquisitions with echo spacings of 2 ms (Panels a and c) and 8 ms (Panels b and d). As indicated in the legend to Panel a, the data are shown for control muscles (black lines) and acidic muscles (gray lines). In order to highlight the muscle region of the T₂ spectrum, the region from 10–400 ms is shown. The vertical dashed lines indicate the boundaries of the intracellular T₂ component, as discussed in the text.

The mean and SD of the R_2,i values are reported in Table 1. Regardless of echo spacing or whether the experiments were localized or non-localized, R_2,i decreased as pH_i decreased. In non-localized experiments, the difference in R_2,i between the acidic and control muscles (ΔR_2,i) was 8.1 ± 1.5 s⁻¹ for the 2 ms TE spacing and 3.4 ± 1.0 s⁻¹ for the 8 ms TE spacing (P = 0.002 and 0.013, respectively). For localized experiments, the ΔR_2,i values were 4.5 ± 1.9 s⁻¹ and 3.6 ± 0.8 s⁻¹ for the TE = 8 ms and 30 ms spacings, respectively. The ΔR_2,i values are shown in Figure 5 and reveal that ΔR_2,i was significantly greater for the non-localized, TE = 2 ms spacing than for the non-localized, TE = 8 ms spacing (P = 0.020) and for the localized, 30 ms TE spacing (P = 0.023); a similar trend was noted for the 8 ms TE, localized experiment (P = 0.066).

Table 1.

Influence of pH_i on R_2,i, the latter assessed using non-localized and localized acquisitions and with different TE spacings. The first two rows of data report the mean and standard deviation (N = 7).

		Non-localized R2,i (s−1)		Localized R2,i (s−1)
		2 ms	8 ms	8 ms	30 ms
Means at pHi ~	7.0	35.2 ± 3.1	25.8 ± 2.2	34.0 ± 2.7	31.5 ± 0.3
	6.5	27.0a ± 3.0	22.4b ± 2.6	29.5c ± 3.8	27.9b ± 1.9

^ap < 0.05;

^bp < 0.01;

^cp = 0.052

Figure 5.

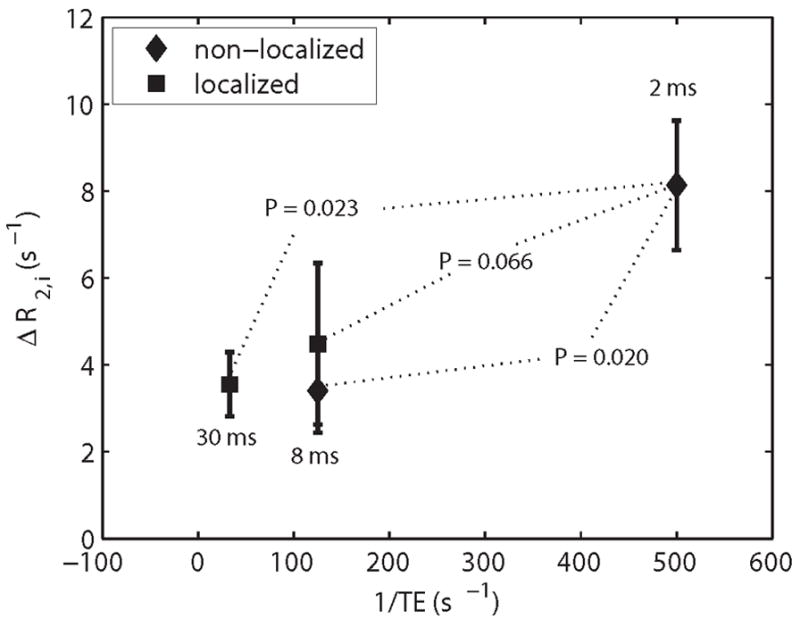
The differences in R_2,i (ΔR_2,i) between acidic and control muscles are plotted as a function of the refocusing rate for the non-localized and localized experiments. The error bars represent the standard error of the mean. In both cases, localized and non-localized, as the echo spacing time is decreased, ΔR_2,i becomes larger. The P values reported are relative to the shortest TE of 2 ms.

In general, R_2,e did not change significantly with pH_i. The only exception was the localized experiment with an echo spacing of 8 ms, in which the R_2,e for the control muscle was 8.3 ± 2.2 s⁻¹ and for the acidic muscle was 13.7 ± 3.3 s⁻¹ (P < 0.001). Irrespective of the type of measurement, F_i was ~80% in the control condition. In the non-localized measurements the F_i values did not differ significantly between the control and acidic muscles. However, the F_i values derived from the localized R_2,i measurements differed significantly between the control and acidic muscles, decreasing to 60% in the acidic condition.

Magnetization Transfer

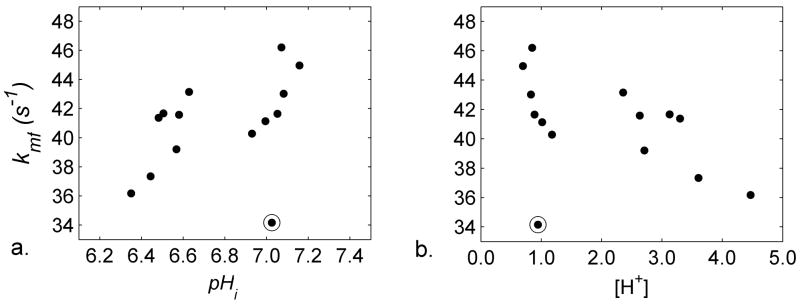
Sample selective inversion recovery data are shown for the acidic and control conditions in Figure 6. Figure 7 presents the raw data illustrating the relationships among k_mf, pH_i, and [H⁺] and Table 2 reports the MT parameters under control and acidic conditions. A data point in the control group (the circled point in Figure 7) was identified as an outlier with respect to k_mf, and so the data from it and its paired, acidic muscle were removed from the following statistical comparisons. k_mf and R₁⁻ decreased in acidic muscles; p_m/p_f did not change. Table 2 also reports the correlations between k_mf and pH_i and between k_mf and [H⁺]. Significant or nearly-significant linear correlations existed between each variable pair, regardless of whether the acidic muscles only, control muscles only, or all muscles were considered.

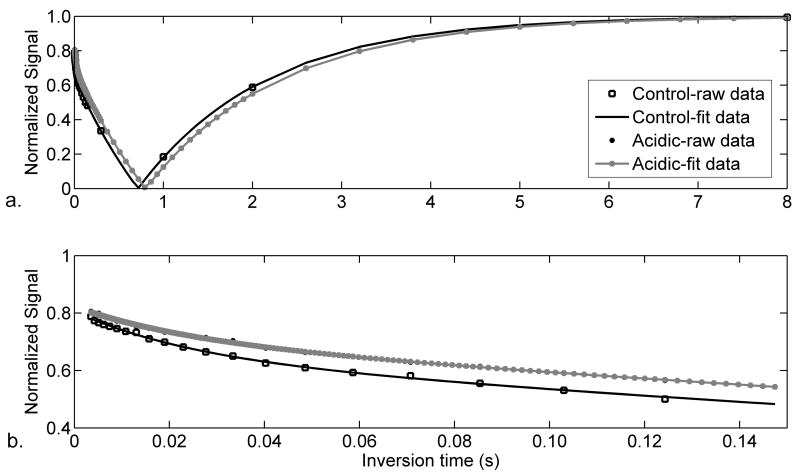
Figure 6.

Representative MT-inversion recovery data for control (squares and black lines) and acidic (gray points and lines) muscles. A) Full inversion-recovery curve. b) The early portion of the recovery (0 – 200 ms) in Figure 7a, revealing the bilinear recovery resulting from MT.

Figure 7.

Scatterplots illustrating relationships among pH and k_mf parameters. a) Plot of k_mf as a function of pH_i. The circled point was identified as an outlier and so the data from it and its acidic muscle pair were removed from all descriptive and inferential statistical tests involving the MT parameters. b) Plot of k_mf as a function of [H⁺].

Table 2.

Effect of pH_i on the MT rates, k_mf and k_fm, from the solid pool (with population fraction p_m) to the free water pool (with population fraction p_f) and in the reverse direction, respectively. The third and fourth rows report the correlations between pH_i, [H⁺], and the MT parameter estimates.. Pool size ratio (p_m/p_f) and slow recovery rate ( $R_1^{-}$) are also reported. Statistical tests reflect removal of outlying point and its pair (see Text). Significant findings are indicated with superscripts; the same notation as in Table 1 has been continued here. For means, significance reflects differences from control condition. Note that because k_fm is not an independent measurement, statistics for this variable are not presented.

		Kmf (s−1)	kfm (s−1)	pm/pf	$R_1^{-}$ (s−1)
Mean±SD at pHi ~	7.0	42.88 ± 2.31	3.56 ± 0.42	0.08 ± 0.01	0.68 ± 0.02
	6.5	39.82 a ± 2.71	3.16 ± 0.18	0.08 ± 0.00	0.65b ± 0.02
Correlations with pHi		0.85a (acidic)
		0.78c (control)
		0.76b (all)
Correlations with [H+]		−0.85a (acidic)
		−0.78c (control)
		−0.73b (all)

^ap <0.05;

^bp <0.01;

^cp=0.066.

DISCUSSION

An important finding of this study is that decreases in pH_i decrease R_2,i in isolated muscle preparations. This finding is consistent with that of Fung and Puon’s study of permeabilized muscle strips in solutions with pH values of 5, 7 and 9, in which they showed that the R₂ is directly related to pH_i (12); with the observations of Bertram et al, who demonstrated that the T₂ of extracted myofibril preparations also increases at low pH (21); and with those of our previous work at 7.0T, in which R_2,i was directly related to pH_i throughout the physiological range (6.5 –7.4) (7). In addition, this study shows that the influence of pH_i on R_2,i exists for all acquisition conditions studied, including localized and non-localized data acquisitions and for refocusing intervals ranging from 2 – 30 ms. The magnitude of R_2,i varied with the refocusing period, however, with the pH_i effect on R_2,i being larger at 2 ms intervals than with longer intervals. Additionally, the pH_i induced variations in R_2,i correspond to changes in the rates of MT between macromolecular protons and free intracellular water and of longitudinal relaxation for free intracellular water. Collectively, these data provide new insights into the specific biophysical phenomena that contribute to the R_2,i change of exercise, the exchange processes among the various tissue proton pools, and the biophysical influences on relaxation in general.

Multi-exponential Transverse Relaxation in Muscle

A common finding in transverse relaxometry studies of ex vivo muscle (7,9,22,23), and in certain studies of in vivo muscle (5,24), is that this tissue exhibits multi-exponential relaxation. In the present study, the ¹H transverse relaxation of muscle water was non-monoexponential in all of the measurements performed, as reflected in the non-linear semi-log plots in Figure 2 and the complex T₂ spectral patterns in Figures 3 and 4. We conducted phantom studies that demonstrated that T₂ components larger than 400 ms, but unassociated with the Ringer’s solution (T₂ ~2 s), were artifactual in origin. In order to characterize the information in the biological portion of the spectrum simply and in a manner that would permit statistical comparisons, we interpreted the shorter T₂ portions as reflecting water in a single compartment of the intracellular space of muscle and the longer T₂ portion of the spectrum as representing water in a single compartment of the interstitial space (9,22,23). In addition, a T₂ component <10 ms has been inconsistently reported for muscle, which has been attributed to hydration shell of macromolecules (9). The long TE spacings used for most of the T₂ measurements in the present study precluded the observation of this component.

pH_i and Intracellular Water Content

Despite the potential for activation of Na⁺-H⁺ exchange and regulatory volume changes in the cells, we argue for several reasons that the MT and R_2,i changes observed in this study are a direct and exclusive effect of intracellular acidification. Most importantly, the densities of the control and acidic muscles did not differ significantly and were uncorrelated with the R₂ and MT findings. Second, intracellular water accumulation would tend to increase F_i. However, F_i either did not change (non-localized R₂ measurements) or decreased (localized R₂ measurements). We consider these two possibilities further below; but for the present argument, the salient point that neither behavior is consistent with the requirement of a higher F_i under this alternative hypothesis. Finally, p_m/p_f should decrease with intracellular water accumulation; but this variable did not differ between the two conditions. For these reasons, we conclude that the decrease in pH_i was the most significant, and probably the only, effecter of the R_2,i decrease in these experiments.

pH_i and R_2,i

A consistent finding in this work is that the pH_i is directly related to R_2,i. Moreover, the magnitude of this effect varies with TE spacing, with larger effects being observed with shorter spacings. This finding resolves the discrepancy between our previous findings with short TE spacings (7), which indicated a direct effect of pH_i on R_2,i, and those of Meyer et al. with long TE spacings (8), which suggested that there was no additional effect of exercise-induced pH_i changes on the whole-muscle T₂ beyond that already exerted by intracellular water accumulation. Because the present data demonstrate that the dynamic range of the pH_i effect on T₂ at long TE spacings is small, it may have been that in the Meyer et al. study, the effect of pH_i on T₂ was below the limit of detectability. Moreover, the ability to resolve distinct intracellular and interstitial T₂ components in the present study may have increased the sensitivity of our measurement as well. The dependence of R_2,i on pH_i under all acquisitions studied indicates that the possibility for systematic, group-wise variations in the pH_i response to exercise, such as might occur in metabolic conditions such as myophosphorylase deficiency and phosphofructokinase deficiency (25) or with peripheral vascular disease (26), must be taken into account when interpreting R₂ or T₂ data from exercising muscles.

While the analyses performed resulted consistently in the identification of a pronounced effect of pH_i on R_2,i, there was ambiguity with regard to the effects of pH_i on R_2,e and F_i. In the non-localized experiments, neither R_2,e nor F_i changed significantly, while in the localized experiments, R_2,e decreased and F_i increased. This discrepancy may have been due to differences in SNR, difficulty in fitting the non-localized data due to the large signal contribution from the buffer, and/or B₁ inhomogeneity in the refocusing pulses used in the non-localized acquisitions. Without a standard measurement such as a sucrose or inulin space determination, it is not possible to resolve this discrepancy. For this reason, and because several studies using different methods have demonstrated that the T₂ change of exercise is primarily an intracellular phenomenon, we focus the remaining portions of this discussion dealing with transverse relaxation on R_2,i.

pH_i and MT

Gochberg and Gore (17) previously reported values for k_mf and p_m/p_f of 46 s⁻¹ and 0.108, respectively, in the skeletal muscle of a single ferret maintained at 37°C. The mean value for k_mf that we report here under control conditions, 42.88 s⁻¹, is slightly lower than the value reported by Gochberg and Gore and probably relates to the temperature difference between the experimental preparations. We found also that the R₁ of water, k_mf, and k_fm all decreased with pH_i (that is, with increasing [H⁺]). A likely explanation for this effect lies in hydrogen exchange between water and amide protons, as the reaction is base-catalyzed for pH values greater than ~5 (27–29) and a recent preliminary report has indicated the presence of a pH-sensitive peak at the amide proton frequency in the CEST spectrum of similarly treated amphibian skeletal muscles (30). Also similar to the present observations, Gochberg et al. have observed decreases in R₁⁺ (≈k_mf) in acrylamine and methacrylamine polymer gels with decreasing pH (31). While simple acid-base chemistry would predict that the reaction rate would depend inversely and linearly on [H⁺] and exponentially with pH (27–29,32), we observed linear dependences of k_mf with both pH and [H⁺], regardless of experimental condition (Table 2 and Figure 7). The linear dependence of k_mf on pH may exist if the proteins function as polyprotic acids, whose multiple pK_a’s would make for a complex dependence of reaction rates on pH that could appear linear within the 0.8 pH-unit range that we studied. In addition, there are well recognized roles for acidic and basic side chains of proteins in determining protein structure, as their pH-dependent ionization states affect the number of opportunities for hydrogen bonding (33). In structural motifs such as the leucine zipper, there are periodic alterations in amide exchange rates that depend on the local tertiary structure, with the amide proton exchange rate corresponding to hydrogen bond length (34). Because the secondary and tertiary structures of muscle proteins including sperm whale apomyoglobin (35) and the myofibrillar proteins (21) are altered by pH, alternative and/or additional mechanisms for the non-exponential dependences of the MT rates on pH_i also exist. These include secondary or tertiary structural changes in the proteins that remove exchange opportunities between functional groups on the proteins and water or that alter the amount of interfacial (hydration) water.

pH_i, R_2,i, and MT

As noted above, the dependence of R_2,i on TE spacing, the correspondence between the MT and R_2,i changes, and the field strength dependence of exercise-induced R_2,i changes previously reported (7) are consistent with a chemical exchange mechanism of transverse relaxation (14). However, it should be noted that alternative explanations exist, at least in principle. Diffusion through magnetic field gradients such as those generated by heme-containing molecules would create a refocusing rate dependence of R₂. However, this mechanism cannot explain the present findings, as Carr and Purcell (36) showed that the effect of diffusion on R₂ should increase with the square of the echo spacing time; conversely, we observed larger R_2,i changes with lower echo spacing times. Also, while we did not measure the transmembrane water exchange rate explicitly, we consider that any such changes are unlikely, as there was no coherent pattern to the effect of pH_i on R_2,e and the permeability of the aquaporin-4 water channel is not affected by acidic deviations from neutrality (37). In light of these considerations, and taking into account also the correspondence of the R_2,i changes to the MT rate changes, we conclude that a pH_i-mediated effect on chemical exchange was the predominant mechanism of the transverse relaxation rate change.

The measured muscle MT and relaxation rates dependences on pH_i, with k_mf, k_fm, $R_1^{-}$, and R_2,i all showing small decreases with pH_i, are similar to those seen previously in BIS gel dosimeters with varying chemical side groups (31) and in 1-palmitoyl-2-oleoylphosphatidyl-choline linked with either cholesterol or galactocerebroside (38). This connection between k_mf and R₂ is consistent with a third small pool of interfacial protons providing a connection between the free water and macromolecular proton pools and effecting both MT and relaxation. However, a simple three pool model would predict that (ΔR₂)/(Δk_mf) would be roughly equal to the pool size ratio (31). Our results for (ΔR_2,i)/(Δk_mf) depend on the echo spacing, but are always at least an order of magnitude greater than the pool size ratio. These results preclude using a simple three pool model for explaining the relaxation and MT results. Instead, they are consistent with the possible existence of polyprotic acids and pH-induced alterations in protein structure and interfacial water content discussed above.

Conclusions

We have shown that pH_i affects the intracellular muscle R_2,i without significant changes in either the muscle density or the ratio of macromolecular to free protons. Further, the R_2,i change occurs regardless of TE spacing and with both non-localized and localized CPMG pulse sequences; however, shorter TE spacings are more sensitive to pH_i changes than long TE spacings. There are corresponding changes in the MT properties of the muscle, with decreases in k_mf and k_fm with decreasing pH_i. These changes suggest a role for amide proton exchange in the MT and transverse relaxation processes in muscle, and that these processes are modulated by based-catalyzed exchange and/or changes in protein structure. Comparison of the MT and transverse relaxation data reveals that transverse relaxation in muscle cannot be explained by a simple three-pool model.