Proximal Femoral Anatomy in the Normal Human Population

Abstract

In this study, we developed a complete description of the morphology of the proximal femur. Then, using this framework, we (1) determined normal population means, standard deviations, and ranges; (2) established differences among subpopulations; and (3) showed correlations among the various measurements. To accomplish these objectives, we analyzed 375 adult femurs. Specimens were digitally photographed in standardized positions, measurements being obtained using ImageJ software. Three parameters of the head-neck relationship were assessed. Translation was examined through four raw offset measurements (anterior, posterior, superior, inferior) used to calculate anterior-posterior and superior-inferior ratios. Rotation was investigated through anteroposterior (AP) and lateral physeal angles. Concavity was examined using alpha, beta, gamma, and delta angles. Two parameters of the neck-shaft relationship were assessed, neck version and angle of inclination. Average anterior-posterior and superior-inferior ratios were 1.14 and 0.90. Average AP and lateral physeal angles were 74.33° and 81.83°, respectively. Averages for alpha, beta, gamma, and delta angles were 45.61°, 41.85°, 53.46°, and 42.95°, respectively. Average neck version and angle of inclination were 9.73° and 129.23°, respectively. Differences existed between males and females and between those younger and older than 50 years. Correlations were observed between translation and concavity, and translation and the neck-shaft relationships.

Level of Evidence: Level II, prognostic study. See the Guidelines for Authors for a complete description of levels of evidence.

Introduction

The morphology of the proximal femur, specifically the relationships among the head, neck, and proximal shaft, has been a subject of interest and debate in orthopaedic literature dating back to at least the middle of the 19th century [2]. As an area susceptible to numerous pediatric and adult disorders, many of which may correlate with variations in this morphology or whose treatment might benefit from a detailed understanding of this area’s anatomy, a substantial body of research aimed at academically defining and pragmatically measuring the proximal femur’s dimensions has developed. These efforts have led to a robust vocabulary for discussing proximal femoral anatomy and abundant methods for its quantification through various linear and angular measures.

Perhaps as a result of their ease of appreciation, the earliest efforts to quantify the proximal femur beyond mere description of its components began with examining the relationship between the femoral shaft and neck. Two well-known parameters have long defined this relationship, angle of inclination (also referred to as neck-shaft angle) and neck version. Although the former of these two measurements has a widely accepted theoretical definition, average value (135°), and standard radiographic method of determination [9], the latter has produced more than a century’s worth of investigation regarding its true definition, normal values, and preferred method of measurement [1, 3–5, 8, 11, 16, 20–23, 26, 29]. Regardless of the volume of research each has generated, however, both are firmly grounded statutes of modern orthopaedics.

Although the relationship between the shaft and neck of the femur has been quantitatively scrutinized by numerous authors for more than a century, critical evaluation of the head-neck relationship is still in relative infancy. Although an abnormal relationship between the head and neck had been a speculated cause of impingement, joint destruction, and osteoarthritis [6, 7, 15, 17, 18, 25, 27, 28] since the mid-20th century, only more recently have efforts been made to quantify this relationship beyond the qualitative descriptions provided by Murray [17] and Stulberg et al. [27]. Specifically, from the desire to further investigate femoroacetabular impingement (FAI), two new classes of measurements have been devised during the last decade to evaluate the relationship between the femoral head and neck.

The first measurement, called the alpha angle, was devised by Nötzli et al. [19] and attempted to quantify the concavity of the anterior head-neck junction. Although only tested against a small sample, this measurement was successful in differentiating individuals with FAI resulting from deficient concavity of the anterior head-neck junction from matched controls. Such results tentatively support the use of the alpha angle for continued quantification of the head-neck relationship.

The second measurement, head-neck offset, recently was quantified [10, 24]. This linear measurement can be used to determine the position of the head relative to the borders of the femoral neck in a plane perpendicular to the femoral neck axis. Like the alpha angle, it has been successful in differentiating individuals with FAI resulting from deficient offset of the anterior head-neck junction from matched controls and is a promising method for quantification of an aspect of the head-neck relationship.

Despite the consideration given to describing and defining proximal femoral anatomy by previous authors, there are at least two essential informational deficiencies in the available literature. First, with perhaps the exception of neck version, there has yet to be a published study that rigorously determined the normal values and degrees of variability in a healthy (normal) population for the defining characteristics of the proximal femur. Second, to the knowledge of the senior authors (AS, DRC), no description exists regarding the angular relationship between the capital physeal scar and the femoral neck axis, a measure that would quantify rotation movements of the head on the neck. The purpose of our study, therefore, was to combine the existing measurements of the proximal femur (or modifications and expansions of these measures as necessary) with an original measure of femoral head rotation to create a complete description of the proximal femur’s anatomy and use this devised framework to produce a global assessment of this area’s morphology in healthy individuals. Specifically, our objectives were to (1) assess the relationship between the head and neck in terms of translation, rotation, and head-neck junction concavity; (2) assess the relationships between the neck and proximal shaft in terms of neck version and angle of inclination; (3) show what differences exist between various subpopulations based on gender and age; and (4) determine if major correlations exist between any of the various measurements.

Materials and Methods

The Hamann-Todd osteologic collection at the Cleveland Museum of Natural History consists of nearly 3000 disarticulated human skeletons gathered approximately at the beginning of the 20th century from the unclaimed dead of the Cleveland city morgue. We obtained 200 adult skeletons from this collection for this study. To select a study sample representing the normal human population, we diversified the sample in terms of race, age, and gender and excluded femora that were anatomically abnormal. Specifically, the initial sample was divided equally among genders and available races (50 white males, 50 white females, 50 black males, and 50 black females being selected at random from the collection’s sample of each subpopulation) and femora from individuals older than 18 years were included, excluding femora not fully mature at the time of death. Additionally, we excluded individual femora that on gross visual inspection appeared anatomically abnormal, ie, those affected by disease, such as arthritis, osteonecrosis, or other deformity. This yielded a total of 375 femora available for study (Table 1).

Table 1.

Sample selection and demographics

Subpopulation	Original number of femora	Number of abnormal femora	Final number of femora	Mean age (years)	Age range (years)
Entire population	400	25	375	44	18–89
Males	200	12	188	44	20–87
Females	200	13	187	43	18–89
Caucasians	200	19	181	47	18–87
African-Americans	200	6	194	40	20–89
Caucasian males	100	10	90	49	21–87
Caucasian females	100	9	91	45	18–80
African-American males	100	2	98	39	20–80
African-American females	100	4	96	42	20–89

Each of the specimens was digitally photographed in two standardized positions, termed AP and lateral. For the AP photographs, we first placed each pair of femora in a supine position on a flat laboratory bench with anterior surfaces directed toward the ceiling and femoral shafts parallel to one another. In this position, specimens rested distally on the convex surfaces of the medial and lateral condyles and proximally on the greater trochanter. The femoral neck then was made parallel to the superior surface of the laboratory bench by either rotating the femoral shaft internally and supporting the lateral condyle if the neck axis was anteverted or rotating the femoral shaft externally and supporting the medial condyle if the neck axis was retroverted. Parallelism between the femoral neck and laboratory bench was determined through visual inspection. The investigator taking the photographs (PAT) used square cards, approximately 1 mm in thickness, to increasingly support the medial or lateral condyle until the axis of the neck appeared parallel to the laboratory bench surface. By taking a photograph from directly overhead (camera lens parallel to the laboratory bench and femoral neck axis as confirmed by a level), we obtained accurate AP pictures; any potential distortion resulting from neck version was eliminated by making all components of the setup parallel. For the lateral photographs, we again placed each pair of femurs on the flat laboratory bench surface with anterior surfaces facing up. The femora then were abducted until the femoral necks were parallel with the plane produced by the edge of the laboratory bench. Parallelism again was determined through visual inspection. The investigator taking the photographs (PAT) increasingly abducted the femoral shafts until the axis of the neck appeared parallel to the laboratory bench edge from overhead. Additionally, each femur was checked to ensure the medial and lateral condyles rested on the surface of the laboratory bench distally, allowing the table surface to represent the transcondylar axis. By taking pictures with the lens of the camera parallel to the edge of the laboratory bench (as confirmed using a T-square ruler) and even with its surface (as confirmed through the camera’s view finder), we obtained accurate lateral images. Any distortion produced by the angle of inclination was eliminated by making the neck axis and camera parallel.

Using ImageJ software (nih.gov), we obtained 12 raw measurements from each specimen, six from each of the two views. These measurements were used to define three parameters of the head-neck relationship (translation, rotation, concavity) and two parameters of the neck-shaft relationship (neck version, angle of inclination).

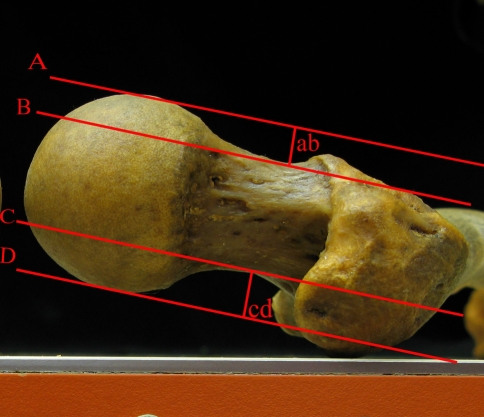
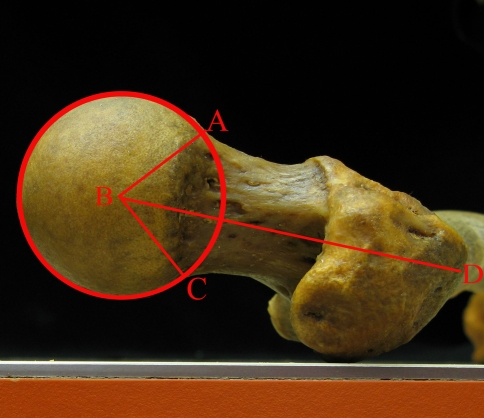
The first parameter of the head-neck relationship examined was head-neck translation. Although the femoral head often is pictured as centered on the axis of the femoral neck, this may not represent normal anatomy. Rather than being perfectly aligned on the neck axis, it is possible normal individuals regularly have minor shifts or translations of the head on the neck in the plane perpendicular to the neck’s axis in AP and/or superior-inferior vectors. To quantify these potential translational movements, four offset measurements were obtained based on descriptions by Ito et al. [10] and Siebenrock et al. [24]: anterior (AOS) and posterior (POS) offsets were acquired from the lateral photographs (Fig. 1); superior (SOS) and inferior (IOS) offsets were acquired from the AP photographs (Fig. 2). Each of these measurements was defined as the minimum distances between two lines drawn parallel to the femoral neck axis on each of the four cardinal surfaces. The femoral neck axis was produced by drawing a straight line that by visual inspection was equidistant from the anterior/posterior or superior/inferior borders of the femoral neck along the neck’s length. The two lines parallel to this axis, needed for the measurements of translation, then were drawn and visually positioned. The first line was drawn tangential to the convexity of the femoral head and the second tangential to the concavity of the femoral neck. The distance between these parallel lines represented the perpendicular distance separating these two contours on a single cardinal surface. We then used these four raw offset measurements to calculate two offset ratios: AOS/POS and SOS/IOS. Femurs with AOS/POS ratios equal to 1 would be offset equally anteriorly and posteriorly and thus have minimal translation in this vector. Similarly, femurs with SOS/IOS ratios equal to 1 would be offset equally superiorly and inferiorly, having minimal translation in this vector. However, femurs with AOS/POS and/or SOS/IOS ratios greater than 1 would be translated more anteriorly and/or superiorly, respectively, whereas femurs with AOS/POS and/or SOS/IOS ratios less than 1 would be translated more posteriorly and/or inferiorly, respectively. These two ratios thus defined translational movements of the femoral head on the neck in both major axes.

Fig. 1.

The AOS was defined as the perpendicular distance (ab) between Lines A and B. Line A was drawn parallel to the neck axis and tangential to the convexity of the femoral head; Line B was drawn parallel to the neck axis and tangential to the concavity of the femoral neck. Similarly, the POS was defined as the perpendicular distance (cd) between Lines D and C.

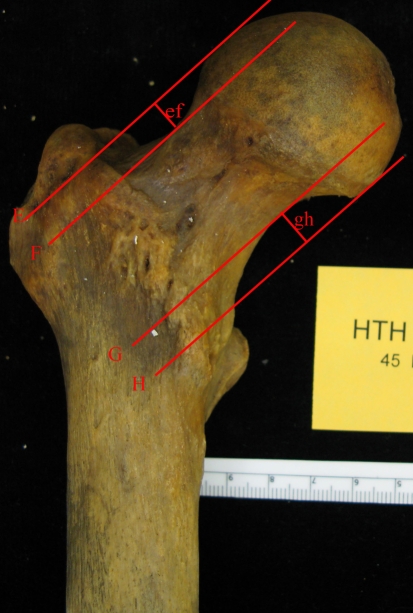
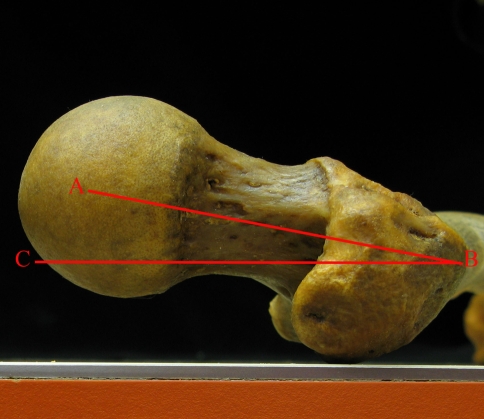
Fig. 2.

The SOS was defined as the perpendicular distance (ef) between Lines E and F. Line E was drawn parallel to the neck axis and tangential to the convexity of the femoral head; Line F was drawn parallel to the neck axis and tangential to the concavity of the femoral neck. Similarly, the IOS was defined as the perpendicular distance (gh) between Lines H and G.

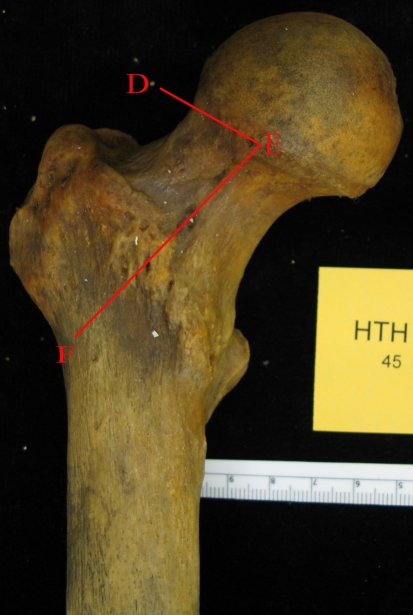
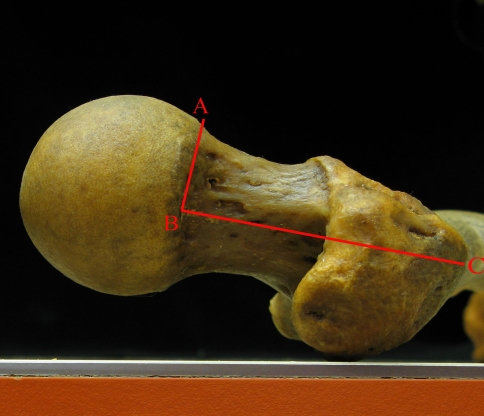
The second parameter of the head-neck relationship examined was head-neck rotation. Although common depictions of the femoral head-neck junction place the physeal scar perpendicular to the axis of the femoral neck, this may not represent normal anatomy. Rather, it is possible even in normal individuals that a certain amount of rotation of this scar (and therefore the femoral head) is present. To quantify these potential rotational movements, two original measurements, termed physeal angles, were devised, one from each of the two available views. The AP physeal angle was defined as the superior-lateral angle made between the intersection of the femoral neck axis, as defined previously, and a line representing the physeal scar in the AP pictures (Fig. 3), whereas the lateral physeal angle was similarly defined as the anterior-lateral angle made between these same lines in the orthogonal, lateral view (Fig. 4). The line representing the physeal scar also was produced by visual inspection and ignored any encroachment of the scar onto the femoral neck. Femoral heads with AP and/or lateral physeal angles equal to 90° would not be rotated with respect to the neck axis. However, femoral heads with AP and/or lateral physeal angles greater than 90° would be adducted and/or retroverted, respectively, whereas femoral heads with AP and/or lateral physeal angles less than 90° would be abducted and/or anteverted, respectively. These two angles thus defined rotations movements of the head on the neck in both major axes.

Fig. 3.

The anteroposterior physeal angle was defined as the acute, superior-lateral angle between Lines DE and EF. Line DE represented the physis; Line EF represented the neck axis.

Fig. 4.

The lateral physeal angle was defined as the acute, anterior-lateral angle between Lines AB and BC. Line AB represented the physis; Line BC represented the neck axis.

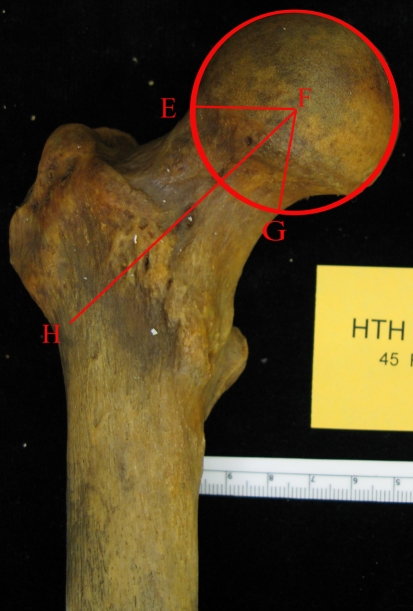
The third and final parameter of the head-neck relationship examined was head-neck junction concavity. Although an idealized femoral head is nearly spherical even as it meets the femoral neck, normal sphericity at the head-neck junction may differ from this idealized conception and among individuals. To quantify sphericity of the head as it joins the neck, four measures of the concavity of this junction, expanded from the description by Nötzli et al. [19], were determined, two (alpha and beta angles) from the lateral view (Fig. 5) and two (gamma and delta angles) from the AP view (Fig. 6). The alpha angle defined the extent of the concavity at the anterior head-neck junction. It was produced by the intersection of two lines. The first was the femoral neck axis. The second was a line formed by connecting two points on the femoral head; Point B was the center of the femoral head as found by ImageJ after visually inscribing a perfect circle around an ideally spherical femoral head and Point A was the point where the anterior cortical surface of the head-neck junction first exited the same perfect circle. The beta, gamma, and delta angles were similarly used to define the concavities of the posterior, superior, and inferior head-neck junctions, respectively. Smaller angles represented concave head-neck junctions and therefore nearly spherical femoral heads. Progressively larger angles represented junctions with increasingly less concavity, ranging from mild flattening of the junction to severe extension of the junction beyond the limits of a spherical head. These four angles thus defined the extent of concavity of the head-neck junction in four cardinal locations.

Fig. 5.

The alpha angle was defined as the acute angle between Lines AB and BD. Line AB was formed by connecting Point B (the center of the femoral head) with Point A (the point where the cortical surface of the head-neck junction first exited a perfect circle drawn around an ideally spherical femoral head); Line BD represented the neck axis. Similarly, the beta angle was defined as the acute angle between Lines BC and BD.

Fig. 6.

The gamma angle was defined as the acute angle between Lines EF and FH. Line EF was formed by connecting Point F (the center of the femoral head) with Point E (the point where the cortical surface of the head-neck junction first exited a perfect circle drawn around an ideally spherical femoral head); Line FH represented the neck axis. Similarly, the delta angle was defined as the acute angle between Lines FG and FH.

The first parameter of the neck-shaft relationship examined was neck version (Fig. 7). This angle was measured from an accurate lateral view, the femoral shafts being abducted allowing the neck axis to be parallel with the camera. It was created by the intersection of the neck axis and transcondylar plane (plane of the laboratory bench) and defined the degree to which the neck was above or below the plane created by the condyles. Angles superior to this plane were arbitrarily positive and angles below it negative.

Fig. 7.

The neck version was defined as the acute angle between Lines AB and BC. Line AB represented the neck axis; Line BC represented the transcondylar axis produced by the superior surface of the laboratory bench.

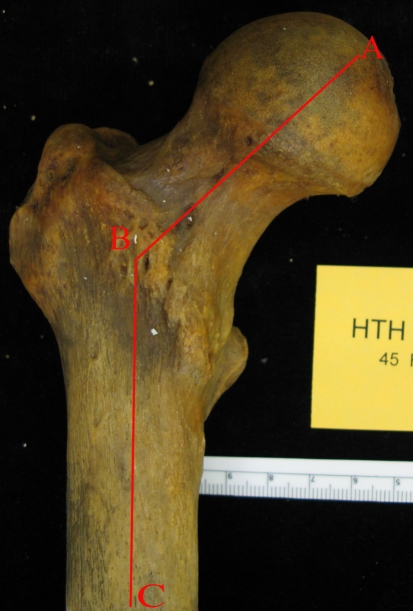
The second parameter of the neck-shaft relationship examined was angle of inclination (Fig. 8). This angle was measured from an accurate AP view, the femoral neck axes being parallel to the camera’s lens. It was created by the intersection of the anatomic axes of the femoral neck and shaft and thus defined the position of the neck relative to the shaft in the accurate AP view. Similar to the method for defining the femoral neck axis, the axis of the proximal shaft was produced by visual inspection and drawn such that it would be equidistant from the medial and lateral cortical surfaces of the shaft along its length.

Fig. 8.

The angle of inclination was defined as the acute angle between Lines AB and BC. Line AB represented the neck axis; Line BC represented the femoral shaft.

Ranges, means, and standard deviations were determined for each of the measurements made for the population as a whole and for the various subpopulations based on gender and age (younger or older than 50 years at the time of death). We used paired Student’s t tests to establish the significance of any noted differences and post hoc power analysis, which revealed a power greater than 0.90 for all examined differences, was used to confirm the adequacy of the sample size. Pearson’s coefficients were calculated to examine correlations between variables.

Results

Examining the three parameters defining the relationship between the femoral head and neck revealed, for the entire sample, the femoral head tended to be translated anteriorly and inferiorly, rotated in abduction and anteversion, and had greater concavity posteriorly and inferiorly (Table 2). Specifically, because the average AOS/POS ratio for the entire sample was greater than 1 (mean, 1.14), the femoral heads of the population were, on average, translated anteriorly. Similarly, because the average SOS/IOS ratio for the sample was less than 1 (mean, 0.90), the femoral heads of the population were, on average, translated inferiorly. Because the average AP physeal angle for the sample was less than 90° (mean, 74.33°), the femoral heads of the population were, on average, abducted. Similarly, because the average lateral physeal angle was less than 90° (mean, 81.83°), the femoral heads of the population were, on average, anteverted. Finally, because the average alpha and gamma angles (means, 45.61° and 53.46°, respectively) were greater than the average beta and delta angles (means, 41.85° and 42.95°, respectively), the femoral head-neck junctions had, on average, greater concavity posteriorly and inferiorly than anteriorly and superiorly.

Table 2.

Summary of measurements for the entire sample

Measurement	Mean	Standard deviation	Range
AOS/POS	1.14	0.40	0.43–3.78
SOS/IOS	0.90	0.39	0.16–2.66
AP physeal angle	74.33°	7.04°	55.04°–98.00°
Lateral physeal angle	81.83°	6.96°	55.19°–102.04°
Alpha angle	45.61°	10.46°	16.87°–78.57°
Beta angle	41.85°	6.92°	22.06°–71.21°
Gamma angle	53.46°	12.68°	31.21°–111.50°
Delta angle	42.95°	4.86°	26.83°–60.80°
Neck version	9.73°	9.28°	−14.63°–35.90°
Angle of inclination	129.23°	6.24°	105.65°–146.29°

AOS = anterior offset; POS = posterior offset; SOS = superior offset; IOS = inferior offset; AP = anteroposterior.

Examining the two parameters defining the relationship between the femoral neck and proximal shaft showed, while neck version and angle of inclination had average results similar to those typically seen clinically [12], both also had ranges including values well beyond these dogmatic means (Table 2). Specifically, although the population’s average neck version (mean, 9.73°) and angle of inclination (mean, 129.23°) were similar to the 10° and 130° listed in Koval and Zuckerman’s Handbook of Fractures [13], both also had ranges greater than 40°.

Evaluating the data for differences based on gender and age revealed males and females varied in at least one parameter of translation, rotation, and concavity, whereas those younger and older than 50 years varied in only one measure of concavity (Table 3). Specifically, regarding translation, with a smaller SOS/IOS ratio (p < 0.01), males had, on average, more inferior offset than females. Regarding rotation, with smaller AP and lateral physeal angles (p < 0.01), males had, on average, more abduction and anteversion than females. Finally, regarding concavity, with a larger alpha angle (p < 0.01), males and those older than 50 years had, on average, less concavity of the anterior head-neck junction (and so less sphericity of the femoral head) than females and those younger than 50 years. No differences based on gender or age were found to be important for either of the two measurements defining the neck-shaft relationship.

Table 3.

Differences based on gender or age

Measurement	Gender/age	Mean	Standard deviation	p Value	Power
SOS/IOS	Male	0.84	0.37	< 0.01	0.91
	Female	0.97	0.39	< 0.01	0.91
AP physeal angle	Male	72.75°	6.74°	< 0.01	0.99
	Female	75.93°	7.01°	< 0.01	0.99
Lateral physeal angle	Male	80.66°	7.23°	< 0.01	0.91
	Female	83.01°	6.49°	< 0.01	0.91
Alpha angle	Male	47.50°	10.71°	< 0.01	0.94
	Female	43.71°	9.88°	< 0.01	0.94
Alpha angle	< 50 years	43.70°	9.68°	< 0.01	0.99
	> 50 years	49.62°	10.93°	< 0.01	0.99

SOS = superior offset; IOS = inferior offset; AP = anteroposterior.

Consistent correlations existed among measures of translation and head-neck junction concavity and each translation ratio and a measure of the neck-shaft relationship. Regarding the relationship between translation and concavity, as translation increased in a particular cardinal direction, concavity tended to increase at the corresponding head-neck junction (Table 4). Specifically, as the AOS/POS ratio became larger (increased anterior translation), the alpha angle became smaller (increased anterior concavity). Similarly, as the AOS/POS ratio became smaller (increased posterior translation), the beta angle became smaller (increased posterior concavity). Likewise, as the SOS/IOS ratio became larger (increased superior translation), the gamma angle became smaller (increased superior concavity) and, as the SOS/IOS ratio became smaller (increased inferior translation), the delta angle became smaller (increased inferior concavity). Regarding the relationship between translation and the neck-shaft measurements, as either neck version or angle of inclination increased, translation in the same direction (anterior for neck version, superior for angle of inclination) at the head-neck junction tended to decrease (Table 4). Specifically, as neck version increased, the AOS/POS ratio decreased and, similarly, as the angle of inclination increased, the SOS/IOS ratio decreased. No other consistent correlations existed between any other measures.

Table 4.

Correlations between measurements of translation and concavity/neck-shaft

Translational measurement	Concavity/neck-shaft measurement	Pearson’s coefficient (R)
AOS/POS	Alpha angle	−0.50
AOS/POS	Beta angle	0.52
SOS/IOS	Gamma angle	−0.50
SOS/IOS	Delta angle	0.73
AOS/POS	Neck version	−0.38
SOS/IOS	Angle of inclination	−0.35

AOS = anterior offset; POS = posterior offset; SOS = Superior offset; IOS = inferior offset.

Discussion

Despite the existence of a substantial body of literature dedicated to describing and defining the anatomy of the proximal femur, a quantitative, global examination of the relationships among the shaft, neck, and head had yet to be produced. With this informational deficiency as an impetus, we attempted to (1) assess the relationship between the head and neck in terms of translation, rotation, and head-neck junction concavity; (2) assess the relationships between the neck and proximal shaft in terms of neck version and angle of inclination; (3) show what major differences exist between various subpopulations based on gender and age; and (4) determine if major correlations exist between any of the various measurements.

This study had certain limitations. With regard to data collection, the available specimens were approximately 100 years old, and thus these individuals lived during a period when rickets and other bony abnormalities were more likely to develop and go untreated. In addition, it is unknown how differences in lifestyle and general nutrition might affect the generalizability of these results to contemporary populations. Also, regarding the clinical applicability of these results, the methods of measurement for a cadaveric sample, which allow observation of an entire, cleaned bone, obviously cannot be used in living patients. Although the necessary imaging technology currently exists to obtain these measurements from patients, before these data can be translated into clinical practice, such images must become routine. Specifically, although previous authors have used study-specific MRI sequences to determine measurements of translation and concavity [10, 19, 24], such imaging sequences are not used routinely in the contemporary evaluation of patients. Many institutions do not insist on absolutely eliminating version when obtaining plain AP films and thus do not collect accurate AP views as we used in this study. Until collection of derotated plain films becomes routine, the clinical applicability of the data from this study is somewhat limited.

Although comparative data were not available for the unique measures in this study (AOS/POS, SOS/IOS, AP physeal angle, lateral physeal angle, beta angle, gamma angle, delta angle), the similarity between our results and those of others was very good when such data did exist. The average alpha angle reported by Nötzli et al. [19] for healthy controls (without FAI) was 42°, similar to our 45.61°. The average neck version as reported by Kinsley and Olmsted [11] was 8.021°, similar to our 9.73°. The average angle of inclination as reported by Hoaglund and Low [9] was 135°, similar to our 129.23°. These results suggest the validity of our data in general and substantiate the novel method by which it was collected.

In addition to providing benchmark data, the previously unestablished measures of femoral head translation and rotation contest certain assumptions of the head-neck junction. Although the femoral head often is depicted as centered on the neck, our data suggest its true position is more often slightly anterior and inferior. Similarly, although the physeal scar might be assumed to be perpendicular to the axis of the neck, our results show it more often is substantially anteverted and abducted. Such findings question the validity of modern surgical techniques not taking such head-neck variables into account. As an example, femoral head resurfacing, a bone-conserving alternative to traditional THA, relies on the assumptions of negligible translation and rotation (AOS/POS and SOS/IOS ratios of 1, AP and lateral physeal angles of 90°) for positioning of the resurfacing prosthesis. Data from this study suggest, however, such idealized positioning may not reproduce an individual’s native anatomy, potentially reducing the lifespan of the prosthesis and altering biomechanical and patient function.

Also of concern to modern surgical technique were the large standard deviations and ranges recorded for the various measures, exemplified by the values obtained for neck version and angle of inclination (Table 2). Although the average values for these parameters were similar to those historically accepted (10° and 130°, respectively), their standard deviations were substantial (9.28° and 6.24°, respectively) and their ranges included values well beyond what modern prostheses currently are able to reproduce. Such results highlight the degree of variability likely to be encountered in a surgical population and challenge surgeons to be mindful of the impact that individual anatomic variation might have on outcomes for procedures not taking this variability into consideration.

The generated data also showed previously undescribed major differences between genders and ages in all three parameters of the head-neck junction. Regarding translation, males more often had inferior positioning than females. Similarly, with respect to rotation, males had, on average, more abduction and anteversion. Finally, examining concavity, males and those older than 50 years had, on average, larger alpha angles (and so less concavity) than females and those younger than 50 years, respectively. Given the higher incidence of osteoarthritis of the hip in elderly men [14], these results reinforce the results of numerous studies, which implicate abnormalities of the anterior head-neck junction in this condition [6, 7, 10, 15, 17–19, 24, 25, 27, 28]. And more broadly, observation of these anatomic differences raises questions of the need for recognition of these differences during THA and whether such differences could be correlated to the incidence of various disorders among genders and ages. Although disorders were consciously excluded from our study sample, their correlation to these gender- and age-dependent variations may be grounds for further research.

Finally, because this study collected multiple dimensions of the proximal femur simultaneously, numerous formerly unexamined correlations could be investigated, yielding noteworthy results. First, each of the four measures of concavity correlated with a measure of translation. Specifically, as the AOS/POS ratio increased, and thus the femoral head became more anteriorly positioned, the alpha angle decreased (more concave anteriorly) while the beta angle increased (less concave posteriorly). Similarly, as the SOS/IOS ratio increased, and thus the femoral head became more superiorly positioned, the gamma angle decreased (more concave superiorly) while the delta angle increased (less concave inferiorly). The explanation for these results may be that there are two sources of decreased concavity at the head-neck junction, only one of which is taken into account by the alpha, beta, gamma, and delta angles. The first, and perhaps intended, source these angles are supposed to detect is abnormal extension of the epiphysis onto the femoral neck (Fig. 9). The second source is decreased offset (Fig. 10). Regardless of the amount of epiphyseal encroachment, smaller offsets seemingly decrease concavity as determined by these angles. This result does not refute the usefulness of these measures for further clinical application but does suggest they would be more accurately interpreted if considered concurrently.

Fig. 9.

This specimen had a decrease in anterior concavity resulting from the encroachment of the epiphysis on the femoral neck.

Fig. 10.

This specimen had a decrease in anterior concavity resulting from a small anterior offset.

Second, both measures of translation correlated with a defining parameter of the neck-shaft relationship. Specifically, as neck version and angle of inclination increased, the AOS/POS and SOS/IOS ratios decreased, respectively; the position of the femoral head relative to the neck thus somewhat compensating for increasingly severe angles between the neck and shaft. Although speculations regarding the etiology of these correlations are provisional, the neutralizing nature of the translations and lack of major differences in translation, neck version, and angle of inclination between populations younger and older than 50 years perhaps suggests an embryologic or developmental origin. Also, although previous research has not shown a correlation between angle of inclination and slipped capital femoral epiphysis [12], our results suggest the relationship between angle of inclination and the development of a translational disorder such as slipped capital femoral epiphysis might be worth revisiting.