Foveal contour interactions and crowding effects at the resolution limit of the visual system

Abstract

We describe several experiments on contour interactions and crowding effects at the resolution limit of the visual system. As test stimuli we used characters that are often employed in optometric practice for testing visual acuity: Landolt C's, Snellen E's and rectangular gratings. We tested several hypotheses that have been put forward to explain contour interaction and crowding effects. In Experiment 1 and Experiment 2, Landolt C's were the test stimuli, and bars, or Landolt C's, or gratings served as distractors. In Experiment 1, we showed that neither scale invariance, nor spatial frequency selectivity are characteristic of foveal crowding effects. These results allowed us to conclude that mechanisms other than lateral masking contribute to observers' performance in ‘crowded’ tasks. Hess, Dakin & Kappor (2000) suggested that the spatial-frequency band most appropriate for target recognition is shifted by the surrounding bars to higher spatial frequencies that cannot be resolved by observers. Our Experiment 2 rejects this hypothesis as the experimental data do not follow theoretical predictions. In Experiment 3 we employed Snellen E's both as test stimuli and as distractors. The masking functions were similar to those measured in Experiment 1 when the test Landolt C was surrounded by Landolt C's. In Experiment 4 we extended the range of test stimuli to rectangular gratings; same-frequency or high-frequency gratings were distractors. In this case, if the distracting gratings had random orientation from trial to trial, the critical spacing was twice larger than in the first three experiments. If the orientation of the distractors was fixed during the whole experiment, the critical spacing was similar to that measured in the first three experiments. We suggest that the visual system can use different mechanisms for the discrimination of different test stimuli in the presence of particular surround. Different receptive fields with different spatial characteristics can be employed. To explain why crowding-effects at the resolution limit of the visual system are not scale invariant, we suggest that a range of stimuli, slightly varying in size, may all be processed by the same neural channel – the channel with the smallest receptive fields of the visual system.

Introduction

Stimuli that are localised in space, and located near to one another, interact in psychophysical tasks. This is the case both for stimuli having a limited spatial frequency range (i.e., gratings with a limited number of cycles, Gabor patches) and for stimuli not narrowly tuned in the spatial frequency domain (i.e., alphanumeric characters). Depending on the parameters of the stimulus, these interactions can be both inhibitory and facilitative.

In the case of narrowly tuned stimuli, the most often cited study is that by Polat and Sagi (1993). They showed that the detection threshold of a test Gabor patch flanked by two laterally displaced Gabor signals depends on the separation between the test and the flanks: at small separations, detection is impaired, but with increasing spatial separation, facilitation of detection is found. This kind of interaction (i) is selective for orientation and for spatial frequency (if the masking Gabor patches differ in orientation or in spatial frequency from the test patch, then masking is much weaker); and (ii) scales with the test frequency (the spatial extent of the interaction is constant if expressed in periods of the test spatial frequencies). The authors attributed these interactions to a pattern of excitatory-inhibitory connections in the space or spatial-frequency domains (Polat & Sagi, 1993).

In the case of broad-band stimuli (e.g. letter opto-types), impaired performance has also been found when the subjects were required to identify a test letter in the presence of nearby objects. This phenomenon is referred to as a ‘crowding effect’ and the objects causing the deterioration are called distractors. Dr. H. Strasburger (personal communication at 18^th ECVP in Tubingen, 1995) traced the usage of the term ‘crowding’ to H. Ehlers who in 1936 (Ehlers, 1936) distinguished the separations needed for reading when the letters are isolated and when they are ‘in close type (provided the reading distance and the size of types are the same)’; and in 1953 Ehlers wrote that ‘if the visual field is crowded with letters, the area of the visual field in which the letters can be recognized narrows’ (Ehlers, 1953). Later Stuart and Burian (1962) adopted the term ‘crowding phenomena’ instead of ‘separation difficulty’. At present, many other words are used to describe the impaired perception of letters surrounded by different types of distractors; and conversely, the word ‘crowding’ is applied to various visual phenomena not involving letter discrimination (for example, see Parkes et al. (2001)). Alternative terms include lateral masking or lateral interference (Huckauf, Heller, & Nazir, 1999; Taylor & Brown, 1972; Townsend, Taylor, & Brown, 1971; Wolford & Chambers, 1983), mutual, or cognitive inhibition (Woodrow, 1938), lateral inhibition (Townsend et al., 1971), and contour interaction (Flom, Weymouth, & Kahneman, 1963b; Jacobs, 1979; Kooi, Toet, Levi, & Tripathy, 1992). When researchers talk about letter recognition, all of the above terms are used as synonyms, although Flom (1991) made a distinction between contour interactions, which he considered as a loss of information within the visual system, and crowding effects, which are more complex phenomena and include both contour interactions and attentional factors. For simplicity, we follow this distinction in our paper and refer to crowding effects when talking about complex surrounds (letters etc.) and contour interaction when the distractors are simple bars. However, under ‘crowding phenomena’ we shall include all types of distractors.

Since the studies of Ehlers (1936, ​1953) and Woodrow (1938), contour interaction and crowding effects have been studied both in central and peripheral vision, and for small or large characters surrounded by various distractors. Good reviews can be found in several papers (Chung, Levi, & Legge, 2001; Levi, Klein, & Hariharan, 2002b; Pelli, Palomares, & Majaj, 2004; Strasburger, Harvey, & Rentchler, 1991).

In our present paper we consider a very specific class of stimuli that are of practical interest, since they are all used as optotypes in optometry charts. In a series of experiments we studied crowding effects and contour interactions with high-contrast Landolt C's, Snellen E's and rectangular gratings presented in the fovea at the resolution limit and surrounded by either similar letters, or gratings of different frequencies, or tangential bars. The work represents a summary of our experiments carried out in the period 1993-2005. The results were partially reported at ECVP and ARVO meetings in 1995-2002 and have been published in Russian journals.

At the resolution limit, Flom et al. (1963b) were the first to measure the observers' ability to report the orientation of a Landolt C as a function of the separation between the target and four tangential flanking bars that acted as distractors. They showed that the critical spacing in a contour interaction task extends over a distance approximately equal to the smallest letter size that a particular observer can resolve, and corresponds to 4-5 units of the minimal angle of resolution (MAR). As far as we know, there have been no comparative studies of contour interactions and crowding effects employing at the same time Landolt C's, Snellen E's and rectangular gratings.

Since we are interested in optotype-like foveal targets at the resolution limit, from the previous studies we will only mention two main characteristics of this particular case:

• 1) in normal vision the sizes of critical spacing are proportional to MAR units (Flom, 1991; Flom et al., 1963b; Hess & Jacobs, 1979; Jacobs, 1979);

• 2) viewing the test Landolt C with one eye and the tangential bars with the other eye does not eliminate contour interactions (Flom, Heath, & Takahashi, 1963a), a result suggesting cortical and not retinal origin.

Several hypotheses have been offered to explain the crowding phenomena. Woodrow (1938) suggested that the effect ‘depends upon the entailed distribution of attention and the amount of confusion or cognitive inhibition resulting from the spatial closeness and the degree of visibility of the letters’. Flom and his colleagues attributed the decline in resolution to one or more of the following factors: (1) optical spread of the retinal image; (2) interaction at the neural level; (3) conflict of tasks; (4) eye movements (Flom et al., 1963b). The extent of the interaction they related to the size of the receptive fields that are most sensitive to the test stimulus. Receptive fields increase with eccentricity; this fact can explain the increasing critical spacing when the targets move into the periphery.

Since impaired recognition of Landolt C's in the presence of laterally displaced bars or other distractors falls into the general category of visual masking, a number of authors have suggested (Bondarko & Danilova, 1995; Chung et al., 2001; Pelli et al., 2004) that effects similar to those recorded in the lateral masking paradigm (Polat & Sagi, 1993) also contribute to the impaired recognition of letters in contour interaction and crowding effects. If this hypothesis is true, two characteristic features should be observed: (i) spatial frequency selectivity of the interactions and (ii) spatial scaling of the critical spacing if expressed in relative units, i.e., periods of the test grating, or the critical spatial frequency in the case of letters.

A simpler explanation based primarily on the ‘physics of the stimulus’ was offered by Hess et al. (2000) to explain the contour interaction phenomenon at the resolution limit. They suggested that at a stage where spatial frequency selectivity takes place, in the presence of flanks, the spatial frequency critical for detection of the orientation of the test Landolt C is shifted towards higher frequencies that are beyond the resolution limit of the human visual system. This spatial frequency was theoretically suggested by Bondarko & Danilova (1997) and tested experimentally by Hess et al. (2000) and was found to be approximately 1.15-1.30 cycles per letter. Throughout the paper, we will call this frequency ‘the critical frequency’ (CF).

Despite many experiments on letter interaction in various visual displays and in reading, crowding phenomena continue to attract the attention of researchers, who mainly test the masking hypotheses (Chung et al., 2001; Levi, Hariharan, & Klein, 2002a; Levi et al., 2002b; Palomares, Cardazone, Green, Levi, & Pelli, 1998; Palomares, LaPutt, & Pelli, 1999; Pelli et al., 2004). These experiments employ letters of various sizes and contrasts filtered with different frequency filters, or employ spatial frequency selective stimuli in central or peripheral vision. They typically conclude that in central vision the crowding effect resembles ordinary lateral masking (Levi et al., 2002b), but that in peripheral vision it does not (Levi et al., 2002a; Pelli et al., 2004). However, neither of the latter studies measured performance in ‘crowded’ tasks for high-contrast targets at the resolution limit of the human visual system, targets such as Landolt C, Snellen E or rectangular gratings. Following the work of Flom and his colleagues, studies of foveal contour interactions and crowding effects have used targets such as a standard Landolt C (Hess & Jacobs, 1979; Jacobs, 1979), or letters (Loomis, 1978), or a stylized Landolt C (Nazir, 1992), or gratings (Latham & Whitaker, 1996). But in these studies the authors were most interested in differences between the central and peripheral performance and did not systematically study the same target as the distractors varied; nor did they compare different targets.

Our task in this paper is twofold. First, we use a target Landolt C surrounded by either bars, or Landolt C's, or gratings, to test the two above mentioned hypotheses: (a) that crowding is a form of lateral masking and (b) that the critical spatial frequency is shifted to irresolvable frequencies in the presence of nearby distractors. Experiment 1 was intended to check whether contour interactions and crowding effects exhibit the two characteristic features of lateral masking: Scaling of the interaction area with test size and selectivity for spatial frequency. Neither of these tests gave a positive result and we therefore reject the hypothesis that lateral masking mechanisms underlie contour interaction and crowding effects at the resolution limit. Experiment 2 tested the hypothesis of Hess et al. (2000) and its result showed that the physical explanation holds for a particular contour interaction case, when the test Landolt C is surrounded by 4 bars, but fails for the crowding effects.

Second, we extend our experiments to other types of targets – Snellen Es (Experiment 3) and rectangular gratings (Experiment 4). Experiment 4 reveals that when a rectangular grating with the same CF as the Landolt C is used as the test stimuli, the critical spacing is noticeably larger than in Experiment 1, but only for a surround with random varying orientation.

We conclude that even at the resolution limit of the visual system, the contour interaction and crowding effects are complex phenomena implying combination of several mechanisms.

Methods

Stimuli and apparatus

This section of the paper gives general overview of the apparatus and procedures used; the details (such as the viewing distance and the corresponding size of the pixels) will be given in each section of the paper. In Experiments 1, 2 and 3 stimuli were presented on a SONY 100 monitor (resolution 640×480), or a CTX PR711T monitor (resolution 1028×768) driven by a standard graphics board. In Experiment 2, a SONY 200PST monitor was driven at the resolution 1280×1024 by a VGS2/2 graphics board (Cambridge Research Systems). The refresh rate was at least 60 Hz. The viewing distance varied from 1.7 to 8.1 m and the corresponding pixel sizes were in the range 0.16 to 0.47 arcmin. Letters were presented as high-contrast black figures (5 cd/m²) on a bright background (either 70 cd/m², or 80 cd/m² or 210 cd/m²); this difference in the background luminance resulted in a contrast change only from 94 to 98 %). Experiments were run in a room dimly lit with tungsten bulbs.

Landolt C's, Snellen E's and rectangular gratings were used as stimuli. The test Landolt C either was presented alone, or it was surrounded by four tangential bars (Fig.1, a), as in the original experiments by Flom and co-authors (Flom et al., 1963b), or by four identical Landolt Cs (Fig.1, b), or by four rectangular gratings (Fig.1, c, d, e) of varying spatial frequency. The Landolt C was nominally constructed in the same way as for opticians' charts: The width of the letter and the size of the gap were equal to 1/5 the size of the letter, but because of the discrete size of the pixels and varying visual acuity of different observers, these proportions were not always held strictly. The rectangular gratings were surrounded by four identical rectangular gratings of the same (Fig.1, f) or higher (Fig.1, g) spatial frequency. Only Snellen E's (Fig.1, h) were used as distractors for the test Snellen E.

Figure 1.

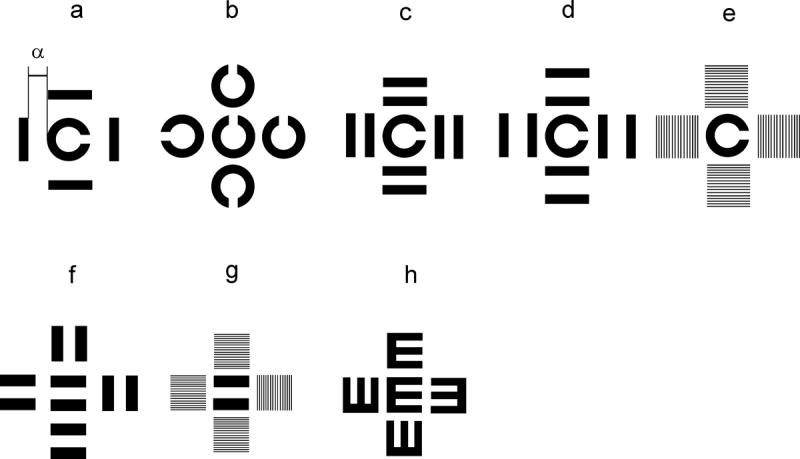
Examples of the stimuli used in the experiments.

Procedure

The observer's task was to detect one of four possible orientations of Landolt C's and Snellen E's (i.e. the location of the gap: top, bottom, left, right), or to discriminate between vertical or horizontal orientations for the rectangular gratings. The orientation of the test stimulus and the spacing α between the edges of target and distractors (defined as in Fig.1, a) varied randomly from trial to trial. Viewing was binocular; stimulus duration was 500 msec. Over different experimental days, we accumulated at least 100 presentations of each stimulus, i.e. the test stimulus and distractors at a particular separation or the single test stimulus without distractors. The size of the test stimulus was chosen individually for each observer so that the percent of correct responses to the isolated target exceeded random level, but did not reach 100%. We recorded the percent of correct responses. The critical spacings were defined by comparing the percent of correct responses obtained when the test stimulus was surrounded by distractors and the percent of correct responses to the isolated stimulus. Using a χ²-criterion or a t-test for mean percent correct, the critical spacing was defined as the spacing between the target and the distractors at which the two values differed significantly at the 5% level. The results of the two statistical tests do not contradict each other in our case. Experiments 1, 2, 3 and 4 are listed in chronological order.

Observers

For all experiments we recruited naïve observers, who were not aware of the purpose of the experiments: KM, IB, JS, IK, PF in Experiment 1, NK and KT in Experiment 2, EL, TA and IR in Experiment 3, AN, NN and OV in Experiment 4. The two authors also served as subjects in most of the experiments. All observers had normal or corrected-to-normal vision.

Experiment 1: Test of the lateral masking hypothesis

The first experiment tested whether crowding effects and contour interactions exhibit the two characteristic features of lateral masking shown by Polat & Sagi (1993). Landolt C's were used as targets. The assumption was that if the same mechanisms underlie spatial frequency masking and crowding effects then (1) the critical spacings should increase with increasing size of the test Landolt C (CF becomes lower, its period becomes larger (Bondarko & Danilova, 1997); (2) rectangular gratings having CF should produce maximal masking.

Therefore, we ran two experimental series: in one series, we varied the size of a test Landolt C surrounded by four tangential bars (Fig.1, a), in the second series we varied the spatial frequency of rectangular gratings surrounding the target Landolt C (Fig.1, c, d, e).

In the first series, the diameter of the test Landolt C was 10 pixels, and the viewing distance was varied to obtain different sizes of the test. Only one type of distractor was used – four tangential bars (Fig.1, a). The separation between the edges of the target and distractors varied in 1 pixel steps.

In the second series, the pixel size was 0.26 arcmin; the spacing α varied in steps of 2 pixels. Five types of distractors were used (Fig.1, a – e). Surrounding Landolt C's were identical to the target one (Fig.1, b). The width of the tangential bars (Fig.1, a) and lines forming rectangular gratings (Fig.1, c, d) was the same as the stroke width of the test Landolt C. Two types of rectangular gratings consisted of only two bars. The gaps were either equal to the width of the lines (Fig.1, c) or were twice larger (Fig.1, d). The latter grating had the CF of the Landolt C (Bondarko & Danilova, 1997). The orientation of the distractors was fixed from trial to trial (as shown in Fig.1). The spatial frequency of the third grating (Fig.1, e) was beyond the resolution limit: its frequency was 77 c/deg. The gap between its bars was equal to 1 pixel while the width of the bars was 2 pixels. This grating filled a square whose size was equal to the diameter of the Landolt C. The sizes of test Landolt C are given in the top row in Table 2 for each observer.

Table 2.

Critical spacing for five types of distractors (see also Fig.3)

Type of distractors	Sizes of the critical spacings
	PF (5.3 arcmin)	IK (3.1 arcmin)	VB (2.6 arcmin)	MD (3.1 arcmin)
CF 1:1	4.8/0.91	2.1/0.68	1.6/0.62	2.1/0.68
CF 1:2	5.3/1.0	2.1/0.68	2.1/0.81	2.6/0.84
High frequency	4.8/0.91	2.6/0.84	2.1/0.81	2.1/0.68
Bars	5.3/1.0	2.1/0.68	1.6/0.62	2.1/0.68
Landolt C	4.2/0.79	2.6/0.84	1.6/0.62	2.1/0.68

Fig. 2 shows the results of the first series where variable sizes of the test Landolt C were used for all 5 observers participating in the study. All the masking functions are U-shaped as was found by Flom et al. (1963b). Some observers (KM, VB) exhibit facilitation effects at large separations.

Figure 2.

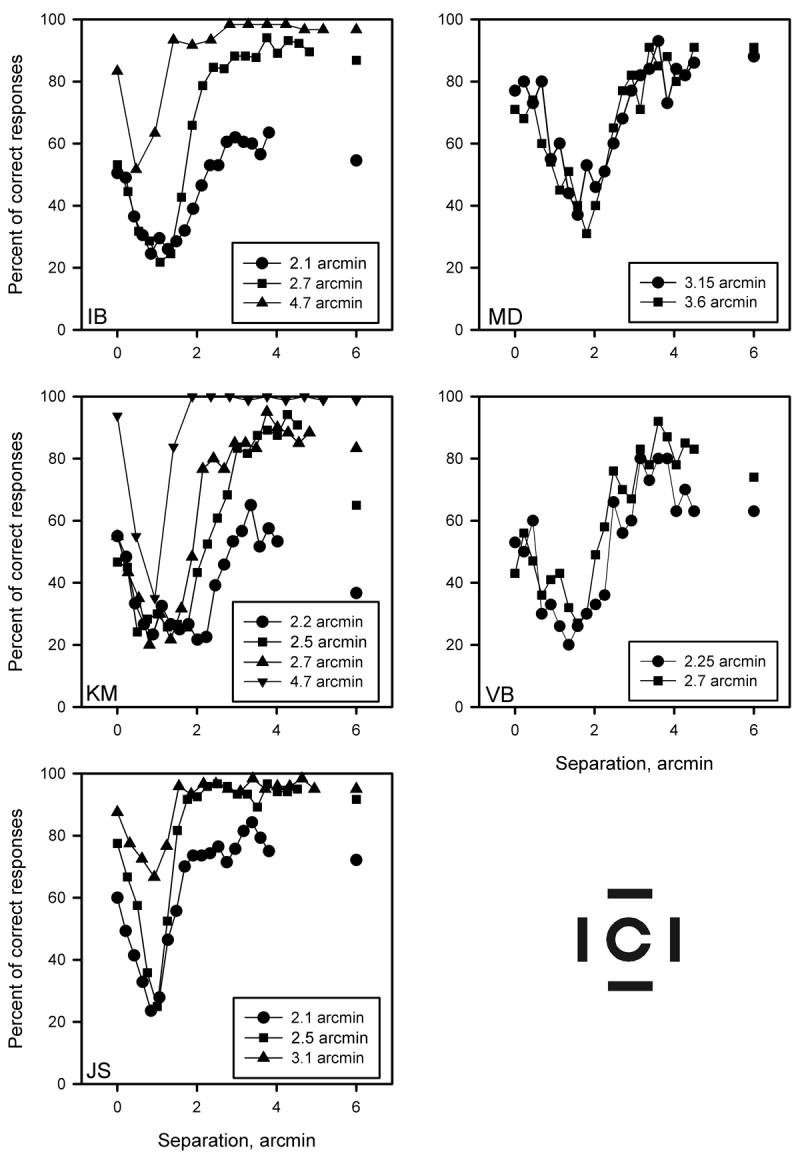
Percent of correct responses is plotted against spatial separation between the test Landolt C and four surrounding bars (see Fig.1, a) for five observers. The legends on each graph give the sizes of the test Landolt Cs.

The numerical data are shown in Table 1. The second column of Table 1 shows the size of the test Landolt C for each observer in arcmin. The third column gives the critical spacing in arcmin defined as described in Methods, using a χ²–criterion. The maximal confidence interval in this experiment (200 presentations) is 7% for a significant difference between the percent of correct responses to the isolated Landolt C and the percent correct for the same Landolt C surrounded by four bars. The maximal errors in defining the critical spacings are equal to ½ the step size which was 1 pixel in this set of Experiment 1. We give these numbers in the same column. The fourth and fifth columns show the relative sizes of the test stimuli and of the corresponding critical spacing. The relative values were calculated relative to the smallest test stimulus for each observer. The correlation between the fourth and fifth columns is significant (r=0.612, p < 0.05).

Table 1.

Sizes of test Landolt Cs and the corresponding critical spacing (see also Fig.2)

Observer	Size of Landolt C (arcmin)	Critical spacing (arcmin)	Relative size of Landolt C	Relative size of the critical spacing
JS	2.1	1.3±0.11	1.0	0.62
	2.5	1.0±0.12	1.19	0.48
	3.1	0.9±0.15	1.48	0.43
KM	2.2	2.2±0.11	1.0	1.0
	2.5	2.2±0.12	1.14	1.0
	2.7	1.9±0.13	1.23	0.86
	4.7	1.4±0.23	2.14	0.64
IB	2.1	1.9±0.11	1.0	0.9
	2.7	1.9±0.13	1.29	0.9
	4.7	0.9±0.23	2.24	0.43
VB	2.25	2.2±0.11	1.0	1.0
	2.7	1.8±0.11	1.23	0.82
MD	3.15	2.7±0.11	1.0	0.87
	3.6	2.5±0.11	1.19	0.81

Fig. 3 shows the result of the second series of the experiment for observers IK and PF; other observers show the same pattern of results. Table 2 gives numerical values for all the observers. Each column shows the critical spacing defined as described in Methods using a χ²–criterion for one observer and for all types of distractors. The first number in each cell is the absolute value in arcmin, the second number is the corresponding relative size (ratio between the critical spacing and the test Landolt C size). In this part of Experiment 1 the pixel size was the same for all observers and the spacing was varied with a step 2 of pixels; hence the maximal error in defining the critical spacing (½ size of the step size) was 0.26 arcmin. A one-way ANOVA showed that the factor ‘type of distractors’ is not significant (F[4]=0.59, p=0.67). The grating of CF did not produce a larger critical spacing or stronger masking, a result that contradicts the prediction of the spatial-frequency masking hypothesis.

Figure 3.

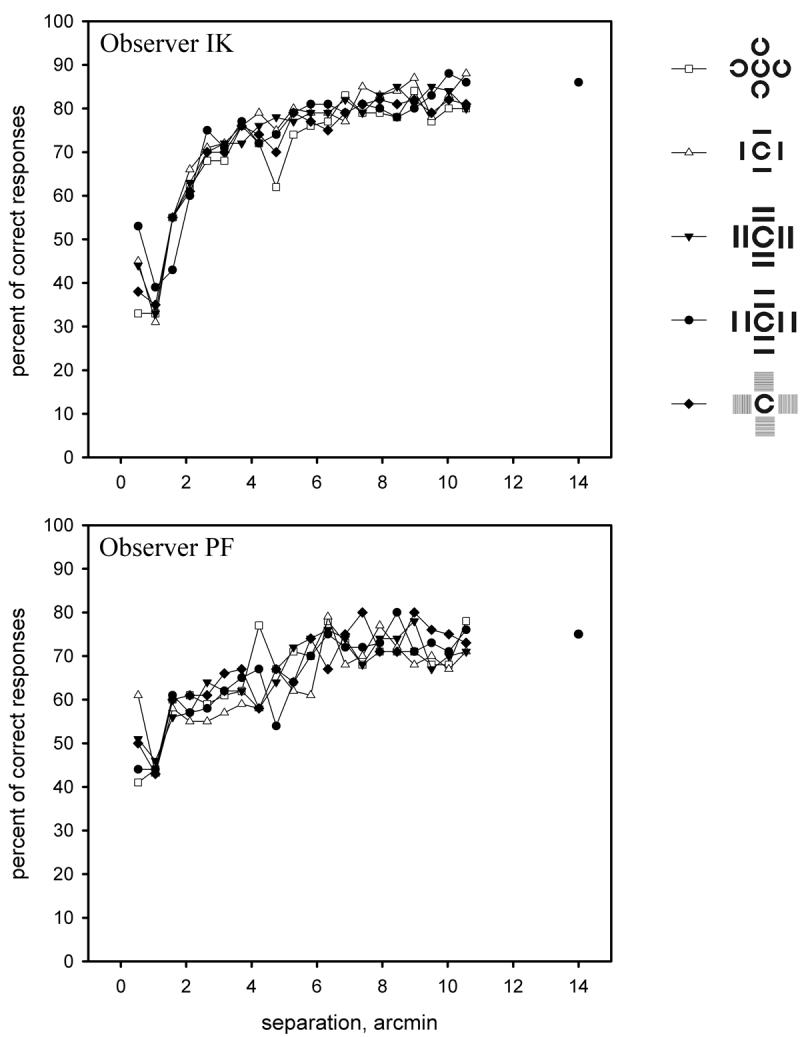
Percent of correct responses is plotted against spatial separation between the test Landolt and surrounding bars (Fig.1, a), or surrounding Landolt Cs (Fig.1, b), or rectangular gratings of different frequency (Fig.1, c, d, e).

To conclude, under our conditions, (i) the critical spacing did not scale with the target size, but decreased when we increased the target slightly above the resolution limit, and (ii) there was no selectivity for spatial frequency. We conclude that foveal crowding effects are unlike foveal lateral masking. Here we should mention that all the types of surroundings that we used were rather broad-band. This may be one of the reasons why we did not observe spatial-frequency selectivity of the crowding effect. Although the absence of spatial-frequency selectivity is not strictly proved in our Experiment 1, we did show that scale invariance fails to hold for foveal contour interactions.

Experiment 2: Can foveal crowding-effects be explained by physics?

Hess et al (2000) have suggested that contour interactions can be explained by considering the physics of the stimulus. These authors argued that in the presence of four tangential bars the CF – the spatial-frequency band most relevant for detecting the orientation of the isolated test Landolt C (upwards-downwards vs right-left) – in the presence of flanking bars is shifted towards higher frequencies and becomes unavailable to the observers, i.e., falls beyond their resolution limit. This frequency band peaks at 1.15-1.3 harmonics (Bondarko & Danilova, 1997). In the case when the band is shifted to lower frequencies or no shift occurs, there should not be impaired recognition. Hess et al. (2000) analysed only the case of contour interaction and only one separation, of 1 bar width (second plot from the top in Fig.4). In this condition their assumption was experimentally confirmed. To test their hypothesis for the case of the crowding effects and for a wider range of separations, we ran Experiment 2 which consisted of two parts: (i) we analyzed the difference spectra for the contour interaction case and for several fixed layouts in the crowding effect case and (ii) we experimentally tested the same layouts to see if psychophysical performance in both contour interactions and crowding-effects can be explained by the difference spectra.

Figure 4.

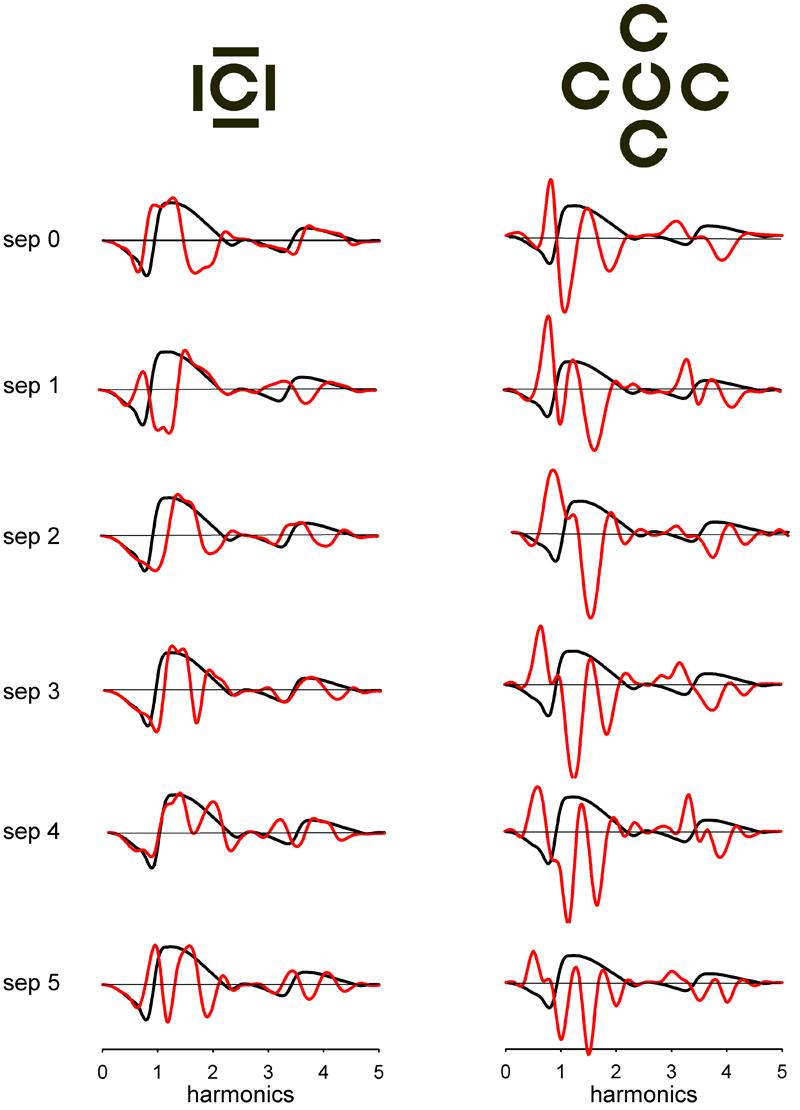
Amplitude difference spectra for a test Landolt C surrounded by four tangential bars, and one of the possible layouts of surrounding Landolt C's (all letters are rotated to the left). Separation between the test and the distractors increases from the top to the bottom from 0 separation (upper curves) to 5 bar widths (bottom curves). Black lines denote amplitude difference spectrum for the isolated Landolt C, red lines show amplitude difference spectrum for the same Landolt C in the presence of the surround.

Spectral considerations

We have analysed and psychophysically tested four patterns. Two of them are presented at the top of Fig. 4 and they are the first and the last patterns in Fig.5. The left panel in Fig.4 shows the same pattern as was used by Flom and his colleagues, by ourselves in earlier experiments (Bondarko & Danilova, 1996) and by Hess et al (2000) in both their analytical and their psychophysical study. The right panel in Fig.4 shows one of the possible layouts: all the distractors are rotated in the same direction (to the left in our case). There was no special preference in choosing this direction and this particular layout. The two other patterns (not shown in the Fig.4, but they are the second and the third patterns in Fig.5) differ in the layout of distractors: the distractors are symmetrically placed 4 Landolt C's with their gaps rotated inwards or outwards. All patterns were analysed for 6 values of α, the separation between the test Landolt C and the distractors. The separations were increased in steps equal to the size of the gap, from 0 (the case where the distractors are adjacent to the test Landolt C) to 5 (when the separation is equal to the outer diameter of the test Landolt C).

Figure 5.

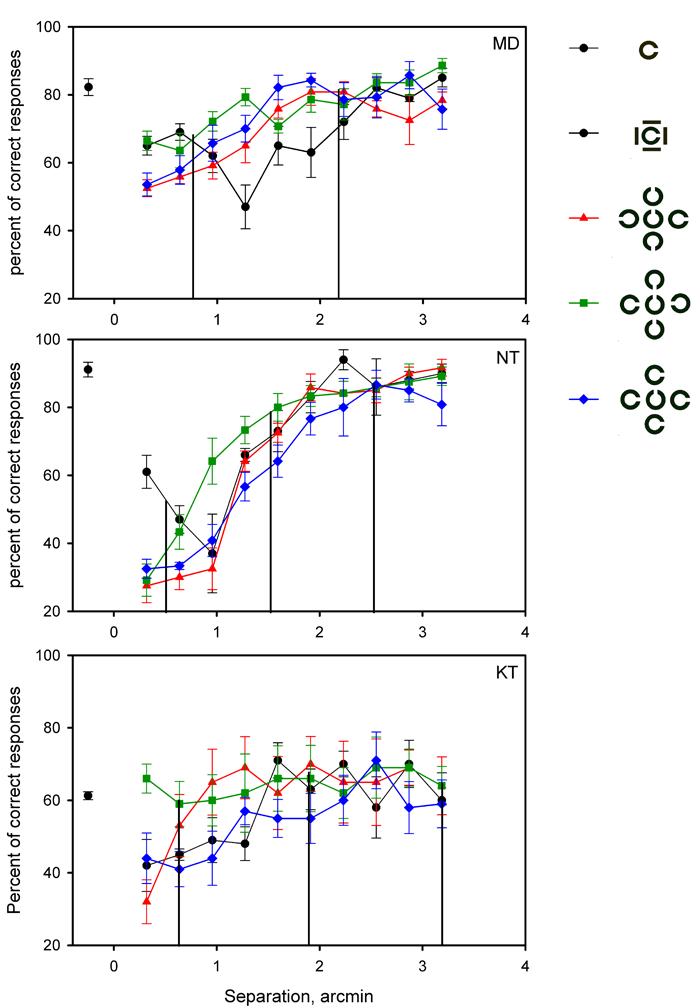
Percent of correct responses plotted against spatial separation for three observers (MD, NT and KT). The Landolt C was surrounded either by four tangential bars (black lines and black symbols), or by four Landolt C's rotated outwards (red lines and red symbols), or by four Landolt C's rotated inwards (green lines and green symbols), or by four Landolt C's facing left (blue lines and blue symbols). The vertical lines correspond to one, three and five gap widths. Error bars represent SEM.

As in our previous study, we calculated amplitude spectra taken in two directions: the direction containing the gap and the direction that does not have a gap. All images were created analytically and then two-dimensional Fourier spectra were calculated with equally spaced steps of frequency. The steps were equal to 1/6 the outer diameter of the test Landolt C. For more details on the method used for these calculations see Bondarko & Danilova (1997).

The graphs in Fig. 4 present the difference spectra for two patterns and for six separations. The abscissa shows the harmonic number, i.e., the spatial frequency relative to the frequency whose period is equal to the outer diameter of the Landolt C. The ordinate is the difference in amplitude spectra in relative units. In all these plots, the red curves show the difference spectra of the displays with varying separations α, and the black lines show the difference spectrum for an isolated Landolt C. In these plots CF corresponds to the largest peak in the difference spectrum and in the presence of distractors is not always equal to 1.2 harmonics as for the single Landolt C (black lines on the graphs). There are both positive and negative peaks in the difference spectra. The negative peaks reflect the case when the larger difference in the spectrum corresponds to the perpendicular orientation of the test Landolt C. This leads to confusion and the subjects will make incorrect discriminations between horizontal and vertical orientations. The difference spectra for the two symmetrical patterns are not shown as they follow the same pattern as was calculated for the single Landolt C.

Contour interaction

What predictions could be made from considering the difference spectra in the contour interaction case (left panel in Fig.4)? According to Hess et al (2000), separation 0 should not produce any masking (in the presence and in the absences of the distractors the peaks in the difference spectra are located at the same frequency); separations 1 and 2 should produce maximal masking (in the presence of the distractors the maximal peak is shifted to the higher frequencies, see red peaks in Fig.4) and hence the worst performance; at separations 3, 4 and 5 there should be no deterioration in psychophysical performance.

Crowding effects

We turn now to the three layouts that we used to study crowding effects. Two of them are symmetrical: in one, all the distracting Landolt C's are rotated outwards relative to the test Landolt C, in the other, all the distracting Landolt C's face inwards. Though they are different, the amplitude difference spectra are very similar and do not change with the separation. We do not show them on the plots, as they are close to the difference spectrum of the isolated Landolt C (black curves on all plots). If the frequency that produces the maximum in the difference spectrum, i.e., CF, is responsible for detecting the orientation of the target in the presence of distractors, then performance for these two layouts should be similar and produce no masking, since there is no shift of CF in the presence of distracting Landolt Cs.

The six difference spectra for the third layout (right panel in Fig.4) have multiple maxima and minima at all analysed separations and it is difficult to predict what will happen in a psychophysical experiment, but since the largest peak in the amplitude difference spectrum is located below one harmonic, we may expect better performance for the ‘crowded’ Landolt C than for the isolated one.

Results

Fig. 5 presents the result of this experiment as percentage of correct responses plotted against separation for the three observers. In a given series, only one type of distractors was used. And the isolated Landolt C was presented in the same series. The pixel size was 0.16 arcmin. The four curves in each graph correspond to the different surroundings: four tangential bars (black filled circles), first ‘crowding’ layout (red triangles), second ‘crowding’ layout (green squares), and third ‘crowding’ layout (blue diamonds). The vertical lines on the plots denote separations approximately equal to one, three and five bar widths for each observer. For MD only two vertical lines are present because the diameter of the test Landolt C for this subject was 3.8 arcmin, and as the separation varied between 0.32 and 3.2 arcmin, 5 bar widths lie beyond the data points.

Contour interaction

For two (MD and NT) of the observers, we found classical masking functions for contour interaction, which resemble those found by Flom et al. (1963b). At the smallest separations, the percent of correct responses was relatively high; it decreased with increasing separation to a minimum at about 2 gap widths and then increased to the plateau level measured for the isolated Landolt C. For observer KT we recorded a function that was flat for separations smaller than 2 gap widths; the percent of correct responses then reached a plateau at relatively small separations between 2 and 3 gap widths.

At separation 0, the difference spectrum predicts the same performance in contour interaction condition as for the isolated letter. A substantial enhancement was reported by Flom and his colleagues (1963b), and later he pointed out that ‘…in many cases recognition was as good with the bars touching the C as when there were no bars in the field. For this reason alone, and there are others, the contour interaction described here should not be referred to as the “crowding effect” or “separation difficulty”. Crowding the bars against the C or being able to separate the C from the bars perceptually is not the problem’. (Flom, 1991, p.238). This finding corresponds to the prediction of the CF shift hypothesis. However, Hess at al. (2000) with their experimental setup failed to find any enhancement. They explained this fact by the possibly better acuity for high-contrast black letters than for the medium-contrast letters that they used. But in our present study not all the subjects showed a high percent of correct responses at the smallest separations in the contour interaction task though we used high-contrast characters. These individual differences cannot be explained by the spectral analysis. However, it explains the performance of those subjects who show almost no contour interaction at the smallest separations of the test Landolt C and the bars. After the experiments were finished, these subjects reported that in this experimental situation they saw a thick Landolt C. KT, who did not show improvement, reported that the bars were too close and made the recognition task difficult.

At separations of 1-2 gap widths the spectral analysis corresponds to the psychophysical data for all the subjects. However, the degree of impairment of performance should be smaller for separation 2 than for separation 1 (the shift is larger in the former case), but the impairment is the same for observer KT. The minimum of performance for the observers NT and MD lies between separation 1 and separation 2, but closer to separation 2. With increasing separations the observers' performance reaches the plateau measured for the isolated letter, as predicted from the difference spectra.

To summarise, the analysis of difference spectra predicts the general shape of masking functions in the contour interaction task: initially good performance at the smallest separations, which then deteriorates reaching a minimum and subsequently, at larger separations, recovers to the level measured for an isolated letter. But not all the observers produce masking functions of the shape that is predicted by the spectral analysis. We speculate here that higher, cognitive levels of the brain may fail to take advantage of information that is formally available to the observer. These levels may be or may not be able to use the information resulting from the low-level processing where spatial frequency selectivity takes place. We already mentioned this fact (Bondarko & Danilova, 1997) and would like to repeat it here. At the resolution limit, to detect the orientation of the test Landolt C, one does not need to see the gap clearly. The non-symmetrical shape of the isolated target, or of the target surrounded by juxtaposed bars, signals the gap's position at relatively low spatial frequencies. If observers are set to detect the position of a gap, they will respond only when they see the gap and may ignore other possible cues. In this case, their performance will drop to chance level. But those observers who can use all the available information will show a high percent of correct responses at the smallest separations, thus confirming the predictions made by the difference spectra.

Crowding effects

According to their difference spectra, the two symmetrical layouts should not produce masking. In fact, only one observer showed no masking and only for one layout (observer KT, when all distracting Landolt C's were rotated inwards). For observers NT and MD within the critical spacings (separations 1-3 gap widths) both the symmetrical layouts masked the test Landolt C. It should be mentioned that distractors rotated inwards produced weaker masking than those rotated outwards. Intuitively, this fact is rather predictable: the gap in the test Landolt C always coincides with the gap of one of the surrounding characters, which makes the gap more visible. Data for the third layout (all distractors rotated to the left) show that this layout produced masking similar to that produced by the second layout for observers NT and MD and stronger than for the two other layouts for observer KT.

In summary, the two symmetrical layouts that should not produce masking according to the prediction from their difference spectra resulted in the usual masking functions. Thus, we conclude that in the case of the crowding effect, the information provided by the amplitude difference spectra is not sufficient to explain the psychophysics: performance depends on the particular layout of the distractors.

Experiment 3: Crowding-effects for Snellen E

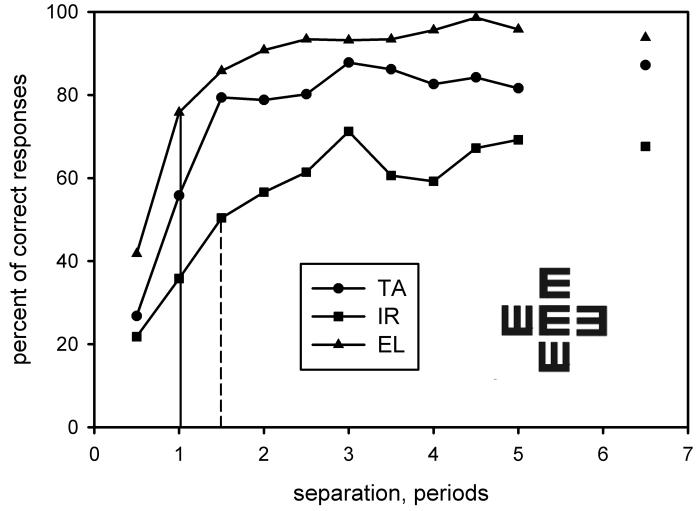
Having measured critical spacings in contour interaction and crowding-effect tasks for one of the characters most commonly used in optometric practice – the Landolt C – we then asked whether other characters behave in a similar way. We start with another well-known character – the Snellen E (Fig.1, h) surrounded by the same Snellen E's. The orientation of each of the four distracting Snellen E's varied randomly from trial to trial. The pixel size was 0.175 arcmin, and the corresponding sizes of the Snellen E were 3.5 for the observer EL and 4.4 arcmin for observers TA and IR.

Fig. 6 presents percent of correct responses as a function of separation between the test E and distractors. We express separation in units of periods where one period is equal to two stroke widths. The right-hand disconnected points correspond to the percent of correct responses measured for an isolated Snellen E. In each graph, the vertical lines denote the critical spacing for each observer. The period of the test E was 1.4 arcmin for observer IR and 1.75 arcmin for observers EL and TA; the critical spacings were equal to one period of the test E for observers EL and TA (1.75 arcmin, solid vertical line), but 1.5 periods for observer IR (2.1 arcmin, dashed vertical line). The critical spacings in this experiment are similar to those found in Experiment 1 for observers (KM, VB, IB) with comparable visual acuity.

Figure 6.

Percent of correct responses is plotted against spatial separation between the test Snellen E and four surrounding Snellen E's (see Fig.1, h). The dashed vertical line shows the critical spacing for observer IR; the solid vertical line shows the critical spacing for observers TA and EL.

In order to compare the critical spacings measured with different test stimuli we express all data in periods of CF. For the Landolt C we use 1.2 harmonic. For the Snellen E, the CF is reciprocal to its period (Bondarko & Danilova, 1997). If we use CF to calculate the critical spacings when Landolt Cs were used as a target (Experiment 1, Table 1 and 2) we obtain similar results. For observer JS the critical spacing is 0.74 periods, for observer KM – 1 .2, for observer IB – 1.08, for observer VB – 1.2, for observer MD – 1.03, for observer IK – 0.9, and for observer PF – 1.1 periods. On average, the critical spacings are very similar in the two tasks and are 1.04 for the Landolt C and 1.17 for the Snellen E.

Experiment 4: Crowding-effects for rectangular gratings

We extend our research to another stimulus that is used in optometry for visual acuity testing – a high-contrast rectangular grating. To some extent, this stimulus resembles Snellen E's and we were curious to see what happens to the observers' performance when rectangular gratings are surrounded by gratings of either the same spatial frequency (Fig.1, f) or a higher frequency (Fig.1, g). The two surrounds were randomly intermixed in the same experiment. The test grating and the surround gratings of the same frequency were composed of two black bars; the bright gap between the bars was of the same width. As in Experiment 1, the high-frequency gratings consisted of thin black bars (two pixels wide) and one pixel wide bright bars. Such a frequency (114 cpd) was beyond the resolution limit of observers as the pixel size was 0.175 arcmin.

In this experiment, two series of runs differed in the way the orientation of the surrounding grating was varied. In one series, the orientation (horizontal or vertical) of each of the four distracting grating varied randomly and independently from trial to trial as in Experiment 3. In the other series, the orientation was constant on all trials as in the second part of Experiment 1 (the layout of the surrounding gratings being as in Fig.1, c or d).

Fig. 7 shows the result of the first series for the three observers. In each graph, the open circles show observers' performance when same-frequency gratings were used as distractors, whereas the filled circles correspond to the high-frequency surround. The sizes of the test gratings were 2.1 arcmin (observer OV), 3.15 arcmin (observer NN) and 2.6 arcmin (observer AN). The corresponding periods of the test gratings were 1.4 arcmin, 2.1 arcmin and 1.75 arcmin. The abscissa shows the spatial separation between the test grating and the distractors in periods of the test grating. The disconnected circles on the right show the percent of correct responses to the test grating in the absence of the surround. Vertical lines denote the critical spacings defined using a χ² criterion as described in General methods. Each point in the graphs is based on 200 presentations accumulated on different experimental days. The maximal confidence interval in this experiment is 7% for a significant difference between the percent of correct responses to the isolated grating and to the same grating in the presence of the distracting gratings. All the data points that are located within the critical spacing in Fig.7 and Fig.8 differ from the isolated point by more than 14%. Solid lines show the areas for gratings of the same frequency, while dashed lines are used for high-frequency gratings.

Figure 7.

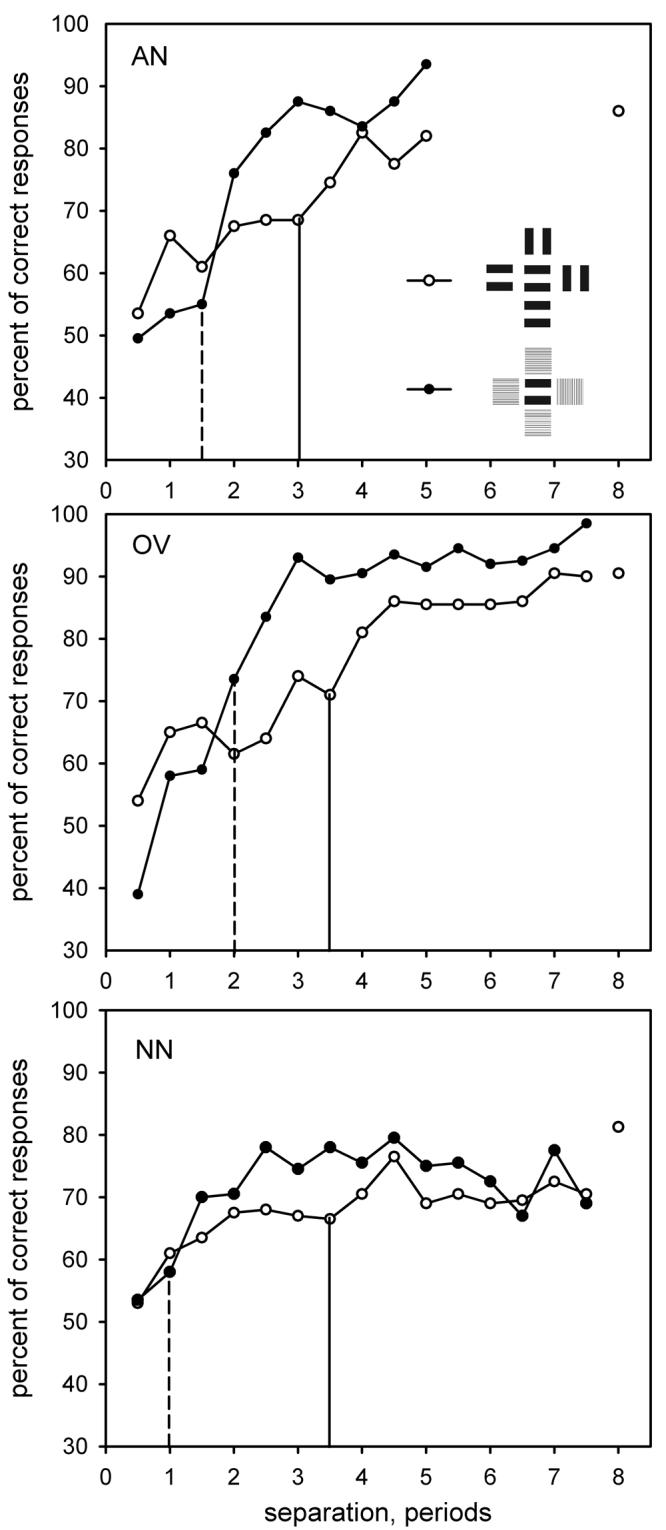
Percent of correct responses is plotted against spatial separation between the test rectangular grating and four surrounding gratings of different spatial frequency having random orientation, Solid vertical lines denote the critical spacing when surrounding gratings had the same spatial frequency as the test grating; dashed lines show the critical spacing when surrounding gratings were of high spatial frequency.

Figure 8.

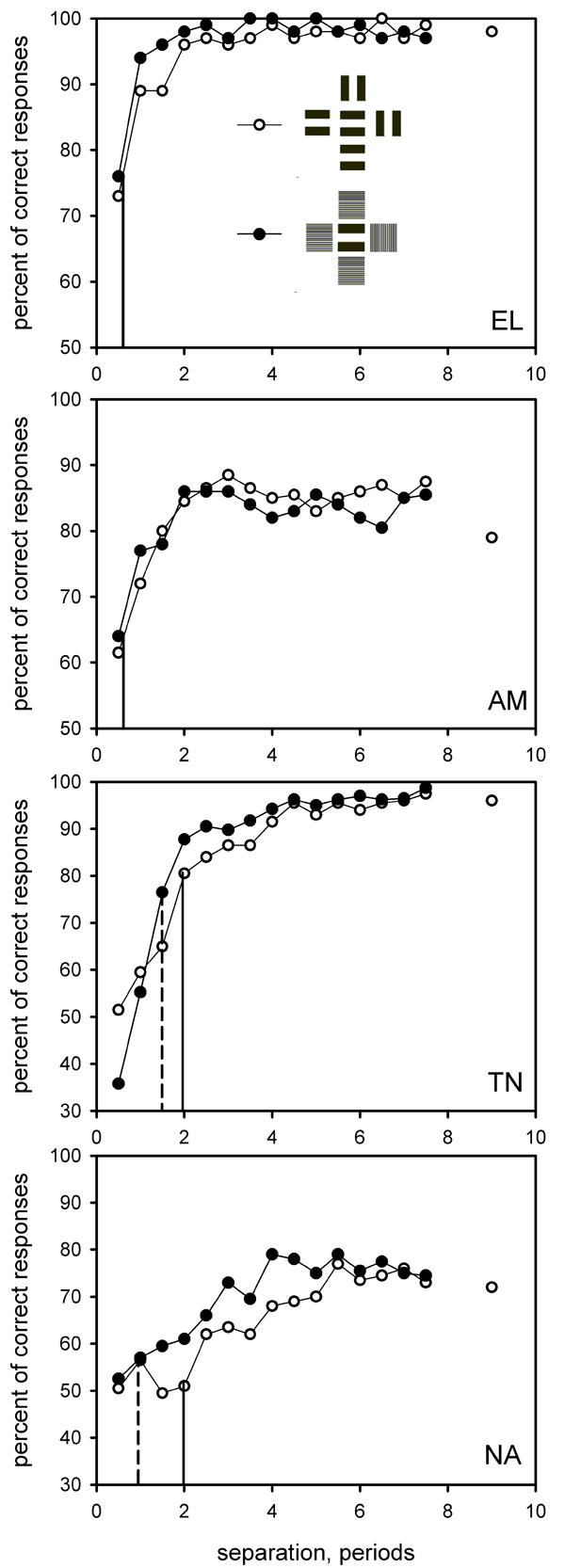
Same as Fig.7, but in this experimental series the surrounding gratings had constant orientation from trial to trial.

The percent of correct responses in general was lower when same-frequency gratings were used as distractors (open circles); this is reflected in the larger critical spacing, which was 3 periods (observer AN) and 3.5 periods (observers NN and OV). When high-frequency gratings surrounded the test grating, the critical spacing was smaller: 2 periods (observer OV), 1 period (observer NN) and 1.5 periods (observer AN). On average, for the three observers, the critical spacing was 3.3 periods for the surrounding gratings of the same frequency and 1.5 periods for the high-frequency gratings. Table 3 shows the values for the three observers; also shown are the data for observers MD, VB, IK and PF (on the gray background) obtained earlier in similar experimental conditions (Bondarko & Danilova, 1998, 1999). A t-test was performed on the 7 observers and showed significant difference between the two types of distractors (t=3.25, p=0.008).

Table 3.

Sizes of the test stimulus and critical spacings. The test gratings were surrounded by same-frequency or high-frequency gratings having random orientation from trial to trial.

Observer	Size of the test grating	Critical spacing
		Same frequency distractors		High frequency distractors
		arcmin	periods	arcmin	periods
AN	2.6	5.2	3.0	2.6	1.5
OV	2.1	4.9	3.5	2.8	2.0
NN	3.15	7.35	3.5	2.1	1.0
MD	3.1	4.2	2.0	3.1	1.5
VB	2.3	3.8	2.5	2.3	1.5
PF	4.5	9.0	3.0	7.5	2.5
IK	3.4	3.4	1.5	2.3	1.0
Mean	3.0	5.3	2.6	3.2	1.6

Fig.8 shows the results of the second series for four observers. The sizes of the test grating were 2.6 arcmin (observers AM. EL. TN) and 2.1 arcmin (observer NA). The corresponding periods were 1.75 arcmin and 1.4 arcmin.

When same-frequency gratings were used as surrounds, the critical spacings were 0.5 periods. or 0.9 arcmin (observers AM and EL); 2 periods, or 3.5 arcmin (observer TN) and 2 periods, or 2.8 arcmin (observer NA). When high-frequency gratings surrounded the test grating, the critical spacings were the same or smaller. Their sizes were 0.5 periods, or 0.9 arcmin (observers AM and EL); 1.5 periods, or 2.6 arcmin (observer TN) and 1 period. or 1.4 arcmin (observer TN). On average, for the four observers the critical spacings were 1.25 periods or 2 arcmin for the same frequency surrounding gratings and 0.9 periods or 1.5 arcmin for the high-frequency gratings.

When gratings with random orientation were used as distractors, the critical spacings were larger than in the other sets. The results for the two groups (the 7 observers. see Table 3 in one group; the 4 observers. see Table 4 in the nother group) were compared using a Mann-Whitney criteria. The critical spacing (in periods) differed significantly in the two series (U=3, p=0.042). Significant differences are also found between critical spacing measured with Landolt C's and gratings crowded by gratings with the same frequency and with random orientation (T=28, p<0.01).

Table 4.

Sizes of the test stimulus and critical spacings. The test gratings were surrounded by same frequency or high frequency gratings having constant orientation from trial to trial.

Observer	Size of the test grating	Critical spacing
		Same frequency distractors		High frequency distractors
		Arcmin	periods	arcmin	periods
AM	2.6	0.9	0.5	0.9	0.5
EL	2.6	0.9	0.5	0.9	0.5
TN	2.6	3.5	2.0	2.7	1.5
NA	2.1	2.8	2.0	1.4	1.0
Mean	2.5	1.95	1.25	1.5	0.875

The comparison of the results from Experiment 1, from Experiment 3 and from Experiment 4 (fixed orientation only) showed that the critical spacings are the same for the different type of test stimuli.

The two series of Experiment 4 showed that the critical spacing in the crowding-effect depends not only on the test stimulus and distractors, but also on the way the same distractors change their orientation. The importance of surround was also underlined by Herzog & Fahle (2002) who showed that masking of a vernier stimulus by gratings is more pronounced than when the same bars do not form a grating.

Individual differences

We should mention the large individual differences between observers. For example, when random orientation of the surrounding gratings was employed, the critical spacings varied from 1.5 periods (observer IK) to 3.5 periods (observers OV and NN). It should be pointed, that observers OV, NN and NA were naive observers and had never participated in psychophysical experiments before, whereas IK, VB and MD are highly experienced observers who had performed several experiments on crowding-effects and contour interactions with a Landolt C target. PF had performed several psychophysical experiments previously, but had never participated in any crowding effect studies, and he resembles the three naive observers. However, experience cannot explain the large variability in the second series, where gratings with constant orientation were used as surrounds (see Table 4). In this case, all four observers were naïve, but the measured critical spacings vary from 0.5 periods (AM and EL) to 2.0 periods (TN and NA). Similar individual differences were reported by Latham & Whitaker (1996): their two observers showed substantial differences in visual acuity values when identifying a rectangular grating consisted of 3 bars in the presence of distractors.

Discussion

In Experiments 1-4 we have shown that the strength and extent of crowding effects depend on the test stimulus (Landolt C, Snellen E and rectangular gratings); but also for the same stimulus, the masking depends on the layout of the distractors (Experiments 2 and 4). We have considered several hypotheses that have been used to explain contour interaction and crowding effects observed at the resolution limit of the visual system.

Physics of the stimulus

Hess et al (2000) suggested that “under conditions of contour interaction or ‘crowding’, the most relevant physical spatial frequency band of the test letter is displaced to higher spatial frequencies and that foveal vision tracks this change in spatial scale”. They argued that the maximum in the difference spectra to discriminate vertical vs. horizontal location of the gap is shifted beyond the resolution limit for the test Landolt C in the presence of distracting bars and thus leads to impaired performance. The present Experiment 2 showed that psychophysical performance confirms their hypothesis in the case of contour interaction, but not in the case of crowding effects. We found that in the case where the difference spectra in the presence of four distracting Landolt C's are very similar to the difference spectra of the isolated Landolt C (two symmetrical layouts when all four distracting Landolt C's are rotated either inwards or outwards), and where no deterioration of performance should be measured according to Hess and co-authors, we obtained the standard masking functions.

In our theoretical analysis we used only those calculations that were used by Hess et al. (2000) in their study. We are aware that this is not a complete analysis and other combinations of amplitude and phase spectra might account for the psychophysical results. We did not consider other spectral characteristics (such as phase) and thus the analysis is far from being complete.

A more detailed analysis of the amplitude difference spectra of the same stimulus (Landolt C or square Landolt C surrounded by four bars) was made by Liu (2001) who analytically derived the dependence of peaks in the amplitude difference spectrum on the harmonic number. He showed that at very small separations (smaller than one bar width), the maximal peak is close to the maximal peak for an isolated Landolt C – 1.2 harmonics. With increasing separation (starting from about 0.8 bar width, his Fig. 5), the peak is shifted towards higher harmonics; this shift is not continuous, but rather abrupt. Then the peak location very quickly returns to the position 1.2 harmonics (when bars are moved by only 1.7 bar widths away from the target). The function is periodic and with increasing separations there should be more changes in psychophysical performance if the physics of the stimulus plays a major role in contour interactions, though no variations were observed in psychophysical experiments with separations larger than 5 bar widths. According to Lui (2000), even contour interaction cannot be accounted for by pure physics of the stimulus: physiology is needed.

Lateral masking

During the past decade, several experiments have tested the validity of the lateral masking hypothesis (Chung et al., 2001; Levi et al., 2002b; Pelli et al., 2004). In the study by Chung et al. (2001) the two lateral masking predictions were tested in experiments employing letter trigrams. The test letter was filtered by spatial filters having different peak object spatial frequencies; they were flanked from two sides by other filtered letters. Both the tests and the flanks were drawn from the 26 letters of the Latin alphabet. The results confirmed that spatial frequency selectivity is present: the experimental curves relating peak masking frequency and target frequency are linear with a slope of 0.73 (see their Fig.5). However, the authors showed failure of scale-invariance: The critical letter spacing (i.e. spacing at which threshold elevation is 0) did not depend on the target frequency and was about 0.5 deg in the fovea. In a more recent paper, Levi et al. (2002b) using a tumbling E composed of Gabor or Gaussian elements, showed that (i) the critical spacings are proportional to the overall target size and (ii) high-contrast flanks produce similar threshold elevation in a direction-identification crowding task and in a detection task with similar stimuli, a result that led the authors to conclude that foveal crowding is simple contrast masking.

Another group of authors (Pelli et al., 2004) rejected lateral masking as the main cause of the crowding effect. First, their data confirmed Bouma's (1970) finding that the critical spacing depends on the target eccentricity, but not on the size of the target or of the flanks, and hence in crowding there is no tuning to size. Second, at an eccentricity of 4 degrees they found that in crowding, the recognition threshold is a sigmoid function of the flank contrast, which gives a log-log slope of 2 at small spacing and falls with increasing spacing. Such behavior is unlike ordinary masking, where threshold contrast is proportional to the masking contrast in double log-log coordinates with a slope of 0.5–1 (Legge & Foley, 1980). The authors concluded that some features of masking are present in crowding, but crowding occurs at a different processing stage than masking: after feature detection, but while integration of features takes place.

Ehrt and Hess (2005) also came to the conclusion that contour interaction and crowding phenomena differ from lateral masking, as the relationship between detection and discrimination of the same target Landolt C depended on its size: for small letters, detection was not affected by the flanks, for large letters, detection and discrimination in the presence of flanks showed similar behavior, but flanks with opposite polarity also were effective and facilitation was observed at small, but not large separations.

Our present experiments differ from the above mentioned studies in their experimental paradigm and more resemble the classical studies of crowding effects by Flom and his colleagues (Flom, 1991; Flom et al., 1963a; Flom et al., 1963b). We used high- contrast targets at the resolution limit of the visual system surrounded by high-contrast flanks and we recorded percent of correct responses as a function of spacing. Under our conditions, we (i) found that critical spacing did not scale with the target size, but decreased when we increased the size of the Landolt C slightly above the resolution limit; (ii) did not find spatial frequency selectivity for the test Landolt C's. Therefore, we conclude that foveal crowding effects at the resolution limit do not show characteristic features of lateral masking of the Polat & Sagi kind (Polat & Sagi, 1993).

Speculations

The variability of our results with respect to the critical spacing and test stimuli may be explained by assuming several mechanisms underlying foveal crowding effects at the resolution limit. Different mechanisms at the different levels of the visual system can account for different combinations of the targets and distractors.

Shape of the masking functions in the case of the test Landolt C

In all our experiments, except when surrounding bars were used, we obtained masking functions of similar shape for all distractors: The percent of correct responses increases with increasing separation and reaches a plateau at 3-5 gap widths. The plateau corresponds to the level of performance recorded for the isolated Landolt C. A U-shaped function was found in the contour interaction case.

The shape of the masking functions for both contour interaction and crowding effects at the resolution limit is described well by a simple model. The model incorporates integration of the spatial profile of a receptive field with the luminance profile of the test Landolt C and distractors (Bondarko & Danilova, 2002; Danilova & Bondarko, 2000). As receptive fields we chose spatial elements corresponding to the highest spatial-frequency channel described by Wilson and Gelb (1984). They considered only even spatial elements – bar-detectors (Fig.9, top panel, left). In the case of symmetrical surrounds, the responses of bar-detectors approximate well the shape of the masking functions and the strength of masking recorded in Experiments 1 and 2 (Fig.9, bottom panel). We also introduced odd elements – edge-detectors – as we need them to discriminate top-down and left-right orientations of the test Landolt C (Fig.9, top panel, right). The model performs spatial-frequency filtering of an image, the algorithm for which is similar to that for lateral masking. By assuming only one spatial element at the resolution limit, we make this model different from lateral masking models, which allow an almost continuous variation in the sizes of receptive fields. At the resolution limit, the same highest spatial-frequency element may be responsible for processing a set of stimuli that have small differences in size, thus giving the absence of scale invariance in Experiment 1. In this experiment we showed that when the size of the stimulus is relatively large and the percent of correct responses reaches almost 100% (the largest sizes of the tests for observers JS, IB and KM, see Fig.2), the critical spacings are very small, about 1 arcmin. The minimal size of critical spacing probably depends on the optical point-spread function.

Fig.9.

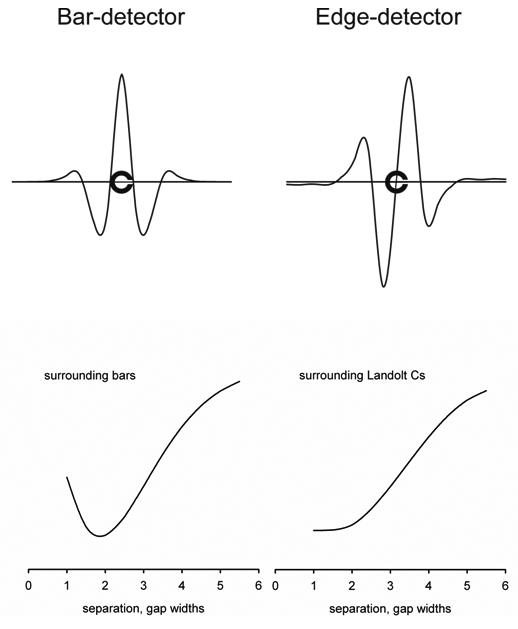
Top panel: Weighting functions of bar-detectors (left) and edge-detectors (right). The test Landolt C is superposed so that its centre coincides with the centre of the weighting functions; the diameter is equal to the size of excitatory area of the line detector. Bottom panel: Theoretical masking functions calculated using bar- and edge detectors.

In the case of observers with normal visual acuity, the weighting functions of these highest-frequency bar- and edge-detectors match the optical point-spread function and spatial arrangement of photoreceptors (Shelepin & Bondarko, 2002). A mismatch in these characteristics in impaired vision may result in abnormal crowding and can explain the different results obtained for amblyopics (Flom, 1991; Hariharan, Levi, & Klein, 2005; Hess, Dakin, Tewfik, & Brown, 2001).

Other types of tests and distractors

In Experiment 4 we obtained different critical spacing for different layouts of distractors, but the same test stimulus: when the orientation of the grating was random, the spacing was almost double. This discrepancy in critical spacing can be explained by either other types of spatial elements than bar- and edge-detectors at the filtering stage, or by interaction between the elements at the higher levels of the visual system.

Since the work of Hubel and Wiesel (1962) several types of receptive fields have been shown in the visual cortex. Psychophysical correlates of the receptive fields were shown in various studies (Bondarko, Gauzelman, & Glezer, 1983; Glezer & Kostelyanets, 1975; Kulikowski & King-Smith, 1973; Shapley & Tolhurst, 1973): bar-, edge- and grating detectors. While in the case of a test Landolt C, bar- and edge-detectors work well, we suggest that when gratings are used as a test stimulus, the grating-detector can be used to perform the horizontal vs. vertical discrimination task. Grating detectors have larger size, are phase-insensitive (Glezer, 1995) and may be more effective when the orientation of the surround is random (In this experiment both symmetrical and non-symmetrical stimuli are mixed in the same series).

In the case of Snellen E. the critical spacing is similar to that found for the test Landolt C, but the smallest size of the test Snellen E is larger than the minimal size of a test Landolt C. For processing such a stimulus, bar- and edge detectors can be used, but also high-frequency spatial elements with a larger number of periods can be considered, as was suggested by some authors (Glezer, 1995; King-Smith & Kulikowski, 1981).

We suggest that at the resolution limit, the crowding-effect is observed when both the test and its surround fall on to the same detectors. Thus we come back to the initial hypothesis suggested by Flom et al. (1963b) with regard to the receptive field that is optimal for detecting the orientation of a Landolt C target. In our version of the hypothesis, the visual system finds the most appropriate receptive field(s) considering as the stimulus the combination of the target and the surround. We don't exclude the possibility that the choice of the receptive field is influenced by top-down processes (Mollon & Danilova, 1996).

Conclusion

Our experiments show that foveal contour interaction and crowding effects are not simple phenomena that can be explained by a single process – either lateral masking, or the physics of the stimulus, or physiological inhibition, or optical factors. We would like to note, that the ‘physical’ hypothesis and lateral masking hypothesis, or other possible hypotheses, do not exclude each other: the combination of several mechanisms can contribute to the crowding-effects even in its more simple form at the resolution limit of the visual system.

It is likely that by choosing a particular experimental condition, different authors favor one contributing mechanism and attenuate the others. In our very specific case of high contrast optotypes at the resolution limit of the visual system, interaction between the test stimulus and its surround probably leads to an appropriate choice of a processing element depending on the combination of the target and distractors.