Changes in the Relationship Between Movement Velocity and Movement Distance in Primary Focal Hand Dystonia

Abstract

The authors examined the relationship between movement velocity and distance and the associated muscle activation patterns in 18 individuals with focal hand dystonia (FHD) compared with a control group of 18 individuals with no known neuromuscular condition. Participants performed targeted voluntary wrist and elbow flexion movements as fast as possible across 5 movement distances. Individuals with FHD were slower than controls across all distances, and this difference was accentuated for longer movements. Muscle activation patterns were triphasic in the majority of individuals with FHD, and muscle activation scaled with distance in a similar manner to controls. Cocontraction did not explain movement slowing in individuals with dystonia, but there was a trend toward underactivation of the 1st agonist burst in the dystonic group. The authors concluded that slowness is a consistent feature of voluntary movement in FHD and is present even in the absence of dystonic posturing. Underactivation of the 1st agonist burst appears to be the most likely reason to explain slowing.

Focal hand dystonia (FHD) is often described as a disorder associated with involuntary muscle activity that causes abnormal posturing of the hand and arm. However, mounting evidence suggests that dystonia is a disorder that also affects voluntary movement control, even in the absence of dystonic muscle spasms. Individuals with dystonia are slower than individuals with no known neuro-muscular condition at both wrist (MacKinnon, Velickovic, Drafta, Hesquijarosa, & Brin, 2004) and elbow joints (van der Kamp et al., 1989) during movement with one degree of freedom and during whole-arm movement (Agostino, Berardelli, Formica, Accornero, & Manfredi, 1992; Curra et al., 2000; Inzelberg, Flash, & Korczyn, 1990; Inzelberg, Flash, Schechtman, & Korczyn, 1995). However, single-joint studies have been concerned with movement distances of 30° or less, and researchers have examined no more than two target distances within a study (15° and 30°; van der Kamp et al., 1989). When ballistic self-paced movements are performed over a wide range of movement distances in individuals with no known neuromuscular condition, movement velocity scales with movement distance. This relationship is approximately linear (Bahill, Clark, & Stark, 1975; Newell, Hancock, & Robertson, 1984). Pfann, Buchman, Comella, and Corcos (2001) showed the slope of the relationship between peak velocity and target distance to be impaired in basal ganglia disorders such as Parkinson’s disease. Because dystonia is related to abnormal basal ganglia functioning, it is of interest to know whether the relationship between peak velocity and movement distance is also impaired in primary dystonia. It is also not clear whether muscle activation patterns are modulated appropriately across movement distance in individuals with dystonia.

In addition to deficits in movement control, deficits in force control have also been shown in individuals with focal dystonia even when they do not manifest dystonic symptoms during the performance of a task. For example, in the absence of dystonic posturing, individuals with FHD have been shown to be weaker than controls in producing maximal isometric wrist and elbow force (Prodoehl, Mackinnon, Comella, & Corcos, 2006c) and slower than controls in rapidly turning on and off submaximal isometric force at the wrist and elbow (Prodoehl, MacKinnon, Comella, & Corcos, 2006b). Because larger forces are required to rapidly move to targets at long distances compared to short distances, force production deficits may lead to greater impairment of movement at longer distances in individuals with dystonia. In addition, attenuated and delayed force output could disrupt the timing and magnitude of the triphasic pattern of muscle activation that is typically observed in participants with no known neuromuscular disorder (Cooke & Brown, 1990). Such a deficit would suggest a more wide-spread dysfunction of motor control that is independent of the task that normally triggers the dystonic cramp.

Therefore, the purpose of this study was to provide a quantitative examination of the relationship between movement velocity and movement distance in a relatively large homogenous group of individuals with FHD compared with controls. We chose to examine kinematics during rapid voluntary movements at the wrist joint and elbow joint. In addition, we evaluated whether slowness was accompanied by a generalized change in the muscle activation patterns of dystonic participants or by participant-specific changes in muscle activation patterns.

Method

Participants

Participants were 18 individuals with writer’s cramp (see Table 1) and 18 age-, height-, weight-, and gender-matched participants with no known neuromuscular condition. These were the same participants as those whom we tested during some of our previous work (Prodoehl, Corcos, & Vaillancourt, 2006a; Prodoehl et al., 2006b, 2006c). Individuals with FHD were recruited from two surrounding medical centers. Inclusion criteria for participation included a diagnosis of writer’s cramp, an age range of 20–65 years, no history of other neurological problems or injury involving the arms, and at least a 4-month time period since receiving any botulinum toxin injection treatment for dystonia. The most clinically affected hand was tested in all individuals with dystonia that was the dominant side. All experiments were conducted in accordance with the Declaration of Helsinki, and we obtained informed consent from each participant prior to testing. The Institutional Review Board of the University of Illinois at Chicago approved the protocol.

TABLE 1.

Characteristics of Individuals with Focal Hand Dystonia

Participant	Age (yr)	Hand dominance	Gender	Duration of symptoms (yr)	Diagnosisa	Duration since previous treatment with botulinum toxin	Movement scale severity (arm)b	Pattern of symptoms during writingc
S1	57	R	F	14	DC	N/A	2	Thumb ext, F2–4 flex
S2	57	L	F	7	DC	N/A	6	Wrist, thumb, & F1–2 flex, F2 abd
S3	61	R	F	17	WC	13 months	9	F2–3 flex, wrist UD
S4	63	R	F	17	DC	N/A	9	Wrist flex & UD, F5 finger abd
S5	24	R	F	16	WC	N/A	4	Wrist flex & UD, F2 flex
S6	53	R	F	41	DC	N/A	9	Wrist flex & UD, F2–5 flex
S7	57	L	F	6	WC	N/A	2	Wrist, thumb, & F2 flex
S8	53	R	F	12	WC	N/A	2	Wrist flex & F2 ext
S9	45	R	F	2.5	WC	N/A	2	Wrist UD, thumb & F2 flex
S10	51	R	M	6	DC	4 months	6	Wrist flex
S11	47	R	M	10	WC	N/A	2	Wrist ext, F2 abd & flex
S12	48	L	M	2.5	DC	6 months	4	Wrist UD, F5 flex
S13	56	L	M	5	WC	N/A	2	Wrist ext, F2 flex
S14	53	R	M	5	WC	4 months	2	Thumb & F2 ext
S15	42	R	M	7	WC	14 months	2	Wrist ext, F2 flex
S16	45	R	M	23	DC	13 months	12	Wrist UD & flex, thumb & F2–3 ext
S17	33	R	M	5	WC	37 months	2	Wrist ext, F1–2 ext,thumb abd
S18	44	R	M	5	WC	N/A	2	Thumb ext

^aWC = writer’s cramp (only writing affected); DC = dystonic cramp (more than one task involved; M. P. Sheehy & C. D. Marsden, 1982).

^bArm subscale from the Burke-Fahn-Marsden (R. E. Burke et al., 1985) rating scale, with higher scores indicating more severe arm involvement (maximum score = 16).

^cExt = extension, flex = flexion, UD = ulnar deviation, abd = abduction, add = adduction, F1–5 = fingers 1–5.

Materials and Procedure

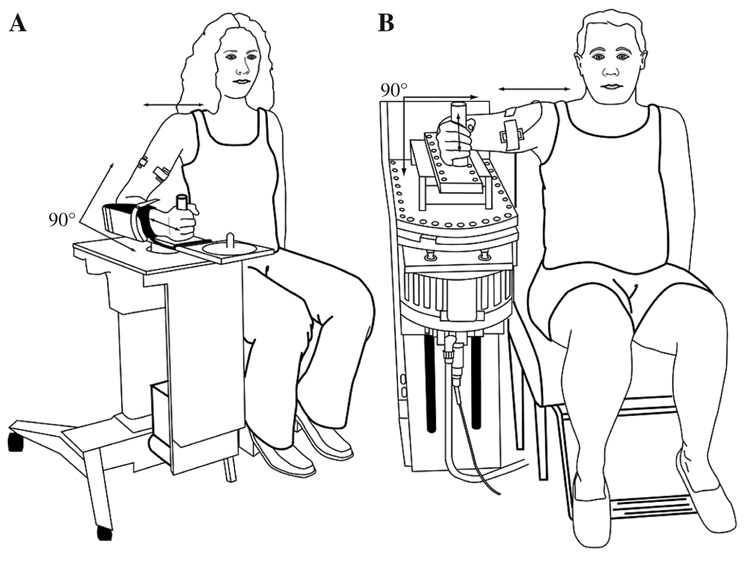
The equipment that we used in this study was exactly as described previously in detail (Prodoehl et al., 2006c). In brief, manipulanda devices were used for testing at the wrist and elbow joints (see Figure 1). Participants performed flexion movements as fast as possible to targets at 11°, 18°, 36°, 54° and 72° from a consistent starting position of either 35° of wrist extension or 35° of elbow flexion. For testing with the wrist manipulandum, 0° corresponded to a neutral position in regard to flexion and extension of the wrist. For testing with the elbow manipulandum, 0° corresponded to full extension of the elbow joint. Joint angle was measured by a capacitive transducer attached to the motor shaft at the axis of rotation of each manipulandum. At the wrist joint, joint velocity and acceleration were calculated through differentiation of the position signal. Angular acceleration at the elbow joint was determined from a high-quality servo accelerometer (Allied Electronic, Inc., Fort Worth, TX) mounted on the elbow manipulandum bar, and velocity was obtained through an analog differentiating circuit of the angle signal. All mechanical signals were digitized at 1000 Hz and stored on a computer for off-line analysis. We digitally low-pass filtered mechanical signals at 20 Hz by using a second-order Butterworth filter, dual-passed.

FIGURE 1.

Experimental setup at the (A) wrist and (B) elbow joints.

We displayed joint angle on a computer screen as a vertical marker. A second stationary marker bar on the screen showed the correct starting joint position. A broad vertical marker bar represented target location. The width of the target always corresponded to 6° of angular rotation. To start the trial, we asked the participant to align the angle marker to the starting position marker. A computer-generated preparatory tone alerted the participant to prepare for a movement. A second “go” tone was generated 1 s after the preparatory tone as a signal to the participant to begin the movement. Participants were instructed to make each movement as fast as possible from the starting position marker and to try to land in the target zone. Participants were asked to perform 5 practice trials immediately prior to data collection at each movement distance. We collected 13 movement trials at each movement distance at the wrist and the elbow joints with a 3-s intertrial interval. Instructions were given at the beginning of each practice block and repeated at the beginning of each block of 13 trials. No additional feedback (apart from the participant’s viewing the cursor on the computer monitor) was given to the participant during or after each series of 13 movements. We randomized the testing order of which joints were tested first and the movement distance within each joint across dystonic participants. Control participants performed the same joint and movement distance sequence as their matched dystonic counterparts. It is important to note that this task did not induce dystonic posturing in any of the individuals with FHD except for participant S16, who was the most impaired participant. However, kinematic results from this participant were similar to those of the rest of the group.

We recorded surface electromyograms (EMGs) from a main agonist (flexor carpi radialis) and a main antagonist (extensor carpi radialis longus) at the wrist and a main agonist muscle (biceps brachii) and a main antagonist muscle (lateral head of triceps brachii) at the elbow by using the Delsys Bagnoli-4 EMG system, which filters the signal to a bandwidth of 20–450 Hz. Recorded EMGs were digitally full-wave rectified and low-pass filtered (second-order Butterworth filter at 50 Hz, dual-passed) and sampled at 1000 Hz.

Data Analysis

Data were initially processed in Labview by using custom-written software. Each trial record was visually inspected prior to data analysis. Only trials whose end position fell within the target were accepted for further analysis. In the control group, 13% of all trials were rejected and in the dystonic group, 14% of all trials were rejected. A custom-written algorithm (MATLAB; MathWorks, Natick, MA) was run on the kinematic data to identify peak velocity. EMG data were always aligned with respect to the onset of the agonist EMG. Agonist EMG onset was identified by using a custom-written algorithm (MATLAB) for detecting EMG onset (Vaillancourt, Prodoehl, Verhagen Metman, Bakay, & Corcos, 2004). The marker was manually adjusted only if it clearly fell in the wrong place (e.g., occurred after movement onset), in which case the first sustained deflection above baseline was marked as the agonist EMG onset.

Based on the marked kinematic and EMG points, the following measures were calculated.

Peak velocity (V/sec) was the highest value of movement velocity. This parameter is used to characterize movement slowing.

Q_AG (mV) was the integral of the agonist EMG signal (biceps brachii or flexor carpi ulnaris) from the marked agonist onset to the time of peak velocity for each trial. This parameter is used to characterize the area of the first agonist EMG burst that is responsible for the limb accelerating toward the target.

Q_AG/T (mV/s) was the integral of the agonist EMG signal from the marked onset to the time of peak velocity for each trial divided by the duration of the burst. This parameter is used to characterize the first agonist EMG burst accounting for any changes in burst duration.

Q_ANT (mV) was the integral of the antagonist EMG signal (lateral head of triceps or extensor carpi radialis longus) for each trial from the marked onset of the agonist burst to the end of the movement. This parameter is used to characterize the area of the antagonist burst.

Q_{AG Duration} (s) was time from the marked onset to the marked offset of the first agonist burst. This parameter is used to characterize the duration of the first agonist burst.

Cocontraction (%) was the degree of cocontraction from movement onset up to peak acceleration (Cocontract_PRE) and from peak acceleration to peak velocity (Cocontract-_POST). We calculated it according to the algorithm by Winter (1990). The equation assesses the percentage of overlapping area in each agonist–antagonist EMG pair (Vaillancourt et al., 2004).

$$\mathit{\text{Concon}}=2\cdot \frac{{\displaystyle \int \text{min}[{\mathit{\text{EMG}}}_i(t),{\mathit{\text{EMG}}}_j(t)]dt}}{{\displaystyle \int {\mathit{\text{EMG}}}_i(t)dt+{\displaystyle \int {\mathit{\text{EMG}}}_j(t)dt}}},$$ (1)

where min is the minimum between two signals at time t.

The dependent variables described above were analyzed using mixed-model analyses with heterogenous compound symmetry in a SAS 9.1 statistical package (SAS Institute, Cary, NC). Initial examination of the data revealed heteroscedasticity (i.e., unequal variances across distance), particularly for the peak velocity data. A mixed-model analysis allows modeling of the correlation within participants and does not have an assumption of equal variance (Brown & Prescott, 2006). The mixed model with heterogenous compound symmetry requires all correlations within person to be the same but does not require all the variances to be the same. This pattern is consistent with the observed variances and correlations. The model includes fixed effects for group, for distance in the appropriate units of measurement, and for the interaction term. (Joint is not considered a factor because the data between elbow and wrist were highly correlated, and the average of the two joints was analyzed.) All effects in the mixed model were evaluated as significant when there was less than a 5% chance of making a Type I error (p < .05).

Results

Peak Velocity

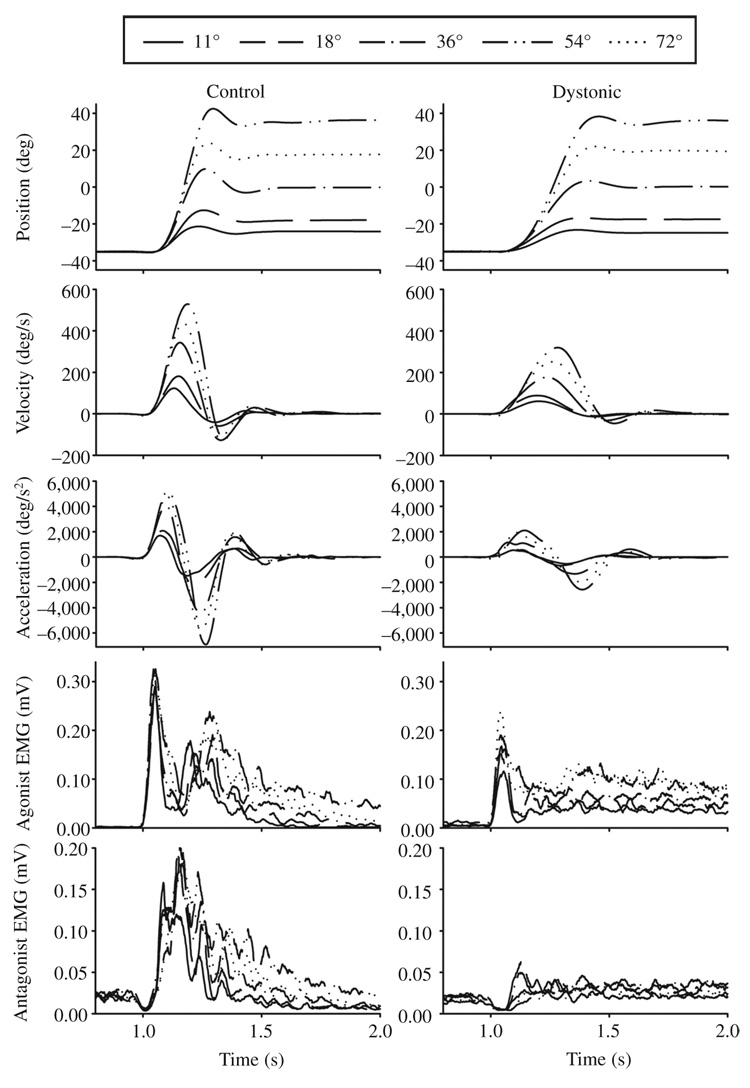
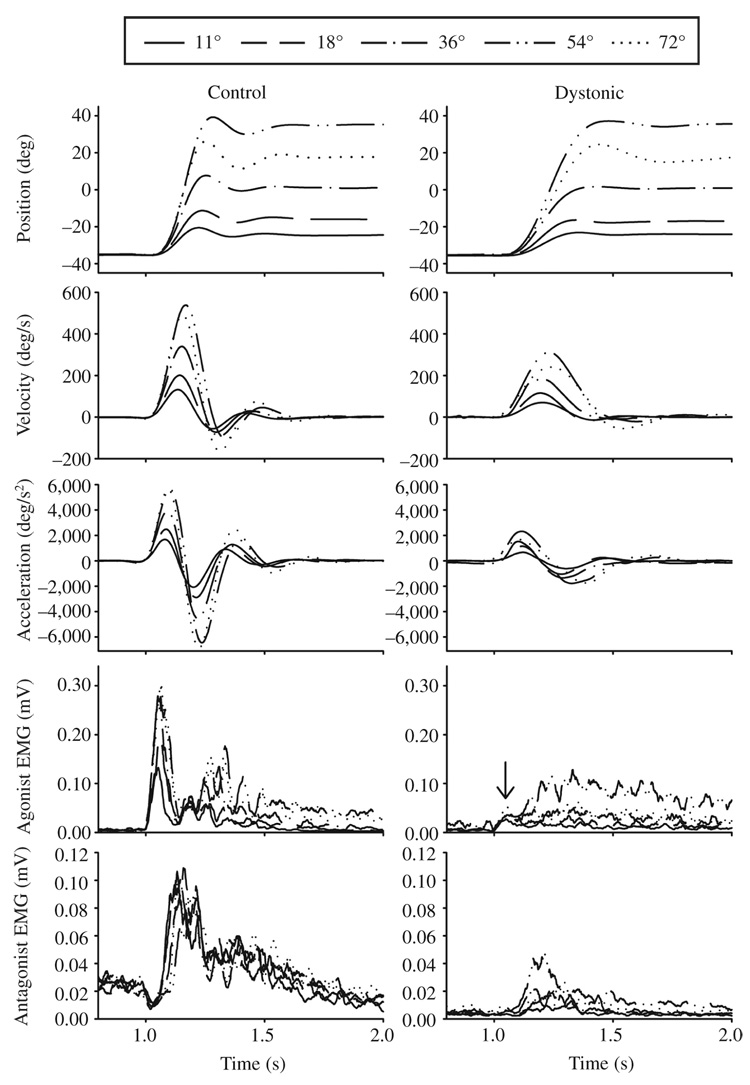
Examples of the averaged kinematics along with EMG profiles during wrist movements to each target in two individuals with FHD and their matched control participants are shown in Figures 2A and 2B. The elbow joint showed similar kinematic and EMG patterns. Note that in both individuals with FHD the peak velocities and peak accelerations and decelerations were reduced relative to the control participant for each target distance. The participant in Figure 2B was the only participant who manifested dystonic symptoms during the task. Despite this, the movement kinematics of this participant were similar in pattern to those of the other dystonic participants.

FIGURE 2.

FIGURE 2A. Wrist flexion movements from 11 to 72°. Average angle, velocity, acceleration, agonist electromyogram (EMG), and antagonist EMG signals are shown across 5 distances in two dystonic and two matched control participants. The two dystonic participants are participants D12 (2A) and D16 (2B). The arrow in the agonist EMG panel of the dystonic individual with FHD in B identifies the small first agonist burst in this individual.

FIGURE 2B. Wrist flexion movements from 11 to 72°. Average angle, velocity, acceleration, agonist electromyogram (EMG), and antagonist EMG signals are shown across 5 distances in two dystonic and two matched control participants. The two dystonic participants are participants D12 (2A) and D16 (2B). The arrow in the agonist EMG panel of the dystonic individual with FHD in B identifies the small first agonist burst in this individual.

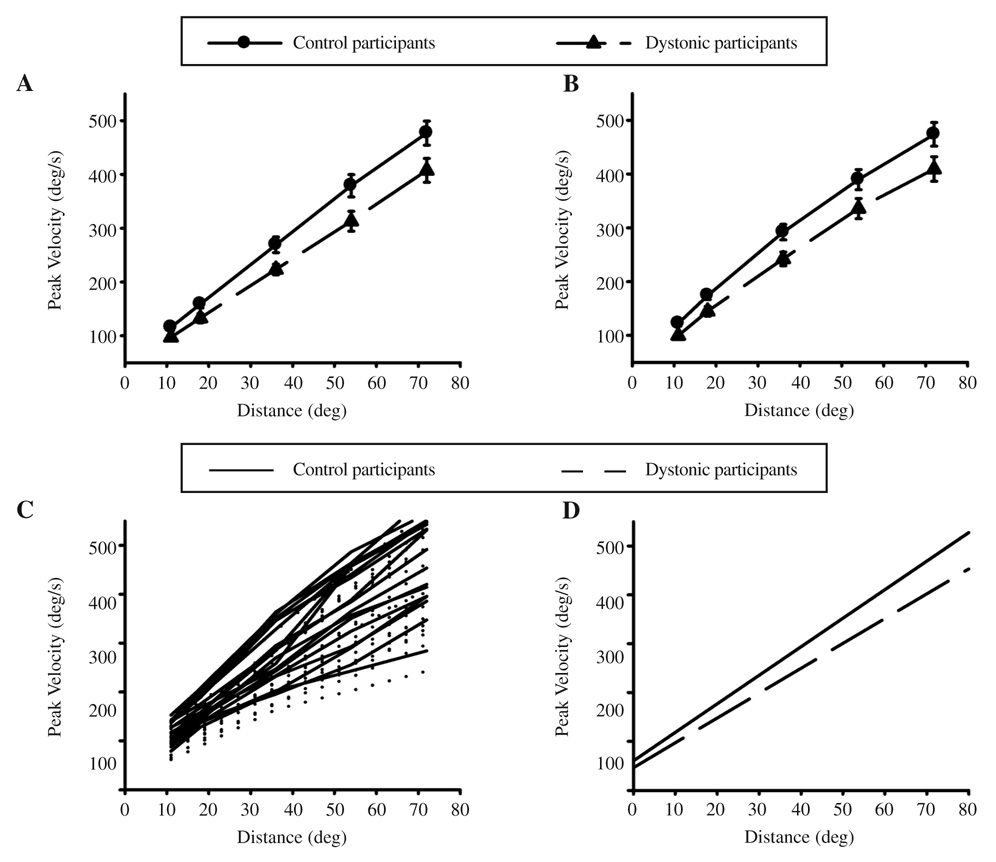
Peak velocity across movement distance averaged across all participants is shown in Figure 3A for the wrist and 3B for the elbow. There was remarkable similarity between the joints for both groups. Dystonic participants were significantly slower than controls across movement distance (see Table 2). The movement velocity–distance relationship for each participant and the linear regression lines for each group derived from the mixed-model analysis are shown in Figures 3C and 3D, respectively. There was a significant difference in the slope between groups (shown by the significant Group × Distance interaction in Table 2). Based on the results of the mixed-model analyses, the relationship between velocity and distance in the dystonic group can be expressed as:

$$\overline{y}\text{dystonic}=53.8746-11.2266+(5.9913-0.8400)*x$$ (2)

(where ȳ = mean peak velocity and x = movement distance). Equation 2 shows that, for any given movement distance, the intercept for the dystonic group will be approximately 11 deg/s lower than controls, and the slope of the relationship between velocity and distance will be significantly lower in dystonics than controls by an increasing amount as movement distance increases (p = .016). Thus, the slopes of each group diverge as movement distance increases (p = .030).

FIGURE 3.

Mean and standard error of peak velocity across 5 distances at the (A) wrist and (B) elbow for control and dystonic participants. C is the average peak velocity across joint for each distance for individual controls and dystonics. D shows the linear regression lines for the data in C (extended to show the intercept).

TABLE 2.

Mixed-Model Analysis Results

	Group		Distance		Group × Distance
Measure	F(1, 34)	p	F(1, 142)	p	F(1, 142)	p
Peak velocity	6.37	.016	846.53	<.0001	4.81	.030
QAG/T	1.13	.296	99.6	<.0001	0.67	.413
QAG Duration	0.39	.535	174.04	<.0001	0.72	.397
QANT	0.24	.0630	108.14	<.0001	0.05	.827
CocontractPRE	2.72	.108	9.24	.0028	1.13	.289
CocontractPOST	1.05	.313	5.03	.0264	2.48	.117

Note. Q_AG/T is the integral of the agonist EMG signal; Q_ANT is the integral of the antagonist EMG signal, Q_{AG Duration} is the duration of the first agonist burst; Cocontract_PRE is the degree of agonist/antagonist cocontraction up to peak acceleration; and Cocontract_POST is the degree of cocontraction from peak acceleration to peak velocity.

EMG Patterns

The EMG patterns in the majority of individuals with FHD (14 of 18) that were not manifesting dystonic symptoms during the task were clearly triphasic in pattern, with no evidence of cocontraction that would be considered abnormal (see Figure 2A). That is, the antagonist muscle turned on shortly after onset of the first agonist burst, was of appropriate size, and turned off appropriately toward the end of the movement. However, we did note some intertrial variability in which occasional trials demonstrated abnormally prolonged second agonist burst activity. We had 1 individual with FHD who manifested dystonic symptoms during the task (see Figure 2B). This individual’s EMG was characterized by a very small initial agonist burst (see the arrow in Figure 2B dystonic agonist EMG), prolonged second agonist burst activity, and an antagonist burst that was essentially normal. However, this abnormally prolonged second agonist burst was also seen in 3 other individuals with FHD who did not demonstrate dystonic posturing or report experiencing dystonic symptoms during the task (Participants S5, S12, and S17 in Table 1) and in 1 control. The presence of dystonic posturing therefore did not produce a uniquely abnormal EMG pattern.

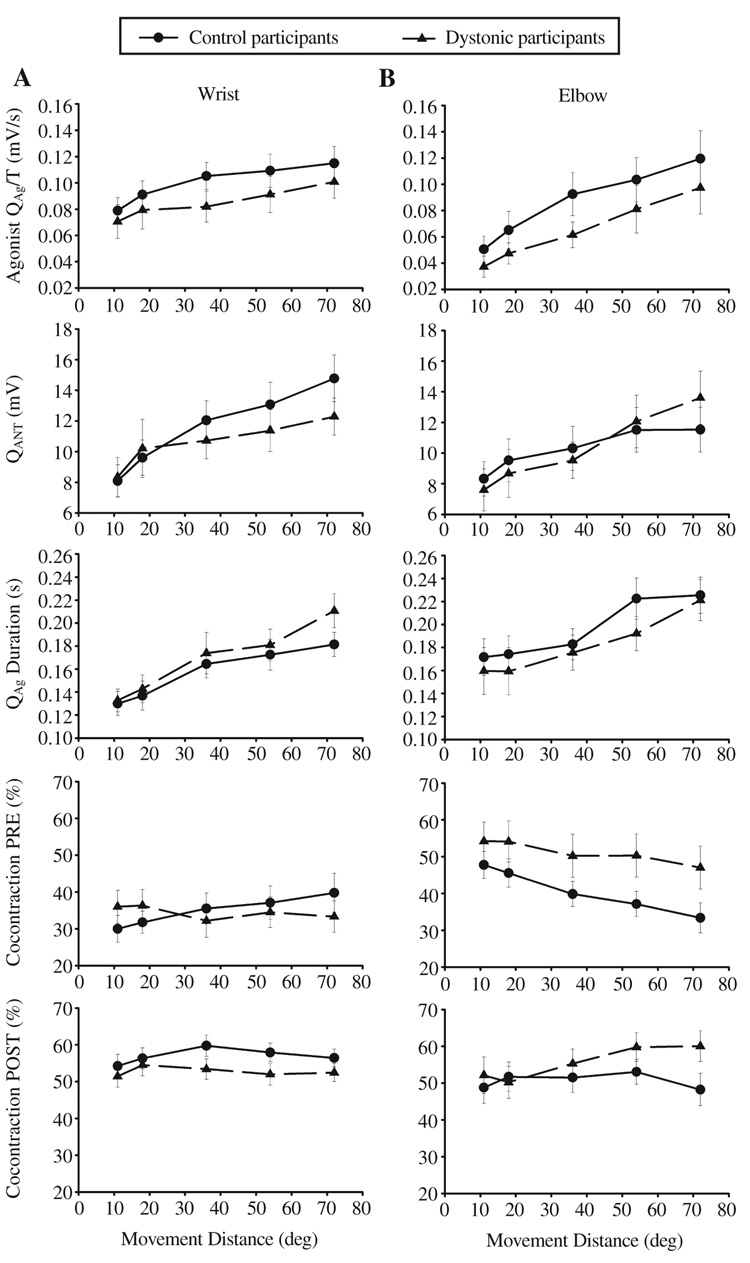
A summary of the statistical analysis of the EMG data is provided in Table 2. In contrast to the kinematic data, there were no significant group effects for any of the quantitative measures of EMG activity. There were also no significant Group × Distance interactions. The relationship between agonist and antagonist muscle activity with target distance is shown in Figure 4. Agonist and antagonist EMGs scaled with movement distance in both groups. The average agonist EMG activity was reduced in the individuals with FHD (15% at the wrist and 28% at the elbow compared to the controls), although this did not reach statistical significance. There was no significant group effect for either measure of cocontraction (see Table 2, Figure 4).

FIGURE 4.

From top to bottom: mean and standard error of the integral of the agonist electromyogram (EMG) divided by time, integral of the antagonist, duration of the first agonist burst, cocontraction from movement onset up to peak acceleration (PRE), and cocontraction from peak acceleration up to peak velocity (POST) across 5 distances at the (A) wrist and (B) elbow joints for control and dystonic participants.

There are two possible reasons for the lack of statistical significance in the EMG measures. The first is that there is only a small difference between the means of the two groups. The second is that the variance of the EMG measures is higher than the variance of the kinematic measures. Figures 3 A and 3B, show that even for the longest movement, the standard error of peak velocity is only 0.05% of the mean for the dystonic participants at the wrist. In contrast, in the EMG data in Figure 4 (see top left panel QAg/T), the standard error is 12.3% of the mean for the same movement distance.

One way to compare measures with different variances is to convert raw scores to standardized scores. A standardized score is a dimensionless quantity derived by subtracting the sample mean from an individual (raw) score and then dividing the difference by the standard deviation:

$$z=\frac{x-\overline{x}}{s},$$ (3)

where z is the standardized score, x is the raw score to be standardized, x̄ is the mean of the sample, and s is the standard deviation of the sample.

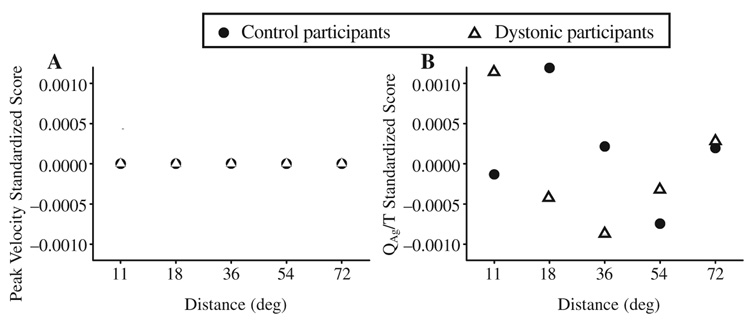
We compared Q_Ag/T and peak velocity across groups as standardized scores (see Figure 5). Standardized scores were calculated for each participant across joint at each movement distance. These scores were then averaged across all participants within a group to give a mean standardized score for each group at each movement distance. Variation between the groups was minimal for the measure of peak velocity for which there is statistical support for a reduced peak velocity across movement distance in the individuals with FHD. However, variation between the groups was high for the agonist EMG measure and that likely accounts for the lack of statistical significance in that measure despite a clear trend of reduced agonist EMG activity in the FHD group.

FIGURE 5.

(A) Peak velocity and (B) the electromyogram (EMG) integral of the first agonist burst averaged across joint converted to standardized scores for control and dystonic participants. The standardized score is negative when the individual raw score is below the population mean.

Discussion

In this study, we performed a quantitative examination of movement velocity at the wrist and elbow joints across distance and EMG activation patterns in a relatively large homogenous group of individuals with FHD compared to a control group. There are two principal findings. First, individuals with FHD were on average 16% slower at both joints than controls. Moreover, the slope of the relationship between movement velocity and movement distance was significantly different in dystonics than controls. Thus, at longer movement distances, the difference in peak velocity between the groups becomes accentuated. Second, the muscle activation patterns underlying movement slowness showed a triphasic pattern of activation in the majority of individuals with FHD tested. Without dystonic posturing, cocontraction did not account for movement slowing. High variability in the EMG data makes finding statistically significant differences challenging, but underactivation of the first agonist burst appears to be a more likely explanation for movement slowing in FHD than muscle cocontraction.

Kinematic Slowing in Dystonia

Movement slowness has previously been shown in individuals with dystonia (Agostino et al., 1992; Curra et al., 2000; Inzelberg et al., 1990; Inzelberg et al., 1995; MacKinnon et al., 2004; van der Kamp et al., 1989). However, this is the first study to show that movement slowing is present across a wide range of movement distances, including both short and long movements, and at both wrist and elbow joints. Movement slowing at both of these joints was present regardless of the presence of dystonic contractions. This is also the first study to show that the relationship between movement velocity and movement amplitude is different in FHD compared with controls. One possibility to explain movement slowing is that dystonic participants simply chose to move more slowly than controls, perhaps to maintain movement accuracy. This possibility cannot be ruled out. Another possibility to explain slowing is that individuals with FHD have a motor execution problem that causes them to move more slowly than if they did not have dystonia. Evidence to support this later possibility is twofold. First, in the present study, each of the 18 individuals with FHD was slower than the control, suggesting that slowing is a more universal feature of the disorder than a participant-specific interpretation of the task. Second, slowness was also found in a previous study utilizing this exact group of participants with FHD performing an isometric task that did not have accuracy constraints. In that study we examined the ability of individuals with FHD to terminate force either passively (i.e., by relaxing) or actively (i.e., by rapidly reversing torque output) without regard to a target (Prodoehl et al., 2006b). Under both the contraction and relaxation task conditions, where accuracy was not a concern, dystonic participants were slower than controls. Regardless of cause, our findings in the present study provide additional support for the idea that bradykinesia is a feature of voluntary movement in individuals with dystonia, present even in the absence of dystonic posturing. This bradykinesia is similar to that seen in other disorders of the basal ganglia such as Parkinson’s disease (PD; Hallett, Shahani, & Young, 1977; Pfann et al., 2001) and Huntington’s disease (van Vugt et al., 2004).

The present study is the first to show that impaired scaling of movement speed across movement distance is present in individuals with FHD. It has previously been shown that the slope of the relationship between movement distance and movement velocity is steeper for control participants than participants with mild PD such that longer movements are associated with a greater degree of slowing in PD (Flowers, 1976; Pfann et al., 2001). The results of the present study show that a comparable impairment in movement scaling is present in individuals with FHD. This finding suggests that, in addition to a generalized slowing of movement, basal ganglia disorders are associated with impaired scaling of movement velocity across distance.

EMG Patterns in Dystonia

Despite clear evidence of movement slowing in dystonia across a wide range of movement distances and two joints, the majority of individuals with FHD showed a triphasic EMG pattern that did not differ from controls. Similarly, agonist burst magnitude, agonist duration, and antagonist burst magnitude scaled with movement distance in a similar manner in both groups (see Figure 4). This is a clear difference from individuals with PD whose EMG is characterized by short multiple agonist bursts which do not increase in duration with increasing movement distance (Hallett et al., 1977; Pfann et al., 2001). Previous studies in dystonia have described patterns of muscle activation underlying movement that range from the normal triphasic pattern (Rothwell, Obeso, Day, & Marsden, 1983; van der Kamp et al., 1989) to tonic EMG activity without any evidence of agonist-antagonist bursting (Cohen & Hallett, 1988; Rothwell et al.; van der Kamp et al., 1989). These discrepancies might be explained by the presence or absence of dystonic posturing during the task. When participants with hand cramps are examined as they exhibit a cramp, clear abnormalities in individual EMG activity can be seen which fall into two abnormal patterns: generalized cocontracting spasms and long cocontracting bursts (Cohen & Hallett). However, we have shown that the presence of hand cramps is not necessary to exhibit movement slowness. In the one individual tested that did manifest dystonic posturing during the task, there was a much larger and prolonged second agonist burst. However, this pattern was also observed in several other participants who did not manifest dystonic posturing during the task and one control. Thus, an abnormal EMG pattern was not unique to the presence of dystonic posturing. Furthermore, movement slowing could not be explained by cocontraction in our study, which is in agreement with results from other studies (MacKinnon et al., 2004; Malfait & Sanger, 2007; Prodoehl et al., 2006c).

The most robust EMG difference that we observed across individual participants with FHD was reduced agonist muscle activation. Reduced agonist activation has previously been shown to be associated with strength deficits in individuals with FHD (Prodoehl et al., 2006c). Reduced agonist activation is consistent with cortical activation and electroencephalogram studies that have shown reduced supplementary motor area and primary sensorimotor cortex activation in dystonic participants performing a task that did not induce dystonic posturing (Ceballos-Baumann et al., 1995; Ceballos-Baumann, Sheean, Passingham, Marsden, & Brooks, 1997; Hamano et al., 1999; van der Kamp, Rothwell, Thompson, Day, & Marsden, 1995). Abnormalities in the processing of primary afferent input have also been reported in individuals with dystonia (Rosenkranz, Altenmuller, Siggelkow, & Dengler, 2000; Siggelkow et al., 2002; Tempel & Perlmutter, 1990). Thus, reduced cortical activity in response to primary afferent input may contribute to an overall reduced central drive during voluntary motor output in dystonia and may contribute to the reduced agonist activity observed during movement. However, this interpretation should be viewed with caution considering that no significant differences were observed in agonist muscle activation between groups.

The absence of a statistical difference in quantitative measures of EMG activity, despite significant differences in limb kinematics, exemplifies the limitations associated with using EMG to compare activation patterns between groups. In the present study we used a larger sample size of individuals with dystonia than is typical for studies of this nature, yet we failed to observe statistical significance for any of our EMG measures. This problem is not unique to dystonia and is attributable in large part to the high variability in EMG measures between participants. Pfann et al. (2001) studied movement control in fourteen individuals with PD. Despite clear evidence of EMG abnormalities in scaling of the magnitude of the first agonist burst with increasing movement distance in individuals with more severe symptoms of PD (their Figure 7), there was no statistically significant effect of disease on magnitude of the first agonist burst or the area of the antagonist EMG. In the present study, there was a trend toward underactivation of the first agonist burst which can be seen in both dystonic participants (see Figure 2) as well the average group data (Figure 4, top panels). When QAg1/T and peak velocity data are converted to standardized scores (see Figure 5), one can see that the spread of scores around the mean for standardized QAg1/T scores is significantly higher than for the standardized peak velocity scores. This highlights how difficult it would be to find statistical significance surrounding EMG measures even given the large differences in peak velocity found between the groups. Previous studies of EMG abnormalities in dystonia have been limited (Cohen & Hallett, 1988; Rothwell et al., 1983; van der Kamp et al., 1989) and mainly descriptive, probably for this very reason. Recruiting significantly more participants with FHD may still not result in statistical significance given the level of variability in EMG data. We therefore suggest that future studies that aim to show EMG changes in FHD may consider using a movement task that requires greater muscle force. For example, a higher inertial load would increase muscle activation demands and therefore may show up impairments more clearly.

In conclusion, we found that not only are individuals with FHD slower than controls, they also show impaired scaling of movement velocity across movement distance such that longer movements are associated with a greater degree of slowing in FHD. EMG patterns can show a triphasic pattern of activation, and agonist and antagonist activity can scale with movement distance in a manner similar to that of scaling in controls. Underactivation of the first agonist burst appears to be the most likely reason that accounts for movement slowing in FHD.

Biographies

Biographical Notes

Janey Prodoehl is a postdoctoral research associate in the Department of Kinesiology and Nutrition at the University of Illinois at Chicago. Her research interests include the role of disordered sensorimotor integration and basal ganglia dysfunction in explaining pathological motor control.

Daniel M. Corcos has been a professor since l997 at the University of Illinois at Chicago. He was executive editor of the Journal of Motor Behavior from 1996 to 2004 and is currently the editor for Rapid Communications.

Sue Leurgans is on the faculty of Rush University, serving as professor of preventive medicine since 1992 and of neurological sciences since 2000. She has taught a variety of statistics courses, with a particular interest in the uses of modern multivariate statistical methods in the quantification of human motion and the study of neurological disorders.

Cynthia L. Comella is a professor of neurological sciences at Rush University Medical Center in Chicago. Her clinical research focuses on Parkinson’s disease, dystonia, and sleep-related movement disorders.

Annette Weis-McNulty was involved in researching basal ganglia dysfunction as explanation for pathological motor control in individuals with dystonia and Parkinson’s disease at Northwestern University in collaboration with the University of Illinois at Chicago. Since 2005, she has continued her medical career in diagnostic radiology with a residency at the University of Illinois at Chicago.

Colum D. MacKinnon is an assistant professor in the department of physical therapy and human movement sciences at Northwestern University. His research interests are in the cortical and subcortical control of movement initiation and execution in individuals with diseases that affect the basal ganglia.