Defining frequent use of an urban emergency department

Abstract

Objective

This study aimed to develop a definition of frequent use of an emergency department (ED) by comparing differences in the observed frequency distribution with that of a theoretical frequency distribution.

Methods

A retrospective analysis of attendance of ED and minor injury unit attendances in one city over 1 year was conducted. From these data, the expected frequency distribution was determined based upon a Poisson distribution.

Results

During the period studied, 75 141 people attended on 98 908 occasions. The theoretical frequency distribution showed that there were 2764 (3.7%) “frequent users” presenting repeatedly due to non‐random events. These patients made 12 316 (12.4%) attendances. Frequent users were older than chance users (mean age 49.7 vs 44.5 years). A greater proportion arrived by ambulance (55.3% vs 27.5%), presented with psychiatric problems (5.8% vs 1.1%) or alcohol intoxication (1.3% vs 0.5%), and were admitted to hospital (37.4% vs 19.6%).

Conclusion

We have identified that there is a group of patients who present repeatedly due to non‐random events, confirming the existence of “frequent users”. Their characteristics are clearly different to other patients in the ED. We propose that “frequent users” be defined as any patient who makes more than four attendances per year.

It is recognised that a small number of patients make frequent use of the emergency department (ED) and account for a disproportionate amount of the total ED workload.¹ Several investigators have studied this area previously. In most cases, the definition of a frequent user has been arbitrarily based upon the number of attendances within a given time frame. The definitions used vary, ranging from 3 to 12 attendances within a year (table 1).¹,²,³,⁴,⁵,⁶,⁷,⁸,⁹,¹⁰,¹¹,¹²,¹³

Table 1 Published definitions of frequent users of EDs.

Authors	Country	Definition*
Lucas and Sanford4	USA	2 in the previous month or 4 in the previous year
Zuckerman and Shen13	USA	3
Genell Andren and Rosenqvist2	Sweden	4
Hansagi et al7	Sweden	4
Olsson and Hansagi8	Sweden	4
Byrne et al11	Ireland	4
Sun et al12	USA	4
Mandelberg et al1	USA	5
Williams et al9	UK	7
Kne et al3	USA	11
Chan and Ovens10	Canada	12
Lynch and Greave5	UK	6 in 6 months
Pope et al6	Canada	Several attendances and potential for heavy future use

*Minimum attendances per year unless otherwise stated.

With changing levels of attendance at EDs, such definitions may not be appropriate today. Equally, differing definitions make comparisons between studies and between different healthcare systems difficult. Correct identification of frequent users of an ED may permit the use of targeted interventions to meet their healthcare needs.

This study aimed to determine the frequency with which people attend the ED and minor injury unit (MIU) in one city and from this determine a definition of frequent use based upon a theoretical distribution of frequency of attendance. Using this definition, we aimed to compare the demographic and clinical characteristics of “frequent” and “chance” users.

METHODS

A retrospective study of routinely collected data from one ED and one MIU in a UK city was conducted. Approval from the research ethics committee was not required for this study.

All patients attending during the 12‐month period of January–December 2003 were included in the study. Both departments were included because the area served by the MIU lies entirely within that served by the ED. The ED and MIU do not treat paediatric cases, which are handled by a separate paediatric ED, and therefore only those patients aged ⩾16 years on their initial presentation were included in the analysis. Planned follow‐up episodes were excluded.

For each patient, the number of occasions on which they had attended the ED or MIU during the study period was determined. Age at first presentation, marital status, and sex were retrieved from the ED/MIU database. For each attendance, the reason for presentation and the outcome of the patient (admission or discharge) from the ED or MIU were determined. The total duration of each episode, defined as the time from arrival until the time at which the patient left the ED or MIU, was calculated.

Data analysis

To analyse frequency of attendance, the ED and MIU were considered as one department. The mean rate of attendance per patient (X) over the year studied was calculated.

To determine the theoretical distribution of frequency of attendance it was initially assumed that the events causing the patient to attend are independent, random events. Consequently, we expect some patients to attend more than once because they have more than one randomly occurring event in the year. Some patients will by chance have three or perhaps four events, for example. If these events are truly random, rather than clustering in frequent attenders, the number of attendances should follow a Poisson distribution.¹⁴ As only those people attending at least once during the period studied were included, the frequency of attendance was therefore assumed to follow a truncated Poisson distribution, with zero attendances not allowed. The probability of a person making x attendances during a year purely as a result of independent chance events, given that they make at least one attendance, can then be calculated from the formula for a truncated Poisson distribution and the observed mean rate of attendance (X) (details of the method and formulae are given in the Appendix). The expected number of patients attending x times over a year, if all attendances are the result of random events, can then be determined by multiplying the probabilities by the total number of patients included in the study. Hereafter, this part of the analysis is referred to as method 1.

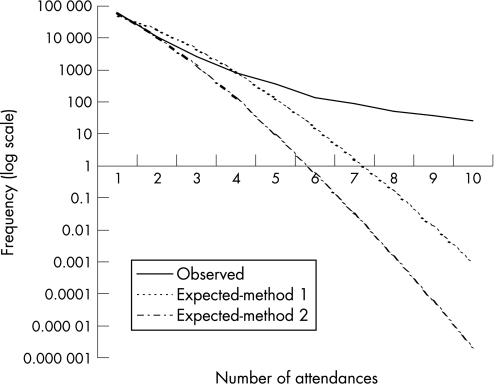
The expected frequencies calculated using method 1 were plotted against the observed frequencies and the two curves were found to cross twice, suggesting that the expected frequencies did not fit our observations and that the assumption that all attendances results from random events is incorrect.

If instead it is assumed that there are some frequent attenders who present due to non‐random events and it is assumed that a user must attend on more than two occasions per year to count as a frequent attender, then the observed frequencies of attendances for patients presenting on more than two occasions will comprise both chance attenders presenting due to random events, and patients who are frequent users presenting due to non‐random events.

By definition, the number of patients attending on one or two occasions must consist entirely of chance attenders, and these two numbers can be used to calculate the rate of true chance attendance, again based on a truncated Poisson distribution. As before, the resulting probabilities can be used to calculate the expected number of attenders attending purely by chance on x occasions in the year. The difference between these numbers and the observed numbers can then be used to calculate the estimated number of frequent attenders. The results obtained by this method are referred to as method 2 below.

To compare differences between chance and frequent attenders in characteristics such as age, sex and duration of episodes, the mean characteristics for chance and frequent attenders were estimated using an iterative process based upon the mean characteristic of all patients attending on x occasions and the proportion of patients attending on x occasions who were determined to be either chance or frequent users by method 2.

RESULTS

During the period studied there were 98 908 attendances, of which 82.9% (n = 81 985) were attendances to the ED, with the remainder attending the MIU. These attendances were made by 75 141 individual patients. The mean number of attendances per patient was 1.32 (maximum 104 attendances).

Table 2 shows the observed frequency of attendance and the expected frequencies determined using the two different methods and fig 1 shows this graphically. The expected frequencies given by method 1 do not fit the observed distribution, suggesting that not all attendances can be due to random events. The calculated frequencies determined from method 2, by definition, fit the observed distribution perfectly for patients attending on one or two occasions per year. The expected frequency of chance attenders falls rapidly with increasing number of attendances per year. Of the patients observed to attend on three occasions per year, 51.6% (n = 1365) could be expected to do so by chance, whereas for patients attending six times per year only 0.45% (n = 1) could be expected to do so by chance. The total number of patients determined to be presenting due to chance was 72 377 (96.3%) and thus there were 2764 (3.7%) frequent attenders.

Table 2 Observed frequency of attendance and expected frequencies calculated from the two methods used .

Attendances (n)	Observed frequency	Expected frequencies
		Method 1	Method 2
			Chance attenders	Frequent attenders
1	59810	50767	59810	0
2	11066	18757	11066	0
3	2646	4620	1365	1281
4	825	853	126	699
5	371	126	9	362
6	133	15.5	0.6	132.4
7	90	1.640	0.030	89.97
8	51	0.151	0.001	51.00
9	37	0.012	0.0	37.00
10	26	0.001	0.0	26.00
11	14	0.0	0.0	14.00
12	18	0.0	0.0	18.00
13	13	0.0	0.0	13.00
14	11	0.0	0.0	11.00
15	3	0.0	0.0	3.00
⩾16	27	–	–	–

Figure 1 Observed and expected frequencies of attendance per year.

Frequent attenders made 12316 (12.4%) attendances. Table 3 shows the characteristics of chance and frequent users and their episodes of care. Chance users are younger and more likely to be married. A lower proportion arrives by ambulance and is admitted to hospital. A greater proportion of attendances made by frequent users is due to psychiatric problems or related to alcohol.

Table 3 Characteristics of frequent and chance users and their episodes of care.

	Chance users	Frequent users
Patient characteristics (n = 75141)
Mean age (years)	44.5	49.7
Male	53.8%	54.2%
Married	37.9%	25.2%
Episode characteristics (n = 98908)
Arrived by ambulance	27.5%	55.3%
Presenting complaint
Psychiatric	1.1%	5.8%
Alcohol intoxication	0.5%	1.3%
Trauma	54.2%	22.8%
Attended ED rather than MIU	81.2%	94.6%
Admitted to hospital	19.6%	37.4%
Mean total duration of episode (mins)*
Admitted	289.1	295.9
Discharged	137.5	184.1

ED, emergency department; MIU, minor injury unit.

*ED attenders only.

The mean total duration of each attendance was calculated for frequent and chance users presenting to the ED. As the number of attendances made by frequent users to the MIU was disproportionately small and waiting times are known to be considerably lower at this facility, episodes presenting to this facility were not included in this part of the analysis. The mean duration of episodes was compared separately for admitted and discharged patients as the pattern of disposal differs between the two groups (table 3). The mean duration of episodes was similar for patients admitted to hospital; however, for those episodes resulting in discharge from the ED, the mean episode duration of frequent users was almost 40 minutes greater than that of chance users.

DISCUSSION

The above distributions demonstrate that not all of the observed attendances can be due to random events and that the concept of a “frequent user” is a genuine one, representing those patients who attend on multiple occasions due to non‐random events. There is no clear cut‐off between chance and frequent users from the expected frequency distribution, as the two distributions in theory continue to infinity. Any cut‐off point must therefore be an arbitrary one. Table 2 shows that 99.99% of chance attenders would be expected to present on ⩽4 occasions per year. A department could therefore determine a threshold based upon the chance of incorrectly classifying a chance user as a frequent user. From the cohort of patients presented above we suggest defining a frequent user as any person who makes >4 attendances per year.

The theoretical distributions determined from method 2 identify two groups of patients, chance users and frequent users, whose clinical characteristics are clearly different. Frequent users are older and less likely to be married than chance users, findings that are in keeping with previous studies.⁵,⁹,¹³ Differences in the cause of presentation exist between the two groups, with frequent users presenting less with injuries than chance users. This probably reflects the high incidence of chronic medical problems previously reported in such cohorts²,¹² and the fact that accidents are more likely to be chance events.

Published evidence suggests that the cause of frequent use of an ED is multifactorial. Although many patients have chronic medical problems, this is often in combination with significant psychosocial morbidity.² Byrne et al have demonstrated that when assessed using the General Health Questionnaire‐12, 72% of frequent users studied showed “clinically significant levels of psychiatric morbidity”, and showed lower perceived levels of social support than infrequent users.¹¹ Olsson and Hansagi, in a qualitative study of frequent ED users, found that for the majority of participants, “their current social circumstances seem precarious, even though none is homeless or totally lacking in means.”⁸

In the current climate of increasing ED utilisation, achieving reductions in workload by decreasing the rate of attendance of frequent users or diverting them to other healthcare services may seem attractive. However, this strategy may not be without risk. One longitudinal study of frequent users found that in the year following commencement of the study, the standardized mortality rate of frequent users was 590% and 740% for men and women respectively.² Frequent users of the ED also tend to demonstrate increased use of other healthcare services⁷,¹¹ and it may therefore not be productive to attempt merely to divert attendances from the ED.

This paper presents a robust method by which frequent users may be identified and therefore provides an opportunity to target specific services at such patients, whom one must assume currently have unmet healthcare needs. Pope et al have shown that a reduction in attendance by frequent ED users can be achieved through the use of an individualised case management programme.⁶ This strategy was multidisciplinary, involving ED, hospital and community medical and social care staff. The median number of visits made by patients enrolled in the programme reduced from 26.5 to 6.5 per year. A notable limitation of this approach, however, is the time involved to implement it, with the authors having to limit new referrals to four per month for this reason. Previous investigators using a similar approach failed to demonstrate a reduction in ED use.¹⁵

Genell Andren and Rosenqvist have demonstrated that without intervention, a number of frequent users of the ED becomes infrequent users over time. In their study, from an initial cohort of 232 patients attending ⩾4 times per year, at the end of a 2‐year follow‐up, 159 patients were alive and living in the area, of whom 43 remained heavy users of the ED.² Other investigators have found similar patterns of reduced use, demonstrating that only 17% of patients remained frequent users of the ED over a 4‐year period.³

In conclusion, it is clear that there is a subset of ED patients who present repeatedly due to non‐random events. The needs of this group are very different from those of other ED patients and they represent a cohort of patients that are at considerable risk.

Abbreviations

ED - emergency department

MIU - minor injuries unit

APPENDIX A

CALCULATION OF RATE OF CHANCE ATTENDANCE

For the purpose of analysing frequency of attendance, the ED and MIU were considered as one department. The mean rate of attendance per patient (X̄) over the year studied was calculated.

To determine the theoretical distribution of frequency of attendance, it was initially assumed that the events causing the patient to attend are independent, random events. Consequently, we expect some patients to attend more than once because they have more than one randomly occurring event in the year. Some patients will by chance have three or perhaps four events, for example. If these events are truly random, rather than clustering in ‘frequent' attenders, the number of attendances should follow a Poisson distribution.¹⁴ As only those people attending at least once during the period studied were included, the frequency of attendance was therefore assumed to follow a truncated Poisson distribution with zero attendances not allowed. The probability of a person making ‘x' attendances during a year (π^x(λ)) purely as a result of independent chance events, given that they make at least one attendance, was calculated according to the formula:¹⁴

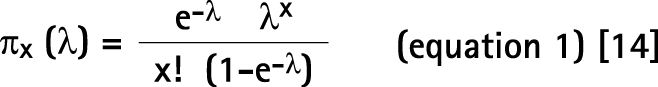To determine the expected probabilities, an estimate of λ is required. If it is assumed that all attendances occur as random events then an estimate of the rate of chance attendances (_λc) is given by the equation below, where X̄ is the mean rate of attendance per patient over the period studied and j takes integer values from 1 to infinity.

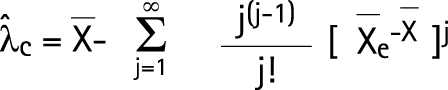The expected number of patients attending x times over a year, if all attendances are the result of random events, can then be determined by multiplying the probabilities determined from equation 1 above by the total number of patients included in the study. This part of the analysis is referred to as method 1 in the paper.

The expected frequencies calculated above were plotted against the observed frequencies and the two curves found to cross twice, suggesting that the expected frequencies did not fit our observations and that the assumption that all attendances results from random events is incorrect. An alternative estimate of λ_c was therefore sought.

If it is assumed that frequent users must attend on more than two occasions per year, then the observed frequencies of attendances for patients presenting on more than two occasions will comprise both ‘”chance attenders” presenting due to random events, and patients presenting due to non‐random events—that is, ‘frequent users'. By definition, the number of patients attending on one (n₁) or two (n₂) occasions must consist entirely of chance attenders. The number of patients making one (n₁) or two (n₂) attendances is given by the probability of patients making one (π₁(λ)) or two attendances (π₂(λ)) multiplied by the total number of patients included in the study. Therefore:

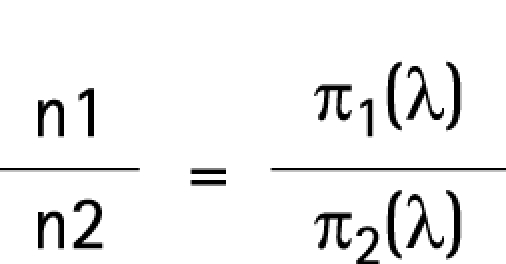Substituting the right side of equation 1 for π₁(λ) and π₂(λ) the above formula becomes:

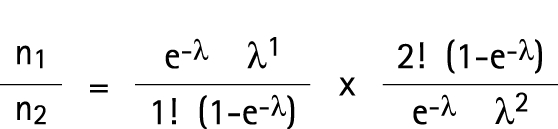Which can therefore be rearranged to give the estimate of λ that describes the frequency distribution of chance attenders, λ_c:

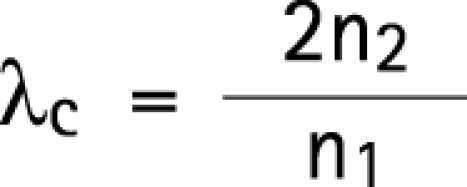The expected probabilities of a person making x attendances were recalculated from equation 1 using the new estimate of λ_c. To calculate the expected frequencies the total number of chance attenders ‘n_tc' must be determined. As n₁, n₂, π₁(λ_c) and π₂(λ_c) are all known, then:

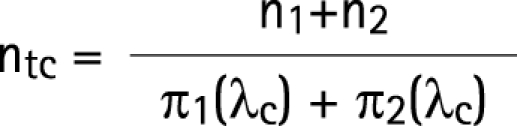Therefore, multiplying the expected probabilities by n_tc gives the expected frequency distribution of chance attenders, with the difference between this and the observed frequency giving the expected frequency distribution of frequent attenders. The results obtained by this method are referred to as method 2 in the paper.