Motor adaptation during dorsiflexion-assisted walking with a powered orthosis

Abstract

A robotic ankle-foot orthosis (AFO) that provides powered assistance could adjust to varying gait dynamics much better than a rigid AFO. To provide insight into how humans would adapt to a powered AFO, we studied the response of neurologically intact subjects walking with an active dorsiflexion assist orthosis proportionally controlled by tibialis anterior electromyography (EMG). We examined the two mechanical functions of ankle dorsiflexors in gait (power absorption at heel strike and power generation at toe-off) by recruiting two groups of healthy subjects: Group One, called Continuous Control, (n=5) had dorsiflexion assistance both at the initial heel contact and during swing; Group Two, called Swing Control, (n=5) had the assistance only during swing. We hypothesized both groups of subjects would reduce tibialis anterior EMG amplitude with practice walking with the powered dorsiflexion assist. Ten healthy subjects were fitted with custom-made orthoses that included an artificial pneumatic muscle providing dorsiflexor torque. We collected lower body kinematics, EMG, and artificial muscle force while subjects walked on a treadmill for two 30-minute training sessions. We found that subjects walked with increased ankle dorsiflexion by 9 degrees but showed different adaptation responses of the two tibialis anterior EMG bursts. The first EMG burst around heel strike had ~28% lower amplitudes (p<0.05) but the second EMG burst during swing had similar amplitudes. These results provide baseline data of EMG controlled dorsiflexion assist in neurologically intact humans that can be used to guide future studies on neurologically impaired individuals.

Introduction

Rigid ankle-foot orthoses (AFO) are frequently prescribed for patients with very weak dorsiflexor recruitment to improve walking ability and prevent tripping. However, drawbacks to rigid AFOs are that they impede active plantar flexion at push-off and do not allow users to make step-to-step changes in motion dynamics (e.g. altering speed, adapting to surface terrain, etc). Many research groups are developing powered orthoses or robotic exoskeletons to help people with gait deficits [1–3] or to augment human performance [4–6]. The orthoses could be used as everyday gait aids to improve mobility or as rehabilitation tools to shape the motor patterns of patients [3]. A critical aspect dictating the success of robotic orthoses for either purpose is how people react to the active devices. Understanding the human response should make it easier to design powered orthoses that achieve either of the goals.

The purpose of this study was to examine how healthy, neurologically intact humans adapt their walking patterns to a powered orthosis that provides dorsiflexion assistance. The advantage of a powered AFO with dorsiflexion assist is that it would not impede plantar flexion at the end of stance and would allow users to make alterations in their gait to accommodate changes in the environment. We used proportional myoelectric control of the powered orthosis because our prior research has shown that humans can quickly adapt to plantar flexion assistance with proportional myoelectric control [7]. The current study allowed us to examine the same type of motor adaptation test for the dorsiflexors.

The tibialis anterior has two main bursts of activity during gait: one at heel strike to slowly lower the foot to the ground and one at toe-off to help provide toe clearance during swing. The former provides mechanical power absorption at the ankle joint and the latter provides mechanical power generation at the ankle joint. To examine these different mechanical functions, we recruited two groups of healthy subjects: (1) one group of subjects received active dorsiflexion assistance both at heel strike and during swing – Continuous Control; (2) another group of subjects received assistance only during swing – Swing Control. Assistance provided at all phases of the gait cycle (Continuous Control) is easiest from an engineering perspective because ongoing EMG signals throughout gait cycle can be used to control timing and amplitude of the assistance. However, our previous studies using artificial pneumatic muscles to power robotic lower limb orthoses have suggested that the human nervous system tends to avoid activating the orthoses for power absorption [7, 8]. As a result, we also wanted to test a control mode that would rely on the orthosis only for power generation. The swing control group allowed us to test if assistance given only during power generation phase (i.e. stance-to-swing transition) resulted in different motor adaptation than assistance during both phases.

We hypothesized that both groups would reduce tibialis anterior EMG amplitude and return to normal joint kinematics with practice walking with the powered dorsiflexion assist. Gordon and Ferris [7] found that healthy subjects walking with a powered orthosis providing plantar flexion assistance under proportional myoelectric control of soleus reduced soleus EMG by ~35% to walk with nearly normal joint kinematics in just two 30-minute sessions. We generated our hypothesis and study protocol based on these prior results studying human locomotor adaptation to a powered orthosis [7].

Methods

Subjects

Ten healthy subjects (five male, five female, age: 18–31 years) gave written informed consent and participated in the study. The University of Michigan Medical School Institutional Review Board approved the protocol.

Experimental design

We constructed a custom-made orthosis (Fig. 1) for left lower limb of each subject. The orthosis consisted of polypropylene shank and foot sections. An artificial pneumatic muscle attached on the two portions powers the orthosis for providing augmented dorsiflexor torque. We implemented proportional myoelectric control (i.e., amplitude and timing) of the artificial muscle through desktop computer and real-time control board (dSPACE Inc.). A custom software program regulated air pressure in the artificial muscle proportional to the processed tibialis anterior EMG. EMG signal from tibialis anterior was high-pass filtered with a second-order Butterworth filter (20-Hz cutoff frequency) to remove movement artifact, full wave rectified, and low-pass filtered with a second-order Butterworth filter (10-Hz cutoff frequency) to smooth the signal. Adjustable gains scaled the control signals and a threshold cutoff eliminated background noise. In Continuous Control, control signals came from the processed tibialis anterior EMG signals throughout the gait cycle. In Swing Control, the right-side footswitch was used for gating control signals.

Fig 1.

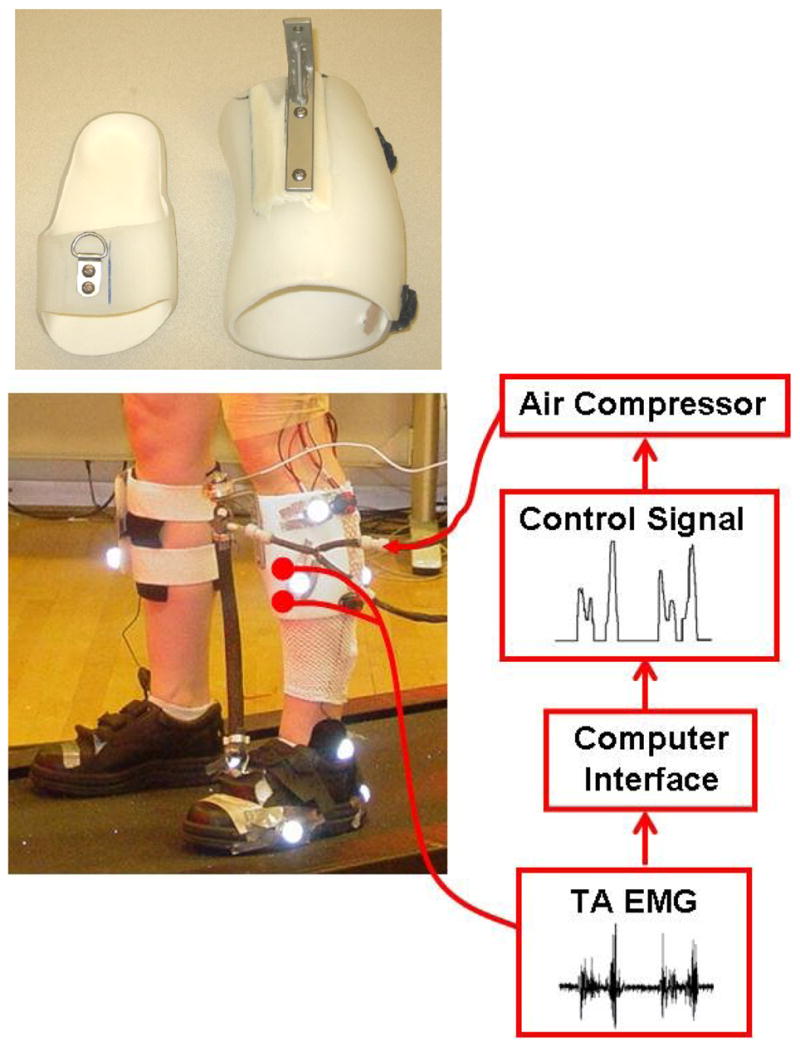
Subjects wore a custom fit orthosis on their left lower limb. The orthosis consisted of polypropylene shank and foot sections. The moment arm length (13.9±0.8 cm, mean±SD) and the pneumatic muscle length (38.4±1.8 cm, mean±SD) varied and depended on the feet size and leg length of the subject. Electrical signals (EMG) of tibialis anterior were recorded and processed to be used to control air pressure in the artificial pneumatic muscle proportionally. As air pressure increased, the artificial muscle started to develop tension and shortened, allowing the powered orthosis to provide dorsiflexor torque controlled by tibialis anterior muscle activation.

Subjects completed two identical testing sessions 72 hours apart. During each session, subjects walked with the orthosis first without power for 10 minutes (baseline), with power for 30 minutes (active), and without power again for 15 minutes (post-adaptation). Before testing, subjects were not given any practice walking with the orthosis. We chose this testing protocol to match a similar study from our laboratory testing the effects of powered plantar flexion assistance using soleus proportional myoelectric control [7].

Data acquisition and analysis

We recorded lower body kinematics, artificial muscle force, and electromyography during the first 10 seconds (~7 full strides) of every minute while subjects walked on a treadmill at 1.25 m/s. The three-dimensional kinematic data were collected by using 8-camera video system (120 Hz, Motion Analysis Corporation, Santa Rosa, CA). Artificial muscle force data were collected with a force transducer (1200 Hz, Omega Engineering). We placed bipolar surface electrodes on the left lower limb to record EMGs (1200 Hz, Konigsberg Instruments Inc.) from tibialis anterior (TA), soleus (SOL), medial gastrocnemius (MG), lateral gastrocnemius (LG), vastus lateralis (VL), vastus medialis (VM), rectus femoris (RF) and medial hamstring muscles (MH). Electrode position was marked on subjects using permanent ink to assure consistent placement.

All data were time normalized to 100% of stride cycle (i.e., from left heel strike to its next one). To quantify changes in muscle activation, we calculated root-mean-square (RMS) amplitude of the high-pass filtered (20-Hz cutoff frequency) and rectified EMG for each burst of tibialis anterior individually. For the first burst, we calculated sum of the RMS over 90–100% and 0–10% of gait cycle. For the second burst, we calculated RMS over 50–90% of gait cycle. For all other muscles, we calculated RMS over whole gait cycle. We normalized them to the last minute of baseline on a given testing session.

The joint angles were calculated from smoothed marker data (4^th order Butterworth zero-lag low-pass filter, 6-Hz cutoff frequency). To examine changes in kinematics across time for ankle, knee and hip joint angles, we linearly correlated the average joint angle profile during the powered condition at each minute to the average joint angle profile at the last minute of baseline on a given testing session using Pearson product moment correlation. As 10 seconds of data were recorded each minute, the correlations reflected the average of about 7 strides of data. The common variance (r²) of the correlation was used as a quantitative measure of similarity in joint kinematics. This method has been quantitatively assessed for validity by Derrick et al (1994) [9].

There are many ways to quantify motor adaptation when studying human movement. A common approach is to use exponential or power law fits of a behavioral parameter. The main drawback to this approach is that mounting literature has established that there are at least two independent processes with different time scales underlying motor adaptation [10]. As a result, quantifying an adaptation rate from an exponential or power fit is inherently flawed. We chose to use a measure of performance variability to quantify adaptation rate [11]. For data with a normal distribution, approximately 95% of all values should lie within the mean ± two standard deviations. Based on this rule of probability, we have defined steady state performance using the mean ± two standard deviations of data from the last 15 minutes on the second day of testing. The slope of a linear regression fit to the last 15 data points on Day 2 was not significantly different from zero (t-test, p>0.05). Using time to steady state as the measure of motor adaptation rate does not make any a priori assumptions about the shape of the motor adaptation data or the number of processes with different time scales involved in the motor adaptation. The method is described in more detail in Noble and Prentice [11] and Gordon and Ferris [7]. We calculated time to steady state for the tibialis anterior RMS EMG and the ankle angle correlation.

To estimate the amount of assistance provided (e.g. percent of normal dorsiflexor torque during walking) by the powered orthosis, we calculated net ankle joint torque and power from ten trials of over-ground walking at 1.25 m/s without wearing orthosis. We used commercial software (Visual3D, C-Motion Inc.) to perform inverse dynamic calculations. Lower limb inertial properties were estimated based on anthropometric measurements of subjects [12].

Statistics

We used repeated measure factorial ANOVAs (2-way) to test for differences in normalized EMG RMS (primary outcome variables were the two bursts of tibialis anterior EMG), joint angle correlation common variances (primary outcome variable was for the ankle joint) between days and conditions (baseline, powered walking minute 1, 15 and 30) (2 days × 4 conditions). Thus, we had three primary outcome variables. We analyzed other parameters as secondary outcome variables to provide insight into the overall adaptation. Included in the secondary outcome variables were the differences in adaptation periods of the two bursts of tibialis anterior EMG RMS and the ankle joint angle correlation common variance between days and groups. We used additional repeated measure factorial ANOVAs (2 days × 2 groups) for the adaptation periods. We set the significance level at p<0.05 and used Tukey Honestly Significant Difference (THSD) post hoc tests for pair-wise comparisons if a main effect was detected.

Results

Joint Kinematics

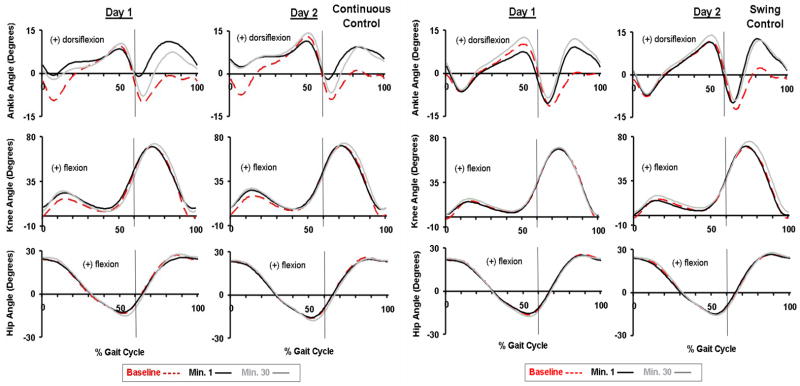
Subjects walked with substantially increased ankle dorsiflexion when the assistance was provided (Fig. 2). Continuous Control subjects increased ankle dorsiflexion both at initial heel contact and during swing by ~9 degrees. Continuous Control subjects also increased knee flexion (by ~8 degrees) during initial stance along with the increased dorsiflexion to walk in a slightly more crouched posture. Swing Control subjects also increased ankle dorsiflexion during swing by ~9 degrees.

Fig 2.

Joint kinematics. Ankle, knee and hip joint kinematic patterns are shown for the last minute of baseline condition (baseline, red dashed line), the first minute of active condition (minute 1, black line), and the last minute of active condition (minute 30, grey line) on the two training sessions for Continuous Control (n=5) and Swing Control (n=5) subjects. Data are the average of all subjects of each group. The vertical lines represent the toe-off. The ankle joint angle profiles showed greatly increased dorsiflexion by ~9 degrees around the initial heel contact for Continuous Control, and swing phase for both groups on both days. The hip and knee joint angle profiles were similar to the baseline across all testing conditions.

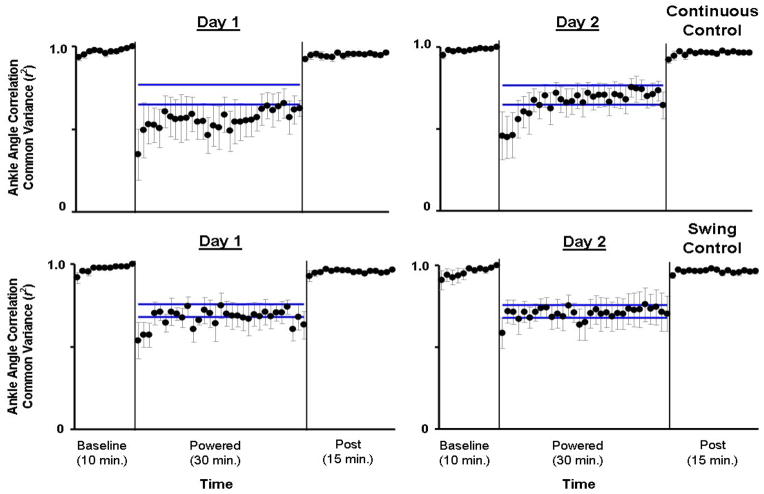
Although both groups of subjects showed some adaptation over the 30-minute session, there were still large differences in ankle joint kinematics between steady state assistance at the end of Day 2 and baseline. For both groups, the ankle angle correlation common variance (r²) at the first minute was the lowest during all testing (Fig. 3). Continuous Control subjects had reduced plantar flexion at push-off during initial powered walking but returned to nearly normal plantar flexion by the end of Day 2. Correspondingly, ankle angle correlation common variance was higher at minute 30 compared to minute 1 for Continuous Control (Fig. 3). However, throughout active trials of both days, ankle angle correlation common variance of Continuous Control subjects was significantly different from baseline (ANOVA, p<0.001, Power=1.00) due to augmented dorsiflexion at initial stance and mid- to late-swing. Swing Control subjects increased ankle dorsiflexion during mid- to late-swing without reducing plantar flexion at push-off. Swing Control ankle angle correlation common variance throughout active trials was also significantly different from baseline (ANOVA, p<0.001, Power=1.00) due to consistent augmented dorsiflexion.

Fig 3.

Ankle joint angle correlation common variance (r²). Mean data (black dots) ± 1 standard error of mean (grey bar) are shown for each minute. The two horizontal blue lines are the mean ± 2 standard deviations from the last 15 minutes of active condition on day 2, representing steady state dynamics. The steady state envelopes shown above from the averaged group data are for display purposes. Steady state dynamics were determined for each subject, individually. Ankle joint angle correlation common variance (r²) increased with practice in the active condition. However, by the end of active condition on Day 2 (Day 2, minute 30), the values of ankle joint angle correlation common variance in both groups were still significantly different from baseline (mean±SD: Continuous Control: 0.64±0.19, Swing Control: 0.70±0.24, THSD, p<0.05).

There were no large differences in knee or hip joint angle profiles for either group with one exception. Continuous Control had greater knee flexion (~8 degrees) during initial stance (p=0.098). Knee and hip joint angle correlation common variances were always greater than 0.96 for all powered trials. There were no significant differences (THSD, p>0.05) between active and baseline conditions in the knee (day 2, minute 30: Continuous Control 0.97±0.02, Swing Control 0.98±0.03, mean±SD) or hip (day 2, minute 30: Continuous Control 0.99±0.01, Swing Control 0.99±0.01) joint angle correlation common variance (r²) for either group after 30-min training.

Orthosis Mechanics

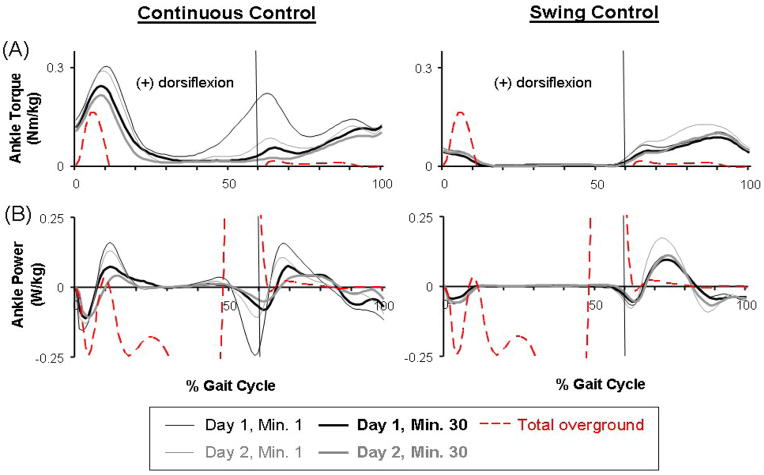
The powered orthosis provided greater peak ankle dorsiflexor torques (Fig. 4) than those normally occurring during walking. At the end of Day 2, the orthosis provided peak dorsiflexor torques of 0.22±0.14 Nm/kg (mean±SD) for Continuous Control subjects around initial heel contact, and 0.12±0.09 Nm/kg for Continuous Control and 0.11±0.06 Nm/kg for Swing Control subjects during swing. The normal peak dorsiflexor torque during over-ground walking for the ten subjects was 0.17±0.07 Nm/kg at heel strike and 0.02±0.00 Nm/kg at swing (mean±SD).

Fig 4.

Mechanics of the powered orthosis. (A) Dorsiflexor torque provided by the orthosis. These averaged data (black and grey lines) from all subjects of each group represent only the portion of ankle torque produced by the orthosis (calculated from artificial muscle force and muscle moment arm) during active condition (the first and last minute on both days). Average ankle torque (red dashed line) during overground walking with no orthosis was calculated from inverse dynamics. The overground walking data shown above is zoomed in to the similar range of the powered orthosis data due to relatively small dorsiflexor torque production during normal walking. (B) Dorsiflexor power provided by the orthosis. The positive value represents power generation and negative value represents power absorption. These averaged data (black and grey lines) from all subjects of each group represent only the portion of ankle power produced by the orthosis during active condition. Average ankle power (red dashed line) was calculated from inverse dynamics during overground walking with no orthosis.

Electromyography (EMG)

During active trials, the shape of the tibialis anterior EMG patterns was similar to baseline trials (Fig. 5) but there were fluctuations in EMG amplitudes. For minute 1 on Day 1, Continuous Control subjects had significantly lower tibialis anterior EMG amplitudes for the first but not the second burst. The Continuous Control reduction in the first burst became more marked on the second day (ANOVA, p<0.001, Power=0.97) (Fig. 5). Compared to baseline, the first tibialis anterior EMG burst had ~28% lower amplitudes at the end of powered walking (day 2, minute 30: 0.72±0.15, mean±SD, THSD, p<0.05). The second tibialis anterior EMG burst was similar in amplitude at the end of the second day (0.86±0.17, THSD, p>0.05). In Swing Control subjects, there was a similar response for the second tibialis anterior EMG burst. On Day 2, the second tibialis anterior EMG burst amplitude was similar to baseline for Swing Control (1.08±0.08 for minute 1 and 1.15±0.28 for minute 30, THSD, p>0.05).

Fig 5.

Tibialis anterior activation patterns (A) and tibialis anterior EMG RMS amplitudes of individual bursts (B and C). (A) Tibialis anterior EMG linear envelopes (rectified and low-pass filtered EMG with 6-Hz cutoff frequency) were averaged from all subjects of each group. During initial walking with the powered orthosis (day 1, minute 1, black line), Continuous Control subjects had lower EMG amplitude of the first burst (swing-to-stance transition, period of pink bar) but had greater EMG amplitude of the second burst (stance-to-swing transition, period of light grey bar with oblique lines). By the end of powered walking on the second day (day 2, minute 30, grey line), the amplitude of the first burst remained reduced but the second burst was similar to the baseline (baseline, red dashed line). During initial walking with the powered orthosis (day 1, minute 1), the amplitude of the first burst in Swing Control also reduced but became similar to the baseline after one training session. In addition, the second burst in Swing Control was similar to the baseline. (B) EMG RMS amplitudes of the first tibialis anterior burst (90–100% and 0–10% of the gait cycle); and (C) EMG RMS amplitudes of the second tibialis anterior burst (50–90% of the gait cycle). Mean data (black dots) ± 1 standard error of mean (grey bar) are shown for each minute. The two horizontal blue lines are the mean ± 2 standard deviations from the last 15 minutes of active condition on day 2, representing steady state dynamics. The steady state envelopes shown above from the averaged group data are for display purposes. Steady state dynamics were determined by each subject, individually.

There were some increases in EMG amplitudes for other lower limb muscles during initial activation but the EMG amplitudes at the end of Day 2 were similar to baseline values. In Continuous Control, soleus, medial gastrocnemius, lateral gastrocnemius, rectus femoris, and medial hamstrings demonstrated increased activation during the initial powered walking. In Swing Control, there were no substantial changes in other lower limb EMG amplitudes. By the end of Day 2, both groups had similar shapes of muscle activation patterns to baseline during powered walking.

Adaptation Rates

Both groups of subjects reached steady state in both kinematics and tibialis anterior EMG amplitude within the two-day training but demonstrated faster adaptation on Day 2 than on Day 1. For ankle angle correlation common variance, both groups reached steady state more rapidly in the second session (ANOVA, p<0.001, Power=1.00) (mean±SD: Day One 28.6±3.1 min, Day Two 6.4±5.5 min for Continuous Control; Day One 29.6±0.9 min, Day Two 11.8±6.3 min for Swing Control) but there was no significant difference between the two groups in time to steady state (THSD, p>0.05) on both days. For tibialis anterior EMG amplitudes, both groups reached steady state more rapidly on Day 2 than Day 1 for both bursts (ANOVA, p=0.001, Power=0.96). For the first tibialis anterior EMG burst, Swing Control subjects reached steady state faster than Continuous Control subjects on Day 1 (THSD, p<0.05) (Day One 25.0±4.7 min, Day Two 8.8±5.1 min for Continuous Control; Day One 12.6±13.6 min, Day Two 0.6±0.9 min for Swing Control) but there was no significant difference in adaptation rates for the second EMG burst (THSD, p>0.05) (Day One 23.8±9.1 min, Day Two 5.2±4.9 min for Continuous Control; Day One 18.6±15.6 min, Day Two 3.4±4.7 min for Swing Control).

Discussion

The powered orthosis provided substantial ankle dorsiflexor torque at the target phases. The ankle kinematics, torque and power data (Fig. 2, 4) indicated that the powered orthosis reduced plantar flexion at initial heel contact and augmented dorsiflexion during swing phase. Development of a portable dorsiflexion assist orthosis that provides similar torques as the one tested in this study may help people with weak ankle dorsiflexors to reduce foot slap at heel strike and improve foot clearance during swing.

Using proportional myoelectric control in post-stroke patients presents both problems and opportunities. For patients with appropriate timing but weak amplitudes, a dorsiflexion assist orthosis with proportional myoelectric control could provide increased ankle motion under direct control by the nervous system. For patients with disordered timing of tibialis anterior, they are likely to have inappropriate initial patterns in orthosis assistance. However, the patients may also demonstrate adaptation in their recruitment patterns as healthy subjects did in this study. The added mechanical torque provided by the orthosis may actually enhance motor learning as it would produce greater errors in movement dynamics for inappropriate recruitment patterns. Error is the driving stimulus for motor learning, so greater error from the power of the orthosis could theoretically enhance motor recovery [13–15]. Future studies need to examine how post-stroke and other neurologically impaired populations respond to practice walking with robotic orthosis under proportional myoelectric control.

The limitation of our current design is that it is not readily portable due to the type of actuator used (i.e. pneumatic artificial muscle). However, the portability could be improved by using a micro-air compressor [16] for pneumatic artificial muscle or to use other types of actuators such as Series Elastic Actuator (SEA) [17, 18] instead. One example of current built dorsiflexion assist devices is the active AFO by Blaya and Herr [1]. They used a series elastic actuator to adjust impedance of the orthosis using information from ankle angle sensor and force sensors beneath the foot.

Our findings indicate that subjects had different adaptation responses for ankle dorsiflexors during the two main phases of activation. Subjects walked with substantially increased ankle dorsiflexion without altering tibialis anterior EMG at the second burst during stance-to-swing transition. This similar response of second burst in both groups (i.e. without decreasing amplitude) indicated that assistance given only during power generation phase did not result in different motor adaptation during the concentric phase of tibialis anterior activation. In contrast, tibialis anterior EMG was reduced by ~28% at the first burst during swing-to-stance transition. During this phase, the tibialis anterior is activated eccentrically to perform negative mechanical work as the foot is lowered to the ground. As a result, the findings only partially supported our hypothesis that subjects would reduce tibialis anterior recruitment when walking with a powered dorsiflexion assist proportional to tibialis anterior EMG.

Different adaptation responses of the two tibialis anterior EMG bursts might be explained by analyzing the costs and benefits of adaptation. The added dorsiflexion assistance during stance-to-swing transition may not provide a very strong stimulus for neuromuscular adaptation. There is no penalty in the mechanics of leg swing to have exaggerated ankle dorsiflexion during swing. The foot simply clears a higher height without added cost to the wearer. From the aspect of gait energetics, leg swing consumes ~30% of net energy required for walking [19] but hip flexors and plantar flexors are the main muscles responsible [20–23]. Ankle dorsiflexion itself consumes a fairly small amount of energy because the foot segment has very low mass. Thus, the energy savings from turning down tibialis anterior activation during the second burst may not be a strong enough stimulus for the nervous system to quickly adapt. In contrast, activation of the tibialis anterior at heel strike helps to smoothly lower the forefoot to the ground. Exaggerated ankle dorsiflexion at heel strike could cause instability because it prevents the foot from contacting the ground. Thus, the user would likely substantially benefit from turning down the first burst of tibialis anterior EMG when dorsiflexion assistance is provided by the orthosis.

The cost-benefit analysis would also explain why subjects using plantar flexion assist rapidly reduced their soleus EMG [7]. During walking, positive work is required to restore the energy lost in redirecting the center of mass velocity from step to step [24] and majority of the positive work is provided by the ankle muscles [25–27]. With such high energy production at the ankle, subjects would likely greatly benefit from having some plantar flexion work replaced by a powered orthosis. In the Gordon and Ferris study (2007) [7], subjects indeed reduced their soleus EMG amplitudes by ~30% with powered plantar flexion assistance. Our recent work has shown that this substitution of biological muscle work with robotic muscle work can significantly reduce the metabolic cost of walking [28].

Conclusion

Healthy subjects wearing dorsiflexion assist orthoses under proportional myoelectric control walked with increased dorsiflexion but did show some reduction in muscle recruitment. The nervous system modulated individual bursts of tibialis anterior EMG (heel strike and toe-off) differently in regard to motor adaptation. Future studies could use active dorsiflexion assist controlled by EMG as a potential rehabilitation intervention for individuals with dorsiflexor weakness.