Episodic Selection and the Maintenance of Competence and Natural Transformation in Bacillus subtilis

Abstract

We present a new hypothesis for the selective pressures responsible for maintaining natural competence and transformation. Our hypothesis is based in part on the observation that in Bacillus subtilis, where transformation is widespread, competence is associated with periods of nongrowth in otherwise growing populations. As postulated for the phenomenon of persistence, the short-term fitness cost associated with the production of transiently nongrowing bacteria can be compensated for and the capacity to produce these competent cells can be favored due to episodes where the population encounters conditions that kill dividing bacteria. With the aid of a mathematical model, we demonstrate that under realistic conditions this “episodic selection” for transiently nongrowing (persisting) bacteria can maintain competence for the uptake and expression of exogenous DNA transformation. We also show that these conditions for maintaining competence are dramatically augmented even by rare episodes where selection favors transformants. Using experimental populations of B. subtilis and antibiotic-mediated episodic selection, we test and provide support for the validity of the assumptions behind this model and the predictions generated from our analysis of its properties. We discuss the potential generality of episodic selection for the maintenance of competence in other naturally transforming species of bacteria and critically evaluate other hypotheses for the maintenance (and evolution) of competence and their relationship to this hypothesis.

BACTERIA may not have sex often, but when they do, it can be really good, at least evolutionarily. Sex, or more precisely recombination, broadly defined to include the acquisition and incorporation of DNA by horizontal gene transfer (HGT) from other organisms, enables bacteria to sample and obtain genes from the entire prokaryotic, archeal, and even eukaryotic DNA “sequence space.” As a result, the rate of adaptive evolution in bacteria need not be limited by the slow pace of sequential selection for small genetic changes generated by mutation. Through horizontal transfer, bacteria can acquire genes that have already passed through the gauntlet of natural selection in the same or even distantly related species living in different habitats.

For many bacterial species it is clear that genes from without play a prominent role in adaptive evolution as a source of genetic variation and particularly so for habitat-and-niche expansion (Shea et al. 1996; Bergstrom et al. 2000; Levin and Bergstrom 2000; Ochman et al. 2000; Koonin et al. 2001; Cazalet et al. 2004; Thomas and Nielsen 2005; Coleman et al. 2006; Gal-Mor and Finlay 2006). Not so clear is how the capacity for HGT evolved and is maintained. Despite its considerable value for the adaptation and long-term survival of bacterial populations, the ability to acquire genes from without, to “explore the fitness landscape” (Dubnau 1999), need not have been the selective force responsible for the evolution and maintenance of the machinery required for that capacity. In fact, for two of the three mechanisms of HGT, conjugation and transduction, it has been postulated that recombination is a coincidental by-product of plasmids' and phages' need for continuous transmission to new hosts to be maintained and the host's recombination system (Levin 1988; Redfield 2001).

On first consideration it would seem that coincidental evolution is unlikely to be responsible for recombination mediated by natural transformation, a complex process that generally requires the concerted action of many chromosomal genes (Berka et al. 2002; Barbe et al. 2004; Dagkessamanskaia et al. 2004; Chen et al. 2005; Thomas and Nielsen 2005). Nevertheless, coincidental evolution is implicit in two of the three existing hypotheses for the evolution and maintenance of transformation. In accord with those hypotheses, competence evolved and is maintained to acquire templates for the repair of double-stranded breaks in DNA (Bernstein et al. 1987; Hoelzer and Michod 1991) or as source of food or nucleotides (Stewart and Carlson 1986; Redfield 1993b; MacFadyen et al. 2001; Redfield et al. 2005). In the third hypothesis, genetic recombination is the selective force responsible for the evolution and maintenance of transformation. This transformation-for-recombination hypothesis (Bacher et al. 2006; Baltrus et al. 2008) is a prokaryotic variant of the classical explanation for the evolution of sex: a mechanism to accelerate evolution by shuffling beneficial mutations and genes among individuals in a population and preventing the accumulation of deleterious mutations (Fisher 1930; Muller 1932) (for a superb review of this classical literature see Felsenstein 1974).

In this report we present a new eclectic, individual-level selection hypothesis for the maintenance of competence and transformation, episodic selection. Central to our hypothesis is a theoretical prediction: When bacterial populations periodically encounter agents that kill replicating cells at a higher rate than nongrowing cells, persister subpopulations (Bigger 1944; Balaban et al. 2004; Wiuff et al. 2005) could have a selective advantage over faster-growing populations without this ability (Kussell et al. 2005; also see Kussell and Leibler 2005). Some 45 years ago E. W. Nester and B. A. D. Stocker (Nester and Stocker 1963) demonstrated that competent cells of Bacillus subtilis are refractory to penicillin-mediated killing and postulated that this is because they are not growing. More recently, Haijema et al. (2001) presented direct evidence in B. subtilis that competence for DNA uptake is expressed in a subpopulation that does not grow for a number of hours after its stationary phase culture is supplied with fresh medium.

With the aid of a mathematical model and computer simulations of the population dynamics of competence formation, transformation, and antibiotic-mediated selection, we demonstrate a priori that within populations, episodic traumas affecting growing cells in a population will favor bacteria that can generate subpopulations of competent nongrowing cells capable of natural transformation. Using experimental cultures of B. subtilis 168 and competence mutants, we test the validity of the assumptions behind the construction of this model and the hypotheses generated from our analysis of its properties. The results of our experiments are consistent with the episodic selection model for the maintenance of competence and natural transformation. We elected to not address the broader issue of the selective pressures responsible for the evolution of all of the genes necessary for natural competence. In our discussion we consider the potential generality of episodic selection in other species of naturally transforming bacteria and then critically review other hypotheses for the maintenance of transformation and their relationship to this episodic selection hypothesis.

THEORETICAL METHODS: A SERIAL PASSAGE MODEL FOR THE POPULATION AND EVOLUTIONARY DYNAMICS OF COMPETENCE AND TRANSFORMATION IN B. SUBTLILIS

To provide a framework for the design and interpretation of our experiments and to illustrate a priori that with realistic parameter values, episodic selection could favor the maintenance and possibly the evolution of competence and transformation, we use a simple mathematical model and numerical solutions. To appreciate this model it is essential to recall that competence in B. subtilis 168 is expressed bistably in ∼15% of the cells in a genetically competent population. In this model, there are four bacterial populations with densities (bacteria per milliliter) designated as S for genetically competent (com⁺) cells that are not phenotypically competent; C, for competent cells produced by S; N, for com⁻ mutants that cannot produce competent cells; and T, for transformants (competent cells that have taken up DNA with a specific marker that is under positive selection). For convenience we use the variables S, C, N, and T as the designations of these bacterial populations as well as their densities.

The populations grow at a rate proportional to the concentration of a resource, R μg/ml, via a Monod function (Monod 1949) V_x(R/(R + k_m)), where V_x hr⁻¹ is the maximum growth rate of that cell line and k_m is the concentration of the limiting resource where rate of growth is half its maximum value. To account for the fact that competent cells are not produced at a substantial rate until the population approaches stationary phase, we let the rate of competent cell production, S → C, be a decreasing function of the resource concentration, θ_C(R) = f(1 − R/(k_r + R)), where f hr⁻¹ is the maximum rate of competence formation and k_r is the resource concentration where competence formation is half its maximum value. In accord with this assumption, competent cells are produced continually throughout stationary phase (between serial transfers). We assume the rate at which competent cells lose competence, C → S is directly proportional to the resource concentration, θ_S(R) = gR/(k_r + R), where g hr⁻¹ is the maximum rate at which competent cells produce noncompetent cells. In the presence of antibiotics the rate of growth of each of the populations is determined by a Hill function so that when the concentration of the antibiotic A μg/ml and the concentration of the resource R μg/ml, the rate of growth of the x th population is

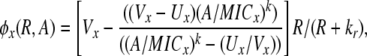where U_x is the minimum growth rate (maximum kill rate) of the x strain, the concentration of the antibiotic, and k is the Hill coefficient, which determines the shape of the function (Regoes et al. 2004). With these definitions, the changes in the density of the component populations, resources, and antibiotics during the course of a transfer are given by

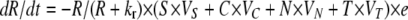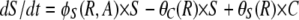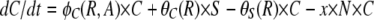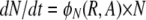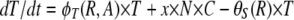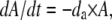where e μg/ml, the conversion efficiency (Stewart and Levin 1973), is the concentration of resource needed to produce a new cell, d_a is the decay rate of the antibiotic, and x is a rate constant of recombination. This parameter is a variant of the rate constant of recombination considered in Levin (1981) in which competent cells, C, are recipients and N are the donors. We assume that the transformants are initially a competent population but like C are converted into an S state that would be a different clone from S because it has a potentially selected gene acquired from N. For simplicity, we do not consider the ST population or the continuation of this episodic selection process.

In our computer simulations, we assume that the introduction of antibiotics and transformation are stochastic processes. A transfer ends at 24 hr at which time each population is diluted by a factor dil (0 < dil < 1), and R_a μg/ml of the resource is added. At the start of each transfer there is a probability p that an antibiotic will be added; for this, at each transfer we generate a random number y (0 < y < 1). If y < p, A μg/ml of the antibiotic is added. At each hour, there is a probability et that transformants will acquire a fitness advantage. To simulate this, a random number, z, from a rectangular distribution (0 < z < 1) is generated. If z < et × Δt, the other populations' growth rates are reduced by a factor (1 − s), where Δt is the step size and s the selection coefficient (0 < s < 1).

THEORETICAL RESULTS

Computer simulations:

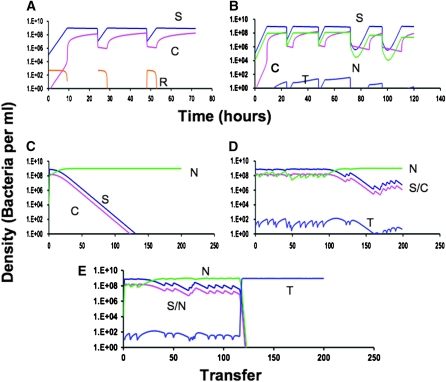
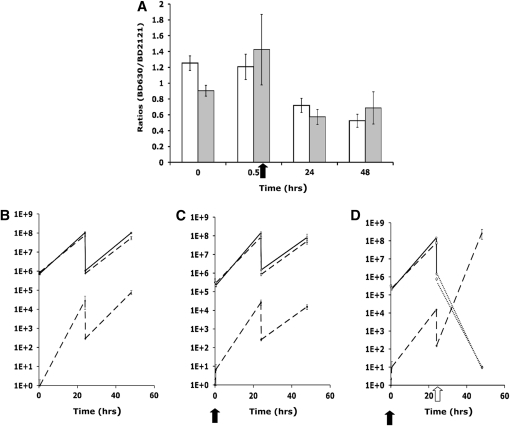
In Figure 1A we illustrate the dynamics of population growth, competence formation, and the competence loss process for three 1:100 successive transfers each at 24 hr in a population that initially bears no competent cells (C = 0). The exponential growth rate and antibiotic minimum inhibitory concentration (MIC) and other Hill function parameters are in a range anticipated for Escherichia coli (Regoes et al. 2004) and B. subtilis (see the experiments below) and bactericidal antibiotics. With the parameters in Figure 1, f = 0.01 and g = 0.10, by the end of a transfer, the C population is nearly 17% of the total population, which is in the range measured for B. subtilis 168 in competence medium (Haijema et al. 2001). At the start of a new transfer when resources are abundant, the S population increases while the frequency of C declines for a while and then increases as the resources become depleted. Within short order, the relative frequency of C at any given time after the start of a transfer is the same in successive transfers (Figure 1A). In Figure 1B we illustrate the dynamics of competition between com⁺ and com⁻, S, and N with equal maximum growth rates, but where there are episodes of antibiotic treatment. Because the com⁺ S population produces cells that grow at a very low rate V_C = 0.001, the S population has a disadvantage relative to N and during the first transfers the relative frequency of N increases. In this simulation, there were two successive episodes of antibiotic introductions and as a result the N population, which does not produce nongrowing antibiotic-refractory cells, has a temporary disadvantage. In the absence of episodes where nongrowing, competent cells are not killed, the N population continues to increase and the C population wanes (Figure 1C). With these episodes, the rate at which the C population declines can be markedly reduced (Figure 1D).

Figure 1.—

Simulation results depicting the population dynamics of competence formation and transformation in serial transfer culture. Changes in the densities of the component populations are as follows: dark blue, S (com⁺); pink, C (competent cells produced by com⁺); green, N (com⁻); blue, T (transformants); orange, resource concentration, R. Parameter values are R_a = 500, d = 0.01, d_a = 0.50, V_S = V_N = 1.0, V_T = 1.0, V_C = 0.001, U_S = U_T = U_N = −2.0, U_C = −0.01, MIC = 1 for all, f = 0.01, g = 0.10, k_m = k_r = 0.25, and x = 10⁻¹⁶. (A) The dynamics of competence formation: changes in the densities of S, C, and the concentration of the resource R. (B) Competence formation and the fitness of the competent population in a mixed culture with a population, N, that does not produce competent cells. The changes in densities of S, C, and N are displayed before and after two sequential episodes of antibiotic pulses of 10 μg/ml at the starts of the 72- and 96-hr transfers. (C) Long-term dynamics of the S, C, N, and T populations in the absence of antibiotic pulses. (D) Long-term dynamics of the S, C, N, and T populations with antibiotic pulses, p = 0.1 (on average once every 10 transfers with 10 μg/ml added). (E) Long-term dynamics of the S, C, N, and T populations with antibiotic pulses, p = 0.1 with 10 μg/ml added, and episodic selection for transformants, et = 0.0005 (on average once every 2000 hr) with an 80% fitness advantage for transformants. The densities plotted are those at the end of each transfer [that immediately before the fraction, d (0.01) of the population is transferred to fresh medium].

In this simulation, the rate parameter of transformation, x = 10⁻¹⁶, is about four orders of magnitude less than what we estimate for B. subtilis 168 (see the appendix). This conservative estimate shows that as long as the competent population, S + C, is present at a substantial density and there is a source of DNA from another population, transformants will be generated. When the population encounters situations where these transformants are favored, the competent population in the guise of transformants will prevail even in situations where the persistence effect does not give the competent, S, population an advantage (Figure 1E). In this simulation, we end with T. In reality, T is another competent population derived from S and the process would continue, where T produces its own competent subpopulation and so on.

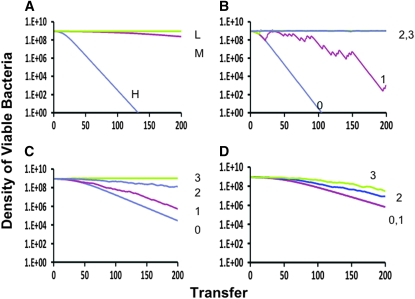
In the Figure 1 simulations, we assume that at the start of a transfer, a substantial fraction of the population is competent for transformation, on the order of 17%. While this is in the range observed for the laboratory strain B. subtilis 168, there is evidence that the frequency of competent cells in natural populations of B. subtilis is substantially lower (Cohan et al. 1991) (H. Maamar and D. Dubnau, unpublished results). Moreover, it is also possible that the fitness cost of maintaining all the machinery for transformation is greater than that due to the production of transiently nongrowing, competent cells. To explore the effects of these realities on the persistence effect of competence formation, we ran these simulations with different levels of competence and different regimes of antibiotic-mediated selection for persistence. The results of these simulations are presented in Figure 2.

Figure 2.—

Change in the density of competent cells (S + C) with different levels of competence and antibiotic exposure episodes and different levels of competence formation. H, g = 0.01, f = 0.10 (∼0.17 competent cells); M, g = 0.001, f = 0.10 (∼0.02 competent cells); L, g = 0.0001, f = 0.01 (∼0.002 competent cells). To simplify, we have not included the N population. When the S + C populations are declining the N population is increasing and the inverse. (A) No fitness cost other than that associated with production of competent cells. (B–D) The competent population S has an additional 1% (0.01) fitness disadvantage relative to the noncompetent, N, population. (B) Antibiotic treatment regimes: 0, no antibiotics; 1, Ad = 10 μg/ml, p = 0.10 (on average every 10th transfer); 2, Ad = 20 μg/ml, p = 0.10 (on average every 10th transfer); 3, Ad = 10 μg/ml, p = 0.20 (on average every 5 transfers). (B) High-level competence, H; (C) medium level of competence, M; (D) low-level competence, L. Other parameters are the same as in Figure 1. The densities plotted are those at the end of each transfer [that immediately before the fraction, d (0.01) of the population is transferred to fresh medium].

In Figure 2A we consider the effects of different levels of competence on the change in the density of a competent population in competition with the noncompetent N population (the density of which is not shown, but can be surmised as the total density was constant). In these simulations, the only fitness cost associated with the competent population is that due to the production of nongrowing competent cells. Under these conditions, the rate of decline in the density of the competent population, the fitness cost of competence, increases with the fraction of the population that is competent. With a maximum level of competence of 0.002, there is almost no selection against the competent population. As can be seen in Figure 2, B–D, where we allow for an additional 1% cost to the competent population, due to episodes of exposure to antibiotics that kill growing cells at a higher rate than nongrowing cells, the fitness costs associated with competence can be mitigated. Indeed, with a sufficiently frequent or a high enough dose of antibiotics, the competent C + S population can prevail and eliminate the noncompetent one (Figure 2, B and C). For any specific regime of antibiotic exposure, the extent of this mitigation of the cost of competence is proportional to the fraction of the population that is competent and thereby transiently refractory to the antibiotic-mediated killing. Thus, although the population with the highest frequency of competent cells has the greatest fitness burden in the absence of antibiotics, by producing this large fraction of nongrowing cells less antibiotic exposure is required to overcome the fitness cost than with strains having lower frequencies of competent cells.

Assumptions and hypotheses:

The assumptions behind the construction of this model and the predictions generated from our analysis of its properties are as follows:

• Assumption 1: Cultures where competent cells are present at substantial frequencies will be more refractory to antibiotics than those where competent cells are rare.

• Assumption 2: In pairwise competition between otherwise isogenic com⁺ and com⁻ bacteria, the com⁻ cells will have a selective advantage over the com⁺ in antibiotic-free media where competent cells are produced but not in media where they are not produced.

• Prediction 1: In pairwise competition between otherwise isogenic com⁺ and com⁻ bacteria in media where competent cells are produced, pulses of antibiotics will increase the fitness of the com⁺ relative to the com⁻.

• Prediction 2: In pairwise competition between otherwise isogenic com⁺ and com⁻ bacteria in media where competent cells are produced and donor bacteria carrying the appropriate genes are present, com⁺ transformants will rapidly ascend when the culture is confronted with the selecting antibiotics.

These assumptions and predictions have been validated and tested experimentally with B. subtilis 168.

EXPERIMENTAL MATERIALS AND METHODS

Bacterial strains:

We used B. subtilis BD630 (his leu met) and BD2121 (his leu met comK∷kan) as respective isogenic com⁺ and com⁻ strains (Berka et al. 2002). A third strain of B. subtilis, which we designate BD630-1 (his leu met nal amyE∷cat), was used as the com⁺ variant in the competition and transformation experiments. We constructed this derivative of BD630 in the following way: The spectinomycin (spc) chloramphenicol (cat) resistance-encoding plasmid, pDG1662 was isolated from E. coli TG1 (Bacillus Genetic Stock Center), using a Qiaprep spin miniprep kit (QIAGEN, Valencia, CA). The cat gene on this plasmid is flanked by sequences from the B. subtilis amyE locus. Cam^R Spc^S pDG1662 transformants of BD630 were obtained by selective plating on Luria–Bertani (LB) agar (Difco, Detroit) containing chloramphenicol (5 mg/liter) and then patching potential Spc^S transformants on agar with spectinomycin (100 mg/liter). These Cam^R Spc^S transformants were the products of double-crossover events, as opposed to Campbell-like integration in the amyE locus. A spontaneous nalidixic acid-resistant mutant of this transformant was isolated by plating concentrated overnight cultures onto LB agar containing nalidixic acid (Nal) (30 mg/liter). Serial transfer experiments were performed to confirm the stability of BD630-1 (Nal^R Cam^R) and BD2121 (Kan^R) phenotypes in the absence of the selecting antibiotic.

Media:

Cell densities were estimated by serial dilution and plating on LB agar with or without kanamycin (25 mg/liter), chloramphenicol (5 mg/liter), and nalidixic acid (10 mg/liter). Competence media GM1 and GM2 were prepared as described in Yasbin et al. (1975) with the omission of CaCl₂ in GM2. The induction of competence was done using the method described in Boylan et al. (1972). Briefly, B. subtilis cells were grown in GM1 for 4 hr at 37°, 220 rpm, or until OD data suggested the end of exponential growth. The culture was then diluted 1/10 in 37° GM2 and incubated with vigorous shaking for another 90 min at which time transforming DNA (>1 μg) was added to the culture. Incubation was continued with gentle aeration for another 30 min at 37°, and the culture was plated onto LB agar with kanamycin added to select for transformants. The production of transformants was used as the criterion for competence. The DNA for these experiments was isolated from B. subtilis, using a blood and cell culture DNA midi kit (QIAGEN) according to manufacturer's protocol.

Penicillin-G time-kill experiments:

To ascertain whether the com⁺ cells were more refractory to antibiotics than the com⁻ cells, we performed time-kill experiments using a protocol similar to that in Nester and Stocker (1963) to compare the killing kinetics of com⁺ (BD630) and com⁻ (BD2121) strains. We also performed transformation time-kill assays by adding DNA from BD2121 and selecting for Kan^R in the penicillin-G-exposed BD630. In these experiments we grew the com⁺ and com⁻ clones in competence medium to an OD where we anticipated high frequencies of competent cells in the com⁺ culture and diluted these cultures 1/5 in prewarmed GM2 medium. In the cultures where the time-kill kinetics of transformant survival were measured, 20 μg/ml DNase1 (Sigma, St. Louis) were added and incubation was continued for 2 min before 100 μg/ml penicillin G were added. These time-kill experiments were performed at 32°. Samples were taken at different times and plated on LB agar. To control for postplating penicillin-G killing, 400 μg/ml penicillinase (β-lactamase from Enterobacter cloacae, Sigma) were added to samples diluted to an extent <10⁻³.

Serial transfer experiments:

Single strains of BD630 and BD2121 were grown in GM1 until exponential growth ceased (as determined from OD₆₅₀ data), at which time the culture was diluted 1/10 into fresh, prewarmed, 37° GM2 broth. The transferred cultures were incubated until net growth was noted from an increase in OD. At this time, 250 μl of each strain were mixed into 10 ml warm (32°) GM2 medium. The densities of the com⁺ and com⁻ competitors were estimated by plating on LB agar with and without kanamycin (25 μg/ml). These serial transfer experiments were performed with and without pulses of penicillin G and with and without selection for transformants. The methods used for these variants of the serial transfer cultures are described in the experimental results section.

Competition assays:

Pairwise competition experiments were performed to estimate the relative fitness of BD630 and BD2121 in LB and GM2 media. The com⁺ and com⁻ clones were grown overnight in GM1 at 32° and the following morning diluted 1/10 and grown in single-clone GM1 or LB culture for ∼4 hr (until exponential growth ceased) and then diluted 1/10 in fresh GM2 or LB broth. At time 0, a 1:1 ratio of each competitor was added to fresh GM2 or LB broth. The initial and final ratios of the two competitors were estimated and the relative fitness was calculated using the Malthusian parameter estimate of fitness described in Lenski et al. (1991). To ascertain the effect of penicillin G in these competitions 100 mg/liter of this antibiotic were added to these cultures and 2 hr later penicillinase was used to abort the penicillin killing.

EXPERIMENTAL RESULTS

Time-kill experiments—test of the validity of assumption 1:

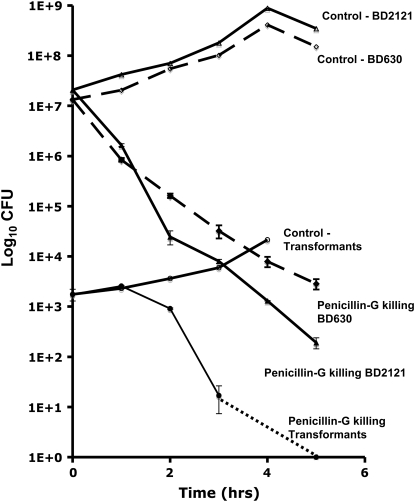
In accord with our model, competent wild-type (BD630) bacteria would be more refractory to penicillin G than the otherwise isogenic comK mutant (BD2121) because competent cells do not grow upon dilution into fresh medium. The results of our time-kill experiments with BD630, BD630-transformants, and BD2121 are consistent with this hypothesis. During the first 2 hr of exposure to penicillin G the extent of killing of BD630 cultures bearing substantial frequencies of competent cells is less than that of the com⁻ BD2121 (Figure 3).

Figure 3.—

Penicillin-G killing of BD630 (com⁺), BD630 transformants, and BD2121 (com⁻). All time-kill experiments were performed in triplicate. Error bars show 95% confidence intervals. The control population was not subjected to penicillin treatment.

Also consistent with this hypothesis is the observation in Figure 3 that during the first 2 hr of exposure to penicillin, transformants (competent cells receiving DNA conferring kanamycin resistance) are relatively refractory to the antibiotic. As time proceeds, the rate of killing of the com⁺ cells and of the transformants should approach that of the com⁻ cells because of the conversion of phenotypically Com⁺ cells into phenotypically Com⁻ cells during exponential growth. This is also evident in Figure 3. As the transformants start growing penicillin G kills them, slowly at first and then faster as the fraction of dividing transformant cells increases.

Competition experiments—test of the validity of assumption 2 and prediction 1:

Our model predicts that in the absence of episodic selection, com⁺ strains would be at a disadvantage in competition with com⁻ strains in fresh medium due to the production of an initially nongrowing subpopulation of competent cells. The results of our pairwise competition experiments with BD630 (com⁺) and BD2121 (com⁻) are consistent with this hypothesis. As can be seen in Figure 4, in competence medium without penicillin pulsing, the com⁻ strain has a selective advantage relative to the com⁺ strain. This advantage is not obtained when the com⁺ and com⁻ strains compete in LB medium where competence is not induced.

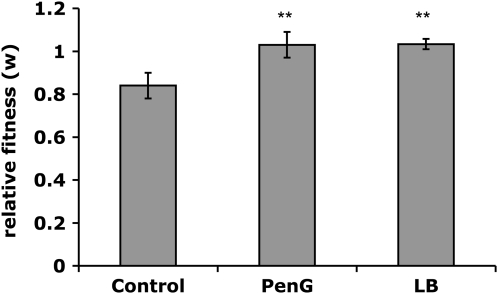
Figure 4.—

Estimated fitness, w, of com⁺ (BD630) in pairwise competition with com⁻ (BD2121). Control: competence medium w = 0.84 ± 0.06 (mean and 95% confidence interval for nine independent replicas). PenG: 2-hr pulse of penicillin G, w = 1.04 ± 0.06. LB: w = 1.03 ± 0.02. **P < 0.001, PenG vs. control and LB vs. control.

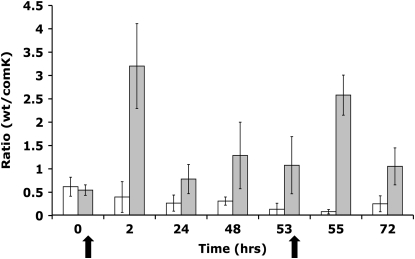
Our model predicts that because competent cells are relatively refractory to antibiotics that kill growing cells, exposing the cultures to penicillin shortly after transfer to fresh medium would mitigate the advantage of the com⁻ strain in competition with the com⁺ strain in competence-inducing medium. This is indeed what we observed when a 2-hr pulse of penicillin G was added upon transfer to fresh medium and then quenched with the addition of penicillinase (Figure 5).

Figure 5.—

Ratios of BD630/BD2121 during the course of a serial transfer experiment. Thick arrows indicate the addition of 100 mg/liter penicillin G followed by the addition of penicillinase 2 hr later. Open bars are untreated controls. Shaded bars are the cultures with penicillin pulsing. Means of three parallel experiments ±95% confidence intervals are shown.

The fitness of com⁺ cells is augmented in populations confronted with an agent that kills growing cells (Figure 5). Following each pulse of penicillin, the frequency of com⁺ cells increased precipitously relative to the controls, which did not receive penicillin. This experiment also supports the hypothesis that in the absence of selection for nongrowing cells, the com⁻ strain has a selective advantage over the com⁺ strain. In the intervals between penicillin pulses the com⁺ frequency declines dramatically.

Competition and selection for transformants—test of the validity of prediction 2:

Our model predicts that a com⁺ population would have an additional advantage over the com⁻ in an environment where transformants have a selective advantage and the right DNA is available. To test this hypothesis, we performed serial transfer experiments similar to those above but with 0.5-hr rather than 2-hr pulses of penicillin G and a pulse of antibiotics that would select for com⁺ transformants. Serial transfer experiments in GM2 were performed with mixtures of the com⁺ BD630-1 (Nal^R, Cam^R) and com⁻ BD2121 (Kan^R). To provide an environment that favors com⁺ Cam^R Kan^R transformants, chloramphenicol (5 mg/liter) and kanamycin (25 mg/liter) was added to one set of these serial transfer cultures. The densities of these transformants were estimated on LB agar containing nalidixic acid, kanamycin, and chloramphenicol. It should be noted that no free DNA was added in these experiments and thus transformants arose from uptake of DNA from the com⁻ Kan^R bacteria in the mixed culture experiments. The Nal marker was used to distinguish com⁺ transformants from potential com⁻ Cam^R mutants. Single-clone, high-density cultures were used to control for the appearance of Kan^R com⁺ and Cam^R com⁻ cells by mutation. These were not detected (data not shown).

The results of these experiments (Figure 6) are consistent with the hypothesis that selection would favor com⁺ transformants under conditions where penicillin pulsing is not sufficient to provide an advantage to the com⁺ cells. With and without penicillin pulsing the com⁺ cells do not increase in frequency relative to the com⁻ cells (Figure 6, A–C). On the other hand, when kanamycin and chloramphenicol were added, the density of transformants increased precipitously. While we cannot exclude the possibility that a minority of populations of the parental strains remained present, by 24 hr com⁺ transformants were the dominant, if not the sole, bacterial population.

Figure 6.—

Competitions between BD630 and BD2121 under conditions where penicillin-G pulses are insufficient to provide an advantage to com⁺ over com⁻ cells. (A) Ratios of BD630-1/BD2121 in pairwise competitions with (shaded bars) and without (open bars) a single 0.5-hr pulse of penicillin G. (B–D) Changes in the density of com⁺ (top dashed line) and com⁻ (top solid line) parental strains and com⁺ transformants (ascending dashed line). (B) No penicillin pulse; (C) 0.5-hr penicillin pulse; (D) 0.5-hr penicillin pulse followed by the addition of both kanamycin (25 mg/liter) and chloramphenicol (5 mg/liter). Means and 95% confidence intervals of the replicas transferred to fresh medium at 24 hr are shown. The thick solid arrows indicate penicillin pulse; the open arrow indicates the introduction of kanamycin and chloramphenicol. Note that we were unable to detect parental com⁺ or com⁻ cells at 48 and 24 hr after antibiotics were added to select for transformants.

DISCUSSION

We present theoretical and experimental support for a new hypothesis for the selective pressures responsible for the maintenance of natural transformation in B. subtilis. In accord with our hypothesis, two forces act synergistically to maintain competence for the uptake and integration of exogenous DNA in populations of naturally competent bacteria: (1) exposure to conditions where replicating members of the population are killed at a greater rate than a growth-arrested subpopulation and (2) confrontation with environmental conditions that favor bacteria that have acquired DNA bearing specific gene(s). As a consequence of episodes of the first type, the fitness cost of producing competent cells is transiently abated in competition with noncompetent populations. This could either reduce the rate at which competence is lost (buying time for episodes of the second type) or, if type-1 episodes are sufficiently frequent, provide the competent bacteria with a fitness advantage. The fitness advantage of the competent population is further and dramatically enhanced when rare episodes of the second type occur and transformants with newly acquired beneficial genes ascend to dominance.

Central to the first element of this episodic selection hypothesis is that B. subtilis competent for transformation are transiently growth arrested (Nester and Stocker 1963; Haijema et al. 2001) and that encounters with agents that kill growing bacteria would favor populations that produced these nongrowing subpopulations (Kussell et al. 2005). Our time-kill experiments, conducted under conditions where competence is induced, show that the com⁺ wild-type strain is killed at a lower rate and to a lesser extent than the otherwise isogenic com⁻ mutant. Moreover during this period, transformants are relatively more refractory to penicillin than nontransformants. Also, as assumed in our model and anticipated from the observations of Haijema et al. (2001), this reprieve from penicillin-mediated killing of the competent subpopulation is short term, reflecting the transience of competence expression.

As assumed in our model, competent populations of B. subtilis would have a selective disadvantage over otherwise identical populations that are not competent. This is due to the production of transiently nongrowing competent cells in the wild-type population. The results of our pairwise competition experiments with mixtures of otherwise isogenic com⁺ and com⁻ B. subtilis 168 are consistent with this assumption. Whether this disadvantage is solely because of the production of transiently nongrowing, competence-induced cells is not clear, but this issue is irrelevant for the present purposes; for whatever reason there is a cost to competence, and that burden needs to be mitigated.

Because of this disadvantage, the frequency of com⁺ cells would continually decline in mixed cultures with com⁻. But, as postulated by our model, the fitness cost of the com⁺ population (which produces persisters in the form of competence-induced cells) could be reduced or even overcome by episodes where the replicating cells are killed at a higher rate than those that are not replicating. Our pairwise competition experiments with mixtures of com⁺ and com⁻ B. subtilis with pulses of penicillin support this prediction.

Finally, our experiments support the prediction our model made for the second element of episodic selection hypothesis, transformation. When there is exogenous DNA bearing a gene that can augment the fitness of competent cells and selection favors competent cells acquiring that gene, transformants bearing that gene will ascend. In our experiments as well as in our model, the source of exogenous DNA was a competing population of bacteria that was not competent for transformation.

In our experiments, the fitness advantage of the transformants was intense, but presumably when a bacterial population encounters a novel habitat, antagonistic agents, or organisms, the intensity of selection for genotypes capable of replication would be profound. Early experiments with B. subtilis 168 in seminatural habitats (peat pots) (Graham and Istock 1979) suggest that more modest selection forces may also provide an advantage for transformants. While the nature of the selection favoring transformants was not identified in these experiments, particular groups of recombinants (for known markers) had an advantage over other groups as well as two parental genotypes that were mixed to initiate the experiment. It has also been postulated that when bacteria competent for natural transformation invade new niches and acquire DNA from other species (interspecific transformation), recombination may speed the process of speciation in bacteria (Cohan 2001, 2002). In this interpretation, recombination through transformation has the opposite effect of that postulated for a sexually reproducing organism. It promotes rather than prevents incipient speciation as recombination is believed to do for animals and plants.

Generality:

It should be noted that this episodic selection mechanism, like the selective pressures responsible for the maintenance of mutator genes, accessory genetic elements, or second-site compensatory mutations, is a nonequilibrium phenomenon (Bergstrom et al. 2000; Levin and Bergstrom 2000; Tanaka et al. 2003). For episodic selection to operate, the bacteria must be continually challenged by stresses that provide an advantage to nongrowing cells and an ever-changing environment and/or continuous opportunities to invade novel habitats or confront new physical or biological conditions.

On first consideration, it may seem that B. subtilis is going through a lot of trouble to generate persistent subpopulations by transient growth arrest of competence-induced cells just to maintain competence by episodic selection. Nontransforming bacteria, like E. coli and Staphylococcus aureus, produce persistent subpopulations with presumably far fewer genes than required for competence. We conjecture, however, that growth arrest in competent B. subtilis is secondary to its primary function, the uptake of exogenous DNA. In this coincidental by-product interpretation, growth arrest is required for the uptake and processing of this DNA and has the secondary consequence of maintaining competence by episodic selection. The growth-arrest element of competence is likely to have evolved because it provides populations with an advantage when competence for natural transformation is induced (note that this is independent of acquirement of adaptive genes). During the course of transformation, competent cells can take up massive amounts of DNA and recombination can result in nicks, mismatches, and single-strand gaps. By arresting DNA replication, those potential errors would have time to be repaired (Mongold 1992; Haijema et al. 2001). (See Claverys et al. 2006 for a potential mechanism for this repair.)

How general is episodic selection as a mechanism for the maintenance of competence and transformation in B. subtilis? Are the results presented here an artifact of our use of the laboratory strain 168 for our experiments? Frequencies of competence formation as high as 10–20% obtained with laboratory strains are substantially higher than those estimated with natural isolates of B. subtilis (Cohan et al. 1991) (H. Maamar and D. Dubnau, unpublished results). Our model suggests, however, that the frequency of competence is not critical to the episodic selection hypothesis. If a smaller fraction of the population is competent, the fitness cost of producing these transiently nongrowing cells would be low and the rate at which the competent population declines between episodes favoring nongrowing cells and transformants would be reduced (see Figure 2). How general is episodic selection for other naturally competent and transforming bacteria? We postulate that other naturally transformable species that display competence-induced dormancy will have the same selective advantage, as reported here for B. subtilis, under conditions where stressors killing growing cells are present in their environments. However, to the best of our knowledge other than in B. subtilis, evidence for competence-induced transient growth arrest has been presented only for S. pneumoniae. When competence is induced globally by the introduction of competence-stimulating peptide (CSP) to growing populations of S. pneumoniae, a distinct but transient arrest of population growth is observed (Oggioni et al. 2004). This growth arrest is not observed in the absence of added CSP, as would be expected if the population is heterogeneous in the timing of the induction of competence. Even if a subpopulation of competent cells ceased growth, population growth at large would remain exponential. In fact, competent cultures of S. pneumoniae may well be heterogeneous (Guiral et al. 2005; Claverys et al. 2007). While there is no evidence for growth arrest in other competent bacteria, this has to our knowledge not been specifically investigated.

We conjecture that growth arrest of competent cells is common for naturally transforming bacteria and indeed may be a necessary concomitant of transformational recombination as suggested above. If this is the case, episodic selection of the sort considered here will play a role in the maintenance of competence and transformation and may well have contributed to the evolution of this mechanism for HGT for other naturally transforming bacteria. Whether our conjecture has general merit or not is experimentally testable. We predict that in untested naturally transformable species, periodic pulsing of antibiotics will increase the frequency of competent bacteria in mixed cultures with noncompetent mutants.

There are abundant ways that the acquisition of genes from other bacteria may provide a selective advantage to competent cells; less clear is how commonly nongrowing cells in an otherwise growing population, persistence, would be favored. The phenomenon, in the guise of persistence, has been observed for a number of very different antibiotics (Wiuff et al. 2005) and a number of toxic metals can also enrich for persisters (Harrison et al. 2005). It is well known that most phage do not replicate on stationary phase bacteria. There is recent evidence that persistent cells are protected from induction of Lambda prophage but not adsorption by lytic Lambda (Pearl et al. 2008). Not so clear is whether the adsorption rate of phage to persistent cells is lower than that of the growing members of the population. If this was the case, persistence could be favored by phage-mediated selection, a hypothesis to test for another time.

An array of compatible hypotheses:

There are currently three hypotheses for the evolution and maintenance of transformation, which we briefly described in the Introduction to this report. In two of these existing hypotheses, transformation (recombination) is a coincidental by-product of the uptake of DNA. In accord with these hypotheses, exogenous DNA is taken up for the repair of double-stranded breaks (Bernstein et al. 1987; Wojciechowski et al. 1989; Hoelzer and Michod 1991) or used as a source of food or nucleotides (Redfield 1993b, 2001; MacFadyen et al. 2001; Redfield et al. 2005). Both the DNA repair and the gastronomy hypotheses have what population geneticists see as the virtue of parsimony: Selection for DNA uptake operates at the level of individual bacteria; a competent bacterium would have an advantage in a population of otherwise isogenic cells that are not competent. These hypotheses also have what some, particularly molecular, biologists may see as the liability of profligacy: The uptake of DNA requires the coordinated action of large number of genes (Berka et al. 2002; Barbe et al. 2004; Dagkessamanskaia et al. 2004; Chen et al. 2005; Thomas and Nielsen 2005). But parsimony and profligacy arguments are not tests of hypotheses. At this juncture, however, direct tests of these hypotheses have been limited and the results obtained may be seen as equivocal, at least for the generality of these hypotheses.

Experiments with B. subtilis are consistent with the repair hypothesis. When provided with undamaged or damaged DNA, the population density of transformed cells increased relative to nontransformed cells with an increasing dosage of ultraviolet light (Wojciechowski et al. 1989; Hoelzer and Michod 1991). On the other hand, the results of experiments with Haemophilus influenzae are inconsistent with the DNA repair hypothesis. Although exposure to DNA increased the rate of survival of UV-treated H. influenzae, the increase was obtained when the DNA carried only 1 min of the H. influenzae chromosome (Mongold 1992). This is far too small a fraction to account for the repair of widespread double-stranded DNA breaks responsible for bacterial death (see also Redfield 1993a).

The observation that starvation induces competence in some naturally transforming species is interpreted as evidence in support of the hypothesis that competence evolved and is maintained for the acquisition of DNA as a source of food or nucleotides (Redfield 1993b). Also consistent with this gastronomy hypothesis is the abundance of DNA in the external environment of many naturally transforming bacteria (Ahrenholtz et al. 1994). Although we know of no experiments presenting direct evidence in naturally competent bacteria supporting this food hypothesis, there are observations that are inconsistent with it. Exogenous DNA does not provide a growth benefit to competent Acinetobacter baylyi strains and increasing the concentration of DNA reduces the growth rate of competent cells to an extent that appears to be greater than it does for noncompetent mutants (Bacher et al. 2006).

Gastronomy as the sole reason for the evolution and maintenance of transformation is also not a particularly parsimonious hypothesis. The uptake of DNA, including the incorporation and expression of exogenous DNA by naturally competent bacteria, is a complex and profligate process; cells go to an inordinate amount of trouble in handling that DNA, playing with their food as it were, and then discarding one strand of it (Jarosik and Hansen 1994; Dubnau 1999). Also, many of the genes expressed under competence control in B. subtilis, H. influenzae, and S. pneumoniae such as recombination proteins and those that protect DNA from degradation (RecA, DprA, SsbB) (Jarosik and Hansen 1994; Berge et al. 2003; Kramer et al. 2007) seem superfluous for digesting the DNA they take up. It is also notable that B. subtilis exhibits localization of these DNA-protective proteins to the cell poles, where they associate with uptake proteins at the sites of DNA transport (Hahn et al. 2005; Kidane and Graumann 2005; Kramer et al. 2007).

The only direct experimental evidence we know of in support of the food hypothesis comes from E. coli K12, which is apparently incapable of natural transformation. E. coli can utilize externally supplied macromolecular DNA as a source of nutrients, thereby deriving a fitness advantage in a competitive situation (Finkel and Kolter 2001; Palchevskiy and Finkel 2006). This capacity is suggestive, but in the absence of evidence that the DNA is first transported across the inner membrane in macromolecular form, it cannot be accepted as evidence for the plausibility of the “transformation for food” hypothesis. It is of course also possible that the use of DNA for food is an accidental by-product of DNA for recombination, rather than the other way around.

The third hypothesis for the evolution and maintenance of transformation is a prokaryotic variant of the classical explanation for the evolution of sex (Fisher 1930; Muller 1932; Felsenstein and Yokoyama 1976). In accord with this hypothesis selection operates at the level of the collective, the group, rather than individuals; populations capable of transformation evolve more rapidly than those without this capacity. While group- or population-level selection may not have the parsimonious appeal of individual selection, in theory at least there are conditions where they can occur (Levin and Kilmer 1975; Szollosi et al. 2006). In theory there are also conditions where at least for sexually reproducing eukaryotes recombination could be favored within a population, by individual selection (Felsenstein and Yokoyama 1976). Moreover and more importantly, in addition to some very nice theory (Evans 1986), there have been direct tests of the hypothesis that recombination augments the rate of adaptive evolution in experimental populations of bacteria. E. coli B bearing an F'lac plasmid adapt to culture conditions at a higher rate than bacteria incapable of conjugation-mediated recombination (Cooper 2007). This also appears to be the case for Helicobacter pylori. Competence-proficient wild-type populations adapt to culture conditions at a rate greater than that of nearly isogenic competence-deficient mutants (Baltrus et al. 2008). Presumably, but not as clearly as in the E. coli B–F'lac study, the advantage of competence in this H. pylori investigation can be attributed to the more rapid assembly of beneficial mutations in bacteria that are capable of recombination relative to those that are not. But alas, there is also evidence from studies with experimental populations of A. baylyi and E. coli inconsistent with this transformation-evolved-for-recombination hypothesis (Souza et al. 1997; Bacher et al. 2006). Of course, these negative results indicate only that the conditions for recombination to augment rates of evolution are not universal.

Not so clear in either of these studies with monocultures is how individual bacteria with the capacity for transformation would fare in populations dominated by otherwise isogenic bacteria not carrying F'lac plasmid or expressing the plethora of genes required for competence. Plasmids are anticipated to engender a fitness cost, and particularly so if they are permanently derepressed for conjugative pilus synthesis (Levin 1980; Dahlberg and Chao 2003). As demonstrated here as well as by Bacher et al. (2006), in naturally transforming bacteria competent cells have a disadvantage over otherwise isogenic bacteria that are not competent. Even if recombination accelerates the rates of adaptive evolution in single populations, in mixed populations with sexually more reticent, but higher fitness competitors, it may not provide the recombining population with a selective advantage.

Caveats and limitations can be pointed out for each of the existing hypotheses for the maintenance of competence and transformation and we expect that the astute reader can do the same for the episodic selection hypothesis we present here. But we cannot reject any of these hypotheses or the possibility that competence and transformation are maintained by more than one mechanism. Nature, unlike those of us who study it, has no need to favor a single hypothesis. Gastronomy and repair (including competence-associated delays for repair) are processes that can provide an advantage to populations that take up exogenous DNA in times of dearth or DNA damage and in this perspective could also be seen as forms of episodic selection for competence. Any of these mechanisms would act synergistically with the episodic selection process considered here to overcome the fitness cost associated with maintaining the machinery for competence and transformation. In this liberal interpretation, as a consequence of these individual-level selection mechanisms, naturally transforming populations can reap the long-term benefits of maintaining a mechanism of natural competence for transformation.

APPENDIX: RATE PARAMETER OF RECOMBINATION

To estimate the rate parameter of recombination, x, the B. subtilis 168 strains BD2121 (Kan^R) and BD630-1 (Cam^R Nan^R) were grown overnight in competence media. These overnight cultures were mixed with initial densities of 8.9 × 10⁵ and 7.12 × 10⁵ cells/ml of BD630-1 (Nal^R Cam^R) and BD2121 (Kan^R). To control for the initial density of transformants, three samples of 100 μl each were plated onto agar containing chloramphenicol, kanamycin, and nalidixic acid (Cam Kan Nal). None were observed in this initial mixture. In terms of our model, BD630-1 (Nal^R Cam^R) is the competent, recipient population, S + C; BD2121 (Kan^R) is the donor population, N; and the Nal^R Cam^R Kan^R cells are the transformants, CT. After 24 hr of growth, the densities of the parental strains were estimated on agar containing Cam or Kan and the density of transformants was estimated on Cam Kan Nal agar. These densities were, respectively, (BD2121) 1.06 × 10⁸ and (BD630-1) 7.6 × 10⁷ and 3.0 × 10⁴ for the transformants.

By adjusting the value of x in repeated simulations with our model (Equations 1–4) we estimated the value of this parameter that with initial densities of donors and recipients in the range of the experiment would yield approximately the observed densities of donors, recipients, and transformants in a single 24-hr cycle. In these simulations, we used the competence formation parameters and other parameters presented in Figure1A, but adjusted the initial concentration of the resource to provide ∼2 × 10⁸ cells. We also assumed that at the start of the experiment, 15% of the recipient populations were competent, C. On the basis of these simulations, we estimate x to be between 1 × 10⁻¹² and 2 × 10⁻¹² (ml cell⁻¹ hr⁻¹).