Heritability of human cranial dimensions: comparing the evolvability of different cranial regions

Abstract

Quantitative craniometrical traits have been successfully incorporated into population genetic methods to provide insight into human population structure. However, little is known about the degree of genetic and non-genetic influences on the phenotypic expression of functionally based traits. Many studies have assessed the heritability of craniofacial traits, but complex patterns of correlation among traits have been disregarded. This is a pitfall as the human skull is strongly integrated. Here we reconsider the evolutionary potential of craniometric traits by assessing their heritability values as well as their patterns of genetic and phenotypic correlation using a large pedigree-structured skull series from Hallstatt (Austria). The sample includes 355 complete adult skulls that have been analysed using 3D geometric morphometric techniques. Heritability estimates for 58 cranial linear distances were computed using maximum likelihood methods. These distances were assigned to the main functional and developmental regions of the skull. Results showed that the human skull has substantial amounts of genetic variation, and a t-test showed that there are no statistically significant differences among the heritabilities of facial, neurocranial and basal dimensions. However, skull evolvability is limited by complex patterns of genetic correlation. Phenotypic and genetic patterns of correlation are consistent but do not support traditional hypotheses of integration of the human shape, showing that the classification between brachy- and dolicephalic skulls is not grounded on the genetic level. Here we support previous findings in the mouse cranium and provide empirical evidence that covariation between the maximum widths of the main developmental regions of the skull is the dominant factor of integration in the human skull.

Introduction

The human skull is an important source of information for phylogenetic and population-genetic studies (Strait, 2001; González-José et al. 2003; Ackermann & Cheverud, 2004a,b). The complex morphology of the skull is usually decomposed in a series of craniometric measurements and it has been demonstrated that moderate amounts of genetic heritable variation underlie these traits (Sjøvold, 1984; Sparks & Jantz, 2002; Carson, 2006a). To some extent this suggests that skull morphology has substantial potential to evolve and that craniometric characters have the potential to provide consistent phylogenetic signals. Nevertheless, most studies have disregarded the integrated nature of the skull (Lieberman et al. 2000a,b; McCarthy & Lieberman, 2001; Bookstein et al. 2003; González-José et al. 2004; Bastir et al. 2004; Bastir & Rosas, 2004, 2005, 2006). Morphological integration can constrain the evolvability of traits (Merilä & Björklund, 2004) and bias the results of phylogenetic analysis (Strait et al. 2007; Lockwood, 2007; Sherwood et al. 2008).

Although the most appropriate approach to address this issue is to account for genetic and phenotypic covariation patterns of multivariate skull shape (Klingenberg & Leamy, 2001; Klingenberg, 2004, 2005), an alternative approach is to assess both the patterns of genetic variation and the correlation of univariate craniometric measurements. Here we explore the genetic architecture underlying the skull following this latter approach, which is relevant for evolutionary biology because craniometric traits are still in full use. For instance, recent studies have extensively applied population-genetics-based models using classical measurements (Roseman, 2004; Neves & Hubbe, 2005; Schillaci & Stojanowski, 2005; Harvati & Weaver, 2006). Our goal is to reconsider the evolutionary potential of craniometric traits, accounting both for their heritabilities and for the patterns of genetic and phenotypic correlation among them. Furthermore, we will test hypotheses of cranial integration formulated on the basis of these kind of traits (Enlow & Hans, 1996; Hallgrímsson et al. 2007).

Genetic variation in the human skull

The estimation of the genetic and non-genetic components underlying the phenotypic variation of the human skull has long been a major focus of anthropological research (Boas, 1912; Kohn, 1991; Varela & Cocilovo, 1999; Konigsberg, 2000). The first studies addressing this issue date back to the first decades of the 20th century (Dahlberg, 1926) but the interest increased at the end of the century because evolutionary biologists reconsidered the use of skeletal remains to unravel human microevolutionary paths (Relethford & Lees, 1982; Relethford, 1994). This new paradigm was built upon the growing evidence that suggested that human patterns of craniofacial variation reflected the underlying genetic patterns of variation (Cheverud, 1988; Buikstra et al. 1990). Craniometric traits were thus regarded as useful tools to study the structure and history of human populations (Relethford & Lees, 1982) and population-genetic models were adapted to be used after craniometric traits (Relethford & Blangero, 1990; Relethford, 2002, 2004). The heritability of complex metric traits, considered in the narrow sense, expresses the proportion of total phenotypic variance due to additive genetic variance (Falconer & MacKay, 1996). Heritability provides a measure of the proportion of variance in a trait explained by genetic transmission and is therefore a key parameter in models of evolution of quantitative traits (Konigsberg, 2000).

A wide range of studies have estimated the heritability of craniofacial traits (Vandenberg, 1962; Hiernaux, 1963; Nakata et al. 1974; Susanne, 1975, 1977; Sjøvold, 1984; Devor et al. 1986; Sharma, 1987; Sharma & Susanne, 1991; Konigsberg & Ousley, 1995; Nikolova, 1996; Sharma, 1998; Sparks & Jantz, 2002; Arya et al. 2002; Johannsdottir et al. 2005; Carson, 2006a). The general conclusion of these studies is that human craniofacial traits have moderate to high degrees of genetic variation. However, the comparison of results from different studies is controversial as they have been computed on very different kinds of samples (living humans or skeletal remains) from different geographical regions, accounting for different familiar relationships (twins, nuclear or extended families) and using different statistical methods (regression, anova, path analysis or maximum likelihood analysis (ML). ML methods are considered the most efficient methods to estimate genetic parameters in natural populations (Konigsberg, 2000). However, they have not been used until recently because they are computationally highly demanding (Roff, 1997).

Moreover, one of the main problems concerning the heritability estimation of cranial measurements in humans is that suitable, large and pedigree-structured skull series are almost non-existent. Such a collection of skulls with genealogical-associated data exists in Hallstatt (Austria), and has been previously studied to measure the heritability of metric and non-metric cranial traits (Sjøvold, 1984; Carson, 2006a,b). The work by Sjøvold (1984) was one of the first surveys of heritability on a human skull pedigreed series and the heritabilities of cranial traits were estimated using regression analysis. Sjøvold (1984) concluded that most of Howell's measurements were significantly hereditable and suggested that the structures showing the highest heritabilities were those connected to the size of the brain, the orbits, the nose and the masticatory apparatus. In a recent study, Carson (2006a) used an ML method to provide alternative estimates of the heritability of Howell's measurements. The main conclusion of this study was in agreement with Sjøvold's study and reported that craniometric traits show low to moderate narrow sense heritabilities. However, Carson (2006a) pointed out some differences and concluded that facial dimensions and cranial breadth measures are the less heritable characters of the skull. According to Carson (2006a) these differences stem from the different statistical techniques used for the heritability estimation.

The patterns of genetic variation of craniometric traits have thus been analysed previously, but the patterns of genetic correlation among them are nearly unexplored. This issue is of crucial importance because morphological integration is pervasive in the human skull (Lieberman et al. 2000a,b; Bookstein et al. 2003; González-José et al. 2004; Bastir et al. 2004; Bastir & Rosas, 2004, 2005, 2006) and integration between characters can limit the evolvability of traits and determine their evolutionary response (McGuigan, 2006).

Morphological integration in the human skull

Integration is expressed through covariation between traits and it plays a key role in the evolution of complex morphological structures such as the human skull, as it can enhance or constrain the evolution of its morphology towards certain directions of shape change (Klingenberg, 2004, 2005). Morphological integration assumes that functionally and/or developmentally related traits will be coinherited and will produce coordinate responses to evolution (Olson & Miller, 1958; Cheverud, 1982, 1984, 1995, 1996a).

The human skull comprises three regions with different developmental origins and functional requirements (Carlson, 1999): the cranial base, the cranial vault and the face. The cranial base is formed from endochondral bone that arises from a cartilaginous precursor originated from mesoderm (Mooney et al. 2002). The base supports the inferior parts of the brain as well as the pons, the medulla oblongata and the brain stem (Richtsmeier, 2002). The cranial vault is formed from membranous bone of paraxial mesodermal and neural crest origin and it gives room and protects the cerebral hemispheres and the cerebellum (Sperber, 2001). The facial skeleton ossifies intramembranously from neural crest precursors (Sperber, 2002) and it surrounds the pharynx as well as the oral, respiratory and orbital cavities, supporting the functions of feeding, breathing and vision. The cranial base is the most ancient structure and has been highly preserved through phylogeny (Carlson, 1999). Therefore, it is considered that the cranial base is under stronger genetic control than the cranial vault and the face (Schilling & Thorogood, 2000; Sperber, 2001). Moreover, it is assumed that the face is the most sensitive skull region to non-genetic factors because it plays a key role in foraging and adaptation to environment and because facial growth is more extended into the postnatal period (Siebert & Swindler, 2002).

The level of integration between these skull regions is a matter of current research. Most studies of morphological integration in the skull of mammals (Hallgrímsson et al. 2004, 2006; Goswami, 2006, 2007), non-human primates (Cheverud, 1982, 1995; Marroig & Cheverud, 2001; Hallgrímsson et al. 2004; Ackermann & Cheverud, 2004b) and humans (Lieberman et al. 2000a,b; McCarthy & Lieberman, 2001; Bookstein et al. 2003; González-José et al. 2004; Bastir et al. 2004; Bastir & Rosas, 2004, 2005, 2006) have considered integration at the phenotypic level. However, researchers have not identified yet which phenotypic units reflect morphogenetic units (Lieberman et al. 2004) and little is known about genetic integration and constraint in the functional and developmental regions of the skull.

The first studies of cranial integration in primates were developed by Cheverud (1982, 1995) and evidenced that functionally and developmentally related traits were in fact integrated. These findings provided support to the functional matrix hypothesis (Moss & Young, 1960), which predicts that covariation within functional units is stronger than covariation within individual bones or osseous subdivisions with different developmental/tissue origins. Afterwards, Hallgrímsson et al. (2004) reported that this functional/developmental pattern of craniofacial integration was consistent in rhesus macaques but not in mice. More recent studies of modularity in mammals (Goswami, 2006, 2007) and primates (Ackermann & Cheverud, 2004b) have identified six phenotypic cranial modules, corresponding to four functional regions of the face (namely the oro-nasal, the molar, the orbital and the zygomatic-pterygoid regions), one neurocranial region (the vault) and one basicranial region (the basicranium). The patterns of covariation within and among regions indicated that the face (the oro-nasal and the molar regions) and the cranial base were the highest integrated structures of the skull, whereas the cranial vault showed differing levels of integration across taxa. According to Ackermann & Cheverud (2004b), the zygomatic region is one of the main sources of facial integration in African apes and humans. Furthermore, they report that the loose integration of the cranial vault provided the skull with more capability to evolve in response to encephalization.

Other studies (Lieberman et al. 2000a,b; Bastir & Rosas, 2004) support the existence of two modules in the human skull, namely the face and the braincase. Lieberman et al. (2000a,b) consider that the basicranium and the neurocranium form a highly integrated morphological unit, the neuro-basicranial complex, which is partially independent from the face. However, Bastir et al. (2006) highlighted that the cranial base can not be interpreted as an integrated unit, at least at the ontogenetic level, as midline and lateral basicranial structures show different growth patterns. Further differences in growth may also explain the lack of integration between the braincase and the face: whereas the basicranium and the neurocranium grow jointly following a rapid neural trajectory (Bastir et al. 2006), facial growth extends more into the postnatal period and is more influenced by environmental factors (especially mechanical loadings). According to this, the face would be more prone to plastic responses (Kohn, 1991; Strand Vidarsdóttir et al. 2002; Bastir & Rosas, 2004), and it has been suggested that from the phylogenetic point of view, facial traits would not be as informative as neuro- and basicranial traits, which are more conservative and would reflect more reliably the underlying genetic patterns (Collard & Wood, 2000; Collard & O’Higgins, 2001).

In the primate skull, the cranial base appears to have a key integrative role (Lieberman et al. 2000a,b; Bookstein et al. 2003; Zollikofer & Ponce de León, 2004). Anatomically, it is a hinge-structure between the face and the cranial vault and developmental and growth studies support this view. Enlow & Hans (1996) suggested that the craniofacial architecture is based on a system of hierarchical modules organized into several craniofacial levels, in which the basicranium responds to modifications of the brain and translates them epigenetically into changes of facial proportions along a cerebro-mandibular gradient. Therefore, the base is the structural foundation that sets out the spatial development of the face and to some extent regulates the overall cranial development via integration with the brain and the cranial vault. Regarding human craniofacial variation, Enlow & Hans (1996) considered that there are two extreme headform types along a continuous spectrum: the dolicocephalic type, which is characterized by a long and narrow skull associated with a flat base and a supero-inferiorly longer face; and the brachycephalic type, in which a short and broad skull is associated with a more flexed cranial base and the face reveals a decreased anterior height and increased breadths. However, this traditional hypothesis of integration is not supported by developmental models of craniofacial biology (Lieberman et al. 2000a; Bastir & Rosas, 2004).

Recent experimental research using mice as animal models (Hallgrímsson et al. 2007) suggests that integration in the mammalian skull is highly structured following a hierarchical scheme that is dominated by strong covariation between the widths of the neurocranium and the basicranium and also with that of the face, but to a lesser extent. This study has further emphasized the stronger integration of the neurocranium and the basicranium with respect to the face, which is more independent but still covaries with the braincase (Hallgrímsson et al. 2007). After analysing the influence of epigenetic factors in craniofacial variation, the authors conclude that phenotypic variation arises from a few key developmental processes (such as brain growth) that channel the underlying genetic variation towards certain phenotypic expressions that maintain an integrated functional skull.

In the present study we reanalyse the pedigreed skull collection from Hallstatt (Austria) to explore the genetic patterns of variation determining the phenotypic expression of the skull and to assess the levels of correlation in craniometric characters. This will allow us to account for both the heritable and the integration patterns of the human skull. Here we test several hypotheses regarding these issues.

Hypotheses

Hypothesis 1 (H1) examines the heritability patterns of facial, neurocranial and basicranial dimensions and tests whether there are differences in the amounts of genetic variation underlying these regions. The null hypothesis states that there are no significant differences among the heritability of each region, whereas rejection of the null hypothesis indicates differential genetic contribution to the phenotype of each region, suggesting that they are subject to different evolvabilities and levels of plasticity.

Hypothesis 2 (H2) explores genetic and phenotypic patterns of correlation of specific suites of craniofacial traits within and among major and minor developmental/ functional regions of the skull. The null hypothesis implies no correlation between the genetic (G) and phenotypic (P) matrices; that is, the patterns of phenotypic correlation do not reflect the genetic ones and show different strengths of morphological integration. The null hypothesis is rejected if the correlation of G and P is high and significant, which would suggest that genetic and environmental effects on development produce similar patterns of phenotypic variation. Thus, in those cases where G is not available, P could be used as a good proxy to G in population quantitative genetic models (Cheverud, 1988).

Hypothesis 3 (H3) tests the traditional hypothesis of integration of the human skull (Enlow & Hans, 1996). Under this hypothesis, maximum cranial breadth should be positively correlated with facial breadth and negatively correlated with facial height, neurocranial length and neurocranial height. The null hypothesis is rejected if the observed patterns of correlation between these pairs of distances do not fit the expected patterns of integration.

Hypothesis 4 (H4) tests whether the overall pattern of genetic integration in the human skull is dominated by the covariation between the maximum widths of the major developmental regions, namely, the face, the neurocranium and the basicranium. This hypothesis was put forward by Hallgrímsson et al. (2007), who investigated the influence of epigenetic factors in the patterns of morphological integration of mice skull. The null hypothesis predicts that the genetic correlations between facial, neurocranial and basicranial width are high and significant.

Materials and methods

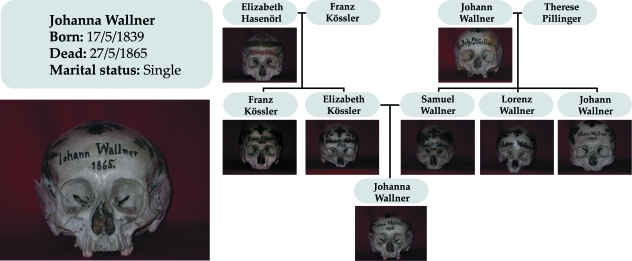
The sample examined here derives from the Hallstatt skull collection, which is a large sample of human skulls with identified familial relationships. It provides the unusual opportunity to perform quantitative genetic analysis in a human skeletal sample. This unique collection is made up of more than 700 decorated skulls that have been accumulating in the charnel house of Hallstatt from the beginning of the 18th century. It stems from a local tradition to honour predecessors (Burgstaller, 1961). On the request of the families, the gravedigger recovered the skeletal remains of their relatives, decorated their skulls with floral paintings and wrote the name of the individual on them (Fig. 1). This custom was widespread in Austrian and German regions surrounding the Alps (Sauser, 1952), but Hallstatt is the only place where it has provided such a large skull series and has endured for so long, the last skull being incorporated in 1996. The series covers a temporal span of more than 250 years, but most of the identified skulls date back to the 19th century.

Fig. 1.

Example of genealogy. The original name (Johanna Wallner) was misspelled (Johann Wallner) when the skull was repainted because of the fading decoration. Sjøvold's photographic records dating back from the seventies revealed the original name. In fact, discriminant analysis on sex confirmed that the skull belonged to a female.

Skull identification and genealogy reconstruction

The name and type of decoration of the skulls allowed us to identify at the parish demographical records almost 60% of the individuals. To reconstruct the genealogies of the Hallstatt population, we compiled the complete parish records of births, deaths and marriages from 1602 to 1900, a total of 18 134 individuals. The most complete families range back up to seven generations, including all kinds of familial relationships from first to fourth degree (Fig. 1). Most of the identified skulls are preserved at the charnel house in Hallstatt (n = 374), but a few of them are on loan to several Austrian Museums: the Musealverein in Hallstatt (n = 3), the Naturhistorisches Museum Wien (n = 17), the Österreichisches Museum für Volkskunde in Vienna (n = 1), and the Anatomisches Institut in Innsbruck (n = 11). Since the first surveys carried out by Sjøvold in 1974 and 1975, 25 identified skulls have disappeared from the charnel house and the names of several individuals have been changed because of recent renewed decoration (Fig. 1).

Morphometric analyses

In this study, we analysed a sample of 355 complete adult skulls of both sexes (144 females and 211 males), 317 of which fall into the extended, multigenerational genealogies. From the total sample of identified skulls (n = 406), subadult (n = 35) and fragmentary individuals (n = 16) were excluded to avoid sample bias. Adulthood was assessed by skeletal criteria, as determined by a fully closed spheno-occipital synchondrosis.

A set of 65 anatomical landmarks (Table 1) was recorded on each skull with a 3D digitizer (Microscribe, Inc.) in two consecutive recordings because it was impossible to access all the landmarks from a single orientation. The two recordings were matched automatically using a custom reference frame in the MUS software (Microscribe Utility Software) defined by three landmarks (nasion, bregma and hormion). Using this reference frame, landmark coordinates were also oriented along the sagittal plane. This means that sagittal landmarks had a z coordinate equal to 0, and symmetrical right and left landmarks only differed in the sign of the z coordinate, one being negative and the other one positive (for more details see Martínez-Abadías, 2007).

Table 1.

List of digitized landmarks. Codes and definitions used are provided (r, right; l, left)

Code	Landmark	Definition
aam l	Anterior auditory meatus	Most anterior point at the external auditory meatus
al r/l	Alare	The most lateral point on the margin of the nasal aperture
alv l	Alveolar point	Posterior limit of the maxillary alveolar arch at the pterygo-alveolar suture
ast r/l	Asterion	The point where the lamboidal, parietomastoid, and occipitomastoid sutures meet
b	Bregma	The ectocranial point where the coronal and sagittal sutures intersect
ba	Basion	The midline point on the anterior margin of the foramen magnum
ek r/l	Ectoconchion	The most lateral and posterior point on the orbital margin
eu r/l	Euryon	The point of greatest breadth of the brain case perpendicular to the sagittal plane
fmo r/l	Frontomalare orbitale	The point where the frontozygomatic suture intersects the orbital margin
fmt l	Frontomalare temporale	The point where the frontozygomatic suture crosses the temporal line
ft r/l	Frontotemporale	The point where the temporal line reaches its most anteromedial position
g	Glabella	The most anterior midline point on the frontal bone, above the frontonasal suture
gle l	Glenoid fossa	The most posterior point on the margin of the glenoid fossa
ho	Hormion	The most posterior midline point on the vomer
i	Inion	Ectocranial midline point at the base of the external occipital protuberance
iam l	Inferior auditory meatus	Most inferior point at the external auditory meatus
izt l	Inferior zygo-temporal	Inferior point at the suture between temporal and zygomatic bones
ju r/l	Jugale	Depth point of the notch between the temporal and frontal processes of the malar
l	Lambda	Midline point of the intersection of the sagittal and lamboidal sutures
m	Metopion	Midline point where the elevation above the chord from n to b is greatest
mf r/l	Maxillofrontale	The point where the anterior lacrimal crest meets the frontomaxillary suture
ms l	Mastoidale	The most inferior point on the mastoid process
mw r/l	MW	Tip of the process at the infratemporal crest
n	Nasion	The midline point where the two nasal bones and the frontal intersect
nar r/l	Nariale	The most inferior point on the nasal aperture
o	Opisthion	The midline point at the posterior margin of the foramen magnum
oc l	Optic canal	Most superior, medial and anterior point of the optic canal
op	Opisthocranion	The posterior-most point of the skull in the medial sagittal plane
or r/l	Orbitale	The lowest point on the orbital margin
pam l	Posterior auditory meatus	Most posterior point at the external auditory meatus
pns	Posterior nasal spine	Vomer–palatin junction
po l	Porion	The uppermost point on the margin of the external auditory meatus
pt r/l	Pterion	The point where the frontal, parietal, temporal and sphenoides bones meet
ra r/l	Radicular	Lateral point on zygomatic process of the temporal bone at the postglenoid
ss	Subspinale	The deepest point seen in the profile below the anterior nasal spine
stf l	Stylomastoid foramen	Stylomastoid foramen
szt l	Superior zygo-temporal	Superior point at the suture between temporal and zygomatic bones
v	Vertex	Midsagittal superior point of the cranium when the skull is in Frankfurt
zy r/l	Zygion	The point of maximum lateral extent on the surface of the zygomatic arch
zym r/l	Zygomaxillare	The most inferior point of the zygomaticomaxillary suture
zyma r/l	Zygomaxillare anterior	The most anterior point on the zygomaticomaxillary suture
zyo r/l	Zygoorbitale	The point where the orbital rim intersects the zygomaticomaxillary suture

From the total set of landmarks, five landmarks from the alveolar region (prosthion, inner prosthion, ectomolare right and left, and palate) were removed because they were missing in more than 50% of the cases due to tooth loss and high levels of alveolar bone resorption.

Measurement error was evaluated by a repeated recording of a subsample of 91 individuals each of which were digitized twice. Analysis of shape variation (anova on repeated measurements implemented with SAS) showed that the component of variation among individuals is 11.5 times the component of variation between repeated measurements. Thus, repeatability (the proportion of variance due to individual differences rather than measurement error) is 92%. Outlier points were detected by means of Box and Whisker plots assuming an outlier coefficient of 1.5. These points were deleted and considered missing data.

The overall percentage of missing values was 2.18%, and these were replaced by two different methods. If the missing landmark had a symmetric counterpart (as for example the asterions), it was directly replaced by coordinate reflection. As landmark coordinates were oriented along the sagittal plane, this was done by copying the x, y, z coordinates of the symmetric landmark and changing the sign of the z coordinate. If missing landmarks did not have a symmetric counterpart, they were replaced by multivariate regression.

To validate the individual identifications made by the gravediggers who decorated the skulls, we confirmed their sex assignment by performing a discriminant function analysis, considering sex as the discriminant variable. It is acknowledged that this is just a partial appraisal, as it only detects those cases in which a female and a male have been confused and neglects those cases where two individuals of the same sex may have been exchanged. Other kinds of errors are almost impossible to detect, but they are expected to be negligible because if any identification mistake was made, the most probable is that members of the same family buried within the same grave were confused. Thus, their influence is expected to produce slight underestimates of the additive genetic component of the morphological phenotype. Results showed that only eight skulls have an overall posterior probability higher than 0.85 of being the opposite sex. These individuals were considered misidentifications and were not taken into account for the estimation of the genetic parameters.

Finally, we estimated 58 linear inter-landmark distances from the three-dimensional landmark coordinates by applying the Pythagorean theorem. The linear distance between two landmarks is estimated using the following formula:

where (x₁, y₁, z₁) are the landmark coordinates of landmark 1, and (x₂, y₂, z₂) the landmark coordinates of landmark 2. Of these distances, 24 correspond to Howell's measurements (Howells, 1973) or are close approximations to them (i.e. the prosthion is substituted by the subspinale). The distances were assigned to the three major regions of skull, which have different developmental origins: the face, the neurocranium and the basicranium (Cheverud, 1995; Hallgrímsson et al. 2004, 2007). Distances within the face were also assigned to minor functional regions, such as the nasal, the orbital and the zygomatic regions (González-José et al. 2005; Sardi & Ramírez-Rozzi, 2007). Distances covering several regions were grouped into another category, the inter-regional dimensions.

All these calculations were performed with statistica 6.0 software package (Statsoft, Inc.).

Quantitative genetic analyses

Maximum likelihood methods were used to estimate the components of variance of the cranial measurements. With these estimates, the narrow sense heritability of each distance was computed as the proportion of phenotypic variance attributable to additive genetic effects (Lynch & Walsh, 1998). ML methods are usually applied under a mixed linear model that jointly accounts for fixed and random effects to describe the phenotype of each individual (Lynch & Walsh, 1998). The phenotypic variance is broken down into its components of additive genetic value and other random environmental and fixed effects. The components of variance are estimated by an iterative procedure that maximizes the likelihood of observing the actual data (Lynch & Walsh, 1998). ML analytical methods are advantageous compared with parent–offspring regression or sib analyses because they incorporate multigenerational information from unbalanced datasets (Konigsberg, 2000).

We computed the variance components of the craniometric traits using the solar 4.0.4 software package (Almasy & Blangero, 1998), which is available online at http://www.sfbr.org/solar/. solar provides estimates of the additive genetic variance and the variance of the residual errors, and computes the narrow sense heritability of the analysed traits. The significance of the heritability estimates is tested using likelihood ratio tests, in which the obtained likelihood of the model with the additive genetic variance component and covariates is compared with the obtained likelihood of the model with the additive genetic variance component constrained to be zero.

The mixed model used in this study included sex, year of birth and the interaction of sex and year of birth as covariates. Moreover, as 12.4% of the individuals showed slight dysmorphologies possibly related to craniosynostosis, deformation was also considered as a covariate. This kind of dysmorphology (occipital flattening and prominent forehead) was also reported in a very similar skull sample from Berg (Austria) (Howells, 1989). This author pointed to cradling practices as possible causes of these deformations, but did not rule out other non-artificial or genetic effects.

solar tested the significance of each covariate and computed the amount of variation explained by the significant ones at the P < 0.10 level. This is done by comparing the likelihood of a model that included each of the covariates separately with the likelihood of a model that excluded them. The final model used to compute the narrow sense heritabilities of the traits only retained the significant covariates.

Before analysis, the traits were inverse normalized using the ‘inormal’ procedure implemented in solar to avoid potential problems of kurtosis of continuous metric traits. Inverse normalization forces marginal (univariate) normality, but not bivariate normality. As all traits have a mean near 0.0 and a standard deviation near 1.0 after normalization, the variances and covariances are no longer available and only patterns of heritability and correlations can be explored. To compute the inverse normalization, the trait values are sorted from the minimal to the maximal value, and for any value a quantile is computed for it by the formula I/(N + 1), where I is the position in the sorted list and N is the total number of observations of the list. For example, the skull with the smallest value of a given measurement would receive a score of 0.0028 [the quantile 1/(355 + 1)], whereas the skull with the highest score, a score of 0.9971 [355/(355 + 1)]. The inverse normal cumulative density function is computed for each quantile and stored in an array keyed by the identification number of the skull. When different individuals show the same value of a measurement, the inverse normal is computed for each applicable quantile. Then the inverse normal is averaged and this average is the value that is stored for each individual.

To test whether there are differences in the amounts of genetic variation at each region (H1), we performed a two-tailed t-test that compared the average heritability estimations of the three sets of measurements (representing the facial, neurocranial and basicranial regions). To analyse the genetic and phenotypic correlation patterns of the skull (H2–H4), we computed the correlation between all possible pairs of distances of maximum breadth, height and length within and among major and minor developmental/functional regions of the skull. To estimate the genetic correlation between pairs of distances we used the solar bivariate models. The bivariate models work similarly to the univariate models, but also estimate the correlation between two traits: the phenotypic correlation is broken down into genetic and residual correlations (Lynch & Walsh, 1998). To estimate the phenotypic correlation we calculated the parametric Pearson's correlation using statistica 6.0. The correlations of each of the trait pairings were computed separately and then the correlation matrices were constructed manually in an excel spreadsheet.

To test the similarity between the genetic and the phenotypic correlation matrices (H2) we used a matrix correlation (Cheverud, 1988) and assessed its significance with a Mantel test (Mantel, 1967) after 100 000 permutations of the original matrices. According to Cheverud (1988), the level of heritability influences the similarity between genetic and phenotypic correlation patterns: if heritability is high it increases both the accuracy of the genetic correlation estimates and the similarity of G and P; if it is low or moderate, the accuracy is reduced and similarity of G and P suggests that genetic and epigenetic factors are channelled through the same developmental process. In this latter case, the levels of genetic correlation usually exceed that of phenotypic correlations. To assess the reliability of the genetic correlation estimates, we measured the effective sample size (N_es) used in our analyses, as suggested by Cheverud (1988). This is a rough measure of the actual sample size and is derived as the product of the number of nuclear families on which the estimation of the genetic parameters and the mean heritability of the traits are based. Previous evidence suggests that an effective sample size of at least 40 should be used to guarantee the reliability of the data (Cheverud, 1988).

Finally, to test the integration hypotheses (H3 and H4) we compared the expected patterns of genetic correlations between the involved measurements with the observed ones. The null hypothesis is rejected if the observed patterns of correlation between these pairs of distances do not fit the expected patterns of integration.

Results

The univariate maximum likelihood estimates of heritability of facial, neurocranial and basicranial dimensions are presented in Tables 2–5. As a summary of these results, we present in Fig. 2 the five most heritable traits of each region. The obtained heritabilities are comparable between regions because the estimation of the phenotypic and genetic variance components was always based on the same number of individuals. Results show that craniofacial traits are low to moderate heritable characteristics. Heritability values ranged from 0.00 to 0.43, and 72.2% of them were significant at the 0.05 level. Regarding the regional patterning of heritabilities, the face is the skull region with a highest number of significantly heritable traits (81%) and the highest mean heritability (0.26), followed by the basicranium (73% and 0.23) and the neurocranium (61.5% and 0.19). The percentage of significant heritability estimates within the inter-regional dimensions was 50%. Despite these slight differences, there is no clearcut difference among regions and the t-test showed that the comparisons of the genetic amounts of variation among regions were not statistically different. The statistical significance of the differences between the average heritability of the three regions was as follows: facial vs. neurocranial (P = 0.053); facial vs. basicranial (P = 0.433); and neurocranial vs. basicranial (P = 0.336). Therefore the H1 null hypothesis cannot be rejected.

Table 2.

Facial dimensions: Narrow-sense heritability estimations (h²) and associated standard errors (SE). Statistical significant estimations (α = 0.05) are in bold. The proportion of variation explained by the significant covariates (α = 0.1) is also provided. Each measure corresponds to the distance between two landmarks (see Table 1 for definitions) and has been assigned to a minor function region within the face (although some distances may cover several regions)

Distance							Covariates
Measure		Landmarks	Howells	h2	SE	P	Variance explained	Significant covariates
Total	height	ss>n	NPH*	0.34	0.13	0.002	0.15	sex
	length	ss>ba	BPL*	0.32	0.12	0.001	0.14	sex
	breadth	zy r>zy l	ZYB	0.28	0.13	0.008	0.43	sex, year birth
Orbital	breadth	fmor r>fmo l	FMB	0.40	0.13	0.001	0.19	sex
	length	or l>oc l		0.35	0.14	0.004	0.06	sex
	breadth	ek r>ek l	EKB	0.34	0.14	0.005	0.17	sex, year birth
	breadth	mf r>mf l	DKB*	0.33	0.13	0.003	0.05	sex
	height	or l>fmo l		0.29	0.14	0.015	−	−
	breadth	mf l>ek l	OBB*	0.28	0.14	0.013	0.07	sex
Nasal	height	n>nar r/l	NLH	0.43	0.13	0.000	0.13	sex
	length	ss>pns		0.38	0.14	0.001	0.19	sex, year birth
	breadth	al r>al l	NLB	0.00	0.00	0.500	0.05	sex, year birth
Zygomatic	height	szt l>izt l		0.38	0.13	0.001	0.11	sex, deformation, year birth
	length	zym l>gle l		0.37	0.12	0.000	0.15	sex, year birth
	height	zyma l>fmo l		0.34	0.13	0.004	0.10	sex
	length	izt l>mw l		0.28	0.11	0.002	0.15	sex
	height	zym l> or l	WMH	0.24	0.12	0.014	0.13	sex
	height	zyo l>fmo l		0.23	0.14	0.029	0.32	sex
	length	or l>izt l		0.22	0.12	0.024	0.17	sex, year birth
	length	fmo l>fmt l		0.22	0.12	0.020	0.04	sex
	length	zyma l>izt l	IML	0.22	0.13	0.037	0.13	sex, deformation, year birth
	length	zyo l>izt l	XML	0.20	0.11	0.018	0.23	sex, deformation, year birth
	breadth	ju r>ju l	JUB	0.19	0.13	0.071	0.38	sex, year birth
	height	zyo l>zyma l		0.09	0.10	0.143	0.13	sex
	breadth	zymar>zyma l	ZMB	0.07	0.10	0.232	0.23	sex, year birth
	height	or l>zyma l		0.03	0.10	0.364	0.13	sex

^*A close approximation to the exact Howell's measurements (1973).

Table 5.

Inter-regional dimensions: Narrow-sense heritability estimations (h²) and associated standard errors (SE). For coding details see Table 2

Distance							Covariates
Measure		Landmarks	Howells	h2	SE	P	Variance explained	Significant covariates
Other	length	zym l>ra l		0.34	0.12	0.001	0.19	sex
	length	n>op	NOL	0.34	0.13	0.001	0.15	sex
	length	po l>ss		0.32	0.12	0.003	0.24	sex, year birth
	breadth	ho>alv l		0.29	0.16	0.034	0.30	sex
	height	op>i		0.13	0.12	0.116	0.04	sex, deformation
	length	n>b	FRC	0.11	0.12	0.161	0.14	sex, deformation
	length	po l>n		0.07	0.11	0.267	0.21	sex, year birth
	height	po l>b		0.03	0.12	0.383	0.22	sex, deformation

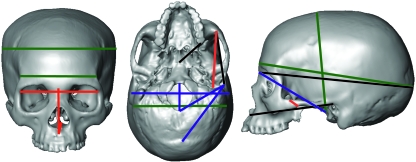
Fig. 2.

Frontal, lateral and inferior views of a human skull showing the cranial dimensions with higher heritabilities. Colours indicate dimensions from the facial (red), neurocranial (green), and basicranial (blue) regions. Inter-regional dimensions are depicted in black.

Regional heritability estimations

Total facial dimensions (maximum facial breadth, length and height) have moderate heritabilities, showing that additive genetic variation accounts for approximately 30% of the phenotypic variation of these traits (Table 2). Minor functional regions within the face show diverse patterns of genetic variation: the orbital and the nasal regions show some of the highest amounts of genetic variance and thus the highest heritabilities of the skull (Fig. 2), whereas the zygomatic or masticatory apparatus tend to show lower estimates (Table 2). The mean heritabilities of the nasal, orbital and zygomatic regions are 0.27, 0.33 and 0.22, respectively. Total breadth, length and height orbital measurements show moderate to high significant heritability estimates. Other breadth measures such as the bi-orbital breadth and the interorbital breadth also show moderate heritabilities. Nasal height and length show high heritability estimates, but nasal breadth shows no additive genetic variance at all (Table 2). The t-test comparison for functional facial regions showed that the orbital region is significantly more heritable than the zygomatic (P = 0.044). Further comparisons did not provide any significant differences.

Total neurocranial dimensions (Table 3) also have moderate significant heritabilities. The anterior breadth measure and the maximum cranial breadth measure have indeed high estimates (Fig. 2). The other neurocranial measurements tend to show low heritability estimates, whereas Howell's chord distances show no genetic variation at all. All neurocranial breadth measures are significantly heritable, except the distance between pterions.

Table 3.

Neurocranial dimensions: Narrow-sense heritability estimations (h²) and associated standard errors (SE). For coding details see Table 2

Distance							Covariates
Measure		Landmarks	Howells	h2	SE	P	Variance explained	Significant covariates
Total	breadth	eu r>eu l	XCB	0.36	0.14	0.002	0.17	sex, year birth
	length	g>op	GOL	0.31	0.12	0.002	0.18	sex
	height	b>ba	BBH	0.24	0.12	0.016	0.18	sex, deformation
Other	breadth	ast r>ast l	ASB	0.23	0.14	0.034	0.05	sex
	breadth	ft r>ft l		0.23	0.12	0.024	0.07	sex
	length	m>b		0.22	0.12	0.020
	breadth	pt r>pt l		0.21	0.15	0.072	0.13	sex, year birth
	height	g>m		0.20	0.12	0.031	0.16	sex, deformation
	length	v>l		0.19	0.12	0.043	0.03	sex
	breadth	mw r>mw l	WCB*	0.16	0.11	0.050	0.05	sex
	length	b>l	PAC	0.06	0.10	0.262	0.07	sex
	height	l>op	OCC	0.04	0.12	0.379	0.02	sex, year birth
	length	b>v		0.00	0.00	0.500

The heritability estimates of the basicranial region (Table 4) were moderate and significant, except for the distances between the inion and the opisthion, the mastoid height and the otic height. The length of the foramen magnum as well as the auricular breadth showed some of the highest heritability estimates, whereas total cranial base length and height show more moderate estimates (Fig. 2).

Table 4.

Basicranial dimensions: Narrow-sense heritability estimations (h²) and associated standard errors (SE). For coding details see Table 2

Distance							Covariates
Measure		Landmarks	Howells	h2	SE	P	Variance explained	Significant covariates
Total	breadth	ba>po l		0.29	0.12	0.005	0.20	sex
	length	n>ba	BNL	0.24	0.10	0.003	0.17	sex
Other	breadth	ra r>ra l	AUB*	0.40	0.12	0.000	0.19	sex
	length	o>ba	FOL	0.38	0.13	0.001	0.13	sex
	breadth	i>po l		0.27	0.14	0.011	0.17	sex, year birth, sex*year birth
	breadth	adml>pam l		0.23	0.14	0.030	−	−
	breadth	po l>ho		0.21	0.14	0.049	0.22	sex, year birth
	breadth	ba>ho		0.20	0.12	0.034	0.04	sex
	length	i>o		0.16	0.12	0.080	0.02	year birth
	height	ms l>stf l		0.15	0.12	0.081	0.15	sex
	height	po l>iam l		0.00	0.12	0.486	0.07	sex, deformation, year birth

Finally, inter-regional dimensions show two different patterns (Table 5). Those distances that mostly cover the face show moderate and significant heritabilities, whereas those mostly covering the cranial vault tend to show low and non-significant estimates, although one exception is the distance from the nasion to the opisthocranion (Fig. 2).

Regarding the covariates included in the analyses (Tables 2–5), sexual dimorphism was the most important effect as it affected more than 90% of the measurements, especially the facial ones. The second most important effect was the temporal span of the sample, which could be reflecting morphological secular trends: year of birth significantly affected facial (41.7%), neurocranial (25%) and basicranial dimensions (40%). Finally, deformation had a smaller effect but significantly affected three facial dimensions, two neurocranial, one basicranial and three inter-regional dimensions. The joint effect of sex and year of birth only influenced one measurement from the cranial base.

Genetic and phenotypic integration

Our results show that the observed genetic and phenotypic correlation patterns of skull integration are consistent in our sample. The matrix correlation between G and P was high (r = 0.74) and the Mantel test revealed that it was highly significant (P < 0.000). Thus, we reject the H2 null hypothesis, which expected independence between these matrices. This suggests that P can be used as a good proxy of G. However, a closer look at the correlation matrices (see Appendix 1) reveals that genetic integration is more constrained to specific dimensions, whereas phenotypic integration is more widespread throughout the skull. Almost all phenotypic correlations were highly statistically significant, even when the correlation was low. Genetic correlations were usually higher than the phenotypic ones, but few of them were statistically significant due to large standard errors. This was an expected result as heritabilities were all low to moderate. To confirm that the genetic correlations were well estimated, we computed the effective sample size (N_es) and we found that it exceeds the minimal threshold value suggested by Cheverud (1988). In fact, there were skull data for 209 families, the mean heritability was 0.23 and thus the effective sample size was 47.4. This result confirms that the genetic correlations are reliable and that G and P are similar because both genetic and environmental components of phenotypic variation are channelled through the same developmental pathways.

Regarding integration hypotheses, the patterns of genetic and phenotypic correlations between facial and neurocranial dimensions do not follow Enlow's expected pattern of craniofacial variation and headform in humans (Enlow & Hans, 1996). As predicted by the hypothesis, maximum cranial breadth is positively correlated with facial breadth (r = 0.89, P = 0.007), but it does not correlate negatively with facial height (r = 0.47, P = 0.11), neurocranial length (r = 0.49, P = 0.06) or neurocranial height (r = 0.16, P = 0.72). Thus, we reject the H3 null hypothesis because neither the genetic nor the phenotypic observed patterns of correlation fit the pattern expected by the traditional hypothesis of integration (Enlow & Hans, 1996).

Finally, our results confirm the hypothesis of strong covariation between the breadth measures of major developmental regions of the skull (Hallgrímsson et al. 2007). The genetic correlations of facial, neurocranial and basicranial breadth measures were high and statistically significant and dominate the patterns of integration of the human skull (r_f-b = 0.90, P = 0.014; r_n-b = 0.93, P = 0.007; r_f-n = 0.89, P = 0.007). Therefore we do not reject H4 null hypothesis and support the hypothesis that this correlation pattern prevails in integration of the skull.

Discussion

This study explored the levels of genetic variation and correlation of craniometric traits through a developmental/functional approach to assess the evolutionary potential of the human skull. The above results confirm that the human skull has substantial amounts of genetic variation, which confers to the skull a high ability to evolve (Tables 2–5). However, evolvability is compromised by complex patterns of genetic integration that may constrain the potential evolution of the skull towards certain directions of change (Appendix 1). That is, free evolution of the skull is unlikely because of morphological integration, and this suggests that the developmental system plays an important role, channelling the paths through which genetic and environmental components of phenotypic variation can be expressed (Cheverud, 1988, Lieberman et al. 2004).

It has been suggested that the different cranial regions could be subject to different levels of evolvability and/or plasticity (Kohn, 1991; Strand Vidarsdóttir et al. 2002; Bastir & Rosas, 2004). We tested this assumption in hypothesis H1 and we did not find significant differences between the amounts of genetic variation underlying the three major developmental regions of the skull. Craniometric traits from the face, the cranial vault and the base show similar percentages of significant heritability estimations and low to moderate levels of genetic components of variation. This result confirms previous evidence indicating that within the primate skull basicranial, neurocranial and facial dimensions show similar levels of heritability (Cheverud & Buikstra, 1982; Sjøvold, 1984; Cheverud, 1996b). Moreover, there is no evidence suggesting that the face is the most plastic region of the skull. For instance, our results showed that some facial dimensions associated with functional regions (such as the nasal, the orbital and the zygomatic regions) have some of the highest heritabilities of the skull (Fig. 2). Characters with no heritability, with all their variation due to environmental effects, are not limited to the face but are widespread through the whole skull and can also be found at the neurocranium and the basicranium (Tables 2–5).

Our results support the hypothesis that the cranial base is more conservative and may be under slightly stronger genetic control, as most distances within the basicranium show moderate and significant heritabilities, and phenotypic and genetic correlations between the width and length of the cranial base are strong (Appendix 1). Also, we corroborate the hypothesis that the cranial base acts as the ‘skull's central integrator’ (Lieberman et al. 2000a,b, 2002). In fact, the cranial base strongly influences the overall cranial shape, constraining facial breadth, height and length, as well as neurocranial breadth and length. This mechanism would contribute to preventing the different regions from evolving independently and would preserve the functional and architectural requirements of the skull.

Craniofacial traits have substantial amounts of genetic variation, but are significantly affected by other non-genetic factors such as sex and year of birth, as revealed by the genetic analyses. This may be reflecting the influences of sexual dimorphism and secular trends in the Hallstatt population. Sexual dimorphism is one of the main sources of intraspecific variation in skull morphology, which is probably the result of allometric factors and differences in body composition between males and females (O’Higgins & Dryden, 1993; Rosas & Bastir, 2002). Therefore, it is not an unexpected result that sex was a significant covariate affecting most of the measurements; especially because linear distances were not corrected for size.

Secular changes are also a well-known source of morphological variation involving both genetic and environmental components (Jantz & Meadows Jantz, 2000). Secular trends may have been driven by random genetic changes by gene flow (Lahr, 1996) and admixture, as well as by specific adaptations due to a release in selective pressures due to masticatory, dietary, and technological changes (Larsen, 1997). Secular trends have been reported in American (Jantz & Meadows Jantz, 2000) and European (Rösing & Schwidetzky 1979; 1984) populations, including Hallstatt (Sjøvold 1990, 1995; Carson, 2006a). These studies showed that the secular trends detected in Hallstatt follow the general trend of gracilization of European modern populations (Henneberg et al. 1978; Rösing & Schwidetzky, 1979, 1984). In Hallstatt, at least from the transition between the 18th and the 19th centuries, there was a reduction of maximum cranial breadth, accompanied by an increase of neurocranial height (Sjøvold 1990, 1995; Carson, 2006a). Here, we have not assessed the temporal gradient of cranial measurements, but we have found evidence that the minor functional regions (i.e. the zygomatic and nasal regions) of the skull are the most affected (Table 2), which could be reflecting dietary and climatic changes (Jantz & Meadows Jantz, 2000). Neurocranial and basicranial dimensions are also affected, especially the maximum cranial breath (Table 3), and these changes might be caused both by genetic and non-genetic factors.

Hypothesis 2 (H2) tested the similarity between the genetic and the phenotypic correlation matrices, and the Mantel test revealed that they are significantly similar. This is important because many studies are using phenotypic data in population genetic models without any knowledge of the genetic architecture of the skull (Steadman, 2001; González-José et al. 2003, 2005, 2007; Ackermann & Cheverud, 2004a; Roseman, 2004; Schillaci & Stojanowski, 2005; Stojanowski, 2005; Martínez-Abadías et al. 2006; Stojanowski & Schillaci, 2006). This is done assuming that the G and P matrices are similar and proportional, a conclusion drawn from Cheverud's (1988) work. This study compared genetic and phenotypic correlation matrices obtained from 23 published studies, which included a wide range of animals (from human to amphipods) and of kinds of traits (from morphological to cognitive). Here we provide empirical data exclusively for human craniometric traits and support the view that G and P display consistent patterns of morphological variation (Cheverud, 1988).

The proportionality of G and P, however, is not a straightforward consequence of the similarity between these correlation matrices. We could not directly assess the proportionality of G and P variance-covariance matrices because variances and covariances are not available after the inverse normalization. However, in another study (Martínez-Abadías, 2007) we tested this assumption using a set of multivariate landmark data representing the shape of the cranium and applying geometric morphometric methods. Our data strongly contradicted this expectation (Martínez-Abadías, 2007). This result, along with earlier findings from mice (Klingenberg & Leamy, 2001) and humans (Sherwood et al. 2008), supports theoretical arguments (Willis et al. 1991) and suggests that phenotypic data may introduce a potential bias in population and quantitative genetic studies unless the sample size is sufficiently large or familial information is available (Sherwood et al. 2008). In conclusion, our analyses suggest that the genetic and the phenotypic covariation matrices are similar but not identical or proportional (Martínez-Abadías, 2007). Genetic covariation matrices show more complex and structured patterns of morphological integration than the phenotypic covariation matrices. This should be taken into account in studies using P as a proxy of G variance-covariance matrix.

Regarding the pattern of genetic correlations between facial and neurocranial dimensions considered in H3, our results show that these patterns do not follow Enlow's expected pattern of craniofacial variation and headform in humans (Enlow & Hans, 1996). Under this hypothesis, maximum cranial breadth should be positively correlated with facial breadth and negatively correlated with facial height, neurocranial length and neurocranial height. However, we only found a significant correlation between neurocranial and facial breadth, as has been previously hypothesized (Weidenreich, 1941) and supported by studies of artificial cranial deformation (Antón, 1989). Therefore, we conclude that the traditional classification between dolico- and brachycephalic skulls does not reflect the genetic architecture of the human skull or provide any valuable hypothesis of morphological-genetic integration. This is relevant because many bio-anthropological issues are still being synthesized in terms of dolico- vs. brachycephalic forms. For instance, the classical study of Boas on European immigrants to USA (Boas, 1912; Gravlee et al. 2003; Relethford, 2004), studies of morphological variation among ancient and modern Native Americans (Gonzalez et al. 2003; Fiedel, 2004) and studies analysing the relationship among head shape and climate (Beals, 1972; Goodman, 1995, 1997) still use this terminology to describe human craniofacial variation.

The clearest integrated module is formed by breadth dimensions covering the neurocranium, the basicranium and the face: the overall pattern of integration in the human skull is dominated by the covariation between the maximum widths of the major developmental regions. This pattern was first reported in the mice cranium (Hallgrímsson et al. 2007) and here we extend it to humans. Evolutionary developmental studies use model organisms such as mice to identify candidate genes that are involved in the phenotypic expression of skull morphology (Lieberman et al. 2004; Hallgrímsson et al. 2004, 2006, 2007). To extrapolate the results obtained from such organisms to humans it is important to compare them with other primate species. Hallgrímsson et al. (2004) compared phenotypic and genetic correlations in macaques and two strains of mice and did not find a consistent pattern of modularity in these groups. Therefore, it is relevant to find the same predicted pattern of integration in humans and mice. This suggests that covariation between cranial widths is an integrated feature that has been conserved across the evolution of the mammalian craniofacial form.

The present study presents similarities but also some differences to previous analyses carried out with the Hallstatt skull collection (Sjøvold, 1984, Carson, 2006a). Although they are all grounded on the same population, results are not totally coincident. However, this is not an unexpected output as each study took its point of departure from different familiar data, accounted for different sources of covariation and did not use exactly the same crania. As sample size is limited, standard errors are substantially large (Falconer & MacKay, 1996) and slight differences in sample composition, model definition and data treatment can alter the results. Therefore, general trends are more reliable quantitative parameters than the exact value of the heritability estimations. In common, all studies have shown that craniometric traits are low to moderate hereditary characteristics. However, we do not confirm previous evidence suggesting that breadth and facial dimensions are the less heritable characters of the human skull (Carson, 2006a). This study reports low to moderate heritability estimates for breadth measures (Tables 2–5) and has tested statistically that there are no significant differences in the amount of genetic variation underlying the main developmental regions of the skull. Although we used the same statistical method to estimate heritability (ML), inconsistencies between studies might also arise due to other methodological issues regarding the number of skulls included in the analyses and the complexity of the pedigree structure. In this study, we extended and revised the pedigrees constructed by Sjøvold (1984), checked the identifications made by the gravedigger by sex confirmation, and thanks to Sjøvold's photographic records from the mid seventies we could identify the original names of the individuals (Fig. 1). In comparison with previous studies, our analysis included a larger skull sample, did not contain missing values and used larger and more complex genealogies since the whole population was reconstructed.

Understanding the patterns of morphological integration among skull regions will improve our ability to make evolutionary and phylogenetic inferences about human evolution. The use of craniodental characters in phylogenetic analyses of primate and hominid evolution is widespread (Strait et al. 1997; Strait & Grine, 1999; Strait et al. 2007; Lockwood, 2007) and they are essential because cranial remains are one of the main sources of information on extant and fossil species (Ackermann & Cheverud, 2004b; Lockwood, 2007). Although skull morphology is affected to some extent by environmental factors and is under lower genetic control than molecular traits, it is accepted that craniometric traits are phylogenetically informative (Collard & Wood, 2007; Lockwood, 2007). However, as there is strong evidence that morphological integration plays an important role in evolutionary biology and can bias the results of such cladistic analyses (Strait et al. 2007; Lockwood, 2007), further understanding about how and why morphological complexes arise in the skull is needed.

Our analysis reports that the human skull has substantial amounts of genetic variation that are constrained by integration. Furthermore, it demonstrates that craniometric traits from the face, the neurocranium and the basicranium do not differ in their heritability patterns. We also provide empirical evidence that genetic and phenotypic correlation patterns in the human skull are consistent and show similar morphological variation patterns. Regarding integration, results suggest that traditional integration hypotheses (Enlow & Hans, 1996) do not have a genetic basis, but confirm recent modularity patterns found in mice, emphasizing strong covariation between relative widths of the neurocranium, the basicranium and the face as the most dominant integration pattern in the mammal skull (Hallgrímsson et al. 2007).

Our results concerning the heritability and correlation patterns of craniometric traits shed light into the genetic architecture of the human skull. Also, they are especially useful to provide an evolutionary context based on quantitative genetics for classic morphometric studies and databases using univariate measurements. For a greater comprehension of modularity and integration patterns in the skull, future analyses should account for the multivariate nature of shape (Klingenberg, 2004). This could be achieved by combining quantitative genetic methods with geometric morphometric tools, as suggested by Klingenberg & Leamy (2001). It would then be possible to discuss in greater detail the genetic and modular basis of complex phenotypes.

Appendix 1

Genetic correlations (lower left) and phenotypic correlations (upper right) among cranial distances. For genetic correlations, the associated standard errors (SE) are also provided. Significant correlations at the 0.05 level are in bold. Note that the genetic correlations involving nasal breadth were non-computable because its heritability was 0.00. B, breadth; H, height; L, length

		Facial			Neural			Basal		Nasal			Orbital			Zygomatic
		B	H	L	B	H	L	B	H	B	H	L	B	H	L	B	H	L
Facial	B		0.47	0.43	0.68	0.41	0.47	0.7	0.49	0.25	0.46	0.49	0.42	0.17	0.4	0.61	0.45	0.56
	H	0.57 ± 0.24		0.24	0.31	0.31	0.41	0.41	0.34	0.05	0.88	0.42	0.37	0.28	0.14	0.42	0.55	0.37
	L	0.42 ± 0.24	0.42 ± 0.26		0.21	0.3	0.48	0.37	0.8	0.2	0.18	0.72	0.27	−0.11	0.41	0.43	0.06	0.37
Neural	B	0.89 ± 0.11	0.47 ± 0.25	0.46 ± 0.24		0.28	0.33	0.52	0.26	0.18	0.28	0.28	0.31	0.15	0.24	0.36	0.3	0.29
	H	−0.01 ± 0.45	0.17 ± 0.39	0.43 ± 0.30	0.16 ± 0.40		0.36	0.49	0.52	0	0.29	0.34	0.25	0.04	0.33	0.3	0.23	0.33
	L	0.56 ± 0.20	0.69 ± 0.21	0.50 ± 0.21	0.49 ± 0.20	0.61 ± 0.25		0.41	0.61	0.11	0.41	0.44	0.31	0.07	0.33	0.33	0.29	0.34
Basal	B	0.90 ± 0.13	0.78 ± 0.26	0.62 ± 0.26	0.93 ± 0.14	0.40 ± 0.35	0.50 ± 0.24		0.5	0.14	0.38	0.42	0.25	0.1	0.34	0.45	0.3	0.41
	L	0.43 ± 0.23	0.45 ± 0.24	0.96 ± 0.07	0.63 ± 0.22	0.56 ± 0.25	0.75 ± 0.15	0.79 ± 0.23		0.13	0.33	0.59	0.36	−0.01	0.4	0.39	0.19	0.46
Nasal	B	1	−1	1	−1	1	1	1	1		0.17	0.07	0.21	0.05	0.23	0.36	0.14	0.06
	H	0.57 ± 0.23	0.94 ± 0.05	0.23 ± 0.24	0.48 ± 0.23	0.27 ± 0.34	0.52 ± 0.21	0.61 ± 0.26	0.25 ± 0.23	−1		0.36	0.35	0.31	0.16	0.42	0.58	0.28
	L	0.71 ± 0.18	0.77 ± 0.16	0.86 ± 0.08	0.61 ± 0.19	0.75 ± 0.23	0.60 ± 0.17	1	0.91 ± 0.10	1	0.57 ± 0.19		0.28	−0.02	0.36	0.4	0.21	0.45
Orbital	B	0.19 ± 0.37	0.98 ± 0.36	0.60 ± 0.34	0.35 ± 0.32	0.26 ± 0.47	0.00 ± 0.33	0.27 ± 0.40	0.45 ± 0.30	−1	0.64 ± 0.32	0.75 ± 0.29		0.26	0.18	0.31	0.32	0.25
	H	−0.08 ± 0.30	0.59 ± 0.30	−0.63 ± 0.22	0.1 ± 0.32	−0.44 ± 0.38	−0.16 ± 0.30	−0.07 ± 0.35	−0.43 ± 0.26	1	0.65 ± 0.26	−0.62 ± 0.24	0.31 ± 0.41		−0.05	−0.01	0.52	0.08
	L	0.00 ± 0.33	0.08 ± 0.31	0.36 ± 0.24	0.45 ± 0.27	1	0.30 ± 0.26	0.66 ± 0.29	0.71 ± 0.22	1	0.03 ± 0.27	0.23 ± 0.26	−0.03 ± 0.38	−0.41 ± 0.28		0.41	0.15	0.25
Zygomatic	B	0.72 ± 0.37	0.17 ± 0.55	0.79 ± 0.41	0.68 ± 0.43	0.53 ± 0.72	0.16 ± 0.51	1	0.73 ± 0.56	−1	0.23 ± 0.48	0.82 ± 0.28	0.87 ± 0.63	−0.22 ± 0.60	0.41 ± 0.54		0.34	0.34
	H	0.19 ± 0.33	0.82 ± 0.19	0.03 ± 0.31	0.49 ± 0.29	−0.42 ± 0.52	0.12 ± 0.30	0.27 ± 0.32	−0.04 ± 0.31	1	0.90 ± 0.17	0.34 ± 0.28	0.12 ± 0.41	1	−0.28 ± 0.34	0.02 ± 0.66		0.34
	L	0.61 ± 0.24	0.30 ± 0.32	0.49 ± 0.25	0.51 ± 0.31	0.03 ± 0.52	0.70 ± 0.27	0.78 ± 0.22	0.47 ± 0.26	1	0.27 ± 0.30	0.77 ± 0.15	−0.11 ± 0.50	−0.60 ± 0.40	0.30 ± 0.34	0.14 ± 0.66	−0.40 ± 0.48