Excitation–contraction coupling gain in ventricular myocytes: insights from a parsimonious model

Abstract

We present a minimal mathematical model of Ca²⁺ spark triggering under voltage-clamp conditions in ventricular myocytes. The model predicts changes in excitation–contraction coupling ‘gain’ that result from diverse experimental interventions. We compare model results to several sets of data, and, in so doing, place apparent constraints on physiologically relevant model parameters. Specifically, the analysis suggests that many L-type Ca²⁺ channel openings can potentially trigger each Ca²⁺ spark, but the probability that an individual opening will trigger a spark is low. This procedure helps to reconcile contradictory results obtained in recent studies; moreover, this new model should be a useful tool for understanding changes in gain that occur physiologically and in disease.

In cardiac myocytes, the central event linking excitation to contraction is Ca²⁺-induced Ca²⁺ release (CICR), whereby Ca²⁺ crossing the cell membrane triggers the release of a larger quantity of Ca²⁺ from the sarcoplasmic reticulum (SR). The primary trigger for CICR is L-type Ca²⁺ current (I_Ca), and release occurs through SR Ca²⁺ release channels known as ryanodine receptors (RyRs). The cellular SR Ca²⁺ release flux results from the triggering of thousands of individual units, Ca²⁺ sparks (Cheng et al. 1993), each of which reflects the activation of a cluster of RyRs (Bers, 2002; Guatimosim et al. 2002). In whole-cell voltage-clamp experiments examining CICR, a measure frequently derived is excitation–contraction (EC) coupling ‘gain’, usually computed as the peak SR Ca²⁺ release flux divided by the peak flux of Ca²⁺ across the cell membrane (Wier et al. 1994; Shannon et al. 2000; Song et al. 2001). When these are expressed in equivalent units, EC coupling gain quantifies the amplification provided by CICR. In addition, the dependence of gain on membrane voltage (V_m) supplies important information. For instance, the decline in gain seen with an increase in V_m (e.g. from −20 to +20 mV) provides evidence of the ‘local control’ of CICR in heart cells (Wier et al. 1994). In addition, changes in gain with pathology or under different experimental conditions have been used to make inferences about the microscopic structures responsible for Ca²⁺ sparks (Gomez et al. 1997; Altamirano & Bers, 2007).

Some investigations of cardiac EC coupling gain seem in conflict, however. For instance, whereas early studies indicated that individual openings of L-type Ca²⁺ channels (LTCCs) can trigger Ca²⁺ sparks (Cannell et al. 1995; Lopez-Lopez et al. 1995; Santana et al. 1996; Collier et al. 1999), more recent investigations have emphasized that each RyR cluster is associated with multiple LTCCs (Inoue & Bridge, 2003; Altamirano & Bers, 2007; Polakova et al. 2008). Moreover, two recent papers have reached opposite conclusions regarding ‘coupling fidelity’, the probability that an individual LTCC opening will trigger a Ca²⁺ spark. Whereas Altamirano and Bers suggested that the coupling fidelity is relatively high at 0 mV (Altamirano & Bers, 2007), Polokova et al. used a different method of analysis to reach the opposite conclusion, that coupling fidelity is low (Polakova et al. 2008). The lack of a general framework for interpreting the V_m dependence of gain makes these divergent viewpoints difficult to reconcile.

Here we address this shortcoming in our understanding by presenting a minimal model of Ca²⁺ spark triggering under voltage-clamp conditions. This algebraic model, which is a generalization of previous calculations (Santana et al. 1996; Cannell & Soeller, 1999; Wier, 2007), can be used to understand changes in gain seen with experimental interventions. The model's simplicity allowed us to explore the parameter space thoroughly and to determine how changes in variables made the model predictions either consistent with, or inconsistent with, diverse sets of experimental data. The comparison to data identified a limited number of parameter sets that produced output simultaneously consistent with all experimental results, and this allowed us to infer probable constraints on physiologically important parameters. The analysis suggests that the number of LTCC openings that can trigger each Ca²⁺ spark is large, but the probability that an individual channel opening will trigger a spark is low.

Methods

To provide insight into the V_m dependence of EC coupling gain, we present a minimal model of the activation of a cluster of RyRs. The RyR cluster is associated with several LTCCs, and Ca²⁺ current through any of these channels can trigger a Ca²⁺ spark from the cluster. We assume that LTCCs contribute independently to spark triggering such that the probability a spark is triggered is the complement of the probability that all LTCCs fail to trigger. We further assume that (1) each LTCC opens either one or zero times during the voltage-clamp step; (2) all LTCCs that open do so at the same time; (3) LTCC opening duration is constant and independent of V_m; and (4) all RyR clusters in the cell have identical characteristics.

With these assumptions, only three relationships are required to predict the cellular SR Ca²⁺ release flux, J_release, in response to a voltage-clamp depolarization. These are: (1) the probability, as a function of V_m, that a given LTCC will open during the voltage step (P_openingvs. V_m); (2) single-channel current vs. voltage (i_Cavs. V_m); and (3) the probability that a single-channel current of a given magnitude will trigger a Ca²⁺ spark (P_trigvs. i_Ca). The first two relationships can be measured directly and are therefore constrained by data. We assume that (1) P_openingvs. V_m obeys a Boltzmann equation, with parameters taken from a model of the rat ventricular myocyte (Pandit et al. 2001), and (2) i_Ca depends linearly on V_m, with single-channel conductance 2.5 pS (at 1 mmol l⁻¹ external [Ca²⁺]) and reversal potential +60 mV (Guia et al. 2001) (Fig. 1A). We note that the variable P_opening used in these calculations differs from the ‘open probability’ that is measured in studies of ion channel gating. The latter refers to the time-averaged probability that a channel is open whereas the former gives the probability that an LTCC will ever open during a voltage-clamp depolarization. However, if the probability of multiple LTCC openings is low, these quantities will be approximately proportional and have the same dependence on V_m. Since, under typical experimental conditions, activation of I_Ca and triggering of Ca²⁺ sparks occurs during the first few milliseconds of a voltage step (Cannell et al. 1995), this is a reasonable assumption for computations of EC coupling gain. Given these assumptions, peak I_Ca at a given V_m is proportional to N×P_opening×i_Ca, where N is the number of LTCCs.

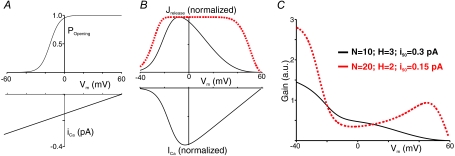
Figure 1. Example EC coupling gain computations.

A, the probability that an LTCC will open (P_opening) and single channel LTCC current (i_Ca) as a function of membrane potential (V_m). These experimentally measured relationships are kept fixed in the simulations. B, normalized SR Ca²⁺ release flux (J_release) and whole-cell LTCC current (I_Ca) vs. V_m calculated with two sets of parameters. C, EC coupling gain calculated from the two sets of curves in B.

The third relationship, P_trigvs. i_Ca, called the ‘coupling fidelity’ in other studies (Wang et al. 2001; Altamirano & Bers, 2007; Polakova et al. 2008), is not known precisely. However, it stands to reason that P_trig will be zero at i_Ca= 0 and will reach a saturating level at large values of i_Ca. Since a Hill function can describe such a relationship with two parameters, we assume that P_trig=i_Ca^H/(i_Ca^H+i₅₀^H), where H is the Hill exponent and i₅₀ sets the sensitivity of the RyR cluster. Since all RyR clusters are identical, J_release is proportional to the probability, P_spark, of triggering a spark from a single cluster. The probability that a given LTCC triggers a spark is the product P_opening×P_trig, and (1 −P_opening×P_trig) defines the probability that it does not. To compute the probability of release from the cluster, we subtract from unity the probability that all LTCCs fail to trigger.

A V_m-dependent quantity proportional to EC coupling gain can then be computed as the ratio P_spark/I_Ca. Because the voltage dependences of P_opening and i_Ca are considered fixed, the model has only three free parameters: N, i₅₀ and H.

Results and Discussion

Figure 1B and C show I_Ca, J_release and EC coupling gain as a function of V_m computed using two sets of parameters. With N= 10, i₅₀= 0.3 pA, H= 3 (continuous line), gain declines monotonically between −40 and +60 mV. With N= 20, i₅₀= 0.15, H= 2, however, gain declines, increases, then declines again as V_m increases (dashed line). Based on a qualitative comparison with experimental data (Wier et al. 1994; Song et al. 2001; Altamirano & Bers, 2007) the former results would be considered provisionally realistic whereas the latter would be deemed clearly unrealistic.

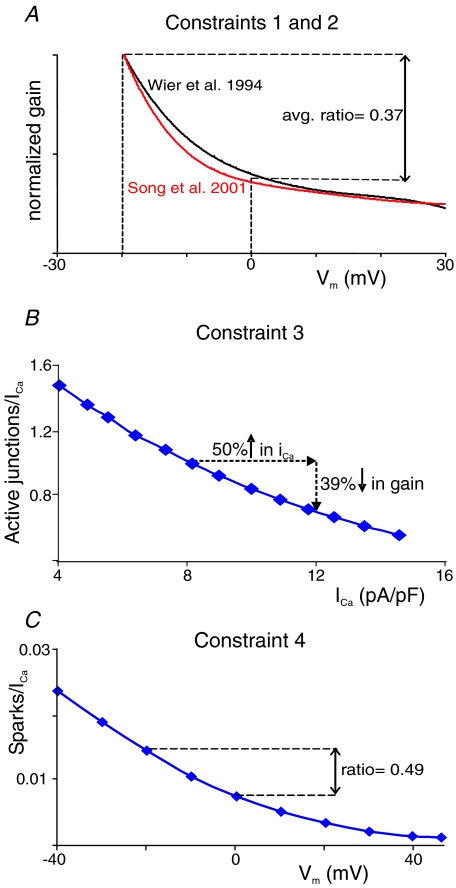
We sought to make the comparison of model output to experimental results more systematic and quantitative. To that end, we selected four characteristics of gain that have been measured in rat ventricular myocytes (Fig. 2). First, several studies have observed a monotonic decrease in gain as V_m is increased from −20 to approximately +20 mV (Wier et al. 1994; Song et al. 2001; Altamirano & Bers, 2007). At more positive V_m, gain can appear to increase, particularly if Na⁺ is present in the pipette (Litwin et al. 1998) and reverse-mode Na⁺–Ca²⁺ exchange can augment the trigger (Sobie et al. 2008). Over the narrower range considered, however, a decrease in gain is consistently observed. Second, two studies that have examined gain quantitatively have indicated that the ratio of gain at 0 mV to gain at −20 mV is approximately 0.37 (Wier et al. 1994; Song et al. 2001). We therefore considered model-produced gain ratios between 0.27 and 0.47 to be realistic (Fig. 2A). Third, Altamirano & Bers showed that when external [Ca²⁺] is increased from 1 mm to 3 mm, single channel i_Ca increases by roughly 50%, and gain measured at 0 mV decreases by 40% (Altamirano & Bers, 2007). We simulated this experiment and considered 30–50% decreases in gain to be consistent (Fig. 2B). Fourth, a few studies have blocked I_Ca by approximately 95% with ∼1 μm nifedipine and quantified gain as the number of Ca²⁺ sparks divided by peak or integrated I_Ca (Santana et al. 1996; Gomez et al. 1997). When measured in this manner, gain also decreases monotonically with an increase in V_m, but the shape of the curve changes in subtle ways. Specifically, the gain curve is somewhat ‘flatter’ such that the 0 mV to −20 mV ratio is larger than when measured under control conditions. We simulated this experiment by reducing N by 95%, and considered ratios between 0.41 and 0.61 to be realistic (Fig. 2C).

Figure 2. Illustration of experimental data used to place constraints on the model parameters.

A, gain functions published by Wier et al. (1994) and Song et al. (2001) normalized to gain at −20 mV and plotted on the same scale. These curves illustrate the first two constraints, that: (1) gain must decrease monotonically from −20 to +20 mV, and (2) the ratio of gain at 0 mV to gain at −20 mV should be between 0.27 and 0.47. B, re-plotted experimental data from Altamirano & Bers (2007) illustrate constraint 3, that a 50% increase in i_Ca, achieved by altering extracellular [Ca²⁺], leads to a decrease in gain at 0 mV. The data indicate a 39% decrease in gain; we consider 30–50% decreases to be realistic. C, re-plotted experimental data from Santana et al. (1996) illustrate constraint 4, that blocking 95% of LTCCs (1 μm nifedipine) alters the ratio of gain at 0 mV to gain at −20 mV. As a ratio of 0.49 was observed, we considered ratios between 0.41 and 0.61 to be acceptable.

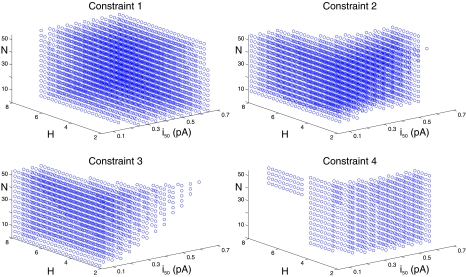
We systematically varied the model's three free parameters over a wide range. For each parameter set, we determined whether the model output was consistent with each of the four experimental constraints. As shown in Fig. 3, a wide variety of parameter values could produce model results consistent with any individual constraint: of the 4550 parameter combinations tested, 2974, 2560, 1478 and 794 were consistent with constraints 1, 2, 3 and 4, respectively. However, the consistent sets of parameters corresponding to the four constraints occupied different regions of parameter space. For instance, low values of i₅₀ tended to yield results consistent with constraint 3, regardless of H and N, whereas small values of H and larger values of i₅₀ made the model more likely to satisfy constraint 4. As a result of this, only 7 of 4550 combinations (< 0.2%) produced model output that was simultaneously consistent with all four constraints. We refer to these hereafter as the ‘fully consistent’ parameter sets.

Figure 3. MOdel parameter analysis.

Locations, in H, i₅₀, N space, of parameter sets that generate model output consistent with each of the four constraints.

The fully consistent parameter sets (see Table 1) share the following characteristics: (1) N is between 17 and 53; (2) H is between 4 and 5.75; (3) i₅₀ is between 0.2 and 0.3 pA. These characteristics indicate that for our simple model to recapitulate several sets of data, the number of LTCCs that can trigger each Ca²⁺ spark must be large, the relationship between P_trig and i_Ca must be steep, and the sensitivity of the RyR cluster must be relatively low. The seven i₅₀ values between 0.2 and 0.3 pA correspond to i_Ca at V_m between −20 and −60 mV. Thus, in the seven fully consistent parameter sets, the coupling fidelity is less than 50% at any V_m positive to −20 mV.

Table 1.

Model parameters of the seven ‘fully consistent’ parameter sets

i50	H	N	Coupling fidelity at 0 mV
0.20	5	17	0.192
0.20	5.25	17	0.181
0.20	5.5	21	0.170
0.20	5.75	21	0.161
0.25	4.5	37	0.091
0.25	4.75	41	0.081
0.30	4	53	0.059

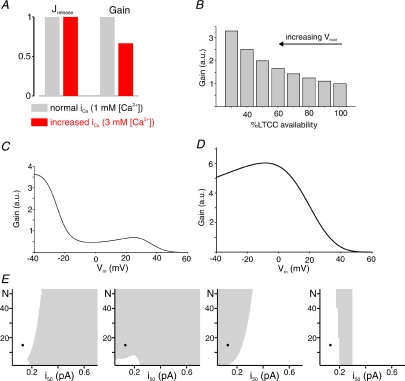
Our inferences about RyR cluster sensitivity and LTCC triggering of sparks are consistent with some prior conclusions but inconsistent with others. For instance, two recent studies used different analyses to conclude, as we have, that many LTCC openings can potentially trigger each Ca²⁺ spark (Altamirano & Bers, 2007; Polakova et al. 2008). These conclusions confirmed results previously obtained in myocytes from rabbit (Inoue & Bridge, 2003), a species in which, based on measurements of LTCC and RyR protein levels (Bers & Stiffel, 1993), we may expect N to be even larger. Regarding the coupling fidelity, however, our results conflict with the conclusions of Altamirano & Bers (2007), who inferred from their data that ‘the amplitude of i_Ca at 0 mV… may be sufficient to effectively trigger uniform SR Ca²⁺ release.’ For the fully consistent parameter sets chosen through comparison with experimental data, the coupling fidelity at 0 mV ranges from 5.88% to 19.2%. This is consistent with the analyses of Wier (2007) and Polokova et al. (2008), who showed that a system with low coupling fidelity can produce results similar to those observed by Altamirano & Bers (2007). Our analysis goes further, however, by predicting that a high coupling fidelity at 0 mV will cause behaviour that is inconsistent with data. Figure 4 shows an example of such unrealistic model behaviour when the coupling fidelity is high (0.66 at 0 mV). These results were generated with N= 13, H= 4.5 and i₅₀= 0.13. With these parameters, the model does indeed reproduce the experimental observations that: (1) an increase in i_Ca decreases gain at 0 mV (Fig. 4A), and (2) a decrease in channel availability (N) increases gain at 0 mV (Fig. 4B). However, these parameters lead to a non-monotonic gain curve that increases between −3 and +25 mV, inconsistent with experimental observations (Fig. 4C). Moreover, when 95% of LTCCs are blocked, simulating 1 μm nifedipine, the ratio of gain at 0 mV to gain at −20 mV is greater than unity (Fig. 4D), much different from the ratio of roughly 0.5 that has been measured in experiments. Figure 4E shows more systematically that if parameters are chosen such that the coupling fidelity at 0 mV is large (i.e. i₅₀ < 0.2), at least one experimental constraint is always violated. Our simulations therefore support the hypothesis that coupling fidelity in rat ventricular myocytes is low at 0 mV (and above), but multiple LTCC openings ensure that a large percentage of Ca²⁺ spark sites are triggered with each beat.

Figure 4. High coupling fidelity at 0 mV generates unrealistic model behaviour.

Model results obtained with H= 4.5, i₅₀= 0.13 and N= 13 show that, if the coupling fidelity at 0 mV is large (0.66 in this case), at least one model constraint will be violated. A, increasing i_Ca by 50%, simulating a change in external [Ca²⁺] from 1 to 3 mm, causes little increase in J_release at 0 mV and a ∼30% decrease in gain. B, decreasing channel availability (N) by increasing the holding potential causes an increase in gain at 0 mV. These two results are consistent with data obtained in analogous experiments by Altamirano & Bers (2007). C, these parameters, however, lead to a gain curve that increases between −3 and +25 mV. D, when 95% of LTCCs are blocked, simulating application of 1 μM nifedipine, gain at 0 mV is nearly identical to gain at −20 mV. These latter two predictions are inconsistent with experimental results. E, more systematic examination of which parameter combinations are consistent with (grey), or inconsistent with (white), the four constraints. All plots generated with H= 4.5; values from 3 to 5.5 show qualitatively similar behaviour. Models with a high coupling fidelity at 0 mV (i₅₀ < 0.15) tend to be consistent with constraints 2 and 3, but inconsistent with constraints 1 and 4. Black circles indicate the parameters used to generate results in A–D.

The analysis illustrates the strength of the approach we have taken. The model's simplicity allowed us to explore the parameter space thoroughly and compare the predictions to several sets of experimental data. In future work, constraints can be added, removed, or modified quantitatively as additional data become available. This simple model, however, has several limitations that should be considered. One is the assumed relationship between i_Ca and coupling fidelity. For the sake of convenience, we represented this with a Hill function, but this simple equation may not capture the full complexity of the spark-triggering process. Simulations employing a more detailed, stochastic model of Ca²⁺ spark dynamics (e.g. Stern et al. 1999; Sobie et al. 2002) can address the adequacy of this assumption. Another potentially important limitation is the model's lack of time dependence. Unique values, representing peak I_Ca and peak J_release, are computed at each V_m, and the model does not consider phenomena such as variable latencies to LTCC openings, re-openings of LTCCs, Ca²⁺-dependent LTCC inactivation, or depletion of SR [Ca²⁺]. Because EC coupling gain is computed based on the maximal rate of SR Ca²⁺ release, and most Ca²⁺ sparks are triggered during the first few milliseconds of a voltage step (Cannell et al. 1995), we do not expect these limitations to alter the study's main conclusions. Time-dependent phenomena such as latencies to LTCC opening may need to be considered, however, to capture aspects of gain seen at very positive V_m, where delays to spark triggering are more variable. These limitations would also need to be addressed to simulate the decreased synchrony of spark triggering observed in disease states (Litwin et al. 2000; Song et al. 2006). Future studies will investigate the balance between model simplicity and mechanistic detail.

In conclusion, we have presented a minimal model of Ca²⁺ spark triggering by openings of associated LTCCs. Altering the model's three free parameters can cause the model predictions to be either consistent with or grossly at odds with experimental data. A quantitative comparison with several independent sets of results suggests that many LTCCs are associated with each RyR cluster in rat ventricular myocytes, and, since the probability of an individual opening activating a spark is low, multiple LTCC openings are required to ensure a ‘safety factor’ for Ca²⁺ spark triggering. More generally, the model can serve as a valuable tool for interpreting experiments that measure gain. Constraints and parameters can be varied as needed for quantitative examinations of EC coupling in different species, after physiological perturbations such as β-adrenergic stimulation (Song et al. 2001), in transgenic animals that show altered LTCC or RyR function, or in disease states such as heart failure, where many factors are altered (Gomez et al. 1997; Song et al. 2006).