Abstract
A combination of abiotic and biotic factors probably restricts the range of many species. Recent evolutionary models and tests of those models have asked how a gradual change in environmental conditions can set the range limit, with a prominent idea being that gene flow disrupts local adaptation. We investigate how biotic factors, explicitly competition for limited resources, result in evolutionarily stable range limits even in the absence of the disruptive effect of gene flow. We model two competing species occupying different segments of the resource spectrum. If one segment of the resource spectrum declines across space, a species that specializes on that segment can be driven to extinction, even though in the absence of competition it would evolve to exploit other abundant resources and so be saved. The result is that a species range limit is set in both evolutionary and ecological time, as the resources associated with its niche decline. Factors promoting this outcome include: (i) inherent gaps in the resource distribution, (ii) relatively high fitness of the species when in its own niche, and low fitness in the alternative niche, even when resource abundances are similar in each niche, (iii) strong interspecific competition, and (iv) asymmetric interspecific competition. We suggest that these features are likely to be common in multispecies communities, thereby setting evolutionarily stable range limits.
Keywords: adaptive surface, fitness surface, interspecific competition, Lotka–Volterra, niche, species borders
1. Introduction
Some species borders are set because the change in the environment is so dramatic that all individuals fail to survive and/or reproduce beyond the border, e.g. at a coastline. However, many range limits do not appear to be set in this way, because environmental conditions across the limit change more gradually. In this case, we need to ask why adaptation in populations at the range edge does not result in an increase in population size, hence range expansion (Mayr 1954; Haldane 1956; Kirkpatrick & Barton 1997). Two explanations currently predominate (Hoffmann & Blows 1994; Hoffmann & Kellermann 2006; Bridle & Vines 2007; Bridle et al. 2008). In the first, adaptation is prevented because relevant genetic variation is absent (Blows & Hoffmann 2005; Kellermann et al. 2006). The absence of genetic variation may apply in some places at some times, but it is unlikely to be general, for the simple reason that related species (often congeners) extend beyond the focal species' range limit. This implies that, given the right sequence of environments, selection can produce phenotypes adapted to conditions where the species is not currently found. In the second explanation, immigration of individuals adapted to the conditions at the centre of the range prevents adaptation to the different conditions at the species border (Mayr 1954; Haldane 1956; Kirkpatrick & Barton 1997). This model is receiving increasing theoretical attention (Case & Taper 2000; Goldberg & Lande 2006; Filin et al. 2008) and has provided the stimulus for much current empirical research (e.g. Angert & Schemske 2005; Bridle et al. 2009). It is not clear, however, how often in nature the immigration of maladapted genotypes occurs at a rate sufficient to disrupt adaptation (Case & Taper 2000; Bridle & Vines 2007). Under some conditions, immigration can actually facilitate adaptation (Barton 2001; Bridle et al. 2009).
In this paper, we consider a third, and in many ways simpler, explanation for evolutionarily stable range limits. It relies on biotic interactions, notably competition. Theoretical work has shown that competition can set range limits in many ways (reviewed by Case et al. 2005). For example, given a pair of species in which the intensity of interspecific competition is similar to that of intraspecific competition, the species with the higher carrying capacity will exclude the other: a range limit is set across a varying environment, where the ranking of the carrying capacities changes (MacLean & Holt 1979). Purely ecological models of this situation do not consider the possibility that a species might evolve to escape the constraining influences of the other, leaving unresolved the issue about whether these types of range limits can be evolutionarily stable. In this paper, we show that species borders set by interspecific competition are often stable in both ecological and evolutionary time, even if all relevant traits remain heritable, and gene flow has no influence on adaptation.
By way of example, consider that a finch's beak size correlates with the size of the seed it can most efficiently harvest (Schluter & Grant 1984). Suppose that a small finch species exploits a small seed and a large finch species exploits a large seed. If the density of the small seed declines along a geographic transect, but the large seed remains abundant everywhere, the small finch species will correspondingly decline, but the large finch species remain common. As small seeds become rare and in the absence of the other species, the small finches should come under directional selection and evolve to efficiently use large seeds; the population can then persist by exploiting the alternative niche and there is no range limit. When the large finch is already exploiting the large seeds, however, individuals of the smaller finch species may be at a severe competitive disadvantage on those seeds. Consequently, the small finch remains under stabilizing selection to eat small seeds, and is driven to extinction when those seeds become sufficiently rare. Patterns approximating these scenarios have been observed in the population of medium ground finches, Geospiza fortis on I. Daphne Major, Galápagos (Grant & Grant 2006). Over a drought in 2003–2004, the population crashed and natural selection favoured small body size, attributed to the presence of a population of large ground finches, Geospiza magnirostris, which monopolized the larger seeds (Grant & Grant 2006). Across an earlier drought (1977), the population of medium ground finches declined, but the large ground finch was not on the island, and larger individuals persisted as a result of exploiting the large seeds, resulting in a selection favouring large body size (Boag & Grant 1981).
In extreme cases, it is easy to see how an evolutionarily stable limit can be set by a lack of resources, even in the absence of competition. Continuing with the finch–seed example, it may be impossible for small finches to consume large seeds, because no individuals in the population are able to crack them. Then, as the small seed disappears, despite the presence of other resources, the small finch species is trapped and disappears too. This is an example where a species is simply unable to use alternative niches in the environment. A similar situation occurs when a few extreme individuals are able to use the alternative resource, but somewhat less extreme individuals are at a strong fitness disadvantage. If the valley in the individual fitness function is sufficiently large, again the population will not evolve to the neighbouring higher fitness peak (Kirkpatrick 1982). While these scenarios are possible, it seems more likely that the distribution of resources does not have impossibly deep valleys, and so when one part of the resource spectrum declines, a consumer species will evolve to use another part of the spectrum if there are no competing species. Our question specifically focuses on the outcome when competitors are present. We show that competitors set evolutionarily stable range limits even in quite non-intuitive cases where a species would rapidly and easily evolve to exploit alternative resources in the absence of the competitor.
Previous models of evolutionarily stable range limits have focused on the role of gene flow disrupting adaptation. These models explicitly considered the movement of individuals between locations (Kirkpatrick & Barton 1997; Case & Taper 2000). Without movement, a range could not extend (no individuals would ever appear beyond the current limit) and gene flow could not prevent adaptation at the limit. In this paper, we considerably simplify the analysis by not explicitly modelling movement. This is justified, because our goal is to show that at a range limit, a species remains under stabilizing selection to efficiently exploit resources in its own niche even as that niche disappears, rather than being placed under directional selection to exploit resources available in another species niche. If the niche disappears, colonists exploiting that niche cannot persist, and if stabilizing selection is present, colonist populations at the range limit cannot be rescued by adaptation.
2. Model and results
Following Case & Taper (2000), we build a Lotka–Volterra model of two species competing in continuous time. The intrinsic growth rate for an individual of species i with phenotype z at time t is
| (2.1) |
The first of the three terms on the right is the maximum growth rate in the absence of competition: reflects the resources available in the environment to individuals with trait value z. The second term represents intraspecific competition and the third term interspecific competition. In those terms, cij(z,z′) is the effect of an individual of species j with phenotype z′ on the growth rate of individuals of species i with phenotype z, and ni(z′,t) is the number of individuals of species i with phenotype z′ at time t.
We assume that the evolutionary change in the trait mean is described by the classic ‘breeder's equation’ of quantitative genetics (Falconer & MacKay 1996). Then, following the argument of Case & Taper (2000, p. 585, with a typographical error corrected and terms rearranged), the rate of evolutionary change in the trait mean of species i is
| (2.2) |
Here is the heritability of the trait in species i and pi(z,t) is the frequency of phenotype z. The rate of change in the total number of individuals of species i is
| (2.3) |
where is the mean intrinsic growth rate. Equations (2.2) and (2.3) give the evolutionary and demographic dynamics of the model.
To complete the model's description, we need to specify the phenotypic distributions pi(), the competition functions cij(), and the maximum growth rate rmax(), which describes the individual fitness function in the absence of intra- or interspecific competitions. We assume that the phenotypic distribution is normal, and denote its variance in species i as . The heritability and phenotypic variance are constant in time. Regarding the competition function, we assume the Gaussian kernel used by Slatkin (1980) and others
| (2.4) |
where aij and bij measure the intensity of competition between phenotypes. In particular, large values of aij imply the maximum amount of interspecific competition is intense, while large values of bij mean that competitive effects decrease rapidly as phenotypes become more different (Slatkin 1980). Written in this way, the effects of inter- and intraspecific competition can differ, and the effects of species i on species j can differ from the effects of j on i.
The final assumption regards the form of the maximum growth rate . To capture situations in which the environment has two intrinsic niches, we consider bimodal functions of the form
| (2.5) |
This form can be visualized in terms of the (discrete time) fitness function, which is simply the exponential of r. Then (2.5) becomes the sum of two Gaussian functions whose maxima are at z=±θ, heights are H1 and H2, and widths (variances) are 1/(2D). The fitness function has two modes when H1 and H2 are not too different, and θ is sufficiently large relative to D; otherwise the function is unimodal (Kirkpatrick 1982). We explore situations where the fitness function is bimodal, as in figure 1, as well as the case where the fitness function is unimodal, which is the model considered in the previous analyses of character displacement (Slatkin 1980; Case & Taper 2000; Goldberg & Lande 2006).
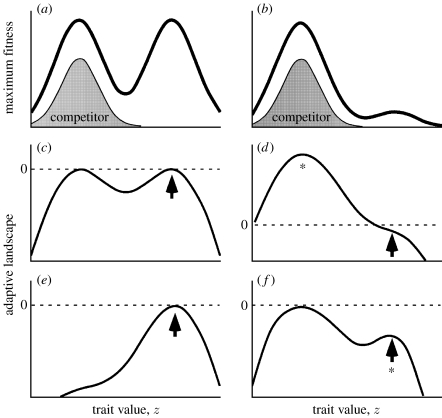
Figure 1.
(a,b) Maximum individual fitness plotted as a function of the trait value z, reflecting the resource distribution in the environment. Resources associated with the right peak are (a) abundant at the range centre and (b) very low at the range edge. Fitness is calculated as w(z)=exp{rmax(z)}. The shaded area shows the phenotypic distribution of the competing species at its evolutionary equilibrium. (c–f) Adaptive landscapes for the focal species (i.e. the one occupying the right peak). Dashed lines show zero population growth. (c,e) Adaptive landscapes at the range centre for the focal species at demographic and evolutionary equilibrium, when the competitor is (c) absent and (e) present. The curves were calculated from equations (2.1), (2.4) and (2.5) by averaging over the phenotypic distribution for a given value of the trait mean, . Arrows indicate the equilibrium mean trait value. (d,f) Adaptive landscapes at the range edge for the focal species when it is at low population density. Arrows indicate the mean phenotype of the focal species when it is first introduced to this habitat, and asterisks the mean phenotype at the evolutionary equilibrium. Parameters are a11=a22=a12=a21=1.0 (for simplicity, we set interspecific competition equal to intraspecific competition), b11=b22=b12=b21=0.05, D=0.15, θ=5 and ==4. H1=H2=10 (a,c,e) and H1=10, H2=1 (b,d,f).
In figure 1, we show example fitness functions for a bimodal case. Figure 1a,b illustrates the maximum fitness of an individual of a given phenotype, in the absence of any inter- and intraspecific competition, which could be considered a measure of the abundance of resources limited by some factor not in the model. Figure 1c–f describes ‘adaptive landscapes’ for the species that occupies the right-hand peak. The adaptive landscape is a plot of the log of the population's mean fitness as a function of the trait mean (note the distinction from the individual fitness function, given by equation (2.5)). Here, the landscape is constructed as the mean intrinsic growth rate . In the absence of frequency dependence, the adaptive landscape indicates the positions of equilibria and directions of evolution towards those equilibria (Lande 1976), and this is true also when the frequency dependence results from symmetrical intraspecific competition (Lande 1976; Case & Taper 2000), as in this case.
Figure 1c,e shows the landscape experienced by the focal species when it is at an evolutionary and demographic equilibria at the range centre. The mean population growth rate is =0 (i.e. =1), and the mean phenotype lies at a peak in the adaptive landscape. With or without the competitor, the focal species persists because it is exploiting an abundant resource. The species experiences a different situation at its range edge. Figure 1d,f shows the adaptive landscape when the focal species is rare, corresponding to a small propagule arriving from the range centre. Now the mean population growth rate is negative because its resource is so sparse, and population size starts to decline. Whether or not it can be rescued depends on whether the population can escape evolutionarily to the left-hand peak. That occurs when the competitor is absent (figure 1d), but not when it is present (figure 1f).
Similar results apply under the less intuitive case when intrinsic resource distributions have a single peak so that, in the absence of any competition, intermediates would have high fitness. An example is illustrated in figure 2. We constructed a unimodal function for rmax by broadening the width of the two Gaussian curves in equation (2.5). We set resources at the upper end of the distribution to decline towards the edge of the focal species range (by decreasing the value of H2 in equation (2.5)). In this case, it is clear that in the absence of a competitor, a single species would persist everywhere with a mean phenotype near the maximum in the fitness function.
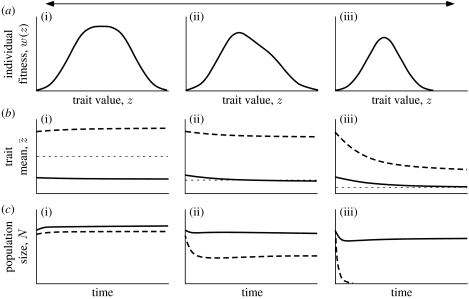
Figure 2.
Example of a stable range limit when the fitness function set by resources is unimodal. (a(i)–(iii)) Maximum individual fitness functions in the absence of inter- and intraspecific competitions (same as figure 1a,b). Trajectories of the population mean phenotypes (b(i)–(iii)) and population sizes (c(i)–(iii)) when environmental conditions are abruptly changed from those at the range centre to the alternatives assuming populations are initially close to the equilibrium expected at the range centre. The focal species, shown by the dashed curves, persists at the range centre (i) but goes extinct at the range edge (iii). The competitively dominant species, shown by the solid curves, persists everywhere. Dotted horizontal lines in (b) show the equilibrium for the trait mean if only one species is present. Computed using equations (2.1)–(2.5) in the text. Parameters are: a11=a22=1, a12=0.7, a21=1.1 (i.e. species 1 is the superior competitor that persists at the range limit of species 2), b11=b22=b12=b21=0.1, ==4, ==0.5, D=0.02, θ=5, H1=H2=2 (i); H1=2, H2=1.2 (ii); H1=2, H2=0 (iii).
In the presence of a competitor species, a second, focal, species exploiting the upper end of the resource distribution can persist wherever its favoured resources are abundant, but it reaches an evolutionarily stable range limit as these resources decline. To show this, we illustrate the dynamics for the mean phenotypes and the population sizes for both species as they evolve towards the equilibria imposed by the different environmental conditions (figure 2b,c). We set population sizes and mean phenotypes close to the equilibrial values expected at the range centre, and then considered how they changed when placed in alternative environments. In this example, we also set the focal species to be an inferior competitor.
At the range centre both species coexist, where they use different parts of the resource spectrum. As resources decline, the superior competitor comes to use the abundant resources represented by the peak of the resource abundance (rmax) curve. The focal species can still persist, albeit at low population size, by exploiting the scanty resources far to the right (figure 2(ii)). With an even lower resource base, however, it goes extinct (figure 2(iii)). Note that the mean trait value of the inferior competitor is not under directional selection at the range limit, but rather under stabilizing selection towards an equilibrial value set by interspecific competition from the numerically dominant species to the left, and an intrinsic decline in available resources to the right. Thus the range limit is evolutionarily stable. We verified these conclusions by integrating equations (2.2) and (2.3) for the cases shown in both figures 1 and 2.
We have not extensively explored the conditions under which populations using a vanishing resource can be rescued by selection. However, several factors make population extinction more likely. First, any change in the intrinsic resource distribution that leads to the presence of a deeper valley in the individual fitness function makes it more difficult for a species to escape evolutionarily to a neighbouring resource peak. Second, the range of parameters over which selection can rescue the population is reduced as interspecific competition increases. Interspecific competition may result from direct aggression of the dominant species over the subordinate, or from more efficient resource depletion by one or both species. Third, ongoing adaptation of the species to the niche it occupies may lead to a decline in individual fitness in the alternative (currently unexploited) niche, again making a transition less likely and extinction more likely. Some examples are considered in the discussion. Fourth, increased adaptation to one's own niche can lead to trade-offs intraspecifically: adaptation to resources at the centre of the distribution, where most of the population is, can lower fitness of phenotypes at the edge (Holt & Gaines 1992). This will deepen the valley in the fitness function and again broaden the range of parameters over which extinction occurs.
3. Discussion
Competitive exclusion is thought to be a common mechanism setting range limits (Case & Taper 2000; Case et al. 2005). Although abiotic factors have been assumed to be important in setting range limits, particularly at high latitudes or altitudes (Darwin 1859; MacArthur 1972; Case et al. 2005), it seems likely that the competition is generally involved in these directions too (Darwin 1859; Case et al. 2005). For example, Darwin (1859, p. 121) noted: ‘in so far as climate chiefly acts to reduce food, it brings on the most severe struggle between individuals’.
Here we have shown that range limits set by competition can be stable in evolutionary time, without the need to invoke the disruptive effect of gene flow. The specific example we have considered is that of two species competing for limiting resources. When one species exploits a rare resource, that species is often not under selection to exploit the resources that are already being used by a competitor. Instead, those individuals of the rare species that most efficiently exploit their own resource have higher fitness than other members of their species. The result is that as a rare species' resources decline to unsustainable levels, the population goes extinct; it cannot be rescued by selection. We find that this result holds for a wide variety of alternative forms for the resource distributions and competitive interactions. It includes many cases where the resource distribution imposes a single peak in the fitness function, so that, in the absence of the competitor, the focal species would readily evolve to use alternative resources if its own become rare (e.g. figure 2). The only escape is a dominant macromutation that makes individuals competitively superior to other species or able to exploit an entirely different kind of resource.
By example, we review studies on a small insectivorous bird, the yellow-browed leaf warbler, Phylloscopus humei, which is one of the most abundant species in northern India in the winter (Gross & Price 2000; Price & Gross 2005). It forages in the crowns of broadleaf trees, and its northern range limit coincides with the loss of leaf from these trees. The ecologically most similar common species at the northern range limit is the related and similar-sized lemon-rumped warbler, Phylloscopus chloronotus, whose range extends beyond that of P. humei. P. chloronotus forages in bushes, which remain evergreen further north. It seems likely that in the absence of P. chloronotus, P. humei could exploit the bush habitat, thereby extending its range north, for it does so in localized areas where P. chloronotus is absent (T. Price, unpublished data, 1994, 1998). Bushes and tree crowns are ecologically different in several ways, including vegetation structure and competitors (Gross & Price 2000) and probably predators and parasites (see Garvin & Remsen (1997) for parasite associations with foraging height in another system), as well as the light environment. The implication is that if species are ecologically segregated to different foraging heights through competition, secondary adaptations to these different heights increase fitness in the occupied habitat, and this will often decrease fitness in the non-occupied habitat. As is clear from our analysis, the result is that population extinction is more likely for the species whose niche disappears. Thus, despite the apparent ability of P. humei to successfully forage in bushes, competition with P. chloronotus could set its range limit, and natural selection would be unable to rescue it. Measurements of fitness surfaces with and without the competitor could potentially be used to test this proposition.
In the above example, a decrease in resource diversity is accompanied by a decrease in species numbers (from two to one). Alternatively, one species replaces an ecologically similar species across space, resulting in parapatric distributions (Case & Taper 2000; Bridle & Vines 2007). The southern range limit of P. humei occurs where food is abundant, but it encounters another species (Phylloscopus trochiloides), which forages in a very similar way and is also found in the tree crowns, but is 40 per cent larger. (Note that these are non-breeding distributions: one common explanation for parapatric distributions of closely related species is that they are set by hybridization between them (Case et al. 2005; Goldberg & Lande 2006), but this cannot apply here.) A reasonable hypothesis is that P. humei is competitively excluded by P. trochiloides, perhaps by aggression, and that P. trochiloides is limited by declining food abundance to the north. P. trochiloides also consumes larger food items than P. humei, even where they co-occur (Gross & Price 2000) and as one moves further north the abundance of large prey appears to decline at a steeper rate than prey abundance in total (Katti & Price 2003). Given these differences, the range limits of both species may well be evolutionarily stable.
Owing to the large number of parameters, the lack of empirical information on the shape of adaptive surface and the restriction of our model to just two species, we have not extensively investigated parameter space. However, we suggest that, particularly among multiply interacting species that have been present in the community for a long time, many range limits remain evolutionarily stable indefinitely. Despite the gradual environmental change across space, range limits set by competition may be more akin to a coastline, with each species simply unable to successfully exploit the other's niche.
We have focused here on cases where range limits are set in space as the resource distribution changes. Clearly, the same processes can also operate in time. It is easy to envision a species adapted to exploiting resources in the alpine zone of mountain tops that is excluded from lower altitudes by the presence of a competing species (Colwell et al. 2008; Moritz et al. 2008). As the alpine zone shrinks and then disappears with a changing climate, the species goes extinct despite the presence of abundant resources in the subalpine vegetation that now occupies the mountaintops. It is prevented from adapting to the environmental change by a competitor that usurps those resources. Thus abundant genetic variation for adaptation to a shifting resource distribution is no guarantee of evolutionary solace from extinction caused by environmental change.
Acknowledgments
We thank Kate Behrman, Nick Barton, Ted Case and Aaron Kandur for their comments. T.D.P. and M.K. acknowledge financial support from the National Science Foundation (USA).
Footnotes
One contribution of 17 to a Special Issue ‘Geographic range limits of species’.
References
- Angert A.L., Schemske D.W. The evolution of species' distributions: reciprocal transplants across the elevation ranges of Mimulus cardinalis and M. lewisii. Evolution. 2005;59:1671–1684. doi:10.1554/05-107.1 [PubMed] [Google Scholar]
- Barton N.H. Adaptation at the edge of a species' range. In: Silvertown J., Antonovics J., editors. Integrating ecology and evolution in a spatial context. Blackwell; New York, NY: 2001. pp. 365–392. [Google Scholar]
- Blows M.W., Hoffmann A.A. A reassessment of genetic limits to evolutionary change. Ecology. 2005;86:1371–1384. doi:10.1890/04-1209 [Google Scholar]
- Boag P.T., Grant P.R. Intense natural selection in a population of Darwin's finches (Geospizinae) in the Galápagos. Science. 1981;214:82–85. doi: 10.1126/science.214.4516.82. doi:10.1126/science.214.4516.82 [DOI] [PubMed] [Google Scholar]
- Bridle J.R., Vines T.H. Limits to evolution at range margins: when and why does adaptation fail? Trends Ecol. Evol. 2007;22:140–147. doi: 10.1016/j.tree.2006.11.002. doi:10.1016/j.tree.2006.11.002 [DOI] [PubMed] [Google Scholar]
- Bridle J.R., Gavaz S., Kennington W.J. Testing limits to adaptation along altitudinal gradients in rainforest Drosophila. Proc. R. Soc. B. 2009;276:1507–1515. doi: 10.1098/rspb.2008.1601. doi:10.1098/rspb.2008.1601 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Bridle, J. R., Polechová, J. & Vines, T. H. 2008 Patterns of biodiversity and limits to adaptation in time and space. In Speciation and patterns of diversity (eds R. K. Butlin, J. R. Bridle & D. Schluter), pp. 77–101. Cambridge, UK: Cambridge University Press.
- Case T.J., Taper M.L. Interspecific competition, environmental gradients, gene flow, and the coevolution of species' borders. Am. Nat. 2000;155:583–605. doi: 10.1086/303351. doi:10.1086/303351 [DOI] [PubMed] [Google Scholar]
- Case T.J., Holt R.D., McPeek M.A., Keitt T.H. The community context of species' borders: ecological and evolutionary perspectives. Oikos. 2005;108:28–46. doi:10.1111/j.0030-1299.2005.13148.x [Google Scholar]
- Colwell R.K., Brehm G., Cardelus C.L., Gilman A.C., Longino J.T. Global warming, elevational range shifts, and lowland biotic attrition in the wet tropics. Science. 2008;322:258–261. doi: 10.1126/science.1162547. doi:10.1126/science.1162547 [DOI] [PubMed] [Google Scholar]
- Darwin C. J. Murray; London, UK: 1859. On the origin of species by means of natural selection. [Google Scholar]
- Falconer D.S., MacKay T.F.C. 4th edn. Longman's Green; Essex, UK: 1996. Introduction to quantitative genetics. [Google Scholar]
- Filin I., Holt R.D., Barfield M. The relation of density regulation to habitat specialization, evolution of a species' range, and the dynamics of biological invasions. Am. Nat. 2008;172:233–247. doi: 10.1086/589459. doi:10.1086/589459 [DOI] [PubMed] [Google Scholar]
- Garvin M.C., Remsen J.V. An alternative hypothesis for heavier parasite loads of brightly colored birds: exposure at the nest. Auk. 1997;114:179–191. [Google Scholar]
- Goldberg E.E., Lande R. Ecological and reproductive character displacement on an environmental gradient. Evolution. 2006;60:1344–1357. doi:10.1554/05-696.1 [PubMed] [Google Scholar]
- Grant P.R., Grant B.R. Evolution of character displacement in Darwin's finches. Science. 2006;313:224–226. doi: 10.1126/science.1128374. doi:10.1126/science.1128374 [DOI] [PubMed] [Google Scholar]
- Gross S.J., Price T.D. Determinants of the northern and southern range limits of a warbler. J. Biogeogr. 2000;27:869–878. doi:10.1046/j.1365-2699.2000.00440.x [Google Scholar]
- Haldane J.B.S. The relation between density regulation and natural selection. Proc. R. Soc. B. 1956;145:306–308. doi: 10.1098/rspb.1956.0039. doi:10.1098/rspb.1956.0039 [DOI] [PubMed] [Google Scholar]
- Hoffmann A.A., Blows M.W. Species borders: ecological and evolutionary perspectives. Trends Ecol. Evol. 1994;9:223–227. doi: 10.1016/0169-5347(94)90248-8. doi:10.1016/0169-5347(94)90248-8 [DOI] [PubMed] [Google Scholar]
- Hoffmann A., Kellermann V. Revisiting heritable variation and limits to species distribution: recent developments. Isr. J. Ecol. Evol. 2006;52:247–261. doi:10.1560/IJEE_52_3-4_247 [Google Scholar]
- Holt R.D., Gaines M.S. Analysis of adaptation in heterogeneous landscapes: implications for the evolution of fundamental niches. Evol. Ecol. 1992;6:433–447. doi:10.1007/BF02270702 [Google Scholar]
- Katti M., Price T.D. Latitudinal trends in body size among over-wintering leaf warblers (genus Phylloscopus) Ecography. 2003;26:69–79. doi:10.1034/j.1600-0587.2003.03264.x [Google Scholar]
- Kellermann V.M., van Heerwaarden B., Hoffmann A.A., Sgro C.M. Very low additive genetic variance and evolutionary potential in multiple populations of two rainforest Drosophila species. Evolution. 2006;60:1104–1108. doi: 10.1554/05-710.1. doi:10.1554/05-710.1 [DOI] [PubMed] [Google Scholar]
- Kirkpatrick M. Quantum evolution and punctuated equilibria in continuous genetic characters. Am. Nat. 1982;119:833–843. doi:10.1086/283958 [Google Scholar]
- Kirkpatrick M., Barton N.H. Evolution of a species' range. Am. Nat. 1997;150:1–23. doi: 10.1086/286054. doi:10.1086/286054 [DOI] [PubMed] [Google Scholar]
- Lande R. Natural selection and random genetic drift in phenotypic evolution. Evolution. 1976;30:314–334. doi: 10.1111/j.1558-5646.1976.tb00911.x. doi:10.2307/2407703 [DOI] [PubMed] [Google Scholar]
- MacArthur R.H. Harper and Row; New York, NY: 1972. Geographical ecology. [Google Scholar]
- MacLean W.P., Holt R.D. Distributional patterns in St. Croix Sphaerodactylus lizards: the taxon cycle in action. Biotropica. 1979;11:189–195. doi:10.2307/2388038 [Google Scholar]
- Mayr E. Change of genetic environment and evolution. In: Huxley J., Hardy A., Ford E., editors. Evolution as a process. Allen & Unwin; London, UK: 1954. pp. 157–180. [Google Scholar]
- Moritz C., Patton J.L., Conroy C.J., Parra J.L., White G.C., Beissinger S.R. Impact of a century of climate change on small-mammal communities in Yosemite National Park, USA. Science. 2008;322:261–264. doi: 10.1126/science.1163428. doi:10.1126/science.1163428 [DOI] [PubMed] [Google Scholar]
- Price T., Gross S. Correlated evolution of ecological differences among the Old World leaf warblers in the breeding and non-breeding seasons. In: Greenberg R., Marra P., editors. Birds of two worlds. Smithsonian Institution Press; Washington, DC: 2005. pp. 359–372. [Google Scholar]
- Schluter D., Grant P.R. Determinants of morphological patterns in communities of Darwin's finches. Am. Nat. 1984;123:175–196. doi:10.1086/284196 [Google Scholar]
- Slatkin M. Ecological character displacement. Ecology. 1980;61:163–177. doi:10.2307/1937166 [Google Scholar]