Mitochondrial Free Calcium Regulation during Sarcoplasmic Reticulum Calcium Release in Rat Cardiac Myocytes

Abstract

Cardiac mitochondria can take up Ca²⁺, competing with Ca²⁺ transporters like the sarcoplasmic reticulum (SR) Ca²⁺-ATPase. Rapid mitochondrial [Ca²⁺] transients have been reported to be synchronized with normal cytosolic [Ca²⁺]_i transients. However, most intra-mitochondrial free [Ca²⁺] ([Ca²⁺]_mito) measurements have been uncalibrated, and potentially contaminated by non-mitochondrial signals. Here we measured calibrated [Ca²⁺]_mito in single rat myocytes using the ratiometric Ca²⁺ indicator fura-2 AM and plasmalemmal permeabilization by saponin (to eliminate cytosolic fura-2). The steady-state [Ca²⁺]_mito dependence on [Ca²⁺]_i (with 5 mM EGTA) was sigmoid with [Ca²⁺]_mito< [Ca²⁺]_i for [Ca²⁺]_i below 475 nM. With low [EGTA] (50 μM) and 150 nM [Ca²⁺]_i(±15 mM Na⁺) cyclical spontaneous SR Ca²⁺ release occurred (5–15/min). Changes in [Ca²⁺]_mito during individual [Ca²⁺]_i transients were small (~2–10 nM/beat), but integrated gradually to steady-state. Inhibition SR Ca²⁺ handling by thapsigargin, 2 mM tetracaine or 10 mM caffeine all stopped the progressive rise in [Ca²⁺]_mito and spontaneous Ca²⁺ transients (confirming that SR Ca²⁺ releases caused the [Ca²⁺]_mito rise). Confocal imaging of local [Ca²⁺]_mito (using rhod-2) showed that [Ca²⁺]_mito rose rapidly with a delay after SR Ca²⁺ release (with amplitude up to 10 nM), but declined much more slowly than [Ca²⁺]_i (time constant 2.8 ±0.7 s vs. 0.19 ±0.06 s). Total Ca²⁺ uptake for larger [Ca²⁺]_mito transients was ~0.5 μmol/l cytosol (assuming 100:1 mitochondrial Ca²⁺ buffering), consistent with prior indirect estimates from [Ca²⁺]_i measurements, and corresponds to ~1% of the SR Ca²⁺ uptake during a normal Ca²⁺ transient. Thus small phasic [Ca²⁺]_mito transients and gradually integrating [Ca²⁺]_mito signals occur during repeating [Ca²⁺]_i transients.

Introduction

In cardiac myocytes, cytosolic Ca²⁺ concentration ([Ca²⁺]_i) transiently increases during each heart beat, initiating cellular contraction, and decreases during diastole to allow relaxation. The majority of the energy needed for contraction and intracellular ion homeostasis is provided by mitochondria via oxidative phosphorylation. Mitochondrial ATP production is sensitive to intra-mitochondrial free [Ca²⁺] ([Ca²⁺]_mito) as several key enzymes of the Krebs cycle and the F₁F_oATPase are activated by micromolar Ca²⁺ [1]. [Ca²⁺]_mito may therefore provide important feedback between cellular energy demand and supply, especially when increased cardiac inotropy is mediated by increased [Ca²⁺]_i (e.g. during beta-adrenergic stimulation) [2]. Numerous participants and regulators of mitochondrial Ca²⁺ in- and efflux contribute to the complex regulation of [Ca²⁺]_mito which is important to maintain cellular function [3].

Ca²⁺ influx into mitochondria occurs via the mitochondrial Ca²⁺ uniporter (mCU), a low affinity (K_0.5 ~tens of micromolar), high capacity uptake mechanism which utilizes the large electrochemical gradient for Ca²⁺ across the inner mitochondrial membrane [3]. So far, the molecular entity of the mCU has not been identified, but was recently functionally characterized as an inwardly rectifying Ca²⁺-selective channel [4]. Additionally, mitochondrial Ca²⁺ uptake has been proposed to occur via a rapid mode of Ca²⁺ uptake (RaM) [5] and via mitochondrial ryanodine receptors [6]; however, the contribution of these putative mechanisms to the regulation of [Ca²⁺]_mito in cardiomyocytes is unclear.

The low Ca²⁺ affinity of the mCU may limit Ca²⁺ entry during diastole and normal Ca²⁺ transients (where spatially averaged [Ca²⁺]_i is 0.1 – 1 μM). However, because parts of mitochondria are in close spatial proximity to sarcoplasmic reticulum (SR) Ca²⁺ release sites, the local [Ca²⁺]_i near ryanodine receptors or IP₃-receptors may greatly exceed bulk [Ca²⁺]_i allowing more rapid mitochondrial Ca²⁺ uptake via mCU [7].

Mitochondrial Ca²⁺ efflux occurs predominantly in a Na⁺-dependent manner (K_0.5~4–10 mM) via the mitochondrial Na⁺/Ca²⁺-exchanger (NCX_m) [8]. Mitochondrial Ca²⁺ regulation is therefore sensitive to variations in cytosolic [Na⁺] ([Na⁺]_i) which may occur during ischemia/reperfusion [9] or during heart failure [10]. Although Na⁺-independent mitochondrial Ca²⁺ efflux has been proposed [11], in the heart its contribution to [Ca²⁺]_mito seems negligible [12].

It is well established that cardiac mitochondria can take up a small fraction of the cytosolic Ca²⁺ involved in excitation-contraction (EC) coupling [8, 13], potentially shaping cellular Ca²⁺ signals [14]. However, two aspects remain unclear and controversial. First, it is unresolved whether [Ca²⁺]_mito rapidly follows the beat-to-beat variations of [Ca²⁺]_i or whether [Ca²⁺]_mito changes slowly, integrating variations in [Ca²⁺]_i over many beats [8, 15]. Second, [Ca²⁺]_mito measurements have generally not been calibrated, making it difficult to know how large the [Ca²⁺]_mito transients are and how important the phasic [Ca²⁺]_mito signals are relative to Ca²⁺ fluxes during EC-coupling.

In this study, we investigated calibrated mitochondrial [Ca²⁺] changes in response to both steady changes in [Ca²⁺]_i (in heavily buffered solution) and in response to SR Ca²⁺ release in permeabilized rat ventricular cardiomyocytes. During SR Ca²⁺ release, [Ca²⁺]_mito rises rapidly with a delay after the [Ca]_i rise, but shows much slower decline, resulting in beat-to-beat integration. During steady-state SR Ca²⁺ releases, mitochondrial Ca²⁺ uptake is small and Ca²⁺ influx and efflux are balanced. The amplitude of the [Ca²⁺]_mito transient during an individual [Ca²⁺]_i transient is less than 10 nM. These data provide novel insights into mitochondrial Ca²⁺ regulation in cardiac myocytes.

Methods

Cardiac myocyte preparation, dye loading and cell permeabilization

Cardiac ventricular myocytes were isolated from adult male Sprague-Dawley rats (250–300 g) using protocols approved by the UC Davis Institutional Animal Care and Use Committee (IACUC) (see Online Supplement for details). Freshly isolated cells were plated on laminin-coated glass cover slips for at least 45 min before dye loading. All experiments were performed at room temperature (RT) (22–23°C).

Cells were loaded with 10 μM fura-2 AM for 45 min or with rhod-2 AM for 30 min at RT in nominally Ca²⁺ free Tyrode’s solution (in mM: HEPES 5, NaCl 140, KCl 4, MgCl₂ 1, glucose 10; pH adjusted to 7.4 with NaOH). 30 min were allowed for de-esterification. Before permeabilization the bath solution was changed to a Na⁺-free/Ca²⁺-free Tyrode’s solution (in mM: HEPES 10, tetraethylammonium chloride 140, KCl 4, MgCl₂ 1, EGTA 2, glucose 10; pH adjusted to 7.4 with Trisma base) for 3–5 min. The cell surface membrane was permeabilized with saponin by exposure to a solution containing 50 μg/mL saponin and (in mM) HEPES 10, K-aspartate 135, MgCl₂ 0.7, EGTA 2, reduced glutathione 10, MgATP 5, glucose 10 for 30 s or less.

Solutions

A highly Ca²⁺-buffered, Ca²⁺-free, Na⁺-free internal solution contained (in mM): EGTA 5, HEPES 20, K-aspartate 100, KCl 40, MgCl₂ 1, maleic acid 2, glutamic acid 2, pyruvic acid 5, KH₂PO₄ 0.5, BDM 15, pH 7.2 adjusted with trisma base. To control [Ca²⁺]_i, 1.00 M CaCl₂ solution (Fluka, #21115) was added as calculated with Max-Chelator (http://www.stanford.edu/~cpatton/maxc.html). Free [Ca²⁺] was confirmed by Ca²⁺-sensitive electrode measurements. Na⁺-free internal solution with low Ca²⁺ buffering capacity and 150 nM free [Ca²⁺] contained (in mM): EGTA 0.05, CaCl₂ 0.0234, HEPES 20, K-aspartate 100, KCl 40, MgCl₂ 0.551, maleic acid 2, glutamic acid 2, pyruvic acid 5, KH₂PO₄ 0.5, MgATP 5, reduced glutathione 10, pH 7.2 adjusted with trisma base. When NaCl was added to these solutions, equal amounts of KCl were omitted. Calibration solutions contain 2–3 μM ionomycin, 10 μM FCCP and 20 μg/mL oligomycin.

Fluorescence microscopy

Fura-2 fluorescence was measured (Nikon DIAPHOT 200, 40× oil-immersion objective) with excitation at 345±10 and 380±5 nm (F₃₄₀ and F₃₈₀), with emission at 505±50 nm. The signals were corrected for background and autofluorescence by subtracting averaged signals from unloaded cells (n=6, determined every day). Autofluorescence (unloaded cell signal minus empty field signal) is ≤10% of background corrected Fura-2 signals. Laser scanning confocal microscopy (Zeiss, LSM5 Pascal, 40× water immersion objective) was used for imaging. For mitochondrial localization Fura-2 was loaded as above and 500 nM Mitotracker Orange was included during de-esterification. Fura-2 and Mitotracker Orange were excited sequentially at 405 nm (diode laser) and 453 nm (helium-neon laser) with emission at 505–530 nm and 560–615 nm bandpass filters, respectively. Mitochondrial Rhod-2 fluorescence was measured with the same confocal microscope (543 nm excitation, emission >560 nm) as 2-dimensional images or in line-scan mode (786 ms/frame and 3–26 ms/line). In some rhod-2 experiments, [Ca²⁺]_i was measured simultaneously with [Ca²⁺]_mito using 2 or 5 μM K₅fura-2 in the internal solution (405 nm excitation, 505–530 nm emission). In permeabilized cells not loaded with rhod-2/AM, 50% of the fura-2 fluorescence intensity measured at 505–530 nm was detected in the rhod-2 window (>560 nm), contaminating the rhod-2 signal. The corrected rhod-2 signal is F₅₄₃^corr = F₅₄₃ + (F₄₀₅⁰ – F₄₀₅)/2, where F₅₄₃ is the raw rhod-2 fluorescence, F₄₀₅⁰ is the resting fura-2 signal and F₄₀₅ is the fura-2 signal (which decreases during Ca²⁺ transients).

A Ca²⁺-insensitive, isosbestic signal (F_c) was calculated from the Ca²⁺-sensitive fluorescence signals as described by Zhou & Neher [16] (see Results and Online Supplement). In situ calibration of mitochondrial fura-2 was performed (see Fig. 2C-D and Online Supplement for details) using 2–3 μM ionomycin, 10 μM FCCP and 20 μg/mL oligomycin to allow [Ca²⁺]_i - [Ca²⁺]_mito equilibration. This allowed transformation of mitochondrial fura-2 signals to [Ca²⁺]_mito.

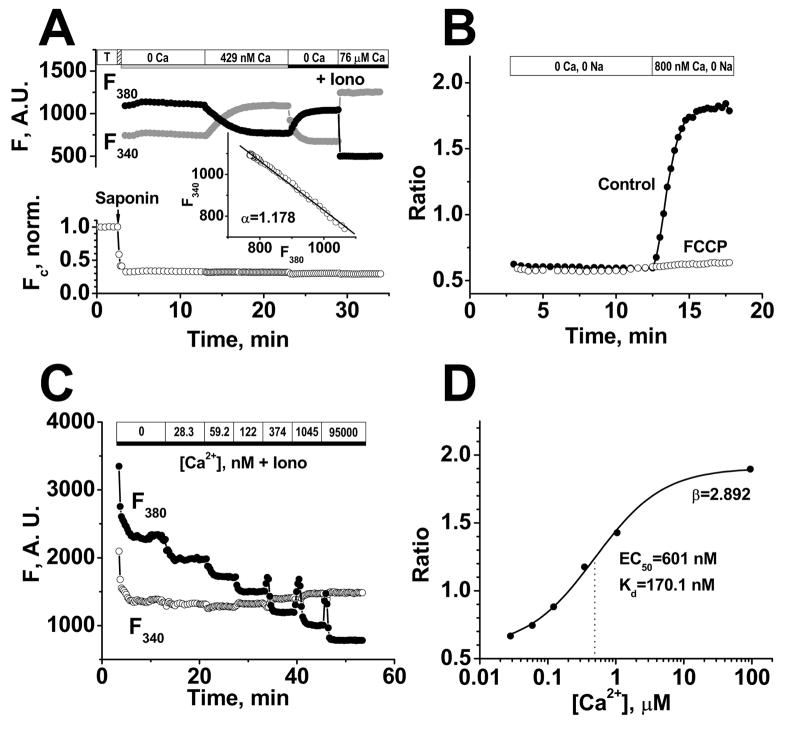
FIGURE 2. Mitochondrial free [Ca²⁺] measurements with fura-2.

A, Top traces show background-corrected fura-2 fluorescence at 340 and 380 nm excitation after permeabilization. The inset shows plot of F₃₄₀ vs. F₃₈₀ as [Ca²⁺]_mito varies to determine αand allows measurement of F_c (Ca²⁺-independent fura-2 fluorescence) in bottom normalized trace showing loss of 70% of fura-2 upon permeabilization. B, Rise in [Ca²⁺]_mito in the presence of 800 nM Ca²⁺ and in the absence of Na⁺ (±2.5 μM FCCP added 2 min prior to increasing [Ca²⁺]_i). C, In situ calibration of background subtracted fura-2 fluorescence in permeabilized cells in the presence of 3 μM ionomycin (Iono), 10 μM FCCP and 20 μg/mL oligomycin. D, Fura-2 ratio dependence on [Ca²⁺]_i for data in C fit to [Ca²⁺] = K_dβ(R-R_min)/(R_max-R), where EC₅₀ = K_dβ and β = F_380-max/F_380-min.

Drugs and Statistics

Indicators were obtained from Invitrogen (Eugene, OR), Ru360, CGP-37157 from CALBIOCHEM (La Jolla, CA) and other chemicals from Sigma (St. Louis, MO). Data are presented as mean ± SD of n measurements. Comparison between groups was performed with One-Way ANOVA (significant at P < 0.05).

Results

Mitochondrial free [Ca²⁺] measurements in rat myocytes

To measure calibrated [Ca²⁺]_mito in isolated ventricular rat cardiomyocytes we used the ratiometric Ca²⁺ indicator fura-2 under conditions promoting mitochondrial fura-2 loading, and removed cytosolic indicator by permeabilization. Saponin-induced permeabilization allows tight control of the cytosolic milieu (e.g. free [Ca²⁺], [Na⁺], Ca²⁺-buffering capacity) while allowing periodic SR Ca²⁺ release when desired.

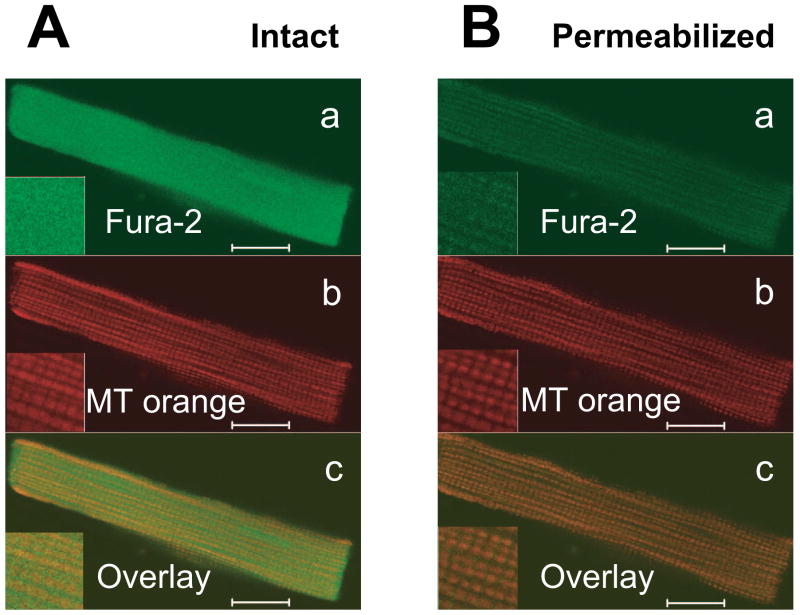
Figure 1A shows confocal images of an intact cardiomyocyte after loading with fura-2 (Fig. 1Aa, green) and mitochondrial staining with Mitotracker Orange (Fig. 1Ab, red). Saponin permeabilization (Fig. 1B) did not appreciably alter the characteristic mitochondrial pattern with Mitotracker Orange, but washed out most of the fura-2, leaving residual fura-2 matching the mitochondrial pattern.

Figure 1. Mitochondrial localization of fura-2 in permeabilized myocytes.

Confocal images of intact rat ventricular cardiomyocyte simultaneously loaded with fura-2 and Mitotracker Orange before (A) and after sarcolemmal permeabilization by saponin (B). Top panels (a) are fura-2 signals, middle panels (b) are Mitotracker Orange, showing typical mitochondrial pattern and lower panels (c) show merged images. Fura-2 only colocalizes with mitochondria after permeabilization. Scale bars are equal 10 μm.

To measure the fraction of total fura-2 loading that was mitochondrial, we used the Ca²⁺-insensitive fura-2 fluorescence (F_c) before and after permeabilization (Fig. 2A). F_c was calculated F_c = F₃₄₀ + αF₃₈₀, where -α is the slope of the F₃₄₀ vs. F₃₈₀ curve when [Ca²⁺]_mito changes (top parts of Fig. 2A, where [Ca²⁺]_mito was altered by raising [Ca²⁺]_i from 0 to 429 nM). The F_c trace in Fig. 2A shows that saponin permeabilization released 70% of the total fura-2 loaded into the intact myocyte (note that F_c did not change with [Ca²⁺]_mito). The remaining 30% of fura-2 was compartmentalized in mitochondria. The stability of F_c after permeabilization indicates no substantial mitochondrial fura-2 leakage over 30 min.

To confirm that the measured fura-2 fluorescence reflect [Ca²⁺]_mito, we applied the protonophore FCCP (2.5 μM) to dissipate the mitochondrial membrane potential (Ψ_m) and therefore the driving force for the mitochondrial Ca²⁺ uniporter (the main mitochondrial Ca²⁺ influx pathway). Figure 2B shows that FCCP abolished the rise in fura-2 ratio induced by raising [Ca²⁺]_i to 800 nM (in Na⁺-free solution). This demonstrates that the fura-2 fluorescence changes under our conditions depend on an intact Ψ_m and are therefore a valid measure of [Ca²⁺]_mito.

Since fluorescent indicator properties can depend on environment [17] we performed in situ calibration of mitochondrially compartmentalized fura-2 in rat ventricular myocytes (Fig. 2C–D). Myocytes were equilibrated with solutions of different [Ca²⁺] (0–95 μM containing Ca²⁺ ionophore ionomycin (3 μM), the protonophore FCCP (10 μM) to collapse Ψ_m, and oligomycin (20 μg/mL) to inhibit the F₁F_oATP-synthase). We found an in situ K_d of 170 nM in this experiment and results were very consistent among cells (K_d = 170±0.3 nM, n = 3).

Steady-state dependence of [Ca²⁺]_mito on cytosolic [Ca²⁺]

Next, we determined the steady-state dependence of [Ca²⁺]_mito on the [Ca²⁺]_i in the presence of 10 mM [Na⁺]_i with Ca²⁺ buffered by 5 mM EGTA. This EGTA concentration allows the precise control of [Ca²⁺]_i and prevents spontaneous SR Ca²⁺ releases (which are examined below). The internal solution contained no ATP or ADP and contained 15 mM BDM to avoid cell contraction at high [Ca²⁺]_i.

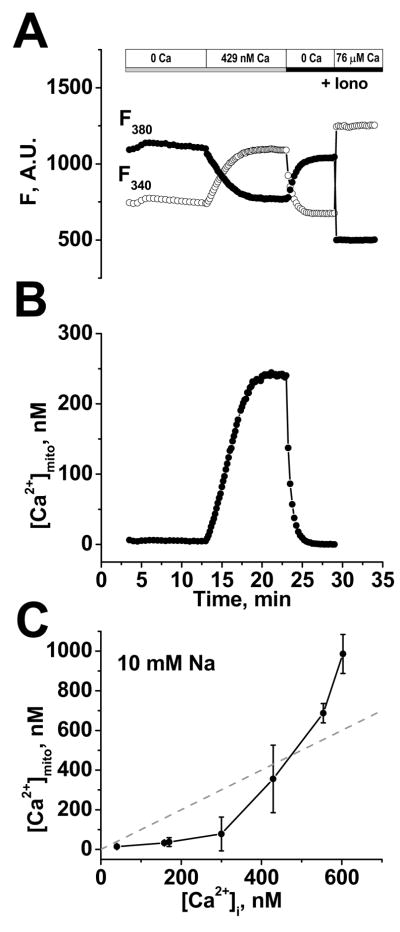
Fig. 3A shows background corrected F₃₄₀ and F₃₈₀ immediately after permeabili-zation (at 3 min in Fig. 3A), in Ca²⁺-free internal solution (10 min) followed by 10 min at 429 nM [Ca²⁺]_i. After reaching steady-state, a two point calibration was performed using Ca²⁺-free and high [Ca²⁺]_i solution (50–100 μM) with ionomycin (3 μM), oligomycin (20 μg/mL), and FCCP (10 μM) to determine R_min, R_max, F_380-max and F_380-min. Using these and the measured in situ K_d, the fura-2 fluorescence was transformed into [Ca²⁺]_mito (Fig. 3B; using the equation in Fig. 2D legend). Figure 3C shows the steady-state [Ca²⁺]_mito vs. [Ca²⁺]_i relationship. Below an apparent threshold of ~300 nM [Ca²⁺]_i, [Ca²⁺]_mito had a very shallow dependence on [Ca²⁺]_i and increased only very little as [Ca²⁺]_i increased. At cytosolic [Ca²⁺] > ~300 nM, [Ca²⁺]_mito showed a steep dependence on [Ca²⁺]_i and exceeded [Ca²⁺]_i for [Ca²⁺]_i > 500 nM.

FIGURE 3. Steady-state dependence of [Ca²⁺]_mito on [Ca²⁺]_i.

A, example of background-corrected raw F₃₄₀ and F₃₈₀ 380 nm signals, where [Ca²⁺]_i was raised from 0 to 429 nM, with R_min and R_max measured at the end using ionophores (Iono). B, calibrated [Ca²⁺]_mito calculated from A. C, Steady-state dependence of [Ca²⁺]_mito on [Ca²⁺]_i in the presence of 10 mM Na⁺ (Mean ±SD, n=3–7 myocytes).

Mitochondrial [Ca²⁺] uptake during spontaneous SR Ca²⁺ release

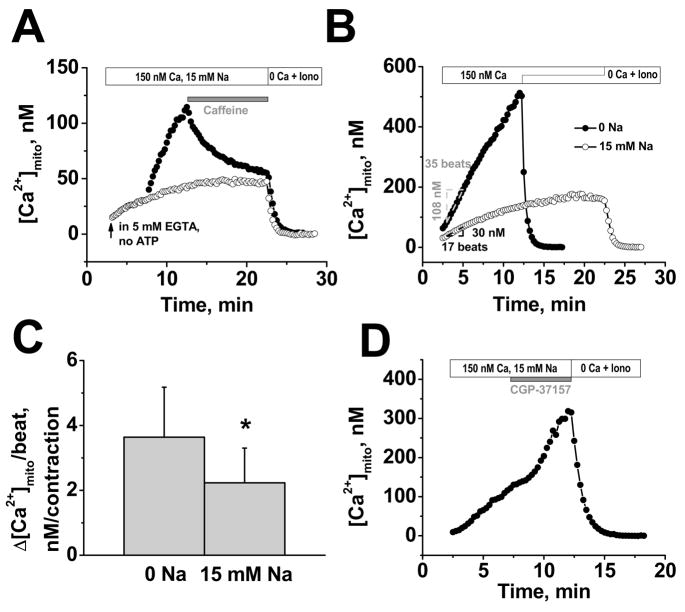
While these steady-state measurements provide a solid foundation, we next sought to test how [Ca²⁺]_mito changes during phasic SR Ca²⁺ release events which may better mimic mitochondrial Ca²⁺ regulation in vivo. By reducing [EGTA] to 50 μM and with [Ca²⁺]_i =150 nM (and physiological internal solution) spontaneous SR Ca²⁺ release events (Ca²⁺ waves) occurred at a regular frequency of 5–15 min⁻¹.

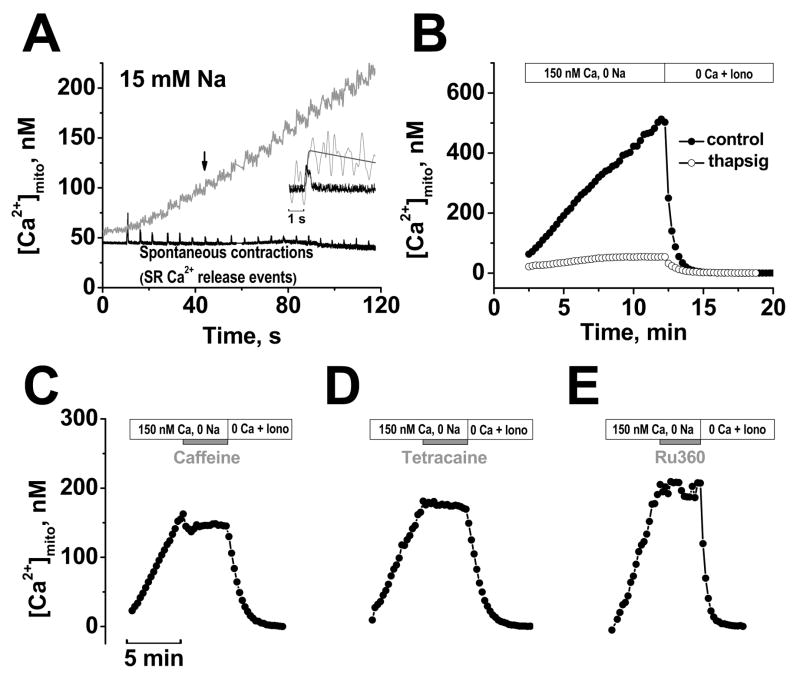
Fig. 4A shows a typical continuous [Ca²⁺]_mito recording (gray trace) and cell contraction (black trace) starting after permeabilization. For this experiment, [Na⁺]_i was 15 mM and 40 μM Cytochalasin-D was added to reduce, but not abolish cell contraction during cytosolic Ca²⁺ waves. The small residual contractions were used to measure SR Ca²⁺ wave occurrence. The inset in Fig. 4A shows a [Ca²⁺]_mito transient with curve-fit and cell contraction. Because the fura-2 loaded cardiomyocytes were incubated in Ca²⁺-free solution before permeabilization and permeabilized in Ca²⁺-free internal solution, [Ca²⁺]_mito was very low at the beginning of the measurement. [Ca²⁺]_mito was stable at ~57nM during the first 10 sec before the first Ca²⁺ wave occurred. The first 3 contractions after permeabilization in Fig. 4A were associated with step-wise increases in [Ca²⁺]_mito without substantial mitochondrial Ca²⁺ extrusion. Only in subsequent Ca²⁺ transients did mitochondrial Ca²⁺ extrusion become evident (as a declining phase of [Ca²⁺]_mito). [Ca²⁺]_mito gradually increased to ~110 nM after 8 waves and to 216 nM after 20 waves. Control experiments using TMRM to assess Ψ_m, showed no appreciable change in Ψ_m during the phasic or integrated rise in [Ca²⁺]_mito.

FIGURE 4. [Ca²⁺]_mito during cyclical SR Ca²⁺ release.

A, Simultaneous recording of [Ca²⁺]_mito (grey) and contractions (black) in the presence of 150 nM Ca²⁺ and 15 mM Na⁺. 40 μM cytochalasin D was included to limit contraction. Inset shows expanded superimposed traces for the 7^th contraction (traces smoothed by fast Fourier transform). B, [Ca²⁺]_mito under control conditions and after pretreated with 1 μM thapsigargin for 30 min. Mitochondrial Ca²⁺ uptake was halted by acute application of 10 mM caffeine (C), 2 mM tetracaine (D), or 1 μM Ru360 (E). Each experiment ended with Ca²⁺-free solution with ionophores (Iono) to start calibration.

To test whether SR Ca²⁺ release was responsible for this dynamic [Ca²⁺]_mito uptake we inhibited SR function (Fig. 4B–D). To limit cellular photo damage and allow long-term [Ca²⁺]_mito measurements over 20 min, we measured [Ca²⁺]_mito every 15 s for a duration of 2 s (each point in Fig. 4B–E is average [Ca²⁺]_mito during the 2 s).

Fig. 4B shows that blocking SR Ca²⁺ uptake by pretreatment with the specific SR Ca²⁺ATPase (SERCA) inhibitor thapsigargin (1 μM for 30 min before permeabilization to deplete SR Ca²⁺) nearly abolished the rise in [Ca²⁺]_mito. Under these conditions no spontaneous SR Ca²⁺ release events occurred and the small residual rise in [Ca²⁺]_mito to ~54 nM is explained by the slow steady-state equilibration at the ambient 150 nM [Ca²⁺]_i (in this case with [Na⁺]_i=0 which inhibits NCX_m). Figure 4C–D shows that either acute SR Ca²⁺ depletion with 10 mM caffeine or inhibition of SR Ca²⁺ release by 2 mM tetracaine terminated the [Ca²⁺]_mito rise abruptly. Notably, in both cases Ca²⁺ waves ceased, but [Ca²⁺]_mito failed to decline in the Na⁺-free solution ([Ca²⁺]_mito declines less than 5 nM/min, n=3), consistent with a dominant role of NCX_m in mitochondrial Ca²⁺ extrusion. Selective blockade of the mitochondrial uniporter with Ru360 (Fig. 4E) also stopped the rise in [Ca²⁺]_mito, although spontaneous cytosolic Ca²⁺ waves continued.

These results demonstrate that the observed mitochondrial Ca²⁺ uptake occurs as a direct consequence of SR Ca²⁺ release (we maintain the structural organization of the cardiac myocyte regarding the crosstalk between the SR and mitochondria), and that the major Ca²⁺ influx pathway under these conditions is the mitochondrial Ca²⁺ uniporter.

Quantitative analysis of the rise of [Ca²⁺]_mito per beat

In Figures 4C and 4D [Ca²⁺]_mito failed to decline in Na⁺-free solution after Ca²⁺ transients were blocked by caffeine or tetracaine. Figure 5A shows that with 15 mM [Na⁺]_i, caffeine still abruptly stopped the rise in [Ca²⁺]_mito (filled circles), but [Ca²⁺]_mito then declined exponentially (τ=3.4 min) to reach the same level as seen in the steady-state measurement protocol (with the same [Na⁺]_i, open circles). This shows that mitochondrial Ca²⁺ is removed effectively via a Na⁺-dependent pathway when mitochon-drial Ca²⁺ uptake is stopped.

FIGURE 5. Calibrated rise in [Ca²⁺]_mito per beat and [Na⁺]_i-dependence.

A, 10 mM caffeine stops [Ca²⁺]_mito, but with 15 mM Na⁺ [Ca²⁺]_mito declines (filled symbols); mitochondrial Ca²⁺ uptake in presence of 5 mM EGTA (empty symbols). B, examples of [Ca²⁺]_mito increase per contraction (±15 mM Na⁺). C, Average increase of [Ca²⁺]_mito per contraction (±15 mM Na⁺). D, Blockade of NCX_m (5 μM CGP-37157) increases rate of [Ca²⁺]_mito rise in presence of 15 mM Na⁺. Each experiment ended with Ca²⁺-free solution with ionophores (Iono) to start calibration.

Figure 5B shows representative experiments monitoring the rise in [Ca²⁺]_mito and the number of spontaneous contractions (±15 mM [Na⁺]_i). In the absence of [Na⁺]_i the [Ca²⁺]_mito rose linearly until the calibration was initiated. In contrast, with 15 mM [Na⁺]_i, the [Ca²⁺]_mito increased linearly initially, but then reached a steady-state within ~15 min. This demonstrates that Na⁺-dependent [Ca²⁺]_mito removal is required to allow [Ca²⁺]_mito to reach a steady-state during regular SR Ca²⁺ release. The rise in [Ca²⁺]_mito per SR Ca²⁺ release (Δ[Ca²⁺]_mito/wave) can be calculated from the linear part of the uptake measurements. The rate of [Ca²⁺]_mito rise in the absence and presence of Na⁺ during the indicated 35 and 17 beats in Fig. 5B was 3.1 and 1.8 nM/wave, respectively. Mean data in Fig. 5C show this rise in [Ca²⁺]_mito/wave to be 3.6±1.5 nM (n=16) and 2.2±1.1 nM (n=19) in the absence and presence of 15 mM [Na⁺]_i, respectively.

Figure 5D shows that abrupt block of the NCX_m with 5 μM CGP-37157 during the rising phase of [Ca²⁺]_mito with 15 mM [Na⁺]_i increased the rate of rise of [Ca²⁺]_mito per wave. The mean acceleration induced by CGP-37157 in 4 cells was 74%, similar to the 63% higher rate of [Ca²⁺]_mito rise per wave in Na⁺-free solution in Fig. 5C.

Spatially resolved [Ca²⁺]_mito measurements during SR Ca²⁺ release

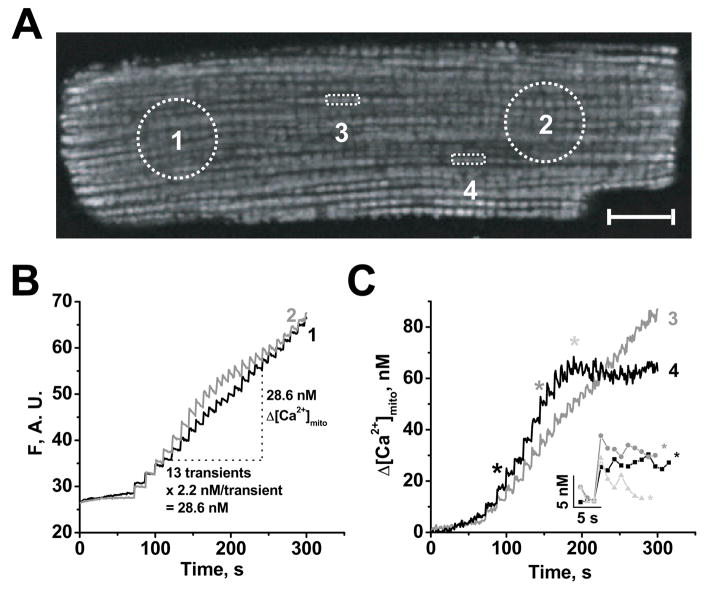
The above calibrated [Ca²⁺]_mito measurements with fura-2 and measures of [Ca²⁺]_mito rise per Ca²⁺ wave allows us to extend these studies with additional spatio-temporal detail using confocal microscopy and the cationic fluorescent Ca²⁺ indicator rhod-2 (which is less readily calibrated). Figure 6A shows a rhod-2 loaded cardiomyocyte 5 min after permeabilization after [Ca²⁺]_mito has risen by ~50 nM (see Fig. 6B). The movie (in Online Supplement) shows the pulsatile emergence of the clear mitochondrial pattern as [Ca²⁺]_mito and rhod-2 fluorescence rise. We focused our analysis on intermyofibrillar mitochondria because some subsarcolemmal mitochondria were less consistent.

FIGURE 6. Spatially resolved [Ca²⁺]_mito during SR Ca²⁺ release.

A, confocal image of permeabilized rat ventricular myocyte loaded with 10 μM rhod-2 AM, after 5 min of spontaneous Ca²⁺ transients in 15 mM Na⁺ (with 80 μM cytochalasin D) with 4 regions of interest (ROI) indicated. Scale bar is equal 10 μm. B-C, Time courses of [Ca²⁺]_mito in 4 ROIs, either as fluorescence (F in arbitrary units, B) or calibrated Δ[Ca²⁺]_mit (C) using averaged Δ[Ca²⁺]_mito/beat from Fig. 5C (see B and text). C, ROI 3 and 4 contain 3 mitochondria and inset shows the indicated [Ca²⁺]_mito transients expanded.

Figure 6B shows mitochondrial fluorescence during Ca²⁺ waves (5–6 min⁻¹) from two large regions of interest (ROI1 and 2 in Fig. 6A) at opposite ends of the cell. As in Fig. 4A, [Ca²⁺]_mito increased during the first transients in a step-like manner without appreciable [Ca²⁺]_mito decay. The rhod-2 fluorescence changes in ROI 1 and ROI 2 were very similar (and like the whole cell), suggesting that [Ca²⁺]_mito is relatively homogeneous when a large fraction of the cellular mitochondria is compared. Additionally, the [Ca²⁺]_mito peaks in ROI 1 and 2 were within 1 s of each other, indicating relatively homogeneous cytosolic Ca²⁺ changes.

Using the [Ca²⁺]_mito gain per Ca²⁺ wave measured with fura-2 (2.2 nM/wave; Fig. 5C), we infer that the [Ca²⁺]_mito rise for the 13 transients indicated was 28.6 nM for both ROI 1 and ROI 2. This indicates that one fluorescence unit reflects a Δ[Ca²⁺]_mito of ~1.44 nM [Ca²⁺]_mito. Next, we used this approximation to estimate [Ca²⁺]_mito changes in two smaller ROIs (Fig. 6C, ROI 3 and 4 shown in Fig. 6A). These smaller ROIs (with only 3 mitochondria) differed slightly. ROI 3 showed a linear increase much like ROI 1 and 2, whereas [Ca²⁺]_mito in ROI 4 reached a plateau after gaining ~60 nM in 3 min. The upstroke amplitudes of individual [Ca²⁺]_mito transients in the inset of Fig. 6C were ~10–12 nM (for the 3 indicated transients). These results suggest that individual mitochondria or small clusters of mitochondria can respond differently to a homogenous cytosolic Ca²⁺ transient and that the amplitudes of [Ca²⁺]_mito transients are much smaller (~100x) than the amplitude of bulk cytosolic Ca²⁺ transients.

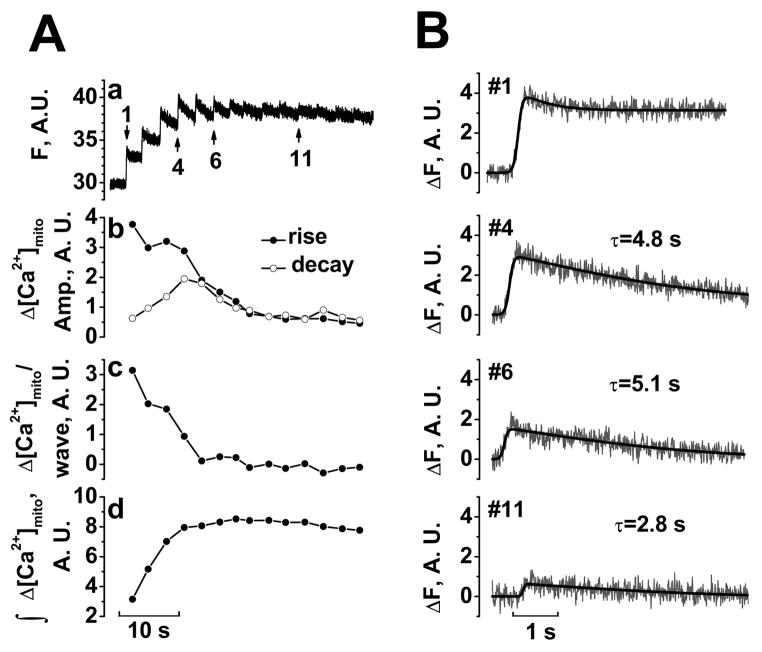
Kinetics of [Ca²⁺]_mito changes during SR Ca²⁺ release

To enhance temporal resolution, we used line-scan confocal microscopy along a 40.5 μm line (~20 mitochondria). Figure 7A shows [Ca²⁺]_mito which rose to a steady-state within a small number of Ca²⁺ waves (expanded time scale in Fig. 7B; typical of 5 other cells). Figure 7Ab shows the [Ca²⁺]_mito rise and fall amplitudes for each beat. The first [Ca²⁺]_mito transient had a large upstroke and small decline amplitude, leading to a large rise in [Ca²⁺]_mito with little decay in [Ca²⁺]_mito (Fig. 7B#1). As mitochondria gained Ca²⁺ (Fig. 7Ad), upstroke amplitudes decreased and decline amplitudes increased until influx and efflux balanced (Fig. 7B#6 and #11) and [Ca²⁺]_mito stopped increasing. The time constant of [Ca²⁺]_mito decay was typically 2.5–5 s (Fig. 7B). At steady-state, [Ca²⁺]_mito transients were very small. Thus, mitochondria can rapidly follow cytosolic [Ca²⁺] changes when [Ca²⁺]_mito is low, but during steady-state, [Ca²⁺]_mito does not change appreciably in response to cytosolic Ca²⁺ transients.

FIGURE 7. Mitochondrial [Ca²⁺] balance during SR Ca²⁺ release.

Aa, [Ca²⁺]_mito measured in line-scan images in permeabilized myocyte loaded with 5 μM rhod-2 AM in 15 mM Na⁺ (with 80 μM cytochalasin D; subjected to 5 point smoothing). Ab, Amplitudes of rise (filled symbols) and decay phase (empty symbols). Ac, Net gain in [Ca²⁺]_mito per wave. Ad, Integral of change in [Ca²⁺]_mito. B, Expanded and curve fitting for the transients indicated in A.

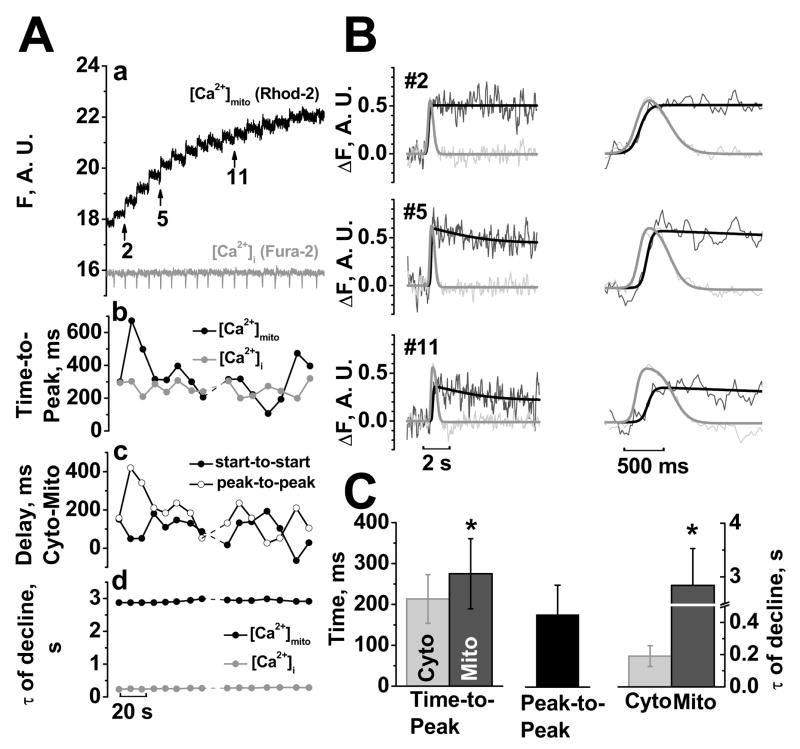
In the next experiments, we compared the kinetics of [Ca²⁺]_mito and [Ca²⁺]_i transients, simultaneously measured with mitochondrial rhod-2 and cytosolic fura-2 using the confocal line-scan mode (fura-2 salt was in the cytosolic solution). Figure 8A shows a typical experiment starting 40 s after permeabilization (with expanded traces in Fig. 8B with fura-2 signal inverted). The [Ca²⁺]_i signals in Fig. 8 were not calibrated, but parallel calibrated experiments with the same protocol showed Ca transients of 1.3–2.5 μM (i.e. typical of spontaneous waves in intact myocytes). Time-to-peak for [Ca²⁺]_mito and [Ca²⁺]_i transients is shown in Fig. 8Ab (note that this is the rise time for each trace independently). The delay between the [Ca²⁺]_i and [Ca²⁺]_mito peaks (peak-to-peak) and start-to-start delays are shown in Fig. 8Ac.

FIGURE 8. Kinetics of [Ca²⁺]_mito vs. [Ca²⁺]_i signals.

Aa, [Ca²⁺]_mito and [Ca²⁺]_i measured in line-scan images in permeabilized myocyte with mitochondrial rhod-2 and 5 μM cytosolic fura-2 salt (15 mM Na⁺, 80 μM cytochalasin D, 5 point smoothing). Ab, Time-to-Peak (rise time for either [Ca²⁺]_mito or [Ca²⁺]_i). Ac, Delay of [Ca²⁺]_mito after [Ca²⁺]_i as start-to-start and peak-to-peak, Ad, time constant τof decline. B, Curve fitting and expanded mitochondrial (black) and cytosolic (grey) transients indicated in Aa. C, Difference in mitochondrial and cytosolic kinetics (n=3 cells, 25 transients).

Figure 8B shows that [Ca²⁺]_i transients preceded [Ca²⁺]_mito transients. On average, [Ca²⁺]_i started to rise 111±60 ms before [Ca²⁺]_mito. [Ca²⁺]_i rose to peak in 213±59 ms, whereas [Ca²⁺]_mito rose slightly slower (in 275±86 ms, p<0.05, n =25 transients, 3 cells, Fig. 8C). Due to the later start and the slower upstroke, [Ca²⁺]_mito peaked 177±72 ms after [Ca²⁺]_i (Fig. 8C). [Ca²⁺]_mito decline was dramatically slower than [Ca²⁺]_i decline (time constant were 2.8±0.7 s vs. 0.19±0.06 s, respectively, Fig. 8C). Additionally, the time constant of [Ca²⁺]_mito decline was relatively constant from transient-to-transient suggesting that extrusion was not activated by increasing [Ca²⁺]_mito (Fig. 8Ad).

Discussion

Cardiac mitochondrial Ca²⁺ uptake may be important in controlling energy supply-demand balance (via activation of mitochondrial dehydrogenases), protecting myocytes from transient Ca²⁺ overload and mediating cell death pathways (necrosis or apoptosis) [14, 18–20]. Mitochondrial Ca²⁺ uptake occurs in response to elevated [Ca²⁺]_i (which in heart occurs transiently with each beat) and it is increasingly clear that some Ca²⁺ enters the mitochondria with each heartbeat [21–25]. However, most prior studies of [Ca²⁺]_mito in cardiac myocytes lack calibration, and in some cases contamination of the [Ca²⁺]_mito signal by cytosolic indicator has not been completely ruled out. These aspects limit the quantitative understanding of mitochondrial Ca²⁺ regulation, and motivated the present study. Here we show carefully calibrated [Ca²⁺]_mito signals in permeabilized cardiac myocytes both at rest (where we characterized the steady-state [Ca²⁺]_mito vs. [Ca²⁺]_i relationship) and during regular SR Ca²⁺ release events. We find that the largest rise in [Ca²⁺]_mito during a local SR Ca²⁺ release is ~10 nM (e.g. when [Ca²⁺]_mito begins very low), and the rise occurs rapidly (275 ms time to peak) within a 100–200 ms delay of the rise in [Ca²⁺]_i. However, the [Ca²⁺]_mito decline is ten times slower than that of [Ca²⁺]_i (2.8 vs. 0.19 s time constant). The slow [Ca²⁺]_mito decline between beats leads to integration of [Ca²⁺]_mito (even at the low frequency of spontaneous Ca²⁺ waves here) to a level many times higher than the amplitude of a single [Ca²⁺]_mito transient. While we acknowledge that these myocytes are not in a truly physiological setting, these results provide useful novel quantitative data to help understanding mitochondrial Ca²⁺ balance in cardiac myocytes.

Limitations

We have measured [Ca²⁺]_mito in quantitative detail in a relatively physiological mitochondrial environment using saponin-permeabilized myocytes, selective mitochon-drial ratiometric Ca²⁺ indicator and intrinsic spontaneous large SR Ca²⁺ release events [26–28]. However, the control gained by permeabilization makes the situation less physiological in several ways. First, small diffusible molecules may be lost by the myocyte, and we cannot rule out that this could alter regulation of mitochondrial Ca²⁺ transport mechanisms (although the impact of this is unknown). Second, it is possible that the saponin exposure changes the physical geometry between the SR Ca²⁺ release junctions and the mitochondria. While we have no reason to expect this, it also cannot be ruled out. Third, and perhaps most important, we are limited in the frequency of SR Ca²⁺ release events, to that which occurs spontaneously (up to ~0.2 Hz). This is much lower than the frequency of the normal heartbeat (even at our sub-physiological 23°C). At higher frequencies, we would expect more dramatic [Ca²⁺]_mito integration (i.e. [Ca²⁺]_mito would rise higher at steady-state), but we do not expect that would increase the transient Δ[Ca²⁺]_mito associated with a single SR Ca²⁺ release. Indeed, the phasic components might be expected to be smaller at higher [Ca²⁺]_mito in the steady-state (as in cases here as the steady-state is approached in Fig. 7 and 8). Fourth, the spontaneous SR Ca²⁺ releases occur as propagated waves (which travel at ~100 μm/s) through the cytosol. While this will spread out the time course of [Ca²⁺]_i and [Ca²⁺]_mito kinetics, it should do so in a parallel manner, such that relative comparisons are still well justified. Furthermore, our most critical kinetic analysis is done in local regions containing 3–30 mitochondria, where this concern is less of a limitation. One might also wonder if the local SR Ca²⁺ release during these waves may be less than during physiological Ca²⁺ transients. However, these events occur only at high SR Ca²⁺ loads, and if anything are likely to be higher at the local level than the normal physiological Ca²⁺ transient [29]. On the other hand, using permeabilized cells allows the measurement of purely mitochondrially derived fluorescence without having to deal with potential problems associated with Mn²⁺-quench (e.g. inhibition of the mitochondrial Ca²⁺ transport or inhibition of oxidative phosphorylation by Mn²⁺) [3, 30], an approach which has been used to minimize cytosolic fluorescence [31, 32]. Thus, while trade-offs are required for the quantitative confidence we have in our results, this work is highly relevant for understanding physiological mitochondrial Ca²⁺ regulation.

Quantitative changes in [Ca²⁺]_mito

Our experiments start from Ca²⁺ depleted mitochondria, because of pre-incubation in low [Ca²⁺]_o prior to permeabilization and permeabilization in Ca²⁺-free solution. Starting from this low level, we see the largest increases of [Ca²⁺]_mito during the first several Ca²⁺ transients. Note that the [Ca²⁺]_i signals usually do not change appreciably (see Fig. 8A), so the change in [Ca²⁺]_mito transients is not secondary to [Ca²⁺]_i transient differences. The first few [Ca²⁺]_mito transients have the largest rising phases and often exhibit little decline (consistent with little Ca²⁺ efflux at very low [Ca²⁺]_mito). Indeed, some of the slight decline in [Ca²⁺]_mito in the first transient in Fig. 8 might be due to buffering of [Ca²⁺]_mito. Notably, the rising phase of [Ca²⁺]_mito becomes much smaller (almost 10 times, Fig. 8Ab) as integrated [Ca²⁺]_mito approaches steady-state. This might be partly due to faster Ca²⁺ extrusion as [Ca²⁺]_mito rises. However, because the Ca²⁺ efflux kinetics are so slow (τ=2.8 s even at higher [Ca²⁺]_mito, Fig. 8Ad), speeding efflux by 2-fold should only reduce amplitude <10%. Thus the reduced amplitude may also reflect reduced Ca²⁺ influx (although the mechanism is not obvious). We also showed that NCX_m is required for Ca²⁺ efflux from mitochondria. [Na⁺]_i was held constant here at 15 mM, but when [Na⁺]_i changes physiologically this would be expected to alter the steady-state [Ca²⁺]_mito and rate of [Ca²⁺]_mito decline [23, 33]. In any event, at steady-state (where [Ca²⁺]_mito stops rising) influx and efflux must be the same and both appear to be much lower than the maximal rising phase of [Ca²⁺]_mito when influx starts.

The maximal rise of [Ca²⁺]_mito during a single SR Ca²⁺ release is ~10 nM (Fig. 6C) with typical rise values of 2–4 nM (Fig. 5D). If we assume that intra-mitochondrial Ca²⁺ buffering is 100:1 (as in cytosol), and use the highest value (10 nM) this would translate to 1 μM rise in total mitochondrial Ca²⁺ content, which corresponds to removal of 0.5 μmol/l cytosol. Surprisingly, this agrees well with Bassani et al. estimates of the quantitative contribution of mitochondrial Ca²⁺ uptake to removal of cytosolic Ca²⁺ during E-C coupling by analysis of [Ca²⁺]_i decline by different Ca²⁺ transporters in intact rat ventricular myocytes [13]. They found that ~75 μmol/l cytosol of Ca²⁺ were added and removed to the cytosol at a normal Ca²⁺ transient, and that about 1% was removed by the combination of mitochondria plus sarcolemmal Ca²⁺-ATPase (0.8 μmol/l cytosol) which contribute about equally with each other [34]. Given the completely different methods and limitations, the quantitative agreement encourages us to think we are close to correct.

Local vs. global [Ca²⁺]_i drives mitochondrial Ca²⁺ uptake

Several studies have suggested that mitochondria are in close physical proximity to the sites of SR Ca²⁺ release and consequently that SR (or endoplasmic reticulum) Ca²⁺ release has preferential access to drive mitochondrial Ca²⁺ uptake [7, 35–39]. Indeed, Sharma et al. [36] showed that 1 mM BAPTA (a fast Ca²⁺ buffer), which reduced the caffeine-induced rise in [Ca²⁺]_i by 90% in permeabilized rat myocytes, only decreased the mitochondrial rhod-2 signal by 23%. Our results are consistent with this general idea and provide some quantitative context in terms of [Ca²⁺]_mito. Note that when we abruptly switch bath [Ca²⁺] from 0 to 429 nM (heavy [Ca²⁺]_i buffering; Fig. 3B) or pretreat myocyte with thapsigargin (Fig. 4B) the rate of [Ca²⁺]_mito rise is linear over several minutes (0.1–0.7 nM/s). This is much slower than during an SR Ca²⁺ release 36 nM/s (10 nM/0.275 s), where global [Ca²⁺]_i is only transiently raised to a slightly higher level (0.5–1 μM [Ca²⁺]_i). This suggests that phasic mitochondrial Ca²⁺ influx must be driven in large part by the higher local (vs. global) [Ca²⁺]_i during SR Ca²⁺ release. This makes sense with the high K_0.5 for [Ca²⁺]_i of the mCU [3], which is required for the observed mitochondrial Ca²⁺ uptake seen here. We can look at this another way. The 20 Ca²⁺ waves during the 2 min in Fig. 4A only raise average [Ca²⁺]_i from 150 to 200 nM (assuming each has peak [Ca²⁺]_i ~1 μM with kinetics as in Fig. 8). Based on the data in Fig. 3C and with thapsigargin in Fig. 4B, this small rise in global [Ca²⁺]_i alone could not increase [Ca²⁺]_mito to 200 nM. So the SR Ca release events are clearly important drivers of [Ca²⁺]_mito rise.

Two points should be borne in mind here. First, while high local SR Ca²⁺ release accelerates mitochondrial Ca²⁺ uptake, the total amount is still small compared to SR Ca²⁺ uptake or Na⁺/Ca²⁺ exchange in cardiac myocytes [13]. Second, while one end of a large mitochondrion may be close to the site of SR Ca²⁺ release (e.g. 37–270 nm; [36]) the other end of a typical intramyofibrillar mitochondrion is ~1 μm away, at the middle of the sarcomere. Considering the overall mitochondrial geometry, the average place on the mitochondrion within the sarcomere probably senses a similar local [Ca²⁺]_i as does the cytosol. Thus, there may be preferential local Ca²⁺ influx on the end of a mitochondrion that is very close to an SR Ca²⁺ release unit, but this may be diluted and buffered strongly in the whole mitochondrion. Sub-mitochondrial [Ca²⁺]_mito gradients might even exist, at least transiently.

Phasic vs. integrative signaling

Our [Ca²⁺]_mito dependence on [Ca²⁺]_i in highly buffered solutions agrees fairly well with and validates the classical studies in isolated mitochondria [40] and of Miyata et al. [31] who used Mn²⁺ to quench cytosolic indo-1 during slow Ca²⁺ transients mediated by Na⁺/Ca²⁺ exchange (rather than SR Ca²⁺ release; compare our Fig. 3C with their Fig. 7B). Thus, Fig. 3C represents well the static quasi-equilibrium relationship. It seems clear that Fig. 3C is a good representation of the static quasi-equilibrium relationship. While helpful, this alone does not inform the question of dynamic [Ca²⁺]_mito regulation during SR Ca²⁺ release.

Experimental data in favor of both “slow integrating” and “phasic” theories of [Ca²⁺]_mito changes during Ca transients were summarized by Dedkova and Blatter [8]. Among recent studies, rapid switches in [Ca²⁺]_i simulating Ca²⁺ transients in permeabilized cat ventricular myocytes by Sedova et al. [12] supports a “slow integrating” model, whereas in guinea-pig cardiomyocytes Maack et al. [23] observed rapid mitochondrial Ca²⁺ transients during every cytosolic Ca²⁺ transient elicited by voltage clamp pulses to initiate SR Ca²⁺ release. While these were both uncalibrated [Ca²⁺]_mito measurements they indicate extremes. Sometimes phasic [Ca²⁺]_mito transients have not been detected [12, 31, 32]. This could be either because the very small [Ca²⁺]_mito transients were simply below the detection limit, or because local SR Ca release events were not used (and may be essential as above). There may also be species differences in resting [Na⁺]_i or mitochondrial Ca²⁺ transport, such that beat-to-beat changes in [Ca²⁺]_mito have been seen in guinea-pig [41, 42], but not in rat [22, 43] and hamster [44, 45]. The apparently large [Ca²⁺]_mito transients observed by Maack et al. [23] in guinea-pig myocytes (which seem to even limit [Ca²⁺]_i transients) are convincing, but seem to require PKA activation and strong cellular Ca²⁺ loading. We were unable to demonstrate substantially larger [Ca²⁺]_mito transients here by including cAMP to activate PKA (not shown).

Our work suggests that both phasic and integrative changes in [Ca²⁺]_mito occur, and we have added some quantitative framework to better understand the amplitude and kinetics of these components of [Ca²⁺]_mito control.

Abbreviations

SR

Sarcoplasmic reticulum

[Ca²⁺]_mito

Intra-mitochondrial free Ca²⁺ concentration

[Ca²⁺]_i

Cytosolic Ca²⁺ concentration

mCU

Mitochondrial Ca²⁺ uniporter

RaM

Rapide mode of Ca²⁺ uptake

NCX_m

Mitochondrial Na⁺/Ca²⁺-exchanger

[Na⁺]_i

Cytosolic Na⁺ concentration

EC

Excitation-contraction

RT

room temperature

F_c

Ca²⁺-insensitive, isosbestic signal