Tensioning the helix: a mechanism for force generation in twining plants

Abstract

Twining plants use their helical stems to clasp supports and to generate a squeezing force, providing stability against gravity. To elucidate the mechanism that allows force generation, we measured the squeezing forces exerted by the twiner Dioscorea bulbifera while following its growth using time-lapse photography. We show that the development of the squeezing force is accompanied by stiffening of the stem and the expansion of stipules at the leaf base. We use a simple thin rod model to show that despite their small size and sparse distribution, stipules impose a stem deformation sufficient to account for the measured squeezing force. We further demonstrate that tensioning of the stem helix, although counter-intuitive, is the most effective mechanism for generating large squeezing forces in twining plants. Our observations and model point to a general mechanism for the generation of the twining force: a modest radial stem expansion during primary growth, or the growth of lateral structures such as leaf bases, causes a delayed stem tensioning that creates the squeezing forces necessary for twining plants to ascend their supports. Our study thus provides the long-sought answer to the question of how twining plants ascend smooth supports without the use of adhesive or hook-like structures.

1. Introduction

Climbing plants use a diversity of specialized attachment structures to cling to a support and grow upwards (Darwin 1876; Baillaud 1968; Putz & Mooney 1991). Twining is exceptional among climbing mechanisms because the stem itself is the attachment structure, in addition to its ordinary roles of searching for a support and increasing the length of the plant. The process by which a twiner finds a support, generates a helical form, and grips its support has fascinated plant biologists for more than a century (von Mohl 1827; Darwin 1876; von Sachs 1882; Hendricks 1940). Twining plants are remarkable because over an extensive apical region the stem is flexible and undergoes broad circumnutational movements (Darwin & Darwin 1896; Silk & Abou Haidar 1986; Silk 1989; Caré et al. 1998) while farther from the apex, the stem forms a strikingly uniform helix (Darwin 1876; Bell 1958; Baillaud 1968; Putz & Holbrook 1991) that can squeeze a support with considerable force (Silk & Hubbard 1991; Matista & Silk 1997; Scher et al. 2001).

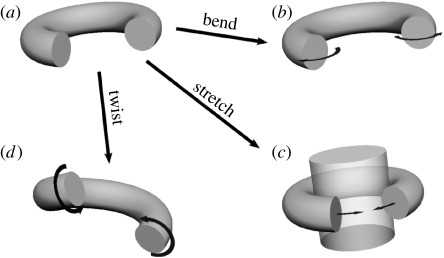
Frictional contact forces are required to prevent twining plants from slipping down their supports (Silk & Holbrook 2005). A normal (squeezing) force is needed to create such frictional interactions, but there is no consensus theory to explain how this squeezing force is produced. A vine can squeeze its support whenever the twining helix has a natural internal radius smaller than the radius of the support. Tightening of the helix can be brought about by twisting, bending or stretching (figure 1), and it is still unclear which of these modes of deformation is the most important. By examining the helical geometry of twining vines on and off the supporting pole, Silk & Hubbard (1991) found significant changes in torsion and curvature in the relaxed shape, suggesting that both modes of deformation could contribute to the squeezing force. The elastic energy stored in the stem was, however, small. Other forces may therefore be involved in maintaining the stability of the vine. Hydraulic inflation of the stem has been proposed to induce twisting and bending, causing a contact force that induces tension in the stem (Silk & Hubbard 1991), although Silk et al. (2000) later found that 50–90% of the twining force remains after dehydration in Ipomoea purpurea and Dioscorea bulbifera, indicating that cellular turgor pressure is not the sole contributor to the twining force. Finally, Scher et al. (2001) proposed that twining forces are produced by shortening of maturing fibres in the stem, but could not find a consistent relationship between the timing of fibre maturation and the generation of squeezing force. More recently, the twining vine problem has been studied in terms of the equilibria of an elastic rod on a cylindrical support (Goriely & Neukirch 2006). However, observation of growing vines indicates that they rarely assume the configurations predicted by this theoretical treatment, suggesting that the details of vine growth and mechanics may recommend different assumptions.

Figure 1.

Three modes of deformation of a helical structure. A ring is shown for clarity, but the same is true for any helix. (a) Relaxed state. (b) The stem can be bent, (c) stretched, or (d) twisted to squeeze the support. Deformations are exaggerated for illustration.

To address the mechanism of force generation in twining plants, we measured the squeezing force exerted by the twiner D. bulbifera and used time-lapse photography to document the trajectory of the apex and the development of the helical form on a cylindrical support. We found that the mature stem is never in continuous contact with the pole; instead, stipules extending from the petiole base form discrete contact points between the vine and its support. Based on the observation that expansion of stipules and stiffening of the stem coincide with the increase in squeezing force, we developed a simple thin rod model that explains how these structures contribute to the twining force and showed that petioles, stem appendages or slight stem thickening can produce a similar effect in other twining plants.

2. Material and methods

(a) Development of squeezing forces during twining growth

We developed a mechanical pole (see figure A1 in the electronic supplementary material), inspired by the TWIFOR of Matista & Silk (1997), to measure the in vivo squeezing forces exerted by twining vines. Each mechanical pole consisted of a 2 m long PVC pipe (Schedule 40; 33.3 mm diameter) from which a 25 cm section had been cut, 50 cm above the bottom end (see figure A1 in the electronic supplementary material). This 25 cm section was split longitudinally and one-half cylinder was rigidly reassembled with the pole. The remaining half-cylinder was anchored to the rest of the pole via two thin-beam load cells (LCL-113G, Omega Engineering, Stamford, CT, USA), forming a hemicylindrical force plate. Vines completed roughly two gyres before touching the force plate and formed six to seven gyres above it. The force plate was spanned by one and a half to two complete gyres of a vine.

Dioscorea bulbifera plants were grown from tubers in a greenhouse and moved to growth chambers (temperature 26°C, 60% humidity, continuous light) when shoots began circumnutating. Pots were placed close to PVC mechanical poles and one stem was allowed to twine around each pole. The correspondence between time course and the arc length distance between the apex and the lower end of the force plate was based on a stem elongation rate of 2.25 mm h⁻¹, calculated from daily length measurements of two stems growing on 33.3 mm diameter poles in the growth chamber for two weeks.

(b) Stipule expansion, stem growth and stem stiffness

We measured stipule width on three twining stems. Nodes from each stem were scanned on a flatbed scanner after distance from the apex was noted; then average stipule width at each node was calculated as the average distance that stipules extended away from the stem on both sides.

A three-point bending method was used to measure stem flexural stiffness (EI, Nm²) (Vincent 1990), from which we calculated the Young modulus (E, MPa) after computing the second moment of area of the elliptic stem cross-section (I, mm⁴) (Niklas 1992). Stretching stiffness is calculated as

$$S=EA$$ (2.1)

with A the stem cross-sectional area. Segments with lengths of 7–34 mm were excised and embedded in paraffin at both ends to retard dehydration. Stems were placed on fixed supports and a load cell (Futek LSB200 with CSG110 amplifier module, Irvine, CA, USA) fixed to a micromanipulator was used to apply successive vertical displacements (30–100 μm) in the middle of the tested stem. Slightly curved segments were loaded in the direction of the curvature. Successive loads were maintained for 4 min in the apical region of the stem and 3 min in mature parts to allow for stress relaxation. Mechanical measurements were carried out on three stems twining on 33.3 mm diameter poles, one circumnutating shoot and one stem growing on a wire mesh.

We measured growth rates in 11 stems by following the displacement away from the vine apex of specks of white or yellow latex paint marked along the stem (Silk & Abou Haidar 1986). The displacement velocity of these marks was used to compute the distribution of the remaining strain (i.e. the elongation growth that a small stem element still has to experience before it exits the growth zone). The strain remaining was calculated as log(V^*/V(s)) where V^* is the vine elongation rate and V(s) is the rate at which a material element at s moves away from the apex (Silk & Abou Haidar 1986).

(c) Force per length from twisting and bending

Twining stems of D. bulbifera tend to coil more tightly when removed from their support, and this tendency could contribute to the normal force. Therefore, we compared estimated forces resulting from change in geometry to estimated forces resulting from stretching. We measured the change in helical configuration of 10 D. bulbifera vines when removed from their support. Support diameters were 21.88 mm (3), 42.1 mm (2), 60.16 mm (1), 89.6 mm (2), and 114.51 mm (2). Vine helices on and off the pole were characterized by the pitch and arc length per gyre over 6–11 gyres (Silk & Hubbard 1991). The helical configuration of a stretched spring can be described by two parameters, yielding estimates of both torsional and bending stiffness (Miller 1902). Therefore, we stretched the same 10 vines horizontally with a mass of 13–30 g suspended over a pulley and measured pitch and arc length per gyre. We used early work on helical springs (Miller 1902) to estimate the force per length imposed by the difference in helical geometry between the resting (off-pole) shape and the realized (on-pole) shape. We calculated the elastic energy of the vine based on thin rod theory, considering only torsion and bending of thin slices along the vine axis but neglecting warping of cross-sections (Love 1927). Sophisticated analyses for springs based on elasticity theory (Ancker & Goodier 1958b,c) give corrections of less than 5 per cent to the approximate theory (Ancker & Goodier 1958a). The elastic energy per length U of a gently twisted and bent thin rod is

$$U=\frac{1}{2}EI{(\mathrm{\Delta }\kappa )}^2+\frac{1}{2}GJ{(\mathrm{\Delta }\tau )}^2,$$ (2.2)

where EI and GJ are the flexural and torsional stiffness of the rod and κ and τ are the curvature and torsion of the rod (including both Frenet torsion and material twist), with Δ indicating difference from the relaxed state (Love 1927). The normal force applied by the vine because of its curvature and torsion follows from differentiation of equation (2.2) with respect to support radius a; approximating derivatives of curvature and torsion with finite differences, the normal force per length p_n on the support is

$$p_n\approx \frac{2U}{\mathrm{\Delta }a},$$ (2.3)

(see also ‘twist and bend calculations’ in the electronic supplementary material).

The helix-stretching experiments also yielded an estimate of the shear modulus G and an estimate of the Young modulus E independent of the three-point bending experiments:

$$E=-\frac{1}{I}\frac{F}{\mathrm{\Delta }\kappa }\frac{ac}{d}G=\frac{1}{J}\frac{F}{\mathrm{\Delta }\tau }\frac{a^2}{d},$$ (2.4)

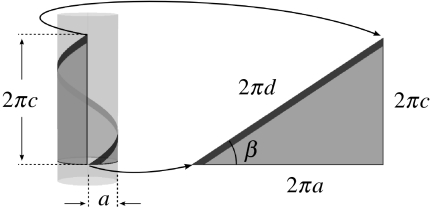
where I is the second moment of area; J the polar moment of area; and a, c and d characterize the on-pole geometry of the vine (figure 2; see ‘twist and bend calculations’ in the electronic supplementary material).

Figure 2.

Characterization of helical geometry. The relationship among helix parameters a, c, d can be pictured by wrapping a right triangle of width 2πa and height 2πc around a cylinder of radius a. The hypotenuse of the triangle, with length 2πd, forms a single gyre. The helix has radius a, pitch c, and arc length per radian d; it has curvature a/d² and torsion c/d² (Silk & Hubbard 1991). From the geometry of the triangle, d²=a²+c²; the angle of ascent β of the helix is given by cos β=a/d.

(d) Force per length from stretching by stipules

Our estimate of the squeezing force arising from stipule growth is based on the observation that much of the stipule growth occurs after the position of stipules on the pole has stabilized. In the most apical gyre of the twining helix, the vine's position on the support shifts with growth and the nutation of the apex; but in the second gyre growth has ceased and the position of the stipules on the pole becomes fixed. Thereafter, the expansion of each stipule results in a simple radial displacement of the vine away from the pole, with no repositioning of the point of contact (see video 2 in the electronic supplementary material). In this scenario, the most important elastic effect is the stretching resulting from the longer path taken by the vine around its support.

The true deformation of the vine in response to stipule extension involves bending and twisting as well as stretching, and it would be possible to compute the force on the support taking into account all modes of deformation and solving the resulting three-dimensional thin rod problem using one of a variety of numerical methods (da Fonseca & de Aguiar 2003; Goriely & Neukirch 2006). However, we feel that a more precise model would yield little additional insight relevant to twining growth in nature given the irregularity of natural supports. Instead, we computed a lower bound on the normal force per length produced by stipule expansion based on stretching alone. The strain based on a stipule height δ is

$$ϵ=\frac{2}{\lambda }\left(\sqrt{{(a+\delta )}^2-a^2+{(\alpha c)}^2}-\alpha d\right),$$ (2.5)

where λ is the internode length; a is the pole radius; c is the pitch (vertical distance per radian); d is the arc length per radian (figure 2); and α=cos⁻¹[a/(a+δ)] is the angle around the axis of the support where the vine is out of contact with the pole on either side of the stipule (see figure A2 in the electronic supplementary material).

We compute the tension from stretching of the gradually stiffening stem by assuming that stiffening during development has no independent effect on the tension in the vine, but rather that an increment in strain ϵ increases the tension given the existing stiffness S. Our reasoning is that the stretching stiffness of a herbaceous shoot arises from the elasticity of its cell walls, and the stiffening of these walls arises from the progressive deposition of wall layers. Mechanically, the system resembles a set of parallel springs, with the addition of new wall material corresponding to the addition of a relaxed spring in parallel with the existing ones. The notion of adding unstressed material to prestressed wall material is supported by observations of Hejnowicz & Borowska-Wykręt (2005), indicating that different layers of cell walls in plant cells are in different states of stress. Under this model, the tension T is given by

$$T={\int }_{t=0}^{t}S(t)\frac{\text{d}ϵ}{\text{d}t}\text{d}t.$$ (2.6)

(e) Tension-based model for twining force

Although the simple calculation above is sufficient to determine the dominant mechanism of force generation on the support, it cannot be used to compute the evolution of the load applied on the force plate for two reasons. First, the strain imposed by the growth of the stipules develops over a period of several days during which the Young modulus of the vine is also evolving. Thus, our computation of the tension must take the gradual increase in vine stiffness into account. Second, the force plate is not a rigid structure—as the stipule grows, the plate yields slightly, relaxing some of the stretching in the vine. Therefore, we measured the effective spring constant of the force plate by deflecting it with an S-beam load cell (Futek LSB200, as above) and measuring its displacement in images captured with a camera (Pixelink PL-A782) through a microscope tube (10X, yielding 5 µm px⁻¹). This experiment indicated that deflection was proportional to force with a spring constant k_p=2 N mm⁻¹. We computed the force on the force plate imposed by the total tension in the vine where it passed from pole to force plate on both sides according to a force balance between the vine and the load cells; we assumed that this tension was not effective in cases where the vine connected the force plate to the vine on the top or bottom of the sensor because the vine could not follow the deflection of the plate. Similarly, we assumed that the natural torsion of the vine would be ineffective in following the deflection of the plate once the vine formed a closed curve over the plate. Taking into account the spring constant k_p of the force plate, the force on the plate is

$$F=2\frac{a}{d}\left(\frac{\mathrm{\Delta }l}{\lambda }\right){\left(\frac{1}{S}+\frac{4}{k_p\lambda }\right)}^{-1},$$ (2.7)

(see the electronic supplementary material for details of the calculation). In the limit where k_p≫S/λ, the behaviour with a rigid support is recovered.

3. Results

(a) Development of squeezing forces during twining growth

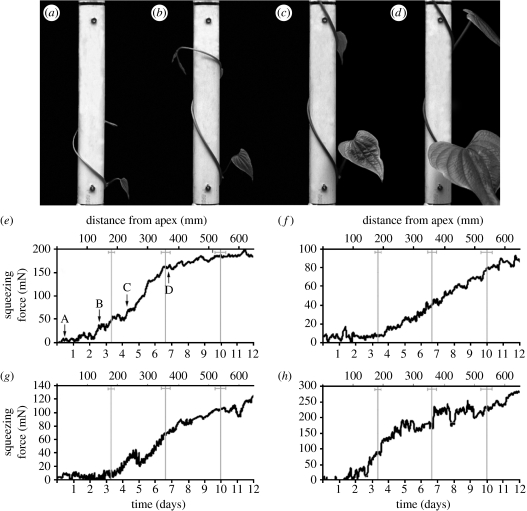
The squeezing force was small until at least one-half gyre of the vine spanned the force plate, after which the force began to rise sigmoidally over a period of approximately 10 days (figure 3e–h; see video 1 in the electronic supplementary material). Time-lapse photography showed that the time for the formation of a single gyre was about 3 days, consistent with the stem elongation rate (2.25 mm h⁻¹) and the average arc length of a gyre on the pole (180±11 mm, n=23). Recorded forces stabilized at 100–300 mN after the completion of approximately three gyres above the bottom of the force plate.

Figure 3.

Development of twining shoot and increase in squeezing force for four stems. (a–d) Stem twining around the force plate (half-pole held in place by two load cells). (e–h) Development of the squeezing force (running average of 12 values, or 2 hours interval) as a function of time and arc length from the shoot apex to the lower edge of the sensing region. Force was proportional to the total output of two load cells holding the force plate. (e) An arrow indicates the position corresponding to the time of each picture (a–d) for the first plant. The positions where gyres are completed are shown by grey vertical lines (±1 standard deviation; standard deviation increases because error accumulates with gyre number).

(b) Stipule expansion, stem growth and stem stiffness

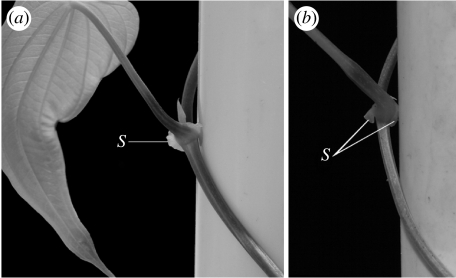
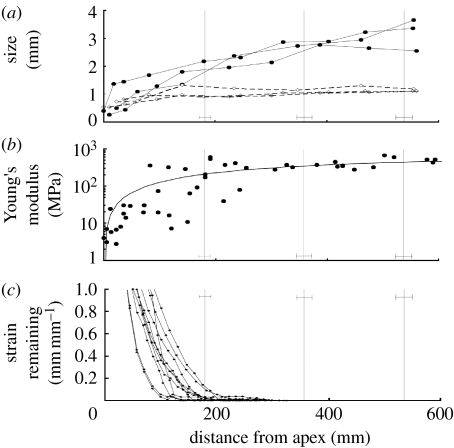
Rigid, flange-like stipules create discrete contact points where the stem is pushed away from the pole (figure 4a,b). Stipule expansion is concomitant with the force increase, both spanning a 600 mm region (about three gyres) just below the apex (figure 5a). At maturity, stipules extend 2–3 mm on both sides of the stem. Stem diameter, however, remains stable beyond approximately 150 mm from the apex (along the first gyre; figure 5a). The stem Young's modulus E increases by more than two orders of magnitude to reach an average value of 624 MPa at 600 mm from the apex (figure 5b).

Figure 4.

(a) Stipule (S) developing at the petiole base in D. bulbifera. (b) The growth of the stipule pushes the stem away from the support.

Figure 5.

Stipule growth, stem radius, the Young modulus and growth strain remaining along the stem of D. bulbifera. (a) Stipule expansion and stem radius along three twining stems. Stipule size is measured as the distance from the stem to the edge of the stipule (filled circles, stipule; open diamonds, stem radius). (b) Stem Young's modulus versus distance from shoot apex for five stems. (c) Growth strain remaining (see §2) versus distance from apex for 10 circumnutating plants.

Stipules can contribute to the twining force by putting the stem in tension as long as their expansion continues after stem elongation has ceased; otherwise the lengthening of the stem due to growth would relax the tension induced by the expansion of stipules. The remaining strain (i.e. the elongation growth that a small stem element still has to experience before it exits the growth zone) falls to zero at approximately 200 mm from the apex (figure 5c), indicating that stem elements have reached their final length there. Therefore, stem elongation ceases prior to the development of much of the squeezing force and prior to the full expansion of stipules.

(c) Force per length from twisting and bending

When D. bulbifera stems were removed from supports, the mean change in torsion was 1.70±0.27 rad m⁻¹ (n=10), while the change in curvature was not significantly different from zero (0.003±0.067 rad m⁻¹, n=10). Spring measurements yielded a Young modulus of 690±100 MPa (n=10) and an effective shear modulus of 248±33 MPa (n=10). From these values, we infer that the force-per-length p_n contributed by the twisting and bending of the vine on an ideal support is 2.96±0.55 N m⁻¹ (n=10; equation (2.3)).

(d) Force per length from stretching by stipules

In the mature stem (greater than 200 mm from apex), internode length λ was 82.8±2.7 mm (n=4, 12–29 leaves from each stem), and the vine geometry was described by a=17.65 mm and c=30.14 mm (figure 2). Raw data for stipule height δ plotted as induced strain (equation (2.5)) gave an ultimate strain of 0.744 per cent. Given this strain and the measured Young modulus, the predicted stem tension at maturity is thus 15 N, corresponding to a force-per-length of 218 N m⁻¹ of a vine spontaneously stretched to the ultimate strain on an ideal (perfectly rigid) support. It is therefore clear that stipule-induced stretching provides a squeezing force well above those due to twisting and bending.

(e) Tension-based model for twining force

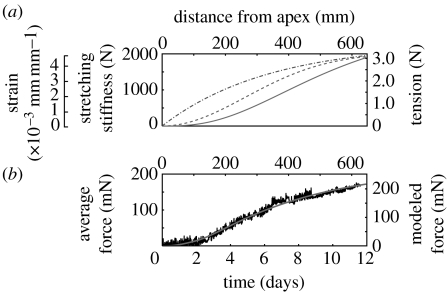
Our more detailed calculations, including the gradual development of stem mechanical properties and the growth of stipules, predict that the tension in the vine develops further along the axis than either the strain or the stretching stiffness (figure 6a), because an increment of strain has a greater effect later in development (when the stretching stiffness S is larger, equation (2.6) and figure 5b). Taking into account the compliance of the force plate as well (see figure A3 in the electronic supplementary material), our calculations from the geometry and elastic properties of the vine (equation (2.6)) predict the shape of the force curves and the order of magnitude of the observed force with surprising accuracy (figure 6b; the predicted force is 0.58 standard deviations above the mean curve).

Figure 6.

Model for development of tension in stem of D. bulbifera. (a) Strain (ϵ, equation (2.5)) and stretching stiffness (S, equation (2.1)) based on data, and predicted tension (T, equation (2.6)) along the axis of a vine on a rigid cylinder. Because the tension is an integral of the stretching stiffness with the derivative of the strain, it evolves further along the axis than either the strain or the stiffness (dot-dashed line, strain; dashed line, stretching stiffness; solid line, tension). (b) Average force recorded from four plants on mechanical poles (figure 2e–h), and force predicted (solid line) by the model taking into account the compliance of the load cells and assuming the average growth rate of 2.25 mm h⁻¹.

The twist measured in Dioscorea, by producing a small normal force near the apex, provides the initial squeezing force required to ‘bootstrap’ the increase in tension along the vine axis (figure 7). To illustrate this behaviour, we made a physical analogue of a vine using a helical rod of elastomer. We chose the helix to have an inner diameter slightly smaller than the PVC support, so that a small normal force was produced on this support by the natural twist of the artificial vine. We show that it is possible to apply strong tension in the middle of the artificial vine without dislodging it (see video 3 in the electronic supplementary material), in much the same way a real vine can sustain a tension. The governing law for the tension along a helically wound rope or stem dictates that the gradient of tension at any point along a gyre can at most equal the local tension T(s) times the stem curvature κ and friction coefficient μ (Silk & Holbrook 2005):

$$\frac{\text{d}T}{\text{d}s}\le \mu \kappa T(s).$$ (3.1)

In other words, the tension along the vine can grow, at most, at an exponential rate. Because stem tension produces a normal force that stabilizes the vine, it is possible for a twining plant to build ever greater tensions once a small normal force has been established in the most apical gyre. To improve the stability of the vine, the growth of stipules must be such that the inequality above is respected. Rapidly expanding stipules just below the apex would simply cause the stem to unravel. Based on the helical geometry in our experiments, a rigid support, and a frictional coefficient of approximately 1 (Silk & Holbrook 2005), the gradient in tension (derivative of tension in figure 6a) matches this frictional limit at approximately 200 mm from the apex, just after the cessation of elongation growth, and continues to decrease thereafter. Therefore, stipules grow just gradually enough to prevent slipping even on a rigid and smooth support.

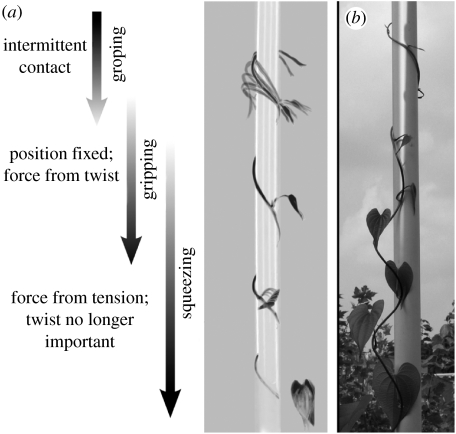
Figure 7.

Principle of squeezing by tension in a twining vine. (a) The most apical portion of the vine moves freely and contacts the support only periodically (image is average of 100 frames of a time-lapse video). Further from the apex, the position of the vine on the support becomes fixed, and a small force is applied by twist in the vine. Finally, this small force starts a frictional interaction that allows the accumulation of axial tension along the vine axis during development. (b) Gently uncoiled twining stem of D. bulbifera still held in place by its most apical gyre. Frictional interactions provide stability under tension. The gravitational load of the uncoiled stem acts similar to the tension in a twining stem.

4. Discussion

The rigid, flange-like stipules of D. bulbifera and its relatives (Burkill 1960; Acevedo-Rodríguez 2005) are distinctive structures in that their sole apparent function is to place the stem under tension. Burkill (1960) noted that stipule growth is delayed until the leaves are ‘below the horizon of twining’ and speculated that they might play a functional role; our model shows how the delay allows the stipules to stabilize the vine. Yet the stipules of Dioscorea, while themselves unusual, reveal a general and, to our knowledge, previously undescribed mechanism for generating squeezing force in vines. Because it is far easier to deflect a thin rod by some distance than to stretch it by the same amount, stretching can produce large forces from very small displacements (Love 1927; Landau & Lifshitz 1986). Our results indicate that small but mechanically dominant stretching of the stem imposed by stipule growth accounts for the shape and magnitude of the twining force applied by D. bulbifera stems on our mechanical poles, whereas both bending and twist produce forces too small to account for the generation of the measured force. This arises from the geometry of twining helices, and not from any special material properties of the vine; our mechanical measurements indicate that a D. bulbifera stem behaves like an isotropic, isovolumetric rod of round cross-section (§2: twist and bend calculations).

Stipules can only account for the later increase in the squeezing force, because in the most apical region the vine is still moving on the pole (groping region, figure 7a). As important as the stipules are, it is clear that they cannot operate alone because the stipules produce a normal force by putting the vine in tension, which seems problematic given that the apical end of the stem is free. The solution to this paradox, however, is known to anyone who has tied a rope under tension to a rigid support. After one turn of the rope around the support, the tension required to hold it in place is much reduced. By the time two turns are made, the tension is nearly imperceptible to someone holding the free end. The explanation for the abrupt decline in tension is simple; the tension in the rope produces a normal force against the support which, in turn, increases the frictional force that keeps the rope from slipping. The high tension applied at one end of the rope thus declines rapidly in the region where the rope is in contact with the support. In D. bulbifera, the small force from twist near the apex allows the later development of a large force from tension by bootstrapping the interaction between tension and friction along the axis (equation (3.1)).

The growth of stipules along the stem axis in D. bulbifera in some ways resembles thickening of the stem. As previously suggested, secondary growth of the host plant or the twining plant itself might tighten the helix later in development (Putz & Holbrook 1991). This mechanism indubitably accounts for large squeezing forces much later in twining plants' development. However, radial expansion of vine stems in the most apical gyres, where attachment to the host is critical, has not been considered important. Two insights from the present study suggest that radial expansion and structures with the effect of thickening, similar to stipules, may be a major contributor to the squeezing force in twining vines. First, in D. bulbifera, after the first gyre the stem does not slide on the support (see video 2 in the electronic supplementary material): the stipules expand gradually enough that tension can gradually build up through positive feedback with friction (equation (3.1)). Second, because stipule expansion is concomitant with stiffening of the stem, a small incremental expansion has a greater effect later in development (figure 6a). This means that the tension in the stem can continue to increase greatly even as expansion becomes less noticeable. Together, these observations suggest that a modest increase in stem diameter away from the apex, if sufficiently gradual to avoid slipping, can produce large tensions in a twining vine.

Without tangential slipping, stem thickening in a twining stem can produce a strain proportional to the radial expansion divided by the support radius; even a radial expansion of one-ten-thousandth of the support radius can lead to significant tensions. For example, by our data, the effective stretching of the stem imposed by stipules was 613 μm for each 82.8 mm internode (strain of 0.74%), equivalent to a total of 131 μm of diameter increment on a rigid support (equation (2.5)). Although producing a large squeezing force, this displacement would be hard to detect by eye. Because all plants exhibit some degree of radial expansion from the apical meristem through the growth zone, it seems worthwhile to revisit the role of radial expansion in generating force in twining vines.

In D. bulbifera, the most obvious deformation of the vine, the stored twist, contributes less to the squeezing force than a small effective contraction generated by the expansion of stipules. We were alerted to the importance of stretching in D. bulbifera because of the macroscopic expansion of the stipules, but less striking structures, such as petiole bases, or even stem expansion, could induce strong tension in the same way. To establish the generality of the tensioning mechanism among twining plants, we performed an extensive search of the botanical literature. Although many twining plants do not have stipules, we have found that other appendages, such as petioles, or even small stem ornamentation, such as winged stems, ridges, or bark extensions, will often serve an equivalent function (see figure A4 in the electronic supplementary material). To our knowledge, von Sachs (1882) provided the first report that petioles in twining plants are often trapped between stem and support but he and following researchers apparently overlooked the significance of this observation for the development of high squeezing forces and the stability of twining vines. Although long ignored in the consideration of twining plants, slight stem expansion, leaf petioles and other stem appendages can make a major contribution to the stability of twining plants.

Supplementary Material

Additional methods and calculations (Figures A1–A3)

Full description of the twining force device and detailed calculations of twist and bend, strain and force with support compliance

Figure A4

Examples of appendages that can produce tension in twining species

Video 1. Dioscorea bulbifera twining around a mechanical pole concomitantly with development of squeezing force for 6 days (data from figure 3b–e)

Squeezing force starts to develop on day 2 after the first gyre has been formed around the support, and increases over time during the formation of new gyres above the force plate. (20 fps with 10 min frame interval)

Video 2. Expansion of D. bulbifera stipule with time

The vine position on the support initially shifts with growth of the apex but stabilizes before full development of the stipule. Expansion of the stipule thus results in a radial displacement of the vine stem away from the support

Video 3. Vine physical analogue made by casting a silicon elastomer in helically wrapped tubing with inner diameter slightly smaller than the PVC pipe

The video shows that this helical rod can sustain large tension without being dislodged. This is explained by small normal forces near the apex that ‘bootstrap’ the tension along the rod, as happens in a real vine