Dynamic cerebral autoregulation during passive heat stress in humans

Abstract

This study tested the hypothesis that passive heating impairs cerebral autoregulation. Transfer function analyses of resting arterial blood pressure and middle cerebral artery blood velocity (MCA V_mean), as well as MCA V_mean and blood pressure responses to rapid deflation of previously inflated thigh cuffs, were examined in nine healthy subjects under normothermic and passive heat stress (increase core temperature 1.1 ± 0.2°C, P < 0.001) conditions. Passive heating reduced MCA V_mean [change (Δ) of 8 ± 8 cm/s, P = 0.01], while blood pressure was maintained (Δ −1 ± 4 mmHg, P = 0.36). Coherence was decreased in the very-low-frequency range during heat stress (0.57 ± 0.13 to 0.26 ± 0.10, P = 0.001), but was >0.5 and similar between normothermia and heat stress in the low- (0.07–0.20 Hz, P = 0.40) and high-frequency (0.20–0.35 Hz, P = 0.12) ranges. Transfer gain was reduced during heat stress in the very-low-frequency (0.88 ± 0.38 to 0.59 ± 0.19 cm·s⁻¹·mmHg⁻¹, P = 0.02) range, but was unaffected in the low- and high-frequency ranges. The magnitude of the decrease in blood pressure (normothermia: 20 ± 4 mmHg, heat stress: 19 ± 6 mmHg, P = 0.88) and MCA V_mean (13 ± 4 to 12 ± 6 cm/s, P = 0.59) in response to cuff deflation was not affected by the thermal condition. Similarly, the rate of regulation of cerebrovascular conductance (CBVC) after cuff release (0.44 ± 0.22 to 0.38 ± 0.13 ΔCBVC units/s, P = 0.16) and the time for MCA V_mean to recover to precuff deflation baseline (10.0 ± 7.9 to 8.7 ± 4.9 s, P = 0.77) were not affected by heat stress. Counter to the proposed hypothesis, similar rate of regulation responses suggests that heat stress does not impair the ability to control cerebral perfusion after a rapid reduction in perfusion pressure, while reduced transfer function gain and coherence in the very-low-frequency range during heat stress suggest that dynamic cerebral autoregulation is improved during spontaneous oscillations in blood pressure within this frequency range.

the maintenance of cerebral perfusion is critical for preserving cerebral function (26, 38). Cerebral blood flow is typically maintained relatively constant over a wide range of perfusion pressures, a phenomenon known as cerebral autoregulation (26, 28). Heat stress (HS) results in a range of thermoregulatory and cardiovascular adjustments to maintain internal temperature and blood pressure within safe limits (18, 30). The effect of HS on the cerebral circulation, however, has not been extensively examined. HS-induced hyperthermia decreases cerebral perfusion in the resting human (40, 41), which is not entirely accounted for by concurrent reductions in arterial carbon dioxide tension (40). Furthermore, orthostatic stress caused a greater reduction in cerebral perfusion that was accompanied by a larger increase in cerebral vascular resistance when individuals were heat stressed (40), which is suggestive of impaired static cerebrovascular autoregulation. Consistent with this observation, HS causes profound reductions in orthostatic tolerance (2, 17, 20, 35, 41). Despite observations of reduced steady-state cerebral perfusion and possibly static cerebral autoregulation (40) during HS, little is known regarding the effects of HS on dynamic cerebrovascular autoregulation, which assesses both the overall effectiveness of autoregulation, as well as the latency between changes in perfusion pressure and corresponding changes in cerebral perfusion (26, 37, 38).

Work by Doering and colleagues reported an increase in a dynamic cerebral autoregulation index to a brief hypotensive challenge in HS humans (12). However, the HS imposed in that study was mild (i.e., ∼0.4°C increase in internal temperature). It may be that, when subjects are exposed to a more severe HS (i.e., following prolonged exercise in the heat, exposure to hot climatic conditions, etc.), dynamic cerebral autoregulation is impaired, which may contribute to heat-related syncope when individuals are upright. Therefore, the aim of this study was to test the hypothesis that passive HS reduces dynamic cerebral autoregulation. This aim was achieved by assessing dynamic cerebral autoregulation using two methods, specifically, 1) examining the relationship between spontaneous changes in arterial blood pressure [mean arterial pressure (MAP)] and associated changes in cerebral blood velocity (V_mean) using transfer function analyses; and 2) evaluating the relationship between changes in cerebral V_mean relative to blood pressure in response to a brief hypotensive challenge (rapid deflation of previously inflated bilateral thigh cuffs).

METHODS

Subjects.

Nine (4 men and 5 women) subjects participated in this study. The subjects' mean ± SD age, height, and weight were 34 ± 7 yr, 175 ± 9 cm, and 77 ± 14 kg, respectively. All subjects were healthy and free from cardiovascular, cerebrovascular, and metabolic diseases. The phase of the menstrual cycle of the female subjects was not controlled. Subjects refrained from alcohol and exercise 24 h and caffeine 12 h before the study. Study procedures were approved by the Institutional Review Boards of the University of Texas Southwestern Medical Center and Presbyterian Hospital of Dallas. Institutional approved, written, informed consent was obtained from all participants before enrolling in the study. The data from the present study were collected simultaneously with data previously reported that assessed the effect of HS on cerebral CO₂ responsiveness (21). However, blood pressure and cerebral V_mean data for this protocol were collected separately from the data obtained during manipulations in arterial CO₂ performed in the cited study (see Experimental protocol below).

Instrumentation and measurements.

Each subject was dressed in a water-perfused, tube-lined suit (Med-Eng, Ottawa, Canada) that covered the entire body, except the head, face, hands, feet, and one forearm. The water-perfused suit permitted the control of skin and core temperature by changing the temperature of the water perfusing the suit. Un-inflated, large pressure cuffs (Aspen Laboratory, Englewood, CO) were placed underneath the suit around the upper thigh of each leg. Core temperature was measured from an ingestible pill telemetry system (HQ, Palmetto, FL). The pill was ingested immediately on arrival at the laboratory, which was ∼2 h before the onset of HS data collection. Mean skin temperature was measured via the weighted average of six thermocouples attached to the skin (36). Heart rate was obtained from an electrocardiogram (HP Patient Monitor, Agilent, Santa Clara, CA) interfaced with a cardiotachometer (CWE, Ardmore, PA). Continuous beat-by-beat arterial blood pressure was recorded from a finger using the Penaz method (Finometer, Finapres Medical Systems, Amsterdam, the Netherlands). Intermittent arterial blood pressure was also measured by auscultation of the brachial artery via electrosphygmomanometry (SunTech, Raleigh, NC). Skin blood flux was measured via laser-Doppler flowmetry using an integrating flow probe (MoorLAB Laser Doppler Perfusion Monitor, Moor Instruments, Wilmington, DE) attached to the forearm not covered by the water-perfused suit. Cutaneous vascular conductance was indexed from the ratio of laser Doppler flux to MAP. Middle cerebral artery (MCA) V_mean was continuously measured using transcranial Doppler ultrasonography. A 2-MHz Doppler probe (Multiflow, DWL Elektronische Systeme, Singen, Germany) was adjusted over the temporal window until an optimal signal was identified. The probe was then fixed using a mold constructed of polyvinylsiloxane impression medium and held in place using a headband strap to prevent subtle movements of the Doppler probe. Cerebrovascular conductance (CBVC) was estimated from the ratio of MCA V_mean to MAP. Subjects were instrumented with a two-way valve attached to a mouthpiece from which end-tidal Pco₂ (Pet_CO2) was continuously measured (VitalCap Capnograph Monitor, Oridion, Needham, MA).

Experimental protocol.

Experiments were performed in a temperature-controlled laboratory (26 ± 1°C) in the morning or early afternoon at least 2 h postprandial. After instrumentation, subjects rested for ∼30 min in the supine position under normothermic (NT) conditions. Water at 34°C was perfused through the suit during this period. After this resting period, 6 min of quiet baseline data were collected during spontaneous respiration, followed by arm cuff measures of arterial blood pressure. The thigh cuffs were then rapidly inflated to a preset suprasystolic pressure (minimum of 40 mmHg above previously obtained systolic blood pressures) and maintained for 5 min. At the end of the occlusion period, both cuffs were rapidly deflated, and data were subsequently collected for 5 min. This leg cuff inflation/deflation maneuver was then repeated. Before each leg cuff inflation maneuver, the water-perfused suit distal to the thigh cuff was removed to reduce the possibility of injuring ischemic skin during the heating procedure. Thereafter, as part of a separate study to address an unrelated question (21), subjects breathed 5% CO₂ for 5 min and hyperventilated for 30 s to transiently manipulate arterial CO₂ levels. Subjects were then exposed to a HS by perfusing 50°C water through the suit for a duration (∼45–60 min) sufficient to increase core temperature ∼1.0°C. Once subjects had reached this target core temperature, the temperature of the water perfusing the suit was gradually lowered to ∼44°C to limit further increases in core temperature during the ensuing data collection periods. The aforementioned baseline data collection period and the two bilateral leg cuff maneuvers were then repeated. For both thermal conditions, manipulation of arterial CO₂ levels (21) was always performed after the baseline and leg cuff inflation/deflation data were collected.

Data analysis.

Data were sampled at 200 Hz via a data-acquisition system (Biopac System, Santa Barbara, CA) and analyzed using a statistical software package (SigmaStat 3.11, Chicago, IL). Data from the last 60 s of each baseline period were averaged for steady-state statistical analyses. Respiratory rate was calculated from breath-by-breath measures of Pet_CO2 data. The MCA V_mean, MAP, Pet_CO2, and respiratory rate responses during baseline NT and HS were compared between thermal conditions using paired T-tests. Where any of these or other data sets did not conform to a normal distribution, a Wilcoxon sign-ranks test was used instead of a paired T-test. All values are reported as means ± SD. P values of < 0.05 were considered statistically significant.

Transfer function and spectral analysis.

Beat-to-beat MAP and MCA V_mean were obtained by integrating analog signals within each cardiac cycle and were then linearly interpolated and resampled at 2 Hz for spectral analysis (42). These data were linearly interpolated and resampled at 2 Hz to convert the unequally spaced beat-to-beat time series to a uniformly spaced time series for spectral and transfer function analyses. The resampling frequency was determined based on the Nyquist theorem (29). The frequency range evaluated was 0–0.5 Hz. Transfer function gain and phase were calculated as previously described (42). The transfer gain and phase reflect the relative amplitude and the time relationship between the changes in MAP and MCA V_mean, respectively, over a specified frequency range. In particular, transfer estimates of gain reflect the relative amplitude between the changes in the input and output signals of the system, with a high gain reflecting reduced autoregulation. Transfer estimates of phase describe the temporal shift required to align the input signal (blood pressure) with the output signal (MCA V_mean). For a working autoregulation output will lead input, whereas, for less effective autoregulation, this phase difference will be reduced (4, 42). Furthermore, the coherence function was calculated to assess the linear relationship between these two variables. A coherence approaching 1 in a specific frequency range suggests a linear relationship between two signals within that frequency range, whereas a coherence approximating 0 may indicate a nonlinear relationship, severe extraneous noise in the signals, or simply no relationship between signals. The spectral power of MAP and MCA V_mean, as well as mean values of transfer function gain, phase, and coherence, were calculated in the very-low- (0.02–0.07 Hz), low- (0.07–0.20 Hz), and high-frequency (0.20–0.35 Hz) ranges. These ranges were specifically chosen to reflect the different characteristics of the dynamic pressure-flow relationship, as previously identified by transfer function analysis, specifically, 1) a low-frequency component (<0.07 Hz) with a low coherence; 2) an intermediate frequency component (0.07–0.20 Hz) characterized by increasing coherence, increasing gain, and decreasing phase; and 3) a high-frequency component (>0.20 Hz) with a high coherence, relatively large gain and minimal phase lead (42). Transfer function gain, phase, and coherence within each frequency range during NT and HS were statistically compared using paired T-tests. Transfer function analyses were performed with commercially available software (DADiSP, DSP Development, Cambridge, MA).

Bilateral leg cuff deflation.

For the bilateral leg cuff inflation-deflation maneuvers, the beat-to-beat MCA V_mean and MAP responses were analyzed using the technique previously reported (1, 23). Control values of MAP and CBVC were calculated from their means during the 4-s period immediately before cuff release, and all subsequent MAP and CBVC data after cuff release were expressed as a ratio of their concomitant control values. During the 1- to 3.5-s period after cuff release, the rate of change, or the rate of regulation (RoR), in CBVC is directly related to cerebral autoregulation (1). The RoR is, therefore, an index of cerebral autoregulation and was calculated as follows:

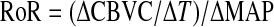where ΔCBVC/ΔT is the slope of the linear regression between CBVC and time (T), and ΔMAP was calculated by subtracting precuff release MAP from the average MAP after cuff release within the evaluated time window. The RoR values for both trials within each thermal condition were averaged.

In addition, the time to the nadir and the time to recovery of the MAP and MCA V_mean responses to the bilateral leg cuff inflation-deflation maneuvers were analyzed using a previously published approach (16). A section of 15-s data before cuff deflation was averaged to represent the precuff deflation baseline data. Beat-to-beat MAP and MCA V_mean data were sampled from the immediate onset of cuff deflation for ∼120 s. The time to reach the nadir of the MAP and MCA V_mean responses following the cuff deflation (T₁), as well as the time to recover (T₂) from the nadir to 90% of the difference between the value at T₁ and baseline were identified. The T₁ and T₂ times for both trials within each thermal condition were averaged. For both the RoR and the T₁ and T₂ analyses, responses between thermal conditions were evaluated via paired T-tests.

RESULTS

Thermoregulatory, cardiovascular, and cerebral responses to passive HS.

Passive HS caused typical thermoregulatory and cardiovascular responses (see Table 1). The group average (±SD) increase in core and mean skin temperature was 1.1 ± 0.2 and 3.7 ± 0.6°C, respectively (both P < 0.001). Heart rate increased (39 ± 12 beats/min, P < 0.001), while MAP was well maintained (−1 ± 4 mmHg, P = 0.36). Forearm cutaneous vascular conductance increased approximately fourfold (2.01 ± 0.42 arbitrary units/mmHg, P < 0.001). HS decreased MCA V_mean (8 ± 8 cm/s, P = 0.01), while Pet_CO2 (−3 ± 4 Torr, P = 0.07) and respiratory rate were not significantly affected (+3 ± 4 breaths/min, P = 0.06).

Table 1.

Thermoregulatory, cardiovascular, respiratory, and cerebrovascular responses during normothermia and heat stress

	Condition		P Value
	Normothermia	Heat stress
Core temperature, °C	36.9±0.3	38.0±0.3	<0.001
Mean skin temperature, °C	34.8±0.5	38.5±0.4	<0.001
Heart rate, beats/min	56±9	95±15	<0.001
Blood pressure, mmHg	91±6	89±6	0.36
MCA Vmean, cm/s	69±14	60±13	0.01
Forearm CVC, AU/mmHg	0.65±0.68	2.32±0.86	<0.001
Respiratory rate, breaths/min	14±4	17±5	0.06
End-tidal Pco2, Torr	42±1	39±1	0.07

Values are means ± SD. MCA V_mean, middle cerebral artery blood velocity; CVC, cutaneous vascular conductance; AU, arbitrary units.

Time series and autospectra.

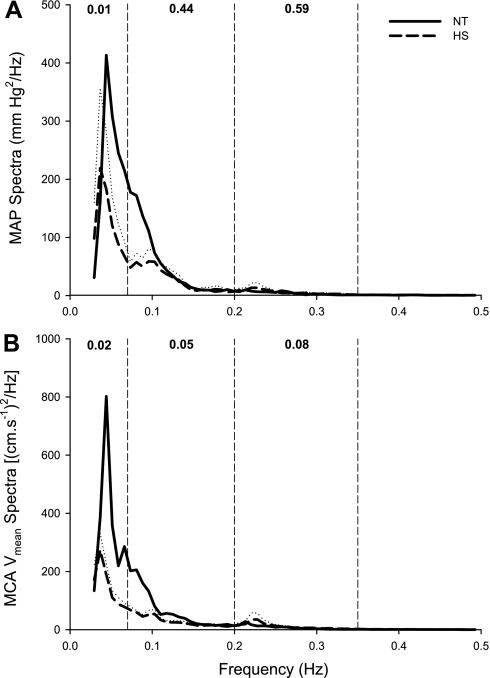
The group-averaged autospectra of beat-to-beat MAP and MCA V_mean are displayed in Fig. 1. The autospectra of arterial pressure demonstrated similar characteristics in NT and HS conditions within all frequency components. The high-frequency peak in the group-averaged spectra in Fig. 1 is blurred as a result of the average processing of individual spectra with different respiratory rates. The spectral power of MAP in the very-low-frequency range was lower during HS (7.95 ± 2.06 vs. 2.90 ± 2.29 mmHg²/Hz, P = 0.01), while spectral power of this variable was unchanged by heating in the low- (2.91 ± 1.33 vs. 2.29 ± 1.64 mmHg²/Hz, P > 0.05) and high-frequency (0.52 ± 0.56 vs. 0.69 ± 0.68 mmHg²/Hz, P > 0.05) ranges. The autospectra of MCA V_mean was decreased by HS within the very low [9.78 ± 8.67 vs. 3.02 ± 1.09 (cm/s⁻¹)²/Hz, P = 0.02] and low [4.29 ± 2.77 vs. 2.77 ± 1.19 (cm/s⁻¹)²/Hz, P = 0.05] frequency ranges. Conversely, the spectral power within the high-frequency range tended to be increased by HS [0.78 ± 0.80 vs. 1.49 ± 1.86 (cm/s⁻¹)²/Hz, P = 0.08].

Fig. 1.

Group-averaged autospectra of beat-to-beat changes in mean arterial blood pressure (MAP; A) and middle cerebral artery blood velocity (MCA V_mean; B) during normothermia (NT; solid line) and heat stress (HS; dashed line). The dotted line represents the SE for the HS data; NT SE data were omitted for clarity. The vertical dashed lines represent the cutoffs for the very-low- (0.07 Hz), low- (0.20 Hz), and high-frequency (0.35 Hz) ranges. The numbers at the top of each frequency band indicate the P values of the comparisons between NT and HS.

Transfer function analysis.

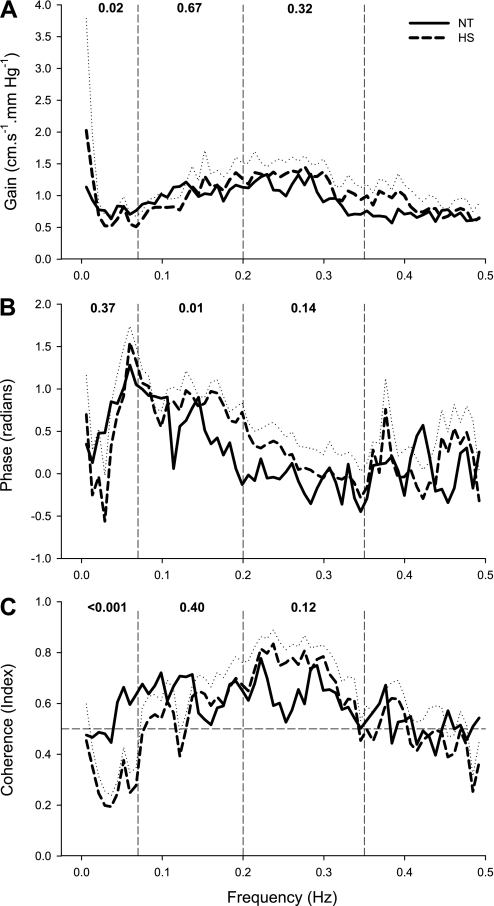
The estimates of transfer gain, phase, and coherence functions are shown in Fig. 2. In both the low- (0.64 ± 0.19 vs. 0.59 ± 0.10, P = 0.40) and high-frequency (0.63 ± 0.19 vs. 0.72 ± 0.14, P = 0.12) ranges, coherence was >0.5 and similar in both NT and HS conditions, respectively, suggesting that changes in velocity are relatively linearly related to the changes in blood pressure. In contrast, in the very-low-frequency range (<0.07 Hz), coherence was >0.5 during NT, but was <0.5 during HS (0.57 ± 0.13 vs. 0.26 ± 0.10, P < 0.001). Transfer gain was reduced by HS in the very-low-frequency range (NT: 0.88 ± 0.38 vs. HS: 0.59 ± 0.19 cm·s⁻¹·mmHg⁻¹, P = 0.02), but was unchanged in the low- (NT: 1.04 ± 0.31 vs. HS: 1.01 ± 0.24 cm·s⁻¹·mmHg⁻¹, P = 0.67) and high-frequency (NT: 1.05 ± 0.38 vs. HS: 1.24 ± 0.40 cm·s⁻¹·mmHg⁻¹, P = 0.32) ranges during HS. Phase was similar during NT and HS in the very-low- (0.92 ± 0.58 vs. 0.71 ± 0.46, P = 0.37) and high-frequency (−0.10 ± 0.20 vs. 0.12 ± 0.71, P = 0.14) ranges, whereas, in the low-frequency range, phase was significantly increased by HS (0.55 ± 0.31 vs. 0.82 ± 0.17, P = 0.01).

Fig. 2.

Group-averaged transfer function gain (A), phase (B), and coherence function (C) between changes in MAP and MCA V_mean during NT (solid line) and HS (dashed line). The dotted line represents the SE for the HS data; NT SE data were omitted for clarity. The vertical dashed lines represent the cutoffs for the very-low- (0.07 Hz), low- (0.20 Hz), and high-frequency (0.35 Hz) ranges. The numbers at the top of each frequency band indicate the P values of the comparisons between NT and HS.

Cuff inflation-deflation.

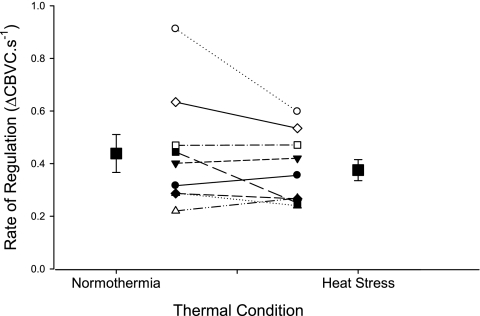
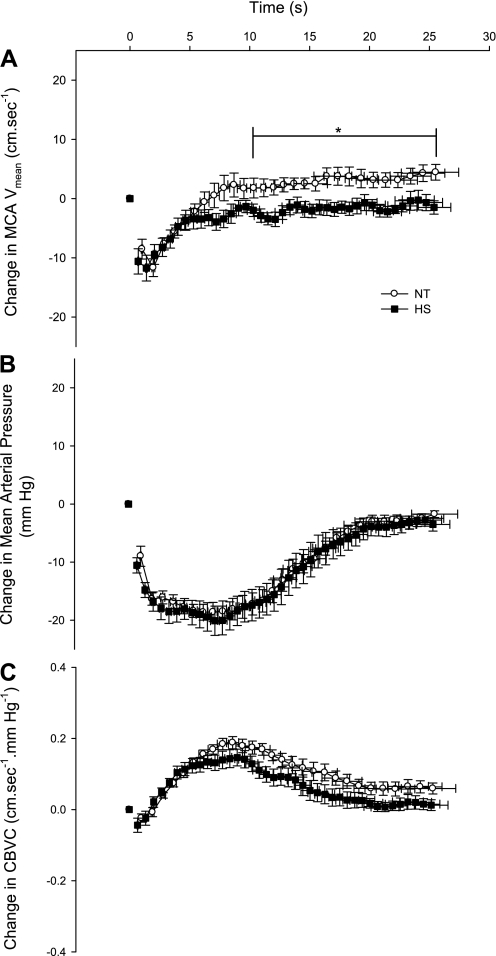
Blood pressure and MCA V_mean responses to cuff inflation-deflation during NT and HS are presented in Table 2. Blood pressure and MCA V_mean decreased in response to cuff deflation, with no difference in the magnitude of the decrease between NT and HS conditions (both P > 0.05, see Table 2). The RoR was not affected by HS (P = 0.16, see Fig. 3). For both thermal conditions, MCA V_mean decreased to its nadir (T₁) 2–3 s earlier than blood pressure T₁ (see Table 2). Interestingly, T₁ for MCA V_mean was slightly shorter during HS (P = 0.05). MCA V_mean recovered to precuff deflation baseline (e.g., T₂) faster than blood pressure for both thermal conditions, while T₂ for MCA V_mean was not affected by HS (P = 0.77, see Table 2). The time for blood pressure to decrease to its nadir (T₁) tended to be slightly shorter during HS (P = 0.07), while T₂ was similar between thermal conditions (P = 0.65, see Table 2). For the 15 s immediately after T₂, MCA V_mean exceeded precuff occlusion baseline under NT conditions, while during HS, MCA V_mean was maintained at precuff occlusion baseline (NT: 2.60 ± 3.67 vs. HS: −1.55 ± 2.54 cm/s, P = 0.02, see Fig. 4A).

Table 2.

Time to nadir and recovery for blood pressure and cerebral blood velocity during the leg cuff deflation procedure in normothermia and heat stress

	Condition		P Value
	Normothermia	Heat stress
Blood pressure
T1, s	4.75±2.1	3.92±2.12	0.07
T2, s	20.24±2.07	19.57±3.96	0.65
ΔMAP, mmHg	20±4	19±6	0.88
ΔMAP, %	21±4	22±7	0.66
MCA Vmean
T1, s	1.97±1.15	1.33±0.73	0.05
T2, s	9.99±7.92	8.74±4.88	0.77
ΔMCA Vmean, cm/s	13±4	12±6	0.59
ΔMCA Vmean, %	20±6	19±7	0.69

Values are means ± SD. T₁, time to nadir; T₂, time to recovery; MAP, mean arterial blood pressure; Δ, change in the indicated value from baseline to T₁.

Fig. 3.

The rate of regulation of cerebrovascular conductance (ΔCBVC) for each subject and the group averages (▪ with SE bars) during NT and HS.

Fig. 4.

Group-averaged decreases in MCA V_mean (A), MAP (B), and CBVC (C) during leg cuff deflation under NT and HS conditions. *P < 0.05 vs. HS.

DISCUSSION

The aim of this study was to test the hypothesis that passive HS reduces cerebral autoregulation evaluated using two methods of dynamic cerebral autoregulation assessment, that is, transfer function analyses and the RoR analyses. The main findings of this study are that HS reduced transfer function gain and coherence in the very-low-frequency range, did not change transfer function gain and coherence within the low- and high-frequency ranges, and did not affect the RoR or the recovery time of the MCA V_mean in response to thigh cuff deflation. Taken together, these findings indicate that, counter to the proposed hypothesis, HS does not impair dynamic cerebrovascular autoregulation.

The observed transfer function gain responses suggest that the ability of the cerebral vasculature to attenuate changes in MCA V_mean in the face of spontaneous changes in blood pressure, within the low- and high-frequency ranges, is unaffected by HS. In contrast, in the very-low-frequency range, transfer function gain was reduced during HS, indicating that smaller changes in MCA V_mean occurred for the same change in blood pressure, e.g., possibly of improved dynamic autoregulation within this frequency range. However, coherence within the very-low-frequency range was greatly reduced by HS, thereby reducing the confidence of this gain calculation (see discussion below). Similarly, HS did not affect transfer estimates of phase in the very low and high frequencies, while phase was increased in the low-frequency range, in contrast to our proposed hypothesis. This latter observation could be interpreted as an improvement in dynamic cerebral autoregulation, similar to an increase in gain in the very-low-frequency range. Data suggestive of improved dynamic autoregulation with HS was unexpected, and the mechanism(s) leading to these responses is unknown. Recent research has reported that sympathetic blockade impairs dynamic cerebral autoregulation, as evidenced by increases in transfer function gain and reductions in transfer function phase (43) and reductions in the cerebral RoR (23). In contrast, passive HS causes pronounced elevations in sympathetic activity (7–9, 11, 31–33). Therefore, HS-induced increases in sympathetic activity, assuming such occurs in the cerebral circulation during HS as recently proposed (40), could have contributed to indexes of improved cerebrovascular autoregulation. In addition, previous studies have reported improved dynamic cerebral autoregulation (increases in transfer function phase) during hypocapnia in NT subjects (3, 13). Although relatively small, HS-induced decrease in Pet_CO2 in the present study could have contributed to improved dynamic cerebral autoregulation within the specified frequency ranges. It is unclear why these observations only occurred in selective frequency ranges (i.e., reduced gain in the very-low-frequency range and increased phase in the low-frequency range). Overall, these observations, coupled with the absence of changes in transfer function gain and phase in other frequency ranges, suggest that, at the very least, HS does not impair cerebrovascular autoregulation.

In the very-low-frequency range, estimates of coherence were lower during HS relative to NT. There are conflicting approaches to the interpretation and application of coherence values. Coherence is proposed to provide an estimate of the statistical reliability of the transfer function estimate of gain and phase (4), with a value of 0.5 typically used as the lower threshold to accept transfer function estimates of gain and phase (26). Alternatively, it has also been put forward that coherence reflects the strength of the linear relationship between arterial blood pressure and MCA V_mean and thus is itself an indicator of dynamic cerebral autoregulation (e.g., for an effective autoregulation, changes in MCA V_mean, in the face of changes in blood pressure, are buffered and result in a lower coherence value) (4, 14). Thus a lower coherence value during HS within the very-low-frequency range (0.57–0.26) in the present study may suggest improved autoregulation with HS, consistent with the transfer gain and phase data within some frequency ranges. It has been recently suggested, however, that univariate coherence values should not be used to reject spectral estimates of gain and phase or be used as an indicator of the extent of autoregulation, because they do not take into account a potentially increasing number of covariates and the intrinsic nonlinearity of dynamic cerebral autoregulation (27). This limitation could also be applied to transfer estimates of gain, which also assume linearity, in the very-low-frequency range. It has also been proposed, from studies of renal autoregulation in rats, that nonstationarity (a change in a system's dynamic characteristics over time) should be considered when analyzing autoregulatory systems (5, 44). The transfer function analyses employed in the present study, and by numerous others, calculates time-invariant estimates of gain and coherence, which assume that the system's dynamic characteristics are stationary. To date, no study has examined the contribution of nonstationarity to cerebral autoregulation in humans, and, despite all data being collected under stable steady-state conditions in the present study, the assumption of stationarity is a potential limitation.

Reductions in spectral power of blood pressure in the very-low-frequency range during HS are consistent with previous data from our laboratory (6, 7). Other groups have proposed that reductions in oscillations of blood pressure reflect a decrease in sympathetic modulation of vasomotor tone (22, 24, 25). In contrast to this proposal, however, we and others have clearly shown that HS causes pronounced elevations in sympathetic nerve activity (7–9, 11, 31–33), and that reductions in spectral power of blood pressure during HS are not due to a reduced oscillation of sympathetic nerve activity (7). The uncoupling between sympathetic nerve activity and blood pressure variability during HS could reflect HS-induced increases in vascular capacitance within the cutaneous circulation that could buffer the magnitude of fluctuations in blood pressure (6) and/or reductions in postsynaptic adrenergic responsiveness (10, 39). The reduction in blood pressure variability with HS could, in fact, reduce the “demand” of dynamic cerebral autoregulation during hyperthermia and thus contribute to a lack of deterioration in dynamic cerebral autoregulation evident with heating in the present study. Similarly, a reduction in cerebral V_mean spectral power in the very-low-frequency range indicates a reduction in cerebral V_mean variability and is, at the very least, in agreement with no changes in dynamic cerebral autoregulation with HS.

The thigh cuff inflation-deflation maneuver has been used to assess dynamic cerebral autoregulation in response to very rapid and pronounced reductions in perfusion pressure (1, 12, 16, 23). Following thigh cuff deflation, the magnitude of the reduction in arterial blood pressure and MCA V_mean was not different between thermal conditions. Moreover, the RoR and timing of the reduction in these variables (T₁), as well as the timing of their recovery (T₂), were not appreciably changed by HS. Findings from the leg cuff procedure are in contrast to prior findings by Doering et al. (12), who used a similar procedure to evaluate dynamic cerebral autoregulation during mild HS. These investigators reported that HS improved dynamic cerebrovascular autoregulation. However, there are some key differences between the present study and that of Doering et al. Foremost was that the HS imposed (body water immersion) in the study of Doering et al. was relatively mild (∼0.4°C increase in internal temperature), and typical responses used to further evaluate the magnitude of the HS (i.e., mean skin temperature, heart rate, skin blood flow, or sweat rate) were not reported. In contrast, in the present study, a more severe HS was imposed, resulting in pronounced elevations in these variables (see Table 1), including an average increase in core temperature of 1.1°C. Similar to the conflicting findings of the present study and that of Doering et al., when assessing dynamic cerebral autoregulation in NT subjects during lower body negative pressure, our laboratory has previously reported a reduction in dynamic autoregulation via increases in transfer function gain (44), whereas others reported no change using the thigh cuff deflation technique (16). Thus the transfer function analysis and thigh cuff deflation techniques may not always produce comparable findings.

Methodological considerations.

Orthostatic tolerance is profoundly reduced under HS, relative to NT, conditions (2, 17, 20, 35, 41). We have previously shown greater decreases in cerebral perfusion and vascular conductance during orthostatic stress in hyperthermic individuals (40), suggestive of impaired static cerebrovascular autoregulation. In the present study, dynamic cerebral autoregulation was examined with subjects in the supine position (e.g., no orthostatic stress). Should dynamic cerebral autoregulation have been assessed during the combination of heat and orthostatic stress, when cerebral perfusion can be severely compromised, then decrements in dynamic autoregulation may have been evident. However, such data are challenging to obtain, given the duration necessary to obtain the data for the evaluated analyses, coupled with substantially reduced orthostatic tolerance when subjects are heat stressed. That said, the thigh-cuff release maneuver could be viewed as mimicking the initial reduction in cerebral perfusion that is encountered during the onset of orthostatic stress, with the present findings suggesting unaltered cerebrovascular autoregulation in heat-stressed subjects. Furthermore, the hyperthermic stress imposed on subjects in the present study was moderate, given an increase in internal temperature of ∼1.1°C. Should the HS have been greater, then perhaps reductions in dynamic cerebral autoregulation may have occurred.

Transcranial Doppler measures of MCA V_mean were used to reflect changes in cerebral blood flow. This assumption is only valid if the diameter of the insonated vessel (i.e., the MCA) remains constant. Direct and indirect measurements of MCA diameters in humans have shown that these diameters do not change during a variety of stimuli, including hypocapnia, known to affect cerebral blood flow (15, 34). Therefore, it is likely that alterations in MCA V_mean reflect changes in cerebral blood flow in the present study.

In the present study, absolute transfer function estimates of gain were analyzed (e.g., expressed as cm·s⁻¹·mmHg⁻¹), while, in some studies, normalized transfer function estimates of gain (e.g., expressed as %/mmHg) are calculated (4, 13). The argument to normalize MCA V_mean data is that differences in baseline MCA V_mean could influence the estimate of transfer function gain due to potential “errors” in equating MCA V_mean to cerebral blood flow, given possible differences in the vessel diameter, as well as the angle of insonation between subjects. This is in contrast to the present protocol in which the position of the Doppler probe did not change, nor is it thought that vessel diameter changes, between thermal conditions. Therefore, absolute changes in MCA V_mean more directly track changes in cerebral blood flow with the present pre/post design, relative to between subject comparisons. It is for this reason that absolute MCA V_mean was selected in estimating transfer function gain, despite cerebral perfusion decreasing by HS. Nevertheless, when MCA V_mean data were normalized between thermal conditions, transfer gain remains unaltered in the low- and high-frequency ranges (low frequency: 1.53 ± 0.41 to 1.73 ± 0.38%/mmHg, P = 0.063; high frequency: 1.55 ± 0.55 to 2.06 ± 0.37%/mmHg, P = 0.061), although there was a tendency for an increase in these gain values by HS. In contrast, normalization of the gain data in the very-low-frequency range removed statistical significance (1.27 ± 0.50 to 1.02 ± 0.29%/mmHg, P = 0.123). When taken together, and despite our contention that normalization of the aforementioned data is less suitable, normalized transfer gain data do not support the hypothesis that dynamic cerebral autoregulation is impaired during HS.

Changes in basal cerebral vascular resistance, and subsequent alterations in myogenic vascular tone, per se, may modulate cerebral autoregulation (19). Therefore, changes in cerebral autoregulation (e.g., transfer gain and phase in the present study) between conditions with differing basal levels of cerebral vascular resistance could be partly explained by a “passive” mechanism through adjustments in baseline vascular characteristics, alongside or even instead of the differences in “active” vascular mechanisms (19).

Perspectives and significance.

The maintenance of cerebral perfusion through local vascular regulation (i.e., cerebral autoregulation) is critical to avoid precipitous reductions in cerebral blood flow. HS results in reductions in steady-state cerebral blood flow, but the effect of HS on dynamic cerebral autoregulation has not been thoroughly examined. We have shown that transfer function estimates of gain, coherence, and phase between spontaneous changes in blood pressure and corresponding MCA V_mean were not diminished, but may even be improved in certain frequency ranges during HS. Furthermore, data derived via the leg cuff release technique also suggest that HS does not alter dynamic cerebrovascular autoregulation. Taken together, a preserved dynamic cerebral autoregulation during HS would serve to attenuate reductions in cerebral blood flow during decreases in blood pressure and thus help protect heat-stressed individuals from further compromised cerebral perfusion.

GRANTS

This research was supported by National Heart, Lung, and Blood Institute Grants HL61388 and HL84072.