Casein Micelle Dispersions under Osmotic Stress

Abstract

Casein micelles dispersions have been concentrated and equilibrated at different osmotic pressures using equilibrium dialysis. This technique measured an equation of state of the dispersions over a wide range of pressures and concentrations and at different ionic strengths. Three regimes were found. i), A dilute regime in which the osmotic pressure is proportional to the casein concentration. In this regime, the casein micelles are well separated and rarely interact, whereas the osmotic pressure is dominated by the contribution from small residual peptides that are dissolved in the aqueous phase. ii), A transition range that starts when the casein micelles begin to interact through their κ-casein brushes and ends when the micelles are forced to get into contact with each other. At the end of this regime, the dispersions behave as coherent solids that do not fully redisperse when osmotic stress is released. iii), A concentrated regime in which compression removes water from within the micelles, and increases the fraction of micelles that are irreversibly linked to each other. In this regime the osmotic pressure profile is a power law of the residual free volume. It is well described by a simple model that considers the micelle to be made of dense regions separated by a continuous phase. The amount of water in the dense regions matches the usual hydration of proteins.

Introduction

Caseins are a family of proteins that make up to 80% of the protein content of cow milk. In native milk, they are associated into large globular aggregates that are called casein micelles. Their main biological function is the transport and delivery of proteins, calcium and phosphate to the young mammals (1). The composition and structure of the casein micelles have been studied for >40 years, and rather precise descriptions are available, although still controversial (2,3). They are made of four distinct caseins, α_s1, α_s2, β, and κ in proportion of 3:1:3:1, and 8% in mass of phosphate and calcium ions. The structural model accepted most widely has a roughly spherical, core-shell structure, with outer diameters ranging from 50 to 500 nm (4,5). The core is now generally described as a homogeneous web of caseins in which calcium phosphate nanoclusters are distributed randomly (6,7). The shell is essentially made of κ-caseins that extend into the aqueous phase as a polyelectrolyte brush and in this way produce short range repulsions between micelles (8).

This model has been extremely useful in providing a simplified view of the micelles as semipermanent objects, and in explaining their colloidal stability (9–12). However, it only provides a snapshot picture of an average, idealized micelle. We still need to understand how the structure and the properties of the micelles result from interactions between their components, how they can respond to changes in physical parameters or chemical composition of the system, and what the association-dissociation equilibria between micelles and nonmicellized components are (13).

To explore how interactions determine structure and properties, a general approach consists in changing these interactions and observing the changes in structure. Interesting results have been obtained by changing the physical parameters (temperature, hydrostatic pressure) and measuring the resulting changes in the average structure or in properties such as the sol-gel transition of the micellar dispersion (6,7,14–17). Further information has been obtained by changing the composition of the aqueous phase (pH, ionic strength, addition of molecules that chelate Ca²⁺ ions) or by carrying out chemical reactions within the micelles (chopping off the brush, cross-linking the core) (7,8,10,18–23).

In this study, we chose a more thermodynamic approach that consists in changing the chemical potential of water using the osmotic stress method. In this method, water is removed from the casein micelle dispersion through dialysis against a polymer solution of known osmotic pressure (24–26). After equilibrium is reached, the amount of water that is retained by the casein dispersion is measured. Similar measurements at each osmotic pressure yield the relation of osmotic pressure to casein concentration, which is the equation of state of the system. This equation of state reflects the balance of all interactions (e.g., casein-water, casein-casein, casein-calcium phosphate) in the system. Moreover, examination of the state of the system (liquid, solid, gel) and of its properties (turbidity) at various osmotic pressures yields further information regarding the structures and interactions of micelles at all concentrations, up to conditions where they have been dehydrated substantially. These results are directly applicable to industrial operations in which casein dispersions are dehydrated through filtration, centrifugation, or drying (27,28).

To our knowledge, the osmotic stress method had not been applied to casein micelle dispersions so far. Previous experiments on sodium caseinate (SC) by Farrer et al. show that the method is appropriate, i.e., the range of concentrations that can be reached through equilibrium dialysis extends from dilute solutions to very concentrated solutions (29). However, casein micelles dispersions are very different from sodium caseinate solutions, with respect to composition, structure, and interactions (30,31). In this work, we have used aqueous dispersions made from native phosphocaseinate powder (NPC) dissolved in a solvent made from ultrafiltration of skimmed milk (UF permeate). It is known that the casein micelles are quite close to their native state in such a reconstituted milk that is depleted in serum proteins (32,33). The use of UF permeate also ensured that the chemical potential of all ions were identical to their values in milk. UF permeate was also used, after addition of a water soluble polymer, as the stressing solution for osmotic stress experiments. In addition, some experiments were also carried out with a stressing solution that had a higher ionic strength. Finally, we studied the effects of osmotic stress cycles in which the casein dispersions were first deswelled to the solid state, and then reswelled with the original ultrafiltration permeate. The aim of these experiments was to provide some answers to the following questions:

• a.In dilute dispersions, is it acceptable to describe the dispersion as a collection of identical “micelles”? If not, what is the collection made of?
• b.In more concentrated dispersions, how do the members of the collection interact? Is there a significant range of concentration where the micelles repel each other through their κ-casein brush? Do they stick to each other when the water that separates them is removed, and if so, what is the casein concentration at this transition?
• c.At still higher concentrations, what is the cost of removing the water that swells each micelle, and what are the consequences of this deswelling?
• d.Are the reverse processes at all possible, i.e., is it possible to reswell and then redisperse the micelles after a deswelling stage? If not, what are the cohesive forces that oppose reswelling and redispersion?

Materials and Methods

Proteins and dispersions preparation

All experiments were done with dispersions made from casein powders (NPC, SC) dispersed in a solvent made from ultrafiltration of skimmed milk (UF permeate). We made this choice for a number of reasons:

• The use of the so-called UF permeate ensures that the chemical potential of all ions is maintained to their values in the native state.

• These casein powders lack the milk serum proteins that could interfere in the osmotic pressure measurements.

• Even if a truly native state cannot be fully guaranteed, it is generally accepted that NPC powder is an adequate model for milk casein micelles (see Huppertz et al. (16) and Muller-Buschbaum et al. (34) for some recent examples of its use). Famelart et al. have shown that, when UF permeate is used as aqueous phase for reconstitution, the main properties (micelle size distribution, behavior toward pH gelation) of NPC dispersions are practically identical to those of skimmed milk (32).

Native phosphocaseinate powders were prepared according to a protocol developed by Pierre et al. (33) and Schuck et al. (35). Briefly, skimmed milk was processed through cross-flow microfiltration (0.1 μm) to separate the casein micelles from the serum proteins. The retentate was washed with 4 volumes of pure water in diafiltration mode. It was then dried in low-temperature conditions through spray-drying. Two batches of powder (NPC powder 1 and 2), prepared from different skimmed milks and at different dates, were used in this study.

Sodium caseinate powder was produced by Armor Protéines (Saint-Brice-en-Coglès, France) according to a protocol similar to that described by Segalen et al. (36). First, the casein micelles from fresh skimmed milk were precipitated through acidification to the isoelectric point of casein at pH 4.6. The acidification also dissociates the calcium phosphate nanoclusters from the micelles (7). Then the precipitated acid casein curd was washed with pure water, re-dissolved in sodium hydroxide solution, and dried through spray-drying.

The composition of the NPC and SC powders are given in Table 1. Caseins and their associated minerals are the main components (total mass >90% of the total solid content). As is usually done for dairy products, the average noncasein and nonprotein nitrogen contents were determined as described in Gaucher et al. (37). The noncasein nitrogen matter is the equivalent protein fraction that does not precipitate at pH 4.6. Pierre et al. (33) showed that this fraction consists mainly of proteose-peptones (i.e., casein fragments of molar mass ∼20,000 Da) that associate into small aggregates when in solution. The rest includes serum proteins (β-lactoglobulin, α-lactalbumin, bovine serum albumin (BSA), immunoglobulin G (IgG), phospholipoproteins) and peptides that were not eliminated through the washing step. The nonprotein nitrogen matter is the equivalent peptide fraction that does not precipitate at extreme acidic condition (15% (w/v) trichloroacetic acid solution). It is usually accepted that most of the small peptides (i.e., molecular mass <10,000 Da) present in solution are found in this fraction (37,38).

Table 1.

Composition of NPC and SC powders

	TS (%, w/w)	Minerals (% TS)	Caseins (% TS)	Noncasein nitrogen matter (% TS)	Nonprotein nitrogen matter (% TS)
NPC 1	90.4	8.1	85.0	5.7	0.6
NPC 2	91.0	8.5	85.6	4.6	0.6/1.8∗
SC	93.4	<3.9	95.7	1.2	0.4/0.6∗

All values are given as averages ± 0.2%. NPC, native phosphocaseinate; SC, sodium caseinate; TS, total solid.

^∗Values determined for NPC and SC dispersions in UF permeate at ∼25 g/L of caseins and after 7 days of incubation at 20°C; for details, see the description of the osmotic stress technique. Details about the peptides present in this fraction are provided in the Supporting Material.

The UF permeate solvent was prepared through membrane ultrafiltration (5000 Da cutoff) of a fresh skimmed milk. Its average ionic composition is: ∼20 mM Na⁺, ∼40 mM K⁺, ∼10 mM Ca²⁺, ∼30 mM Cl⁻, ∼10 mM phosphate, ∼10 mM citrate (see Jenness and Koops (39) for a full description). It also contains lactose (∼150 mM) and a few other low molar mass molecules such as riboflavin, a vitamin that gives it a distinctive yellow color. Thimerosal and sodium azide, both purchased from Sigma-Aldrich (St. Louis, MO), were added to the UF permeate as preservatives at 0.02% and 0.1% (w/w) respectively. The NPC dispersions were prepared by thoroughly mixing the NPC powder in UF permeate for 15 h at 35°C. It has been shown by Gaini et al. (40) that such conditions are sufficient to fully dissociate the protein aggregates that are present in the powder. The SC dispersions were also prepared by mixing for 15 h. In that case, a slightly higher temperature (50°C) was needed for complete dissociation of the SC powder. After total dissolution, the pH of the dispersions was 6.7 ± 0.1 at 20°C, i.e., pH of a fresh skimmed milk.

Experiments at modified ionic strengths were done with dispersions prepared from UF permeates in which NaCl (Sigma-Aldrich) was added at 100 mM or 300 mM. It was necessary in these cases to slightly correct the pH of the final dispersions by addition of drops of a 0.1 M NaOH solution to reach 6.7 ± 0.1 at 20°C.

Osmotic stress technique

The osmotic stress technique is based on water exchange between the sample (i.e., a colloidal dispersion) and a reservoir of known osmotic pressure (24,26). The sample is placed in a dialysis bag that, in turn, is immersed in a reservoir that contains a solute for which the relation between osmotic pressure and concentration is known (generally a polymer). The cutoff of the dialysis bag is chosen so that it only retains the polymer and the colloidal matter of the sample. Conversely the solvent, i.e., water, ions, and small organic molecules can exchange between the two compartments. At equilibrium, the chemical potentials of water on either side of the membrane are equal, and therefore the osmotic pressure of the sample equals that of the polymer in the reservoir. This technique makes it possible to play with interactions in a colloidal system over a wide range of pressure, i.e., usually more than 3 decades.

A poly(ethylene glycol) with a molar mass of 35,000 Da (Fluka, Buchs, Switzerland) was used as the stressing polymer. We determined the osmotic pressures of that polymer for concentrations up to 20% (w/w) at 20°C through membrane osmometry (Osmomat 090, Gonotec, Berlin, Germany) and equilibrium dialysis versus T110 Dextran solutions of known osmotic pressure (24). The results were fitted to the following expression for the osmotic pressure Π (Pa) as a function of PEG concentration [PEG] (%, w/w):

$$\log \Pi =a+b{\left[\text{PEG}\right]}^c$$ (1)

with a = 0.49, b = 2.5, and c = 0.29.

Solutions of PEG at osmotic pressures from ∼250 Pa to ∼500,000 Pa were prepared by dispersing the polymer in UF permeate, modified or not by addition of NaCl. If necessary, the pH of the resulting solutions was adjusted to 6.7 ± 0.1 at 20°C to match the dialysis bags.

Standard regenerated cellulose Spectra/Por 2 dialysis bags with a molecular mass cutoff of 12,000–14,000 Da were used (Spectrum Laboratories, Rancho Dominguez, CA). These bags were chosen to allow exchange of water, ions, and lactose but not caseins or PEG. Before experiments, the bags were washed in deionized water and conditioned in UF permeate at the appropriate ionic strength. Then casein dispersions, i.e., sodium caseinate or casein micelles in UF permeate at a given ionic strength, were placed in the bags and immersed in the polymer solutions kept at 20°C. In some cases, depending on the osmotic pressure of the reservoir, it was necessary to refill the bags with casein dispersion to obtain a sufficient amount of concentrated dispersion. After equilibrium was reached (from 7 days to 50 days), the casein concentration in each bag was determined through drying at 105°C. For that purpose, the relation between total solid content and casein concentration in g/L was initially determined over a wide range of concentrations with model dispersions.

Despite the relative simplicity of the osmotic stress technique, a fair amount of experimental work was required to adapt it to the peculiarities of casein dispersions. Extreme attention was paid to the integrity of the casein micelle during the process of osmotic compression. Specifically, we observed through Urea-PAGE experiments that a small but significant degradation of caseins molecules occurred in NPC dispersions compressed at low osmotic pressure, i.e., Π < 5000 Pa after 7 days of dialysis (see the Supporting Material). This degradation was most likely caused by enzymes, including plasmin, that remained in the NPC powder (41). To minimize the effect of this proteolysis, osmotic stress experiments performed at low pressures were limited to 7 days. The casein concentrations in the bags were measured each day. Accordingly, this dialysis time was in most cases sufficient to reach a stable casein concentration. If not, a new 7 days compression was done starting from a fresh NPC dispersion at a concentration closer to the expected equilibrium casein concentration. In all cases, we found from nonprotein nitrogen measurements (Table 1) that enzymatic proteolysis lead to the presence of a maximum of 2 g of low molar mass peptides for 100 g of caseins after 7 days of dialysis at 20°C. The nature of these peptides was investigated through liquid chromatography-mass spectrometry (LC-MS) experiments, which gave a set of molar mass values ranging between 811 Da and 4168 Da. A detailed description of these experiments is provided as Supporting Material.

For osmotic pressures >5000 Pa, equilibrium was reached between 10 and 50 days of dialysis. Urea-PAGE gels showed that enzymatic degradation was limited to insignificant in these conditions. Indeed, at such pressures, high casein concentrations (>200 g/L) were quickly reached in the dialysis bags, leading to high viscosity liquids or solids in which enzymes have a much lower activity.

Reswelling experiments

Over a certain range of osmotic pressures, the casein dispersions changed into a solid-like state. To evaluate the cohesion of these solids, simple reswelling experiments were carried out as follows. For each dialysis bag in which such a state was observed at equilibrium, a piece of the resulting solid (∼0.1 g) was cut out and immersed in a fixed volume of UF permeate (20 mL) at the appropriate ionic strength and without stressing polymer. That solid was then allowed to reswell and/or dissolve during at least 15 h at ambient temperature and under strong agitation. Agitation was then stopped and the dispersion was kept 4 h at rest to allow sedimentation. The casein concentration in the supernatant was then measured by a simple Bradford detection method that was calibrated beforehand with fresh NPC dispersions (42). Knowing the total casein concentration in the dispersion [Cas]_t, the fraction of casein that did not dissolved after reswelling (= the gel fraction), was calculated according to the following expression:

$$\text{Gel}\text{fraction}(\%)=100\times \left(1-\frac{{\left[\text{Cas}\right]}_{\text{s}}}{{\left[\text{Cas}\right]}_{\text{t}}}\right)$$ (2)

with [Cas]_s the casein concentration in the supernatant.

Results

General features

The osmotic compression of casein micelle dispersions produces dramatic changes in their rheological, mechanical, and optical properties. These changes are illustrated in Fig. 1 for NPC dispersions in UF permeate. Dispersions equilibrated at low osmotic pressures (∼450 Pa) are fluids with a moderate turbidity, due to the scattering of light by the casein micelles. At higher osmotic pressures (∼4500 Pa), the turbidity increases, in line with the concentration of casein micelles, and the dispersions still behave as liquids. Then, for pressures that are in excess of 10,000 Pa, the turbidity starts to decrease, and the content of the dialysis bag becomes a solid with a yellowish color (that was the original color of the UF permeate). This evolution is continued at still higher pressures (45,000 and 450,000 Pa in Fig. 1) where the casein dispersion finally becomes nearly transparent.

Figure 1.

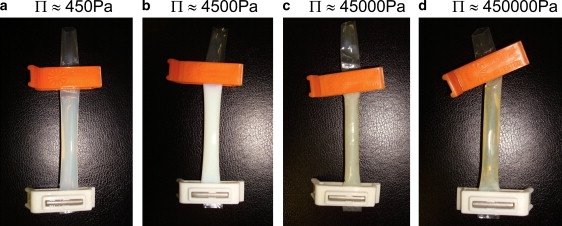
Successive states of the casein micelle dispersions equilibrated at increasing values of the osmotic pressure Π.

Osmotic pressure profiles

Fig. 2 shows the values of osmotic pressures that were applied (through the stressing solution) to reach increasing concentrations of NPC in UF permeate within the dialysis bags. The variations span over 3 decades in osmotic pressures and 2 decades in casein concentrations. Two NPC powders originating from different production batches were used and gave identical results. Hence the osmotic stress method really measures an equation of state or a “material property” of the casein micelle dispersions over an extremely wide range of compositions. At this stage, it is already obvious that this equation of state is made of two very distinct regimes:

• A dilute regime in which the osmotic pressure is proportional to the casein concentration. In this regime, all the NPC dispersions are liquids.

• A concentrated regime in which the osmotic pressure rises much faster, approximately as the sixth power of concentration. In this regime, the NPC dispersions behave as soft-solids or solids.

Figure 2.

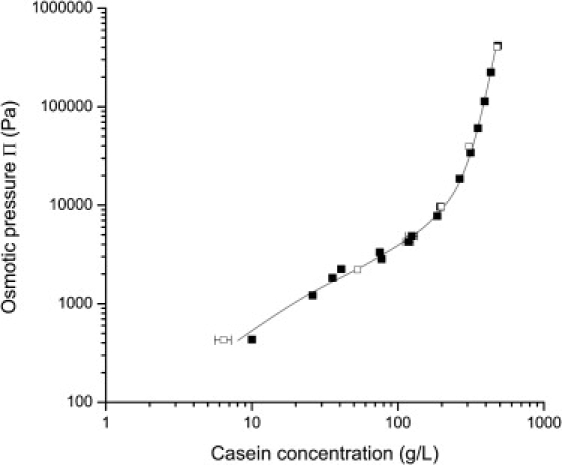
Osmotic pressures of casein micelle dispersions: NPC powder 1 in UF permeate (solid squares); NPC powder 2 in UF permeate (open squares). Vertical scale: osmotic pressure of the stressing solution, in Pa. Horizontal scale, casein concentration within the dialysis bag. The solid line is a guide for the eye.

Similar experiments were carried out with dispersions and stressing solutions made at higher ionic strength through the addition of NaCl (100 mM and 300 mM) to the UF permeate that originally contains 20 mM of Na⁺ and 10 mM of Ca²⁺ for an estimated 80 mM ionic strength. Remarkably, the effects of ionic strength are in opposite directions for the two concentration regimes defined above (Fig. 3 a). In dilute dispersions, the addition of NaCl depresses the osmotic pressure (or makes it easier to concentrate the dispersions). In concentrated dispersions, the addition of NaCl increases the magnitude of the osmotic resistance, but the power law of osmotic pressure versus concentration seems to remain the same. In this regime, dispersions of NPC in UF permeate with 300 mM of added salt had a compression resistance that was nearly twice as high as that of dispersions in UF permeate (Fig. 3 b).

Figure 3.

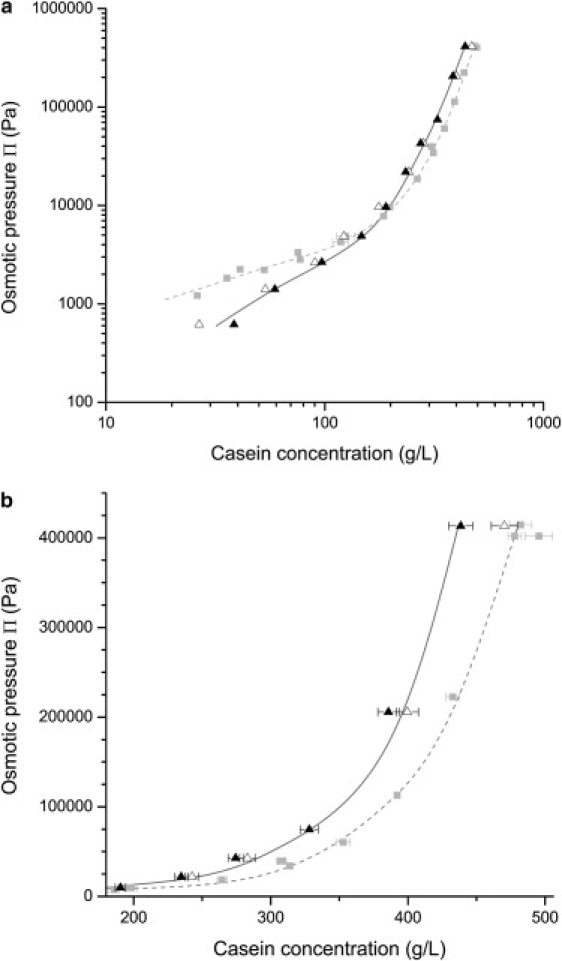
Effect of ionic strength on the osmotic pressures of casein micelle dispersions: NPC powders 1 and 2 in UF permeate (shaded squares); NPC powder 2 in UF permeate + 100 mM NaCl (open triangles); NPC powder 2 in UF permeate + 300 mM NaCl (solid triangles). The dashed and solid lines are guides for the eye. (a) Plot over the whole range of casein concentration, log scale. (b) Plot for high casein concentrations, linear scale.

The osmotic pressures of casein dispersions in UF permeate were also compared with those of sodium caseinate in the same solvent (Fig. 4). The results are markedly different. Indeed, the osmotic pressures of sodium caseinate rise as a single power law of concentration over most of the concentration range, and the exponent is slightly above 2.5. Because both sets of experiments were carried out with the same aqueous solutions (UF permeate), the differences must reflect the different compositions and structures of NPC dispersions versus SC dispersions.

Figure 4.

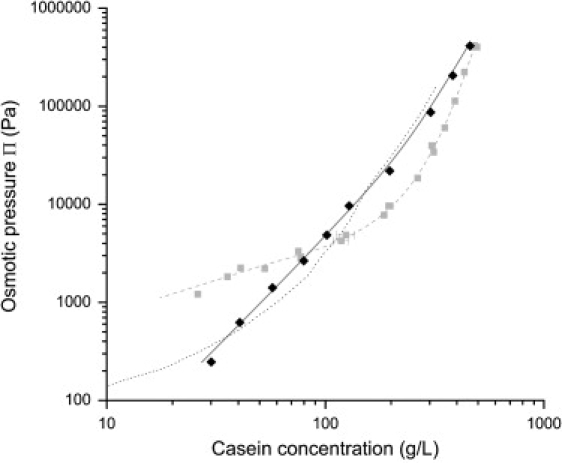
Comparison of casein micelles dispersions with sodium caseinate solutions: NPC powders 1 and 2 in UF permeate (shaded squares); SC powder in UF permeate (solid diamonds). Dotted line: data for sodium caseinate in water + 100 mM NaCl as measured by Farrer et al. (29). The dashed and solid lines are guides for the eye.

Phase behavior

As already mentioned, the NPC dispersions changed from a fluid state to a soft solid state over a range of osmotic pressures and concentrations that we call the transition region. A similar liquid-solid transition was observed for all the systems investigated (NPC powder in UF permeates at different ionic strength and SC powder in UF permeate). To better understand this behavior, the location of the transition range was estimated by direct observations. The reversibility of the phase transition was also assessed through additional experiments for all the dispersions investigated.

Fig. 5 presents the boundaries of the transition region: the lower boundary is taken as the last sample that flows as a homogeneous fluid, and the upper boundary as the first sample that does not flow at all under the effect of gravity (i.e., it has a yield stress). Because there is a unique relation between concentration and osmotic pressure, the boundaries can be traced in either representation (Fig. 5, a and b). For the dispersions of NPC in UF permeate (no added NaCl), the upper boundary was located at a casein concentration of 200 g/L (Fig. 5 a). This is close to the concentration of casein within a micelle (∼230 g/L) that can be deduced from the so-called voluminosity of the casein micelle (estimated by different authors at ∼4.4 mL/g of casein (4,43–48)). Hence, at the upper boundary, the volume fraction that is occupied by the micelles is 0.88 (Fig. 5 a), which is in between the volume fraction for a dense packing of monodisperse spheres (0.74) and that for space-filling packing of polydisperse spheres (1.00). The addition of monovalent salt (NaCl) shifts the transition to lower casein concentrations (150 g/L, i.e., 0.66 volume fraction, with 300 mM of added NaCl) and lower osmotic pressures. For comparison, the fluid-solid transition of sodium caseinate in UF permeate is also indicated: it takes place at higher concentrations and much higher pressures than that of the casein dispersion. Additional information is given by the visual appearance of the dispersions. In the transition region, all NPC dispersions were highly turbid and the upper boundary was near the maximum of turbidity before the dispersions start to progressively acquire a yellowish color (that is the UF permeate color). On the other hand, the optical properties of the SC dispersions were unchanged (translucent and yellow) over the whole range of osmotic pressure, including the transition region.

Figure 5.

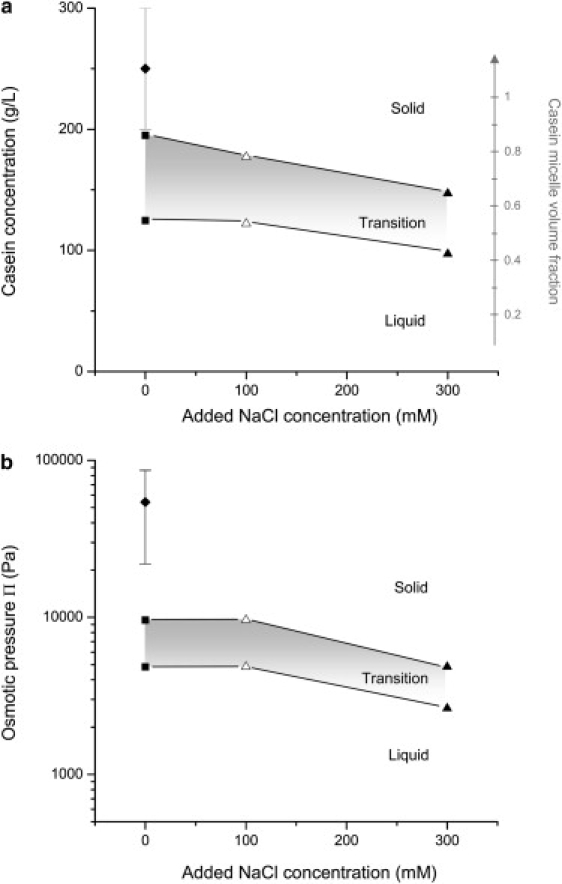
Liquid-solid transition for NPC and SC dispersions in UF permeate, as a function of the concentration of added NaCl. (a) Casein concentration at the transition. (b) Osmotic pressure at the transition. NPC powder 1 in UF permeate (squares, triangles); SC powder in UF permeate (diamond). The volume fraction occupied by the casein micelles was estimated using a micelle voluminosity of 4.4 mL/g (4). It is indicated in a (right vertical axis).

Beyond the liquid-solid transition, i.e., in the concentrated regime, the dispersions were still compressible, even though the casein micelles were densely packed. These extreme compressions produced changes that were not fully reversible. The reversibility of the compression was tested through reswelling experiments. After reswelling, the sample consisted of a gel (mechanically coherent) and a sol (fluid). The remaining gel fraction, as defined in Eq. 2, was then determined. This relatively straightforward test gave remarkable results that are presented in Fig. 6. For all NPC dispersions equilibrated at pressures above the transition, the compression was not fully reversible, and the gel fraction grew with the magnitude of the applied pressure. The extent of irreversibility turned out to be quite sensitive to the nature of the dispersion. The addition of NaCl (100 mM and 300 mM) to the UF solvent caused the gel fraction obtained at ∼10,000 Pa to rise from 20% to 60% of the total mass of casein. This was clearly a direct effect of NaCl on NPC (all other ion concentrations remained the same in all the UF permeates used). On the other hand, the compression of sodium caseinate dispersions was always fully reversible, regardless of the equilibration pressure (Fig. 6).

Figure 6.

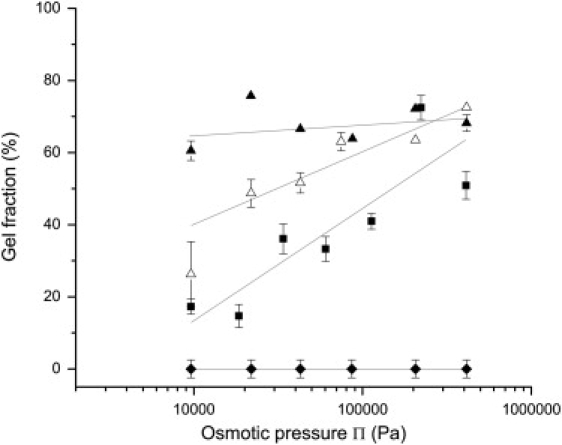
Remaining gel fraction in casein dispersions after compression at pressures ranging from ∼10,000 to ∼450,000 Pa and reswelling in the appropriate solvent: NPC powder 1 in UF permeate (squares); NPC powder 2 in UF permeate with addition of 100 mM NaCl (open triangles); NPC powder 2 in UF permeate with 300 mM NaCl (solid triangles); sodium caseinate in UF permeate (diamonds). The lines are guides for the eye.

Summary of results

The results presented above can be summarized as follows. The osmotic stress method really measures an equation of state of the casein micelle dispersions over an extremely wide range of pressures and concentrations. This equation of state is made of three different stages: i), a dilute regime in which the casein micelles dispersions are liquid and the osmotic pressure is proportional to the casein concentration; ii), a transition range that is complete when the micelles are densely packed, and the dispersion behaves as a coherent solid; and iii), a concentrated regime in which the osmotic pressure rises approximately as the sixth power of concentration. In this last regime, compression removes water from within the micelles, and increases the fraction of micelles that are irreversibly linked to each other within the gel network.

Discussion

The aim of this discussion is to rationalize the osmotic stress behavior of casein dispersions in terms of interactions between or within the different components of these dispersions. This will be done for each of the three compressive stages that have been defined previously.

Dilute regime

The dilute regime comprises the NPC dispersions equilibrated at pressures up to 5000 Pa (Figs. 2 and 3). These dispersions are turbid fluids, with casein concentrations from 10 to 125 g/L. In this regime, the osmotic pressures are approximately proportional to the casein concentration. This is the typical behavior of dispersions in which repulsive interactions are insignificant. It makes sense, because the volume fraction that is occupied by the micelles at such concentrations remains below φ = 0.55 (Fig. 5 a). Therefore volume exclusion effects are not yet important in this regime. The same must be true of ionic interactions between neighboring micelles because they are short range interactions in UF permeate.

If repulsive interactions are insignificant, then the osmotic pressure is a true colligative property, which measures numbers only. It must be the sum of contributions from all the noninteracting species in the dispersion, according to van 't Hoff's law

$$\Pi =\text{R}T\sum _j{c}_j$$ (3)

with noninteracting species i, j, etc. at concentrations c_i, c_j, etc. expressed in moles per unit volume; T is the temperature and R is the ideal gas constant.

Fig. 7 shows the osmotic pressures calculated from Eq. 3 assuming that the casein micelles are the only species in the dispersions (line 1 at the bottom of Fig. 7). The number concentration c_m of casein micelles was estimated from an average molar mass of 2.8 × 10⁸ Da (44). The pressures predicted by the van 't Hoff equation are >3 orders magnitude lower than the experimental ones. Hence the species i, j, etc. that contribute to the osmotic pressure in the dilute regime are not (only) the casein micelles, but also residual species with a much lower molar mass. Because their concentration is low, these species still do not interact with each other, yet they are sufficiently numerous to contribute to the osmotic pressure. This is a classical result: for instance, in latex dispersions, Bonnet-Gonnet et al. (24) have found that the osmotic pressures in the dilute regime (also on the order of 1000 Pa) originate from small macromolecules (average molar mass 3000 g/mol) that coexist with the latex particles.

Figure 7.

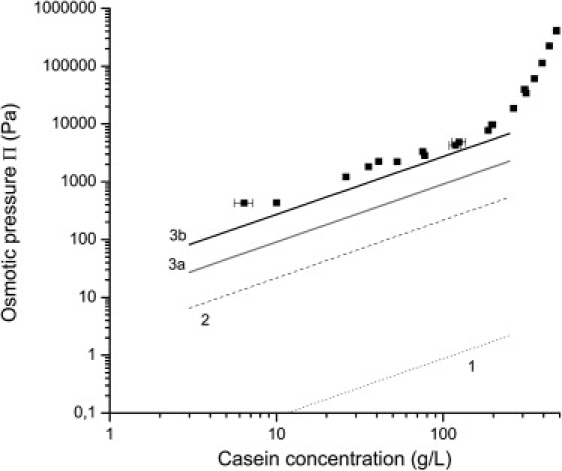
Comparison between the osmotic pressures of NPC dispersions (solid squares) and the predictions of van 't Hoff's law in the dilute regime. The osmotic pressures were calculated through Eq. 3, using the estimated number concentrations of: casein micelles (1, dotted line); serum proteins, serum caseins and proteose-peptones (2, dashed line); low molar mass residual peptides (fresh NPC dispersion: 3a, shaded line; after 7 days of dialysis at 20°C: 3b, solid line).

In the NPC dispersions, different types of macromolecules coexist with the casein micelles:

• Minimicelles: as proposed by Muller-Buschbaum et al. (49), casein “minimicelles” (∼20 nm in diameter) could be in coexistence with ordinary observed casein micelles ranging from 50 to 500 nm in diameter. It is however difficult to assess their number concentration in the NPC dispersions used in this study. On the other hand, a simple calculation shows that the increase in osmotic pressure they could cause is insignificant even if their number concentration is overestimated.

• Residual serum proteins (β-lactoglobulin, α-lactalbumin, BSA, IgG, lactoferrin): these were identified and quantified by reverse phase high-performance liquid chromatography measurements following a method described in Resmini et al. (50) (results not shown). This gave an overall concentration of 0.6% of total solid in the NPC powders.

• Serum caseins: these are caseins (mainly β and α_s1) that are not bound into the micelles. Instead, they form much smaller aggregates from 10 to 25 unimers in conditions similar to those of this study (51–55). The maximum concentration of these serum caseins is generally estimated to be 10% of the total casein concentration (56,57).

• Proteose-peptones: these are casein fragments of molar mass ∼20,000 Da that also associate into small aggregates when in solution (33). The concentration of these fragments is ∼5% of the total casein concentration (noncasein nitrogen in Table 1).

• Peptides: these are smaller protein fragments that have molar masses ranging from 800 to 10,000 Da (the molar masses of the main peptides have been determined through HPLC and MS and are presented in Supporting Material). HPLC results showed that they do not cross the dialysis bags during the 7 days of compression, presumably because the pores are packed with caseins that let through water and small ions but not these peptides (Supporting Material). Peptides are initially present in the NPC powder (0.6% of total solid, nonprotein nitrogen in Table 1) but their concentration increases with dialysis time since proteolysis occurs. For diluted NPC dispersions, after 7 days of dialysis at 20°C, their mass concentration reaches a maximum of 2% of the total casein concentration (Table 1).

Fig. 7 presents the contributions of these different species to the osmotic pressure of the NPC dispersion in UF permeate, calculated according to van 't Hoff's law and with reasonable approximations regarding molar masses and concentrations. The contributions of non micellar caseins, proteose-peptones and serum proteins are small compared to experimental pressures. On the other hand, the contribution of peptides is comparable to the experimental pressures, due to their low molar mass. We may conclude that, in the regime of large dilutions, where the dispersed species do not interact, the osmotic pressure of the dispersion is dominated by the contribution from small peptides. An additional piece of evidence that supports this conclusion is the comparison of NPC dispersions with sodium caseinate solutions (Fig. 4). Indeed, the osmotic pressures from sodium caseinate are much lower, and they rise much more steeply than those of NPC. This is consistent with the fact that sodium caseinate is produced through a precipitation process that minimizes the amount of impurities and residual enzymes compared to the NPC powder (Table 1).

This analysis assumes that the number of free macromolecules remains proportional to the total casein concentration. The observation that the osmotic pressure of casein dispersions in UF remains proportional to the casein concentration indicates that this must be the case. However, in different solvents, the relative numbers of free macromolecules may not be the same. Indeed, osmotic stress experiments performed at higher ionic strengths gave lower osmotic pressures (Fig. 3 a). This effect could be explained by a shift in the association equilibria of the different species in the dispersion: if the small peptides are amphiphilic, then the addition of NaCl may promote the formation of casein-peptides complexes and thus depress the number of free macromolecules that contribute to the total osmotic pressure. The addition of NaCl may also shift other association equilibria, such as the micelle-free caseins equilibrium, but this is expected to have a smaller effect on the osmotic pressures.

Transition range

In this transitional regime, and for all the NPC dispersions, the osmotic pressure is no longer proportional to casein concentration but starts to rise very much faster (Figs. 2 and 3). Simultaneously, the dispersions change from a liquid state to a soft-solid state that is not fully redispersible in its solvent. This section aims at understanding these phenomena and explaining the relationships that exist between them.

On the one hand, the abrupt increase in osmotic resistance cannot be explained by the contribution from the small peptides. Indeed, at casein concentrations that are just before the transition (100–125 g/L), their volume fraction is still quite low (∼0.002) and their interactions are still weak. As a result, their contribution to the osmotic pressure must remain proportional to the total casein concentration. On the other hand, at the onset of the transitional regime, the volume fraction occupied by the casein micelles is φ = 0.55 and the first solid sample was at φ = 0.88 (Fig. 5 a). This is exactly the range of volume fractions where a polydisperse liquid of hard spheres has a transition to a glassy phase: the transition is at φ = 0.63 for a monodisperse distribution of sizes, and closer to φ = 1 for the extreme case of a polydisperse system with an Appolonian distribution of diameters (58,59). Hence, the transitional regime marks the onset of strong interactions between the casein micelles.

This correspondence between the fluid-solid transition of casein micelles and that of the polydisperse hard sphere liquid indicates that the casein micelles do interact with short range repulsions only. Indeed, long-range repulsions would have produced a fluid-solid transition at a lower volume fraction, as in colloidal crystals that are made in deionized water (60). Accordingly, the repulsions produced by the κ-casein brush at the micellar surfaces must be very short range. This is in agreement with a brush thickness usually estimated at 7 nm (8,10).

If the κ-casein brush is compressed, then the micelles may have been forced to come into direct contact. The results of the reswelling experiments might be an indication of that forced contact. Indeed, the samples obtained at the end of the transition were cohesive gels, i.e., they did not redisperse entirely upon transfer from the high osmotic stress conditions to a low osmotic stress environment (Fig. 6). At first, such a gel transition may appear as a surprise, because the κ-casein brush is expected to provide colloidal stability and thus prevent any irreversible sticking of the micellar surfaces. However, previous osmotic stress experiments on latex particles have shown that equilibrium at high osmotic stress makes it possible for particles to bridge through hydrophobic interactions even when they are covered by a polyelectrolyte brush (24). Additionally, it has been suggested that κ-caseins do not homogeneously cover the micelle surface but rather forms small islands surrounded by other, more hydrophobic, caseins (11). This feature may further facilitate irreversible sticking on contact between the micelles.

The experiments carried out at different ionic strengths provide additional information regarding the nature of intermicellar interactions. First, when increasing ionic strength, there is a slight shift of the fluid-solid transition to lower pressures and volume fractions (Fig. 5). As the micelle sizes do not change significantly with ionic strength (21,61), this must be an effect of a shift in the balance of attractions and repulsions. Indeed, particles that attract rather than repel may undergo a fluid-solid transition at lower volume fractions (60,62). Second, there is a major change in the cohesion of the solid, as the gel fraction changes from 15% to 60% (Fig. 6). Again, this must reflect a shift in the balance of attractions and repulsions. Indeed, hydrophobic groups that may be protected by the κ-casein brush at low ionic strength may become more accessible when this brush is partially collapsed at high ionic strength (10). Recently, such an effect of salt addition on the balance of attractions and repulsions between micelles was also reported on casein micelles thin films, i.e., in a concentration regime that resembles the transitional regime discussed here (49).

The behavior of sodium caseinate must be interpreted differently, because there was a liquid-solid transition (at casein concentrations between 175 and 260 g/L) but no gel transition (indeed all samples redispersed entirely when they were transferred from high osmotic stress to a low osmotic stress conditions). It is known that, at such concentrations, the macromolecules of sodium caseinate are interpenetrated (29,30). Hence the fluid-solid transition may result from entanglements of the caseinate macromolecules. However, because no gel transition took place, we must conclude that the attractive interactions that bridge casein micelles together are absent in sodium caseinate. These observations match those from Farrer and Lips (29): “We found that even quite concentrated caseinate solutions (330 g/L) show the viscoelastic behavior of entangled polymer systems rather than of gel networks.”

In summary, the application of moderate osmotic stress, in the range of 5000–10,000 Pa, is sufficient to produce two transitions in casein dispersions: i), a liquid-solid transition, caused by repulsions between κ-casein brushes, that can be compared to the fluid-solid transition of the polydisperse hard sphere liquid; and ii), a gel transition, caused by attractive interactions, that is reminiscent of the sol-gel transitions that take place when the κ-casein brush is degraded through enzymatic treatment (i.e., the well-known renneting process in cheese-making (8)).

Concentrated regime

This regime starts at a casein concentration of ∼200 g/L (Fig. 5 a). As already mentioned, the volume fraction that is occupied by the micelles is then φ = 0.88: most of the water that separates the casein micelles has been extracted and irreversible connections start to be formed between them. Further compression led to casein concentrations up to 500 g/L. Such concentrations are well above the casein concentration within a micelle (230 g/L taking a voluminosity of 4.4 mL/g). This shows that all the water that separates the micelles has been extracted and that compression now causes the micelles to deform and deswell.

Another indication of this transformation to a gel phase is the observation that the gel fraction, which cannot be redispersed by dilution, increases continuously through the concentrated regime (Fig. 6). At high ionic strengths (+100 mM and +300 mM NaCl), these intermicellar connections are established more easily so that the solid cohesion is less sensitive to osmotic pressure.

As a result, the dispersion can now be considered as a continuum (at the scale of the micelle) and the osmotic pressure can be understood as the compression resistance of the casein micelle itself, i.e., the casein micelle interior. At the start of the concentrated regime, the dispersions are still white/turbid, indicating that the distribution of water and proteins within the micelles is still heterogeneous, e.g. dense regions (lumps) separated by a continuous phase. Further compression into this regime causes the turbidity to subside, and the compressed dispersions become totally translucent at 450,000 Pa (Fig. 1). This loss of turbidity indicates that the water that separates the dense regions has been removed.

Models

A simple model for the structural evolution of the micelles through this regime assumes that all the protein and calcium phosphate are in the lumps, and that the continuous phase contains water and small ions only. In this case the resistance to extraction of water must originate from the thermal agitation of the lumps and from their interactions. The simplest version of this model is the hard sphere liquid, in which the micelle interior is described as a collection of nonconnected hard spheres that occupy a fraction φ of the total volume. In this case, the osmotic pressure originates from the thermal agitation of the spheres. The rise of the osmotic pressure with increasing φ is related to the loss of available configurations. This is given by the Carnahan-Starling equation (63),

$$\frac{\Pi }{nkT}=\frac{1+\varphi +{\varphi }^2-{\varphi }^3}{{(1-\varphi )}^3},$$ (4)

with n the number density of spheres.

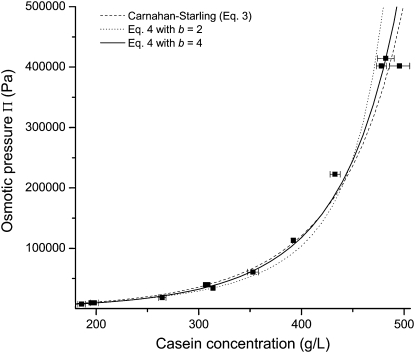
Fig. 8 shows that Eq. 4 fits remarkably well the experimental data with spheres of diameter d_p = 8.8 nm and mass m = 1.56 × 10⁵ Da. With these parameters and knowing the voluminosity of the casein micelle (∼4.4 mL/g of casein), the average distance between these spheres can be estimated if we consider that they are placed on a regular (e.g., face-centered cubic) lattice. Such a calculation leads to a distance d between 12 and 17 nm. Interestingly, this distance is quite close to the correlation length λ that has been measured through neutron or x-ray scattering (λ = 16–18 nm (49,64–66)) for casein micelles and is interpreted commonly as the distance between the calcium phosphate nanoclusters that are present in their internal structure. Moreover, an average casein micelle of 2.8 × 10⁸ Da (44) would contain ∼1800 of these spheres, which is of the same order as the number of nanoclusters in a micelle (∼800 (65)). This correspondence suggests that one could identify the calcium nanoclusters and their direct environment to equivalent objects that interact as hard spheres.

Figure 8.

Osmotic pressures of NPC dispersions in the concentrated regime: NPC powders 1 and 2 in UF permeate (solid squares). The dashed line is calculated through the Carnahan-Starling equation (Eq. 4) for hard sphere particles of diameter 8.8 nm and mass 1.56 × 10⁵ Da in casein. The dotted and solid lines are calculated through Eq. 5 with b = 2 and 4 respectively.

However, it is clear that this model cannot be taken as a realistic model for the internal structure of the casein micelles. Indeed, it assumes that the spheres have no interactions besides excluded volume effects. This would yield very distinct correlations at a distance equal to the sphere diameter (67). These correlations would show up as an enormous peak at a scattering vector q = 0.6 nm⁻¹ in the small angle x-ray scattering (SAXS) and small angle neutron scattering (SANS) spectra. In fact, the experimental SAXS or SANS spectra only show weak oscillations in the corresponding range of q, even at the highest casein concentrations, indicating that the correlations are considerably more complex than those of hard spheres (7,65,66,68).

A more realistic model would be to consider the micelle as made of connected particles (caseins and calcium phosphate nanoclusters) in a continuous phase. Based on recent experimental studies (transmision electron microscopy, SAXS, and SANS), such a network approach is now accepted widely (7,65,68,69). However, several network models exist and differ in the detailed description of the units that are linked by these bonds, and of the resulting correlations. At one extreme, there are uniform protein matrix models in which the only characteristic length is the distance between calcium phosphate nanoclusters (1,6,7). At the other extreme, there are true network models in which the casein molecules are assembled to form branched aggregates, which are cross-linked by the calcium phosphate nanoclusters (69). For the interpretation of osmotic stress experiments, we have no rationale for choosing one model rather than another.

A more model independent approach is based on the free volume concepts that have been used to describe the behavior of polymer solutions (71,72) and also of systems that are jammed by increasingly dense packing (73). In such a model, the system has a limiting concentration C^∗ that would be reached through compression at extremely high pressures. At lower pressures, the actual casein concentration is C, and the divergence of the osmotic pressure upon approaching C^∗ would follow a law of the type

$$\frac{\Pi }{aC}={\left(1-\frac{C}{C\ast }\right)}^{-b}$$ (5)

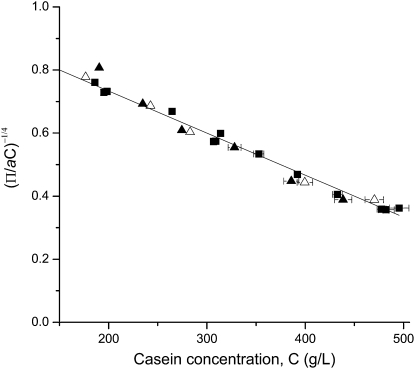
in which a and b are adjustable parameters. A very acceptable fit is obtained for b = 4 (Figs. 8 and 9). In such a case, a = 14.0 and the osmotic pressure diverges for C^∗ = 750 g/L. In the case of a network that contains dense lumps, this value is the concentration of the high density regions in the casein micelle; the work of compression that is carried out in the concentrated regime is used to extract the aqueous phase from the pores that separate these dense regions. It is also interesting to figure out how much water remains in the dense regions at the end of the compression in the dense regime. For that calculation, a weighted average partial specific volume of 0.733 mL/g is taken for the caseins (44). The mass fraction of calcium phosphate is ∼0.08 and it is mainly present as nanoclusters with a density close to 2.1 g/mL (74). This leads to ∼0.5 g of water per gram of dry casein. Interestingly, this value is quite close to the full hydration of a typical globular protein in water (0.3–0.4 g/g (75,76)). This suggests that this residual water is the water that is intimately linked to the caseins. It will only be extracted in another compression regime, at extreme pressures, that lead to total dehydration of the casein molecules.

Figure 9.

Unified model (Eq. 5) for the osmotic pressure of NPC dispersions in UF permeate at different ionic strengths: NPC powders 1 and 2 in UF permeate (solid squares); NPC powder 2 in UF permeate + 100 mM NaCl (open triangles); NPC powder 2 in UF permeate + 300 mM NaCl (solid triangles). The parameter a was taken as 14.0, 20.0 and 21.5 for NPC in UF permeate + 0, + 100 and + 300 mM NaCl respectively. The full line is (1 − C/C^∗) with C^∗ = 750 g/L.

Ionic strength effect

The experiments carried out with NPC dispersions at higher ionic strength required higher osmotic pressures, indicating that the casein micelle was harder to compress when NaCl was added (Fig. 3). This effect of salt addition is fully consistent with the experimental results of Famelart et al. who found an increase in the water content of 75,000 × g ultracentrifugation pellets of NPC dispersions at increasing NaCl concentrations (77). It could be explained through a loss of calcium from the micelle upon addition of NaCl (21,77). According to Huppertz et al. (21), this release of Ca²⁺ is not associated with a loss of phosphorus, indicating that it originates from Ca²⁺ bound to caseins molecules and not from the calcium phosphate nanoclusters. Accordingly, this Ca²⁺/Na⁺ exchange may dissociate some of the calcium bridges that hold the casein molecules together and also increase the ionic pressure inside the micelle. These effects would lead to a network that is less connected, contains more monovalent ions, and therefore would further resist compression.

In the view of a network that contains dense regions (lumps) separated by pores, the analysis of the data according to Eq. 5 shows that the model is able to describe the results obtained at all ionic strengths with b = 4, C^∗ = 750 g/L, and a ranging from 14.0 to 21.5 (Fig. 9). The possibility of using a single value for C^∗ over the range of ionic strengths suggests that the hydration of the dense regions is not modified; only the pores between these regions are harder to compress when Ca²⁺ ions are replaced by Na⁺.

Sodium caseinate

A completely different behavior was obtained with sodium caseinate in UF permeate. Over the whole concentrated regime, the osmotic pressures of sodium caseinate are higher than those of the NPC dispersions, but their rise is not nearly as steep (Fig. 4). These differences make sense because sodium caseinate dispersions no longer contain any calcium phosphate. In terms of interactions, some important attractive forces that contribute to the structure and cohesion of casein micelles are therefore absent from the sodium caseinate dispersions. The lack of any such attractive forces in sodium caseinate dispersions is shown by the observation that their compression is fully reversible (Fig. 6). Accordingly, and as already suggested by Farrer and Lips (29), it may be more appropriate to describe a sodium caseinate dispersion as a polyelectrolyte solution. The polyacrylic acid (PAA) solutions studied by Bonnet-Gonnet et al. (24) are a good example of such a system. Fig. 10 shows the comparison between the osmotic pressures of PAA in water with 100 mM NaCl at two pH values and those of sodium caseinate in UF permeate. For PAA systems, identical pressures were obtained at both pH values, indicating that ionic interactions were largely screened in both cases. Both osmotic pressure profiles have the same exponent (∼2.7), which confirms that the osmotic resistance of sodium caseinate dispersions has the same origin as that of a usual polymer solution, i.e., the entropy of mixing of the polymer segments with the solvent. However, the profiles differ by a constant concentration factor that takes into account the mass per segment of each polymer. This concentration factor is ∼5.5, suggesting that the statistical segment of sodium caseinate is much larger than that of a usual polymer such as PAA.

Figure 10.

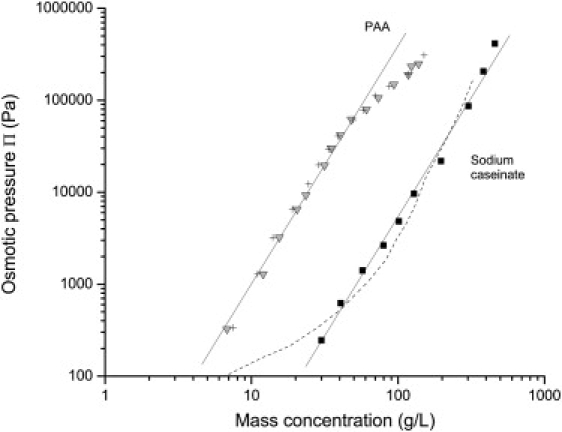
Comparison between the osmotic pressures of sodium caseinate and pure polyacrylic acid (PAA): SC powder in UF permeate (solid squares); Sodium caseinate in water + 100 mM NaCl as measured by Farrer et al. (29) (dashed line); PAA at pH 9 in water + 100 mM NaCl (crosses); PAA at pH 3 in water + 100 mM NaCl (inverted triangles). See Bonnet-Gonnet et al. (24) for details in the experiments carried out with PAA. The lines are guides for the eye but have identical slopes (exponent 2.7).

Conclusions

The osmotic stress technique was successfully applied to dispersions of native casein micelles, i.e., dispersions made from NPC powder in skimmed milk UF permeate. This method measured an equation of state of the dispersions over an extremely wide range of pressures and concentrations. We found that this equation of state has three distinct regimes in which very different phenomena take place: i), a dilute regime in which the micelles (and the other dispersed species) are far from each other and do not interact; ii), a transition range that is complete when the casein micelles are densely packed; and iii), a concentrated regime in which compression removes water from within the micelles.

A detailed analysis of the results obtained in these three different compressive regimes and at different ionic strengths (+0, +100 mM NaCl, +300 mM NaCl) has been conducted. These results were also compared to the osmotic pressures of sodium caseinate in UF permeate. Accordingly, the questions raised in the introduction section of this study can be reasonably answered as follows:

• a.In the dilute regime, the cost of removing water from the NPC dispersions is mainly the cost of the osmotic compression of a solution containing the residual small peptides that are present in such dispersions. In a proper description of casein micelles dispersions made from NPC powders, one consequently needs to take into account these small species.
• b.The application of moderate osmotic stress, in the range of 5000–10,000 Pa, is sufficient to produce two successive transitions in casein dispersions: a liquid-solid transition, caused by repulsions between κ-casein brushes, that is similar to the fluid-solid transition of the hard sphere liquid; and a gel transition, caused by attractive interactions, when the micelles are in contact at ∼200 g/L. That second transition is reminiscent of the sol-gel transition that takes place when the κ-casein brush is degraded through enzymatic treatment such as the well-known renneting process in cheese-making.
• c.At higher concentration, the micelles are in contact and there is no longer any intermicellar continuous phase. The osmotic compression causes the casein micelles to deform and deswell and the turbidity of the dispersions decreases. In a simple model, the micelle core is made of dense regions (lumps) of concentration C^∗ that are distributed in an intramicellar continuous phase from which water is extracted on compression. The pressure diverges at C^∗ when all the water between the lumps is extracted. This concentration corresponds to a hydration of the lumps that is close to the typical hydration of globular proteins.
• d.When the micelles have been brought into direct contact and then compressed further, some of them can no longer be separated through reswelling. Hence some cohesive forces have been turned on by the compression. However, the compression of sodium caseinate, where the calcium phosphate nanoclusters have been removed, is fully reversible. This suggests that these cohesive interactions result from ionic and hydrophobic forces that take place at the surface of the casein micelles. These structure-dependent forces are disrupted when the micelles are dissociated through dissolution of the calcium phosphate nanoclusters.

Supporting Material

Materials, results, three figures, one table, and three references are available at http://www.biophysj.org/biophysj/supplemental/S0006-3495(08)00056-8.