Intra-annual variability of anatomical structure and δ¹³C values within tree rings of spruce and pine in alpine, temperate and boreal Europe

Abstract

Tree-ring width, wood density, anatomical structure and ¹³C/¹²C ratios expressed as δ¹³C-values of whole wood of Picea abies were investigated for trees growing in closed canopy forest stands. Samples were collected from the alpine Renon site in North Italy, the lowland Hainich site in Central Germany and the boreal Flakaliden site in North Sweden. In addition, Pinus cembra was studied at the alpine site and Pinus sylvestris at the boreal site. The density profiles of tree rings were measured using the DENDRO-2003 densitometer, δ¹³C was measured using high-resolution laser-ablation-combustion-gas chromatography-infra-red mass spectrometry and anatomical characteristics of tree rings (tracheid diameter, cell-wall thickness, cell-wall area and cell-lumen area) were measured using an image analyzer. Based on long-term statistics, climatic variables, such as temperature, precipitation, solar radiation and vapor pressure deficit, explained <20% of the variation in tree-ring width and wood density over consecutive years, while 29–58% of the variation in tree-ring width were explained by autocorrelation between tree rings. An intensive study of tree rings between 1999 and 2003 revealed that tree ring width and δ¹³C-values of whole wood were significantly correlated with length of the growing season, net radiation and vapor pressure deficit. The δ¹³C-values were not correlated with precipitation or temperature. A highly significant correlation was also found between δ¹³C of the early wood of one year and the late wood of the previous year, indicating a carry-over effect of the growing conditions of the previous season on current wood production. This latter effect may explain the high autocorrelation of long-term tree-ring statistics. The pattern, however, was complex, showing stepwise decreases as well as stepwise increases in the δ¹³C between late wood and early wood. The results are interpreted in the context of the biochemistry of wood formation and its linkage to storage products. It is clear that the relations between δ¹³C and tree-ring width and climate are multi-factorial in seasonal climates.

Introduction

Tree rings are generally considered to be archives of past climatic conditions and of changes in the environment (Schweingruber 1996; Wimmer 2002), especially when trees were growing under extreme conditions in which changes in climate dominated the response. Despite a long history of climate reconstructions (Fritts 1966), there is still some uncertainty regarding the specific effects of certain climate parameters, especially temperature and drought, on tree-ring width and wood anatomy (Vaganov et al. 2006). This uncertainty is based on the knowledge that seasonal and annual changes in tree-ring anatomy (tree-ring width, number of cells, size of cells, cell-wall thickness and wood density) reflect not only processes at the time of xylem-cell production and maturation, but also additional physiological controls and the availability of storage products at the time of wood formation (Hemming et al. 2001). In addition, the conditions for wood formation may be different for solitary growing trees under extreme environmental conditions, which have been generally sampled for tree ring studies, than for trees growing in forest stands of closed canopies where competition for water, nutrients and space in addition to climate be important. Also, single events, such as late frost or heavy fruiting, may affect wood growth more than average climatic conditions in forest stands.

In addition to wood anatomy, the isotope composition of tree rings may serve as an additional parameter to expand the possibilities of interpreting tree-ring width. The ¹³C/¹²C ratios of wood (cellulose) have also been shown to reflect climatic conditions (Wilson and Grinsted 1977; Leavitt 1993; McNulty and Swank 1995; Duquesnay et al. 1998; McCarroll and Loader 2004). The carbon isotope ratios of carbohydrates are determined by carbon dioxide (CO₂) assimilation and stomatal conductance during the growing season (Francey and Farquhar 1982; Farquhar et al. 1989; Brugnoli and Farquhar 2000). However, these ratios in tree rings may also be influenced by isotopic discrimination during storage and re-mobilization of storage products, including various carbohydrates, lipids and proteins, in leaves (Gleixner et al. 1998; Gessler et al. 2008) and stems (Skomarkova et al. 2006). Storage effects are most important at the end and at the beginning of the growing season (Helle and Schleser 2004; Scartazza et al. 2004; Schulze et al. 2004), although the interactions between storage products and growth are not fully understood. A carry-over effect may lead to autocorrelations among years and mask a climatic signal (Hill et al. 1995; Monserud and Marshall 2001; Kagawa et al. 2006a, b).

The aims of the study reported here were (1) to determine the correlation between climate parameters and tree-ring width (TRW) and wood anatomy (wood density, cell-wall thickness, cell-lumen area) at three sites ranging between boreal and sub-Mediterranean alpine climates; (2) to compare the long-term (decadal) correlations between tree rings and climate parameters with short-term observations; (3) to analyze the seasonal variation of tree-ring δ¹³C in trees exhibiting different growth rates; (4) to investigate relations between present and past years on tree-ring parameters.

Materials and methods

Experimental sites and tree samples

The three study sites are (Table 1; see also http://www.bgc-jena.mpg.de/public/carboeur/sites/index-s.html): (1) an alpine forest in North Italy (Renon, REN), (2) a lowland forest in Germany (Hainich, HAI) and (3) a boreal forest in North Sweden (Flakaliden, FLA). Annual temperatures were highest in HAI and lowest in FLA, and the length of the growing season (>5°C) was longest (211 days) in Germany, 20% shorter at the alpine site and 34% shorter at the boreal site. Annual precipitation and summer rainfall decreased from the alpine REN site to the boreal FLA site. The investigated stands differed in age and stand density. The distribution of forests at the global scale is related to precipitation, but the growth of trees is not only determined by climate but also by nutrition (Linder 1995; Schulze et al. 2006). Table 1 shows that the boreal site has a much lower nitrogen concentration in its needles than the other sites and that the needle nitrogen level reached at FLA indicates deficiency (Oren and Schulze 1989).

Table 1.

Climate and stand characteristics of the Renon site (Cescatti and Marcolla 2004), the Hainich site (Knohl et al. 2003) and the Flakaliden site (Linder 1995; Lundmark et al. 1998)

Site	Renon (North Italy)	Hainich (Central Germany)	Flakaliden (North Sweden)
Longitude	46°36′	51°04′	64°07′
Latitude	11°28′	10°27′	19°27′
Elevation (m)	1,730	445	310
Length of growing seasona (>5°C) (day)	30 April–14 October (167)	31 March–27 October (211)	9 May–27 September (141)
Average annual temperaturea (°C)	3.8	6.8	1.9
Precipitation, annual (mm)	1,008	780	590
Species (no. of samples)	Picea abies (13) Pinus cembra (8)	P. abies (5)	P. abies (5) Pinus sylvestris (5)
Age (years)	182	80	31
Diameter at breast height (cm)	17	40	8
Height (m)	29	31	6
Stand density (trees/ha)	745	679	2100
Needle nitrogen concentration (mmol g−1)	0.84	0.94	0.68

^aTemperature data were corrected according the differences between the elevation of the studied site (1700 m) and that of the meteorological station (1210 m)

Soil conditions differed between sites. The boreal site at FLA is a glacial moraine with a shallow organic layer on unsorted stony material in the whole soil horizon. The lowland site at HAI is a brown earth developed on clay that originated from the weathering of limestone. The alpine site REN is a shallow organic layer that developed on limestone boulders.

In accordance with the climate analysis of Walter and Lieth (1967), all sites have a positive hydrological balance. During the time interval studied in this investigation (Table 2; 1999–2003), the seasonal climate showed very little variation in early season temperatures, but temperatures were colder during the autumn at the boreal site. Average daytime vapor pressure deficit was highest at the HAI site. Early season precipitation was lowest at the boreal site, but low rainfall in the spring is generally considered to be compensated for by snow melt in boreal climates. Late season rainfall was lowest at HAI. Solar radiation was highest at the alpine site REN. Due to the long daylight in the northern summer, solar radiation was higher at FLA than at HAI in the early growing season, but this decreased in the late summer.

Table 2.

Comparison of early and late season climate between sites

Parameter	Renon	Hainich	Flakaliden
Temperature–MJJ (°C)	14.8	15.2	11.6
Temperature–ASO (°C)	12.5	14.8	7.8
Vapor pressure deficit–MJJ (hPa)	4.2	5.8	4.7
Vapor pressure deficit– ASO (hPa)	3.7	4.5	2.6
Precipitation–MJJ (mm)	324	214	209
Precipitation–ASO (mm)	242	177	202
Precipitation-growing season (mm)	566	391	411
Solar radiation-MJJ (MJ m−2)	967	621	665
Solar radiation–ASO (MJ m−2)	686	491	299

MJJ, May–June–July; ASO, August–September–October

Study interval: 1999–2003

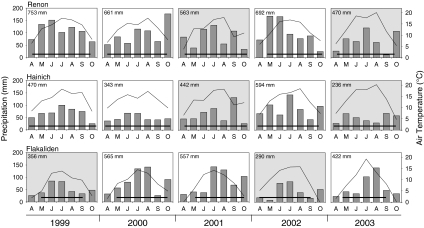
The seasonal averages do not show the inter-annual variability of climate, which is important to this study (Fig. 1). Maximum temperatures increased at all sites between 1999 and 2003. The length of the growing season (days with average temperature >5°C) did not show large inter-annual variability, while precipitation showed the largest variability, with drought being more apparent later in the season. Years with precipitation during the growing season below the long-term average were considered to be dry years. At FLA, 1999 and 2002 were considered to be dry years, with rainfall up to 30% below average; at HAI and REN, 2001 and 2003 were considered to be meteorologically dry years.

Fig. 1.

Inter-annual variability in temperature and rainfall at the three study sites for the period of 1999–2003. Gray shadow indicates dry years, horizontal bars indicate the meteorological length of the growing season (>5°C daily temperature)

Since the CarboEurope climate data cover only 5 years, additionally long-term meteorological data (daily temperature and precipitation) were used from the following weather stations: Costalovara (46°52′N, 11°42′E, 1,250 m a.s.l., data for 1989–2003), Soprabolzano (46°52′N, 11°40′E, 1,206 m a.s.l., data for 1931–1980), Leinefelde (51°20′N, 10°22′E, 440 m a.s.l., data for the 1957–2003) and Stensele (65°04′N, 17°10′E, 330 m a.s.l., data for 1918–2001).

Tree sampling and dendrochronological analysis

Wood samples were collected by coring one core per tree at breast height (1.3 m) with a 5-mm-diameter increment borer. At Renon, eight cores of Norway spruce [Picea abies (L.) H. Karst] and three cores of Swiss stone pine (Pinus cembra L.) were used to measure wood density, and five cores of each species were used to measure δ¹³C and anatomy. For Hainich, three cores each of Norway spruce were used to measure δ¹³C and anatomy, respectively. For Flakaliden, three cores each of Norway spruce and Scotch pine (Pinus sylvestris L.) were used for δ¹³C and anatomy, respectively. The statistical data of wood density and tree-ring width chronologies are based on International Tree-Ring Data Bank (ITRDB; FH Schweingruber) for the sites in Germany and Sweden.

Tree-ring width was measured on all cores using a semi-automatic device LINTAB-III (Cook and Kairiuktis 1990; Rinn 1996; Vaganov et al. 1996). Density profiles of tree rings were measured following Schweingruber (1988) using a densitometer (Dendro-2003; Walsch Electronics, Zurich, Switzerland).

We also used the existing in ITRDB tree-ring width and maximum density chronologies obtained by FH Schweingruber at the end of the 1970s for sites close to the study areas. The analog sites of HAI and FLA are Andreasberg Harz Schlucht (900 m NN) and Gallejour Glommerstark (480 m NN), respectively (http://www.ncdc.noaa.gov/paleo/ftp-treering.html).

Cross-dating of individual cores was carried out based on tree-ring width (Schweingruber 1988) using the program COFECHA (Cook and Peters 1981; Holmes 1992). Absolute values of each tree-ring width were standardized by calculating the ratio of the tree-ring deviation in a certain year and the long-term average of tree-ring width to remove the effect of age and diameter on tree-ring width (Shiyatov 1986; Cook et al. 1990; Vaganov et al. 1996). A spline function was used to determine the long-term trend for each tree core using the program ARSTAN (Cook et al. 1990). The following parameters were calculated according to Schweingruber (1988) as:

1. The standard deviation of relative TRW and maximum density, indicating a measure of tree-ring response to inter-annual climate variability.

2. The “interserial correlation” describes the variation in a tree-ring parameter in a certain year between all sample trees of a stand. This parameter provides information on the heterogeneity of the stand and indicates the similarity of tree growth during inter-annual changes in climate. A high number indicates a high similarity between trees.

3. The common variance of the first eigenvector explains the fraction of the variability in tree-ring parameters by environmental factors common to all trees at each site (Fritts 1976; Cook et al. 1990). A high number indicates a strong climatic effect on all trees of a site.

4. The first-order autocorrelation explains the correlation between the tree-ring width in the previous year (t-1) and ring width in the year under investigation (t). A large number represents a strong effect of the past year on the current year's tree ring.

The effect of temperature and precipitation on the inter-annual variability of tree-ring growth and wood density was estimated through correlations between the chronologies and monthly climatic data from the above mentioned meteorological stations. These calculations were performed for each month of 12 months (from October of the previous year to September of the current year) using the program RESPONSE (Holmes 1983).

Image analysis of tracheid dimensions

Wood cross sections were cut with a microtome (20 μm) and stained by methylene blue (Furst 1979; Vaganov et al. 1985). Tracheid dimension in tree rings was determined using an Image analysis system (Carl Zeiss, Jena; Munro et al. 1996; Vaganov et al. 2006). Radial cell diameter (DR), cell-wall thickness (CWT) and tangential cell diameter (DT) were measured in five radial series of cells from the outside border in each tree ring to the inside border for each year. The five radial series were 100–150 μm (three to five cell rows) apart. Assuming a rectangular shape of the tracheid in cross section, these data were used to calculate cell-wall area: 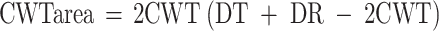, and cell-lumen area: 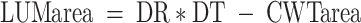. Since tree-ring width varies between years and species, measurements were normalized to a standard number of cells (Vaganov 1990).

Seasonal course of anatomy and isotopes

Tracheids of conifers are organized in rows in which each tracheid was produced by the cambial zone consecutively after the previous one. As such, the position (from the first early wood cell to last late wood cell) of each tracheid in a row is related to a temporal scale. In order to fit the anatomical data measured within a tree ring with calendar dates (dates of season) for some samples, we used the approach described in detail by Vaganov et al. (2006) which transforms the tracheid radial size to the dates of their production by the cambium based on two main assumptions: (1) a linear relationship between growth rate and radial tracheid size, and (2) a known duration of the growth season. In tree-ring studies, the length of the growth season is the period with an average daily air temperature above 5°C (Mikola 1962; Leikola 1969; Hughes et al. 1999; Jones and Briffa 1992; Kirdyanov et al. 2003). Based on this assumption, the linear sequence of tracheid measurements is transformed into a seasonal time scale for each consequent tracheid in the row, accounting for a delay of 25 days, which is needed for cells to reach a final diameter and to start with secondary cell-wall growth (Vaganov et al. 2006).

Carbon isotope analysis

Carbon isotope ratios were determined using a laser ablation-combustion line coupled to an isotope-ratio-mass-spectrometer (YAG 266 nm UV laser; Merchantek–New Wave, Fremont, CA, coupled to a Finnigan Delta +XL) as described in detail by Schulze et al. (2004). The exact location of each ablation spot was visualized with a camera mounted directly on the laser ablation station. The wood samples were enclosed in an aluminum chamber with a quartz window, which was flushed with helium as carrier gas. For quantitative combustion of the ablation particles to CO₂ and H₂O, the sample was passed through an Al₂O₃ tube at 700°C containing CuO wire as the oxygen source. The reaction gas further passed through a gas chromatography (GC) column (HayeSep D, Bandera, TX) to separate CO₂ from other gases. Water was removed with an on-line Nafion water trap. Carbon-stable isotope ratios were expressed in the δ notation on the VPDB scale using NBS22 with a value of −30.03‰ as the scale anchor. The spatial resolution of a laser shot is about 70 μm. A series of four to five shots was needed at one location to collect sufficient material for analysis. The series of shots was repeated radially along the same line every 120 μm, which resulted in ten to fifteen data points per tree ring. Profiles of δ¹³C were measured for a 19-year period.

The δ¹³C values within a tree ring varying within and between years were normalized by calculating the deviation of the measured value at time t from the average of that same year (δ¹³C_t − δ¹³C_avt).

In a separate experiment, the δ¹³C value of ray cells and of adjacent tracheids were measured in the last cells of late wood and in the first cells of early wood in order to detect changes which may be related to carbohydrate storage.

We use the δ¹³C notation rather than discrimination (Δ) because we did not measure the atmospheric δ¹³C. Furthermore, the reconstruction of all discrimination steps during wood formation was not the objective of our study and, therefore, the comparison of δ¹³C values seemed to be more appropriate. The δ¹³C-values represent the isotope ratios of natural wood, including lignin. Schulze et al. (2004) showed that there is an offset between cellulose and natural wood of 1.5‰ in conifers, which is constant and independent of season. Eglin et al. (2008) also confirmed that biochemical composition influences the variability in the carbon isotope composition of tree rings by up to a maximum of only 5%. Consequently the investigation of whole wood samples seems to be appropriate (Harlow et al. 2006; Eglin et al. 2008).

Statistical analysis

Relationships between wood anatomy and carbon isotope ratios and their dependency on climatic variables were analyzed with linear mixed-effects models using the lmer function in the R package lme4 (Bates and Sakar 2006) to account for unbalances in the data set due to different numbers of investigated trees per site and study years. A hierarchical series of models was fitted sequentially, entering site, wood anatomical or climatic variables and their interactions, with site as fixed effects. Tree identity and study year were included as grouping factors in a nested sequence in the random term. The maximum likelihood method was applied, and likelihood ratio statistics were used to appraise model improvement and to test for statistical significance of the fixed effects.

Results

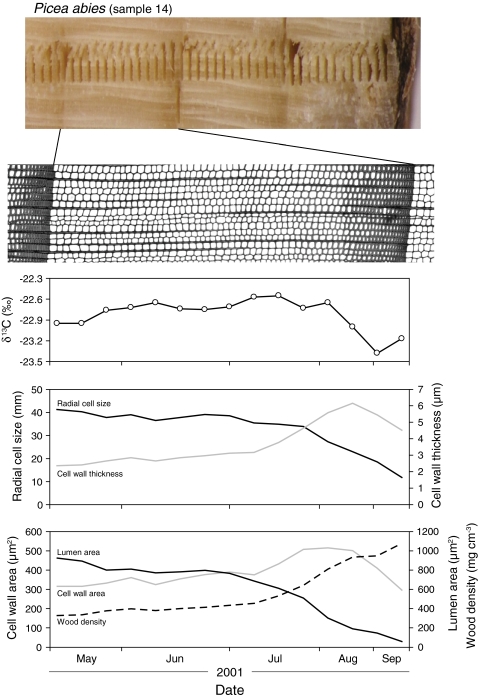
A typical pattern of the anatomical parameters and carbon isotope ratios of the 2001 tree ring of spruce from REN (Fig. 2) shows that the radial size of cells decreases slowly in early spring wood and faster in late early wood and late wood. Early and late wood are clearly visible in the density slide. At the same time, CWT and area increase, reaching a maximum in the first cells of late wood and decreasing again before the cessation of growth at the end of the season. Changes in lumen area parallel the changes in radial cell size. Wood density can be seen to increase in early wood slower than in late wood, reaching a maximum in the last cells of late wood. Values of δ¹³C increase gently in early wood from the beginning of the growth ring to the transition zone between early and late wood and then decrease sharply in late wood. This decrease in δ¹³C (more negative values indicate open stomata) in late wood was unexpected because precipitation was low in August.

Fig. 2.

Example of anatomical, density and isotopic measurements in a spruce tree ring (Renon, Italy; Picea abies, tree number 14): radial cell size, cell-wall thickness, lumen area, cell-wall area, wood density. The laser shots for isotope determination can be seen as vertical bars

Long-term relations between tree-ring width, maximum density and climate

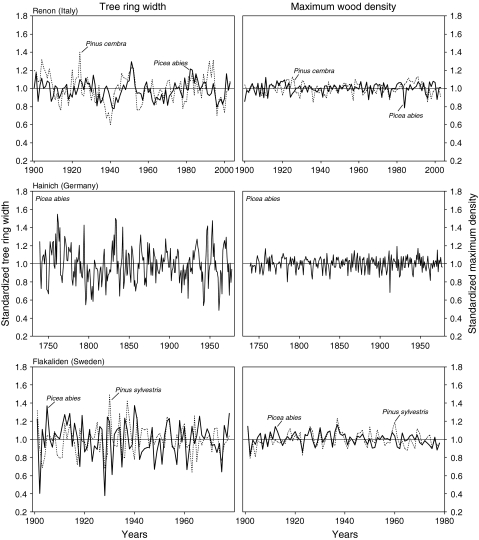
Standardized tree-ring width and maximum wood density of spruce and pine species varied with a standard deviation of up to 20%, and they were similar between both species studied (Table 3; Fig. 3). Both species showed higher inter-annual variability for tree-ring width than for maximum wood density. The standard deviation of the standardized tree-ring width, indicating inter-annual variability, was lower at the alpine REN site [standard deviation (SD) 10%] than at the boreal FLA and the lowland HAI site (SD 20%; Table 3). Inter-annual variability of wood density was very small (6–7%) and similar for all sites and species.

Table 3.

Statistical parameters of tree-ring width and maximum density chronologies for conifers from the studied sites

Species	Parameter	Site	Number of samples	Standard deviation of relative TRW and MaxD	Inter-serial correlation (average ± SD)	Common variance (first Eigen-vector)	First-order autocorrelation
Picea abies	TRW	REN	13	0.10	0.32 ± 0.20	32.3	0.75
	TRW	HAIa	14	0.20	0.68 ± 0.21	61.2	0.54
	TRW	FLAa	13	0.19	0.71 ± 0.46	61.1	0.76
	MaxD	REN	8	0.06	0.60 ± 0.06	54.2	−0.16
	MaxD	HAI	14	0.07	0.74 ± 0.08	69.7	0.03
	MaxD	FlA	13	0.07	0.64 ± 0.09	–	–
Pinus cembra	TRW	REN	8	0.16	0.28 ± 0.18	35.2	0.72
Pinus sylvestris	TRW	FLA	18	0.17	0.58 ± 0.23	55.3	0.58
Pinus cembra	MaxD	REN	3b	0.06	0.30 ± 0.06	37.7	0.19
Pinus sylvestris	MaxD	FLA	18	0.07	0.67 ± 0.09	55.2	0.21

TRW, Tree-ring width; MaxD, maximum diameter; SD, standard deviation; REN, Renon (Italy), an alpine forest in North Italy; HAI, Hainich, a lowland forest in Germany; FLA, a boreal forest in Northern Sweden (Flakaliden, FLA)

^aData from ITRDB (FH Schweingruber)

^bNumber of samples is not enough for significance

Fig. 3.

Standardized site tree-ring width and maximum density chronologies for Picea abies and Pinus cembra. The original data were standardized by correcting for an age-dependent trend

The inter-serial correlation (Table 3) that compares the variation between trees is similar for maximum density and for tree-ring width. The standard deviation of the inter-serial correlation was higher for tree-ring width than for maximum wood density, and it was highest at FLA and lowest at REN. Thus, there was relatively little variation between trees at FLA and HAI, but a large variation between trees at REN. Also, the common variance of the maximum density and tree-ring width was higher at FLA and HAI than at REN, indicating a weaker climatic signal at REN.

The first-order autocorrelation in tree-ring width, which is an indicator of the carry-over effect from one year to the next, is very high at all study sites. In fact, 29–58% of tree ring-width in a current year is explained by the growth rate in the previous year (Table 3). The autocorrelation in the tree rings is much larger than the autocorrelation of climatic parameters, which was 0.06, 0.08 and 0.16 for temperature, and 0.10, −0.12 and −0.06 for precipitation at the REN, HAI and FLA site, respectively (data not shown). This result indicates that only 0.3–2% of the climatic conditions in one year are explained by the climatic conditions in the previous year.

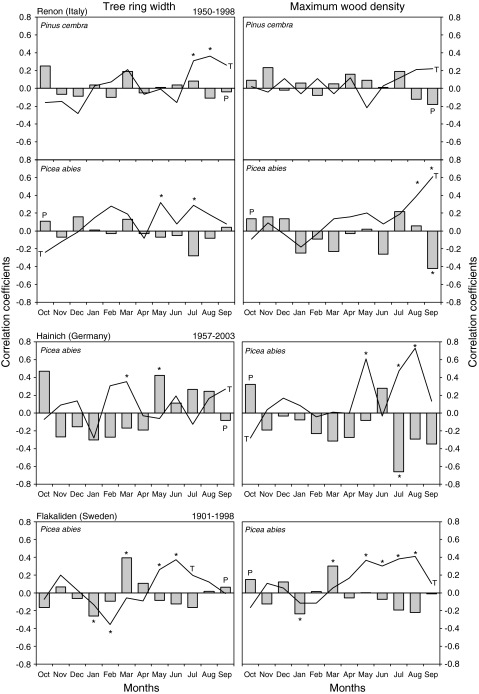
The correlation of long-term chronologies of tree growth and wood density with temperature and precipitation (time series 70–100 years) shows no general pattern between tree-ring width or maximum wood density and monthly mean values for precipitation and temperature (Fig. 4). Site-specific conditions are important. For example, late season temperature (August, September, October) correlated with tree-ring width at the alpine REN and boreal FLA sites, but the early season temperature (May, June, July) was significant at the lowland HAI site. Precipitation had no significant effect on tree-ring width at the alpine site, but late season precipitation correlated with tree-ring width at HAI, and early season precipitation was significant at FLA despite snow melt. In general, there is a stronger climatic effect at higher latitudes (Shiyatov 1986; Vaganov et al. 1996; Briffa et al. 1998) than at the alpine site. The climate signal was not as strongly expressed as that observed for maritime Mediterranean sites (Gonzales and Eckstein 2003).

Fig. 4.

Correlation coefficients of annual tree-ring and maximum density chronologies with climatic data: Lines indicate correlations with monthly mean temperature, columns indicate correlations with monthly precipitation. Asterisks indicate a significant correlation (P < 0.01)

In summary, we found that the long-term correlations between climate and tree-ring width and wood density are not very strong and that they are both site- and species-specific. For tree-ring width, late season temperatures (but not precipitation) are significant at REN, and spring temperatures and precipitations are significant at HAI and FLA. Extreme events of temperature and precipitation, such as in the dry year of 2003 (see Fig. 7), seem to be important.

Fig. 7.

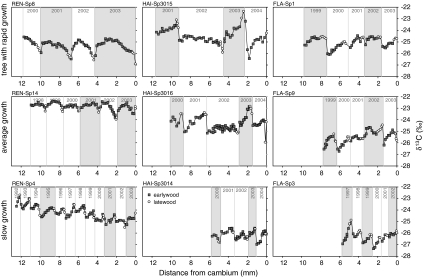
Seasonal courses of δ¹³C during consecutive years, taking a fast-growing tree, a tree with average growth rate and a slow-growing tree as an example. Closed symbols indicate early wood, open symbols indicate late wood. Columns: left Renon (REN), middle Hainich (HAI), right Flakaliden (FLA)

Relations between isotopic composition and cell lumen and tree-ring width

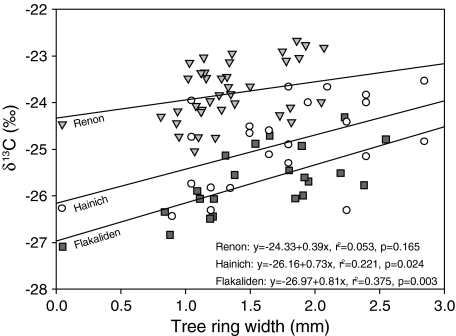
All sites show a positive correlation between the average value of δ¹³C within a tree ring and the width of tree rings (Fig. 5). This was unexpected because a more positive δ¹³C value would indicate an increasing stomatal limitation of photosynthesis with increasing ring width. The main differences between sites are the intercept values and a lower slope at the REN site. In narrow rings, the temperate and the boreal site are more similar than the alpine and the boreal site. The high δ¹³C values at REN could indicate water stress at the alpine site. This was not apparent in the correlations with long-term chronologies (Fig. 4).

Fig. 5.

Correlation between tree-ring width and average value of δ¹³C within tree rings for small (<3 mm) tree rings. The average δ¹³C value represents the numerical average of the individual measurements without taking changes in density into account. The higher density of late wood affects only 10–20% of the δ¹³C data

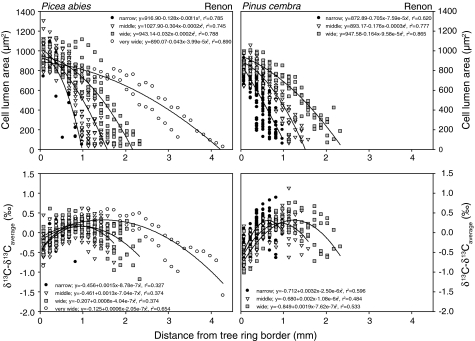
The variations in δ¹³C and wood anatomy within a tree ring were investigated for the REN site (Fig. 6) by calculating the deviation of δ¹³C from the average value for a given tree ring. Cell lumen area was used as a parameter because it is positively correlated with tracheid diameter (overall correlation 0.86, P < 0.0001), and it is negatively correlated with CWT (overall correlation −0.48, P < 0.01), cell-wall area and density (Lambers et al. 1998; Hubbard et al. 2001).

Fig. 6.

Relations between cell lumen area and δ¹³C with tree-ring width as expressed by distance from the tree ring boundary. The Renon site was taken as an example

Cell-lumen area shows the highest values at the beginning of the growing season and continually decreases later in the season. The decrease is steeper in narrow than in wide rings. The maximum values of cell lumen area are slightly higher for spruce (1063–1225 μm²) than for pine (956–1058 μm²). There is no obvious change at the border of early and late wood.

The seasonal changes of δ¹³C with tree-ring width can be seen to differ from the seasonal changes in cell-lumen area (Fig. 6). At the beginning of the season, average δ¹³C was 0.4–0.8‰ lower than the tree-ring average; it then increased with progressing tree-ring formation (see Fig. 5), but decreased again past a maximum. This decrease may only be small in narrow rings, but it is substantial in wide tree rings. Thus, in wide tree rings, the late wood δ¹³C may be lower than in the early wood, while in narrow rings δ¹³C in late wood is higher than in early wood of the same ring. The average amplitude of the intra-annual variation of δ¹³C is about 0.8–1.6‰ depending on years of growth.

The changes in δ¹³C within the tree rings (see Fig. 6) do not correlate with the observed long-term effect of climate on tree-ring width (Fig. 4) and may reflect seasonal changes in the contribution of storage products for tree-ring growth.

The seasonal and inter-annual variation of δ¹³C in spruce

In this section, we depict examples of the seasonal changes in δ¹³C in fast-, average- and slow-growing trees of Picea abies with the aim of demonstrating the tree-by-tree variability as well as inter-annual variability for the three sites (Fig. 7). The different numbers of years in Fig. 7 are due to the sampling procedure, where cores of different lengths were taken from fast- and slow-growing trees. Thus, not all cores reach the same depth from the cambium.

Figure 7 shows that the variation in δ¹³C within years (intra-annual variation) is highly variable between fast- and slow-growing trees. δ¹³C was either constant, increased or decreased over time, with no consistent relations.

In fast-growing trees, the δ¹³C of early wood was on average the same for all three sites (24.9 ± 0.6‰). The seasonal course of δ¹³C in fast-growing trees at REN showed an autumn decrease, even in the dry years of 2001 and 2003. At HAI, dry years (2001 and 2003) differ from wet years in that there was an increase in autumn δ¹³C in the dry years. At FLA also, there may be an increase in δ¹³C in the autumn of wet years, and there was no δ¹³C signal left in fast-growing trees of FLA during the very dry year of 2002.

Slow-growing trees δ¹³C showed a more consistent pattern than fast-growing trees. At REN, δ¹³C generally increased as the season progressed, which was opposite to the seasonal pattern observed in fast-growing trees. At HAI, the effect of a dry year was not apparent in slow-growing trees, while at FLA, the very dry year of 2002 did not leave a signal in slow-growing trees, but the lesser dry year of 1999 and 1997 resulted in a increase in δ¹³C.

The highest values of δ¹³C were found at REN for a tree of average growth rate (−22.8‰) with very little inter-annual and seasonal variation. The δ¹³C values were generally lower in slow-growing trees than in fast-growing ones. The lowest values were observed in slow-growing trees of HAI and FLA (−27‰). It should be pointed out that all trees were dominant in height and that none of the trees was a suppressed tree of the lower canopy.

There was a remarkable stepwise change in some trees in terms of δ¹³C between late wood and early wood of the next year. A decrease in δ¹³C across the tree ring boarder was observed at REN, where the lowest δ¹³C values were observed in autumn. This result contrasts with those obtained at the HAI and FLA sites, where the δ¹³C values of the early wood were generally lower than those of the late wood in autumn.

In order to obtain insight into the processes which link the late wood of one year with the early wood of the next year, we assessed whether the δ¹³C in ray cells differed from the δ¹³C in xylem cells (Table 4). The expectation was that ray cells, which contain the stored carbohydrates in conifers, would contain an additional isotope signal; i.e. the hypothesis was that ray cells would be heavier that the associated tracheids. However, at all sites, the δ¹³C in 1- to 3-year-old ray cells was 0.1–0.2‰ lower than that of the associated xylem. This difference disappeared after 4 years, i.e. ray cells were the same as xylem cells. A lower δ¹³C value could be the result of a continuation of lignin incorporation into ray cells (FH Schweingruber, personal communication), which would lower the δ¹³C of ray cells. Obviously, the bulk measurement of the whole cell is not fully conclusive and does not show the effects of carbohydrate turnover.

Table 4.

Difference between δ¹³C of xylem and ray cells

Year	Early (EW)-Late wood (LW)	Hainich δ13C-xylem	Hainich δ13C-ray	Hainich δ13C xylem-ray	Renon δ13Cxylem	Renon δ13C-ray	Renon δ13C xylem-ray	Flakaliden δ13C xylem-ray
2001	EW	−25.51 + 0.42	−25.98 + 0.42	−0.01 + 0.02
	LW	−25.44 + 0.79	−25.54 + 0.80	0.11 + 0.14
2002	EW	−25.62 + 0.56	−25.69 + 0.58	0.07 + 0.17				0.16
	LW	−25.84 + 0.37	−25.95 + 0.31	0.11 + 0.12	−24.66 + 1.20	−24.68 + 1.20	0.01 ± 0.05	0.21
2003	EW	−25.37 + 0.62	−25.60 + 0.68	0.23 + 0.13	−24.36 + 0.85	−24.48 + 0.83	0.12 ± 0.13
	LW	−23.91 + 1.39	−24.10 + 1.34	0.18 + 0.12	−24.31 + 1.35	−24.40 + 1.35	0.08 + 0.15
2004	EW	−26.11 + 0.61	−26.26 + 0.69	0.15 + 0.12
	LW	−25.44 + 0.79	−25.54 + 0.80	0.09 + 0.07

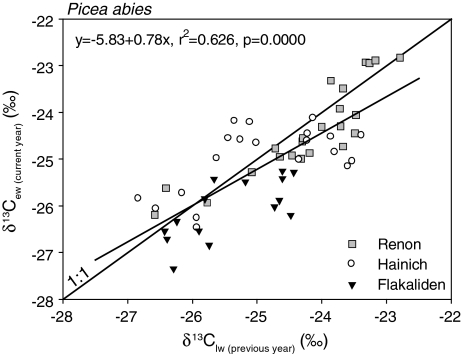
Independent of sites, yearly climate and growth rates (Fig. 8), we found a close correlation between the yearly average δ¹³C of a tree ring in a given year (t) and the average δ¹³C in the immediately preceding year (t-1). At low δ¹³C values, there was a tendency for the yearly average δ¹³C of early wood to be higher than that of late wood; in contrast, at high δ¹³C values, the yearly average δ¹³C in early wood would tend to be lower than that in late wood.

Fig. 8.

Relation between δ¹³C in late wood of previous tree ring and in early wood of current tree ring for spruce. The thin line shows the 1:1 relation. The regression lines are calculated by reduced axis regression

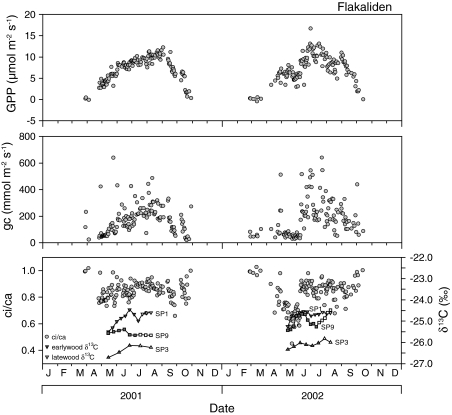
It was not possible to compare δ¹³C in wood with eddy flux data at all sites because the ecosystem flux at the alpine site REN is also determined by the activity of the grass cover on the forest floor, while the stand was too small at the lowland HAI site for eddy flux measurements. At the boreal site FLA, it was only possible to make a comparison for 2001 and 2002 before the eddy flux measurements terminated. The synchronization with growth was made on the basis of independent dendrometer measurements (Sune Linder, personal communication). We used gross primary productivity and canopy conductance to derive a canopy-scale ratio of the intercellular to ambient CO₂ concentration (ci/ca), which is not equal to leaf–level ci/ca, but rather a integrative estimate of ci/ca across the entire canopy confounded by other effects, such as surface evaporation and mixing sun and shade leaves. Figure 9 shows an increase in δ¹³C early in the season in both years, when canopy-scale ci/ca was increasing. Thus, the initial increase in δ¹³C is in contrast to the expectation that gas exchange parameters determine the isotope composition in wood. Also, the δ¹³C in the following year was lower or similar to that in the past autumn, which was contrasted by much lower ci/ca values. Thus, gross primary productivity, canopy conductance and canopy-scale ci/ca do not explain the observed variation in δ¹³C in wood.

Fig. 9.

Seasonal course of gross primary productivity (GPP) as measure of photosynthesis, canopy conductance (gc), the ratio of canopy-scale mesophyll internal and atmospheric CO₂ concentration (ci/ca) estimated based on eddy covariance flux measurements, and δ¹³C in wood for the boreal site at Flakaliden in 2001 and 2002. Closed symbols of δ¹³C indicate early wood, open symbols indicate late wood

The information obtained during our study, which is based on a total of three sites, 22 years of wood analysis and 760 individual observations, was analyzed with linear mixed-effects models to explore relationships between δ¹³C, wood anatomy, yearly climate and their dependency on different study sites (Table 5). Cell-wall area was related to cell lumen in early and late wood, and δ¹³C correlated with cell-wall area and lumen in early and late wood even if the significant interaction terms with site identity indicated differences in these relationships among sites. The relationships to climate parameters are complicated and mostly site-specific. Tree-ring width correlated with early season precipitation and late season temperature (mainly an effect of HAI). Vapor pressure deficit (VPD) and the total incoming radiation (R) were highly significant variables to explain variation in tree-ring width although their effects varied to some degree among sites as well. δ¹³C varied among sites, but it generally did not correlate with precipitation or temperature. However, despite site-specific differences, δ¹³C was highly significantly related to total radiation, VPD and the length of the growing season. The correlation between early wood of one year versus late wood of the immediately preceding year was highly significant.

Table 5.

Summary of linear mixed model analyses of the relationships between wood anatomy and carbon isotope ratios and their dependency on climatic variables

Relationship	Tested variablesa	Site	Variable	Interaction site × variable
All data δ13C vs. anatomy	CWA vs. LUM	4.93	130.29***	21.35***
	δ13C vs. CWA	6.00*	17.45***	14.46***
	δ13C vs. LUM	6.00*	4.62*	64.15***
Early wood	CWA vs. LUM	4.03	265.03***	40.46***
	δ13C vs. CWA	6.94*	16.19***	16.25***
	δ13C vs. LUM	6.94*	29.97***	24.63***
Late wood	CWA vs. LUM	15.60***	60.51***	14.86***
	δ13C vs. CWA	2.95	15.24***	4.14
	δ13C vs. LUM	2.95	6.23*	8.27*
TRW vs. climate	TRW vs. P-MJJ	3.23	7.38**	16.86***
	TRW vs. P-ASO	3.23	0.30	32.60***
	TRW vs. T-MJJ	3.23	1.07	1.64
	TRW vs. T-ASO	3.23	9.40**	2.85
	TRW vs. R-MJJ	3.23	21.67***	6.04*
	TRW vs. R-ASO	3.23	16.51***	9.61**
	TRW vs. VPD-MJJ	3.23	15.01***	11.09**
	TRW vs. VPD-ASO	3.23	18.09***	5.81
δ13C vs. TRW	δ13C vs.TRW	6.00*	3.19	1.83
Early wood	δ13C vs. TRW	6.94*	2.75	1.02
Late wood	δ13C vs. TRW	2.95	4.67*	4.31
TRW vs. growing season	TRW vs. length	3.23	11.09***	9.23**
LUM vs. growing season	LUM vs. length	9.43**	594.07***	0.45
LUM vs. Radiation	LUM vs. R-MJJ	9.43**	1089.27***	1.71
	LUM vs. R-ASO	9.43**	1089.11***	1.12
CWA vs. growing season	CWA vs. length	4.93	18.92***	5.93
δ13C vs. growing season	δ13C vs. length	6.00*	18.92***	2.14
δ13C vs. climate	δ13C vs. P-MJJ	6.00*	<0.01	14.23***
	δ13C vs. P-ASO	6.00*	2.43	8.55*
	δ13C vs. T-MJJ	6.00*	0.44	0.31
	δ13C vs. T-ASO	6.00*	1.08	9.76**
	δ13C vs. R-MJJ	6.00*	45.01***	14.58***
	δ13C vs. R-ASO	6.00*	40.08***	1.95
	δ13C vs. VPD-MJJ	6.00*	47.52***	7.02*
	δ13C vs. VPD-ASO	6.00*	43.60***	9.61**
Early vs. late wood preceding year		*	***	NS

*, **, *** Significant at the 5, 1 and 0.1% level, respectively

Models were fitted by the stepwise inclusion of variables. Listed are the values of likelihood ratio statistics that were applied to assess model improvement and the statistical significance of the variables

^aVariables: TRW, tree-ring width; CWA, cell-wall area; LUM, cell lumen; P, precipitation; T, temperature; R, incoming radiation; VPD, vapor pressure deficit; length, length of the growing season (days with average temperature >5°C); MJJ, early season (May, June, July); ASO, late season (August, Spetember, October)

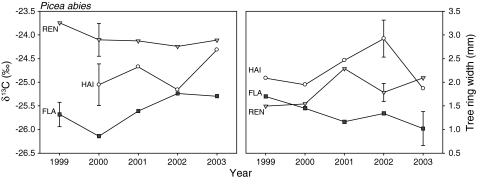
Figure 10 summarizes the yearly averages of all data points in terms of yearly δ¹³C and tree-ring width. There is a strong latitudinal effect of δ¹³C, which was highest at REN and lowest at FLA. Although REN receives 20–40% more solar radiation than HAI and FLA, respectively, tree-ring width at REN varies mostly between that at HAI and FLA. The HAI site receives only 30% more solar radiation than FLA, but the tree-ring width was found to be twofold larger. Despite high rainfall, REN appears to be a water-stressed site due to the special soil conditions, but water stress alone cannot explain the differences. Tree-ring width is lowest in Flakaliden, despite low δ¹³C. There are obviously additional factors which determine tree-ring width and δ¹³C, one of which is plant nutrition. FLA is nitrogen deficient (Table 1), while the nitrogen concentrations of needles were the highest at REN. High nitrogen availability is expected to increase carboxylation capacity and to amplify water stress, leading to an increase of δ¹³C in leaf and wood (Högberg et al. 1993; Livingston et al. 1999) and thus explain part of our observation.

Fig. 10.

Inter-annual variations in absolute values of δ¹³C in tree rings of spruce (all three studied sites) during the last 5 years (1999–2003). Vertical lines show the standard deviations

Discussion

This study was undertaken to investigate correlations between climate and tree-ring width and anatomy at long and short time scales and to explore whether δ¹³C could be used as an additional parameter to interpret tree-ring chronologies. We also wanted to know if there are carry-over effects from one year to the next that affect δ¹³C and growth. We investigated these questions by (1) studying climate correlations of long-term tree ring chronologies, (2) investigating in detail short-term seasonal observations using wood samples which were taken from forest sites ranging between boreal Scandinavia and alpine North Italy where trees grew in competitive interaction as forest stands.

In the long time series, temperature and precipitation correlated with tree-ring width and wood density, but these correlations were highly site and species specific, with spring-time temperatures and precipitations seeming to be most important. In contrast to the long-term correlations, at short time scales, we found only poor correlations between tree-ring width and temperature or precipitation. However, there was a highly significant correlation between tree-ring width, δ¹³C and lumen area and the length of the growing season, total net radiation and VPD. Our investigation also shows that there were large differences between fast- and slow-growing trees even though all trees were dominant canopy trees in which the δ¹³C signal was not affected by soil respiration. It is quite likely that the main driver for δ¹³C could be VPD. We were unable to test for effects of soil moisture because these data were not available as time series for all sites.

Our initial hypothesis was that the seasonal course of δ¹³C would allow an identification of dry years (i.e. years with below-average rainfall during the growing season) as had been described by Ferrio et al. (2003) in Pinus halepensis, Kagawa et al. (2003) in Larix gmelinii or Adams and Kolb (2004) in Pinus ponderosa. We observed a δ¹³C response in a fast-growing tree of HAI during the dry year of 2003; however, the effect disappeared in slow-growing dominant trees, and the δ¹³C increase did not increase at the other sites during years with a below-average rainfall. To the contrary, δ¹³C increased even in wet years at FLA. δ¹³C did not respond at REN. There is clearly an absence of a general and regular response of tree-ring width and δ¹³C to precipitation. The site effects and the effects of slow- versus fast-growing trees override the precipitation effect.

The seasonal increase in δ¹³C at FLA was not caused by drought. Rainfall at FLA is generally lower in August, but this did not affect canopy-scale ci/ca, and the δ¹³C of wood changed independently of canopy-scale ci/ca. We hypothesize that the increase in δ¹³C in autumn is caused by the reserve formation of products which are ¹³C-enriched and which then result in heavy early season wood.

In contrast, at REN, δ¹³C decreased in fast-growing trees but was constant or increased in slow-growing trees. Thus, the seasonal pattern is more complex at REN than at the FLA site and is not simply related to one climatic factor. However, slow- and fast-growing trees could represent different rooting depths at this alpine site, which consists of very stony soils. We cannot rule out that slow-rowing trees at the REN site experience water stress, but this was not apparent in the seasonal pattern of fast-growing trees.

At HAI, δ¹³C generally increased during the year, and this increase occurred concurrently with a 20% decrease in precipitation and a 40% decrease in solar radiation (Table 2). At this site, the effect was most pronounced in fast-growing trees and was not observable in slow-growing trees, which is opposite to the observation at REN.

In summary, TRW is correlated with early and late season precipitation in both the long-term and short-term time series. However, there was no simple relationship between δ¹³C and precipitation. There was a significant correlation with VPD, which is expected to close stomata, and with radiation, which is expected to open stomata. At FLA, the ci/ca remained constant during the autumn with low rainfall but relatively high radiation. A comparison of the three sites indicates that an interaction with nutrition is likely. The site with the lowest nitrogen concentrations in the needles had the lowest δ¹³C values. This result corresponds well with findings from nitrogen fertilization experiments in which low nitrogen availability resulted in low δ¹³C in the needles of Pinus sylvestris L. (Högberg et al. 1993; Betson et al. 2007), Picea abies Karst. (Högberg et al. 1993) and Picea glauca (Moench) Voss (Livingston et al. 1999). Possible mechanisms include changes in carboxylation capacity (Livingston et al. 1999) and an increase in susceptibility to climate-induced water stress with increasing nitrogen availability (Högberg et al. 1993; Betson et al. 2007).

The most striking observation is the close correlation between the early wood of a given year and the late wood of immediately preceding year, which indicates a carry-over effect of stored products (Helle and Schleser 2004; Skomarkova et al. 2006). Thus, our hypothesis was that the δ¹³C in one year is related to the δ¹³C in the previous year through storage products. The use of stored carbohydrates and lipids for growth in consecutive years has been confirmed by Keel et al. (2007) in a permanent ¹³C-fumigation experiment of deciduous trees. The accumulation of reserves involves an accumulation of carbohydrates in the autumn and a depletion of carbohydrates in the spring. It also involves an accumulation of lipids during the summer, which are metabolized in spruce in the autumn (Hoell 1985). However, other tree species, such as Larix, may accumulate lipids during the winter (Sudachkova et al. 2004). The effect of storage has been demonstrated in deciduous trees (Helle and Schleser 2004) which have a larger number of wood parenchyma cells than conifers (Schweingruber et al. 2007). One would not expect major storage pools in conifers, which have only a few ray cells. However, Hoell (1985) did demonstrate the turnover of soluble carbohydrates in conifers, and Von Felten et al. (2007) estimated that 42% of new wood in Larix originates from storage products.

Direct proof that stored carbohydrates of one year contribute to the growth of the next year was presented for conifers by Hansen and Beck (1994), who pulse-labeled young Pinus sylvestris trees with ¹⁴C in the field and demonstrated the incorporation of ¹⁴C into wood of the following year. The process was biochemically further complicated by the fact that starch was transformed into various soluble carbohydrates, such as maltotriose, galactose, arabinose and rhamnose in the winter as a mechanism of cold hardiness (Hansen and Beck 1994). Thus, the breakdown of starch in wood results in the production of triose-phosphates, which results again in a ¹³C depletion step (Gleixner et al. 1998). Obviously, it is also not possible to demonstrate fluxes of ¹³C in retrospect using tree rings and ray cells.

Gessler et al. (2008) measured δ¹³C real time in the phloem sap of Rhizinus and found an increase as well as a decrease in δ¹³C in the phloem sap compared to the immediate photosynthetic product, depending on whether the phloem sugars were derived from sucrose or from starch or from sucrose passing through the cell metabolic pathways, including the formation of triose-phosphates. No fractionation was found during phloem transport. However, this experiment did not include the formation of wood in a seasonal climate, which may be supported by carbohydrates from ray cells in the wood and not involve phloem transport. This situation is different from that of continually fast-growing trees (Pinus radiata: Walcroft et al. 1997; Eucalyptus globulus: Pate and Arthur 1998) where a correlation between the phloem sap isotope signal and δ¹³C in wood has been demonstrated. During the loading of storage cells, carbohydrates move from the phloem via the cambium and the xylem initials into the ray cells of existing wood. If wood formation has priority over storage, the storage products would be expected to become relatively enriched in ¹³C (increasing δ¹³C). However, if storage and cold hardiness has priority, then δ¹³C could also be depleted (decreasing δ¹³C). In contrast, during carbohydrate mobilization in the spring, carbohydrates move from ray cells into the cambium without entering the phloem. It would be the mixture of the enriched mobilized carbohydrates and the phloem-derived carbohydrates from spring photosynthesis which determines the δ¹³C of wood. An additional important step of discrimination takes place during wood respiration due to the breakdown of carbohydrates into triose-phosphates. However, respiratory CO₂ is ¹³C enriched (Brandes et al. 2006), which does not explain the enrichment of δ¹³C in stems (Damesin and Lelarge 2003).

In our study, a stepwise change between late and early wood of consecutive years was observed, but this stepwise change was not always in the same direction, as was predicted by Helle and Schleser (2004). We observed all possible permutations of alternative responses depending on the site, species and climate. Thus, the linkage between the δ¹³C of wood and climate is not constant. There are many ways which link δ¹³C in wood, storage and climate and which govern the relation between tree ring width and δ¹³C. Thus, based on our results, we conclude that tree-ring width is not consistently related to δ¹³C in seasonal climates, and both are only indirectly linked to climate.

Conclusion

The carry-over effect, based on correlations between the δ¹³C of early wood and the δ¹³C of late wood of the immediately preceding year is significant in conifers. However, depending on sites and specific meteorological conditions, other effects, such as whole-wood composition, the isotope signal of storage products or a dry autumn leading to dry spring result in additional variation.

The use of a 5°C average temperature as a proxy for the start of the growing season appears to be robust for boreal, temperate and Mediterranean sites. The correlations in this study are insensitive to this proxy, but more elaborate phasing studies are needed in the future.