Response Linearity of Alert Monkey Non-Eye Movement Vestibular Nucleus Neurons During Sinusoidal Yaw Rotation

Abstract

Vestibular afferents display linear responses over a range of amplitudes and frequencies, but comparable data for central vestibular neurons are lacking. To examine the effect of stimulus frequency and magnitude on the response sensitivity and linearity of non-eye movement central vestibular neurons, we recorded from the vestibular nuclei in awake rhesus macaques during sinusoidal yaw rotation at frequencies between 0.1 and 2 Hz and between 7.5 and 210°/s peak velocity. The dynamics of the neurons' responses across frequencies, while holding peak velocity constant, was consistent with previous studies. However, as the peak velocity was varied, while holding the frequency constant, neurons demonstrated lower sensitivities with increasing peak velocity, even at the lowest peak velocities tested. With increasing peak velocity, the proportion of neurons that silenced during a portion of the response increased. However, the decrease in sensitivity of these neurons with higher peak velocities of rotation was not due to increased silencing during the inhibitory portion of the cycle. Rather the neurons displayed peak firing rates that did not increase in proportion to head velocity as the peak velocity of rotation increased. These data suggest that, unlike vestibular afferents, the central vestibular neurons without eye movement sensitivity examined in this study do not follow linear systems principles even at low velocities.

INTRODUCTION

Responses of mammalian vestibular neurons, both peripheral and central, have been described for various stimulation paradigms. In vestibular afferent fibers, trade-off between the sensitivity of the cell's response and its linear range has been reported. Slow firing but sensitive irregular afferents effectively code acceleration only in the excitatory direction because once the firing rate of the neuron reaches inhibitory cutoff (rate of zero) the response of the cell remains zero (unchanged) with progressively more vigorous stimulation (Goldberg and Fernandez 1971b). Although the dynamics of vestibular afferent responses have been discussed extensively, less well understood is the impact of inhibitory cutoff and other nonlinearities in central vestibular neurons on the function of the vestibular system overall. Despite the known asymmetries in the primary afferent discharge, central mechanisms, especially the presence of a commissural system that provides informational redundancy in the vestibular nuclei, have been proposed to fill in the missing inhibitory information from one side of the midline with excitatory information from the other side (Abend 1978) to enable a linear output. Other authors have speculated on the possibilities of nonlinear processing in the central vestibular system (Minor et al. 1999) and the role of population coding, which might provide linear output despite nonlinear elements (Hospedales et al. 2008).

Previous studies have examined properties of central vestibular neurons. An increase in inhibitory cutoff has been described with increasing peak velocity in vestibular nucleus neurons in the decerebrate cat (Jones and Milsum 1970). These authors also reported on the sensitivity versus frequency relationship of central vestibular neurons in the same preparation (Jones and Milsum 1971). Their findings were expanded by subsequent investigators in nonprimate species (Broussard et al. 2004; Schneider and Anderson 1976; Shinoda and Yoshida 1974) and in alert monkeys (Buttner et al. 1978; Cullen and McCrea 1993; Dickman and Angelaki 2004; Fuchs and Kimm 1975; Keller and Kamath 1975; Ramachandran and Lisberger 2008). Most of these studies concerned the effect of frequency on the sensitivity and phase of responses of individual neurons and demonstrate a relatively flat relationship for frequencies between 0.1 and 2.0 Hz with an increasing phase lead relative to velocity as the frequency of rotation increases. Although studies of central vestibular neurons in vitro have addressed many aspects of response linearity (Bagnall et al. 2008; du Lac and Lisberger 1995; Pfanzelt et al. 2008), little systematic work has been done in vivo to understand how the magnitude of stimulation influences responses of central vestibular neurons. The linearity of the responses of central neurons over a range of rotational stimuli has not been directly addressed in normal, alert animals, although such responses have been reported in cats with unilateral vestibular lesions (Heskin-Sweezie et al. 2007).

The current study was undertaken to investigate how stimulus magnitude is coded in the vestibular nuclei. We limited our analysis to vestibular neurons without sensitivity to eye position or eye velocity (non-eye movement [NEM] or vestibular-only cells) to avoid confounding the analysis by having to parse out the sensory from the motor component of the response of vestibular neurons that carry eye-related information. Similar to the dynamics noted in primary afferent fibers, we hypothesized that the amplitude of neuronal responses would increase linearly proportional to the amplitude of stimulation at lower peak velocities before saturating at higher peak velocities. We found, however, that even at relatively low peak velocities, the relationship between amplitude of rotation and neuronal responses was not linear and, at higher peak velocities, the amplitude of neuronal responses did not increase with increasing velocity.

METHODS

Animal preparation

For this study, vestibular nuclei neurons were recorded from five juvenile rhesus monkeys (Macaca mulatta), with weights ranging from 4.0 to 6.3 kg. The animals were prepared for these experiments in two sterile surgical procedures performed under inhaled isoflurane anesthesia. The first surgery was for placement of a head cap used for subsequent immobilization of the head and placement of recording electrodes. Six inverted stainless steel bolts were implanted into the skull through small T-shaped craniotomies and were secured to dental acrylic. A circular recording chamber holding a Delrin platform with holes drilled every millimeter in a 2-cm-diameter grid was placed stereotaxically over the bilateral vestibular nuclei, such that all areas of the nuclei could be accessed, and was secured with the dental acrylic. A metal sheath oriented parallel to the interaural axis was also secured to the implant with acrylic for later use in securing the animal's head during the experiments.

In the second surgery, the animals were chronically implanted with a three-turn 15.5-mm search coil of braided insulated stainless steel wire (Cooner AS631) sutured directly to the sclera with 7-0 prolene or silk sutures in one or both eyes. Conjunctiva was loosely closed over the coils. After both surgeries, the animals were treated with postoperative antibiotics for 7 days and intramuscular buprenorphine (0.01 mg/kg) for pain control twice a day for 3 days. All surgical procedures were performed according to institutional and National Institutes of Health guidelines and under a protocol approved by the Institutional Animal Care and Use Committee at the University of Texas Medical Branch.

Experimental setup

During the experiments, the monkeys were placed in custom-manufactured primate chairs. A square metal rod was placed transversely through the transverse metal sheath attached to the animal's head implant to immobilize the animals head relative to the upright extensions of the monkey's chair. The primate chair was designed to hold the monkey's head pitched 20° nose-down to place the horizontal canals in a coplanar position to the horizontal. A two-field magnetic search coil system (C-N-C Engineering Systems, Seattle, WA) was mounted on the primate chair centered on the animal's eyes. The chair was suspended from horizontal posts that allowed several degrees of manual pitch rotation (earlier experiments) or was mounted onto a motor-controlled pitch platform (±24°, later experiments). This pitch axis was used only to confirm that all of the neurons recorded were primarily associated with the yaw axis of rotation. The pitch mechanism and chair were in turn mounted onto a motorized turntable in the horizontal plane that was, in turn, mounted onto a linear air bearing that moved along a horizontal granite beam. The air bearing was positioned along the sled using a cable capstan system connected to a motor. The yaw axis and linear sled motors were initially servo controlled using a commercial motor control program (Acroloop; Parker Hannifin, Rohnert Park, CA). Later, the control system and experimental apparatus were upgraded to be motorized in the pitch plane and all three motors (pitch, yaw, and linear) were controlled by custom-written software (LabVIEW, National Instruments, Austin, TX).

Animals were trained to fixate and pursue laser targets projected onto a white screen using a laser projected in an x–y mirror galvanometer system (General Scanning, Billerica, MA). An additional laser system attached to the primate chair allowed for projection of head-fixed targets. Eye coil signals were calibrated by behavior training, rewarding animals for looking at moving and stationary targets with increasing requirements for accuracy as we became more certain that the animal was performing the task as required. This system allowed measurement of horizontal and vertical eye positions in either one or both eyes. Eye position calibration was stored in the data acquisition software but was adjusted daily as necessary. In the current report, the neurons described did not have eye position or velocity sensitivity, but oculomotor training permitted us to keep the animal alert during the recording period. The animal received a juice reward for fixating the target within a window of tolerance and a length of time prescribed by the investigator. Typically, for a well-trained monkey, the window was 2° horizontally or vertically around the target and juice was given for every 0.5 to 2 s of continuous fixation. The laser targets and reward mechanism were controlled by computers running custom software written in LabVIEW.

Neural recordings

Extracellular recordings used epoxy-coated tungsten microelectrodes (2–10 MΩ impedance, FHC, Bowdointam, ME). Ensheathed in 26-gauge guide tubes, electrodes were introduced into the brain via predrilled holes through the acrylic and calvarium aligned with the stereotaxically implanted Delrin grid. The guide tubes placed the tip of the electrode into the ventral cerebellum. Electrodes were then driven through the guide cannula ≤15 mm through the vestibular nuclei using a remote-controlled hydraulic microdrive. Neuronal activity was amplified and filtered (100 to 10,000 Hz). This filtered activity was stored to disk with a sampling rate of 40 kHz for off-line analysis.

To facilitate accurate electrode placement into the vestibular nuclei, we first identified the abducens nuclei bilaterally, relying on the characteristic homogeneous burst-tonic firing pattern (Fuchs and Luschei 1970; Fuchs et al. 1988). Subsequent recordings concentrated on the area posterior (1–5 mm) and lateral (0–4 mm) to the center of either abducens nucleus. Location of the recording sites was confirmed to be in the rostral half of the vestibular nucleus, from the level of the abducens nucleus rostrally, to the caudal extent of the lateral vestibular nucleus caudally, including the rostral medial vestibular nucleus and medial superior vestibular nucleus, in two monkeys. In a third monkey, the histological material was lost by the histology core facility, and the last two monkeys are still alive.

For individual recording sessions the search stimulus to find yaw-sensitive neurons was sinusoidal yaw rotation at 0.5 Hz and 30 or 60°/s with a space-fixed target in place. Once a candidate neuron was identified based on auditory identification of firing in phase with rotation, a standard protocol was followed. Eye movement sensitivity was evaluated during saccades to horizontal and vertical targets (spaced every 5°, for ±20°), untargeted saccades, and pursuit to horizontal targets sinusoidally moving at 0.25 Hz, ±10°. Only neurons without eye position or eye velocity sensitivity are included in this report, although some demonstrated variable degrees of pausing for saccades. Dynamic sensitivity in the pitch plane was tested to exclude neurons related to vertical canal signals.

Next, recordings were made with the animal rewarded for fixating head-fixed targets in the dark during sinusoidal rotations at various frequencies between 0.1 and 2.0 Hz (protocol order was 0.5, 1.0, 2.0, 0.1, 0.2, 0.3, 0.8, then 1.5 Hz), holding the peak velocity constant at 60°/s (Fig. 1 A). At each frequency, from 40 cycles (at 2.0 Hz) to six cycles (at 0.1 Hz) were recorded to give a representative response. The next portion of the protocol was to hold the frequency of yaw rotation constant at 0.5 Hz and vary the peak velocity from as low as 7.5°/s to 210°/s in ascending order, to evaluate for saturation of the firing rate with higher peak velocities and accelerations (Fig. 1C). In the majority of our recording sessions, we tested only the range between 30 and 210°/s, but later we added either 7.5 or 15°/s amplitude. Last, sensitivity to linear acceleration at 0.5 Hz, peak acceleration of 0.2 g, was tested in four to six directions in the horizontal plane [nasooccipital (0°), interaural (90°), and 45° on either side of nasooccipital (−45 and 45°), or at 0, 30, 60, 90, 120, and 150°] by translating the animal along the linear sled. For the purposes of this report, linear sensitivity is included to distinguish neurons that have convergent linear and rotational sensitivities. All recordings were done in a dark room. Light entry to the room was negligible because the doors and other openings to the room were extensively shrouded.

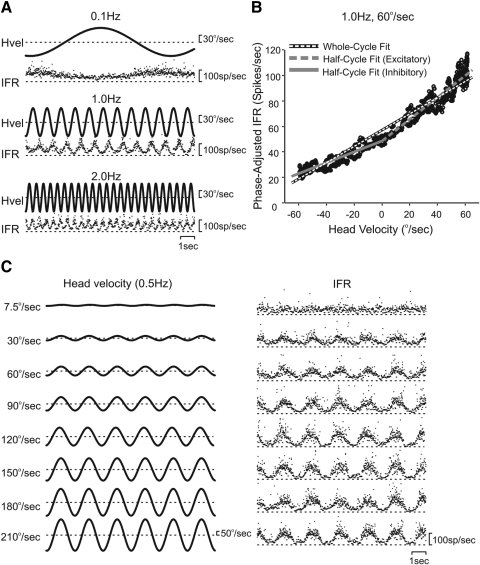
FIG. 1.

Example of one non-eye movement (NEM) neuron's responses to yaw rotation. A: frequency series at peak velocity of 60°/s (only 3 frequencies shown of the 8 tested), 12 s of data shown Hvel, head velocity; IFR, instantaneous firing rate. Sensitivities of this neuron at 0.1, 1.0, and 2.0 Hz are 0.37, 0.68, and 0.51 spikes·s⁻¹/deg·s⁻¹, respectively. B: half-cycle analysis of the middle frequency (1.0 Hz) demonstrating calculation of the half-cycle sensitivity for the inhibitory and excitatory phases of the cycle. Excitatory half cycle sensitivity 0.64 spikes·s⁻¹/deg·s⁻¹. Inhibitory half cycle sensitivity 0.45 spikes·s⁻¹/deg·s⁻¹. Whole-cycle sensitivity 0.55 spikes·s⁻¹/deg·s⁻¹. C: amplitude series when holding the frequency constant at 0.5 Hz and varying the peak velocity from 7.5 to 210°/s. Sensitivities of this neuron at 7.5, 30, 60, 90, 120, 150, 180, and 210°/s are 1.03, 0.79, 0.54, 0.52, 0.44, 0.36, 0.29, and 0.25 spikes·s⁻¹/deg·s⁻¹, respectively.

Data analysis

All data were analyzed off-line using a suite of custom programs written in LabVIEW. Initially, the microelectrode recording was fed into a program that has the primary function of discriminating the spikes and creating a spike train using a combination of feature analysis and a time–amplitude window. A second program converted the raw spike times into an instantaneous firing rate trace by taking the reciprocal of the interval since the previous spike. The eye movement trace could be desaccaded using an eye acceleration filter. The data were desaccaded for all units with any suggestion of pauses for saccades. Rotator velocity and instantaneous firing rate for all selected cycles were averaged together and fit using a Levenberg–Marquardt least-squares fitting algorithm. The relationship between the stimulus and response fit was used to calculate the sensitivity of the response [response amplitude (spikes/s)/stimulus amplitude (deg/s)] and the phase relationship between the stimulus and response. For purposes of later analysis, the peak firing rate was defined as the peak of the response fit to the averaged reciprocal interspike interval data. The sensitivities of the excitatory and inhibitory half-cycles of the response were also calculated individually by first shifting the response relative to the stimulus by the phase relationship calculated with the whole-cycle fit to align the response peak to the stimulus peak and then plotting the response versus the stimulus on an x–y plot (Fig. 1B). Regressions were then fit for the excitatory half-cycle and the inhibitory half-cycle such that the regression lines shared a common origin (the bias firing rate, defined as the rate of firing at the transition from ipsilateral to contralateral rotation, which is the point where head velocity is zero). The slope of the regression in the excitatory direction is the excitatory half-cycle sensitivity. The slope of the regression line in the inhibitory direction is the inhibitory half-cycle sensitivity. For neurons with very low baseline firing rates that were silent for much of the cycle, half-cycle sensitivities could not be reliably calculated. Neurons with unusually unstable bias firing rates, sensitivities, or phases were scrutinized before inclusion in the data set to include all of the natural variability in the unit responses but exclude poorly isolated neurons.

Eye movement sensitivity was evaluated after desaccading the eye movement trace for the smooth pursuit, saccade to targets, and saccade in the dark data by creating a regression of eye position against firing rate and eye velocity against firing rate. Only neurons that did not demonstrate a clear relationship with eye position or velocity were included in this report. No a priori sensitivity cutoff was used to select which neurons were included in the study. Neurons with low sensitivity were included if the phase of the response was consistent across cycles and across amplitudes and frequencies. All such neurons were rechecked to confirm the consistency of the spike waveform throughout the recording. In actuality, all included neurons had sensitivity of ≥0.19 spikes⁻¹/deg·s⁻¹ for at least one of the frequencies tested at peak velocity of 60°/s. Neurons were considered translationally sensitive only if they modulated >20 spikes·s⁻¹·g⁻¹ for one of the orientations at 0.5 Hz with peak acceleration at 0.2 g (with g = 9.81 m/s²), similar to other studies (Dickman and Angelaki 2002; Zhou et al. 2006).

RESULTS

Of 263 neurons that were well characterized, 191 NEM units are included in this study; 72 units with eye movement sensitivity were excluded. Units were classified as type I or type II based on the phase of response (Duensing and Schaeffer 1958). The 135 NEM units that responded with a phase lead or lag within 90° relative to ipsilateral head velocity were assigned to the type I category. The 56 NEM units that responded within 90° of contralateral angular velocity were assigned to type II. Because phase varied with frequency and because some of the central neurons showed responses better aligned with acceleration than with velocity, a few neurons were difficult to classify as either type I or type II. For these units, we classified them according to the phase of their response at 0.5 Hz.

Sensitivity

Figure 2 demonstrates the responses of type I and type II NEM neurons over the frequency range tested with the peak velocity held constant at 60°/s. Most neurons demonstrated a phase lead with respect to either ipsilateral or contralateral velocity that increased with higher frequencies, consistent with previous reports. There was a high-frequency enhancement of response sensitivity for type I neurons [mean ± SD at 0.1 Hz = 0.45 ± 0.35 spikes·s⁻¹/deg·s⁻¹ to 2.0 Hz = 0.81 ± 0.57 spikes·s⁻¹/deg·s⁻¹, ANOVA, F_(7,764) = 9.141, P < 0.0001]. For type II neurons, there was also a sensitivity enhancement at higher frequencies [mean ± SD at 0.1 Hz = 0.25 ± 0.13 spikes·s⁻¹/deg·s⁻¹ to 2.0 Hz = 0.51 ± 0.34 spikes·s⁻¹/deg·s⁻¹, ANOVA, F_(7,299) = 6.196, P < 0.0001]. The sensitivity of type I neurons was greater than the sensitivity of type II neurons at all frequencies tested at peak velocity of 60°/s (t-test, P < 0.001 for all eight frequencies). Phase leads relative to peak velocity were greater for type I than those for type II neurons (Mann–Whitney U test, P < 0.001 for all frequencies except 1.5 Hz, P = 0.027).

FIG. 2.

Bode style plots of sensitivity and phase vs. frequency for type I and type II neurons. Frequency and sensitivity plotted in log–log scale. Sensitivity is calculated based on fitting the entire response cycle. Gray open circles are for individual neurons; black solid circles are averages at each frequency. Due to changes in tuning of the rotator, the actual frequency of the rotation varied around intended frequency for some trials. SE is represented by the error bars, which are small relative to the size of the symbol.

Of the type I neurons, 88 were tested for linear sensitivity. Of these 64% were sensitive to translational motion in the horizontal plane and thus are classified as convergent neurons. Similarly, 64% of the 33 type II neurons tested demonstrated convergence of translational and rotational sensitivity.

Figure 3, A and B demonstrates the effect of peak velocity on the sensitivity of the response with the frequency held at 0.5 Hz. There is a clear decrement in the sensitivity of the response as the peak velocity increases [type I neurons: ANOVA, F_(8,634) = 30.056, P < 0.0001; type II neurons: ANOVA, F_(8,216) = 9.795, P < 0.0001]. The sensitivity of the response tested on individual neurons decreased significantly between 15 and 30°/s for both type I and type II neurons (P < 0.0001, paired t-test and P = 0.007, Bonferroni, respectively).

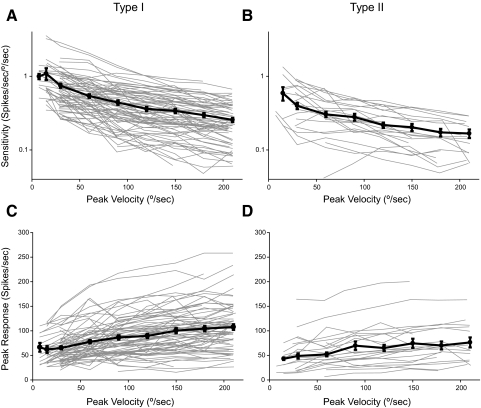
FIG. 3.

Sensitivity (A, B) and peak of the response (C, D) as a function of the peak velocity of rotation for type I (A, C) and type II (B, D) neurons. Faint lines are data for the 74 type I and 24 type II neurons for which data are available for at least 5 velocities of rotation. Solid lines and circles are the average for all units studied. Error bars are SEs. Sensitivity plotted in log scale (A and B).

The sensitivity of the response declined with increasing peak velocity; thus the peak response rate of the neurons did not grow in proportion to the peak of the stimulus. In Fig. 1C, the response of one unit to increasing peak velocities of rotation are shown. The responses at 90–210°/s peak velocity showed little noticeable difference. Figure 3, C and D demonstrates that for both type I and type II neurons, as the amplitude of the stimulus was increased, the peak response rate of the individual neurons saturated for velocities >90°/s. For the type I neurons, there was an effect of peak velocity on peak response [ANOVA, F_(8,634) = 10.292, P < 0.0001], although there was no significant difference in the peak response between the 90, 120, 150, 180, and 210°/s trials (Bonferroni, P > 0.05 for all comparisons). For type II neurons, there was an effect of peak velocity on peak response [ANOVA, F_(8,216) = 2.635, P = 0.009], although no significant difference was found in the peak responses between any of the eight velocities tested (Bonferroni, all P > 0.05).

Response rectification

One source of nonlinearity explored was the proportion of neurons that were silent in some portion of their cycle. Neurons for which the fitted response amplitude was greater than the bias firing rate (sensitivity × stimulus peak velocity > bias firing rate) were classified as rectified because, for these neurons, a portion of the response cycle was below zero and the neurons were thus silent for a portion of the cycle. Table 1 and Table 2 demonstrate the effects of rotational frequency and peak velocity on the proportion of neurons that were silenced (rectified), respectively. The trend toward a higher proportion of neurons silencing during the inhibitory portion of the cycle as frequency increased, while holding velocity constant at 60°/s, was significant for type I but not type II neurons (chi-square, P = 0.017 and P = 0.560, respectively). By holding frequency constant at 0.5 Hz, there was a very strong effect whereby higher peak velocities resulted in a higher proportion of rectified neurons for type I but not for type II cells (chi-square, P < 0.0001 and P = 0.06, respectively).

TABLE 1.

Effect of rectification on the response of neurons by frequency

Type	Frequency, Hz	Amplitude, deg/s	n	Percentage rectified	Rectified			Not Rectified
										Bias, spikes/s	Sensitivity, spikes·s−1/deg·s−1		Bias, spikes/s	Sensitivity, spikes·s−1/deg·s−1
					Whole Cycle	Half-Cycle	Whole Cycle	Half-Cycle
I	0.1	60	70	18.6%	19 ± 22	0.73 ± 0.53	0.71 ± 0.47	50 ± 26*	0.39 ± 0.26*	0.39 ± 0.26*
I	0.2	60	113	23.9%	20 ± 23	0.68 ± 0.42	0.80 ± 0.44	46 ± 23*	0.36 ± 0.23*	0.38 ± 0.27*
I	0.3	60	72	33.3%	23 ± 19	0.74 ± 0.42	0.82 ± 0.46	52 ± 30*	0.39 ± 0.19*	0.40 ± 0.22*
I	0.5	60	127	31.5%	22 ± 19	0.72 ± 0.43	0.81 ± 0.41	53 ± 26*	0.45 ± 0.25*	0.48 ± 0.26*
I	0.8	60	102	33.3%	25 ± 21	0.88 ± 0.50	0.91 ± 0.47	50 ± 24*	0.42 ± 0.24*	0.43 ± 0.24*
I	1.0	60	119	35.3%	25 ± 21	0.89 ± 0.49	0.94 ± 0.46	53 ± 26*	0.46 ± 0.25*	0.50 ± 0.29*
I	1.5	60	76	38.2%	28 ± 18	1.09 ± 0.56	1.11 ± 0.62	56 ± 24*	0.50 ± 0.29*	0.48 ± 0.33*
I	2.0	60	86	44.2%	28 ± 22	1.11 ± 0.62	1.18 ± 0.64	60 ± 30*	0.55 ± 0.31*	0.57 ± 0.40*
II	0.1	60	30	13.3%	6 ± 2	0.21 ± 0.05	0.33 ± 0.10	37 ± 18*	0.25 ± 0.14	0.27 ± 0.19
II	0.2	60	45	15.6%	4 ± 4	0.26 ± 0.14	0.29 ± 0.06	39 ± 21*	0.28 ± 0.15	0.36 ± 0.26
II	0.3	60	25	20.0%	9 ± 7	0.37 ± 0.17	0.49 ± 0.22	43 ± 26*	0.33 ± 0.16	0.41 ± 0.29
II	0.5	60	56	23.2%	7 ± 7	0.41 ± 0.28	0.48 ± 0.26	38 ± 24*	0.27 ± 0.15	0.32 ± 0.22*
II	0.8	60	41	19.5%	12 ± 15	0.41 ± 0.29	0.42 ± 0.33	41 ± 25*	0.31 ± 0.14	0.39 ± 0.20
II	1.0	60	49	22.5%	10 ± 8	0.42 ± 0.30	0.39 ± 0.16	42 ± 24*	0.32 ± 0.15	0.37 ± 0.20
II	1.5	60	25	36.0%	18 ± 10	0.45 ± 0.20	0.25 ± 0.23	55 ± 33*	0.43 ± 0.23	0.44 ± 0.25
II	2.0	60	31	25.8%	12 ± 10	0.74 ± 0.43	0.69 ± 0.25	52 ± 27*	0.43 ± 0.26	0.42 ± 0.38*

Values are means ± SD.

^*P < 0.01 (Mann–Whitney U test), comparing rectified with not rectified.

TABLE 2.

Effect of rectification on the response of neurons by peak velocity

Type	Frequency, Hz	Amplitude, deg/s	n	Percentage rectified	Rectified			Not Rectified
										Bias, spikes/s	Sensitivity, spikes·s−1/deg·s−1		Bias, spikes/s	Sensitivity, spikes·s−1/deg·s−1
					Whole Cycle	Half-Cycle	Whole Cycle	Half-Cycle
I	0.5	15	20	5.0%	NA	NA	NA	46 ± 24	1.07 ± 0.90	1.10 ± 0.95
I	0.5	30	93	17.2%	21 ± 18	1.28 ± 0.65	1.50 ± 0.77	47 ± 24*	0.64 ± 0.34*	0.67 ± 0.34*
I	0.5	60	127	31.5%	22 ± 19	0.72 ± 0.43	0.81 ± 0.41	53 ± 26*	0.45 ± 0.25*	0.48 ± 0.26*
I	0.5	90	76	39.4%	33 ± 20	0.63 ± 0.30	0.68 ± 0.33	55 ± 26*	0.32 ± 0.17*	0.32 ± 0.18*
I	0.5	120	99	45.5%	31 ± 24	0.49 ± 0.25	0.57 ± 0.26	55 ± 27*	0.25 ± 0.14*	0.24 ± 0.14*
I	0.5	150	72	43.1%	34 ± 22	0.47 ± 0.21	0.52 ± 0.24	60 ± 27*	0.24 ± 0.12*	0.22 ± 0.13*
I	0.5	180	71	49.3%	36 ± 22	0.40 ± 0.18	0.44 ± 0.21	61 ± 29*	0.20 ± 0.10*	0.20 ± 0.10*
I	0.5	210	66	50.0%	38 ± 26	0.33 ± 0.15	0.37 ± 0.18	65 ± 28*	0.18 ± 0.08*	0.17 ± 0.08*
II	0.5	15	8	0.0%	NA	NA	NA	37 ± 18	0.66 ± 0.38	0.80 ± 0.41
II	0.5	30	36	13.9%	8 ± 7	0.38 ± 0.16	0.51 ± 0.24	39 ± 26*	0.40 ± 0.24	0.51 ± 0.33
II	0.5	60	56	23.2%	7 ± 7	0.42 ± 0.28	0.48 ± 0.26	38 ± 24*	0.27 ± 0.15	0.32 ± 0.22*
II	0.5	90	22	27.3%	20 ± 15	0.30 ± 0.16	0.18 ± 0.18	50 ± 26	0.27 ± 0.13	0.21 ± 0.17
II	0.5	120	36	41.7%	14 ± 12	0.20 ± 0.10	0.29 ± 0.14	47 ± 26*	0.23 ± 0.11	0.29 ± 0.18
II	0.5	150	22	31.8%	26 ± 13	0.24 ± 0.11	0.27 ± 0.14	48 ± 30	0.28 ± 0.10	0.22 ± 0.18
II	0.5	180	20	35.0%	21 ± 15	0.20 ± 0.12	0.24 ± 0.15	45 ± 19	0.16 ± 0.07	0.17 ± 0.15
II	0.5	210	15	33.0%	26 ± 14	0.22 ± 0.10	0.27 ± 0.12	42 ± 26	0.14 ± 0.07	0.17 ± 0.13

Values are means ± SD.

^*P < 0.01 (Mann–Whitney U test), comparing rectified with not rectified. NA, not applicable.

Because lowering the bias firing rate of a neuron would increase the likelihood that responses would rectify and raising the bias firing rate would decrease the likelihood of rectification, we examined whether the bias firing rate changed as a function of either frequency of rotation (while holding peak velocity constant at 60°/s) or peak velocity of rotation (while holding frequency constant at 0.5 Hz). The mean bias firing rate at 0.5 Hz, 60°/s peak velocity was 43 ± 28 spikes/s for type I neurons and 31 ± 25 spikes/s for type II neurons (P = 0.003). There was no significant interaction between the bias firing rate and frequency with the peak velocity held at 60°/s [type I: ANOVA, F_(7,764) = 0.361, P = 0.925; type II: ANOVA, F_(7,299) = 0.800, P = 0.588] or between bias firing rate and peak velocity for rotations at 0.5 Hz [type I: ANOVA, F_(8,633) = 1.217, P = 0.286; type II: ANOVA, F_(8,216) = 0.669, P = 0.719].

The relationship between bias firing rate, rectification, and sensitivity are explored in Tables 1 and 2. As expected, rectified units have lower rates, on average, than those of nonrectified units; the lower the firing rate of the cell, the lower the amount of modulation required for silencing during the inhibitory portion. Also, rectified units have higher sensitivity, on average, because for two units with the same bias firing rate, the one with the higher sensitivity is more likely to silence during a portion of its cycle. However, in considering both rectified and nonrectified units there was not a consistent relationship between bias and sensitivity (for example, at 0.5 Hz and 60°/s for type I neurons R² = 0.07; for type II neurons R² = 0.00005).

Response saturation

To further understand the output of NEM neurons as a function of stimulus amplitude, we explored the relationship between the sensitivity of responses to peak rotation velocity. The sensitivity of each response was examined in two ways: by looking at the output calculated on the whole-cycle fit, which takes into account both the inhibitory and the excitatory portions of the sinusoidal response, and on the half-cycle fit for the excitatory direction, which ignores the inhibitory portion of the cycle. Because many of the neurons were silenced in the inhibitory direction, the inhibitory half-cycle fit was based on fewer points than the excitatory or whole-cycle fit. For this reason, to consider the impact our style of analysis had on the results, and whether the relationship between the sensitivity of the response and peak velocity of the stimulus primarily influences either the excitatory or the inhibitory half cycle selectively, we directly compared the fit from the whole-cycle (sinusoidal fit) to that of the excitatory half-cycle. Tables 1 and 2 compare the response sensitivity calculated by the excitatory half-cycle to the whole-cycle sinusoidal fit. If the saturation of the response by velocity were limited only to either the on direction (excitatory) or the off direction (inhibitory) portion of the response cycle, then the relationship between the sinusoidal fit whole-cycle sensitivity and the regression fit half-cycle excitatory sensitivity would change as the peak velocity varied. No such relationship is seen. There is no statistically significant difference in the relationship between whole-cycle fits and half-cycle fits across different peak stimulus velocities. In total, the results of this study demonstrate that as the peak velocity of rotation increased, there was little change in the neuronal response rate. As a result, the sensitivity of the response, for both the whole-cycle and the excitatory portion of the cycle, decreased in proportion to the increasing velocity of rotation.

One difference between type I and type II neurons is seen in Tables 1 and 2. Rectification can result from either low bias firing or high sensitivity. For both type I and type II neurons, rectified cells have slower firing rates on average, as one expects. However, for type I neurons rectified neurons also show higher sensitivities. For the type II neurons, there was generally no statistically significant difference in the sensitivity, depending on whether the neuron is silenced for a portion of the cycle.

DISCUSSION

A number of studies have detailed the responses of central vestibular neurons to vestibular stimuli in a variety of preparations. Of interest are those that examined response characteristics to a range of vestibular stimuli. The majority of such studies have concentrated on frequency–response characteristics, including the development of transfer functions to describe the dynamics of the responses in terms of linear systems theory (Angelaki et al. 2001; Buettner et al. 1978; Dickman and Angelaki 2004; Fuchs and Kimm 1975; Jones and Milsum 1970, 1971; Ramachandran and Lisberger 2008; Shinoda and Yoshida 1974). In some seminal literature, investigators varied frequency while holding the amplitude of excursion constant, which varied peak velocity with frequency (Boyle and Pompiano 1981; Buettner et al. 1978; Chubb et al. 1984; Dickman and Angelaki 2004; Fuchs and Kimm 1975; Kasper et al. 1988; Shinoda and Yoshida 1974). Conclusions about the frequency response characteristics of neurons from experiments designed as such rely on the linearity of the system over the range of stimulus velocities that were applied; the findings in this study should influence the interpretation of those results.

A possibility to consider is that somehow the population of cells studied here differs from populations studied previously—although this possibility is unlikely. We used the same stereotaxic techniques as those used by other labs in identifying the vestibular nucleus based on the location of typical abducens nucleus discharge (Dickman and Angelaki 2004; Fuchs and Luschei 1970; Fuchs et al. 1988; Scudder and Fuchs 1992). Sixty-four percent of our NEM rotationally sensitive neurons showed convergence with translational stimuli, compared with 69% of the rotationally sensitive NEM cells recorded by Dickman and Angelaki (2002). Comparison of our data to those earlier studies is supported by the similarity in our findings to those data previously reported for which direct comparison is possible. The mean bias firing rate we found (43 ± 28 spikes/s for type I neurons and 31 ± 25 spikes/s for type II neurons) was similar to spontaneous firing rate values for central vestibular neurons in monkeys [46.9 for type I NEM neurons and 37.1 for type II NEM neurons (Fuchs and Kimm 1975); 48 spikes/s for both type I and type II, eye movement and NEM combined (Buettner et al. 1978); 38 spikes/s for both type I and type II, eye movement and NEM combined (Waespe and Henn 1977)]. Where comparable based on frequency and peak velocity of rotation, our sensitivity data are similar to those found in the study of NEM neurons by Dickman and Angelaki (2004, extrapolated from their Fig. 2: ∼0.8 spikes·s⁻¹/deg·s⁻¹ at 0.5 Hz, 31.5°/s peak velocity vs. our findings of 0.75 spikes·s⁻¹/deg·s⁻¹ at 0.5 Hz, 30°/s peak velocity for type I neurons and ∼0.6 spikes·s⁻¹/deg·s⁻¹ at 0.5 Hz, 31.5°/s peak velocity for type II neurons vs. 0.4 spikes·s⁻¹/deg·s⁻¹ at 0.5 Hz, 30°/s peak velocity in this study), but lower than found in Dickman and Angelaki (2002) (1.4 to 1.1 spikes·s⁻¹/deg·s⁻¹ at 0.5 Hz, 31.5°/s peak velocity for type I and II neurons). Direct comparison of the sensitivity and bias rate found in this study and most of the literature is complicated by the mixing of eye movement sensitive neurons, which have higher spontaneous firing rates and sensitivities than do NEM neurons (Fuchs and Kimm 1975).

A linear system is one that is scalable for the linear range, meaning that the sensitivity of the response is constant over that range of stimuli. A system for which doubling the input does not double the output is not linear. The validity of the assumption of linearity in the vestibular nuclei has been touched on in other studies. Jones and Milsum (1970) studied the response properties of neurons in the vestibular nucleus of decerebrate cats at frequencies between 0.25 and 1.7 Hz. They reported on the responses of five units as the amplitude of sinusoidal yaw rotation at a single frequency was varied between 10 and 100°/s. These authors fit the data with a power function with an exponent of 0.28 and concluded that the nonlinear effect was “apparently very small.” In anesthetized gerbils, Schneider and Anderson (1976) reported on the responses of vestibular afferents and central neurons to sinusoidal yaw rotation at frequencies ranging from 0.05 to 5 Hz. These authors also tested five central neurons for linearity of sensitivity by varying the peak velocity from 32 to 256°/s. They discovered that the two neurons with highest sensitivity at low rotational velocities had “an almost logarithmic decrease in sensitivity with increasing stimulus amplitude,” whereas three central neurons with lower sensitivity had a more linear relationship between amplitude of stimulus and magnitude of response. However, closer inspection of their data reveals that all of their units show decay in response sensitivity by ≥50% from the lowest velocities tested to the highest. They acknowledged that the nonlinearity in responses required that peak velocity be held constant when exploring sensitivity–frequency relationships. Shinoda and Yoshida (1974) examined the dynamics of neurons in the vestibular nuclei as part of a study to describe the transfer characteristics through the vestibuloocular reflex (VOR) pathway in decerebrate cats. They describe linear responses of 19 type I vestibular neurons at low frequencies (0.0083 to 0.5 Hz) and peak velocities (<40°/s), although they comment that the amplitude of rotation at higher frequencies was kept as small as possible to “minimize the nonlinearity of the gain.” By comparing steps to sinusoidal responses, these authors concluded that the vestibular nucleus neurons' behavior was “in agreement with the assumption of a linear system.” Waespe and Henn (1979) considered the response of vestibular neurons with and without eye movement sensitivity using velocity step stimuli in alert macaques. These authors found, similar to our study, that during vestibular stimulation in the dark, type I neurons were more sensitive than type II neurons. In the light, the neurons they recorded had linear responses for rotation velocities ≤120 to 140°/s. They did not directly report the linearity of neurons in the dark; it appears from their figures that they found that the response was linear only for velocities <60°/s, which were dependent on the acceleration of the stimulus. More recently, Heskin-Sweezie et al. (2007) examined linearity of central vestibular responses in alert cats after unilateral canal plugging or labyrinthectomy. Similar to our results in monkeys with intact labyrinths, these authors found neurons with nonlinear input–output relationships with peak velocity over the range of 10 to 120°/s for sinusoidal yaw oscillations at 1.0 Hz.

Other studies have addressed linearity in the vestibular nuclei by looking at neurons with sensitivity to linear motion. Musallam and Tomlinson (2002) found directional asymmetry in the response of vestibular-only (NEM) neurons to linear acceleration transients. In response to unidirectional transient linear accelerations along the nasooccipital and interaural orientations, these authors found that in one direction the cells responded relative to linear velocity, whereas in the other direction the neurons were acceleration sensitive. Schor (1974) found that the sensitivity of lateral vestibular nucleus neurons at 0.2- and 0.3-Hz roll tilts were the same at 5 and 10° tilt amplitudes, suggesting that tilt sensitivity in these neurons is roughly independent of stimulus amplitude. Schor et al. (1985) studied the frequency dependence of tilt-sensitive neurons in decerebrate cats. They performed no “explicit tests for linearity” but the sum of responses to single sinusoids and the response to a sum of sinusoids overlapped, suggesting that the additivity principle was obeyed. Musallam and Tomlinson (2001) reported, in abstract form, that vestibular only (NEM) cells in the vestibular nuclei of rhesus monkeys responding to linear and rotational stimuli violate both the principle of homogeneity (scalability, as shown in the current study) and superposition.

Nonlinearity in the form of rectification (inhibitory silencing) has been documented in nearly all studies examining dynamic responses of central vestibular neurons. Increasing asymmetry in vestibular neurons both with and without eye movement sensitivity is seen with increasing frequency of stimulation in alert cats (Broussard et al. 2004), as we found for type I but not type II neurons over a lower range of frequencies (0.1–2 Hz opposed to 1–8 Hz in the Broussard et al. study). During portions of the cycle where neurons are silent, information is not directly available about the magnitude of the inhibitory stimulus. However, silencing of one neuron may not degrade the linearity of the output of the system, either due to redundancy of information from the contralateral vestibular nucleus (Abend 1978) or population coding of asynchronously firing, intrinsically active neurons (Hospedales et al. 2008).

In the current study, we found that all neurons had reduced sensitivity with increasing peak velocity. The advantages of the current study in contrast to previous studies include the use of an awake primate, classification of the neurons based on eye movement and linear sensitivity, and the number of units studied. Limiting the study to neurons without eye movement sensitivity avoided confusion as to whether the response being studied was sensory or motor. Perhaps the surprising finding in this investigation was that even with seemingly gentle rotations, response sensitivities were not constant as peak velocities increased. This declining sensitivity with increasing velocity at even very modest peak velocities has not been observed in vestibular nerve fibers. Fernandez and Goldberg (1971) found essentially no saturation of sensitivity with intensity for sinusoidal rotation (a 10% sensitivity decrease for canal afferents with a 16-fold increase in peak acceleration). Sadeghi et al. (2007) noted linear responses in primary afferents in rhesus monkeys with increasing peak velocity from 20 to 80°/s. Regular units saturated at 200–300°/s and silence at about 200°/s with a linear response range of ±80°/s for steps. Irregular units saturated at 200°/s and silenced at about 100°/s with a linear response range of ±120°/s for steps. Regular-firing otolith afferents also demonstrate linear behavior over the range of 0.1–0.4 g at 0.1 Hz (Fernandez and Goldberg 1976b). Although primary afferents demonstrate scalability that is not seen in the second-order vestibular neurons in this study, other examples of nonlinearity are present in primary afferents. In squirrel monkeys, Goldberg and Fernandez (Fernandez and Goldberg 1971; Goldberg and Fernandez 1971a), using both steps of acceleration and sinusoidal stimuli, found asymmetry in the on and off directions for canal primary afferents. In contrast, Hullar and Minor (1999) did not find such asymmetry for regular afferents in the chinchilla. Goldberg and Fernandez (1971a) also demonstrated pronounced adaptation of primary semicircular canal afferent responses (usually more marked during inhibitory than during excitatory responses). Fernandez and Goldberg (1976a) noted similar nonlinearities (adaptation and excitatory responses being larger than inhibitory responses for irregular units) in otolith afferent neurons as well.

The functional significance of the nonlinearity of responses noted in this study is not clear. Some vestibular reflexes (like the VOR, which is an open-loop reflex) are linear over a wide range of velocities (Sadeghi et al. 2007), so such compression of the dynamic range of neurons involved in the VOR might be surprising. One explanation for the apparent mismatch between the relative linearity in afferent responses compared with NEM response could be related to the duality of the vestibular nucleus for sensory functions (such as in perception of motion) and direct involvement in short-latency reflexes (such as the VOR). Relative fidelity of the afferent signal entering the vestibular nucleus might be critical to preserving linearity in vestibular reflexes. For more purely sensory functions, the linearity of the input-to-output transformation in the nuclei might not be as pertinent. Alternatively, linearity in the neurons themselves might have less influence on linearity of output than previously thought (Hospedales et al. 2008).

Neuronal cell types in the vestibular nuclei other than NEM cells, specifically position-vestibular-pause and eye-head velocity cells are directly involved in generating the VOR (Scudder and Fuchs 1992). However, neurons with eye movement sensitivity were not studied in this report. The degree to which the neurons studied in these experiments might be involved in VOR processing is not known. NEM neurons likely contribute to a wide range of vestibular outputs such as vestibulospinal (Boyle 1993), vestibuloautonomic (Miller et al. 2008), vestibulocerebellar (Cheron et al. 1996; Zhang et al. 1993), and vestibulothalamic–cortical pathways (Meng et al. 2007), where linearity has not been studied and may not be as important a characteristic as it appears to be in the VOR (Ezure and Sasaki 1978).

In slice preparations, various authors have investigated cellular preparations in a variety of animal models (for review see Straka et al. 2005). One component of central vestibular neurons suspected of contributing to their nonlinear of behavior includes the expression of different voltage-dependent K⁺ conductances (Ris et al. 2001), which make one type of neuron found in rodent slice preparations (type B) display nonlinear current–discharge relationships. However, other data, also in slice preparations, demonstrate remarkably linear input–output relationships for central vestibular neurons to current (du Lac and Lisberger 1995) and synaptic (Bagnall et al. 2008) inputs. Potentially, local circuits may reconcile differences between findings in various preparations and may account for discrepancies in findings regarding linearity of responses in individual neurons (Pfanzelt et al. 2008).

Because the superposition principle does not apply in NEM neurons, the role of these neurons as transfer stations of linear responses from the linear transduction apparatus in the ear to (sometimes) linear outputs from the vestibular nucleus must be reconsidered. One possibility is that NEM neurons are concerned with detecting motion more than accurately relaying the magnitude of that motion. Perception of angular velocity in humans occurs at levels <4°/s (Becker et al. 2000; Kolev et al. 1996). In contrast, primary afferent detection thresholds are around 3.5°/s for regular afferents and 9°/s for irregular afferents (for responses averaged over 10 cycles at 0.5 Hz; Sadeghi et al. 2007). Goldberg and Fernandez (1971a) failed to detect a threshold for primary afferents stimulating at accelerations as low as 5°/s². Although we did not measure detection threshold, we were able to clearly demonstrate reproducible sensitivity and phase measurements over nearly 10 cycles of stimulation for most of the neurons we tested at peak velocities of 7.5°/s. Our data are consistent with those of Waespe and Henn (1979) who reported that 79% of vestibular neurons they tested had rotational thresholds <1.25°/s² to velocity step stimuli. Thus very high sensitivity with low-stimulus amplitudes allow for detection of low-amplitude signals over the inherent noise from the spontaneous activity of these cells. Although we did not calculate the regularity of firing rate of these cells, others have noted that second-order vestibular neurons fire with a range of regularity, but on the whole demonstrate more irregularity than afferents because there is no population of central neurons as regularly firing as regular afferents (Chen-Huang et al. 1997; Iwamoto et al. 1990; Schneider and Anderson 1976).

Because NEM neurons do not appear to encode amplitude of modulation as the amplitude of the stimulus increases, one possibility is that extending the dynamic range of the neurons is a primary concern for the system, avoiding high levels of inhibitory cutoff. Dynamic modulation of neuronal responses to time-varying stimulus amplitudes has been described in other sensory systems (Brenner et al. 2000). Such “adaptive rescaling” is suggested as part of a mechanism to extract features of the sensory signal against the background of noise and signal variance in a biologically efficient fashion (Brenner et al. 2000). This study does not allow determination of what features of vestibular signals are being optimally extracted by NEM cells, but suggests that treatment of vestibular nuclear cells as linear conduits between the periphery and vestibular reflexes responsible primarily for adjustment of response phase is an oversimplification of what are likely more complex processes. The degree of linearity of the system likely results not just from linearity in the individual components but also from the emergent properties of the complex neuronal network.

GRANTS

This work was supported by National Institute on Deafness and Other Communication Disorders Grant R01 DC-006429.