ON THE BIOMECHANICS OF HEART VALVE FUNCTION

Abstract

Heart valves (HVs) are fluidic control components of the heart that ensure unidirectional blood flow during the cardiac cycle. However, this description does not adequately describe the biomechanical ramifications of their function in that their mechanics are multi-modal. Moreover, they must replicate their cyclic function over an entire lifetime, with an estimated total functional demand of least 3×10⁹ cycles. The focus of the present review is on the functional biomechanics of heart valves. Thus, the focus of the present review is on functional biomechanics, referring primarily to biosolid as well as several key biofluid mechanical aspects underlying heart valve physiological function. Specifically, we refer to the mechanical behaviors of the extra-cellular matrix structural proteins, underlying cellular function, and their integrated relation to the major aspects of valvular hemodynamic function. While we focus on the work from the author’s laboratories, relevant works of other investigators have been included whenever appropriate. We conclude with a summary of important future trends.

I – Heart Valve Physiologic Function

1.1 – Introduction

Heart valves (HVs) are fluidic control components of the heart that ensure unidirectional blood flow during the cardiac cycle. Essentially, HVs are passive tissues that are directed to open and close due to inertial forces exerted by the surrounding blood. However, this description does not adequately describe the biomechanical ramifications of their function in that their mechanics are multi-modal and their loading cycle is repeated every second. For example, they must replicate this feat with each heart beat; over a period of 70 years the reader’s HVs will open and close nearly 3×10⁹ times.

Anatomically, there are two types of valves: semilunar and atrio-ventricular (sometimes also known as “sigmodial”). The semilunar valves (pulmonary (PV) and aortic (AV)) prevent retrograde flow back into the ventricles during diastole. Because of their anatomical location, they form nearly a perfect circle and have three, similarly sized leaflets (Schoen, 2005; Thubrikar, 1990) and are called semilunar due to their half-moon shape. The leaflets are attached to the vessel wall at the so-called basal attachment, with their free edges coapting to prohibit flow when closed. Neighboring leaflets meet at the highest point of their basal attachment to form a commissure. The major difference between the AV and PV, besides the dimensions of the leaflets, is that the AV has coronary artery ostioles behind two of the leaflets in the aortic wall. This feature gives rise to the nomenclature of the three AV leaflets: non coronary, right and left coronary. These openings are the source for feeding the myocardium of the heart with freshly oxygenated blood.

The atrioventricular valves, the tricuspid (TV) and mitral (MV) valves, prohibit reverse flow from the ventricle to the atrium during systole. They are more structurally complex as they are not formed of equal sized leaflets and do not have symmetrical geometries. Additionally, the leaflets of the valves are tethered to the inner walls of the ventricles by chord-like structures called chordae tendineae. These serve to prevent the valves from collapsing into the atrium during systole. There are two leaflets of the MV, the anterior, which forms a continuous connection with the non coronary leaflet of the AV, and the posterior. The TV is made up of three leaflets, all of which are of different shapes and sizes. The leaflets themselves (of all the HVs) are tapered as one moves away from the basal attachment; the porcine AV basal attachment is nearly twice as thick as the free edge (Weind et al., 2002).

The gross biomechanics of HV leaflets can be demarcated into three physical loading modes: tension, shear, and flexure. These three are imposed cyclically as the valve opens (flexure), permits blood to pass (shear), closes (flexure), and prevents the reverse flow of blood (tension). Because of the different geometries between the valve types and tethering chordae tendineae of the TV and MV, these three loading types are varied between the valves. Most of the research concerned with HV mechanics has been conducted on the AV (Adamczyk and Vesely, 2002; Billiar and Sacks, 2000b, 2000a; Christie and Barratt-Boyes, 1995; Merryman et al., 2006; Sacks et al., 1998; Vesely, 1998; Vesely and Mako, 1998; Vesely and Noseworthy, 1992) and MV (Gorman et al., 1996; Gorman et al., 2004; Kim et al., 2008; Sacks et al., 2002). The driving force behind this is likely due to the fact that these AV and MV are the most problematic valves and require surgical intervention or replacement more regularly. Additionally, many studies have been conducted with the valve in vivo under the full regime of loading required during normal (or pathological) function (Thubrikar et al., 1977; Thubrikar et al., 1979; Thubrikar et al., 1977; Thubrikar et al., 1980; Thubrikar et al., 1980; Thubrikar et al., 1979, 1981; Thubrikar et al., 1986). Therefore, a complete understanding of the three individual loading modes on each valve at the present time is quite unclear.

Shear stress and flexure are difficult to measure with the valve in situ, and accurate values for what the valves actually experience are not currently known; however, estimates for shear stress on the AV of 10–80 dynes/cm² (Weston et al., 1999) and flexure in native and bioprosthetic valves (Mirnajafi et al., 2005; Mirnajafi et al., 2006) have been made recently. It is reasonable to suspect that the shear stress and flexure experienced by each valve are comparable. The normal physiological transvalvular pressures (TVP) on the valves of a heart at rest are as follows: TV, 25 mmHg; PV, 10 mmHg; MV, 120 mmHg; and AV, 80 mmHg (Guyton, 1976). These values reveal that the left side heart valves experience 5 to 8 fold higher TVPs, which are not equilibrated by the tissue thickness (left side HVs are about 2x thicker) via a simple Law of Laplace calculation.

1.2 - Heart valve disease

According to the American Heart Association (Thom et al., 2006), valvular heart disease resulted in 19,989 deaths directly and was a contributing or underlying cause of death in 42,590 cases in 2003. Of the mortality or contributing mortality numbers, AV disease was the most prevalent with 12,471 and 26,336 cases, respectively; MV disease cases were responsible for 2,759 deaths and 6,600 contributory deaths. Right side valve cases were significantly less (PV: 11 deaths and 35 contributory deaths; TV: 16 deaths and 69 contributory deaths). It is estimated that in 2003 there were 95,000 inpatient valve procedures performed. These numbers indicate that valvular disease is very prevalent and life threatening and additionally that the AV is the most diseased with the highest rate of morbidity.

There exist two types of valvular heart disease: congenital and acquired (Otto, 2004). Congenital valve disease is an abnormality that develops before birth and this subset of patients are who would most likely benefit most from a tissue engineered heart valve. The result of the defect may be related to improper valve size, malformed leaflets, or an irregularity in the way the leaflets are attached. This most often affects the AV or PV. A very common congenital defect is bicuspid AV disease where instead of the normal three leaflets or cusps, the bicuspid AV has only two. Acquired valve disease pertains to problems that develop within valves that were at one time normal. This is typically referred to as age-related degenerative valve disease. In the early part of the 20^th century, the primary cause of acquired valve disease stemmed from rheumatic valve disease; however, this trend has changed dramatically with a decrease in rheumatic valve disease and a concomitant increase in age-related degenerative valve disease (Otto, 2004).

1.3 – Focus of this review

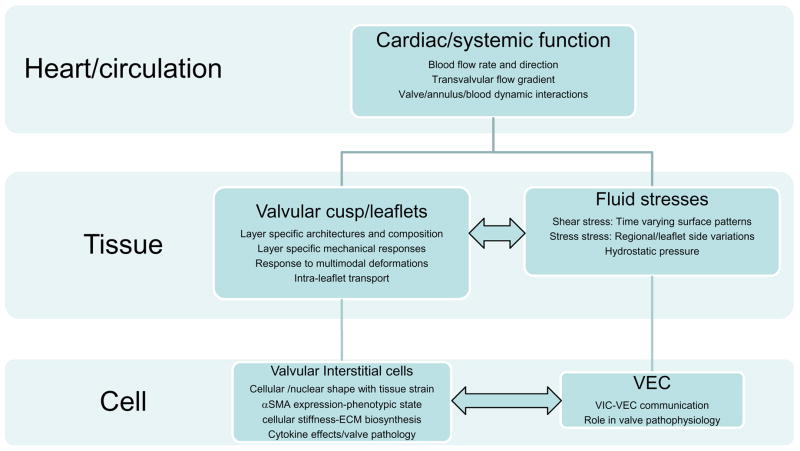
As in many physiological systems, one can approach heart valve biomechanics from a length scale approach, since mechanical stimuli occur and have physiological impact at the system, organ, tissue, and cellular scales (Fig. 1). Yet, despite its clinical importance, the unique and demanding valvular biological/biomechanical environment is relatively unexplored, with most research focusing on valvular prosthetic design. Thus, the focus of the present review is on functional biomechanics, referring primarily to biosolid as well as several key biofluid mechanical aspects underlying heart valve physiological function. Specifically, we refer to the mechanical behaviors of the extra-cellular matrix structural proteins, underlying cellular function, and their integrated relation to the major aspects of valvular hemodynamic function. While not an exhaustive review, the works of most investigators known to the authors have been included. We conclude with a further aim to underscore important future trends. Finally, while the literature is extensive, there are still many gaps. Therefore, in this review we note that experimental data and theoretical models may only exist for certain valves (or even specific leaflets).

Figure 1.

A schematic approach to the biomechanics of heart valve function. As in other physiological systems, valve function can be divided into multiple length scales. While the major drivers are organ-scale hemodynamic phenomena, key to this review are underlying tissue and cellular components that facilitate and regulate these remarkable functional processes of valve function. Moreover, while this system is hierarchical, there are interactions (horizontal arrows) that have not been well described and are likely paramount in valve physiology and function.

2 – Heart valve hemodynamics and fluid-structure interactions

2.1 - Heart valve hemodynamics

The mechanisms ensuring the proper function of the heart valves are essentially controlled by the surrounding hemodynamic environment. Understanding the interactions between the heart valves and the local hemodynamic environment is thus critical to better understand normal valve function and disease progression. Although the four heart valves present profoundly different anatomies and functional (e.g. opening/closing) characteristics, they all essentially function to facilitate the unidirectional flow of blood while maximizing flow rate and minimizing flow resistance. Thus, in the following we focus on AV hemodynamics as an example for all the heart valves. We also note that the effects of valve size or effective orifice area will affect the specific flow behaviors. However, for the purposes of the present review we focus on general behaviors common to valve function.

The AV opens during systole when the ventricle is contracting and then closes during diastole as the ventricle relaxes. In healthy individuals, blood flows through the aortic valve accelerating to a peak value of 1.35 ± 0.35 m/s (Otto, 2001). The valve closes near the end of the deceleration phase of systole with very little reverse flow through the valve. The adverse axial pressure differences causes the low inertial flow in the developing boundary layer along the aortic wall to decelerate then to reverse direction, resulting in vortices in the sinuses behind the aortic valve leaflets (Reul and Talukder, 1989). This action is thought to facilitate movement of the AV cusps (leaflets) away from the sinus wall and toward the closed position. When this force is coupled with the vortices that push the leaflet surfaces toward the closed position, a very efficient and fast closure is obtained. In vitro studies have shown that the axial pressure difference alone is sufficient to close the valve (Reul and Talukder, 1989).

Thus, without the vortices in the sinuses the valve still closes, but its closure is not as efficient as when the vortices are present. However, we note that the AV should functionally be considered part of the entire left ventricular outflow track. Thus, while the presence of the aortic sinuses helps to induce local hemodynamic patterns that facilitate AV function, they may have other functions. For example, during the cardiac cycle the AV annulus expands and contracts, which clearly alters how the AV leaflets function, possible facilitating valve opening. Further, the tissue structures at the leaflet/sinus interface reveal a gradual transition from the collagen rich leaflet tissue to the elastin rich sinus wall (Thubrikar, 1990). Thus, the sinus geometry may also help reduce the effects of flexural stresses at the leaflet/sinus interface. We should always thus keep in mind that various valvular components have multiple functions and interact in a complex but ultimately functionally efficient manner (see next section).

2.2 - Dynamic leaflet deformations

Clearly, a major part of understanding valve dynamics requires knowledge of the dynamic deformations that occur during valve function. Along these lines, investigators have undertaken the task of studying MV dynamics and left ventricular fluid mechanics (Bellhouse and Bellhouse, 1969; Hartiala et al., 1993; Ming and Zhen, 1986; Ormiston et al., 1981; Otsuji et al., 1997; Reul and Talukder, 1989; Schwammenthal et al., 1994). However, due to the complexity of valve anatomy, it is difficult to theoretically determine the functional role and importance of each individual component (Arts et al., 1983). Computational models, such as those described in the next section represent an exciting approach, but have not yet progressed to the point where fully dynamic function can be simulated. Moreover, simulations are currently difficult to fully validate in vivo with available imaging technologies. Several approaches have been taken to attempt to quantify valvular dynamics, including in-vivo (see (Sacks and Yoganathan, 2007) for details) and in-vitro approaches using in-vitro simulated flow conditions (He et al., 2005; He et al., 2003; Sacks et al., 2006; Sacks et al., 2002).

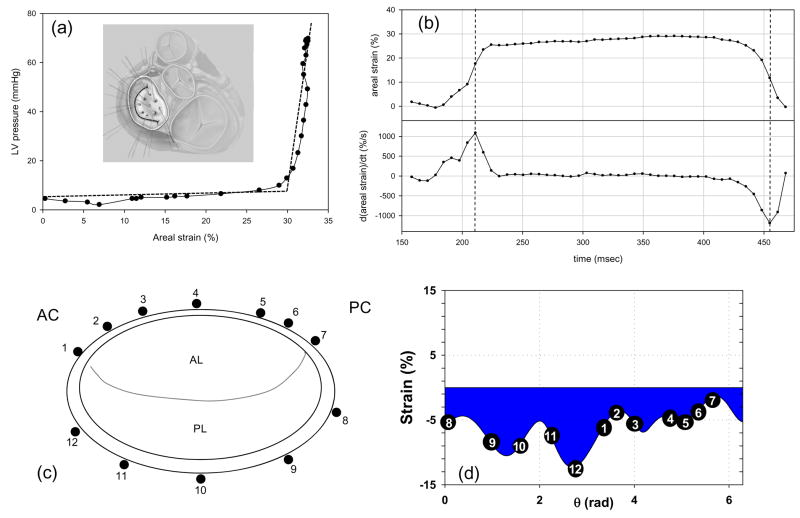
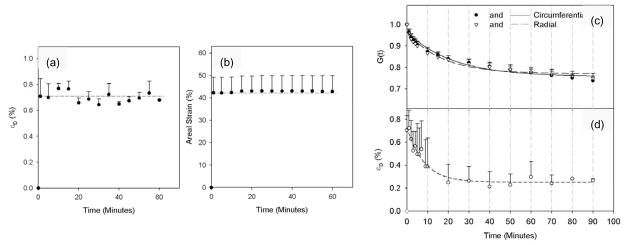
The highly dynamic motions, large anisotropic deformations, complex surface geometries, and thin leaflets clearly puts heart valve imaging beyond the forefront of what is possible with current imaging technologies. Recently, use of sonomicrometry technology has allowed greater insight into the actual in-vivo deformation patterns of the major valve structures (Eckert et al., submitted; Sacks et al., 2006). Specifically, the anterior leaflet of nine Dorsett sheep (35–45 kg) was instrumented with nine 1 mm hemispherical piezoelectric transducers in a 15 mm square array (Fig. 2-a). 3D crystal spatial positions were recorded at 250 Hz over several cardiac cycles, with peak LV pressures varied from 90 mmHg to 200 mmHg. The in-surface Eulerian strain tensor was computed from the crystal displacements. Since the central region demonstrated generally homogeneous strains, and thus we elected to focus the present study on the results from this region. To quantify the net change in leaflet dimensions the areal strain was defined as the change in the area defined by the sonocrystals expressed as percent reference area.

Figure 2.

(a) A representative in-vivo LV pressure-MV areal strain curve, which is analogous to a stress-strain curve for the leaflet. Here we observed a response, analogous to the classic non-linear soft tissue response, where the valve tissue undergoes large strains (up to 30% change in area) with minimal pressures, followed by a linear response highly stiff response corresponding to valve closure. Inset - A schematic of the MV anterior leaflet showing the 9 sonomicrometry transducer array. (b) Representative time-areal strain traces, along with the corresponding areal strain rate data. Strain rates were quite high, on the order of 1000%/sec. (c,d) Using similar techniques for the MV annulus, annular strains were shown to be regionally and temporally variant with stretches of −15% to 6%. This confirms the highly dynamic nature of the MV annulus and may be related to the varied fibrous-muscle structure of the annulus. Taken from (Sacks et al. 2006).

Perhaps the best way understand the key characteristics of valve deformations is to examine the simple relation between trans-valvular pressure and areal strain (Fig. 2-a). Here, one can easily observe very large changes in area (30%) under minimal trans-valvular pressure. Then, a sharp transition is observed accompanied by a highly stiff region corresponding to the coapted, fully pressurized state. Corresponding changes in areal strain with time demonstrated overall smooth deformations, with complete loading of the leaflet occurring in ~50 ms and peak strain rates of 1000%/sec (Fig. 2-b). We should note that valve tissue straining actually occurs over the entire cardiac cycle. Moreover, in a real sense a common reference state for the entire valve leaflet may not truly exist, given the high degree of spatial heterogeneity and constant changing dynamic fluid stresses. However, as a working definition for strain calculations we refer to point of initial contact between the leaflets, but before any significant coaptation can occur. For the aortic valve, we have defined this to be when a transvalvular pressure of ~4 mmHg exists (Sacks et al., 1998), whereas for the central point of the mitral valve anterior leaflet we have utilized the point of minimal LV pressure (Sacks et al., 2006).

In addition to leaflet deformation, the surrounding annulus can have a profound influence on valvular dynamics. We have recently investigated the in vivo annular kinematic data collected from surgically implanted sonocrystals in an ovine model and utilized quintic order hermite shape functions with C² continuity (Eckert et al., submitted) to determine the resulting strain and strain rate information along the entire annulus length (Fig. 2-c). Annular strain was shown to be regionally and temporally variant with minimum and maximum stretch values of near −15% and 6%, respectively, observed. Such information confirms the highly dynamic nature of the MV annulus and may be related to the varied fibrous-muscle structure of the annulus. Moreover, it can lay the basis for dynamic computational models of mitral valve function by allowing for prescription of the annular deformations as a time-varying boundary conditions.

Similarly, studies of normal and pathological aortic root dynamics are limited due to difficulties in performing in-vivo studies (Thubrikar et al., 1993). Dagum et al. (Dagum et al., 1999) have studied the deformation modes of the left, right, and noncoronary aortic root regions using radiopaque markers implanted at the top of the 3 commissures (sinotubular ridge) and at the annular base of the 3 sinuses. Not surprisingly, they determined that the ovine aortic root undergoes complex, asymmetric deformations during the various phases of the cardiac cycle, including elongation, compression, shear, and torsional deformation. Moreover, these deformations varied significantly among the left, right, and noncoronary regions.

Taken as a whole, these responses underscore common behaviors in all valvular tissues, and demonstrate the basic functional requirements at the tissue level:

1. Leaflets (or cusps) cycle between the fully unloaded to fully loaded state every cardiac cycle, unlike other connective tissues that remain preloaded (e.g. blood vessels).

2. Leaflets (or cusps) experienced large, anisotropic stretches during closure in response to respond to small trans-valvular pressure gradients.

3. Corresponding tension levels have been estimated to be in the range of 50 to 100 N/m during peak loading.

4. Peak strain rates are very high, reaching values of 1000%/sec.

5. Once the valve is closed, further leaflet deformation ceases.

6. Valve tissue deformations are highly sensitive to the deformations of the surrounding tissues.

Clearly, the demands on the constituent tissues are substantial to meet these physiological demands. The underlying tissue and cellular basis for these responses are discussed in Sections 3 and 4.

2.3 - Heart valve fluid-solid interactions

Finite element analysis has been the popular technique employed to determine the stress distribution on the leaflets of native and bioprosthetic valves with the blood pressure applied as the load on the leaflets (Kunzelman et al., 1993; Kunzelman et al., 1994; Kunzelman et al., 1998). Such analyses have also been employed to perform stress analysis with native aortic and mitral valves (Grande et al., 1998; Kunzelman et al., 1998). Numerical simulation of native heart valve function is quite challenging, with the necessity of addressing several difficult issues. It is necessary to incorporate the non-linear anisotropic mechanical properties of the leaflets and compute the complex motion of the leaflets due to the external load imparted by the surrounding fluid. In modeling the valve function, the fluid domain is most conveniently described using the Eulerian reference frame in which the fluid moves through a fixed mesh. A Lagrangian formulation is more appropriate for the leaflet motion in which the mesh moves together with the leaflet. The complete simulation of the heart valve function requires a fluid-structure interaction simulation and the two formulations are incompatible for such an analysis.

Two methods have generally been employed to circumvent this problem. De Hart (De Hart et al., 2003) utilized the Lagrange multiplier based fictitious domain method to describe the large leaflet motion within the computational fluid domain. This method is applied to a three-dimensional finite element model of a stented aortic valve. Results show that during systole the leaflets appear to be moving with the fluid in an essentially kinematical process governed by the fluid motion. The second approach is the arbitrary Lagrangian-Eulerian (ALE) method in which the computational mesh is allowed to deform (Lai et al., 2002) and move in space arbitrarily to conform to the moving boundaries and has also been successfully employed in the heart valve simulation. Recently, Vigmostad et al. (Vigmostad et al., 2008; Vigmostad et al., 2008) developed a partitioned, sharp interface approach (Marella et al., 2005) to overcome some of these limitations and employed physiologic Reynolds numbers, realistic material properties, and highly resolved grids. A partitioned approach was chosen, in which blood flow was computed using computational fluid dynamics, structural deformation was computed using an FE solver, and an FSI algorithm was developed to strongly couple these solutions. The standard governing equations for a viscous, incompressible fluid were employed to compute flow. These equations were discretized using a sharp interface, Cartesian grid, finite difference method (Marella et al., 2005). Boundaries and the embedded valve leaflets were represented using level sets, which move freely through the mesh without complex re-meshing. To decrease computation time, both local mesh refinement (LMR) and parallel algorithms were implemented. Using LMR, the Cartesian mesh was automatically refined or coarsened locally based on flow and boundary criteria to efficiently enable accurate results. With parallel processing, the solution was apportioned into multiple processors to enable high resolution without high computation times. Deformation and stresses in the valve leaflet were computed using a finite element model (FEM).

In 3D, an enhanced strain element was used to define the leaflet and membrane, bending, and shear stresses were computed as the leaflet deformed. The leaflet material model utilized a Fung-elastic model that incorporated the effects of in-plane shear and local material axes as defined by the local fiber preferred directions was employed to describe the valve leaflet in 3D, based on work by Sun et al. (Sun et al., 2005; Sun and Sacks, 2005). The FE model used a Lagrangian mesh which describes the leaflet geometry using an 8-node continuum element, and the stress and deformation of the leaflet were computed based on the leaflet properties and governing principles of solid mechanics. Inertial effects of the leaflet were incorporated, and through the no-slip condition were reflected in the flow solution. In order to couple the effects of the blood flow with those of the deforming valve leaflet, a fluid structure interaction method was developed. At every time step, a level set field was developed on the Cartesian grid to describe the current location of the valve leaflet. Based on the current conditions, the flow field was computed, incorporating the leaflet velocity at the previous time step as a boundary condition, so that no-slip was enforced. The pressure and stress in the fluid were computed at the leaflet interface and were used as boundary conditions on the leaflet FE mesh. The leaflet deformed accordingly, and this deformation was fed back to the fluid and the computation continues.

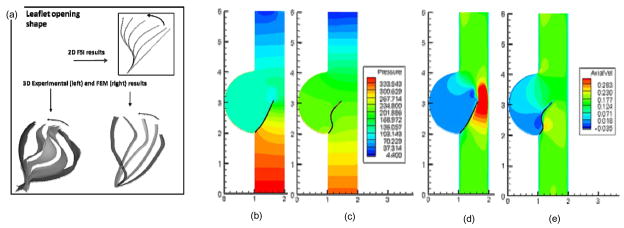
Results for the leaflet shape during valve opening for a 2D FSI simulation and 3D FEM computations (Kim et al., 2008) show good agreement with 3D experimental results (Iyengar et al., 2001), (Fig. 2). The leaflet profile at the fully open position shows good qualitative agreement between the various studies. These computations were performed using high resolution grids, to enable a more accurate solution. This was achieved with the use of LMR and a parallel algorithm, and stability and efficient convergence was ensured through the use of the partitioned, sub-iteration FSI approach, and underscores the importance of the use of physiologic material properties in modeling valve dynamics.

Two simulations were performed which employed the same boundary conditions, but the material properties of the leaflet were different. Valve 1 used the material properties used by de Hart et al. (De Hart et al., 2000), while Valve 2 used actual pericardial heterograft mechanical properties (Sun and Sacks, 2005). It was noted that the pressure contours (Fig. 3) a build-up of pressure for Valve 1, which was not observed in Valve 2. This was due to the high resistance to deformation offered by Valve 1, whereas Valve 2 utilizing a more realistic material model did not offer the same resistance to flow. Figure 3(C–D) shows the axial velocity in the two valves at the same time point. Note the large jet formed by Valve 1, due to the high stiffness of the valve. Valve 2 did not have such a jet, as it offered far less resistance to flow. Although the average displacement of the two leaflets was similar, a very different opening profile was visible. Additionally, the flow fields in the two cases were vastly different. This demonstrated how important physiologically realistic leaflet material properties were for the flow field computations and ultimately for understanding valve failure.

Figure 3.

(a) Stress computed during the opening phase of the cardiac cycle using experimentally determined leaflet material properties and physiologic pressure boundary conditions on the fluid. Leaflet motion computed using 2D FSI simulation (Vigmostad et al., 2008), 3D flow loop experiments (Iyengar et al., 2001), and 3D FEM computations (Kim et al., 2008). (b–e) Here, the same boundary conditions are employed for both simulations, but with different for material properties. Results showing a comparison of the pressure build-up (in Pa) with a high stiffness valve (b) and physiologic valve properties (c). In (d–e), axial velocity (in m/s) is shown for the two cases.

3 – Tissue level structure and mechanical behavior

3.1 – Tissue structure and composition

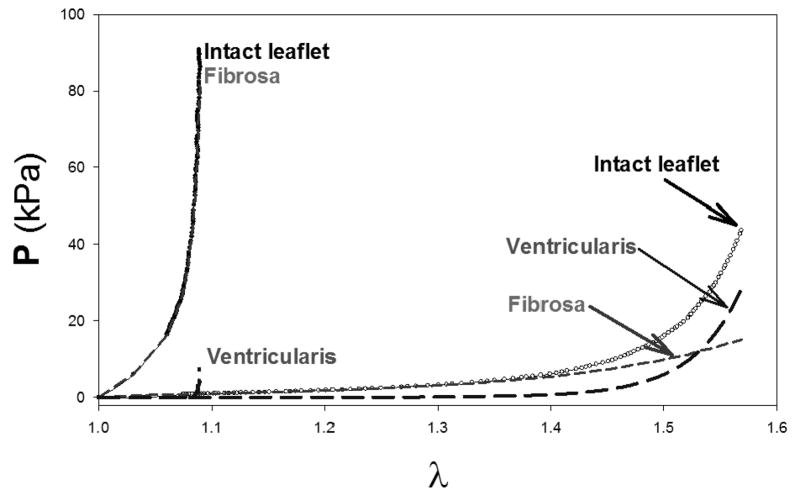
Given the physiological demands on the constituent tissue structures of the heart valve and the available biological materials (e.g. collagens, elastin, proteoglycans, etc), it would seem intuitive that a single, thin collagenous membrane would suffice to form the valve leaflets. Yet, nature has evolved a tri-layered leaflet structure (Schoen, 1997; Thubrikar, 1990). The two main connective tissue layers are the fibrosa and ventricularis (Figs. 4-a,b). The fibrosa is the thickest layer and consists primarily of a dense network of type I collagen fibers. Functionally, it appears to be the main load bearing layer and extends over the entire leaflet surface. As its name implies, the ventricularis (or atrialis for the MV and TV) layer faces the left ventricular chamber (or the left and right atria in the mitral and tricuspid valves, respectively). It is composed of a dense network of collagen and elastin fibers (Vesely, 1998) (Figs. 4-c,d). The elastin fibers within the ventricularis (or atrialis) appear to be radially aligned, as evidenced by their long, fibrous appearance in radial cross-sections (Fig. 4-d). Thus, its physiological function appears to assist in reducing large radial strains that occur during maximum forward flow when the valve is fully opened.

Figure 4.

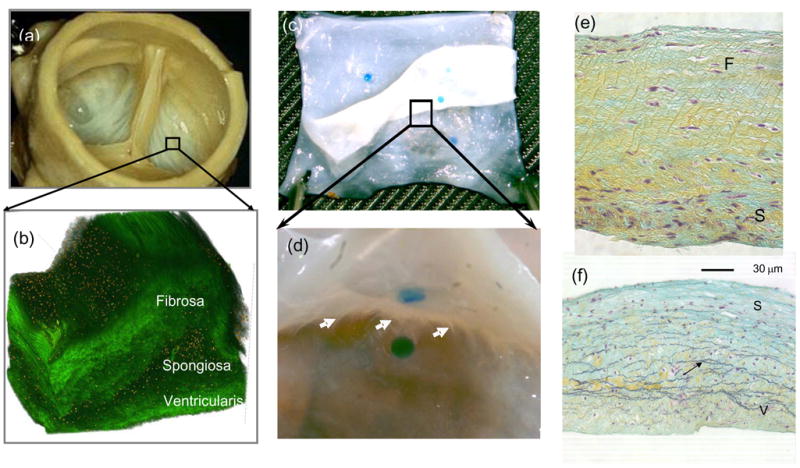
(a) The AV showing, where a 1 mm × 1 mm section was removed to illustrate in (b) the 3D the tri-layered leaflet structure (Courtesy Resolution Science Corporation, CA). (c,d) A magnified view of a partially separated AV leaflet showing the numerous connections found throughout the spongiosa. (e,f) Conventional circumferential histologic cross-sections from separated AV layers, showing that the remaining spongiosa displayed no visible damage induced during separation.

In contrast, the central spongiosa layer contains a high concentration of highly hydrated GAGs and PGs, which are believed to lubricate the fibrosa and ventricularis as they shear and deform relative to each other during leaflet bending and pressurization (Simionescu et al., 2003). Although they are most apparent in the spongiosa, GAGs and PGs are also found throughout the leaflet. It has been demonstrated by transmission electron microscopy that the majority of GAG are located in the interfibrillary spaces (Rothenburger et al., 2002; Simionescu et al., 2003), where they are likely present as components of large proteoglycans such as versican and versican-hyaluronan aggregates (Grande-Allen et al., 2004). GAGs are also visible in specific association with collagen fibrils (Rothenburger et al., 2002; Simionescu et al., 2003), in which they extend orthogonally from the fibrillar surfaces and bridge adjacent fibrils (Liao and Vesely, 2004; Liao et al., 2007). In addition to their mechanical functions, valvular GAGs and PGs are also speculated to have biological activity in regulating the sequestration of soluble mediators such as growth factors (Latif et al., 2005). The hydrated nature of most GAGs would enable the diffusion of nutrients and oxygen through the valvular tissue, which is largely avascular in adults (Duran and Gunning, 1968), to the resident interstitial cells (See section 5).

It is well known that collagen fibers can withstand high tensile forces, but have low torsional and flexural stiffness. Thus, directions in which the fibers are oriented can be identified with the directions in which the tissue is able to withstand the greatest tensile stresses. To quantify the gross fiber architecture of the valve leaflet, we used small angle light scattering (SALS) (Sacks et al., 1997). To simulate on the changes to aortic valve leaflet structure with increasing trans-valvular pressure, fresh porcine aortic valves were fixed at trans-valvular pressures ranging from 0 mmHg to 90 mmHg. Overall, increasing trans-valvular pressure induced the greatest changes in fiber alignment between 0 and 1 mmHg, and past 4 mmHg there was no detectable improvement in fiber alignment. Recently, we have quantified the amount of collagen fiber crimp in the native pulmonary and aortic heart valves (Joyce et al., 2009). Following Hilbert et al. (Hilbert, 1990; Hilbert et al., 1986), we represented the amount of collagen crimp as a percentage of the histological cross-sectional area containing observable crimp structures for each transvalvular pressure level. In this approach, cross-sectional regions displaying observable crimp were identified and the resultant areas measured, and expressed as a percentage of the total cross-sectional tissue image. It was found that at 0 mmHg ~60% of the AV transverse cross-sectional area was occupied by crimp structure. As transvalvular pressure increased, the percent crimp decreased rapidly until 20 mmHg, with minimal decreases in percent crimp thereafter. Thus, we can see that for the valvular structures much of the observed change in collagen structure is due to straightening of the collagen fibers. This is a finely tuned affair; straightening must occur at the right strain level and at the right rate to facilitate coaptation, yet not allow excessive tissue deformations that may lead to regurgitation (e.g. Fig. 2-a).

3.2 - Key biomechanical behaviors of valvular tissues

The results of the preceding section suggest a complex structure-mechanical behavior and interactions for heart valve leaflets. While there have been a few studies on MV tissue (May-Newman and Yin, 1995, 1998), most of the research concerned with HV mechanics has been conducted on the AV (Adamczyk and Vesely, 2002; Billiar and Sacks, 2000b, 2000a; Christie and Barratt-Boyes, 1995; Merryman et al., 2006; Sacks et al., 1998; Vesely, 1998; Vesely and Mako, 1998; Vesely and Noseworthy, 1992) and MV (Gorman et al., 1996; Gorman et al., 2004; Sacks et al., 2002; Stella et al., 2007). The driving force behind this is likely due to the fact that these AV and MV are the most problematic valves and require surgical intervention or replacement more regularly. Although common to all valves, the different valve geometries and effects of tethering by chordae tendineae in the TV and MV, the magnitudes and rates of these loading modes will vary between the valves. It is thus useful to divide the study to the tissue-level biomechanics of HV leaflets into in-plane stretch and flexural deformation modes. These occur cyclically as the valve opens (flexure), experiences surface shear stress from the local blood flow, followed by closure (flexure) then coaptation and full loading (tension). Therefore, behaviors of the individual loading modes are presented in the following. While we and others have made extensive examinations of other heart valve leaflets (also referred to as a “cusp,” but we consider “leaflet” a better term to describe its function), we focus here on the native aortic valve as an example of the intricacies of heart valve leaflet tissue biomechanics.

In-plane responses

The mechanics of soft tissues are well known to be complex: they exhibit a highly non-linear stress-strain relationship, undergo large deformations, complex viscoelasticity, and complex axial coupling behaviors that defy simple experiments and material models (Fung, 1993; Sacks, 2000). Much of this behavior is a direct result of changes in their internal structure with strain, which involves both straightening of highly crimped collagen fibers and rotation of these fibers toward the stretch axis. For valvular tissues, most previous work on the mechanical properties of the native and chemically treated aortic valve has relied on uniaxial mechanical testing (Lee et al., 1984), (Lee et al., 1984), (Vesely and Noseworthy, 1992). These studies demonstrate that chemical fixation of intact valves, especially under pressure, alters the mechanical properties of the leaflets. Marked decreases in the extensibility are generally attributed to “locking” the collagen fibers in the uncrimped state (Broom and Christie, 1982), (Christie, 1992). Tests on thin tissue strips however, cannot mimic the heterogeneous multi-axial deformation fields, combined loading sequences, and native fiber kinematics found in the physiological environment. Mayne et al. (1989) and Christie et al. (1995) have performed equi-biaxial testing (i.e. equal levels of tension applied to each test axis) that overcomes many of the above limitations of uniaxial loading. However, derivation of a constitutive relationship solely from equi-biaxial test data is limited due to multiple co-linearities that confound the ability to obtain reliable, unique model parameter values (Brossollet and Vito, 1995).

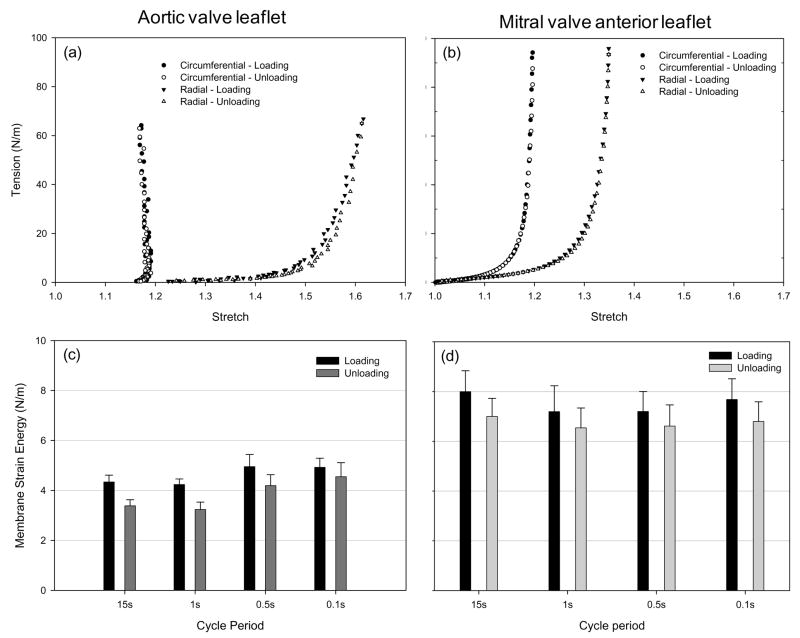
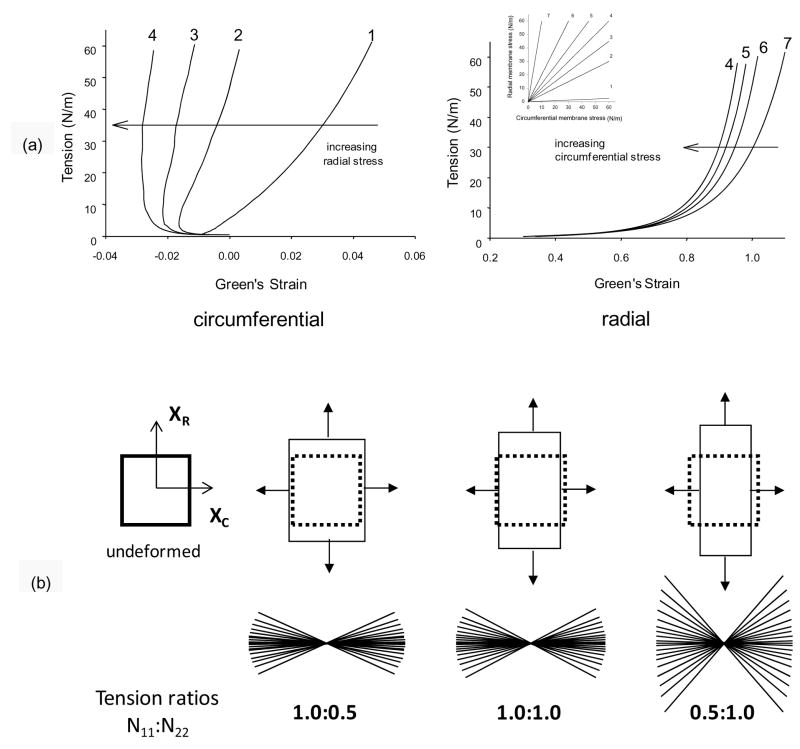
Billiar and Sacks (2000a) generated the first complete biaxial mechanical data necessary for constitutive modeling aortic valve leaflet (See Section 4). Due to the small size and heterogeneous structure of the aortic valve leaflet, testing methods were developed and validated. Leaflet specimens were subjected to biaxial tests utilizing multiple loading protocols to provide a range of loading states that encompass the physiological loading state. The leaflets demonstrated a complex, highly anisotropic mechanical behavior (Fig. 5-a), including pronounced mechanical coupling between the circumferential and radial directions. The MV anterior leaflet, produced similar albeit less anisotropic response (Fig. 5-b). Mechanical coupling between the axes produced negative strains along the circumferential direction and/or non-monotonic stress-strain behavior when subjected to equi-biaxial tension, a behavior noted by Mayne et al. (1989) but was not explained. Moreover, Adamczyk et al. (Adamczyk and Vesely, 2002) measured AV leaflet strains in-situ and found that negative strains can occur in the non-coronary leaflet.

Figure 5.

Representative loading and unloading curves for the (a) AV and the (b) MVAL depicting minimal energy loss. Mean (c) AV and (d) MVAL energy storage and dissipation for all stretch rates, with hysteresis defined as the area under tension-areal stretch curve. Both leaflets demonstrated very small hysteretic losses and neither leaflet demonstrated measureable change in hysteresis with strain rate.

Time dependent behaviors

The above studies focused on the elastic behavior of valvular tissues. There are substantial previous studies using uniaxial testing techniques which have investigated stress-relaxation in valvular tissues and modeled the response using quasi-linear viscoelastic theory (Carew et al., 2000; Carew et al., 1999). However, time dependent behaviors under physiological multi-axial loading states have only recently been quantified. We have conducted novel sub- to physiological strain rate studies under biaxial stretch on the AV and MVAL (Grashow et al., 2006a; Grashow et al., 2006b; Stella et al., 2007). The resulting stress–strain responses were found to be independent of strain rate for both valve leaflets, as was the observed low level of hysteresis (Fig. 5). Stress relaxation and creep results indicated that while the leaflet tissues exhibited significant stress relaxation, they exhibited negligible creep over the three hour test duration. To our knowledge, this response is unique in the biomechanical literature and stands in contrast, for example, to that reported for other dense collagenous tissues such as tendon and ligament (Provenzano et al., 2001; Thornton et al., 2001; Thornton et al., 1997).

However, the underlying micro-structural basis for this unique mechanical behavior is presently unknown. As collagen is the major structural component of the MVAL, we investigated the relation between collagen fibril kinematics (rotation and stretch) and tissue-level mechanical properties in the MVAL under biaxial loading using small angle x-ray scattering (SAXS). A novel device was developed and utilized to perform simultaneous measurements of tissue level forces and strain under a planar biaxial loading state. Collagen fibril D-period strain (ε_D) and the fibrillar angular distribution were measured under equibiaxial tension, creep, and stress relaxation to a peak tension of 90 N/m. Results indicated that, under equibiaxial tension, collagen fibril straining did not initiate until the end of the nonlinear region of the tissue-level stress-strain curve. At higher tissue tension levels, ε_D increased linearly with increasing tension. Changes in the angular distribution of the collagen fibrils mainly occurred in the tissue toe region. Using ε_D, the tangent modulus of collagen fibrils was estimated to be 95.5 ± 25.5 MPa, which was ~27 times higher than the tissue tensile tangent modulus of 3.58 ± 1.83 MPa. In creep tests performed at 90 N/m equibiaxial tension for 60 minutes, both tissue strain and ε_D remained constant with no observable changes over the test length (Fig. 6-a). In contrast, for stress relaxation performed for 90 minutes, ε_D was found to rapidly decrease in the first 10 minutes followed by a slower decay rate for the remainder of the test (Fig. 6-b).

Figure 6.

Using both small angle X-ray and tissue level strain measurements of the porcine MVAL, we observed that (a) collagen fibril D-period strain (ε_D) and (b) tissue areal strain vs. time during creep tests demonstrated no detectable change with time. In contrast, biaxial stress relaxation experiments (c,d) demonstrated continued relaxation over the similar time scales. Data presented as mean±SEM

It is interesting to note that we also evaluated the measured the strain during the relaxation tests. First, our device was able to resolve stretch values to 0.001 or 0.1% (Grashow, 2005). We found that during relaxation experiments the circumferential strain increased by 0.1% (i.e. within measurement error) and the radial component to 0.4%. If the relaxation was an artifact of excessive deformation resulting from the stress concentrations near the sutures, then the strain in the central region should decrease, which it did not. Thus, we have no evidence of decreasing strain due to stress concentrations or other experimental artifacts.

Overall, our results suggest that:

1. Since stress relaxation occurred at both the tissue and fibril levels, it may be a result of an internal slipping mechanism, possibly modulated by non-collagenous components (e.g. proteoglycans).

2. The lack of creep but the occurrence of stress relaxation suggests the internal slipping can only occur when the external strains are held constant, not under constant loads.

3. Overall, while valvular tissues exhibit intrinsic viscoelastic behavior these behaviors do not appear to be functional.

Taken as whole, these results support our observations that valvular tissues are functionally anisotropic, quasi-elastic biological materials. These mechanical qualities also appear to be unique to valvular tissues; indicating an ability to withstand loading without time-dependent effects under physiologic loading conditions.

Flexural response

Any biomechanical study of heart valve leaflets must be designed to elucidate the unique mechanical properties of the tri-layered aortic cuspal structure. This is especially important when determination of the layer mechanical properties of the compliant cuspal tissue in the low stress-strain region is considered. For example, while biaxial mechanical testing techniques successfully quantified the in-plane stress-strain response (Sacks, 2000), they cannot determine the contributions of individual layers. Further, although the cuspal displacements are large the tissue strains are actually small, so that the low strain region of stress-strain curve is of interest. Mechanical properties in the low strain region are difficult to obtain accurately from tensile studies, which also cannot measure compressive stiffness. Flexure represents not only a natural deformation mode of the valve leaflet, it also introduces a deformation mode in which the different layers (unlike uni- or biaxial tensile experiments), experience different strains. Further, the stress-strain response of the individual cuspal layers in the low strain region under both tension and compression can be obtained without the need for dissection or other interventional approaches. Flexure of soft biological materials offers two distinct advantages over tensile mechanical testing: 1) the ability to discern slight changes in stiffness at low-stress strain levels that would not be appreciable in tension and 2) the ability to assess the contributions and interactions of individual layers of multi-layered structures. Thus, multi-layered tissues like the aortic valve leaflet should reveal a distinct bending response depending on the direction of bending. Thus, flexural mechanical testing techniques are a sensitive way to explore the effects of layer contributions.

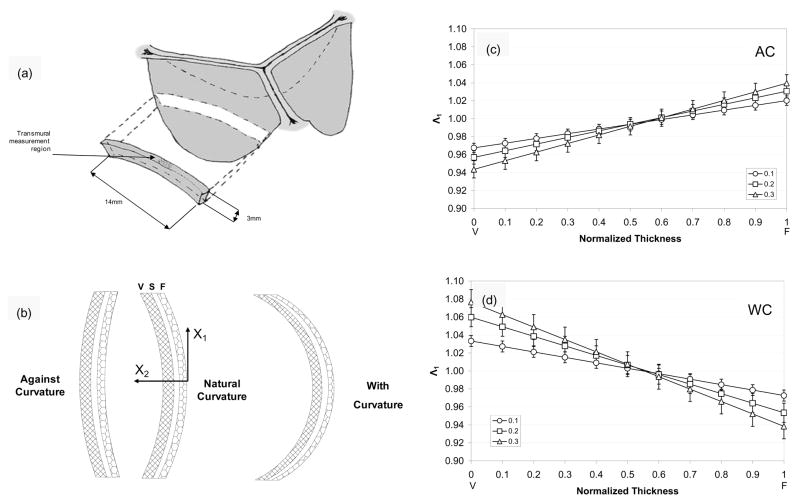
To quantify the flexural properties, we have utilized both 3-point (Engelmayr et al., 2003; Gloeckner et al., 1999; Merryman et al., 2006) and cantilever (Mirnajafi et al., 2006) loading configurations. For the aortic valve leaflet, the effective stiffness measured in the with-curvature (WC) direction is dominated by the tension in the ventricularis, with little contribution from the fibrillar collagen in the fibrosa which is not designed to support compressive loads. Conversely, when the leaflet is bent in the against-curvature (AC) direction, the fibrosa is in tension and the ventricular is in compression (Fig. 7-b). The resulting data is expressed as the bending moment (M) vs. Δκ, from which the effective instantaneous bending stiffness E can be determined using the Euler-Bernoulli equation M = EI Δκ (Frisch-Fay, 1962), where I is the second moment of inertia. Using this technique we have found a linear M-Δκ response in the belly regions (Merryman et al., 2006), in contrast to the highly non-linear in-plane tension response. Interestingly, for the commissure region E varied linearly with the deflection angle φ, indicating a consistent decrease in stiffness with increased flexure. Histological studies of the commissure region indicated that tissue buckling was a probable mechanism for decrease in E with increasing flexure. The observed change in E with flexural angle in the commissural region is a subtle aspect of valve function.

Figure 7.

Schematic showing (a) the orientation of the AV leaflet specimen used for flexural testing, including the location of the transmural measurement region, which was flexed in two directions to alternately subject the tissue layers to tension/compression (b)? The resulting transmural strain distribution of Λ₁ with increasing curvature along the normalized thickness (V=ventricularis and F=fibrosa) for the (c) AC and (b) WC directions. Here, the intersection of the composite plots with Λ₁ of 1 determined the location of the neutral axis, which was near the center and not dependent of flexural direction.

Recently, we have developed a novel, highly sensitive instrument that used an end-loaded configuration that allows for simultaneous measurement of the transmural strain distribution in the specimen center (Lam et al., submitted). Circumferential strips from porcine AV leaflets taken from the belly region (Fig. 7-a) were subjected to bidirectional flexure to the fibrosa and ventricularis layers to alternate states of in tension and compression (Fig. 7-b). The center of the specimen (approximate 2 mm in length) was marked with small ink particles which were tracked using a separate high-resolution imaging system. Transmural images were acquired at Δκ=0.1, 0.2, and 0.3 mm⁻¹ in the AC and WC directions, where Δκ=0.1, 0.2, and 0.3 mm⁻¹ was considered the peak change in curvature, based on in-vitro measurements (Sugimoto and Sacks, submitted). Details of the transmural strain analysis is given in (Lam et al., submitted). Briefly, from the tracked marker displacements the local deformation gradient tensor F determined which was decomposed into the stretch and rotation tensors, U and R, respectively to remove rigid body motion experienced by the tissue during flexure. The net stretch along each axis, Λ_i (i=1,2) was computed using ${\mathrm{\Lambda }}_1=\sqrt{{\text{U}}_{11}^2+{\text{U}}_{12}^2}$ and ${\mathrm{\Lambda }}_2=\sqrt{{\text{U}}_{22}^2+{\text{U}}_{12}^2}$. Thus, the location of the neutral axis, where Λ₁=1, was determined by plotting Λ₁ against the tissue thickness.

Interestingly, the Λ₁-transmural thickness relation quite linear for each flexural level in both bending directions, with Λ₁ ranging from 0.94 to 1.08 (Fig. 7-c). This suggests that the maximum tissue strains were on the order of +/−7%, which is consistent with previous work on bioprosthetic heart valves (Mirnajafi et al., 2005). Moreover, for both bending directions the neutral axis was located at 0.55–0.60 normalized tissue thickness, indicating that the fibrosa was slightly stiffer than the ventricularis in the low strain range. Of interest to layer micro-mechanics, we did not observe any slip or other evidence of shearing between layers in the transmural strain profiles. This is consistent with the highly interconnected layer microanatomy (Fig. 4). Continued work to provide insights into these mechanisms is clearly necessary to better understand native valve function.

4 – Constitutive models for valvular tissues

4.1 – General considerations

Advanced modeling and simulation of heart valves clearly requires sophisticated constitutive models, which are expected to account for regional variations (belly/commissure) and layer properties of valvular tissues. Moreover, they should be able to predict the stress environment of cells to address mechano-physiological questions (Huang et al., 2007). In order to frame the problem, we should consider the some important general characteristics of typical valvular mechanical responses. For example, the response to multiple planar biaxial loading protocols reveals a non-monotonic relationship between circumferential stress and strain (Fig. 8-a). Examination of the progression of the stress-strain curves with decreasing radial stress in non-equibiaxial loading protocols demonstrated that the negative circumferential strain values were due to a very strong mechanical coupling between the axes. This behavior, as well as the overall anisotropic behavior of the cusp, can be explained by the tight angular distribution of collagen fibers (Fig. 8). As one axis is loaded, the radial forces cause the fibers to rotate, which in turn cause a contraction along the circumferential axis. This effect will become more dramatic as the radial loads become larger with respect to the circumferential loads. This effect illustrates that negative strains can be generated even though the stress magnitude is the same along both axes and no buckling of the tissue is observed. Thus, radial loads are ultimately resisted by the highly circumferentially aligned fibrosa collagen fibers, whose rotation as opposed to stretch allows for large radial strains.

Figure 8.

(a) Representative stress-strain data in the circumferential and b) radial directions for a AV leaflet cusp demonstrating the effects of transverse loading (in-plane coupling). The underlying structural basis for this behavior is best shown by a schematic showing the fibrous structure of the cusp depicting the large collagen cords which undergo large rotations with loading (b). As the radial loads become larger with respect to the circumferential loads, the collagen fibers undergo large rotations causing contraction along the circumferential axis without buckling and allows for very large radial strains.

4.2 – Structural model

To capture this response, we utilize a meso-structural approach for constitutive modeling for planar collagenous tissues (Billiar and Sacks, 2000b; Lanir, 1983; Sacks, 2003). It is assumed that a representative volume element (RVE) can be identified that is large enough to represent the processes associated with the microstructure of the material in some average sense, yet small compared to the characteristic length scale of the microstructure, i.e. the tissue thickness. The RVE is treated as a three-dimensional continuum and it is assumed that the material can be modeled as a hyperelastic solid, so that

$$\mathbf{S}=\frac{\partial \text{W}}{\partial \mathbf{E}}$$ (1)

where S and E are the 2^nd Piola-Kirchhoff stress and Green-Lagrange strain tensors, respective, and W is the tissue strain energy density per unit volume. Within the RVE, the following assumptions are made:

1. As with other collagenous membrane tissues, valvular tissues can be idealized as a planar network of collagen fibers embedded in a compliant ground substance (i.e. the matrix).

2. The load required to straighten the collagen fiber is considered negligible compared to the load transmitted by the stretched fibers. Hence, collagen fibers transmit load only if stretched beyond the point where all the undulations have disappeared, and are assumed to be linearly elastic.

3. The fiber strain can be computed from the tensorial transformation of the global strain tensor referenced to fiber coordinates (i.e. the affine transformation assumptions).

4. The strain energy function of the tissue is the sum of the individual fiber strain energies. For the purposes of tissue-level simulations, we consider a homogenized model wherein we consider an ensemble of fibers, with varying degrees of undulations, with an orientation defined by the normal vector N̂ = cos(θ)î + sin(θ)ĵ in the unloaded reference state. The resulting effective stress response was represented using S′ = c₁[exp (c₂ E′) − 1] where c_i are material constants, S′ is the ensemble second Piola-Kirchhoff stress, and E′ is the ensemble Green’s strain determined using E′ = N̂^T EN̂. Note that for valvular tissues, it was initially more convenient to work with membrane stresses due to considerations such as variable total and layer thickness, and heterogeneous layer structure (Billiar and Sacks, 2000a). We also assume that inter-specimen variations in fiber volume fraction V_f and thickness h are negligible, so that the product hV_f can be conveniently absorbed into the material constant c₁. This model worked very well for both the native aortic and chemically modified valvular tissues (Billiar and Sacks, 2000b, 2000a).

Constitutive model that better reflects the physiologically-driven structural kinematics of collagen fibers under deformation is the next logical approach, including the use of. This includes use of fiber recruitment models (Sacks, 2003). However, fiber recruitment models are difficult to implement three dimensional soft tissues since the fiber recruitment behavior is difficult to directly obtain from conventional multi-protocol experimental protocols. There are also practical parameter optimization difficulties when utilize in multi-axial data since recruitment model parameters are non-unique and multiple combinations can fit data equally well, and thus obscure the underlying mechanisms. Thus we need appropriate theoretically-guided experiments to implement structural models for valvular tissues properly.

Our purpose was therefore to demonstrate that the constituent fibers of the left side valve leaflets can be simulated using a fiber recruitment model. As above, the strain energy measure in terms of total strain for a single fiber is given by

$${\text{W}}^{\text{f}}({\text{E}}^{\prime })=\frac{\text{K}}{2}{\left(\frac{{\text{E}}^{\prime }-{\text{E}}_{\text{s}}}{1+2{\text{E}}_{\text{s}}}\right)}^2.$$ (2)

Here, K represents the effective fiber modulus and E_s is the Green’s strain in which the fiber straightens. To link the individual fiber responses to that of the ensemble, we assume that the recruitment of collagen fibers, the ensemble is represented by D(E′) (Lanir, 1983; Sacks, 2003), where the function D represents the probability density distribution characterizing the fiber undulation. The fiber ensemble strain energy can thus be written as

$${\text{W}}^{\prime }({\text{E}}^{\prime })={\int }_0^{{\text{E}}^{\prime }}\text{D}(\text{x}){\text{W}}^{\text{f}}({\text{E}}^{\prime })\text{dx}.$$ (3)

Here, W′ represents the strain energy developed by all fibers contributing to the load transfer at a specific angle θ within the fiber network. The resulting ensemble stress can be determined using

$${\text{S}}^{\prime }=\frac{\partial {\text{W}}^{\prime }({\text{E}}^{\prime })}{\partial {\text{E}}^{\prime }}={\int }_0^{{\text{E}}^{\prime }}\text{D}(\text{x})\frac{\partial {\text{W}}^{\text{f}}({\text{E}}^{\prime })}{\partial {\text{E}}^{\prime }}\text{dx}.$$ (4)

Next, integrating to the tissue level, we have

$$\text{S}(\text{E})=\frac{\partial \text{W}(\text{E})}{\partial \text{E}}={\int }_{-\pi /2}^{\pi /2}\text{R}(\theta )\left\{\text{K}{\int }_0^{{\text{E}}^{\prime }}\text{D}(\text{x})\frac{{\text{E}}^{\prime }-{\text{E}}_{\text{s}}}{{(1+2{\text{E}}_{\text{s}})}^2}\text{dx}\right\}\text{N}\otimes \text{N}\theta ,$$ (5)

where ⊗ indicates external multiplication so that [N⊗N]_ij = N_iN_j and that R(θ) is the angular distribution of the collagen fibers. Based on our architectural data for the aortic valve leaflet (Sacks et al., 1998), we utilize the fact that R(θ) can be represented by $\text{R}(\theta )=exp\left[\frac{-{\theta }^2}{2{\sigma }^2}\right]/(\sigma \sqrt{2\pi })$, where σ is the standard deviation.

A difficulty in implementing eqn. 5 in the homogenized tissue constitutive model is the determination of the fiber recruitment function D. In particular, the tissue response should exhibit a distinct upper bound strain, E_ub, which represents the strain wherein all collagen fibers have been fully recruited. At the tissue level, full recruitment is characterized by a constant stiffness for E_ens ≥ E_ub. Thus, the D must be defined over a finite Green’s strain range E_ens ∈ [0, E_ub], and 0 otherwise, with ${\int }_0^{{\text{E}}_{\text{ub}}}\text{D}(\text{x})\text{dx}=1$. It can be shown that under equibiaxial strain conditions (E₁₁ = E₂₂, E₁₂ = S₁₂ = 0), the fiber ensemble stress-strain law can be obtained directly from the biaxial mechanical data using S′=S₁₁+S₂₂ (Sacks, 2003). From the S′-E′ response curve and eqn. 3, the effective fiber stiffness K and D can be determined. For D, we utilized the following modified beta function

$$\text{D}(\text{x})=\frac{{\text{x}}^{\gamma -1}{(1-\text{x})}^{\delta -1}\mathrm{\Gamma }(\gamma +\delta )}{\mathrm{\Gamma }(\gamma )\mathrm{\Gamma }(\delta )}\text{for}\text{x}\in [0,{\text{E}}_{\text{ub}}],0\text{otherwise},$$ (6)

where γ and δ are constants related to the distribution mean μ = γ/(γ + δ) and variance $\sigma =\sqrt{(\gamma \delta )/\left[{(\gamma +\delta )}^2(\gamma +\delta +1)\right]}$.

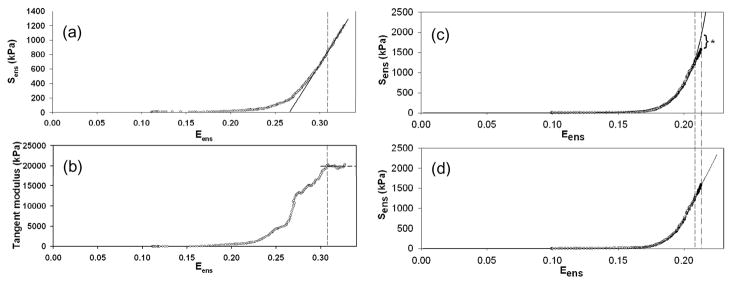
Experimentally, the E_ub is determined by finding the onset of constant stiffness from the S′-E′ response curve, best determined from strain value where the tangent modulus plateaus (Figs. 9-a,b). To determine the ensemble model parameters K, μ, and σ, eqn.4 was fit to the to S′-E′ data using Marquardt-Levenberg non-linear least squares algorithm (Press et al., 1988). Using the post-transition tangent modulus value (equivalent to the maximum tangent modulus) was used as an initial estimate to the collagen fiber elastic modulus K. When applied to the native MVAL, MVPL, and AV The recruitment model was found to fit the data quite well, with an r2 ≅ 0.99. AVL was found to have the Eub = 0.3013 ± 0.0323, in comparison with MVAL (0.2692 ± 0.0487) and MVPL (0.2473 ± 0.01181). Moreover, the model predicted the very similar average K values for each valve leaflet type (mean ± sem, n=6 each): MVAL 123.443 ± 3.324 MPa, MVPL = 124.114 ± 7.830 MPa, and AV=101.220 ± 6.030 MPa. It is interesting to note that these values are very similar to the 95.5 MPa measured using SAXS for the MVAL (Liao et al., 2007).

Figure 9.

(a) A representative example of the effective fiber stress-strain curve of heart valve leaflets (MVAL), showing the characteristic long toe region, transitional region, and subsequent linear region. (b) Corresponding tangent modulus of the fiber stress-strain curve. Note the sharp rise of tangent modulus in the middle of transitional region and the followed plateau that shows a constant tensile modulus in linear region. (c) The standard exponential model fitted to the data predicts a continuous increase of stiffness beyond the used data range, whereas the fiber recruitment model correctly predicates a linear stress-strain relationship beyond the used data range.

In addition to greater structural realism, the recruitment model can also perform better in stress overload situations. For example, both the exponential model and the recruitment model were fitted to the same equi-biaxial strain data (Fig. 9-c,d). We then simulated the trend beyond the used data range and examined the predicted trend with the 10 real datum points. As anticipated, the exponential model predicted a continuous increase in tensile modulus with increased strain, whereas the fiber recruitment model provided accurately predicted the linear stress-strain relationship beyond the data range (Figure 7b). This result indicates the need for realistic constitutive models capable of prediction outside the range used to define the model, especially in situations where stress-overloads need to be accurately predicted.

4.3 – 3D stress model

All existing constitutive models for heart valve leaflet tissues assume either a uniform transmural stress distribution or utilize a membrane tension formulation. Both approaches ignore layer specific mechanical contributions and the implicit non-uniformity of the transmural stress distribution. Both the structural and mechanical data, especially the observation of the extensive network of inter-layer connections (Fig. 4-b) and identical strains under biaxial loading (Stella and Sacks, 2007) and the continuous transmural strain distribution (Fig. 7) suggest that the layers are tightly bonded. More importantly, they call into question the functional role of the spongiosa and whether it is a functional layer. Traditional thinking suggests that the spongiosa enables local movement and shearing between the fibrosa and ventricularis layers during dynamic loading due to its loose organization (Talman, 1996; Talman and Boughner, 2001, 1995). Instead, we view the spongiosa from a functional perspective: as an interior surface specialization of the fibrosa and ventricularis layers that strongly resists delamination through extensive collagen fiber inter-connections.

To develop an actual 3D stress model that accounts for layer specific contributions, we conducted novel studies to quantify the biaxial mechanical behavior of the two structurally distinct, load bearing aortic valve (AV) leaflet layers: the fibrosa and ventricularis (Stella and Sacks, 2007). For a bilayer tissue configuration, we utilized individual layers in their separated, stress free reference configuration and reconstruct the 3D stresses. We modified the equation 5 to include the separate contributions of both layers (Stella and Sacks, 2007), accounting for the different reference states of each layer (Stella and Sacks, 2007). Key results demonstrated that the fibrosa layer dominated the circumferential direction response (Fig. 10). Interestingly, the ventricularis experienced approximately twice the stress level at full physiological deformations. Moreover, the model also indicated that the ventricularis does not contribute to the radial component until a radial stretch of ~1.4. Clearly, layer micromechanics play a substantial role in valve leaflet function.

Figure 10.

Results of the 3D stress model of the AV along with the reconstructed layer stresses under physiological strains, based on biaxial experimental data taken from separated layers (Fig. 4). While the fibrosa layer clearly dominated the circumferential response, the ventricularis contributes ~2/3 of the total stress in the radial direction. Note, however, that the ventricularis only “kicks in” at radial stretches >1.4, suggesting a “over distention” protection role.

5 – Cellular function

5.1– Introduction

Within the three layers of the leaflet tissue, there resides a heterogenic population of interstitial cells (Filip et al., 1986; Messier et al., 1994; Mulholland and Gotlieb, 1996; Taylor et al., 2003). The heterogeneity of the interstitial cells is made up of fibroblasts, smooth muscle cells, and myofibroblasts, which have characteristics of both fibroblasts and smooth muscle cells. Studies of the interstitial cell population in both human and porcine subjects have revealed that the interstitial cell population was not localized to any one region or layer of the leaflet, but was present throughout the tissue (Bairati and DeBiasi, 1981; Merryman et al., 2006). The leaflets of all four HVs are also sheathed by single layer valvular endothelial cells (VECs), shown to be morphologically different from aortic ECs (Butcher and Nerem, 2004; Butcher et al., 2004). Additionally, VECs are aligned with the collagen architecture of the valve, which is circumferentially oriented (Deck, 1986). The VECs are believed to regulate vascular tone, inflammation, thrombosis, and remodeling, and their dysfunction has been linked with multiple disorders (Leask et al., 2003). Primarily, release of cytokines has been shown to cause changes in interstitial cell structure and function (Davies, 1997; Davies and Tripathi, 1993). It has also been speculated that there exists some physical communication between the VECs and VICS. However, to date no direct junctions have been observed between the two cell populations (Filip et al., 1986).

Interest in the myofibroblast cells (typically referred to as VICs) has grown in recent years, as they are believed to be critically important in valve pathophysiology. Primarily, VICs serve to maintain the structural integrity of the leaflet tissue by remodeling via protein synthesis and enzymatic degradation (e.g. MMPs). Their phenotype (which ranges from fibroblast-like to myo-like) is believed to be plastic and reversible, as VICs of normal, healthy valves were quiescent while in developing, diseased, and remodeling valves, the VICs were activated and contractile (Rabkin-Aikawa et al., 2004; Rabkin et al., 2002). While their dualistic nature is not fully understood, the VIC’s multifunctionality may be used for cell-cell communication, tissue remodeling, wound healing, and contraction (Mulholland and Gotlieb, 1996). Further, it is believed that when the phenotype of the resident VIC population is myo-like, the cells are actively remodeling the ECM. This indicates that the VIC phenotypic state at any given time is likely related to the current remodeling demands of the tissue (Rabkin et al., 2002).

5.2 – VIC micromechanical environment

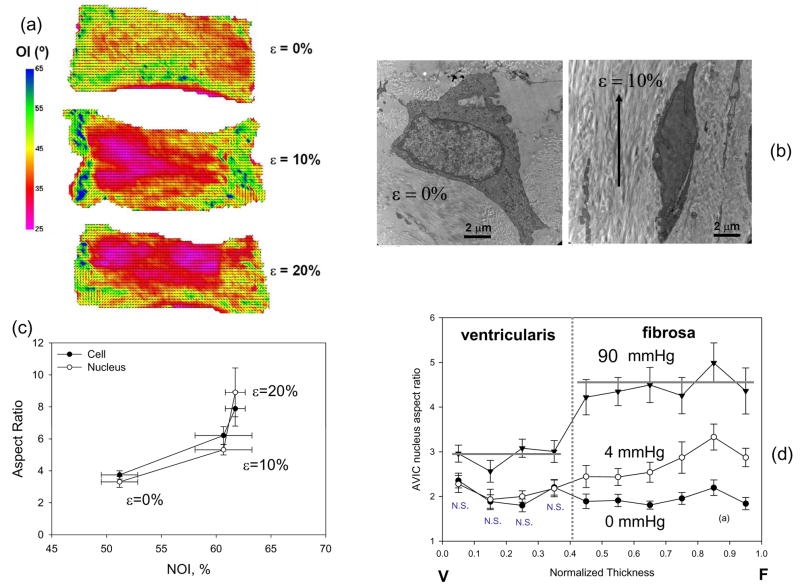
While it is known that AVICs can respond to mechanical stimuli in-vitro, the level of in-vivo AVIC deformation and its relation to local collagen fiber reorientation during the cardiac cycle remain unknown. To elucidate the micromechanics of the valve leaflets to increasing strain and the VIC micromechanical environment, AV leaflets were obtained, dissected from the aortic root, and subjected to a single extension of 0, 10, or 20% strain and fixed with either 2.5% Grade I or Grade II (EM grade) glutaraldehyde (Sigma Aldrich, St. Louis, MO) for 60 min in their strained state. Afterwards, the strips were either analyzed by small angle light scattering (SALS) (Sacks et al., 1997) to determine the change in collagen orientation with increasing strain, or imaged by transmission electron microscopy (TEM) to determine changes in cellular deformations. From the SALS measurements, we determined the normalized orientation index (Gilbert et al., 2006), a percentile scale of fiber orientation. From the TEM images, the orthogonal major and minor axes of the cell and nucleus were measured (SigmaScan, SYSTAT) and used to calculate aspect ratios as reported previously for AVICs fixed under increasing pressure (Huang et al., 2007; Kim et al., 2008).

As found previously (Sacks et al., 1997), increasing diastolic pressure induced increased NOI increased appreciably with increasing strain (Figure 11-a). Note that that there is no change in NOI from 10 to 20% strain demonstrate that 10% strain is sufficient to largely orient the collagen fibers. Since the myofibroblast nucleus makes up a sizeable portion of the cell volume, we found the cell and nucleus aspect ratio increase together with increasing strain (Figs. 11b,c). The nuclear aspect ratios were significantly greater (p<0.05) for the both the cell and nucleus at 20% compared to 0 and 10%. This finding also demonstrates that the nucleus is indeed a reliable cellular strain gage.

Figure 11.

(a) Collagen alignment results for circumferential strips of AV tissue subjected to 0, 10, and 20% strain. (b) Corresponding TEM images of AVICs at 0, 10, and 20% strain, with the drawn arrows show orientation of circumferential strain. (c) Both the cellular and nuclear aspect ratios changed similarly with strain and collagen alignment, supporting the use of NAR as a measure of cellular strain. In the intact valve, at 0 mmHg transvalvular pressure AVIC NAR values were uniform across the leaflet thickness In contrast, for normalized thickness positions between 0.4–1 (i.e., within the fibrosa layer) the AVIC NAR exhibited a trend of increased value with increased thickness position, approaching an average value of ~4.8 at 90 mmHg. These data indicate that AVICs in the different leaflet layers are subjected to dramatically different external stresses.

Based on these studies, we quantified VIC deformation under physiological loading conditions using porcine AV glutaraldehyde fixed under 0 to 90 mmHg transvalvular pressures. The resulting change in nuclear aspect ratio (NAR) was used as an index of overall cellular strain, and dependencies on spatial location and pressure loading levels quantified. Local collagen fiber alignment in the same valves was also quantified using small angle light scattering (SALS). When the VIC nuclear aspect ratios were plotted against normalized leaflet thickness, TVP level was found to have a significant effect on NAR values (Fig. 11-d). At zero mmHg the AVIC NAR maintained a constant transmural value of ~1.8. In the fibrosa layer (normalized thickness range from ~0.4 to 1), the AVIC NAR at 4 mmHg slightly increased. Under peak physiological transvalvular pressure (90 mmHg), the AVIC NAR were not only substantially greater than at low-pressure levels, but also demonstrated substantial transmural variations. In particular, the NAR exhibited greater increases in the fibrosa layer (~4.8 compared to ~3.0 for the ventricularis layer), which were statistically larger than both the corresponding 90 mmHg ventricularis and lower pressure NAR values (p < 0.05). This result indicated additional micromechanical and fiber-compaction effects occur at high pressure levels.

5.3 – VIC mechanical behavior

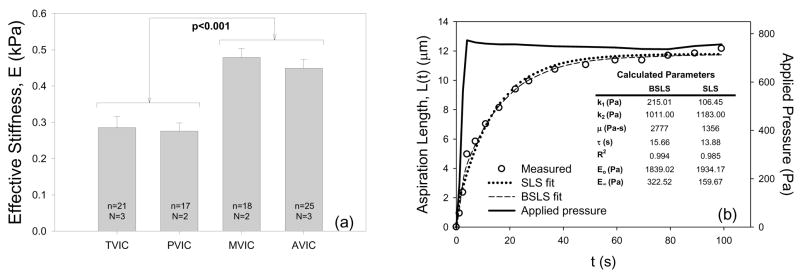
VICs are phenotypically plastic as they transdifferentiate into myofibroblasts during times of valve development, disease, and remodeling (Rabkin-Aikawa et al., 2004). One major indicator of VIC phenotype is the level of smooth muscle α-actin (SMA), which should dramatically affect cell stiffness. To begin to address this question, we have isolated ovine VICs from the four heart valves and subjected them to micropipette aspiration to assess cellular stiffness, and cytoskeletal composition and collagen biosynthesis were quantified using surrogates smooth muscle α-actin (SMA) (Merryman et al., 2006). Results revealed that VICs from the aortic and mitral valves were significantly stiffer (p<0.001) than the pulmonary and tricuspid VICs (Fig. 12-a). Additionally, left side isolated VICs contained significantly more (p<0.001) SMA and Hsp47 than the right side VICs. Mean VIC stiffness correlated well (r=0.973) with TVP; SMA and Hsp47 also correlated well (r=0.996) with one another. Moreover, assays were repeated for VICs in situ, and as with the in vitro results, the left side VIC protein levels were significantly greater (p<0.05).

Figure 12.

(a) Using micropipette aspiration, the effective stiffness, E, of VICs from each valve demonstrated highly significant differences (p<0.001) in mechanical properties between the AVIC and MVIC populations and the PVIC and TVIC populations. N=hearts used and n=cells tested for each valve type. (b) Time dependent responses during the aspiration experiment for AVIC indicated that both the BSLS and SLS model fit well. Inset table shows determined parameters from both models for the representative AVIC (n=1) that was used.

However, the mechanical properties of VICs have been measured under essentially quasi-static time frames, including both by micropipette (Merryman et al., 2006) and atomic force microscopy (Merryman et al., 2007). However, due to the rapid loading time of the AV leaflets during closure (~0.05 s), time-dependent effects may play a role in VIC deformation during physiological function. Recently, we explored AVIC viscoelastic behavior using the micropipette aspiration technique (Merryman et al., in press). We then modeled the resulting time-length data over the 100 sec test period using a standard linear solid (SLS) model which included Boltzmann superposition (Fig. 12-b). To quantify the degree of creep and stress relaxation during physiological timescales, simulations of micropipette aspiration were preformed with a valve loading time of 0.05 s and a full valve closure time of 0.3 s. The 0.05 s loading simulations suggest that, during valve closure, AVICs act elastically. During diastole, simulations revealed creep (4.65%) and stress relaxation (4.39%) over the 0.3 s physiological timescale. Simulations also indicated that if Boltzmann superposition was not used in parameter estimation, as in much of the micropipette literature, creep and stress relaxation predicted values were nearly doubled (7.92% and 7.35%, respectively). We conclude that while AVIC viscoelastic effects are negligible during valve closure, they likely contribute to the deformation time-history of AVIC deformation during diastole.

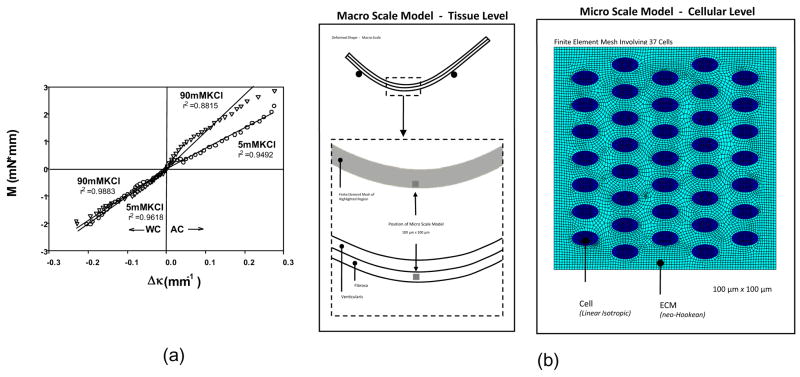
The above studies were conducted on isolated cells, while the role of VIC and extracellular matrix (ECM) interactions are not well understood. For example, the distinct differences in the composition and structure of the AV leaflet layers (fibrosa and ventricularis) have been shown to influence mechanical properties (Stella and Sacks, 2007). Our ability to measure the effects of changes in cellular stiffness in the dense collagenous valvular leaflets (Merryman et al., 2006) offers a unique opportunity to explore the in-situ VIC stiffness and local VIC-ECM mechanical interactions. To better determine the in-situ VIC stiffness, a multi-scale finite element model approach was developed based on our simulations of our flexural stiffness experiment were used to develop effective layer dependent mechanical properties (Fig 13). The bi-layer finite element model described was utilized as a macro scale model providing driving mechanical conditions to the micro cell-based model. The adopted approach is rooted in the mapping of micro region boundary deformations from the macro scale results. A two-dimensional plane stress finite model representing the 100 μm × 100 μm square section of tissue in the fibrosa was developed (Fig. 13). Based on available histological data presented above, cell dimensions were assumed to be 6 μm × 3.3 μm with the micro scale model containing 37 cells distributed in an uniform pattern simulating measured densities. The micro model ECM was modeled using a neo-Hookean material to match the assumption associated with the tissue level model, with the cell regions were modeled as a linear isotropic elastic material. Nodal displacements on the exterior boundary of the cell scale model are defined from interpolation of the displacement field of the deformed tissue scale model.

Figure 13.

(a) M vs. Δκ relations for both the AC and WC directions for specimens tested in 5 mM and 90 mM KCl, showing a highly linear response. To simulate the cellular responses in-situ a macro/micro model cellular level finite element model was developed (b). Here a bilayer model to simulate bending was utilized, the a 100 mm × 100 mm micro model utilized to estimate cellular contributions.

Macro-model simulation results indicated maximum principal strain of less than 5% for curvatures up to 0.12 mm⁻¹, a condition which supports the neo-Hookean material assumption. Further, macro model effectively predicted the nearly linear response observed by flexural testing (Fig. 13-a) (Merryman et al., 2006). Given the assumption that the fibrosa and ventricularis layer properties are dissimilar in a contracted state under AC conditions, the estimated fibrosa layer neo-Hookean modulus was 2.3 times greater than ventricularis layer. For this state, the ventricularis layer was parameterized with normal basal tonus properties (70 kPa) in combination with a fibrosa layer shear modulus of 160 kPa. The effect of basal tonus was to raise the base tissue response from 40 to 70 kPa in both layers.

However, at the micro-scale we determined that, as an independent mechanism, increases in cell stiffness alone cannot lead to a significant increases gross tissue stiffness. This property further suggests that cell contraction precipitates an increase in surrounding ECM. With respect to leaflet mechanical response in a low strain environment, cell contraction serves as a contributing mechanism. In contrast to the fiber dominated response to transvalvular pressure during valve closure, during valve opening the leaflets are highly compliant operating in a flexural mode of deformation. In this flexure dominated environment, the nature of cell-ECM interaction under contractile states may play an important role in regulating AVIC mechanobiological responses during normal valve function. Our present work also showed the potential layer specific effects the contractility of the AVIC population can have, particularly in the oft-diseased fibrosa layer of the AV. Pathologic and etiologic data indicate that nearly all cases of degenerative valve disease initiate and propagate from the fibrosa layer.

6 – Summary and Future trends

Growth and remodeling of valvular tissues

Biomechanics is ideally suited toward the study of valve function as it takes an “integrated” approach rather than a reductionist approach common to the biological sciences. This will be important not only for understanding basic valve pathologies, but also in related valvular issues never explored. For example, although other cardiovascular tissues, such as myocardium and aorta, have been shown to remodel when exposed to chronic changes in hemodynamic stresses, it has been ardently debated whether heart valves have the capacity to remodel in a non-pathological state

Perhaps the most interesting ways to look at valvular remodeling is the remodeling of clinical pulmonary-to-aortic valve transplants. Rabkin-Aikawa et al. (Rabkin-Aikawa et al., 2004) studied the pathologic features, cellular phenotypes, and matrix remodeling of clinical pulmonary-to-aortic valve transplants functioning up to 6 years. Autografts were found to be near-normal trilaminar cuspal structure and collagen architecture and viable valvular interstitial and endothelial cells throughout the time course. In contrast, cusps of homografts used to replace the pulmonary valves in the same patients were devitalized. Collagen content in autografts was comparable with that of normal valves and was higher than that seen in homograft valves. However, autograft walls were damaged, with granulation tissue (early) and scarring, with focal loss of normal smooth muscle cells, elastin, and collagen (late). Thus, the structure of pulmonary valves transplanted to the systemic circulation evolved toward that of normal aortic valves, with key processes including onset of a systemic endothelial cell phenotype and reversible plasticity of fibroblast-like valvular interstitial cells to myofibroblasts. In a related study, Aikawa et al. (Aikawa et al., 2006) performed quantitative histological assessment of 91 human semilunar valves obtained from fetuses at 14 to 19 and 20 to 39 weeks’ gestation; neonates minutes to 30 days old; children aged 2 to 16 years; and adults. They concluded that fetal valves possess a dynamic/adaptive structure and contain cells with an activated/immature phenotype. During postnatal life, activated cells gradually become quiescent, whereas collagen matures, which suggests a progressive, environmentally mediated adaptation

Another model of valvular remodeling is pregnancy, which produces dramatic hemodynamic and hormonal changes. Wells and Moeller have recently shown that (Wells and Moeller, 2008) for the first time, physiological remodeling of mature heart valve leaflets in a non-pathological state. Adaptive remodeling of heart valve leaflets during pregnancy involves dramatic changes in leaflet mechanical properties, dimensions, and composition, and these changes appear to be valve-dependent. These results have significant implications for heart valve tissue engineering. Understanding the mechanical and hormonal triggers of this remodeling will aid in directing the proper structural/functional development of tissue engineered leaflet tissues. These results also imply that engineered heart valves should maintain an implicit capacity for remodeling post-implantation.

The development of aortic valve problems in patients with LVADs has been reported previously (Park et al., 2004). Left ventricular assist device (LVAD) is a pump surgically connected to the heart and aorta in order to boost systemic blood flow (Rose et al., 2001). Aortic dysfunction in LVAD patients can develop within 6–12 months of LVAD implantation. It is estimated that approximately 50% of patients with pulsatile LVADs develop aortic insufficiency (AI) or aortic stenosis (AS) (Park et al., 2004). One institution reports routinely using ultrasound imaging to evaluate patients on VAD support for more than six months, finding that 50% of patients developed inappropriately increased rates and flows and are found to have mild to moderate degrees of native AI (Samuels et al., 2001). Insidious onset and progression of AI may also occur for undefined reasons such as (i) leaflet elongation, (ii) sinotubular dilatation, (iii) annular dilatation or (iv) any combination of the above. LVAD-induced structural abnormalities in the native aortic valve have been found in a significant number of LVAD patients as well, in particular the observation of commissural fusion. Aortic commissural fusion is a remodeling process in which fibrous tissue is deposited at the commissures of the coapted leaflets, adhering them together and preventing complete opening. Fusion can occur in one, two or all three of the cusps and usually covers a total of several mm of commissural length summed over all leaflets (Rose et al., 2000; Connelly et al., 2003). Recent studies have linked LVAD use to higher transvalvular pressures across the aortic valve (Travis et al., 2007; Zamarripa Garcia et al., 2008), and have found that strain in the valve leaflets is increased in LVAD patients, particularly under series flow conditions (Enriquez et al., 2008). These findings suggest that the development of aortic fusion is related to the increase in mechanical stress and strain in the leaflets, as they are subjected to higher transvalvular pressures for a longer percentage of the cardiac cycle than under normal flow conditions. Further investigation into LVAD-induced remodeling and dysfunction of the aortic valve are warranted.

Computational approaches to valve function

In the authors’ opinion, we are now entering a level of bioengineering knowledge of valvular function wherein computational approaches can begin to realistically be applied. For example, recent work from the Einstein et al. on dynamic modeling (Einstein et al., 2004; Einstein et al., 2005) simulated early acoustic detection of changes in mitral valve properties, which may lead to the better management of mitral valve disease. To model the transient vibrations of the mitral valve apparatus bathed in a blood medium, they constructed a dynamic nonlinear fluid-coupled finite element model of the valve leaflets and chordae tendinae. The gross movement and small-scale acoustic vibrations of the valvular structures result from the application of physiologic pressure loads. Material changes that preserved the anisotropy of the valve leaflets were found to preserve valvular function. By contrast, material changes that altered the anisotropy of the valve were found to profoundly alter valvular function. These changes were manifest in the acoustic signatures of the valve closure sounds. Abnormally, stiffened valves closed more slowly and were accompanied by lower peak frequencies.

Recent work by Driessen et al. (Driessen et al., 2003; Driessen et al., 2005) developed a finite-element (FE) model to relate changes in collagen fiber content and orientation to the mechanical loading condition within the engineered heart valve construct. They hypothesized that collagen fibers aligned with principal strain directions and that collagen content increased with the fiber stretch. The results indicate that the computed preferred fiber directions run from commissure to commissure and show a resemblance to experimental data from native aortic heart valves. These models represent only a first step in developing physiologically realistic models of heart valve function. In particular there is a need for thorough experimental validation and true dynamic models that couple tissue (solid) and blood (fluid). For example, Kim et al. have developed (Kim et al., 2007; Kim et al., 2006) have shown for pericardial bioprosthetic heart valves that In addition, the present simulation also demonstrated the importance of including the bending component together with the in-plane material behavior of the leaflets towards physiologically realistic deformation of the leaflets. Moreover, dynamic simulations with experimentally determined leaflet material specification can be potentially used to modify the valve towards an optimal design to minimize regions of stress concentration and structural failure.

Surgical replacement of diseased heart valves by mechanical and tissue valve substitutes is now commonplace and enhances survival and quality of life for many patients. However, repairs of congenital deformities require very small valve sizes that are simply not commercially available. Further, in pediatric applications growth of the replacement valve is essential to eliminate the need for reoperations as the patient grows. There are a variety of devices available for replacement of cardiac valves, but all current devices have significant limitations that result in a continuing risk for morbidity and mortality (Schoen and Levy, 1999). The ultimate heart valve replacement is characterized as non-obstructive, non-thrombogenic living tissue valve substitute that lasts the lifetime of the patient, provides ongoing remodeling and repair of cumulative injury and potentially grows with the recipient is an important goal. Such a valve would be particularly useful in the treatment of the approximately 20,000 children with congenital heart disease born in the United States each year and especially those with valvular disease. In this population the anticoagulation required with mechanical valves is particularly dangerous and tissue valve substitutes undergo accelerated calcification. Moreover, the placement of oversized valves extra-anatomically in the right ventricular outflow necessitates multiple subsequent surgeries for these children as valves and conduit devices repeatedly become stenosed over time. Thus, a fundamental problem inherent to the use of existing mechanical and biological prostheses in the pediatric population is their failure to grow, repair and remodel. Regardless of the design specifics of current prosthetic valve devices, none offers any potential for growth, and therefore pediatric patients requiring valve replacement will require reoperations to place larger devices to accommodate the growth of the patient (Kirklin et al., 1993).

For example, it has been reported the gradual development of a tri-layered structure, including variations in collagen, GAG, and elastin, after 15–20 weeks post implantation (Rabkin et al., 2002). Yet, we do not know if these layers are functionally equivalent to the native valve, and even what mechanisms guide their formation in-vivo, nor do we have no knowledge of the anisotropic mechanical properties of TEHV leaflet tissue, and how well these compare with the native PV (Sacks et al., 2009). In the principals of Functional Tissue Engineering (Butler et al., 2000), it is stated that there a need to establish the minimal functional parameters necessary to produce tissue equivalents. For heart valves, this includes quantification of the anisotropic mechanical properties of TEHV leaflet tissue, which can only be achieved thru multi-axial testing to determine if the developing tissue is a suitable valve replacement. Further, the degree of cellular function and similarity to the native valve has yet to be determined. Yet, despite the fundamental nature of these requirements, we currently have no knowledge of the TEHV functional similarity at the tissue and cellular levels to the native PV, which will ultimately dictate functionality and long-term durability (Sacks et al., 2009).

Ultimately, we would like to connect the organ-scale simulations to evaluate our understanding of AVIC mechano-transduction, it would be beneficial to develop an AVIC phenotypic/biosynthetic model linked to organ-level deformations. In the AVL, transvalvular pressures (TVPs) above ~5 mmHg predominately result in ECM compaction as the collagen fibers become uncrimped and taut. From this ECM compaction, significant AVIC nucleus aspect ratio has been observed with increasing pressures (Fig. 13). Even a phenomenological model could thus simulate the AVIC population with mechanical inputs (quantified by the deformation of the AVIC nuclei) and cytokine activity. It could then be possible to predict the phenotypic and biosynthetic response of AVICs after repeated loading cycles.

NOMENCLATURE

AV

Aortic valve

AC

when referred to flexure, the direction against the natural curvature of the leaflet

AVEC

aortic valve endothelial cell

AVIC

aortic valve interstitial cell

CFD

computational fluid dynamic (modeling)

Δκ

Change in curvature in flexure

E

the effective stiffness in flexure

EC

endothelial cell

ECM

extracellular matrix

GAG

glycosaminoglycans

HSP47

heat shock protein 47

I

second moment of inertia

M

Applied bending moment

MV

mitral valve

MVAL

mitral valve anterior leaflet

MVPL

mitral valve posterior leaflet

PV

Pulmonary valve

SMA

smooth muscle actin (intracellular)

TEHV

tissue engineered heart valve

TV

Tricuspid valve

TVP

transvalvular pressure

VIC

valvular interstitial cell

WC

when referred to flexure, the direction with the natural curvature of the leaflet