Biomechanical Issues in Endovascular Device Design

Abstract

The biomechanical nature of the arterial system and its major disease states provides a series of challenges to treatment strategies. Endovascular device design objectives have mostly centered on short-term challenges, such as deployability and immediate restoration of reliable flow channels. The resulting design features may be at odds with long-term clinical success. In-stent restenosis, endoleaks, and loss of device structural integrity (e.g., strut fractures) are all manifestations of a lack of compatibility between the host vessel biomechanical environment and the implant design. Initial attempts to adapt device designs for increased compatibility, including drug-eluting and bioabsorbable stents, barely begin to explore the ways in which implant design can be modulated in time to minimize risk of failure. Biomechanical modeling has the potential to provide a virtual vascular environment in which new designs can be tested for their implications on long-term tissue reaction. These models will be based on high quality, highly resolved imaging information, as well as mechanobiology experiments from the cellular to the whole tissue level. These models can then be extended to incorporate biodegradation mechanics, facilitating the next generations of devices whose designs (including drug delivery profiles) change with time to enhance healing. The possibility of initiating changes in device design or drug release according to information on vascular healing (through clinical intervention or automated methods) provides the opportunity for truly individualized dynamic device design optimization.

The human cardiovascular system has evolved into a sophisticated biomechanical structure capable of sustaining living tissues having widely different and dynamic metabolic needs. The biomechanical nature of this system is the key to its ability to perform its variety of functions continuously over decades.

The primary purposes of the system, delivery of nutrients to and removal of waste from tissues, are facilitated through an elegant combination of fluid mechanical features. Its primary pump generates sufficient pressure to distribute a fluid approximately four times more viscous than water through a vast network of tubes whose smallest diameter is smaller than a red blood cell. The highly dynamic, near turbulent cardiac output is transformed by the well-tuned elasticity of the larger arteries into a steady, highly viscous flow prior to arrival in the capillaries. The mass transfer occurring at this level is amazingly efficient due to the actions of highly evolved cells in the blood and vessel walls. These biomechanical challenges place high demands on the larger and medium-sized arteries, which are subjected to the most vigorous pressures and flows found in the system. When the fluid delivery capability or structural integrity of any of these vessels is threatened by disease, the consequences can be fatal.

The ability to deliver blood to the distal tissues depends on the resistance presented by the arteries, which is a strongly non-linear function of vessel diameter. The larger and medium-sized arteries normally present a very low resistance to blood flow compared to the arterioles and capillaries. This is exemplified by the fact that the mean blood pressure falls by only a few millimeters of mercury from the heart to the level of the arterioles.¹ When stenotic disease develops, the real danger begins when the resistance of these normally low-resistance vessels becomes high enough to limit flow. Resistance is a strongly non-linear function of percent stenosis, increasing several fold beginning at around 70% stenosis (Fig. 1). Small increases in percent stenosis beyond that point lead to tremendous increases in flow resistance.

Figure 1.

♦ Relationship between percent stenosis and flow resistance, adapted from Logan.⁴⁶ Casts of several diseased human coronary arteries were perfused at a physiological flow rate with a tube whose unobstructed diameter was 3.2 mm. Adapted and reprinted with permission from IEEE Biomedical Engineering. Copyright 1975, IEEE.

The biomechanical danger presented by aneurysms is quite different and primarily related to solid mechanics rather than fluid mechanics. As the aneurysm grows, the stress in the thinning vessel wall increases dramatically, as illustrated by the Law of Laplace for the simplified case of a straight, thin-walled tube:

When the stress at any one point in the structure exceeds the material strength, rupture occurs, resulting in massive blood loss. Bioengineers have employed computational modeling techniques with the aim of providing more accurate rupture prediction protocols than maximum diameter alone.^2–4

BIOMECHANICS OF CURRENT ENDOVASCULAR TREATMENTS

Despite the drastically different biomechanical natures of these diseases, the treatment modalities share a common immediate goal of restoring the primary biomechanical function of effective and reliable fluid transfer. In the case of stenotic disease, endovascular tools, including angioplasty and stenting, strive to minimize the resistance of the diseased vessel to restore flow to distal tissues. For aortic aneurysms, the deployment of endografts intends to serve two purposes. The first is to isolate the vulnerable aneurysm wall from blood pressure (reducing vessel wall stress and thus rupture risk), and the second is to provide a new, sealed conduit for flow. The minimally invasive nature of both stent and endograft procedures provides advantages over open surgery. However, clinical failures involving both procedures remind us that the short-term biomechanical requirements that have been the focal point for device design may be at odds with long-term tolerance by the body and device integrity.

Stented Artery Biomechanics

Clinical failure modes of stent procedures include restenosis due to intimal hyperplasia or thrombosis, as well as vessel closure due to strut fractures. Restenosis can be seen as a lack of long-term compatibility between the stent (including implantation procedure) and the body. The initial expansion of the artery can result in disruption of the internal or external elastic laminae, both of which have been associated with higher risk for restenosis.⁵

The degree to which the elastic laminae are disrupted is directly related to the degree of expansion of the vessel. It is generally desirable to expand the diseased portion of the vessel out to at least the full healthy diameter. However, as seen in Figure 1, one can significantly reduce the resistance to flow using smaller degrees of initial expansion, i.e., restoration of the majority of the flow can be achieved leaving some residual stenosis in place. Of course, one has to foresee the development of some degree of restenosis, so partial expansion would have its own set of risks.

The biomechanical interaction of the stent and artery wall can also be important in the long-term success of the procedure. The stress placed on the artery wall by a permanent implant (unlike angioplasty alone) remains as a trigger for inflammation and vessel wall cellular proliferation long after the implantation procedure. Modeling the stent/artery interaction requires highly sophisticated modeling techniques that stretch the capabilities of even the most modern computational mechanics software packages. Arteries are complex, non-homogeneous, and non-isotropic materials that undergo large deformations not encountered in most engineering materials. Thus, one cannot apply the linear, small deformation mechanical modeling employed in most engineering applications, such as building and bridge design. The contact modeling with the deformable stent structure is another challenging aspect. The models that have been constructed show that the amount and distribution of stress depends heavily on stent design, with more sparse mesh designs (Fig. 2) provoking less stress on the artery wall.⁶

Figure 2.

♦ Color-encoded circumferential artery wall stress at the intimal surface, as modeled computationally in different stent designs. Stress is higher in designs with closely packed struts. Reprinted with permission from Bedoya et al.⁶ in Journal of Biomechanical Engineering. Copyright 2006, ASME.

This stress also depends on the mechanical properties of the plaque.^7–9 Biomechanical studies have shown that the relative stiffness of stenotic plaque and the stent both play important roles in determining stress at the internal elastic lamina and postprocedure lumen diameter. In the presence of a softer plaque, the artery wall stress is not heavily influenced by stent design. For stiffer plaques, stent design has an important effect on artery wall stress. As expected, less rigid stent designs result in a smaller final lumen diameter. Still, it has been demonstrated that modeling tools can be developed to predict stent/artery/plaque behavior given sufficient material property information. These tools should eventually be employed in lesion-specific stenting strategies.

Stents also provoke changes in blood flow patterns that depend on strut configuration (Fig. 3). Near-wall flow stagnation and its effects on convection of blood-borne cells to the artery wall influence the degree of platelet adhesion^10,11 and likely the delivery of inflammatory cells. Endothelial cell regrowth can also be affected by these flow patterns.^12,13 The degree of flow changes also depends on the degree of overexpansion, with larger postprocedure diameters being associated with lower wall shear stress.¹⁴ Flow modeling in stented arteries has demonstrated that areas of low wall shear stress tend to develop thicker neointimas.^15–17

Figure 3.

♦ Blood flow patterns in different stent designs visualized through color-encoded axial wall shear stress (dynes/cm²) taken at the mean flow rate for (A) Wallstent, (B) Bx Velocity stent, (C) Aurora stent, and (D) NIR stent. Reprinted with permission from Duraiswamy et al.⁴⁷ in Journal of Biomechanical Engineering. Copyright 2009, ASME.

Biomechanics play a quite different role when considering stent strut fractures. This phenomenon has been observed most often in the arteries of the lower limbs,¹⁸ but can also occur in coronary arteries.¹⁹ While marketing approval generally requires extensive fatigue testing, the conditions of these tests do not always incorporate all of the relevant physiological forces. Traditional fatigue testing, in which pressure is cycled in a compliant tube, does not include the “off-axis” forces present in vivo. Such forces arise from adjacent tissue movement due to myocardial contraction,²⁰ respiration,²¹ or limb flexion.²² Such complex 3-dimensional (3D) forces present high challenges for biomechanical modeling. First, there is a need to understand the nature of these complex soft tissue/hard tissue interactions in the pre-treatment state. Then, incorporation of the contact between the stent, the artery, and these adjacent tissues can commence. The associated challenges to current modeling techniques and computer hardware are immense, but some initial modeling studies are emerging.²³ These challenges will be best addressed by industry/government/academia partnerships.

Aneurysm Endograft Biomechanics

The lack of consistent reliability of current endograft technology requires careful patient monitoring over the years following implantation. A reliable outcome can be achieved if the endograft can serve either of the two intended functions. If the endograft results in a reduction in aneurysm wall stress to the point that the risk of rupture is eliminated, or if the new, sealed conduit can maintain its integrity even in the presence of a rupture, then patient safety is assured. Unfortunately, a variety of failure mechanisms have manifested, resulting in five endoleak classifications. With the exception of type II endoleaks, all of these failure modes can be seen as a loss of integrity in either the endograft or its sealing points.

Type I endoleaks, which are the result of failure to maintain complete contact between the endograft and the vessel wall, are commonly associated with aneurysm rupture because the endograft has failed in its two major duties. The endoleak allows pressurization of the sac and prevents the development of a new, sealed channel. The belief that forces induced by blood flow may be responsible for type I endoleaks has led many to employ biomechanical modeling to estimate these forces.^24–26 It is, in fact, not difficult to prove, using rather unsophisticated control volume analysis, that forces due to blood pressure dominate over forces due to frictional shear stress and momentum transfer (the so-called “windsock” effect). Thus, one can obtain an accurate estimate of the total force due to blood flow simply by multiplying systolic blood pressure by the cross-sectional areas of the inlet and outlets (using their orientation to obtain the directions of the force vectors). Estimates of these forces are typically in the 5- to 10-Newton range, which coincides with the force necessary to dislodge some endografts based on in-vitro testing.²⁷

A rather underappreciated possible reason for the formation of type I endoleaks is the vessel deformations that can occur due to normal respiration and limb movement. The effects of these motions on deformations in the abdominal aorta and iliac arteries have been estimated as being on the order of only a few millimeters,²⁸ but they are obviously unavoidable, repeating phenomena. Hip flexion can create changes in curvature of the iliac arteries as well, which may result in axial tension or compression of the endograft, depending on individual anatomy. The implications of these movements on endoleak development deserve further investigation. Regardless, it makes sense to incorporate axial extensibility in endograft design to minimize the stress placed on fixation points.

Forces due to complex 3D vessel deformations may also play a role in the formation of type III endoleaks. Contact points between metal and fabric can be focal points of high stress depending on the magnitude, frequency of occurrence, and duration of bending or torsion. These factors are likely to be highly patient specific. Still, measures should be taken to insure that endografts are able to withstand more than simple cycling pressure in a consistently straight tube.

OVERCOMING LONG-TERM BIOMECHANICAL CHALLENGES

Minimizing clinical failures of implantable endovascular devices will involve consideration of both the short-term requirements of a reliable flow channel and the long-term interaction of the implant with the vessel and surrounding tissues. These are biomechanical challenges for which current technologies are only partially prepared. A fuller array of imaging tools, biomechanical models, knowledge of device/tissue interactions, materials technology and drug delivery, and their integration into an iterative device design process is required.

Imaging Requirements

Advances in imaging technologies hold the promise that we can know much more about the target lesion in advance of any treatment procedure. While fluoroscopy, computed tomography (CT), and magnetic resonance imaging (MRI) have provided good quality lumen imaging for some years now, high-resolution intravascular imaging of the vessel wall (normal and diseased portions) can provide important information that can be used to optimize treatment. For stenotic lesions, catheter-based intravascular ultrasound (IVUS) can provide some of this information, but this tool is limited by spatial resolution and in some cases poor contrast between plaque components.²⁹ IVUS can provide a gross approximation of plaque stiffness (elastography) and detect the presence of necrotic cores or fibrofatty tissue (radiofrequency backscatter signal analysis).^30–32 Optical coherence tomography (OCT) offers better potential spatial resolution but requires saline flushing to provide an optically clear path to the artery wall.³³ There are considerable additional benefits of OCT, including sufficient resolution to detect thin fibrous caps and the possibility to distinguish cell types, in particular, superficial macrophage infiltration.^34,35 Multimodality approaches combining high-resolution volumetric imaging with biochemical composition assessment of plaques are also being explored.^36,37

For the sake of model construction, this information from micro-scale imaging modalities must be integrated up to the tissue level. The possibility to construct biomechanical models of device/tissue interactions including non-homogeneous tissue models then becomes more feasible. Holzapfel et al.³⁸ used high-resolution MRI of a diseased human external iliac artery to model its interaction with different stent types. Computational biomechanical modeling of aortic aneurysms is currently limited by insufficient spatial resolution to resolve wall thickness, a parameter of crucial importance in predicting localized regions of peak stress. These kinds of studies indicate great potential for biomechanical modeling, but the challenge of obtaining high-resolution imaging in patients must be overcome for these modeling tools to find widespread clinical application.

Insuring the long-term structural integrity of implants will require imaging information at still higher spatial scales. As mentioned above, movement of adjacent tissue can place complex forces on implants. The work from Taylor's laboratory at Stanford provides some idea of the deformations involved, but does not necessarily allow the determination of the forces to which an implant will be subjected. Placing a stent in a femoral artery, for example, changes the way that artery deforms with knee flexion.³⁹ Implantation of fenestrated endografts can have a significant effect on renal artery motion during respiration, depending on whether or not stents are deployed into the branches.⁴⁰ Images of healthy vessels or even pre-treatment diseased vessel deformations could be used as input to models that estimate the forces involved. It would also be necessary to have a reasonable assessment of vessel and surrounding tissue mechanical properties.

Incorporation of Tissue Adaptation in Biomechanical Modeling and Device Design

Enhanced knowledge of the target lesion will only help patient care if we know more about how endovascular devices interact with specific lesion types on both acute and chronic time scales. The biomechanical models mentioned above are currently limited to the situation immediately post implant. While this can provide some indication of the likelihood of adverse tissue reactions, modeling tools should be developed that incorporate dynamic tissue response components.

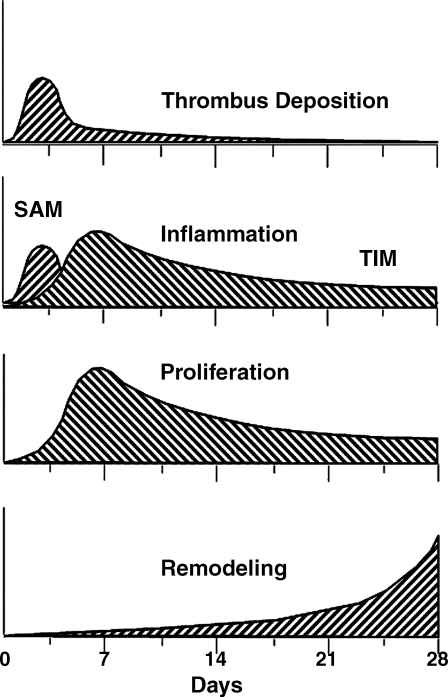
It is well known that artery wall structure adapts to changing mechanical loads, e.g., the thickening in response to hypertension. However, the change in mechanical loading due to stent implantation is sudden, and the stresses induced are well above the normal physiological range. The time course of events (Fig. 4) that follow stent implantation are known in the approximate sense,⁴¹ but not in enough detail to predict in-vivo reactions to stent implantation. A more complete picture of tissue reactions to implants will require a combination of in-vitro studies at both the cell and tissue levels with biomechanical modeling and in-vivo observations (animal and human).

Figure 4.

♦ Time course of artery wall reaction to stent implantation, illustrating the highly time dependent nature. Adapted with permission from Edelman and Rogers⁴¹ in the American Journal of Cardiology. Copyright 1998, Elsevier Health Science Journals. SAM: surface adherent monocytes, TIM: tissue infiltrating monocytes.

There are enough reports in the literature to encourage further research in these directions. It has been known for some time that all of the cells that make up the artery wall respond to mechanical stimuli. Endothelial cells align and elongate with flow direction.⁴² Smooth muscle cells respond to cyclic stretching with alignment perpendicular to the direction of stretch.⁴³ These responses by both cell types are accompanied by changes in mRNA expression, along with changes in protein expression and cytoskeletal reorganization. Adventitial fibroblasts also respond with phenotype changes and, in fact, are the first cells to respond to balloon injury.⁴⁴ To be able to apply the results of cell mechanobiology studies to modeling device/tissue interactions, however, these experiments must be performed under mechanical conditions that are more representative of those following device implantation. Stented arteries experience localized regions of high stress adjacent to struts, with stress tapering off strongly in both the axial and radial directions. These gradients in stress may be important in triggering cell phenotype change and migration. Unfortunately, most cell mechanobiology experiments employ devices that subject cells to uniform stress environments. Devices that employ more physiologically relevant mechanical environments are required.

Integration of cellular level information into models representative of tissue level responses will benefit greatly from in-vitro and in-vivo studies at that scale. Mechanobiology experiments at the tissue level are also possible with excised arteries or tissue engineered constructs, but it is difficult to maintain tissue viability over the time required to observe important reactions to stents. In-vivo studies in animals, or preferably humans, can be used to verify and refine modeling tools, provided the availability of high-quality imaging at multiple time points. The same imaging challenges discussed above will limit the quality of the information gained, as will the lack of minimally- or noninvasive imaging modalities that minimize or eliminate the exposure to potentially harmful contrast agents.

Time-Dependent (Dynamic) Implant Design

Better information on implant biomechanics and long-term tissue reactions should naturally lead to the development of implant designs that change with time to optimize success. There are some attempts in modern devices to account for the time dependency of the reaction to the implant, but these devices should be viewed as a first-order approximation to what eventually should be standard design practice.

The drug delivery profile of drug-eluting stents has been tailored using different polymer formulations to release drug gradually into the wall over a period of weeks. The current strategy of these designs is to restrict one of the principal reactions of the artery wall to the injury caused by the implant, namely, smooth muscle cell (SMC) proliferation. This process takes some time to ramp up (Fig. 4), so it makes sense to try to release the drug gradually. This strategy should be refined to one in which drug release works in concert with the artery's healing process, rather than against it. The emerging evidence that cytostatic drugs aimed at SMCs also affect re-endothelialization provides an example of unintended consequences of drug elution strategies. Technologies to deliver multiple drugs along different time scales are certainly feasible and should be pursued once it is known which drugs should be released for a given target lesion.

There are also a number of companies trying to develop bioabsorbable stents based on the idea that a mechanical scaffold should not be necessary once the artery wall has healed and remodeled. The design challenges are numerous, including deciding when the stent dissolves to the point where it no longer provides structural support and then designing strut configurations for this target time in an environment of dynamic mechanical loading. Our group has begun to develop material models that can be used for this design challenge, provided that information on time-dependent material properties can be obtained.⁴⁵ Bioabsorbable stents are promising technologies for vessels subjected to off-axis forces, since the materials targeted for their construction may be less prone to crack propagation leading to fractures. Perhaps more importantly, the struts would have to withstand these forces only for a few months, rather than multiple years.

Incorporation of time-dependent drug elution and biodegradation offers the possibility of implantable devices whose pharmacological and biomechanical effects on the artery wall moderate and even enhance the healing process. If high quality multi-scale (cell to surrounding tissue) information on the diseased segment can be obtained from imaging modalities at multiple time points, this will provide much of the information required to model the tissue reaction process. The goal of this ambitious modeling quest should be a virtual environment in which new designs can be tested for their short- and long-term effects. Finally, combining with models of implant degradation should enhance the reliability of implants that change with time.

ROADMAPPING THE FUTURE OF ENDOVASCULAR IMPLANT BIOMECHANICS

The use of endovascular devices to restore reliable flow vessel integrity requires both short- and long-term biomechanical considerations. Many of the design challenges confronted in these first decades of endovascular technology focused on short-term needs, such as facilitating the implantation procedure. This initial approach has been successful enough to establish a vibrant market for these less invasive options to surgery. Unfortunately, failures still do occur in a rather unpredictable manner, requiring long-term monitoring, pharmaceutical treatment, or additional intervention.

Many of the clinical failures that limit the applicability of endovascular devices can be avoided or resolved using better biomechanical modeling. From improved device design tools to lesion-specific guidance on implant choice, there is much promise in the application of current and future modeling capabilities. Given the current states of the clinical and engineering arts, the following steps may be envisioned:

Step 1: Incorporate high-quality imaging of the lesion, vessel, and surrounding tissue to improve the design of current permanent implants so that they can withstand the biomechanical challenges presented by tissue deformations of all types. Both computational and experimental tools are currently available to aid in this task, but information on the physiological deformations before and after implantation, as well as tissue properties, is incomplete.

Step 2: Analyze clinical and experimental data to determine which implant designs perform best in certain types of diseased tissue and design devices to address particular lesion types. Extend these capabilities to refine drug delivery strategies.

Step 3: Develop biomechanical modeling strategies that predict tissue reactions to implants. These models should first be applied to improving the design of permanent implants. They should also span multiple scales, from cellular mechanotransduction to the whole tissue level, and should be based on experimental data from studies employing physiological mechanical stimuli.

Step 4: Conceptualize devices whose designs and drug delivery profiles change with time (e.g., through biodegradation) to work in concert with the body's reactions and enhance the healing process. The construction of the modeling techniques above will greatly facilitate the design of these devices.

Step 5: Devise techniques for actively changing devices post implant in response to information from minimally- or noninvasive imaging on the healing process. For example, once it has been determined that arterial wall healing has progressed sufficiently, the interventionist could trigger degradation of the endovascular implant. The related technology of convertible or removable vena cava filters is based on a similar concept.

Step 6: Develop implants that change in response to the various phases of the healing process in an automatic fashion. Perhaps it will eventually be possible to mount small sensors on implants that can distinguish various phases of healing then actuate device changes without clinical intervention.

Clearly, these are ambitious programs with varying time scales to adoption. Accomplishing any of these steps will require extensive collaboration between clinicians, industry, academia, and government organizations.