The elastic modulus of Matrigel™ as determined by atomic force microscopy

Abstract

Recent studies indicate that the biophysical properties of the cellular microenvironment strongly influence a variety of fundamental cell behaviors. The extracellular matrix’s (ECM) response to mechanical force, described mathematically as the elastic modulus, is believed to play a particularly critical role in regulatory and pathological cell behaviors. The basement membrane (BM) is a specialization of the ECM that serves as the immediate interface for many cell types (e.g. all epithelial cells) and through which cells are connected to the underlying stroma. Matrigel is a commercially available BM-like complex and serves as an easily accessible experimental simulant of native BMs. However, the local elastic modulus of Matrigel has not been defined under physiological conditions. Here we present the procedures and results of indentation tests performed on Matrigel with atomic force microscopy (AFM) in an aqueous, temperature controlled environment. The average modulus value was found to be approximately 450 Pa.. However, this result is considerably higher than macroscopic shear storage moduli reported in the scientific literature. The reason for this discrepancy is believed to result from differences in test methods and the tendency of Matrigel to soften at temperatures below 37° C.

Introduction

Cells actively sense and respond to both chemical and physical stimuli in their surrounding microenvironments. Cellular responses to chemical stimuli have been studied for many years, but a number of recent reports suggest that biophysical cues such as substrate topography (Flemming et al., 1999; Karuri et al., 2004; Teixeira et al., 2006) and compliance (Discher et al., 2005; Flanagan et al., 2002; Pelham and Wang, 1997) play an important role in both normal and pathological behaviors. Stem cell lineage specification (Engler et al., 2006), malignant behavior in mammary gland epithelia (Paszek et al., 2005), and fundamental cell behaviors such as migration (Pelham and Wang, 1997; Wong et al., 2003), proliferation (McDaniel et al., 2007; Thompson et al., 2005), and differentiation (Boontheekul et al., 2007; Peyton et al., 2006) have been shown to be affected by substrate compliance. These studies collectively imply that extracellular matrix (ECM) compliance acts as a homeostatic regulator of normal tissue function. This finding directly applies to the biocompatibility of implantable prosthetic devices, the efficacy of tissue engineering processes, and the relevance of cell culture studies. Therefore, it has become apparent that mechanical characterization of the cellular microenvironment, both in its natural state and in the laboratory, is increasingly necessary to develop a more complete description of biological systems.

Within the context of environmental cueing of cell behavior, a specialized layer of ECM known as the basement membrane (BM) is of particular interest. The BM is a protein complex consisting mainly of collagens, laminins, nidogen/entactin, and perlecan (LeBleu et al., 2007). It is in direct contact with the basal layer of epithelial and vascular endothelial cells and is a ubiquitous structure found in a wide range of tissues across all vertebrate species. However, experimentation with BMs is complicated by the relative difficulty and expense associated with obtaining and isolating intact, native samples in a laboratory setting. Additionally, the use of primarily isolated basement membranes from native tissues introduces the intrinsic individual variability associated with genetic differences, age and health of the individual organism.

Matrigel (Kleinman and Martin, 2005), a reconstituted BM-like complex harvested from Engelbreth-Holm-Swarm mouse tumor cultures, is a commercially available simulant of native BMs. It has found widespread use as a model system for the study of tumor cell invasion (Zaman et al., 2006), hepatocyte aggregation (Semler et al., 2000), stem cell differentiation (Ma et al., 2008), and the formation of tube-like structures by vascular endothelial cells (Kobayashi et al., 2004). Matrigel is relatively versatile and can be molded into thick gels, used to coat cell culture substrates with ECM proteins, or geometrically patterned using soft lithography techniques (Sodunke et al., 2007). The nanoscale topographic features of Matrigel have been shown to be similar to those of several native BMs (Abrams et al., 2000), but the appropriateness of its use as a mechanical surrogate has not been established. In order to quantify the stiffness of Matrigel, an atomic force microscope (AFM) was used to perform indentation tests under physiological conditions (37° C, in liquid). AFM has been widely used as a mechanical testing device for soft, hydrated materials because it allows small forces to be applied over short distances in an aqueous environment (Domke and Radmacher, 1998). Therefore, the elastic modulus reported herein is highly relevant because it more closely mimics the conditions experienced by living cells in vivo.

Materials and methods

2.1. Matrigel sample preparation

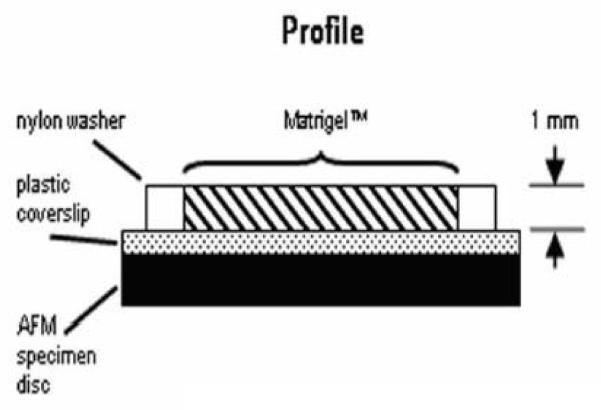
Three different lots of Matrigel (catalog number 354234, BD Biosciences, San Jose, CA USA) were tested and prepared according to the manufacturer. Samples for AFM indentation tests were created by cold-pipetting Matrigel into wells mounted on AFM specimen discs (Fig. 1). Specimens were made approximately 1 mm thick in order to avoid substrate effects during indentation (Domke and Radmacher, 1998). The Matrigel was polymerized for 30 minutes in a humidified incubator at 37° C, and then placed in 1x phosphate buffered saline (PBS — pH 7.4) at 37° C for 24 hours to allow complete gelation. Sample temperatures were not allowed to drop below 37° C before or during experimentation. In order to accomplish this, an AFM environmental chamber was built to maintain a constant temperature of 37° C. The chamber consists of a commercially available thermoelectric heater/cooler (Igloo Products Corp., Katy, TX) modified with a digital temperature controller. All AFM parts, including probe and fluid cell, were preheated in the environmental chamber for at least 30 minutes prior to use, and all tests were conducted in PBS preheated to approximately 45° C to ensure that the system did not drop below 37° C over the course of experimentation. Throughout the experiment the AFM remained inside the environmental chamber and the temperature was maintained at 37° C.

2.2. AFM indentation testing

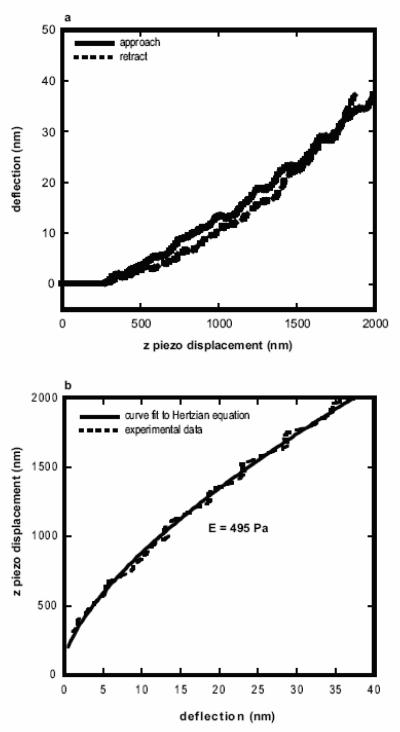
A Multimode AFM with Nanoscope IIIa controller (Veeco Instruments Inc., Santa Barbara, CA, USA ) was used for all indentation tests, as described elsewhere (Dimitriadis et al., 2002). Briefly, the elastic (Young’s) modulus was extracted from a plot of cantilever deflection versus sample (piezo) displacement by fitting the data to a spherical Hertzian contact equation (Sneddon, 1965),

$$(z-z_0)=(d-d_0)+{\left[\frac{0.75k(d-d_0)(1-{\nu }^2)}{E\sqrt{R}}\right]}^{\frac{2}{3}}$$ (1)

where z and d are piezo and deflection coordinates, respectively, z₀ and d₀ are piezo and deflection contact coordinates, respectively, k is the cantilever spring constant, υ is Poisson’s ratio (assumed to be 0.5 for a soft, incompressible material (Boudou et al., 2006)), E is the elastic modulus, and R is the particle probe radius (500 nm). E and z₀ were used as fitting parameters over the first 400 nm — 500 nm of deflection-displacement data.

Spherically tipped cantilevers (1 μm diameter) with nominal spring constants of 0.06 N/m were used in all experiments. Cantilever spring constants were more precisely calibrated by the manufacturer with the Sader method (Sader et al., 2005) prior to use. Indentations were performed at a rate of 2 - 6 μm/sec to probe elastic rather than viscoelastic behavior (Mahaffy et al., 2000), and only the piezo extension data were analyzed to avoid artifacts created by tip adhesion and uncertainty in the contact coordinates. The average maximum force applied to each sample was 3 nN. The moduli of at least four samples of each lot were calculated as an average over 3-10 sites per sample.

Results

AFM was used to obtain deflection-displacement curves on Matrigel at physiological temperatures in an aqueous environment (Fig. 2). Micron sized spherical tips were used because they provide a contact area which is larger than that of conical or pyramidal tips. A larger contact area is desirable because interactions between probe geometry and the nanoscale topography of the sample surface are reduced, and the contact area of a cellular focal adhesions is more closely approximated (Geiger et al., 2001). These factors provide a more biologically relevant measurement.

Deflection-displacement profiles obtained on Matrigel are typical of soft materials, with piezo displacements an order of magnitude larger than resultant cantilever deflections. Results from all samples are summarized in Table 1. The mean elastic modulus for each lot of Matrigel was similar. Lot 1 exhibited an average elastic modulus of 420 Pa ± 280 Pa, while the average elastic modulus of lot 2 was 400 Pa ± 175 Pa. Lot 3 exhibited some variations in modulus within each sample. The majority of the data obtained were consistent with those obtained with each of the other tested lots, with an average modulus of 480 Pa ± 240 Pa. On these samples, however, areas of higher elastic modulus were observed, with modulus values ranging from 1 – 3 kPa. The average modulus value obtained on lot 3 increased to 840 Pa ± 870 Pa when including all data points.

Table 1.

Sample	Elastic modulus, E [Pa]
Lot 1	420 ± 290
Lot 2	400 ± 175
Lot 3	480 ± 240
Lot 3*	840 ± 870
Average (excluding *)	440 ± 250

The overall average, including all three lots, was 443 Pa ± 285 Pa for the elastic modulus of Matrigel (Table 1). Variations in sample moduli are believed to be a result of slight temperature variations among samples and microscale heterogeneities within the material. However, these factors had a marginal impact on calculated moduli, and our method was observed to provide a high degree of reproducibility.

Discussion

Force curves obtained on Matrigel typically exhibited evidence of a small amount of viscoelasticity, with a separation between the approach and retract curves. The viscoelasticity was minimized by choice of a slow indentation rate and was not changed by further decreasing the rate. The approach curve, however, showed a good fit with the Hertz equation and the viscoelasticity was therefore assumed to have a minimal effect on the resulting modulus values. In addition, adhesion of the tip to the sample was occasionally observed. The adhesion may effect modulus measurements, but no difference in modulus was observed between those force curves with and without adhesion, indicating that it is valid to analyze these curves with the Hertz equation.

Although different lots of Matrigel can have varying compositions of biopolymers and protein growth factors, it was unclear if each lot would exhibit identical mechanical properties. Measurements from three separate lots have yielded very similar elastic moduli, with average values of 420 Pa, 400 Pa and 480 Pa, indicating that the mechanical properties are not directly influenced by small changes in Matrigel composition. However, one lot did contain regions of higher elastic moduli. The origin of these areas is unclear, but has been previously attributed to granular regions within the film (Reed et al., 2009).

Previous studies report shear storage modulus values of approximately 55 Pa (Zaman et al., 2006) and 34 Pa (Semler et al., 2000) for Matrigel. Approximating the material as ideally elastic (vanishing shear loss modulus), these elastic moduli are lower than those obtained in our experiments. The above-cited studies employed bulk rheometry, a dynamic mechanical testing technique by which viscoelastic material properties are determined by exposing the specimen to oscillatory shear strains. Discrepancies between rheological and AFM measurements may be due to several factors including length scale differences in test methods, different stress modes (shear versus compression), and temperature conditions. AFM indentation probes highly localized surface properties of a material, and local material properties may differ from those observed with macroscopic test methods. Shear versus compressive forces elicit different modes of mechanical response and may produce anisotropic effects. One report of the elastic modulus of Matrigel with AFM, taken at room temperature, gives a value of 120 Pa, still lower than reported here for samples maintained at 37° C (Alcaraz et al., 2008). Therefore, the most likely explanation for the observed discrepancy is specimen temperature. As observed in our experiments, allowing the specimen temperature to drop below 37° C before or during experimentation resulted in a decrease in sample stiffness. Loss of sample gelation leads to strong probe-surface attraction and adhesion, which made the contact point indiscernible in the deflection-displacement data. Such indiscriminate surface/probe interactions are indicative of a semi-fluid sample surface and rendered indentation tests inconclusive. Therefore we expect mechanical testing of Matrigel without strict temperature control to result in an underestimate of the elastic modulus. Recently, the elastic modulus of Matrigel has been reported after a gelation time of 1 hour to be similar to those reported here, with a median of 650 Pa (Reed et al., 2009), using mechanical interferometry. While it was unclear if the sample temperature was maintained at 37° C, it was stated that the sample chamber was sealed to prevent evaporation.

AFM indentation tests provide experimental conditions that more closely mimic both the length scale and environmental conditions experienced by cells in vivo. The use of micron scale probe geometries, piconewton indentation forces, and aqueous environments at physiological temperatures suggest that AFM is an appropriate instrument for the mechanical characterization of biological materials.

The elastic modulus of Matrigel reported in this study is approximately an order of magnitude lower than determined for cross-linked polyacrylamide gels both prepared in our group and reported (Engler et al., 2004) and poly electrolyte multilayer films (Schneider et al., 2006). These hydrated gels have been used extensively as cell culture substrates because they have tunable mechanical properties, can be cast as thin films, and show a high degree of biological compatibility. This modulus difference may have direct relevance when choosing an appropriate support for the study of cell behaviors. The values obtained are also lower than measurements obtained for native corneal basement membranes (Last et al., 2009).

Conclusions

AFM provides an accurate and reproducible method for characterization of the elastic properties of soft, hydrated, and temperature sensitive materials. However, detailed attention to experimental conditions is necessary for reliable and consistent results. In particular, the delicate temperature sensitivity of Matrigel demands that test samples are kept above 37° C in order to prevent a decrease in sample stiffness. Discrepancies between results reported here and in the literature are believed to be a consequence of different test methods and the tendency of Matrigel to soften at room temperature.