Application of Isotopic Techniques Using Constant Specific Activity or Enrichment to the Study of Carbohydrate Metabolism

Tracer techniques have become an established part of metabolic research and indeed are a fundamental part of our understanding of carbohydrate metabolism in humans. This review is meant to provide a basic overview of the problems and potential pitfalls associated with these techniques in the hope that they are reliably applied to newer areas of research.

A quantitative measurement of the substrate fluxes that determine fasting or postprandial glucose concentrations is central to the determination of the pathophysiology of disease states and of measuring the effect of therapeutic interventions on the disease state. During the fasting state, glucose concentrations are dependent on the rate of endogenous glucose production (EGP) relative to the rate of glucose disappearance; measurement using tracer techniques is quite straightforward. The situation is more complex in the postprandial state, where gastric emptying, glucose absorption, the net sum of splanchnic extraction of ingested glucose (and therefore the rate of systemic appearance of ingested glucose), EGP, and glucose disappearance determine postprandial glucose concentrations (1,2). The tracer-based methodologies used to measure these fluxes, and their potential pitfalls, will be discussed.

Tracers: stable vs. radioisotopes.

The use of a substance (tracer) that can be used to follow a naturally occurring substrate (tracee) requires that such a substance experience the identical metabolic fate as the tracee. The ideal tracer can be detected with such precision as to require administration in trace amounts, thereby avoiding any alteration in the metabolism of the tracee (3). Tracers used for the purpose of metabolic research in humans are usually identical to the tracee, except that one or more atoms differ from the more abundant naturally occurring form of that atom.

Such isotopes are radioactive when they spontaneously disintegrate to form another element, releasing radiation as a by-product of the decay. For example, ³H, which has one proton and two neutrons, emits an electron when decaying to ²He (two protons and one neutron). The energy emitted by such disintegrations is detectable by a scintillation counter. The rate of decay of such isotopes may determine their utility or otherwise in the study of human metabolism; in practice, isotopes with a short half-life are typically not used in metabolic studies except to image metabolic events in specific tissues.

Stable isotopes also differ in the number of neutrons present but do not decay spontaneously and therefore do not emit radiation. Gas chromatography–mass spectrometry is necessary to distinguish such isotopes. To be of use in metabolic research, the natural abundance of a given isotope (and the presence of multiple other isotopes) needs to be low. Other than the absence of radiation exposure associated with the use of stable isotopes, there is another important key distinction from radioisotopes. The presence of naturally occurring stable isotopes within the body prior to tracer infusion means that the tracer (merely) enriches the amount of this isotope. Knowledge of the background abundance of the isotope is necessary to determine the degree of enrichment required.

While scintillation counting is sufficiently sensitive to detect small quantities of radioisotope, permitting infusion of “massless” amounts of tracer, this is not the case with mass spectrometry. The use of stable isotopes generally requires infusion of amounts of labeled compound large enough that the mass of tracer infused is significant and must be accounted for when calculating a metabolic rate using such isotopes.

The assumption that tracers are treated in identical fashion to their naturally occurring counterparts underpins all tracer methodology. However, the presence of radioactive impurities can introduce significant errors into calculations (4,5). This is because a portion of the radioactive material infused is not behaving as a tracer but as a “bystander” playing no role in the reaction rate being measured and with a clearance rate an order of magnitude lower than that of glucose.

The unit of enrichment for a radioactive tracer is specific activity (SA), where:

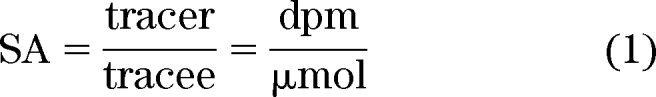Since the tracer is massless, the amount of tracer is represented by disintegrations per minute (dpm). In contrast, due to the fact that stable isotopes are not massless and because stable isotopes require higher enrichment to be accurately measured, their enrichment is:

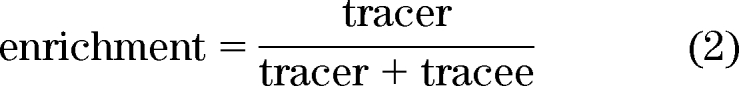Commonly this is expressed as atom percent excess (APE) to account for the presence of naturally occurring stable isotopes:

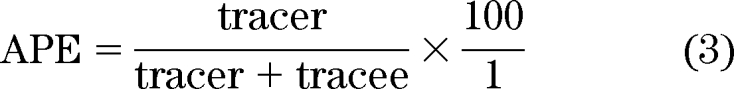There is concern that the degree of enrichment necessary with stable isotopes can induce side effects or directly affect metabolism. To some extent, the variability in measurement of isotopes seems to be greater than any isotope effect (3). However, a recent study using a variant of the deuterated water method suggested that the deuterium on C5 of glucose is lost more rapidly relative to the deuterium on C3 (6).

The Steele equation.

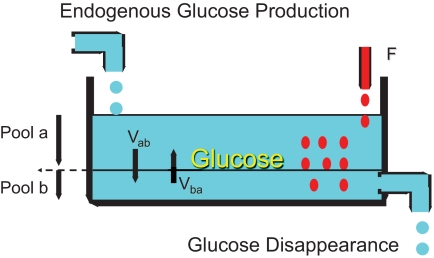
At the simplest level, glucose metabolism in the fasting state can be reduced to a single compartment represented by a bathtub where water enters via a faucet (EGP) and leaves via the drain (glucose disappearance [Rd]). The water level rises if EGP > Rd, falls if Rd > EGP, and is constant when EGP = Rd, as happens during fasting or in a clamp when peripheral glucose concentrations are kept constant. If a dye could be added to the water in such a way that it is instantaneously and uniformly distributed throughout the bathtub, subsequent water loss via the drain will not change the resulting dye concentration in the bathtub. In contrast, water entering from the faucet has no dye and over time will dilute the dye concentration. This underlies the basis of the tracer dilution technique, commonly used to measure EGP where unlabeled glucose dilutes the tracer.

In fact, tracers used to measure metabolic fluxes are usually infused at a (constant) rate so that after a period of time the tracer will be removed at the same rate that it appears. The time to reach that equilibration period (assuming a one-compartment system with constant tracer infusion) depends on the tracer's infusion rate relative to the tracer's clearance (removal from the pool). Assuming that the tracer and tracee are cleared at the same rate (i.e., isotopic discrimination does not occur), the concentration of tracer and tracee in the body pool will reflect their respective rates of infusion/appearance (7,8) (Fig. 1).

FIG. 1.

Schematic diagram illustrating the multiple-pool concept in tracer dilution studies. Pool a represents the readily accessible pool in which tracer distributes rapidly, in contrast to the less readily accessible pool (Pool b). The relative size of each pool can be altered by experimental (or physiological) conditions, as can the flow of tracer (and tracee) from Pool a to b (V_ab) and vice versa (V_ba). F, tracer infusion rate.

As a method for measuring EGP, the model described could feasibly be used to measure EGP assuming that a human behaves like a single-compartment pool, where tracer and glucose are uniformly distributed. However, in most experimental situations, where the steady state is perturbed by a sudden influx of glucose and the secretion of insulin changes the rate of EGP and glucose efflux, these assumptions do not hold because glucose is not entering a uniform pool—the sampled pool does not necessarily reflect the pool into which glucose initially appears. Glucose crosses (and is extracted by) multiple compartments before entering the circulating glucose pool, which is the pool usually sampled.

However, let us persist for a while with the single-compartment bathtub analogy before progressing to different situations. The equation that is most relevant to simple measures of glucose flux is:

where Ra is rate of appearance and F is rate of tracer infusion. Solving for SA:

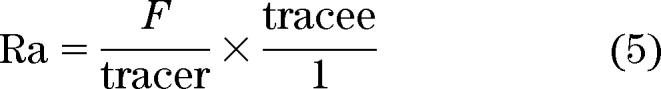Steady state vs. non–steady state and Steele volume.

In situations where the relative concentrations of tracer and tracee are stable, Ra can be calculated accurately. This is because the assumption that a tracer is well mixed with the tracee holds (8) and once the tracer (and tracee) has left the pool, it does not re-enter.

A perturbation to the steady state, e.g., a (sudden) decrease in EGP caused by insulin injection, will decrease dilution of the tracer by cold glucose. The consequent rapid changes in SA decrease the accuracy with which glucose flux can be calculated (9).

To account for the sudden changes in both tracer and tracee concentrations, the equation can be modified to account for these changes over time (dSA/dT). However, because glucose is not distributed in a single pool, the prior assumptions of uniform mixing and a lack of reentry into the pool being sampled may not hold. Therefore, it is reasonable to postulate the presence of at least two pools: a rapidly equilibrating pool and a slow(er)-equilibrating pool (10,11). The complexities of a multiple-pool system can be simplified to that of a single-compartment system by using a pool fraction (p) of the total extracellular glucose pool (V_total) − the Steele volume (V_Steele), e.g., 0.65 of 200 ml/kg. In such circumstances the non–steady-state correction at two given time points T₁ and T₂ is:

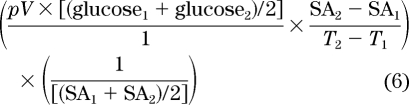The potential problem with this approach is that the size of the pool is probably not constant and changes with time after a given perturbation (12). The same applies to the equilibration rate of tracer between compartments (10). Furthermore, disease states may alter the magnitude of differences between compartments, introducing new errors into the calculation of glucose flux. In such situations, calculation of glucose fluxes (see below) may produce aberrant results, e.g., negative values for EGP. Such errors can be minimized by using an experimental design where perturbations in SA are minimized and frequent sampling occurs (so that these perturbations in SA are very small quantities) or by fitting the data to minimize changes in SA (13).

An alternative approach to the assumption of pV is to measure this volume during the experiment. Issekutz et al. (14) proposed a method in which a (separately) infused isotope of glucose is used to trace the Ra of a second isotope of glucose mixed with exogenously infused glucose. Since the infusion rate and therefore the Ra of both isotopes into the systemic circulation is known, pV can be measured. This methodology has been compared with the non–steady-state calculation when using a variation of the hot-GINF technique (15) (outlined below) and yields similar results (16,17).

Measurement of glucose turnover using a clamp.

A euglycemic-hyperinsulinemic clamp is a straightforward experimental design that approximates the bathtub example used above to describe glucose metabolism. The design is amply suited to measurement of glucose turnover using a single tracer because by design a steady state is reached after a prolonged period of time where the Ra is equal to the Rd and the SA is relatively constant. For example, using 3H³-glucose as the tracer:

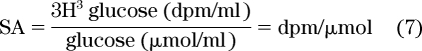Therefore,

where F = rate of tracer infusion (dpm/min).

Provided the tracer is infused for an adequate amount of time, baseline EGP can be calculated in the same fashion. However, if glucose turnover is to be measured at multiple time points with multiple perturbations, e.g., to establish a dose-response curve for insulin, it is best to design an experiment that avoids large changes in the SA, thereby minimizing the need to make assumptions regarding the structure or degree of mixing of accessible and remote glucose pools (13,18).

Changes in SA can be minimized by infusing glucose that contains tracer in amounts that approximate the baseline plasma glucose SA (16,19). A primed, continuous infusion of tracer is initiated and continued till the end of the study. Provided a suitable equilibration period elapses, such an infusion can readily be used to measure fasting EGP as described above. Subsequent to the infusion of insulin and intravenous glucose, the rate of infusion of this tracer is decreased to approximate the anticipated fall in EGP, thereby keeping the ratio of tracer to tracee constant (13,19).

Anticipation of the expected fall in EGP requires a priori knowledge of the degree and pattern of change in this parameter. In one of the examples cited (19), the basal infusion of [3-³H]glucose was altered so as to approximate the anticipated pattern of fall of EGP (0 to 10 min, 90%; 10 to 20 min, 80%; 20 to 30 min, 65%; 30 to 40 min, 55%; 40 to 50 min, 45%; 50 to 75 min, 35%; 75 to 120 min, 40%; 120 to 300 min, 45%; 300 to 360 min, 50%). The necessary information to generate such general patterns can be obtained from other experiments or pilot studies. Error is reduced by more frequent sampling during the 60–90 min subsequent to such a perturbation. The success or otherwise of such a technique can be measured in the degree of perturbation observed in SA and the basal infusion rates adjusted prior to conducting the experiment(s).

The infusion of intravenous glucose delivers “hot” and “cold” glucose-minimizing perturbations in SA. In such circumstances:

Therefore, since

Assuming SA can be kept relatively constant over time, thereby minimizing the non–steady-state correction, such a technique can be effectively used in experiments mimicking the systemic appearance of orally ingested glucose (Fig. 2).

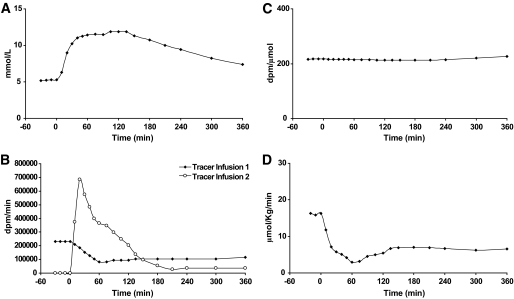
FIG. 2.

An experiment using intravenous infusion of glucose to mimic the systemic appearance of oral glucose (A). The tracer [3-³H]glucose is infused via two separate infusions (B), so that tracer infusion 1 mimics the expected decline in EGP. Tracer infusion 2 reflects the glucose infusion replicating systemic appearance of oral glucose. Despite large, rapid changes in glucose concentration, the SA is relatively constant over time (C), enabling accurate measurement of EGP (D).

An issue that arises when reporting flux is that of data normalization so as to allow interindividual comparison of results. The approach most often adopted is to express turnover per unit of lean body mass or unit of surface area. This needs to be borne in mind when comparing results between different investigators.

So far we have considered perturbations to SA that arise from meal ingestion. However, it is important to appreciate that sudden increases in physical activity can perturb SA by causing an increase in glucose disappearance. Assuming that the tracer infusion rate does not change in anticipation, SA will fall leading to an overestimation in the flux being measured.

Measurement of splanchnic glucose uptake: dual-tracer methodology.

The dual-isotope method was developed in an effort to simultaneously measure both the systemic Ra of ingested glucose (Ra_meal) and postprandial EGP (20). This method uses an infused and an ingested tracer to trace glucose. The appearance of ingested glucose is calculated by multiplying the Ra of the ingested tracer by the tracer-to-tracee ratio of the ingested meal. Initial splanchnic glucose uptake can be calculated by subtracting the portion of the ingested glucose that reaches the circulation from the total amount of glucose ingested. Endogenous glucose production is calculated by subtracting Ra_meal from the total glucose appearance.

This experimental approach has been used by a large number of investigators to study postprandial glucose metabolism in a wide variety of species. However, results have not always been consistent. Estimates of initial splanchnic glucose uptake by this method have varied widely, as has the pattern of EGP, ranging from rapid near-complete suppression to an initial paradoxical rise followed by a subsequent fall in EGP (21). These discrepancies are likely to arise from inadequacies of the model used to calculate turnover in the rapidly changing conditions occurring after carbohydrate ingestion. As previously reviewed, estimates of initial splanchnic glucose with this technique vary between ∼0 and 30% (21).

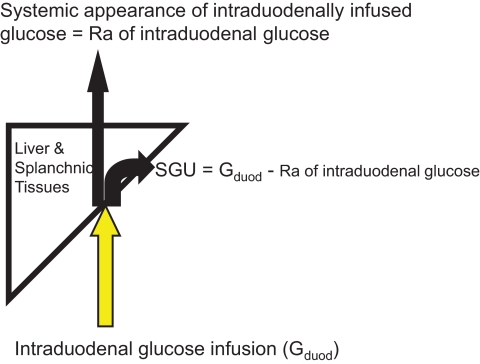
A partial solution to these problems is to use an intraduodenal infusion of labeled glucose whose Ra can be determined by a systemic tracer (22–24) (Fig. 3). In such experiments, 3H³-glucose was infused intraduodenally while [6,6-²H₂]-glucose was used as the systemic tracer. Once steady state is achieved, Ra and Rd is calculated using a derivation of the Steele steady-state equation:

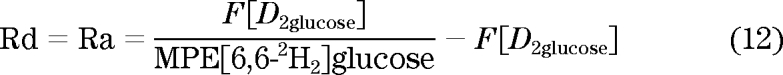where F[D_2glucose] is the [6,6-²H₂] tracer infusion rate and MPE [6,6-²H₂]glucose is the plasma [6,6-²H₂]glucose enrichment. Note that the calculation must account for the fact that the tracer used is not massless, as would have been the case for a radioisotope. The systemic Ra of the intraduodenally infused 3H³-glucose can be calculated as:

where [6,6-²H₂]glucose is the concentration (in μmol/ml) of [6,6-²H₂]glucose in plasma and [3-³H]glucose is the concentration (in dpm/ml) of [3-³H]glucose in the plasma. The systemic Ra of the intraduodenally infused glucose can subsequently be calculated as:

where SA_duod glucose is SA (in dpm/μmol) of the glucose infused intraduodenally. Initial splanchnic glucose uptake (SGU) can subsequently be calculated:

where G_duod is the intraduodenal infusion rate of glucose. EGP is calculated by subtracting the sum of the intravenous glucose infusion rate (F[D_2glucose]) and the systemic Ra of intraduodenally infused glucose from total glucose appearance.

FIG. 3.

Methodology for the determination of splanchnic glucose uptake (SGU) using a nasojejunal tube.

While this methodology is relatively simple, it has three problems: It cannot distinguish between the intestinal and hepatic contribution(s) to SGU. Furthermore, it requires the cumbersome and invasive placement of a nasoduodenal feeding tube, and it does not replicate postprandial conditions.

Glucose turnover in meal studies: triple-tracer methodology.

The inadequacies of the dual-tracer method for meal studies arise in part because a one-compartment model cannot describe the non–steady-state conditions that occur after meal ingestion. In addition, a fixed pool fraction cannot account for incomplete mixing within the compartment, and indeed the “correct” value is uncertain and may vary with time (16,21,25,26). The presence of concentration gradients within the glucose pool results in errors that may depend on disease state (diabetic vs. nondiabetic) and methodology (meal size, rapidity of ingestion/appearance, etc.). Such errors are not necessarily overcome with multiple-compartment models (27,28).

Methodology that can ensure a relatively constant tracer-to-tracee ratio overcomes these problems when measuring glucose turnover using a clamp (see above). Therefore, in an effort to overcome the marked changes in glucose and tracer concentrations that occur after meal ingestion using a dual-tracer approach, the addition of a third tracer was conceived as a refinement of the dual-tracer method. For example, with this design the meal glucose is labeled with a tracer (e.g., [1-¹³C]-glucose), while a second tracer (e.g., [6,³H₂]-glucose) is infused intravenously in a manner anticipated to mimic the systemic appearance of meal-derived glucose.

In this way, the ratio of [6-³H] glucose to [1-¹³C] glucose and of [6,6-²H₂]glucose to endogenous glucose (the portion of glucose derived from EGP) remain relatively unchanged. As before, changes in the ratio between [6,6-²H₂]glucose tracer and endogenous glucose are minimized by infusing [6,6-²H₂]glucose in a pattern that mimics the anticipated pattern of change of EGP. This approach is virtually model independent, yielding essentially the same results when interpreted using steady-state or non–steady-state assumptions and either a one- or two-compartment model. When compared with a dual-tracer approach, minimizing change in the plasma tracer-to-tracee ratios, accurate measurement of the factors involved in the regulation of postprandial glucose tolerance is possible (27).

In this experimental design, [6-³H] glucose is used to trace the systemic appearance of [1-¹³C] glucose. Therefore:

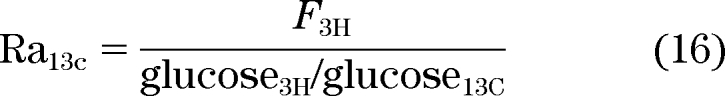Consequently,

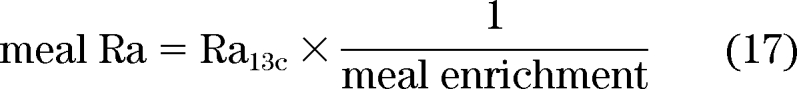where $\frac{1}{\text{meal enrichment}}$ is the ratio of total glucose (tracer + tracee) to tracer in the meal. To calculate EGP, consider that total plasma glucose concentration is the sum of tracee plus two tracers ([6-³H]glucose being massless):

Since G_13C and G_2H can be quantified, the equation can be solved for G_natural. Subsequently, the concentration of glucose derived from EGP can be calculated by subtracting the contribution of ingested natural glucose from G_natural.

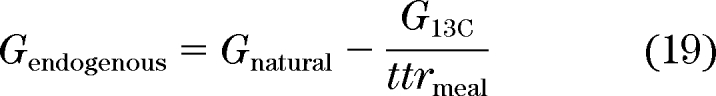where ttr_meal is the ratio of tracer to tracee in the meal.

EGP is then calculated as:

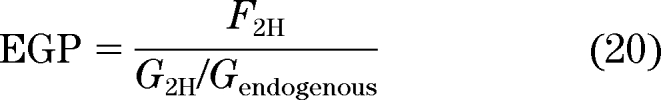where F_2H is the infusion rate of [6,6-²H₂]-glucose. Finally:

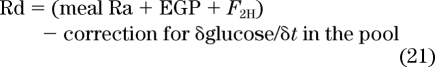Therefore:

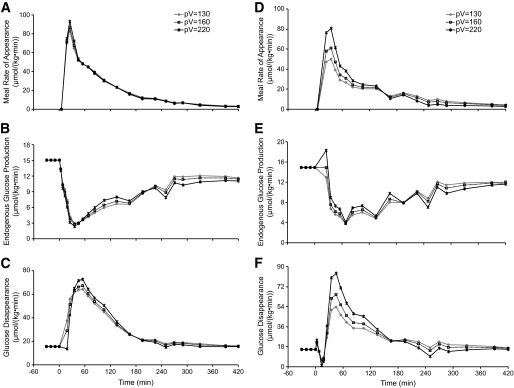
where pV is V_Steele as discussed above and G is glucose concentration. This final calculation depends on multiple assumptions known to be incorrect (see discussion above). However, calculation of Rd using the triple-tracer approach is little affected by different estimates of pool size or a single- versus dual-compartment approach, underlining the model independence of this methodology (Fig. 4).

FIG. 4.

Calculation of the systemic appearance of meal-derived glucose (A), EGP (B), and glucose disappearance (C) using the triple-tracer technique and a one-compartment model. Note that changing the estimates of the pool fraction (pV) has little effect on calculated rates. In contrast, using a dual-tracer approach, changing estimates of pV have marked effects on meal appearance (D), EGP (E), and glucose disappearance (F). Modified and reprinted with permission from Basu et al. Am J Physiol Endocrinol Metab 2003;284:E55–E69.

Tracer choice and its effects on measurement of glucose flux.

An important assumption underling tracer methodology is that the label used in a given tracer does not recycle into the compartment being studied after it leaves the compartment with the tracee. If this is the case, Ra will be progressively underestimated as more and more isotope recycles back into the pool being measured. Glucose tracers typically use a hydrogen isotope on the 2nd, 3rd, or 6th carbon. The label is lost to water at different points during glycolysis or gluconeogenesis, leading to potential differences in Ra measurement depending on the tracer used (3,29).

In the case of glucose labeled on the 2nd carbon, the isotope will be lost in the conversion of glucose-6-phosphate to fructose-6-phosphate (assuming that the isomerase reaction is much more active compared with the formation of glucose-6-phosphate) and reconversion to glucose-6-phosphate. This results in overestimation of glucose utilization (4,30). Since the glucose/glucose-6-phosphate cycle results in hydrolysis of ATP but without glucose metabolism, this has been termed futile cycling (31).

On the other hand, label is lost from the 3rd carbon after cleavage of fructose-6-phosphate to dihydroxyacetone phosphate (DHAP) and glyceraldehyde-3-phosphate (G-3P). The label is present on the 1st carbon of DHAP but is then lost after isomerization to G-3P.

In the case of glucose labeled on the 6th carbon, label is lost from the 6th carbon after entry into the tricarboxylic acid cycle. The action of uridine-diphosphoglucose dehydrogenase causes loss of tritium from carbon 6 when uracil-diphosphate (UDP)-glucose reenters glucose metabolism (32). Another potential mechanism by which the label on the 6th carbon is lost is when glucose is metabolized to fructose biphosphate and then recycles back to glucose. A variety of (in vitro) studies have demonstrated that futile cycling can occur at the fructose-6-phosphate/fructose diphosphate level (33–35). However, in vivo studies suggest that in postabsorptive humans, futile cycling at this level is insignificant (36,37). The use of 6,6-²H₂-glucose as a tracer overcomes some of these problems, as the 6-²H₁-glucose product of recycling can be distinguished from the underlying tracer (38).

There is no net loss of carbon in glycolysis, and therefore the use of a carbon-labeled tracer in measuring glucose metabolism avoids some of the potential pitfalls associated with the use of hydrogen labeling. Generally, 1-¹³C-glucose, 6-¹³C-glucose, or universally labeled glucose (U-¹³C-glucose) is used. Use of the latter tracer decreases potential problems associated with the other tracers of recycling label that underestimate glucose production (39). This assumes that the likelihood of two triply labeled 3-carbon precursors (re-)combining to form U-¹³C-glucose during gluconeogenesis is extremely small. Such potential pitfalls and the likely presence of such cycling during the study conditions need to be considered when choosing a tracer for a given study (40,41).

Conclusions.

The evolution of tracer techniques enabled an understanding of basic physiologic processes in free-living organisms. Established techniques such as those outlined above are routinely used to measure carbohydrate metabolism and subsequent changes in response to a given intervention. Newer methodologies, while more esoteric and perhaps less widely applicable, nevertheless depend on the same principles outlined in this review. Ultimately, the aphorism that to detect a change one has to be able to measure it applies to metabolism too—we hope that properly applied tracer methodology will continue to drive innovative metabolic research in the near and distant future.