Skip to main content
PMC home page
NIHPA Author Manuscripts logoLink to NIHPA Author Manuscripts
. Author manuscript; available in PMC: 2010 Aug 25.
Published in final edited form as: J Biomech. 2009 Jun 18;42(12):1941–1946. doi: 10.1016/j.jbiomech.2009.05.008

Reduced Nucleus Pulposus Glycosaminoglycan Content Alters Intervertebral Disc Dynamic Viscoelastic Mechanics

John I Boxberger 1, Amy S Orlansky 1, Sounok Sen 1, Dawn M Elliott 1
PMCID: PMC2756063  NIHMSID: NIHMS119206  PMID: 19539936

Abstract

The intervertebral disc functions over a range of dynamic loading regimes including axial loads applied across a spectrum of frequencies at varying compressive loads. Biochemical changes occurring in early degeneration, including reduced nucleus pulposus glycosaminoglycan content, may alter disc mechanical behavior and thus may contribute to the progression of degeneration. The objective of this study was to determine disc dynamic viscoelastic properties under several equilibrium loads and loading frequencies, and further, to determine how reduced nucleus glycosaminglycan content alters dynamic mechanics. We hypothesized (1) that dynamic stiffness would be elevated with increasing equilibrium load and increasing frequency, (2) that the disc would behave more elastically at higher frequencies, and finally, (3) that dynamic stiffness would be reduced at low equilibrium loads under all frequencies due to nucleus glycosaminoglycan loss. We mechanically tested control and chondroitinase-ABC injected rat lumbar motion segments at several equilibrium loads using oscillatory loading at frequencies ranging from 0.05 to 5 Hz. The rat lumbar disc behaved non-linearly with higher dynamic stiffness at elevated compressive loads irrespective of frequency. Phase angle was not affected by equilibrium load, although it decreased as frequency was increased. Reduced glycosaminoglycan decreased dynamic stiffness at low loads but not at high equilibrium loads and led to increased phase angle at all loads and frequencies. The findings of this study demonstrate the effect of equilibrium load and loading frequencies on dynamic disc mechanics and indicate possible mechanical mechanisms through which disc degeneration can progress.

Introduction

The intervertebral disc provides load support, permits motion, and dissipates energy during normal daily activity, functioning over a range of loading regimes including axial loads across a spectrum of frequencies at varying states of compressive equilibrium (Andersson et al., 1978; Wilke et al., 1999). Under extreme loading conditions matrix damage may accumulate compromising disc integrity (Adams and Hutton, 1983; Adams and Hutton, 1985; Gordon et al., 1991). Microdamage including annulus fibrosus delamination occurs under aberrant strains and may permanently alter disc mechanics (Iatridis et al., 2005). While disc cells are capable of remodeling the damaged tissue to some extent, during degeneration progression mechanically induced damage accumulation may exceed repair. Certain mechanical conditions may exacerbate damage accumulation in a relatively healthy and asymptomatic disc, and biochemical changes occurring in early degeneration may further elevate susceptibility to mechanically induced damage and progression of degeneration.

Among the earliest changes in disc degeneration is a reduction of nucleus pulposus glycosaminoglycan (GAG) content (Pearce et al., 1987; Antoniou et al., 1996; Roughley et al., 2002). This loss directly impacts the mechanical function of the nucleus (Iatridis et al., 1998; Johannessen and Elliott, 2005; Perie et al., 2006) and the motion segment (Boxberger et al., 2006; Johannessen et al., 2006; Cannella et al., 2008). Additionally, alterations in disc viscoelasticity occurring during degeneration, including increased creep and creep rate (Kazarian, 1975; Keller et al., 1987), can be partly attributed to reduced nucleus GAG (Keller et al., 1988). Increased hypermobility and creep may contribute to mechanical progression of degeneration as the resulting alterations in local stress and strain lead to damage accumulation. While this is plausible, it remains unknown how reduced nucleus GAG alters disc mechanics under dynamic loading, as the available data are limited to quasi-static conditions. Furthering the understanding of the dynamic mechanical behavior of both healthy intervertebral discs and those with reduced nucleus GAG will lend insight into the mechanical progression in disc degeneration.

Disc mechanical behavior may be differentially altered under various applied loads and frequencies, and thus, the objective of this study was to determine disc viscoelastic properties in axial loading over varying equilibrium loads and frequencies. Further, this study sought to determine how reduced nucleus GAG altered the mechanical behavior compared to healthy discs at these equilibrium load and frequency combinations. To complete our objectives, we dynamically tested rat lumbar discs, which offer mechanical and biochemical properties consistent with human discs (Elliott and Sarver, 2004; Beckstein et al., 2008) and provide a platform to controllably alter nucleus GAG with chondroitinase ABC (ChABC) (Boxberger et al., 2006; Boxberger et al., 2008). Discs were tested dynamically at incrementally increasing compressive equilibrium loads and analyzed using linear dynamic viscoelastic theory. We hypothesized that dynamic stiffness would be elevated both with increasing equilibrium load and with increasing frequency, and further, that the disc would behave more elastically with increasing frequency. As a result of nucleus GAG loss, we hypothesized that disc stiffness would be reduced at low loads.

Materials and Methods

Sample Preparation

Sixteen motion segments from L2L3 to L5L6 were selected at random from 6 male retired breeder Sprague Dawley rats (age 7-9 months, weight 450-500g), with remaining levels utilized in other studies. Following euthanasia, lumbar spines were extracted. Transverse processes, posterior elements, and all musculature and ligaments were removed. Individual bone-disc-bone motion segments were isolated by making parallel axial cuts through each vertebral body.

Treatment Groups

Motion segments were randomly assigned to either a control or treatment group (n=8 per group). One μL containing 0.00025 units of ChABC (Seigaku Corp., Tokyo, Japan) in phosphate buffered saline (PBS) (Boxberger et al., 2006; Boxberger et al., 2008) was injected into the nucleus of discs assigned to the treatment group to reduce nucleus GAG by levels consistent with early degenerative changes in the human (Antoniou et al., 1996). Injections were administered using a gas tight microsyringe (Hamilton Co., Reno, NV) with a custom 33 gauge needle inserted anteriorly into the disc to a depth of 2.5 mm. Control discs recieved no needle insertion or injection. All discs, including the controls, were kept at 37°C in a PBS bath for 18 hours allowing for enzyme activity and then frozen at -20°C until mechanical testing.

Mechanical Testing

Each motion segment was thawed in room temperature PBS for 1 hour. Vertebral bodies were gripped in microvises attached to an Instron 5848 (Instron, Canton, MA) with testing performed in room temperature PBS. Twenty preconditioning cycles from 4.5 N compression (∼1× body weight) to 3 N tension were applied at 0.1 Hz. The dynamic loading protocol (Figure 1) was then run. Discs were held at 0 N applied load for 30 minutes (equilibrium load A). Displacement was zeroed after the hold, and 5 cycles of a sinusoidal waveform with amplitude ±0.005 mm were applied across a spectrum of frequencies: 0.05, 0.1, 0.2, 0.5, 1, 2, and 5 Hz. After the final cycle, a step displacement was applied with a stop criterion of 1 N compression. Upon reaching 1 N, displacement was fixed and load relaxed for 30 minutes (equilibrium load B). The frequency sweep with amplitude ± 0.005 mm was again applied. This sequence of step displacement followed by load relaxation to equilibrium and frequency sweep was repeated at step displacements corresponding to 4.5 N (equilibrium load C) and 9N (equilibrium load D). Samples were then rehydrated for 1 hour at room temperature and refroze at -20° C until biochemical analysis.

Figure 1.

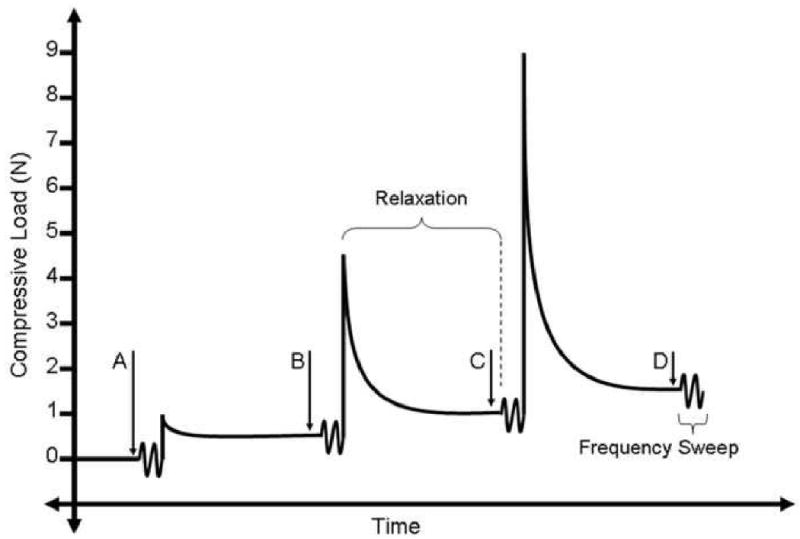
Figure 1

Open in a new tab

Dynamic compressive testing with amplitude +/- 0.005 mm was performed at frequencies ranging from 0.05 to 5 Hz at four different equilibrium loads (A, B, C, D). In order to reach each state, a step load was applied to the motion segment followed by load relaxation at fixed displacement.

The final cycle at each equilibrium load and frequency combination was analyzed with linear dynamic visocelastic theory (Lakes, 1999). At each frequency, force-time and displacement-time data were curvefit to 4 parameter sinusoidal functions (Equations 1-2) using least squares in MATLAB (The Mathworks, Inc., Natick, MA). The parameters L, d, and t represent measured force (N), displacement (mm), and time (sec), respectively. The following parameters were then obtained from the curvefit: L0 and d0 are load (N) and displacement (mm) amplitudes, ω is frequency (rad/sec), θL and θd are phase terms (rad), and Li and di are equilibrium load (N) and displacement (mm).

d=d0⋅sin(ωt+θd)+di (Equation 1)
L=L0⋅sin(ωt+θL)+Li (Equation 2)

Dynamic stiffness (K*, N/mm) was calculated as K∗=L0d0 and phase angle (δ, deg) as δ=θL−θiπ×180°. Goodness of fit of the displacement-time and load-time response with the sine wave model was determined using a Bland-Altman analysis (Bland and Altman, 1986).

Biochemical Analysis

Each disc was excised from the frozen motion segment and placed on a freezing stage. Each complete nucleus pulposus was removed using a 1.5 mm diameter punch and dried at 65° C for 24 hours. Each nucleus was then digested for 18 hours in 2.5 mg/mL proteinase K solution at 65° C. Finally, nucleus GAG content was quantified using the 1-9 dimethylmethylene blue assay (Farndale et al., 1986).

Statistics

A two factor ANOVA was performed on the control disc data to determine the effects of factors equilibrium load and frequency on disc mechanical properties. Next, Student's t-tests comparing the ChABC treatment group versus control were performed evaluating mechanical parameters at each equilibrium load and frequency combination. Significance was set a p < 0.05. Trends were defined as 0.05 ≤ p < 0.1.

Results

Linear Viscoelastic Model

Displacement-time and load-time responses were well described by the sine wave model. The bias of the displacement fits had a mean value of -0.08% while the bias of the load fits was 0.91%. 95% limits of agreement (representative of ±2 standard deviations of the residuals) were ±3.19% for the displacement fits and ±3.86% for the load fits. This data combined with observed elliptical load-displacement responses (Figure 2) confirmed the deformation amplitude utilized was sufficiently small to allow for piece-wise linearity and appropriate usage of linear dynamic viscoelastic theory.

Figure 2.

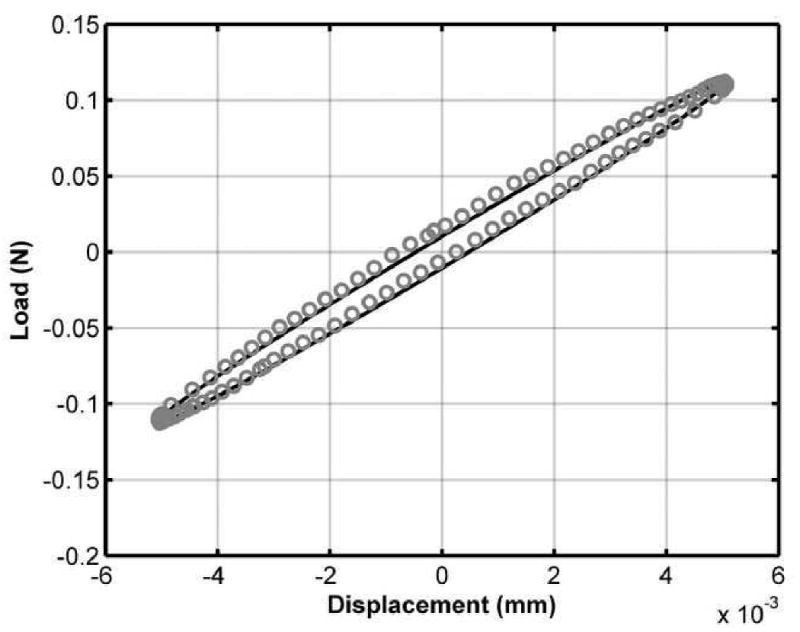
Figure 2

Open in a new tab

An elliptical load versus displacement response demonstrates the applicability of linear viscoelastic theory. Deviations from the elliptical path would indicate non-linearity.

Equilibrium Load and Frequency

Equilibrium load A was at 0 N and 0 mm displacement. Equilibrium load B was 0.35 ± 0.09 N (65% load relaxation from applied 1N) at displacement 0.046 ± 0.033 mm. Equilibrium load C was 0.98 ± 0.33 N (78% load relaxation from applied 4.5 N) at displacement 0.152 ± 0.078 mm. Equilibrium load D was 1.58 ± 0.52 N (82% load relaxation from applied 9 N) at displacement 0.312 ± 0.114 mm. No differences existed in step displacements or equilibrium loads between controls and ChABC treated discs, thus the preceding data represent combined values.

Equilibrium load significantly affected dynamic stiffness (p<0.05) while frequency had no significant effect (p>0.1), as shown in Figure 3. As equilibrium load increased, dynamic stiffness of controls increased from 31.52 ± 9.41 N/mm at equilibrium load A to 64.77 ± 17.70 N/mm at equilibrium load D (averaged across all frequencies). In contrast, phase angle was unaffected by compressive equilibrium load (p>0.1) but significantly decreased with increasing frequency (p<0.05) (Figure 4), ranging from 5.7 ± 1.7° at 0.05 Hz to 1.7 ± 1.3° at 5 Hz (averaged across all equilibrium loads). No significant interactions were detected (p>0.1) between equilibrium load and frequency.

Figure 3.

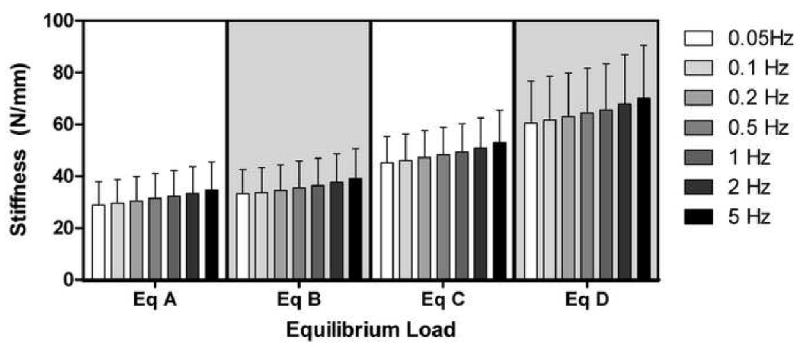
Figure 3

Open in a new tab

Intervertebral disc dynamic stiffness increased as equilibrium load was increased (control data shown) from equilibrium state A to D (x-axis). Dynamic stiffness was not significantly altered by frequency (color shades), though mean values tended to rise in magnitude with increasing frequency.

Figure 4.

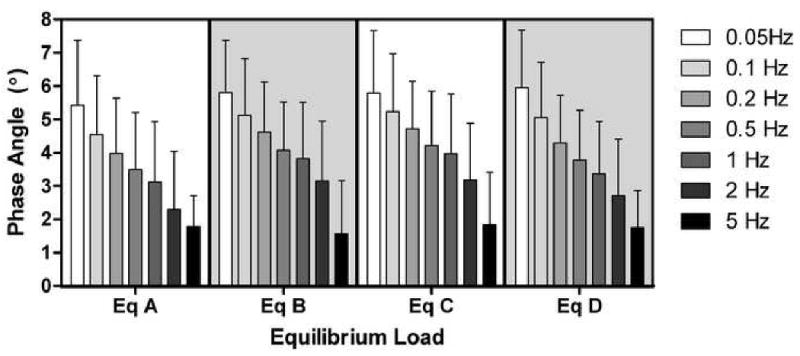
Figure 4

Open in a new tab

Phase angle was not significantly altered with increasing equilibrium load (control data shown) from state A to state D (x-axis); however, phase angle was significantly decreased with increasing frequency (color shades).

ChABC Treatment

Nucleus pulposus GAG content of the ChABC treated group (24.3 ± 7.4 μg) was 33% lower than control (36.2 ± 16.6 μg). Values normalized by tissue weight are presented in table 1. As dynamic stiffness values were independent of frequency in both control discs and ChABC discs (not shown), values were averaged across frequencies at each equilibrium load for comparison between treatments. Dynamic stiffness (Figure 5) in ChABC treated discs was 39% lower than control (p<0.05) at equilibrium load A, 35% lower (p<0.05) at equilibrium load B, and 21% lower at equilibrium load C (trend, p<0.1). At the highest equilibrium load, D, no difference in dynamic stiffness between control and ChABC treated discs was detected (p>0.1). Phase angle did not vary with equilibrium load; thus, comparisons between control and ChABC treated discs were made at each frequency using mean values across all equilibrium loads. Phase angle was 1.8 ± 0.3° higher in ChABC discs compared to control over all frequencies; a difference which was either statistically significant (p<0.05) or a trend (p<0.1) at all frequencies (Figure 6).

Table 1.

Normalized nucleus GAG values (mean ± standard deviation)

Control ChABC
GAG/wet weight (μg/mg) 14.7 ± 5.0 10.3 ± 4.1
GAG/dry weight (μg/mg) 92.2 ± 65.1 70.5 ± 39.3
Open in a new tab

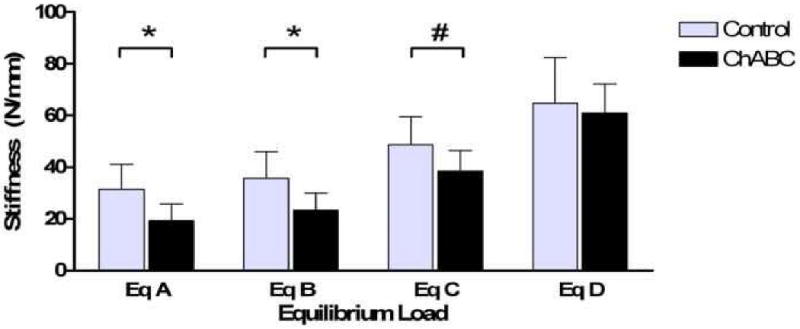
Figure 5.

Figure 5

Open in a new tab

Reduction in nucleus GAG content by ChABC injection led to a decrease in motion segment dynamic stiffness at low equilibrium loads, though had no significant effect at high loads. Dynamic stiffness was not significantly altered by frequency, so data presented are averaged across all frequencies within a particular equilibrium load state. (‘*’ = p<0.05; ‘#’ = 0.05≤p<0.1)

Figure 6.

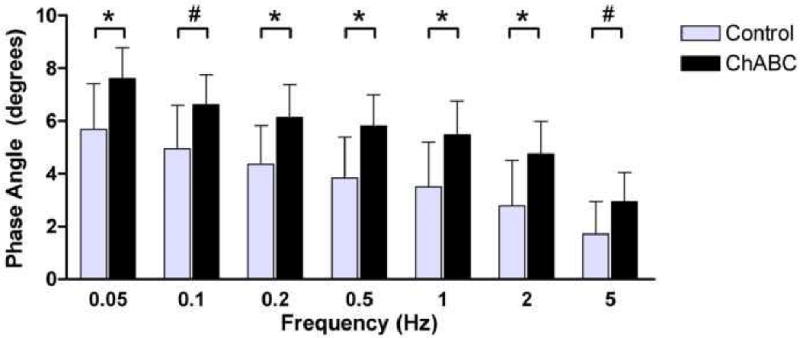
Figure 6

Open in a new tab

Reduction in nucleus GAG content by ChABC injection led to an increase in phase angle at all frequencies examined. Phase angle was not significantly altered by equilibrium load, so data presented are averaged across all equilibrium loads within a particular frequency. (‘*’ = p<0.05; ‘#’ = 0.05≤p<0.1)

Discussion

The intervertebral disc experiences a wide range of applied loads across a range of frequencies and experiences varying equilibrium states (Andersson et al., 1978; Wilke et al., 1999). In order to better comprehend mechanical contributions to degeneration progression, understanding of disc mechanics at these equilibrium loads and applied frequencies is needed. The present study 1) quantified the dynamic viscoelastic behavior (dynamic stiffness and phase angle) of the intervertebral disc over a range of compressive equilibrium loads and frequencies likely to be encountered in regular activities, and 2) determined how the early degenerative change of reduced nucleus GAG affected dynamic mechanics.

The rat lumbar disc behaved non-linearly, demonstrating higher dynamic stiffness at elevated compressive loads irrespective of frequency, consistent with load dependent changes reported in previous dynamic loading studies in porcine lumbar and bovine tail motion segments (Kaigle et al., 1998; Race et al., 2000; Korecki et al., 2008). Though the motion segment is nonlinear, behavior was piece-wise linear under the small oscillatory deformations applied, making the linear viscoelastic model appropriate. Similar to the dynamic properties of the medial collateral ligament at multiple equilibrium strains (Bonifasi-Lista et al., 2005), the data interpretation extends from the linear to non-linear case. The mechanism for load-dependence of dynamic stiffness is likely the non-linearity of the annulus fibrosus in circumferential tension, which is well established under quasi-static (Galante, 1967; Acaroglu et al., 1995) and dynamic loading (Sen et al., 2008) and closely matches the motion segment nonlinearity observed here. In going from zero load to the highest compressive equilibrium the increasingly pressurized nucleus pulposus transmits loads radially to the confining annulus fibrosus. The annulus resists deformation through generation of tensile hoop stresses which show significant load dependence.

The dynamic stiffness did not depend on frequency of loading. This was unexpected, as Costi et al. (2008) demonstrated a significant 19% increase in compressive stiffness over a similar two decades of frequency (0.01 Hz to 1 Hz) in the human disc, and the dynamic tensile stiffness of annulus fibrosus correlates with increasing frequency (Sen et al., 2008). Examination of mean values in the current study revealed a non-significantly (p=0.1) 18% greater dynamic stiffness at 5 Hz than at 0.05 Hz, potentially implicating a type II statistical error. Differences may also be attributed to differing loading rates implemented due to varying disc size between human and rat. It is evident, however, that varying frequency by the two decades here had less of an impact on dynamic stiffness than varying compressive equilibrium load.

In contrast to dynamic stiffness, phase angle was not affected by compressive equilibrium load but was reduced as frequency increased (Figure 4). This finding is consistent with the behavior of human lumbar discs in axial compression (Costi et al., 2008). Phase angle represents the lag between load and displacement and can be physically interpreted as the ratio of energy dissipated to energy stored or as a measure of internal friction (Lakes, 1999). The results indicate a disc which behaves more elastically at higher frequencies. Thus, energy may be transmitted along the spine as opposed to being dissipated, potentially elevating the damage risk within other spinal structures. This may help explain connections between high frequency occupational loading and low back pain, while also supporting the inability to connect these high frequency loads with progressive disc degeneration (Battie et al., 2002). However, in addition to mechanical damage, frequency may affect biological factors in progressive degeneration including altered expression of anabolic and catabolic factors, apoptosis, and matrix compositional changes (Ching et al., 2004; MacLean et al., 2004; Walsh and Lotz, 2004).

The impact of reduced nucleus GAG content on disc mechanical properties varied with equilibrium load. At low loads, reduced GAG decreased dynamic stiffness, but did not cause changes at high equilibrium loads. This is consistent with previous work showing that nucleus GAG content plays a role in the neutral zone, the low stiffness regime between compressive and tensile axial loads (Boxberger et al., 2006). The decrease in dynamic stiffness is consistent with human motion segment hypermobility during early degeneration, where moderate GAG loss occurs (Brown et al., 2002). The hydrostatic swelling pressure of the nucleus decreases as GAG content declines, and thus the load distribution within the disc is altered requiring higher loads and deformations for the nucleus to generate sufficient pressure to directly resist applied compression or transfer loads radially to the annulus. With reduced nucleus GAG, the annulus may not being tensioned into its linear stiffness region at low compressive loads, which may elevate the likelihood of collagen degradation (Lotz et al., 2008). Reduced nucleus GAG also led to a higher phase angle relative to controls. This increase in phase angle can relate to an increase in internal friction in the disc, possibly relating to damage mechanisms where fissures form in the disc matrix, as occurs in cartilage (Silver et al., 2004). It is evident that reduced nucleus GAG content results in changes in mechanics which could be implicated in the mechanical progression of degeneration.

The findings presented here should be considered in light of the limitations. A comparison was made between unaltered control samples and specimens which underwent an injection to induce GAG reduction. Needle puncture with a large gauge needle leads to decreased dynamic modulus in bovine tail discs (Korecki et al., 2008) and an anterior-lateral (60° from sagittal plane) injection into the rat disc with a 33 gauge needle may alter neutral zone mechanics (Elliott et al., 2008). The injection procedure used in the current study is, however, through the disc anterior which is the region of greatest height and was identical to that used in a study of quasi-static behavior of rat lumbar discs which produced no significant alterations in axial mechanics (Boxberger et al., 2006). Further, preliminary studies were performed to verify that dynamic viscoelastic axial mechanics were not altered by the injection procedure. Thus we conclude that the observed alterations are due to the glycosaminoglycan reduction, not the injection procedure.

In this study we used a random assignment of discs spanning from L2L3 to L5L6 into treatment groups. Mechanical differences between levels, as observed in the rabbit (Kim et al., 2005), may be contributing to the high standard deviations. While standard deviations may be somewhat elevated due to this randomization, we expect the direction of magnitude of changes to demonstrate the same treatment dependency if a single level was used for the entire study. Further, an added benefit of this randomization is that results can be interpreted more broadly throughout the entire lumbar spine and not limited to one particular level. We report structural properties here, as opposed to material properties, though we expect the structural parameters to be representative of their material counterparts due to level randomization. No differences due to treatment were detected in terms of height loss, and average starting heights and areas were likely similar between groups due to the random sampling. Further, despite the rat lumbar disc being a valid quasi-static model of human lumbar discs, it may behave differently under dynamic conditions as natural frequencies may vary between the two structures. Finally, some matrix degradation may have occurred during the incubation and mechanical testing. Values from fresh discs may vary from those reported here; however, we expect the relationships between load, frequency, and biochemistry to hold as all discs underwent identical incubation times and temperatures.

In summary, this study lends insight into the dynamic behavior of the intervertebral disc and how changes in nucleus GAG content contribute to altered mechanics over multiple compressive loads and frequencies. Increasing equilibrium load led to an increase in disc dynamic stiffness while increasing frequency led to a decrease in phase angle and behavior more akin to an elastic solid. Reducing nucleus GAG content decreased low load dynamic stiffness while ubiquitously increasing phase angle. These findings indicate possible mechanical mechanisms through which disc degeneration can proceed after reduced nucleus GAG content. In addition to elucidating information regarding the interplay between altered biochemistry and mechanics in degeneration, these data provide target mechanical trends for intervertebral disc models and replacements under a range of dynamic axial loading conditions.

Acknowledgments

Funded by the NIH (AR-050052)

Footnotes

Conflict of Interest Statement: In regards to the submitted manuscript “Reduced Nucleus Pulposus Glycosaminoglycan Content Alters Intervertebral Disc Dynamic Viscoelastic Mechanics”, no authors have any financial or personal relationships with other people or organizations which could inappropriately influence this work.

Publisher's Disclaimer: This is a PDF file of an unedited manuscript that has been accepted for publication. As a service to our customers we are providing this early version of the manuscript. The manuscript will undergo copyediting, typesetting, and review of the resulting proof before it is published in its final citable form. Please note that during the production process errors may be discovered which could affect the content, and all legal disclaimers that apply to the journal pertain.

References

  1. Acaroglu ER, Iatridis JC, Setton LA, Foster RJ, Mow VC, Weidenbaum M. Degeneration and aging affect the tensile behavior of human lumbar anulus fibrosus. Spine. 1995;20:2690–2701. doi: 10.1097/00007632-199512150-00010. [DOI] [PubMed] [Google Scholar]
  2. Adams MA, Hutton WC. The effect of fatigue on the lumbar intervertebral disc. Journal of Bone & Joint Surgery - British. 1983;65:199–203. doi: 10.1302/0301-620X.65B2.6826631. [DOI] [PubMed] [Google Scholar]
  3. Adams MA, Hutton WC. Gradual disc prolapse. Spine. 1985;10:524–531. doi: 10.1097/00007632-198507000-00006. [DOI] [PubMed] [Google Scholar]
  4. Andersson G, Ortengren R, Nachemson A. Quantitative studies of the load on the back in different working-postures. Scandinavian Journal of Rehabilitation Medicine - Supplementum. 1978;6:173–181. [PubMed] [Google Scholar]
  5. Antoniou J, Steffen T, Nelson F, Winterbottom N, Hollander AP, Poole RA, Aebi M, Alini M. The human lumbar intervertebral disc: Evidence for changes in the biosynthesis and denaturation of the extracellular matrix with growth, maturation, ageing, and degeneration. Journal of Clinical Investigation. 1996;98:996–1003. doi: 10.1172/JCI118884. [DOI] [PMC free article] [PubMed] [Google Scholar]
  6. Battie MC, Videman T, Gibbons LE, Manninen H, Gill K, Pope M, Kaprio J. Occupational driving and lumbar disc degeneration: A case-control study. Lancet. 2002;360:1369–1374. doi: 10.1016/S0140-6736(02)11399-7. see comment. [DOI] [PubMed] [Google Scholar]
  7. Beckstein JC, Sen S, Schaer TP, Vresilovic EJ, Elliott DM. Comparison of animal discs used in disc research to human lumbar disc: Axial compression mechanics and glycosaminoglycan content. Spine. 2008;33:E166–173. doi: 10.1097/BRS.0b013e318166e001. [DOI] [PubMed] [Google Scholar]
  8. Bland JM, Altman DG. Statistical methods for assessing agreement between two methods of clinical measurement. Lancet. 1986;1:307–310. see comment. [PubMed] [Google Scholar]
  9. Bonifasi-Lista C, Lake SP, Small MS, Weiss JA. Viscoelastic properties of the human medial collateral ligament under longitudinal, transverse and shear loading. Journal of Orthopaedic Research. 2005;23:67–76. doi: 10.1016/j.orthres.2004.06.002. [DOI] [PubMed] [Google Scholar]
  10. Boxberger JI, Auerbach JD, Sen S, Elliott DM. An in vivo model of reduced nucleus pulposus glycosaminoglycan content in the rat lumbar intervertebral disc. Spine. 2008;33:146–154. doi: 10.1097/BRS.0b013e31816054f8. [DOI] [PMC free article] [PubMed] [Google Scholar]
  11. Boxberger JI, Sen S, Yerramalli CS, Elliott DM. Nucleus pulposus glycosaminoglycan content is correlated with axial mechanics in rat lumbar motion segments. Journal of Orthopaedic Research. 2006;24:1906–1915. doi: 10.1002/jor.20221. [DOI] [PubMed] [Google Scholar]
  12. Brown MD, Holmes DC, Heiner AD. Measurement of cadaver lumbar spine motion segment stiffness. Spine. 2002;27:918–922. doi: 10.1097/00007632-200205010-00006. [DOI] [PubMed] [Google Scholar]
  13. Cannella M, Arthur A, Allen S, Keane M, Joshi A, Vresilovic E, Marcolongo M. The role of the nucleus pulposus in neutral zone human lumbar intervertebral disc mechanics. Journal of Biomechanics. 2008;41:2104–2111. doi: 10.1016/j.jbiomech.2008.04.037. [DOI] [PubMed] [Google Scholar]
  14. Ching CT, Chow DH, Yao FY, Holmes AD. Changes in nuclear composition following cyclic compression of the intervertebral disc in an in vivo rat-tail model. Medical Engineering & Physics. 2004;26:587–594. doi: 10.1016/j.medengphy.2004.03.006. [DOI] [PubMed] [Google Scholar]
  15. Costi JJ, Stokes IA, Gardner-Morse MG, Iatridis JC. Frequency-dependent behavior of the intervertebral disc in response to each of six degree of freedom dynamic loading. Spine. 2008;33:1731–1738. doi: 10.1097/BRS.0b013e31817bb116. [DOI] [PMC free article] [PubMed] [Google Scholar]
  16. Elliott DM, Sarver JJ. Young investigator award winner: Validation of the mouse and rat disc as mechanical models of the human lumbar disc. Spine. 2004;29:713–722. doi: 10.1097/01.brs.0000116982.19331.ea. [DOI] [PubMed] [Google Scholar]
  17. Elliott DM, Yerramalli CS, Beckstein JC, Boxberger JI, Johannessen W, Vresilovic EJ. The effect of relative needle diameter in puncture and sham injection animal models of degeneration. Spine. 2008;33:588–596. doi: 10.1097/BRS.0b013e318166e0a2. [DOI] [PubMed] [Google Scholar]
  18. Farndale RW, Buttle DJ, Barrett AJ. Improved quantitation and discrimination of sulphated glycosaminoglycans by use of dimethylmethylene blue. Biochimica et Biophysica Acta. 1986;883:173–177. doi: 10.1016/0304-4165(86)90306-5. [DOI] [PubMed] [Google Scholar]
  19. Galante JO. Tensile properties of the human lumbar annulus fibrosus. Acta Orthopaedica Scandinavica Suppl. 1967;100:101–191. doi: 10.3109/ort.1967.38.suppl-100.01. [DOI] [PubMed] [Google Scholar]
  20. Gordon SJ, Yang KH, Mayer PJ, Mace AH, Jr, Kish VL, Radin EL. Mechanism of disc rupture. A preliminary report. Spine. 1991;16:450–456. doi: 10.1097/00007632-199104000-00011. [DOI] [PubMed] [Google Scholar]
  21. Iatridis JC, MaClean JJ, Ryan DA. Mechanical damage to the intervertebral disc annulus fibrosus subjected to tensile loading. Journal of Biomechanics. 2005;38:557–565. doi: 10.1016/j.jbiomech.2004.03.038. [DOI] [PMC free article] [PubMed] [Google Scholar]
  22. Iatridis JC, Setton LA, Foster RJ, Rawlins BA, Weidenbaum M, Mow VC. Degeneration affects the anisotropic and nonlinear behaviors of human anulus fibrosus in compression. Journal of Biomechanics. 1998;31:535–544. doi: 10.1016/s0021-9290(98)00046-3. [DOI] [PubMed] [Google Scholar]
  23. Johannessen W, Cloyd JM, O'Connell GD, Vresilovic EJ, Elliott DM. Trans-endplate nucleotomy increases deformation and creep response in axial loading. Annals of Biomedical Engineering. 2006;34:687–696. doi: 10.1007/s10439-005-9070-8. [DOI] [PubMed] [Google Scholar]
  24. Johannessen W, Elliott DM. Effects of degeneration on the biphasic material properties of human nucleus pulposus in confined compression. Spine. 2005;30:E724–729. doi: 10.1097/01.brs.0000192236.92867.15. [DOI] [PubMed] [Google Scholar]
  25. Kaigle A, Ekstrom L, Holm S, Rostedt M, Hansson T. In vivo dynamic stiffness of the porcine lumbar spine exposed to cyclic loading: Influence of load and degeneration. Journal of Spinal Disorders. 1998;11:65–70. [PubMed] [Google Scholar]
  26. Kazarian LE. Creep characteristics of the human spinal column. Orthopedic Clinics of North America. 1975;6:3–18. [PubMed] [Google Scholar]
  27. Keller TS, Hansson TH, Holm SH, Pope MM, Spengler DM. In vivo creep behavior of the normal and degenerated porcine intervertebral disk: A preliminary report. Journal of Spinal Disorders. 1988;1:267–278. [PubMed] [Google Scholar]
  28. Keller TS, Spengler DM, Hansson TH. Mechanical behavior of the human lumbar spine. I. Creep analysis during static compressive loading. Journal of Orthopaedic Research. 1987;5:467–478. doi: 10.1002/jor.1100050402. [DOI] [PubMed] [Google Scholar]
  29. Kim JG, An HS, Masuda K, Inoue N. Transactions of the Orthopaedic Research Society. Washington, DC: 2005. Dynamic viscoelastic properties of rabbit lumbar discs. [Google Scholar]
  30. Korecki CL, Costi JJ, Iatridis JC. Needle puncture injury affects intervertebral disc mechanics and biology in an organ culture model. Spine. 2008;33:235–241. doi: 10.1097/BRS.0b013e3181624504. [DOI] [PMC free article] [PubMed] [Google Scholar]
  31. Korecki CL, MacLean JJ, Iatridis JC. Dynamic compression effects on intervertebral disc mechanics and biology. Spine. 2008;33:1403–1409. doi: 10.1097/BRS.0b013e318175cae7. [DOI] [PMC free article] [PubMed] [Google Scholar]
  32. Lakes RS. Viscoelastic solids. In: Kulacki FA, editor. Crc mechanical engineering series. CRC Press; Boca Raton: 1999. [Google Scholar]
  33. Lotz JC, Hadi T, Bratton C, Reiser KM, Hsieh AH. Anulus fibrosus tension inhibits degenerative structural changes in lamellar collagen. European Spine Journal. 2008;17:1149–1159. doi: 10.1007/s00586-008-0721-y. [DOI] [PMC free article] [PubMed] [Google Scholar]
  34. MacLean JJ, Lee CR, Alini M, Iatridis JC. Anabolic and catabolic mrna levels of the intervertebral disc vary with the magnitude and frequency of in vivo dynamic compression. Journal of Orthopaedic Research. 2004;22:1193–1200. doi: 10.1016/j.orthres.2004.04.004. [DOI] [PubMed] [Google Scholar]
  35. Pearce RH, Grimmer BJ, Adams ME. Degeneration and the chemical composition of the human lumbar intervertebral disc. Journal of Orthopaedic Research. 1987;5:198–205. doi: 10.1002/jor.1100050206. [DOI] [PubMed] [Google Scholar]
  36. Perie DS, Maclean JJ, Owen JP, Iatridis JC. Correlating material properties with tissue composition in enzymatically digested bovine annulus fibrosus and nucleus pulposus tissue. Annals of Biomedical Engineering. 2006;34:769–777. doi: 10.1007/s10439-006-9091-y. [DOI] [PMC free article] [PubMed] [Google Scholar]
  37. Race A, Broom ND, Robertson P. Effect of loading rate and hydration on the mechanical properties of the disc. Spine. 2000;25:662–669. doi: 10.1097/00007632-200003150-00003. [DOI] [PubMed] [Google Scholar]
  38. Roughley PJ, Alini M, Antoniou J. The role of proteoglycans in aging, degeneration and repair of the intervertebral disc. Biochemical Society Transactions. 2002;30:869–874. doi: 10.1042/bst0300869. [DOI] [PubMed] [Google Scholar]
  39. Sen S, Boxberger JI, Schroeder Y, Espinoza Orias A, Elliott DM. Effect of degeneration on the dynamic viscoelastic properties of human annulus fibrosus in tension. Proceedings of the ASME 2008 Summer Bioengineering Conference; Marco Island, FL. 2008. [Google Scholar]
  40. Silver FH, Bradica G, Tria A. Do changes in the mechanical properties of articular cartilage promote catabolic destruction of cartilage and osteoarthritis? Matrix Biology. 2004;23:467–476. doi: 10.1016/j.matbio.2004.08.003. [DOI] [PubMed] [Google Scholar]
  41. Walsh AJ, Lotz JC. Biological response of the intervertebral disc to dynamic loading. Journal of Biomechanics. 2004;37:329–337. doi: 10.1016/s0021-9290(03)00290-2. [DOI] [PubMed] [Google Scholar]
  42. Wilke HJ, Neef P, Caimi M, Hoogland T, Claes LE. New in vivo measurements of pressures in the intervertebral disc in daily life. Spine. 1999;24:755–762. doi: 10.1097/00007632-199904150-00005. [DOI] [PubMed] [Google Scholar]

RESOURCES