The Q_γ component of intra-membrane charge movement is present in mammalian muscle fibres, but suppressed in the absence of S100A1

Abstract

S100A1 is a Ca²⁺ binding protein that modulates excitation–contraction (EC) coupling in skeletal and cardiac muscle. S100A1 competes with calmodulin for binding to the skeletal muscle SR Ca²⁺ release channel (the ryanodine receptor type 1, RyR1) at a site that also interacts with the C-terminal tail of the voltage sensor of EC coupling, the dihydropyridine receptor. Ablation of S100A1 leads to delayed and decreased action potential evoked Ca²⁺ transients, possibly linked to altered voltage sensor activation. Here we investigate the effects of S100A1 on voltage sensor activation in skeletal muscle utilizing whole-cell patch clamp electrophysiology to record intra-membrane charge movement currents in isolated flexor digitorum brevis (FDB) muscle fibres from wild-type and S100A1 knock-out (KO) mice. In contrast to recent reports, we found that FDB fibres exhibit two distinct components of intra-membrane charge movement, an initial rapid component (Q_β), and a delayed, steeply voltage dependent ‘hump’ component (Q_γ) previously recorded primarily in amphibian but not mammalian fibres. Surprisingly, we found that Q_γ was selectively suppressed in S100A1 KO fibres, while the Q_β component of charge movement was unaffected. This result was specific to S100A1 and not a compensatory result of genetic manipulation, as transient intracellular application of S100A1 restored Q_γ. Furthermore, we found that exposure to the RyR1 inhibitor dantrolene suppressed a similar component of charge movement in FDB fibres. These results shed light on voltage sensor activation in mammalian muscle, and support S100A1 as a positive regulator of the voltage sensor and Ca²⁺ release channel in skeletal muscle EC coupling.

Skeletal muscle excitation–contraction (EC) coupling is the process by which membrane depolarization triggers sarcoplasmic reticulum (SR) Ca²⁺ release and subsequent contractile activation. Depolarization of the transverse (T) tubule system is detected by the voltage sensor of EC coupling, the dihydropyridine receptor (DHPR), an L-type Ca²⁺ channel (Ca_V1.1). Upon depolarization there is a reorientation of positively charged residues in the transmembrane domain of DHPR that triggers Ca²⁺ release from the coupled ryanodine receptor (RyR1) in the SR membrane (Schneider & Chandler, 1973; Horowicz & Schneider, 1981b; Huang, 1988; Ríos & Pizarro, 1991).

RyR-mediated Ca²⁺ release is modulated by Ca²⁺ and Mg²⁺, as well as by endogenous proteins such as FKBP12, calmodulin, calsequestrin and, more recently, S100A1 (Tripathy et al. 1995; Ikemoto et al. 1989; Jayaraman et al. 1992; Meissner, 1994; Treves et al. 1997). S100A1 is a small Ca²⁺ binding protein highly expressed in striated muscle and localized to the Ca²⁺ release units of skeletal and cardiac muscle cells (Haimoto & Kato, 1987; Zimmer et al. 1991; Most et al. 2003; Prosser et al. 2007). S100A1 interacts with both skeletal and cardiac RyR isoforms and enhances SR Ca²⁺ release. In the heart, S100A1 improves Ca²⁺ cycling and contractile performance (for a detailed review see Most et al. 2007), and is being rapidly pursued as a gene therapeutic agent for the treatment of cardiomyopathy (Pleger et al. 2007; Vinge et al. 2008). The ablation of S100A1 results in ventricular conduction defects and increased mortality following myocardial infarction (Ackermann et al. 2008; Desjardins et al. 2009). While skeletal muscle research has been less extensive, several groups have demonstrated direct effects of S100A1 on skeletal muscle EC coupling. Specifically, S100A1 binds to RyR1 with nanomolar affinity and increases the open probability of the channel (Treves et al. 1997), and exogenous S100A1 enhances Ca²⁺ release and force in skinned slow and fast twitch muscle fibres (Most et al. 2003). We recently have shown that S100A1 competes with calmodulin (CaM) for the CaM binding site on RyR1, and have mapped this interaction using NMR spectroscopy (Prosser et al. 2007; Wright et al. 2008). Furthermore, we showed that compared to muscle fibres from wild-type (WT) animals, muscle fibres from an S100A1 knock-out (KO) animal demonstrate smaller and more slowly developing Ca²⁺ transients when stimulated with an action potential, which can be reversed with adenoviral delivery of S100A1 (Prosser et al. 2007).

Enhancement of SR Ca²⁺ release could be the result of a direct interaction between S100A1 and RyR1, as supported by studies with the purified channel (Treves et al. 1997). A second, not mutually exclusive possibility is that modulation of the voltage sensor mediates S100A1's effect on Ca²⁺ release. This hypothesis is supported by the following. (1) S100A1 modulates the DHPR L-type Ca²⁺ channel in cardiomyocytes (Reppel et al. 2005). (2) S100A1 binds to the CaM binding site on RyR1 (Prosser et al. 2007; Wright et al. 2008), a site proposed to bind and stabilize the C-terminal tail of the DHPR (Sencer et al. 2001). (3) S100A1 binds RyR1 and enhances Ca²⁺ signalling, which may contribute to bi-directional crosstalk between RyR1 and DHPR, a previously documented pathway (Nakai et al. 1996; Avila & Dirksen, 2000). For these reasons we sought to explore the effect of S100A1 on the activation of the voltage sensor of EC coupling.

A classical approach to studying DHPR activation in skeletal muscle is to record gating currents elicited by sarcolemmal and transverse tubule depolarization. This is performed by eliminating ionic and linear capacitive currents, thereby allowing the isolation of non-linear capacitive currents arising primarily from the movement of intra-membrane charged residues in the DHPR (Schneider & Chandler, 1973; Chandler et al. 1976). Initial studies in frog muscle showed that a certain amount of charge must be moved to trigger Ca²⁺ release and fibre contraction (Kovacs et al. 1979; Horowicz & Schneider, 1981a). Since then, recording of charge movement has been used extensively to study DHPR mediated activation of Ca²⁺ release in skeletal muscle, at first largely in amphibian muscle (Hui, 1983; Pizarro et al. 1989; Csernoch et al. 1991; García et al. 1991), and more recently in mammalian muscle (Wang et al. 1999; Avila & Dirksen, 2000; Royer et al. 2008). Here we utilize whole-cell patch clamp electrophysiology to record charge movement currents in mammalian skeletal muscle fibres from WT and S100A1 KO animals. We show that WT mammalian fibres exhibit two components of charge movement, including a temporally delayed, steeply voltage-dependent component reminiscent of ‘Q_γ’ previously identified in amphibian muscle fibres (Adrian & Almers, 1976; Adrian & Peres, 1977). We also demonstrate for the first time that the absence of an endogenous protein can markedly affect voltage sensor charge movement, as Q_γ is suppressed in fibres lacking S100A1. Furthermore, we show that Q_γ can be restored in KO fibres by transient application of S100A1, and is at least partially suppressed by the RyR1 inhibitor dantrolene. These results shed light on the nature of voltage sensor charge movement in mammalian muscle, as well as furthering our understanding of S100A1's modulation of EC coupling. A detailed examination of the relationship between voltage sensor charge movement and RyR Ca²⁺ release in both wild-type and S100A1 deficient muscle fibres is provided in a companion paper.

Methods

Ethical approval

All animals were housed in a pathogen-free area at the University of Maryland, Baltimore. The animals were killed according to authorized procedures of the Institutional Animal Care and Use Committee, University of Maryland, Baltimore (Baltimore, MD), by regulated delivery of compressed CO₂ overdose followed by decapitation.

FDB fibre preparation

Fibres were prepared using enzymatic dissociation of FDB muscles of 6- to 7-week-old C57 × 129 WT and S100A1 KO mice, and were cultured as previously described (Liu et al. 1997). S100A1^−/− animals and WT littermate control animals were obtained from Dr Danna Zimmer, Texas A&M University. The generation and genotyping of these animals has been previously reported (Prosser et al. 2007).

Solutions

For whole-cell Ca²⁺ current measurements bath (external) solution contained (in mm): 150 TEA-CH₃SO₃, 2 CaCl₂, 10 Hepes, 1 MgCl₂, 0.001 TTX, 1 4-aminopiridine, 0.025 N-benzyl-p-toluene sulphonamide (BTS), pH adjusted to 7.4 with CsOH. For non-linear capacitive current recording we use a similar external solution, except we added 0.5 CdCl₂ and 0.5 CoCl₂, and CaCl₂ was reduced to 1 to keep the total divalent concentration constant.

The standard pipette solution (internal solution) contained (in mm): 140 Cs-CH₃SO₃, 10 Hepes, 20 EGTA, 4 MgCl₂, 4 Na₂ATP, 14 creatine PO₄, 0.3 Na₂GTP and 0.1 leupeptin, pH adjusted to 7.4 with CsOH. The choice of such solution was the result of a large series of trial and error tests in preliminary experiments. Compared with large non-permeable inorganic (NMG⁺ and TEA⁺) or organic (aspartate and glutamate) cations, we obtained with a Cs⁺-based solution a large improvement in sealing, durability, blocking of ionic currents, and comparability with experiments at the level of ionic current.

Alexa-Fluor-488 labelled S100A1 protein was a generous gift from Drs Nathan Wright and David Weber, University of Maryland, Baltimore.

Electrophysiology and data analysis

Membrane current measurements were performed using the whole-cell configuration of the patch-clamp technique (Hamill et al. 1981) as previously detailed by Wang et al. (1999) for mammalian FDB fibres but with some modifications. We use an EPC-10 (HEKA Instruments Inc., Bellmore, NY, USA) amplifier and Parafilm coated pipettes of borosilicate glass (WPI-TW150F3) with resistance of 1.2–1.6 MΩ when filled with the internal solution. Once the whole-cell configuration was established, cells were held at −60 mV and the capacitance compensation partially adjusted. Series resistance was compensated to 50–65%. Fibres with signs of qualitative clamp error, such as unstable series resistance, unstable holding current, or a stable holding current above 8 nA, were rejected from the analysis. Measurements were started 20 min after whole-cell configuration was established.

Voltage protocols were generated and current responses were digitized and stored using Patchmaster and Fitmaster (HEKA Instruments). Command pulses were delivered at 10 s intervals to the levels and duration indicated in each figure from a holding potential of −80 mV, unless otherwise indicated. Currents were typically low-pass-filtered at 3–10 kHz (3-pole Bessel filter). Currents were sampled at 10 kHz. Linear capacitive and ionic currents were routinely subtracted by a P/4 protocol from a subholding potential of −20 mV. Gating charge moved during each test depolarization (Q_ON) was quantified by calculating the area under the curve of each trace of non-linear current using the post-transient level of each trace as a steady-state value of non-linear ionic current. Total charge moved during repolarization (Q_OFF) was calculated similarly. Total charge movement was normalized to the linear fibre capacitance, which was estimated using the following equation: C=Q/V. C was determined by measuring linear capacitive current elicited by a ±5 mV test pulse from the holding potential and integrating the area under the capacitive current trace to estimate Q.

Temporal differences in charge development at different voltage steps and between WT and KO groups were considered in setting the time to integrate during a typical 80 ms pulse. To minimize ionic contamination of the ON current, the time of integration for Q_ON was set to the nearest approximation of the end of Q development. As such, for steps to −70 through −40 mV, and for steps above 0 mV, the time of integration for the ON current was set between 15 and 25 ms. For steps to −30 through 0 mV, the time of integration was increased to between 25 and 35 ms due to the presence of a temporally delayed component in WT fibres. In KO fibres lacking a temporally delayed component, an increase in integration time was not required. As a visual depiction of these different integration time points, hash marks have been included on the ON current traces in Fig. 1C to mark the end of the integration time. The time of integration for the OFF current was always set to 50 ms after the pulse. Quantification of charge movement will be considered further in the results.

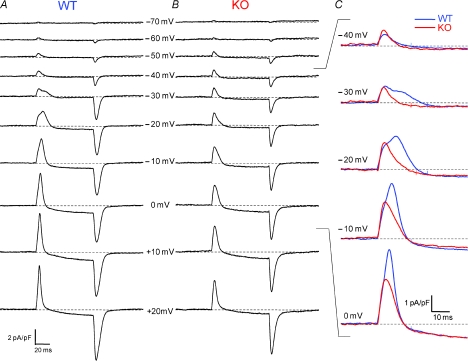
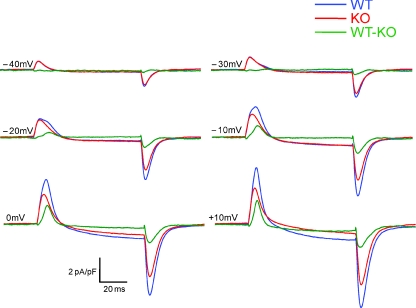
Figure 1. Whole-cell patch clamp recordings of non-linear capacitive (charge movement) currents in WT and KO FDB fibres.

A and B, representative charge movement current traces elicited by 80 ms depolarizing steps from a holding potential of −80 mV. In all traces dotted line represents the zero baseline. C, zoom in of the ON current of superimposed WT and KO current traces demonstrates suppression of temporally delayed ‘hump’ component (Q_γ) in KO fibres. Typically WT fibres developed a noticeable hump around −20 mV, whereas no distinct second component was seen in KO fibres. Colour-coordinated hash marks on current traces represent integration times to establish ‘Q’ for WT and KO fibres, set to 20, 25, or 30 ms depending on the appearance of a temporally delayed component. A single hash mark implies that the same time to integration was used for both traces.

Western blotting

Western blots were performed as in Liu et al. (2009) with modifications. Tibialis anterior muscles were isolated from WT and S100A1^−/− animals, frozen in liquid nitrogen, and stored at −80°C. Muscles were ground to a fine powder at −20°C with mortar and pestle, then lysed and centrifuged, and the soluble fraction collected as previously described (Liu et al. 2009). Protein content was determined using the Bradford assay (Bio-Rad, Hercules, CA, USA). Approximately 50 μg of protein from each sample was separated by SDS-PAGE using a 4–12% gradient gel and transferred to PVDF membrane. Blots were then processed and probed with the appropriate antibodies. The antibody for DHPR α₁s was purchased as tissue culture supernatant from Developmental Studies Hybridoma Bank (Iowa City, IA, USA) and used at a 1: 100 dilution. The antibody for glyceraldehyde phosphate dehydrogenase (GAPDH) was from Sigma (St Louis, MO, USA). Immunoreactive bands were visualized by ECL Western Blotting Detection Reagents (GE Healthcare (Amersham), Piscataway, NJ, USA).

Fluo-4AM Ca²⁺ transient recordings

Action potential evoked Ca²⁺ transients were monitored using the fluorescent indicator Fluo-4AM and ultra high-speed confocal microscopy (Zeiss LSM 5 LIVE system) as previously described (Prosser et al. 2007).

Statistical analysis

All statistical analysis was performed using OriginPro8.0 (OriginLab Corp., Northampton, MA, USA). All data are presented as mean values ± standard error of the mean (s.e.m.) unless otherwise noted. Normality of data sets was ensured for each statistical calculation. Significance was determined using Student's t-test and set at P < 0.05.

Results

Non-linear charge movement currents are different in WT and S100A1 KO fibres

Figure 1 presents non-linear capacitive current records obtained from a FDB fibre from an S100A1 KO mouse (Fig. 1B) and from a WT littermate (Fig. 1A). The current components were elicited with 80 ms depolarizing test pulses to voltages ranging from −70 to +20 mV in 10 mV increments. The displayed records are typical of those obtained from 11 fibres from KO mice and 12 fibres from WT littermates. The transient positive (outward) non-linear current at the start of each depolarization was primarily due to outward movement of intramembrane charged voltage sensors. The more slowly developing or maintained inward or outward current following the transient charge movement current during the larger pulses represents slowly developing or constant voltage-dependent ionic current. The transient negative (inward) current at the end of the pulse was primarily due to the return of these mobile intramembrane charges when the membrane potential was restored to the holding voltage, with a possible additional contribution from non-linear tail currents flowing through ion channels activated during the depolarization. Ionic current interference with the charge movement currents was typically minimal for depolarizations to voltages ranging from −70 to −10 mV, but became increasingly important for larger depolarizations. Procedures for minimizing the influence of such ionic current components on charge movement characterization and quantification will be presented in the following section.

Simple visual comparison of the representative non-linear current records in Fig. 1 reveals several similarities and differences between charge movement currents recorded from the KO and WT fibres. (1) For depolarizations to −70 to −40 mV, the amplitude of the transient currents and their relatively simple time course were similar for KO and WT fibres. (2) During pulses to −30 to 0 mV the charge movement records from the WT fibres exhibited a temporally delayed ‘hump’ component after the initial peak, whereas KO fibres continued to display a time course consisting of a relatively rapid rise followed by a simple decay (see superimposed records in Fig. 1C). A temporally delayed component of charge movement that arises at intermediate depolarizations and produces a ‘hump’ in non-linear current records has been previously described in amphibian fibres and termed Q_γ (Adrian & Almers, 1976; Adrian & Peres, 1977). We will adapt this terminology for the temporally delayed component seen in mammalian fibres here. Due to the similar initial phase in WT and KO fibres, but the presence of a delayed extra component only in the WT fibre, the charge moved by the charge movement current became larger for the WT than for the KO fibre over this intermediate voltage range. (3) For even larger depolarizations (to +10 and +20 mV), a kinetically delayed component was no longer obviously distinguishable. However, the amplitude of the transient charge movement current (and its area) continued to be larger for the WT than for the KO fibre, indicating greater charge moved in the WT fibre. These observations were generally characteristic of WT and KO fibres studied.

Isolation, verification and quantification of charge movement currents

Before quantifying the observations in the preceding paragraph, it is important to establish measures of charge movement that are not appreciably contaminated by non-linear ionic current contributions. The successful elimination of ionic current results in a charge movement current that saturates at extreme voltages, and demonstrates an equal amount of charge moved during depolarization (Q_ON) and repolarization (Q_OFF). As seen in Fig. 2B, Q_ON and Q_OFF demonstrated ON/OFF equality with minimal ionic contamination during and after 80 ms steps to −70 mV to −10 mV (Fig. 2A, top and 2B). However, Q_OFF became significantly larger than Q_ON after 80 ms steps to 0 mV and more positive voltages, suggesting ionic contamination of the OFF recordings, as can be visualized with the longer depolarizing step in Fig. 2A, bottom. To minimize possible non-linear ionic current contamination of the OFF current, we used shorter, 25 ms steps to 0, +10 and +20 mV. This step was of adequate duration to achieve complete charge movement at these voltages, and minimizes the ionic contamination of the OFF, as seen in the ON/OFF equality of the open and filled circles in Fig. 2B and with the shorter depolarizing step in Fig. 2A, bottom. For approximately half the fibres tested we also used 25 ms steps to +30 mV and +40 mV, and found that despite increasing ionic contamination, ON and OFF charge movement were completely saturated at these voltages. These short steps could not be used for the less depolarized voltages, as fibres that exhibited a temporally delayed component elicited charge movement currents lasting longer than 25 ms (demonstrated in Fig. 1C). Ionic contamination of the ON current also appears to have led to a slight underestimation of charge moved at the most positive steps. For this and the other reasons detailed above, to achieve our measure of charge movement (Q) we integrated the ON current traces for 80 ms steps from −70 to −10 mV, and averaged the ON and OFF integrations from short 25 ms pulses for steps from 0 to +20 mV. Following these parameters fibres demonstrated ON/OFF equality, as demonstrated by the linear relationship between the ON and OFF charge seen in Fig. 2C.

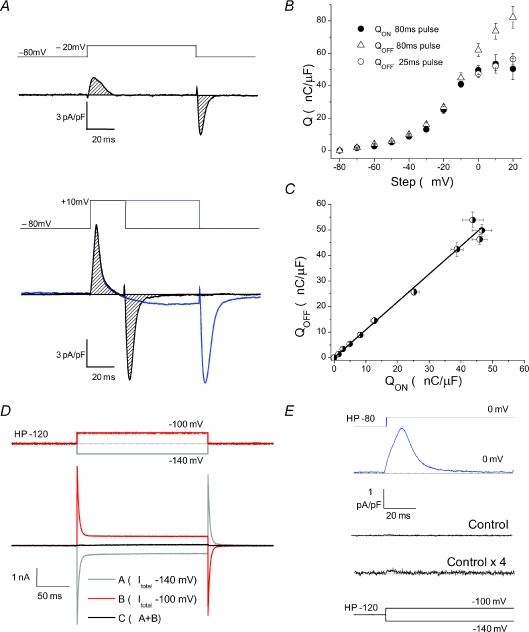
Figure 2. Quantification of charge movement recordings.

A, top, charge movement current trace elicited by an 80 ms step to −20 mV demonstrates ON/OFF conservation with minimal ionic interference. Shaded region marks the area of integration to establish Q_ON and Q_OFF, which are quantified in Fig. 2B. Bottom, at steeper depolarizing, 80 ms steps (such as to +10 mV, blue trace) significant ionic contamination leads to an overestimation of Q_OFF, as seen by the open triangles in Fig. 2B. For this purpose we used shorter, 25 ms steps (black trace) to minimize ionic contamination. Integrating these ON and OFF currents from shorter steps showed charge conservation, quantified with the open and filled circles in Fig. 2B. B, Q_ON and Q_OFFvs. voltage relationship in WT fibres. Filled circles are Q_ON established by integrating non-linear current traces during an 80 ms pulse. Open triangles are Q_OFF from the same 80 ms pulse; note overestimation of Q_OFF at steeper depolarizations, a clear indication of ionic contamination of the OFF records. Open circles are Q_OFF during a 25 ms pulse. Based on the above we averaged Q_ON and Q_OFF from a 25 ms pulse to establish ‘Q’ at steeper depolarizations. C, Q_ONvs. Q_OFF relationship demonstrates charge conservation when using above criteria, using Q_ON values from 80 ms pulses, and Q_OFF values from 80 ms pulses for steps to −80 through −10 mV and from 25 ms pulses for steps to 0 through +20 mV. The data were fitted with a linear function with a slope of 1.09 and R²= 0.986. D, sum response (black) to opposite polarity 20 mV voltage steps (red and grey traces) from a holding potential (HP) of −120 mV indicates negligible non-linearity of membrane properties over voltage range used to obtain leak templates with the P/4 leak subtraction protocol. E, non-linear capacitive current elicited by a test pulse of 80 mV amplitude (from −80 mV to 0 mV; top current trace) using P/4 method. Below is the amplitude of the P/4 leak template (Control, middle current trace) and full leak template (Control × 4; bottom current trace) for comparison.

As the P/4 protocol was used to subtract linear capacitive currents and isolate non-linear charge movement currents during depolarizing pulses, we sought to ensure linearity of membrane properties over the voltage range used to obtain these subtraction templates. Figure 2D demonstrates a sum response (black trace) to opposite polarity 20 mV voltage steps from a holding potential of −120 mV. The red and grey traces represent the total current from the depolarizing and hyperpolarizing steps, respectively. As can be seen the sum of these two currents is essentially zero, suggesting linearity of membrane currents at these very negative potentials. Figure 2E shows the remaining non-linear current elicited by an 80 mV step to 0 mV in a representative WT fibre (blue trace), after subtraction with the P/4 protocol. Below is the amplitude of the subtraction template (middle current trace) and the template scaled up by a factor of 4 (bottom current trace), as would be used in the subtraction from an 80 mV step. Remaining non-linear currents in the control pulses appear negligible compared to the relatively large charge movement currents, validating the use of this technique to isolate non-linear capacitive currents in FDB fibres.

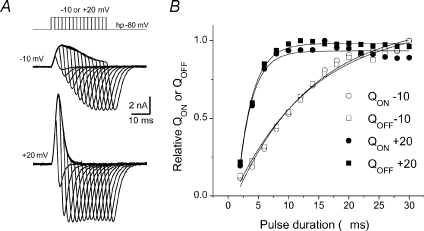
Figure 3 further demonstrates charge movement conservation in WT FDB fibres. Charge movement currents were recorded in response to depolarizing pulses of different duration (starting at 2 ms and increasing in 2 ms increments) to −10 mV (Fig. 3A top), where we typically saw a pronounced temporally delayed component in WT fibres, and to +20 mV (Fig. 3A bottom), where a temporally delayed component was not apparent. Figure 3B shows the relationship between Q_ON or Q_OFF and pulse duration for the set of pulses in Fig. 3A. The normalized charge-pulse duration relationship was fitted to a single exponential function (continuous lines) of the form:

(1)

The calculated Q_ON time constants were 12.7 ms for pulses to −10 mV, and 2.3 ms for pulses to +20 mV. Similar values were obtained for Q_OFF.

Figure 3. Charge conservation.

A, charge movement currents recorded in response to depolarizing pulses to −10 and +20 mV of increasing duration (2 ms increments) to evaluate charge movement conservation. B, Q_ON or Q_OFFvs. pulse duration for the complete set of pulses of corresponding traces illustrated in A. The normalized charge-pulse duration relationships for both Q_ON and Q_OFF are fitted to single exponential functions (continuous lines). The calculated Q_ON time constants were 12.7 ms for pulses to −10 mV, and 2.3 ms for pulses to +20 mV.

Charge movement in S100A1 KO fibres lacks the temporally delayed, steeply voltage-dependent Q_γ component

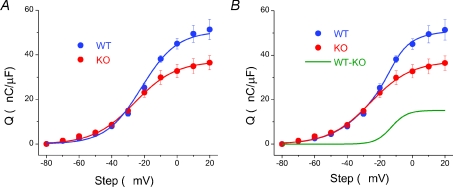
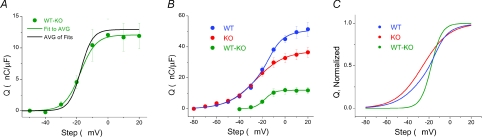
Figure 4A shows the average charge vs. voltage (Q–V) relationship of 12 WT and 11 KO fibres. Consistent with simple visual comparison of charge movement currents between WT and KO fibres, the Q–V relationship was very similar between groups for steps to −70 to −30 mV. However, at −20 mV the data began to diverge and the charge moved in WT fibres (Q_WT) was considerably larger than their KO counterparts (Q_KO), consistent with the development of a second component. As a first approximation, the Q–V relationship of each individual fibre tested was fitted to a single Boltzmann function, as described by the equation:

(2)

where Q_max gives the maximum charge movement, V_half defines the potential where Q= 0.5 of Q_max and 1/k is a measure of the steepness of the Q–V relationship. The KO data were typically well fitted by a single Boltzmann, with average Boltzmann parameters of Q_max= 37.3 ± 3.2 nC μF⁻¹, V_half=−25.8 ± 1.04 mV and k= 13.0 ± 0.9 mV. A single Boltzmann fit to each WT data set gave average parameters of Q_max= 53.0 ± 4.9 nC μF⁻¹, V_half=−21.5 ± 2.5 mV and k= 9.5 ± 0.5 mV. Using these parameters both Q_max and k values were found to be significantly different between WT and KO fibres (P= 0.016 and P= 0.003, respectively).

Figure 4. Q–V relationship of WT (blue) and KO (red) fibres.

A, KO data was well fitted by a single Boltzmann function with parameters of Q_max= 37.3 nC μF⁻¹, V_half=−25.8 mV and k= 12.6; n= 11 fibres. A single Boltzmann function did not accurately fit the WT data. B, based on the second, temporally delayed component of charge movement seen in WT, but not KO fibres, a double Boltzmann fit was applied to the WT data using fixed KO parameters for the first Boltzmann function and free values for the second component. This double Boltzmann function very accurately fitted the WT data. The second component, analogous to the theoretically derived difference component, had Boltzmann parameters of Q_max= 15.2 nC μF⁻¹, V_half=−13.0 mV and k= 4.8; n= 12 fibres. A curve with these parameters is simulated in the WT – KO (green) trace.

Upon inspection of the fits to the average data in Fig. 4A, it is clear that while a single Boltzmann function accurately fits the KO data (R²= 0.998), several points are missed along the WT curve (R²= 0.955). Based on the similar Q–V relationship of WT and KO fibres at lesser depolarizations and the presence of a second, Q_γ component of charge movement seen in WT, but not KO fibres, a double Boltzmann fit was applied to the WT data:

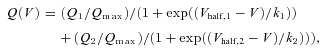(3)

where Q_max is the limiting charge movement, Q₁ and Q₂ are the amplitudes of the charge components, and V_half and k are half-activation voltage and slope factor for each component, respectively. For this fit we used all fixed Q₁, V_half,1, and k₁ parameters from the KO fibres for the first Boltzmann component and allowed free values for the second component. As can be seen in Fig. 4B, this double Boltzmann function very accurately fitted the WT data (R²= 0.998). As all parameters were fixed to KO values for the first component, the improved fit cannot be attributed to an increased number of free parameters; instead it appears the WT and KO data contained a very similar first component, with a second component only present in the WT fibres. The second component of the double Boltzmann fit displayed a steep voltage dependence, with parameters of Q_max= 15.2 nC μF⁻¹, V_half=−13.0 mV and k= 4.8 mV. A curve of this theoretically derived difference component (Q_WT-KO) is simulated in green, and represents Q_γ suppressed in the KO fibres.

To evaluate the properties of the current elicited by the difference component of charge movement we subtracted the average KO current records from the average WT current records for each depolarizing voltage step. This average difference current, I_WT-KO, is plotted vs. the average WT and KO charge movement currents (I_WT and I_KO, respectively) in Fig. 5. I_WT-KO was negligible with steps to −70 through −40 mV. At −30, and more prominently −20 mV, I_WT-KO began to develop a temporally delayed ‘hump’ which further increases in amplitude and area with steps to −10 and 0 mV while shifting back to earlier in time during the pulse. This current saturates in area around 0 mV.

Figure 5. Average recordings of WT (blue) and KO (red) fibres plotted in time with average difference records (green).

Note virtually identical charge movement recordings at −40 and −30 mV steps, followed by development of delayed, steeply voltage dependent hump component (Q_γ) suppressed in KO fibres. At steeper depolarizations this component shifts back earlier in time and saturates in area.

To quantify Q_WT-KO we subtracted the average I_KO record from individual I_WT records for steps to −50 through +20 mV. We then integrated the resulting difference records to get a Q–V relationship for each individual difference component. These Q–V relationships were averaged and fitted to a single Boltzmann function, as plotted in Fig. 6A (AVG Q–V fit). The curve displays parameters Q_max= 12.1 nC μF⁻¹, V_half=−17.7 mV and k= 5.0, which agree nicely with the steeply voltage-dependent component theoretically derived in Fig. 4B. The individual Q_WT-KOQ–V relationships were also fitted to single Boltzmann functions, and their subsequent Boltzmann parameters averaged. These revealed average parameters of Q_max= 12.9 ± 2.4 nC μF⁻¹, V_half=−17.4 ± 2.9 mV and k= 3.7 ± 0.6 mV. A simulated curve from these average parameters (AVG of Fits) is superimposed over the fit of the average Q–V relationship. The most noticeable difference in the curves was the increased steepness of the fit to the average parameters, quantified by the lower k value. The fit to the average Q–V relationship was slightly less steep due to the variable appearance of Q_γ along the voltage axis, which manifested itself as a larger range for V_half (range =−27 mV to −10 mV) and blurring of the steepness of the curve. This slight variability in the voltage dependence of Q_γ also accounted for the larger variation in WT V_half parameters compared to KO V_half parameters. Furthermore, it blurred the kinetic properties of the two distinct components in WT fibres when viewed as an average current record (Fig. 5). A more accurate visual depiction of the two temporal components is seen in traces from individual fibres, as in Fig. 1A, Fig. 7B and Fig. 9B. Figure 6B plots Q_WT-KOvs. Q_WT and Q_KO for comparison. Figure 6C plots the Q–V curves normalized to Q_max, allowing a better visualization of the steeply voltage dependent difference component.

Figure 6. The Q–V relationship of WT–KO difference component, Q_γ.

A, the average KO recording was subtracted from each individual WT current trace, and the resulting difference trace was integrated to establish Q during the step. The green trace represents the fit to the average difference Q–V, with Boltzmann parameters of Q_max= 12.1 nC μF⁻¹, V_half=−17.7 mV and k= 5, similar to those from the theoretically isolated difference component seen in Fig. 3B. The black trace is the slightly steeper curve generated from averaging the Boltzmann parameters of the individual fits to the difference Q–Vs. B, average difference (WT – KO) Q–V plotted in scale with WT and KO Q–V relationships from Fig. 3B. C, normalized Q–V relationships of fits from panel B.

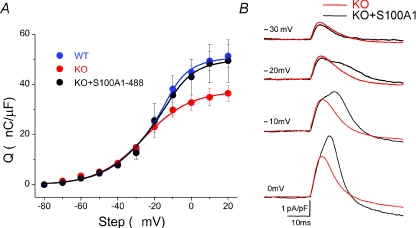
Figure 7. Rescue of Q_γ with purified S100A1.

A, KO fibres dialysed, via the patch pipette, with 10 μm Alexa-488-S100A1 show restoration of Q–V relationship to WT levels. S100A1 protein concentration in KO fibres was estimated using confocal fluorescence microscopy and averaged 2.8 ± 0.21 μm at the time of recordings. B, zoom in of ON current traces from KO AVG and representative KO + S100A1 fibre show that kinetic properties of charge movement currents can be restored in KO fibres dialysed with S100A1, specifically the temporally delayed Q_γ component.

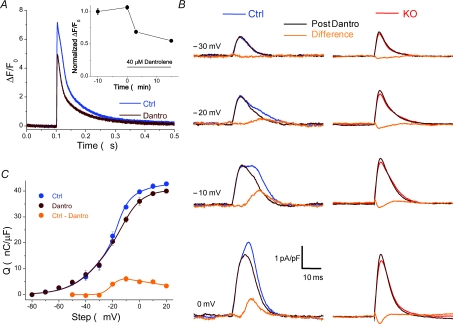
Figure 9. Effect of the RyR1 inhibitor dantrolene on charge movement.

A, exposure of control fibres to 40 μm dantrolene for 3 min suppressed the action potential evoked Ca²⁺ transient by about 35%, measured with high speed confocal microscopy and Fluo4-AM Ca²⁺ indicator dye. This reduction in the Ca²⁺ transient recapitulates the suppression seen in KO fibres (Prosser et al. 2007). The inset shows the normalized peak values of the Ca²⁺ transient (reported as ΔF/F₀±s.e.m.) as a function of time of exposure to dantrolene. B, charge movement currents from a control fibre (left), before (blue trace) and after (brown trace) 3 min exposure to 40 μm dantrolene in the bath, and the resulting difference current (orange trace). As can be seen, the RyR inhibitor dantrolene suppressed a temporally delayed hump component at intermediate voltages, without affecting the initial component of charge movement currents, similar to the difference current between WT and KO fibres. On the right of the figure are charge movement currents elicited by the same voltage steps in 4 KO fibres exposed to the same concentration of dantrolene (red traces). There was no suppression of charge movement in any of these fibres. C, Q–V relationship of 6 control fibres before and after treatment with dantrolene. The steeply voltage-dependent difference component (Ctrl – Dantro; orange Q–V) was fitted to a single Boltzmann function to −10 mV and displayed parameters Q_max= 6.3 nC μF⁻¹, V_half=−21.5 mV and k= 3.0 mV. At higher voltages the difference component decreased monotonically with voltage and was therefore fitted to a linear function.

It is important to note that there was some variability in the presence or absence of the Q_γ component within the groups of KO and WT fibres. Two out of a total of 11 KO fibres did show clear indications of a delayed Q_γ component at one or more intermediate depolarization, and 1 out of 12 WT fibres did not exhibit a clear delayed Q_γ component over the same voltage range.

Thus, the presence or absence of the delayed Q_γ component is not absolutely determined by the presence of absence of S100A1. In the companion paper we show that the presence of the delayed Q_γ component in a few atypical KO fibres and its absence in a few atypical WT fibres is correlated with a relatively larger Ca²⁺ release in these atypical KO fibres, and a relatively smaller Ca²⁺ release in the atypical WT fibres. If these atypical fibres were excluded from the Boltzmann fits to the Q vs. V data sets for each individual fibre, the mean parameter values for the KO fibres (without noticeable delayed Q_γ component) were Q_max= 34.2 ± 2.6 nC μF⁻¹, V_half=−25.8 ± 1.1 mV and k= 13.8 ± 0.8 mV. Excluding the atypical WT fibres lacking clear Q_γ and using mean Q₁ parameter values obtained only from KO fibres without any obvious delayed Q_γ component, the mean parameter values for the second charge component from the double Boltzmann fit are Q_max= 16.1 ± 2.6 nC μF⁻¹, V_half=−16.1 ± 3.1 mV and k= 2.8 ± 0.4 mV.

These refined values are likely to reflect a better estimate of Q_β and Q_γ than obtained by using all KO fibres to define Q_β and all WT fibres (together with all KO fibres for Q_β) to define Q_γ.

Intracellular application of S100A1 protein restores Q_γ in S100A1 KO fibres

Next we sought to evaluate whether the Q_γ component of charge movement suppressed in the KO fibres could be rescued by transient intracellular application of S100A1. To this end, we dialysed KO fibres with purified S100A1 protein and recorded charge movement currents. After our standard 20 min dialysis time, but now with 10 μm Alexa-488-S100A1 added to the pipette solution, non-linear capacitive currents were recorded using the same pulse protocol as detailed in the description of Fig. 1. Additionally, a confocal fluorescence image was taken of the dialysed fibre and a second image was taken of the tip of the pipette. Comparing the fluorescence in the pipette (with a known 10 μm Alexa-488-S100A1) to that of the fibre we could estimate the protein concentration achieved in the cell at the time of the recordings. The mean concentration of purified Alexa-488-S100A1 in the fibre at the time of charge movement recordings was 2.8 ± 0.2 μm, approximating the concentration of endogenous S100A1 in fast twitch skeletal muscle (Haimoto & Kato, 1987; Zimmer et al. 1991). As can be seen in Fig. 7A, dialysis of S100A1 into KO fibres almost completely restored the Q–V relationship of charge movement to the WT level, with restoration of both the total charge moved and the steepness of the curve. These data were well fitted by a double Boltzmann function (R²= 0.998), again using fixed KO parameters for the first Boltzmann and free parameters for the second. The restored (second) component obtained from this fit demonstrated Boltzmann parameters of Q_max= 14.8 nC μF⁻¹, V_half=−12.7 and k= 6.1, similar to the difference component between WT and KO fibres. Figure 7B compares the ON current record of a KO fibre dialysed with Alexa-488-S100A1 compared to the average KO fibre recording. It is clear that transient application of S100A1 can restore the kinetic properties of the charge movement current in KO fibres, as the characteristic temporally delayed hump component of charge movement becomes evident after application of S100A1.

S100A1^−/− fibres do not have significant alteration in L-type channel expression or Ca²⁺ current activation

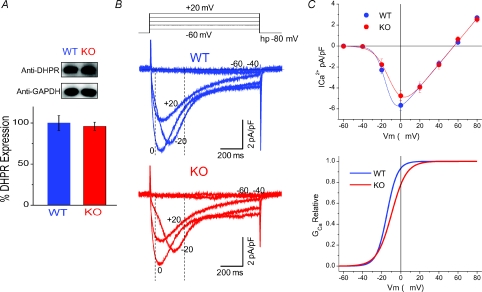
Suppressed maximal charge movement could be the result of decreased DHPR expression in KO muscle. To test this hypothesis, we performed Western blot analysis for the α₁s subunit of the DHPR in WT and KO muscle lysates. Figure 8A shows that protein expression of the DHPR was not altered in KO muscle, suggesting that decreased channel expression did not account for the differences in charge movement seen in KO fibres. Moreover, although S100A1 KO fibres lack Q_γ, they did not exhibit any noticeable difference in both the amplitude and the activation of L-type Ca²⁺ currents compared to WT fibres. Figure 8B presents representative time courses of Ca²⁺ currents from WT and KO fibres in response to 1 s depolarizing steps to −60 mV to +20 mV as illustrated above the graph. Current onset was slow and maximum inward current peaked at approximately 80 ms with a pulse to 0 mV. The voltage dependence of activation and peak current density appear similar between WT and KO fibres. The current vs. voltage (I–V) relationship for pooled data from 11 WT and eight KO fibres is shown in Fig. 8C. There were no significant differences in current density at any of the voltages tested, including the maximum inward current elicited by a step to 0 mV (WT I_Ca=−5.8 ± 0.5 pA pF⁻¹, KO I_Ca=−5.1 ± 0.5 pA pF⁻¹, P= 0.27). I–V plots from WT and KO fibres were fitted to a Boltzmann Ohmic function, described by the following equation (Nakai et al. 1996):

(4)

where G_max is the maximum conductance, V is the membrane potential, V_rev is the reversal potential, V_half is the half-activation potential, and k is a measure of the steepness. Average parameter values for G_max, V_half and k for WT fibres were 108.7 pS pF⁻¹, −14.7 mV and 5.4 mV, respectively. For KO fibres G_max, V_half and k were 106.0 pS pF⁻¹, −9.6 mV and 7.6 mV, respectively. These parameters were used to derive the activation curves for WT and KO fibres, as plotted in Fig. 8C (bottom). Other than a slight rightward shift in the V_half of KO fibres, there were no noticeable differences in L-type current activation. This suggests that despite suppression of Q_γ in KO fibres, the activation of L-type Ca²⁺ currents was not significantly affected.

Figure 8. L-type Ca²⁺ current density, voltage dependence and channel expression are not significantly changed in KO fibres.

A, representative Western blots with anti-α₁s from lysates of WT and S100A1^−/− muscle show similar DHPR expression, with GAPDH used as a loading control. The bar graph is the result of triplicate experiments in WT and KO muscle, showing that the expression of the DHPR is not altered with the ablation of S100A1. Data are presented as a percentage of the average WT DHPR expression, normalized to the GAPDH signal. B, representative macroscopic Ca²⁺ current recordings of WT (blue) and KO (red) fibres. In these experiments Cd²⁺ and Co²⁺ were eliminated from the external solution and Ca²⁺ was increased to 2 mm. Vertical dashed lines illustrate the time used to measure peak current values to create the I–V plots. C, top, I–V relationship of average data from WT and KO fibres reveals no significant differences in L-type channel current density and voltage dependence. The data are fitted to a Boltzmann-Ohm function. At bottom is the activation profile of WT and KO fibres derived from parameters of a Boltzmann-Ohm function. These results suggest that suppressed charge movement in KO fibres is not linked to changes in L-type Ca²⁺ channel expression and voltage-dependent activation.

Application of dantrolene suppresses the Q_γ component in WT fibres

It has been suggested that the Q_γ component of charge movement identified in amphibian fibres is a consequence of Ca²⁺ released from the SR (Csernoch et al. 1991). If the decreased charge movement in KO fibres is a consequence of their decreased Ca²⁺ release (Most et al. 2003; Prosser et al. 2007), then a pharmacological intervention that reduces Ca²⁺ release should suppress the second component of charge movement. Therefore, we sought to reduce Ca²⁺ release with dantrolene, an inhibitor of RyR1, and measure the effect on charge movement. To this end we first identified a suitable concentration of dantrolene that mimicked the reduction of the Ca²⁺ transient inherent to fibres lacking S100A1. As shown in Fig. 9A, we found that after 3 min exposure to 40 μm dantrolene the peak amplitude of the action potential evoked Ca²⁺ transient was reduced by about 35%. The effect was consistent, relatively stable over time, and in general mimicked the reduction of the Ca²⁺ transient previously reported for fibres lacking S100A1 (Prosser et al. 2007). We then recorded the effect of this concentration of dantrolene on charge movement in control fibres. Figure 9B (left) shows representative ON charge movement currents of a control fibre before and after 3 min exposure to dantrolene, and the resulting difference record. Exposure to dantrolene did not affect the first component of charge movement but at least partially suppressed the temporally delayed component seen at intermediate depolarizations. Figure 9B (right) shows the average ON charge movement currents of four KO fibres before and after dantrolene, and the resulting difference current. There was no temporally delayed component in these KO fibres prior to exposure to dantrolene, and, notably, exposure to dantrolene caused no further suppression in charge movement in any of these fibres. The only detectable difference was a slight increase of the Q_β component of charge movement, as indicated by the minor negative deflection in the difference current. Figure 9C shows the pooled Q versus V relationship from six control fibres before and after treatment with dantrolene. The Q–V relationship of the integrated difference records is also displayed for comparison. The difference component over the voltage range of −50 mV to −10 mV was fitted by a single Boltzmann function with parameters Q_max= 6.3 nC μF⁻¹, V_half=−21.5 mV and k= 3.0 mV. It should be noted that this fit only accurately described the Q vs. V relationship of the dantrolene isolated difference component for steps to −50 mV to −10 mV. Upon further depolarizations the difference component appears to decrease monotonically with voltage, and therefore has been fitted with a straight linear function over this voltage range. These findings suggest that 40 μm dantrolene partially suppresses and/or shifts the voltage dependence of Q_γ in control fibres to higher potentials, but has minimal or no affect on KO fibres which initially lack Q_γ.

La³⁺ profoundly modifies charge movement currents in FDB fibres, but Cd²⁺ and Co²⁺ do not

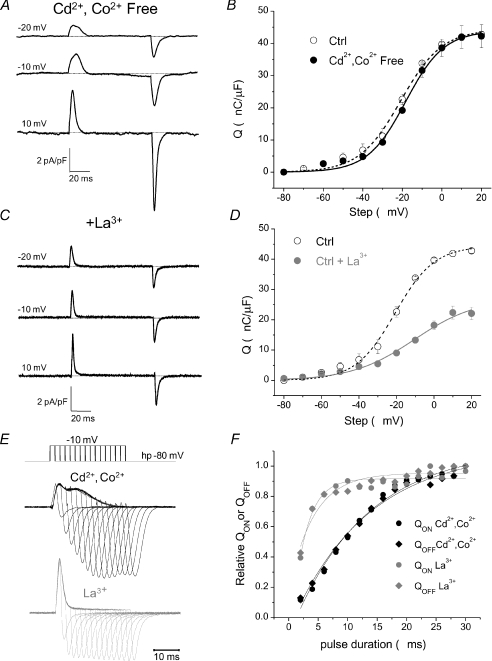
As the temporally delayed Q_γ component of charge movement has not been consistently demonstrated in mammalian fibres, we next evaluated possible explanations for this discrepancy. Since Cd²⁺ has been suggested previously to alter the kinetic properties of charge movement (Francini et al. 2001), we first evaluated charge movement in the absence of Cd²⁺ and Co²⁺ by using our standard internal and external solutions, except with the elimination of Cd²⁺ and Co²⁺ from the external solution. To block inward Ca²⁺ currents and allow the evaluation of charge movement currents, we added 10 mm Mg²⁺ to the external solution. This set of control experiments, both for Cd²⁺/Co²⁺ and for La³⁺ (see below), were carried out on FDB fibres from mice of the C57Black strain. Figure 10A shows representative recordings from one such control fibre bathed in the high Mg²⁺, Cd²⁺ and Co²⁺ free external solution. The presence of a temporally delayed ‘hump’ component is clearly evident in the current records at similar voltages to where it is typically seen in control fibres bathed in our standard Cd²⁺/Co²⁺ based external solution. Figure 10B shows the pooled Q vs. V relationship of three control fibres exposed to high Mg²⁺, Cd²⁺ and Co²⁺ free external solution and three control fibres exposed to Cd²⁺/Co²⁺solution. The Q vs. V relationship of fibres treated with high Mg²⁺, Cd²⁺ and Co²⁺ free solution was very similar in both slope and amplitude to that of fibres exposed to Cd²⁺/Co²⁺, with only a slight, 2–3 mV shift in the half-maximal voltage. The data were fitted by a double Boltzmann function with the following parameters: Q_max,1= 33.8 nC μF⁻¹, Q_max,2= 14.2 nC μF⁻¹, V_half,1=−19.0 mV, V_half,2=−13.0 mV, k₁= 12.8 mV and k₂= 4.0 mV.

Figure 10. La³⁺ profoundly modifies intra-membrane charge movement.

A, charge movement currents recorded using an external solution lacking Cd²⁺ and Co²⁺ exhibit a temporally delayed component and similar current kinetics to fibres bathed in our standard control solution. Ca²⁺ was reduced to 0.5 mm and 10 mm Mg²⁺ was added to the external solution to block inward ionic components. B, fibres bathed in control external solution (open circles, dashed line) demonstrate a very similar Q vs. V relationship to fibres bathed in Cd²⁺ and Co²⁺ free external solution (filled circles, continuous line). C, charge movement currents recorded using an external solution supplemented with 0.3 mm La³⁺ exhibit rapid current kinetics and no clear temporally delayed ‘hump’ component. D, fibres bathed in our control external solution supplemented with La³⁺ demonstrate decreased maximal charge moved and a rightward shift in the voltage dependence of charge movement when compared to fibres bathed in our standard external solution. E, charge movement currents recorded in control and La³⁺-treated fibres in response to depolarizing pulses to −10 mV of increasing duration (2 ms increments) to evaluate charge movement conservation. F, Q_ON or Q_OFFvs. pulse duration for the complete set of pulses of corresponding traces illustrated in E. The normalized charge–pulse duration relationships for both Q_ON and Q_OFF are fitted to single exponential functions (continuous lines). The calculated time constant for charge development in the Cd²⁺/Co²⁺ exposed fibre was 13 ms, compared to 2.4 ms for the fibre exposed to La³⁺.

La³⁺ is another common and widely used Ca²⁺ channel blocker. In preliminary experiments using a recording solution containing La³⁺ we did not detect the presence of two kinetically distinct components of charge movement in FDB fibres. We therefore sought to further evaluate the effects of La³⁺ on charge movement. Figure 10C shows representative charge movement currents from fibres exposed to 0.3 mm La³⁺ in our standard external solution containing Cd²⁺/Co²⁺. These fibres exhibited dramatically increased current kinetics and did not display any clear temporally delayed ‘hump’ component in the current records. Figure 10D plots the Q vs. V relationship of pooled data from five control fibres exposed to La³⁺ compared with the control fibres exposed to Cd²⁺/Co²⁺ from Fig. 10B. As a first approximation the La³⁺Q vs. V data were fitted to a single Boltzmann function, with parameters Q_max= 27.0 nC μF⁻¹, V_half=−10.4 mV and k= 16.6 mV, R²= 0.987. Therefore, the addition of La³⁺ led to a reduction in both Q_max and the steepness of the Q vs. V relationship, as well as a rightward shift in the voltage dependence of charge movement.

Figure 10E compares charge development with increasing pulse duration from a step to −10 mV in a fibre treated with our standard external solution (top) vs. one supplemented with 0.3 mm La³⁺ (bottom). A temporally delayed hump component is clearly distinguishable in the fibre exposed to Cd²⁺/Co²⁺, and noticeably absent in the La³⁺ exposed fibre, which exhibits markedly increased current kinetics. Figure 10F plots the charge vs. pulse duration relationship for these two fibres. The calculated time constant for charge development in the Cd²⁺/Co²⁺ exposed fibre was 13 ms, compared to 2.4 ms for the fibre exposed to La³⁺. Thus the addition of La³⁺ to our standard external solution dramatically increased the kinetics of charge movement and decreased the amount of charge moved in control fibres.

Discussion

Gating charge movement in muscle

Gating charge movement was first predicted by Hodgkin & Huxley (1952) to explain the voltage-dependent origin of Na⁺ and K⁺ conductance in the nerve. The first recordings of these gating currents were applied to the activation of muscle contraction by Schneider & Chandler (1973), who proposed that voltage-dependent intra-membrane charge movement was a key step in skeletal muscle EC coupling. Evidence has accumulated demonstrating that the α1 subunit (α_1S) of the DHPR is the primary source of this charge movement (Tanabe et al. 1988; Nakai et al. 1996) that triggers Ca²⁺ release from the mechanically linked RyR1 (Block et al. 1988; Protasi et al. 2002) and promotes skeletal-specific muscle contraction (Horowicz & Schneider, 1981a).

While the majority of the seminal work on muscle charge movement was done in amphibian fibres, recently the focus has switched to mammalian muscle and expression systems. One striking difference between recordings in amphibian and mammalian muscle is the kinetic properties of charge movement currents. In amphibian fibres two distinct components of charge movement have repeatedly been identified: a relatively fast initial component, Q_β, and a temporally delayed ‘hump’ component, Q_γ (Adrian & Almers, 1976; Horowicz & Schneider, 1981a; Melzer et al. 1986; Csernoch et al. 1991; Jong et al. 1995). On the contrary, in charge movement recordings in mammalian fibres and myotubes Q_γ has been conspicuously absent; charge movement currents appear generally as a rise to peak followed by a simple decay (Hollingworth & Marshall, 1981; Simon & Beam, 1985; Delbono et al. 1991; Wang et al. 1999; Avila & Dirksen, 2000; Bannister & Beam, 2005; Royer et al. 2008). It should be noted that Simon & Beam (1985) and Lamb (1986a) did report that in a minority of their mammalian fibres charge movement recordings exhibited a slight temporally delayed component resembling Q_γ, and Francini et al. (2001) were able to detect a delayed component in rat EDL muscle. However the overall discrepancy between amphibian and mammalian charge movement records remains unresolved.

Recent groups using the whole cell patch-clamp technique on FDB fibres have demonstrated charge movement currents with a single rise and simple decay (Wang et al. 1999, 2002; Royer et al. 2008). Here using this same technique we demonstrate a clear, consistent, temporally delayed component in the ON charge movement currents of WT murine FDB fibres. As an explanation for this discrepancy, we propose that the use of the trivalent cation lanthanum as a Ca²⁺ channel blocker (Wang et al. 1999, 2002; Royer et al. 2008) may alter charge movement currents and mask Q_γ in mammalian fibres. We use a channel blocking cocktail of Cd²⁺ and Co²⁺, with no added La³⁺, to minimize ionic currents; in our hands the addition of La³⁺ dramatically increased the kinetics of charge movement currents and abolished any temporally distinct components (Fig. 10C, E and F), leaving recordings that kinetically resemble those of these recent reports. We also noted a reduction in the maximum charge moved (Q_max) and rightward shift in the voltage dependence of charge movement (V_half) in the presence of La³⁺, when compared to our control solution. The La³⁺Q_max value reported here is about 30% less than that reported by Wang et al. (1999) and Royer et al. (2008). Potential explanations for this discrepancy include different strains and age (5–7 weeks here vs. 5–7 months in the study of Wang et al.) of mice studied. Additionally, the studies by both Wang et al. and Royer et al. used a higher concentration of Mg²⁺ in their external solution (2 and 3.5 mm, respectively) than our 1 mm Mg²⁺. Increased Mg²⁺ could potentially blunt some of the effects of La³⁺ on the voltage sensor. A final possibility to consider is that part of the decrease in charge movement seen here in the La³⁺ containing solution could be due to a partial detubulation of the fibres in La³⁺. There was a slight, but not significant, decrease in the total fibre linear capacitance from 1.33 ± 0.05 nF and 1.32 ± 0.05 nF for WT and KO fibres (n= 38 and 29, respectively) measured in our standard external solution to 1.20 ± 0.11 nF for the 13 fibres measured in La³⁺ containing solution (P= 0.24), indicating that detubulation was likely not to be a major factor. To our knowledge the effects of La³⁺ on skeletal EC coupling have not been thoroughly explored; however La³⁺ has been shown to alter both sodium and potassium voltage-dependent channel gating in a manner that cannot be explained by surface charge considerations alone (Armstrong & Cota, 1990; Tytgat & Daenens, 1997). We additionally observed both time- and use-dependent effects of La³⁺ on charge movement currents and therefore suggest that the use of this trivalent cation as an L-type Ca²⁺ channel blocker should be approached with caution.

Effects of S100A1 on voltage sensor charge movement

A striking finding of this work is the modulation of intra-membrane charge movement by an endogenous Ca²⁺ binding protein, S100A1. Q_γ was selectively suppressed in fibres lacking S100A1, resulting in reduced sensitivity to activation (decreased steepness of the Q–V relationship), and reduced maximal activation (decreased Q_max) of the voltage sensor. Notably, the first component of charge movement (Q_β) appeared unaffected by ablation of S100A1, as can be seen by the identical current traces at voltages hyperpolarized to the development of Q_γ (Fig. 4), as well as by the accurate double Boltzmann fit to the WT Q–V relationship with fixed KO parameters describing the first component. These data suggest a selective suppression of a distinct component of charge movement in fibres lacking S100A1.

Moreover, several lines of evidence presented here support that effects on charge movement appear to be specific to S100A1, and not a result of compensatory changes in KO animals. First, a 20 min intracellular application of S100A1 restored both the total charge moved and the kinetics of Q_γ in KO fibres, arguing against a developmental role of S100A1 in the modulation of charge movement. Second, Western blot analysis suggests that protein expression of the DHPR is not changed in KO fibres. Third, the L-type Ca²⁺ current density and voltage-dependent activation do not appear to be significantly altered in KO fibres. The last two pieces of evidence suggest that the total expression of DHPR Ca²⁺ channels, as well as the functional fraction of these Ca²⁺ channels, is preserved in fibres lacking S100A1, implying that the suppressed charge in KO fibres is independent of channel expression and/or developmental or compensatory changes due to genetic manipulation. This is a unique finding, as multiple groups have demonstrated a reduction in charge movement with the alteration of endogenous proteins, but as a by-product of reduced functional L-type channels. For example, mutations to the β1 subunit of the DHPR lead to reduced α_1S expression at the membrane and a concomitant decrease in charge movement (Gregg et al. 1996; Schredelseker et al. 2009), and expression of the RGK family member Rem reduces the functional number of DHPRs and charge movement in myotubes (Bannister et al. 2008). To our knowledge, this specific modulation of gating charge by S100A1 is the first example of an endogenous protein regulating skeletal muscle charge movement independent of channel expression.

This reduction in charge movement is consistent with our previous findings that fibres lacking S100A1 have depressed SR Ca²⁺ release (Prosser et al. 2007). In that work we showed that action potential evoked Ca²⁺ transients are smaller in KO fibres compared to WT counterparts, a finding predicted if there is decreased activation of the voltage sensor. Additionally, as DHPR expression levels and L-type Ca²⁺ currents in KO fibres do not differ from their WT counterparts (Fig. 8B and C), the suppressed Q_γ component does not appear to be linked to gating of the DHPR Ca²⁺ channel, but instead is likely to be linked to the excitation–contraction coupling machinery. One interpretation of these findings is that S100A1 modulates intra-membrane voltage sensors that regulate Ca²⁺ release, but not the gating of the DHPR Ca²⁺ channel. However, we have also previously proposed that S100A1's modulation of Ca²⁺ release is a direct effect of S100A1 binding to RyR1 and enhancing RyR1 release. This was supported by previous reports of S100A1 increasing the open probability of the purified RyR1 in single channel recordings (Treves et al. 1997), and our demonstration, in conjunction with our collaborators, that S100A1 competes with calmodulin for binding the CaM binding domain (CaMBD) of RyR1 (Prosser et al. 2007; Wright et al. 2008), a site previously demonstrated to modulate the channel's activation (Tripathy et al. 1995; Moore et al. 1999; Rodney & Schneider, 2003).

While these two proposed mechanisms for S100A1's modulation of EC coupling may appear contradictory, they are not mutually exclusive. The DHPR and RyR interact in both an ‘orthograde’ manner, where the DHPR signals RyR1 to open, and a ‘retrograde’ fashion, where RyR1 prevents DHPR inactivation (Nakai et al. 1996). Interestingly, the CaMBD where S100A1 binds RyR1 is involved with mechanical coupling and bi-directional signalling with the DHPR. Specifically, the CaMBD of RyR1 interacts with the carboxyl-terminal tail of the DHPR (Sencer et al. 2001), and the region of RyR1 containing the CaMBD elicits a retrograde signal to the DHPR (Nakai et al. 1998). It is tempting to speculate that S100A1 interacting with this same region of RyR1 may affect retrograde coupling between the two proteins, enhancing voltage sensor activation and/or Ca²⁺ release. However, studies on retrograde signalling in myotubes suggest that while L-type Ca²⁺ currents are dramatically affected by interaction with RyR1, voltage sensor charge movement is minimally influenced, undermining this hypothesis (Nakai et al. 1996). Nonetheless, myotubes lack Q_γ and demonstrate less than 20% of the total charge moved in adult fibres (Nakai et al. 1998; Avila & Dirksen, 2000), validating the exploration of the interaction between the CaMBD of RyR1 and the DHPR and its effects on charge movement in adult fibres.

Origin of Q_γ

An alternative explanation for the enhanced charge movement in the presence of S100A1 is that Q_γ appears as a consequence of RyR-mediated Ca²⁺ release. This is not a novel concept; the nature of the delayed component has been widely debated. Several groups proposed Q_γ to be the primary charge moved by the voltage sensor that causes Ca²⁺ release (reviewed in Huang, 1988). Yet Q_γ has also been well argued to be a consequence of release, in a model put forth primarily by Pizarro et al. (1991). Our findings with the RyR1 inhibitor dantrolene support this hypothesis. Dantrolene rapidly suppressed the temporally delayed hump in charge movement currents of control fibres, isolating a component with similar initial voltage dependence to the component suppressed in KO fibres. These results suggest that Q_γ may be a function of optimal RyR-mediated Ca²⁺ release. In KO fibres, where Q_γ is already suppressed (in conjunction with suppressed release, as is documented in the companion paper), dantrolene treatment caused no further suppression of charge, as would be expected if Q_γ is a consequence of optimal release.

However, our data with dantrolene treatment of control fibres do not completely mimic the difference in charge movement between WT and KO fibres. The difference component isolated by dantrolene peaks around −10 mV and then begins to decrease monotonically with voltage, suggesting a rightward shift in the voltage dependence of Q_γ. We cannot determine whether this difference component would diminish to zero upon further depolarization beyond +20 mV, mostly due to the presence of ionic contamination at these large depolarizations which precluded an accurate isolation of charge movement currents. In contrast to the dantrolene isolated component, we did not see rescue of Q_γ at larger depolarizations in KO fibres, where differences in maximal saturating charge in WT compared to KO fibres were maintained even at steps to +30 or +40 mV. These findings suggest a more complex mechanism than simply the amount of Ca²⁺ release dictating the presence or absence of Q_γ (with Q_γ defined here as the difference component between WT and KO fibres). A detailed examination of the relationship between charge movement and Ca²⁺ release in WT and KO fibres is needed to address these questions, and is provided in the companion paper (Prosser et al. 2009).

Regardless of this discrepancy, it is clear from our studies and previous work that Q_γ is selectively affected when the RyR1 Ca²⁺ release channel is altered (Lamb, 1986b; Hollingworth et al. 1990; Csernoch et al. 1991; García et al. 1991; Gonzalez & Rios, 1993). This does not appear to be a function of global myoplasmic [Ca²⁺], as all of our recordings were performed in high intracellular EGTA. Instead our data suggest a process, dependent on optimal RyR1 activation and/or local Ca²⁺ release, that can be modulated by S100A1, and that positively feeds back on voltage sensor charge movement. The mechanistic underpinnings of the relationship between S100A1, Ca²⁺ release and Q_γ are further explored in the companion paper.

Summary

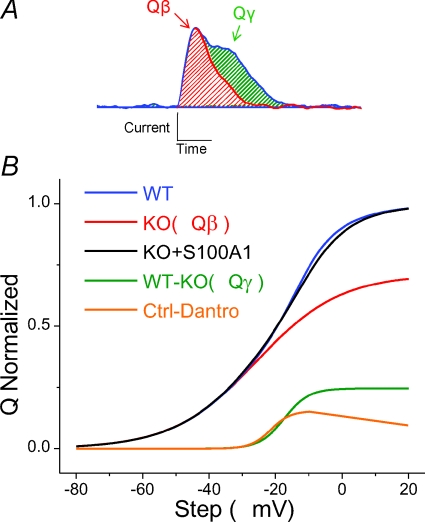
In conclusion, here we demonstrate that mammalian muscle fibres exhibit two distinct components of intra-membrane charge movement (Fig. 11A), a relatively rapid initial rise to peak (Q_β), and a temporally delayed hump component (Q_γ). The use of La³⁺ as a Ca²⁺ channel blocker altered the kinetics and voltage dependence of intra-membrane charge movement. We showed that Q_γ is selectively suppressed in muscle fibres lacking S100A1 (Fig. 11B, red curve), a protein known to bind to RyR1 and enhance Ca²⁺ release. We also found that the RyR-inhibitor dantrolene selectively suppresses a similar component of voltage sensor charge movement (Fig. 11B, orange curve), suggesting that Q_γ may be a product of cross talk between RyR1 and DHPR. Transient application of S100A1 rescues Q_γ in KO fibres (Fig. 11B, black curve), suggesting a specific effect of S100A1 on charge movement. These results further support S100A1 as a positive regulator of the t-tubule voltage sensor and SR Ca²⁺ release channel in skeletal muscle-type EC coupling.

Figure 11. Components of charge movement in mammalian fibres.

A, charge movement in WT fibres consisted of two components, the first eliciting a current with an initial rise to peak and simple decay, similar to Q_β (red), and the second, a temporally delayed, steeply voltage dependent component, similar to Q_γ (green). Q_γ was suppressed in fibres lacking S100A1, consistent with decreased RyR-mediated Ca²⁺ release. B, comparison of the Q–V relationships of WT (blue; total charge movement) and KO (red; Q_β) fibres, along with difference Q–V plot (WT−KO, green; Q_γ). Transient application of S100A1 restored Q_γ, suggesting that this suppression of charge movement is not due to developmental or compensatory effects of S100A1 KO (KO+S100A1, black). Inhibition of RyR with dantrolene also suppressed a temporally delayed, steeply voltage dependent component similar to Q_γ (Ctrl – Dantro, orange), suggesting this component may be a product of crosstalk between the voltage sensor of EC coupling, DHPR, and the mechanically coupled SR Ca²⁺ release channel, RyR. All curves are Boltzmann fits to average data.

Glossary

Abbreviations

CaM

calmodulin

CaMBD

calmodulin binding domain

DHPR

dihydropyridine receptor

EDL

extensor digitorum longus

FDB

flexor digitorum brevis

KO

knock out

RyR

ryanodine receptor

SR

sarcoplasmic reticulum

Author contributions

B.L.P. performed the majority of experimentation, analysis, and writing involved with this work. E.O.H. contributed to all aspects of this work, particularly to experimental design and interpretation of results. D.B.Z. designed and provided the S100A1^−/− animal and WT counterparts, and also critically revised and approved the final manuscript. M.F.S contributed to experimental design, analysis, and discussion. All the experiments presented above were performed in the lab of M.F.S., at the University of Maryland, Baltimore.