The Potential for Brain Injury on Selected Surfaces Used by Cheerleaders

Abstract

Context:

Although playground surfaces have been investigated for fall impact attenuation, the surfaces that cheerleaders use have received little attention.

Objective:

To determine (1) the critical height for selected surfaces used by cheerleaders at or below which a serious head impact injury from a fall is unlikely to occur, (2) the critical heights for non–impact-absorbing surfaces for comparison purposes, and (3) the effect of soil moisture and grass height on g_max (which is defined as the multiple of g [acceleration due to gravity at the earth's surface at sea level: ie, 32.2 feet·s⁻¹·s⁻¹] that represents the maximum deceleration experienced during an impact) and the Head Injury Criterion (HIC) at the critical height for a dry grass surface.

Design:

Observational study.

Settings:

A local cheerleading gym, indoor locations within the authors' institution, and various outdoor locations.

Main Outcome Measure(s):

g_max, HIC, and critical height.

Results:

Critical heights for the surfaces tested ranged from 0.5 ft (0.15 m) for concrete and vinyl tile installed over concrete to more than 11 ft (3.35 m) for a spring floor. Increases in grass height and soil moisture resulted in an increase in the critical height for grass surfaces. Only spring floors and 4-in (0.10-m)–thick landing mats placed on traditional foam floors had critical heights greater than 10.5 ft (3.20 m), thus providing enough impact-absorbing capacity for performance of 2-level stunts.

Conclusions:

The potential for serious head impact injuries can be minimized by increasing the shock-absorbing capacity of the surface, decreasing the height from which the person falls, or both. Cheerleaders and cheerleading coaches should use the critical heights reported in this study to compare the relative impact-absorbing capacities of the various surfaces tested, with critical height as an indicator of the impact-absorption capacity of the surface. The findings of this study can be used to select the most appropriate surface for the type of maneuver to be performed, based on the maximum height expected to be achieved by the cheerleader(s) during execution of the maneuver. Cheerleaders should not perform maneuvers at heights that exceed the critical height for the surface on which they are performing.

Key Points

• For the surfaces tested, critical heights ranged from 0.5 ft (0.15 m; concrete, vinyl tile over concrete) to more than 11 ft (3.35 m; spring floor).

• Only spring floors and 4-in (0.10-m)–thick landing mats over foam floors provided sufficient impact absorption for the performance of 2-level cheerleading stunts.

• Selecting the most appropriate surface for the cheerleading maneuver being performed may help to minimize the potential for serious head impact injuries.

Considerable attention has been given to establishing safety thresholds for playground surfaces with respect to fall impact attenuation,^1–12 yet to date, nothing has been published regarding the impact attenuation of surfaces that cheerleaders use for practices and performances. Head impact injuries from a fall have the potential to be life threatening. About 75% of all fall-related deaths reported to the US Consumer Product Safety Commission since 1973 involved head injuries.¹³ Mueller and Cantu¹⁴ published a description of 59 catastrophic cheerleading injury cases, and 44 of these cases (75%) were fall related. Head injuries were sustained in 24 (54%) of these falls, 2 (4%) of which resulted in death. Also, concussions and closed head injuries were the most serious type of injury sustained by cheerleaders 5 to 18 years of age who were treated in US emergency departments from 1990 through 2002, accounting for 3.5% of cases.¹⁵

The potential for life-threatening head impact injuries from a fall can be minimized by increasing the shock-absorbing capacity of the surface, decreasing the height from which the person falls, or both.¹⁶ Extensive research^17–23 has been conducted on concussion in collegiate and professional football, focusing on the reconstruction of game impact biomechanics. These authors have used various biomechanical measures of head impact, such as linear acceleration, rotational acceleration, impact duration, and impact location. Greenwald et al²⁴ found that a single biomechanical measure, such as linear deceleration, was not the most sensitive biomechanical measure for determining concussion risk. However, measuring peak deceleration and the Head Injury Criterion (HIC) provides a quick and easy method to determine when a fall-related impact injury to the head may be life threatening. The HIC, defined by the National Highway Traffic Safety Administration in 1972 and used to assess head injury potential with automobile crash test dummies,²⁵ is a measure of impact severity that considers the duration over which the most critical section of the deceleration pulse persists, as well as the peak level of that deceleration.¹⁶ The formula used to calculate the HIC is presented in the American Society for Testing and Materials (ASTM) guideline F1292-04.¹⁶ Both peak deceleration and the HIC can be measured using a Triax 2000 Portable Surface Impact Tester (Alpha Automation, Trenton, NJ). The Triax 2000 is easy to use in the field and provides data that allow for comparisons of impact-absorbing capacities among various surfacing materials.

The critical height for a surfacing material is defined as an approximation of the fall height below which a life-threatening head impact injury would not be expected to occur. It is measured as the height at which g_max (defined as the multiple of g [acceleration due to gravity at the earth's surface at sea level: ie, 32.2 ft·s⁻¹·s⁻¹] that represents the maximum deceleration experienced during an impact¹⁶) is less than or equal to 200g and HIC is less than or equal to 1000.^13,16 In our study, we used critical height as an indicator of the impact-absorbing capacity of the surfacing material. When a falling object is halted by impact, the deceleration of the object rises to a peak and then decreases back to zero.¹³ The force applied to the object as it strikes the surface is directly proportional to the deceleration at any instant. If the surface is hard, such as in the case of concrete or asphalt, the time duration of the impact pulse will be short, causing the peak deceleration and the corresponding force to be high.¹³ Therefore, the critical height will be low, and the high deceleration caused by the short pulse may result in a serious head injury. In contrast, when a falling object strikes a softer surface, such as a cheerleading mat, the surface deforms upon impact, the time to bring the object to a halt is extended, the peak deceleration and corresponding force are reduced, the critical height is increased, and the likelihood of a life-threatening injury is decreased.¹³

The objectives of this study were to determine (1) the critical heights for the most common surfaces on which cheerleaders practice and perform, (2) the critical heights for non–impact-absorbing surfaces for comparison purposes, and (3) the effect of soil moisture and grass height on g_max and HIC at the critical height for a dry grass surface.

METHODS

Surfaces Tested

The following surfaces were tested to determine critical height: artificial turf (new style), asphalt, carpet, concrete, dirt, grass, landing mat, rubberized track, spring floor, traditional foam floor, vinyl tile floor, and wood gym floor. Detailed descriptions of these surfaces are presented in Table 1. A diagram illustrating the construction of a spring floor is available at http://www.theamericangym.com/Floorinfo.htm. The landing mat, spring floor, and traditional foam floor were tested at a local cheerleading gym. Carpet and the vinyl tile floor were tested in the authors' office building. All other surfaces were tested at various local outdoor sites.

Table 1.

Descriptions of Surfaces Tested

Testing Procedure for Artificial Turf and Wood Gym Floor

The artificial turf and wood gym floor tests were conducted on samples of the materials provided by a sports surfacing manufacturer. Testing was performed by placing the wood sample on a concrete surface and the artificial turf sample on a dirt surface (typical surfaces over which each would be installed) and dropping the headform onto the sample surface. We used samples of the material to test these surfaces, because dropping the headform onto surfaces installed at an athletic facility could leave permanent indentations in the surface.

Measurement of Ambient Air Temperature, Percentage of Relative Humidity, and Soil Moisture

For each surface, at the time of testing, the ambient air temperature and percentage of relative humidity were measured using a humidity and temperature pen (model 44550; Extech Instruments, Waltham, MA). For soil surfaces, the soil temperature was measured using a Luster Leaf Rapitest stainless-steel dial soil thermometer (model 1630; Luster Leaf Products, Inc, Woodstock, IL), and soil moisture content was measured using a Rapitest moisture meter (model 1820; Luster Leaf Products, Inc). Both the soil thermometer and soil moisture meter probe were inserted 3 in (0.08 m) into the soil, and the temperature and moisture content were recorded after 3 minutes, as specified by the manufacturer.

Triax 2000 Portable Surface Impact Tester

We used the Triax 2000 to perform the surface impact- attenuation tests in accordance with the ASTM specification F1292-04: “Impact Attenuation of Surfacing Materials Within the Use Zone of Playground Equipment.”¹⁶ The Triax 2000 system consists of a handheld data acquisition unit, hemispherical headform, coiled cable to connect the handheld unit and the headform, and electromagnetic holding device mounted to an adjustable-height tripod (Figure 1). The tripod allows the headform to be suspended at an accurate distance above the test surface and then precisely released to ensure an impact perpendicular to the test surface. The tripod and handheld unit combination measure the time of the drop, from release to initial impact, allowing the handheld unit to calculate and display the actual drop height and impact velocity. This feature enables the operator to be certain that the cabling did not slow the fall of the headform and is required for ASTM F1292-04 test procedure compliance. Data displayed on the handheld unit after each drop include g_max, HIC, and actual headform velocity. The maximum drop height for the Triax 2000, as stated by the manufacturer, is 12.5 ft (3.81 m). Variations of ±20% for g_max and ±40% for HIC were found during an interlaboratory study conducted by the ASTM²⁶ on 8 playground surfacing materials. For non–loose-fill materials tested during the same study, variations of ±11% for g_max and ±24% for HIC were noted.²⁶

Figure 1.

Triax 2000 (Alpha Automation, Trenton, NJ) positioned for performance of a drop test on a landing mat placed on a traditional foam floor. A, Tripod leg attachment site at the top of the tripod assembly. B, Attachment of the headform to the electromagnetic holding device. C, Handheld data acquisition unit. D, Telescoping feature of the tripod legs for height adjustment. E, Cable ratcheting system to raise and lower headform assembly.

Drop Test Procedure

Assessing the Resolution, Accuracy, Precision, and Calibration of the Triax 2000

The following calibration methods and tolerance limits were provided by the manufacturer. Before conducting the drop tests, we tested the calibration of the Triax 2000 by placing the reference pad (an 8-in [0.20-m] square rubber pad supplied by the manufacturer) on a concrete surface and performing 3 sequential headform drops from a height of 3 ft (0.91 m). The average g_max and HIC values were calculated for the second and third drops. These mean values were compared with the factory calibration data to ensure that they agreed (within ± 15%) with the factory calibration values.

In addition, the actual velocity of the headform was compared with the theoretical velocity for every drop to ensure that it did not deviate by more than ± 0.5 ft/s (0.15 m/s). If it did, the drop was repeated. The theoretical velocity was calculated using the following formula: 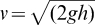, where v is the velocity at impact (ft/s), g is the acceleration due to gravity (32.2 ft·s⁻¹·s⁻¹ and h is the actual drop height (ft).

Drop Tests for Dry Surfaces

The drop tests were performed on each dry surface according to the methods specified by the “Installed Surface Performance Test (Field Test)” in ASTM F1292-04.¹⁶ Briefly, the tripod was erected on the surface, the distance between the bottom of the headform and the surface was measured with a steel tape measure, and 3 successive drops of the headform were made onto the surface. Drop height, g_max, and HIC were recorded for each drop. The actual impact velocity was compared with the theoretical velocity, and the drop was repeated if these values did not agree within ± 0.5 ft/s (0.15 m/s). The average of the g_max and HIC values for the second and third drops were calculated. This process was repeated at increasing heights until the average value for g_max was at least 200 and the average value for HIC was at least 1000. This height was designated as the critical height. The tripod, cables, and headform were kept at the settings used for the critical height drops, and the entire unit was moved to 3 additional sites. At each of these additional sites, the distance from the surface to the bottom of the headform was measured to ensure that it did not differ from the critical height by more than 0.5 in (0.01 m). Three drops were then performed, and g_max and HIC were recorded. Tests at the 3 additional sites allowed us to assess variation among the average g_max and average HIC values for the surfacing material and to ensure that the critical height for the surface was correct. We were unable to test the artificial turf and wood gym floor at 3 additional sites, because the size of the surfacing samples provided by the manufacturers was too small to accomplish this.

Assessing the Effect of Soil Moisture on g_max and HIC

Two grass surfaces were used for this assessment: 2-in (0.05-m)–high and 4-in (0.10-m)–high Kentucky bluegrass. The critical height for dry grass was determined as previously described. After we measured the soil temperature and soil moisture for the dry test site, we left the thermometer and moisture meter probe in the soil and 1 gal (3.79 L) of water was slowly poured onto the test site. The water was allowed to percolate through the soil for 5 minutes, after which the soil temperature and soil moisture were recorded for the “wet grass” surface and the thermometer and probe were removed from the soil. The critical height for the dry surface was held constant (tripod, cable, and headform positions were not changed after conducting the critical height drops), and 3 successive drops of the headform were made onto the wet surface. Drop height, g_max, and HIC were recorded for each drop. The average of the g_max and HIC values for the second and third drops was calculated. This procedure was repeated at 2 additional test sites for each of the grass surfaces. For each of the 3 test sites on each of the grass surfaces, the differences in the average g_max and HIC values between the dry and wet surfaces were calculated.

RESULTS

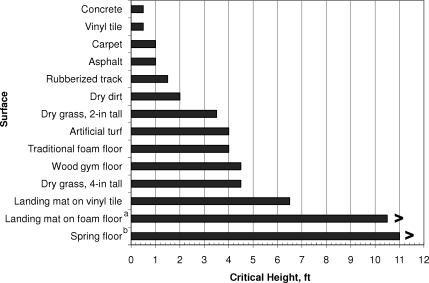
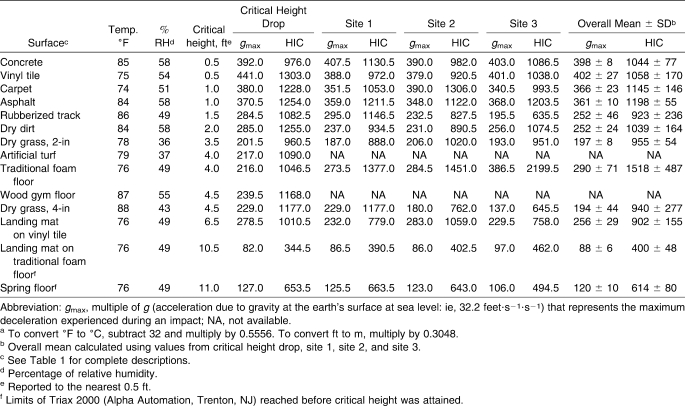
The critical height for each of the surfaces tested is presented, to the nearest 0.5 ft (0.15 m), in Figure 2. This figure is meant to be used as a quick reference guide for comparing the critical heights among the various types of surfaces. Table 2 presents the critical height, g_max, and HIC by surface type and test site, as well as the ambient temperature and percentage of relative humidity at the time of the drop tests. Critical heights ranged from 0.5 ft (0.15 m) for concrete and vinyl tile installed over concrete to more than 11 ft (3.35 m) for a spring floor. The height limit of the Triax 2000 tripod was reached before the critical height was attained for the landing mat on a traditional foam floor and the spring floor. These 2 surfaces had the highest critical heights of the cheerleading surfaces tested: more than 10.5 ft (3.20 m) and more than 11 ft (3.35 m). Although the Triax 2000 can be used at up to 12.5 ft (3.81 m), we were not able to achieve that height. When we placed the device on the spring floor with the tripod legs at maximum length and fully extended from the top of the tripod (Figure 1A and D), the maximum height that we could achieve was 11 ft (3.35 m). Although additional height could be achieved by moving the tripod legs closer to the center, the spring floor was too unstable to safely support such an arrangement. Part of this instability resulted from the construction of the telescoping legs on the tripod (Figure 1D): the thinnest of the 3 segments of each leg was at the bottom of the tripod, while the thickest was at the top. When placing the Triax over the landing mat on the traditional foam floor (Figure 1), a safe, stable configuration for the tripod could only be achieved by straddling the mat with the tripod legs. This resulted in a loss of 4 in (0.10 m) from the 11 ft (3.35 m) we were able to achieve on the spring floor. Because critical heights were reported to the nearest 0.5 ft (0.15 m), the critical height for the landing mat (10.5 ft [3.20 m]) was 0.5 ft (0.15 m) shorter than that for the spring floor (11 ft [3.35 m]).

Figure 2.

Critical height for cheerleading surfaces. ^a Landing mat on traditional foam floor. Limits of Triax 2000 were reached before critical height was attained. ^b Limits of Triax 2000 were reached before critical height was attained.

Table 2.

Ambient Conditions, Critical Height, g_max, and Head Injury Criterion (HIC) by Surface Type and Test Site^a

Ambient temperature and percentage of relative humidity are presented in Table 2 for documentation purposes only, as specified by ASTM F1292-04.¹⁶ The values for g_max and HIC varied among the 4 sites tested (critical height site and 3 additional sites) for each surface when measured at the critical height for the surface. In all cases the criterion of 200g was reached before reaching the criterion of 1000 for HIC. The overall means and SDs for g_max and HIC in Table 2 were calculated using the values from each of the 4 sites tested.

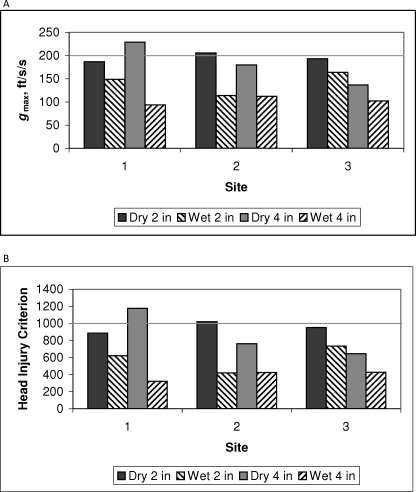
Taller grass (4 in [0.10 m]) was associated with an increase in critical height of 1 ft (0.30 m) compared with shorter grass (2 in [0.05 m]): 4.5 ft (1.37 m) and 3.5 ft (1.07 m), respectively (Figure 2). Increased values for soil moisture (wet grass) were associated with decreased values for g_max and HIC when measured at the critical height for dry grass. For short grass (2 in [0.05 m]), a mean increase in soil moisture of 3.5 ± 2.8 units on a 0 to 10 scale was associated with mean decreases in g_max and HIC of −52 ± 35 g and −361 ± 209, respectively. For tall grass (4 inches [0.10 m]), a mean increase in soil moisture of 5.5 ± 0.4 units was associated with mean decreases in g_max and HIC of −79 ± 52g and −470 ± 338, respectively. Figure 3 presents the values of g_max and HIC for dry and wet grass, as measured at the critical height for dry grass, by height of the grass and site on the grass surface. Critical heights for wet grass (tall and short) were not determined because we were not able to precisely replicate the wet grass conditions at each of the test sites on the surface while in the field. These tests would need to be performed in a laboratory setting under controlled conditions.

Figure 3.

Measured values of A, g_max (which is defined as the multiple of g [acceleration due to gravity at the earth's surface at sea level: ie, 32.2 feet·s⁻¹·s⁻¹] that represents the maximum deceleration experienced during an impact), and B, Head Injury Criterion at the critical height for dry grass, by height of grass, soil moisture, and site on the grass surface.

DISCUSSION

This is the first study to investigate the potential for brain injury on selected surfaces used by cheerleaders, employing a method used to measure the impact attenuation of surface systems under and around playground equipment.¹⁶ Cheerleading-related falls from 15 and 20 ft (4.57 and 6.10 m) have been reported.^14,27 Brain injuries, and even death, have resulted from cheerleading-related falls.¹⁴ In 2006, a collegiate cheerleader fell 15 ft (4.57 m) from a pyramid, landed on her head on a wood gym floor during a time-out performance for a basketball game, and sustained a chipped neck vertebra and a concussion.²⁸ In our study, the critical height for a wood gym floor was 4.5 ft (1.37 m). Based on our data, we believe the cheerleader should not have been performing this type of maneuver on a wood floor.

Falls onto an impact-absorbing surface are less likely to cause a serious injury than are falls onto a hard surface, and the potential for life-threatening head impact injuries can be minimized by increasing the shock-absorbing capacity of the surface, decreasing the height from which the person falls, or both.¹⁶ Bare earth, grass, asphalt, and concrete are categorized as non–impact-absorbing surfaces,⁶ yet Hutchinson²⁹ recommended that cheerleaders practice on grass rather than a hard gym floor. We found that the critical height for dry grass ranged from 3.5 to 4.5 ft (1.07–1.37 m), which is comparable with the 4.5 ft (1.37 m) for a wood gym floor (hard gym floor). Based on our data using critical height as an indicator of the impact-absorbing capacity of the surface, grass is not more impact absorbing than a wood gym floor. Therefore, the above-mentioned recommendation by Hutchinson²⁹ would not be expected to improve cheerleading safety.

Many variables can affect the impact-absorbing capacity of a surface. Examples include temperature, moisture, age of the surfacing material, and thickness of the surfacing material. We demonstrated that the height of the grass and the moisture content of the soil can affect the impact-absorbing capacity of a grass surface. In cold temperatures, the resiliency of some surfaces may decrease, thus decreasing the impact-absorbing capacity of the surface. Temperature changes may also affect moisture retention by surfaces such as soil and grass, thereby altering the impact-absorbing capacity of these surfaces.

The American Association of Cheerleading Coaches and Administrators^30,31 created rules for cheerleading safety that address the types of surfaces that are appropriate for cheerleading and that limit the height of pyramid formations and partner stunts. The rules for collegiate cheerleaders state that “technical skills should not be performed on concrete, asphalt, wet or uneven surfaces, or surfaces with obstructions.”³¹ All pyramids and partner stunts are limited to 2 persons high for high school cheerleaders³⁰ and 2 1/2 body lengths for pyramids formed by collegiate cheerleaders.³¹ Basket and elevator or sponge tosses and similar multibase tosses are prohibited on surfaces other than grass (real or artificial), a mat, or rubberized track,^30,31 and pyramids of 2 1/2 body lengths are prohibited on surfaces other than grass (real or artificial) or a mat.³¹ Based on the results of the present study, we believe that some of these rules should be revised. The critical heights reported in our study for artificial turf, grass, a 4-in (0.10-m)–thick landing mat on a vinyl tile floor, and a rubberized track are all lower than the heights that are achieved during cheerleading tosses and pyramids of 2 and 2 1/2 body lengths. Therefore, performing these maneuvers over these surfaces could place cheerleaders at risk for serious brain injury in the event of a fall. In our study, only the spring floor and a landing mat placed on a traditional foam floor were suitable for performing these maneuvers.

Data from our companion study³² illustrate the potential effectiveness of using our findings to help prevent brain injuries among cheerleaders. All the concussions reported in that study were sustained by cheerleaders who fell from higher heights than the critical heights reported in the present study for the surfaces on which they landed. These falls included a 6-ft (1.83-m) fall onto grass while performing a single-based stunt; a 5-ft (1.52-m) fall onto a wood floor while performing a single-leg stunt; a 5.5-ft (1.68-m) fall onto grass while performing a single-leg stunt; and a 6-ft fall onto artificial turf while performing a transition. It is customary to report fall heights as the distance between the surface the person was standing on before the fall and the surface the person landed on during the fall. This was the case for these 4 injury event descriptions. Therefore, the fall heights of the cheerleaders' heads in these 4 cases were actually greater than the fall heights reported. In other studies, fall height may be defined as the difference in the positions of the person's center of gravity at the start and end of the fall. Further research is needed to determine if these findings can be replicated with a larger sample size and among different populations of cheerleaders.

It is important to note that the critical heights we report in this study assume an unobstructed, vertical fall, in which the primary impact with the surface is made by the head. In some cases, a cheerleader may land on another cheerleader (spotter or base) during the fall, thus decreasing the deceleration of the head when it strikes the surface. It is also possible that another part of the body makes the primary impact with the surface and that the head makes a secondary impact. In this case, head deceleration upon impact is also reduced. Therefore, the critical height for each surface reported in our study represents a conservative estimate of the height at or below which a serious head impact injury from a fall is unlikely to occur on that surface.

Limitations

The most important limitation of our study is that the critical heights for surfaces used by cheerleaders only apply to the potential for sustaining brain injuries. Using these critical heights as guidelines to select an appropriate cheerleading surface may not ensure protection from other types of injuries. Studies^8,10,11,33 have been conducted to determine the critical height for fractures. These authors noted that fall heights higher than 2 ft (0.61 m) posed a significant risk for wrist fractures,³³ and the risk for arm fractures increased for fall heights above 3.3 ft (1.01 m) and peak deceleration above 100g.¹¹ However, none of these researchers presented conclusive data.

Another limitation of the study was the variability in the values of g_max and HIC during the calibration test and during each of the 3 sequential drop tests performed on the same surface and at the same location on the surface. According to the Triax 2000's manufacturer, up to 15% deviation from the factory calibration values for g_max and HIC during the calibration test is permissible and still ensures that the device is performing in accordance with the specifications required by ASTM F1292-04.¹⁶ Furthermore, up to 20% variation in g_max and up to 40% variation in HIC can be expected when determining the critical height for a surface.²⁶ Surface compaction, resulting from repetitive use of particular areas on a surface, may also account for some of the variability.

Several biomechanical factors (linear acceleration, rotational acceleration, impact duration, and impact location) are linked to mechanisms of concussion.^17,34–37 Angular acceleration, also known as rotational acceleration, may be more damaging to the brain than linear acceleration, even though both are present in head impact.³⁸ The Triax 2000 headform contains a triaxial accelerometer that measures only linear accelerations and the duration of these accelerations. Therefore, the HIC, as measured in the present study using the Triax 2000, does not account for the angular acceleration of the head.³⁵ Despite this limitation, HIC is a widely used predictor of brain injury, and our findings will still allow cheerleaders and cheerleading coaches to compare the relative impact-absorbing capacity of the various surfaces tested using critical height. From these comparisons, they can choose the surface that is most appropriate for the type of maneuver being performed and the maximum height expected to be achieved by the cheerleader(s) during execution of the maneuver.

Lastly, the list of cheerleading surfaces presented in this study is not comprehensive. Cheerleaders use other types of surfaces for practices and performances, and the critical heights reported in this study cannot be applied to surfaces that were not tested.

CONCLUSIONS

We are the first to investigate the potential for brain injury on selected surfaces used by cheerleaders, employing a method used to measure the impact attenuation of surface systems under and around playground equipment.¹⁶ Catastrophic injuries and death have resulted from cheerleading-related falls, and many of them involved brain injuries.¹⁴ Falls onto an impact-absorbing surface are less likely to cause a serious injury than are falls onto a hard surface, and the potential for life-threatening head impact injuries can be minimized by increasing the shock-absorbing capacity of the surface, decreasing the height from which the person falls, or both.¹⁶

We reported the critical height for selected surfaces used by cheerleaders at or below which a serious head impact injury is unlikely to occur. Cheerleaders and cheerleading coaches should use these critical heights to compare the relative impact-absorbing capacities of the various surfaces tested. With these data, the most appropriate surface for the type of maneuver to be performed can be selected, based on the maximum height expected to be achieved by the cheerleader(s) during execution of the maneuver. Cheerleaders should not perform maneuvers at heights that exceed the critical height for the surface on which they are performing. Spring floors and 4-in (0.10-m)–thick landing mats placed on traditional foam floors were the only surfaces we found to be impact absorbent enough for the performance of 2-level stunts and tosses. Cheerleaders performing at basketball and football games should restrict sideline cheering activities to chants, dances, and tumbling routines. During halftime performances, they can perform pyramids, partner stunts, and tosses if an appropriate impact-absorbing surface is available. Further research is needed to test other surfaces used by cheerleaders and to determine critical heights for other types of injuries besides brain injuries.