Hutchinson's duality: The once and future niche

Abstract

The duality between “niche” and “biotope” proposed by G. Evelyn Hutchinson provides a powerful way to conceptualize and analyze biogeographical distributions in relation to spatial environmental patterns. Both Joseph Grinnell and Charles Elton had attributed niches to environments. Attributing niches, instead, to species, allowed Hutchinson's key innovation: the formal severing of physical place from environment that is expressed by the duality. In biogeography, the physical world (a spatial extension of what Hutchinson called the biotope) is conceived as a map, each point (or cell) of which is characterized by its geographical coordinates and the local values of n environmental attributes at a given time. Exactly the same n environmental attributes define the corresponding niche space, as niche axes, allowing reciprocal projections between the geographic distribution of a species, actual or potential, past or future, and its niche. In biogeographical terms, the realized niche has come to express not only the effects of species interactions (as Hutchinson intended), but also constraints of dispersal limitation and the lack of contemporary environments corresponding to parts of the fundamental niche. Hutchinson's duality has been used to classify and map environments; model potential species distributions under past, present, and future climates; study the distributions of invasive species; discover new species; and simulate increasingly more realistic worlds, leading to spatially explicit, stochastic models that encompass speciation, extinction, range expansion, and evolutionary adaptation to changing environments.

For decades, it has been customary for ecologists to cite Hutchinson's famous Concluding remarks (1) as his most important contribution to the concept of the niche, and this practice continues, even in discussions focusing specifically on the niche (e.g., refs. 2–5). The richest source of Hutchinson's views on the niche, however, appeared much later, in his 1978 book, An Introduction to Population Biology (6). A 60-page chapter on the niche (nearly a quarter of the book) details his ideas on the subject 20 years after Concluding remarks. This masterful, but thoroughly idiosyncratic, book, cast as a “textbook” (but as far as we know rarely used as one) was a compilation of whatever Hutchinson, at age 75, considered important or interesting, not only in population and community ecology, but also in philosophy, art, literature, and aesthetics.

It is in this curious volume that we find the clearest expression of the conceptualization that we call “Hutchinson's duality,” the reciprocal correspondence between niche space and what Hutchinson called biotope space. We will show how this idea, as applied to biogeography, is transforming the way we model past, present, and future distributions of life on Earth. First, however, given the context of this article in a symposium honoring the work and influence of Joseph Grinnell (7), we take a brief look at Hutchinson's debt to Grinnell and Charles Elton.

Hutchinson, Grinnell, and Elton

The extensive footnotes in An Introduction to Population Biology (6) are a treasure trove of scholarly gems and scholarly trivia, seasoned with quotations and citations from sources in any of the five or six languages that Hutchinson had mastered, including, of course, Latin and classical Greek. On some pages, footnotes cover more space than the text; one, on the history of the concept of territoriality, covers 2½ pages. From the footnotes in the chapter on the niche, we can learn something about Hutchinson's intellectual links to Grinnell and Elton, whose differing views of the niche guided the early development of the concept (8–11) and continue to be usefully distinguished (12, 13).

In a long biographical footnote on Grinnell, Hutchinson (6) calls him “perhaps the greatest student of North American birds and mammals whom the continent has yet produced.” Hutchinson praises Grinnell not only for his acumen as a naturalist, but for his abstract conceptualization of a competitive community, in which “… the masses of different species press against one another, like soap bubbles, crowding and jostling …as one species acquires, through modification of food-getting powers and perfected adaptability to other conditions, some advantage over another” (14).

In another footnote, we learn that Hutchinson had been inspired by Elton's Animal Ecology (15), shortly after its publication. Elton's introduction of his own version of the niche concept in Animal Ecology is widely considered to be intellectually independent of Grinnell's considerably earlier (14) conception of the term (8, 11). In a footnote, however, Hutchinson points out that Elton, in Animal Ecology, referred to Grinnell and Storer's Animal Life in the Yosemite (16). Elton (15) praised the work as an “… extremely fine account … in which are given accurate data of the distribution of vertebrates in relation to life zones, together with a mass of interesting notes on the ecology of the animals.”

Rather pointedly, Hutchinson mentions that Grinnell and Storer wrote, in Animal Life in the Yosemite (16), that “each species occupies a niche of its own.” Hutchinson adds, tersely, “This statement was apparently overlooked by Elton [in litt. (in correspondence) August 26, 1976].” A tale lies implicit in this short sentence. Elton and Hutchinson were almost exact contemporaries, born in 1900 and 1903, respectively, dying 16 days apart in May, 1991, but they were not close (L. B. Slobodkin, personal communication). Nonetheless, in 1976, we may guess that Hutchinson, perhaps in preparing An Introduction to Population Biology (published 2 years later), wrote to Elton to ask whether Elton's own niche concept had been inspired by Grinnell and Storer's discussion of the niche. Apparently, Elton wrote back to say that it had not. Like Hutchinson, we will have to take Elton's word for it.

The Niche and the Biotope

Hutchinson's conception of the niche as a multidimensional volume, defined in a hyperspace with permissive conditions and requisite resources as its axes, was not simply an abstract formalization of the ideas of Grinnell, Elton, Gause, and others. Whatever the differences in their conceptualization of the niche (8, 10, 13, 17), both Grinnell and Elton already viewed the niche as an abstraction, a place or role in the environment or community that might be filled at one time or place by a certain species, and at a another time or in another place by some different species, or might even be empty in one place and filled in another.

Grinnell wrote that the niche of the California thrasher was “one of the minor niches which with their occupants all together make up the chaparral association” (18). Noting that both the arctic fox and the spotted hyena eat bird eggs and carrion, Elton (15) concluded that the fox and the hyena each occupy “the same two niches,” the egg-eater niche and the carrion-feeder niche. Thus Elton, but not Grinnell, considered not only that one species might occupy two niches, but also that a single niche might be occupied by more than one species, presumably even in the same community: “… we might take as a niche all of the carnivores that prey on small mammals” (such as weasels and foxes) or all of the animals that browse on live corals (such as parrotfish and holothurians) (15). For both Grinnell and Elton, although the niche was abstract, particular niches were nonetheless attributes of particular environments (chaparral, grassland, or coral reefs) and thereby associated with particular places.

Without losing any of the utility of these ideas, Hutchinson (1, 6) transformed them and turned them on their head (2) by declaring the niche an attribute of a population or species, in relation to its environment, both abiotic and biotic (8–10). No more empty niches (any imaginative naturalist can dream up any number of such plausible angels), although empty niche space still made sense (as we will discuss later). In Hutchinson's view, ecological and evolutionary opportunities take the form of environmental conditions, available resources, and fitness gradients, not vacant niches. There would be no more niche double-dipping by the arctic fox or the spotted hyena and no more niche cohabitation by weasels and foxes. By definition, each population or species has its own niche and only one niche (except in the case of polymorphisms). The placental mole and the marsupial mole, famous for their evolutionary convergence, do not share the same niche, by Hutchinson's definition, but their niches may be remarkable for their similarity.

This transformation had a profound and enduring consequence: Hutchinson's redefinition of the niche severed all direct ties between the physical world of particular places and the abstract hyperspace in which niches were to be defined and modeled. The California thrasher and the arctic fox still lived in their accustomed habitats, in their accustomed communities in the physical world, but their niches had been hijacked into hyperspace. Hutchinson resolved this disjunction by defining a duality, a reciprocal correspondence between multidimensional niche space and the physical spaces in which species live. In mathematics, a duality is a formal, reciprocal way of translating one system of concepts or constructs into a different system. Although we do not pretend to apply the concept of duality in any formal mathematical way, it is worth noting that, in some mathematical dualities (for example, in the study of topological vector spaces; ref. 19), the correspondence between a representation and its dual need not be one-to-one; the dual of the dual may be broader or narrower than the original representation. This partial reciprocity proves to be a characteristic and powerful feature of the niche–biotope duality.

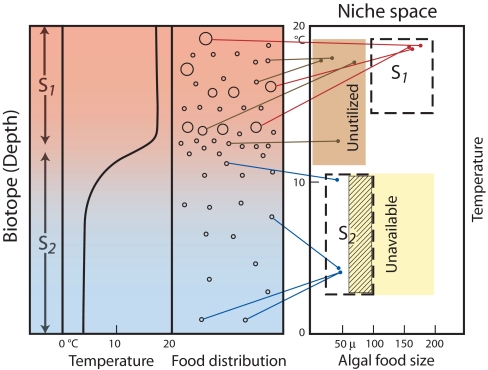
In 1957 Hutchinson (1) called the physical space biotope space or simply the biotope, “ordinary physical space,” the physical setting of an ecosystem, such as a lake or a forest. In 1978 (6), he wrote: “A biotope is any segment of the biosphere with convenient… boundaries.” The archetype of the niche–biotope duality is a diagram that Hutchinson published in each of three decades (1, 6, 20), with increasing clarity and simplicity. Fig. 1 is based closely on the version of this figure from his 1978 book (6). Not surprisingly, from “the father of American limnology,” the biotope in this example is a lake. The niche space has two axes, one a condition and one a resource [or one “scenopoetic” and the other “bionomic,” as Hutchinson (6) and Soberón (13) prefer]: water temperature and algal food particle size. The niches of two hypothetical species of microalgae-eating creatures, perhaps cladocerans, appear in the niche space, charismatically named S₁ and S₂. The former is a warm-water species that feeds on large algae, and the latter is a cool-water species that feeds on small algae. Their vertical distributions in the water column are shown in Fig. 1.

Fig. 1.

Hutchinson's illustration of the niche-biotope duality for a temperate lake with algae and two consumers of algae (species S₁ and S₂). The 1D biotope (red and blue rectangle) is a stratified water column with a strong summer thermocline. The two environmental factors characterizing the biotope (water temperature and food size, both as a function of depth) correspond to niche axes in niche space shown on the right. Red (S₁) and blue (S₂) lines connect representative algal cells of different sizes and at different depths with their corresponding points in the niches of the two consumers. The distribution of species S₁ and S₂ in the water column is the projection of their niches on the biotope. Brown lines show algae that map onto an unutilized region of niche space (small algae in warm water). The yellow region of niche space (large algae living in cold water) is unavailable (not represented in this biotope). The cross-hatched portion of the niche of consumer S₂, which overlaps unavailable niche space, is unexpressed in the biotope. Based on Hutchinson's figure 101 in ref. 6.

The lake biotope is depicted by a single dimension, depth, as a thermally stratified water column, with a strong summer thermocline producing a nonlinear decrease in temperature with depth (Fig. 1). Adjacent to the temperature plot in Fig. 1 is a graphical depiction of the frequency distribution of three sizes (species or groups of species, presumably) of algal cells as a function of depth. All three sizes are present above the thermocline, but only the smallest of the three sizes is found below it. The blue, red, and brown lines connect representative algal cells of different sizes and different water temperatures (different depths) with their corresponding positions in niche space. The blue and red lines show food-size/temperature combinations that map, respectively, within the niche of consumers S₁ or S₂. Notice that algae of a single size, but found at different depths, map to the same part of niche space because of nearly constant temperatures above (or below) the thermocline.

In Fig. 1 the brown lines indicate food–size/temperature combinations that map onto the brown area of niche space, which is unutilized by consumers in this lake: no warm-water consumer of small algae is present to exploit this region of niche space. The yellow region of niche space, large algae living in cold water, may be called unavailable because it is not represented in this biotope. Because the niche of consumer S₂ includes part of this unavailable region (presumably based on data from other lakes or on laboratory experiments), the cross-hatched part of its niche is unexpressed in the biotope. As Hutchinson (6) put it, “Any point in niche space can correspond to many points in the biotope, but not all points in niche space are represented in any given biotope.” Finally, notice that the separation between the niches of the two consumers on the temperature axis in niche space projects into the biotope as a gap between the vertical distributions of the two species in the water column (Fig. 1). The distributional gap is small because the thermocline is steep.

With this simple, but wisely, crafted example, Hutchinson was able to show features characteristic of almost any real-world example of the niche–biotope duality, each of which, we will show, has exact counterparts in biogeographical applications of the duality: (i) reciprocal mapping between distributions in the biotope and niches in niche space, (ii) roles for both conditions and resources in defining niches, (iii) nonlinear correspondence between niche axes and dimensions of physical space, (iv) one-to-many projections of points in niche space onto the biotope, (v) unutilized regions of available niche space that correspond to unexploited opportunities in the biotope, (vi) regions of niche space that are unavailable (not represented) in the biotope, and (vii) portions of niches that are unexpressed in the biotope. The last four of these features are specific forms of partial reciprocity in the niche–biotope duality.

Extending the Duality to Biogeography

Conceptually, extending Hutchinson's duality to biogeographical scales is straightforward. On large spatial scales, the biotope (in our extended usage, far grander than what Hutchinson had in mind) is represented as a map, each point (or cell) of which is characterized by its geographical coordinates and by the local values of n environmental attributes (which may be envisioned as n geographic information system map layers). Exactly the same n environmental attributes define the corresponding niche space, as niche axes. Just as in Hutchinson's original conception, the duality works both ways. Points or regions of the n-dimensional niche space can be projected onto the geographical space (biotope space), guided by the n environmental layers of the map. Moreover, as in Hutchinson's lake example (Fig. 1), the rules that define the duality are not reciprocal. Although each point in the biotope (at a given time) corresponds to exactly one point in niche space, a single point in niche space may correspond closely to many in the biotope or to none. Indeed, if the duality were strictly one-to-one, it would have little utility at all, as we will show by examples. It might be objected that, with a large enough n and sufficient precision, the chance of one point in niche space mapping to more than a single point in the biotope is very small so that, strictly speaking, the duality is one-to-one. But at biologically meaningful levels of precision and spatial scale the rule stands: a region of niche space may correspond to more than one place in the biotope. This rule is pivotal to the reciprocity between niche and distribution.

Notice that we have not yet mentioned niches or geographical distributions (or the species they belong to), with regard to biogeography, only niche space and biotope. In fact, this aspect of the duality has been used, reciprocally, to characterize, classify, and map ecoregions or climate zones on geographical scales and project them in time, without any reference to species. For example, Saxon et al. (21) treated climatic, edaphic, and topographic variables for the United States and western Canada, mapped on a 5-km grid scale, as orthogonal axes in niche space. Each geographic map cell (the biotope) corresponded to a single point in this niche space. Using a clustering algorithm, they defined nonoverlapping regions of niche space (“environmental domains”) that, collectively, included all of the points from the map. In the final step, each of the domains in niche space was projected back onto geographical (biotope) space, classifying each grid cell according to its membership in one of the environmental domains. Using projected climate maps under alternative Intergovernmental Panel on Climate Change scenarios, the study predicted geographic shifts in environmental domains in terms of novel and disappearing environments. Hargrove and Hoffman (22) projected ecoregion distributions not only for future climates but for past climates.

Among the earliest rigorous studies that linked the biogeographical distribution of individual species explicitly with their niches in multivariate niche space were Green's (23) classic study of bivalve mollusks in Canadian lakes in 1971 and Austin et al.'s 1984 (24) study of the “qualitative environmental realized niche” of several Eucalyptus species in Australia, based on georeferenced specimen and environmental data. By defining “available niche space” as the union of the sets of conditions in all lakes (plotted on discriminant function axes), in relation to species' niches in particular lakes, Green suggested than one might be able to tell where introductions of particular species would fail or succeed. In doing so, he touched on the partial reciprocity of Hutchinson's duality (in this case, projection from limited geographical space into niche space and back to a broader geographical space) and anticipated by decades the use of the duality to predict the spread of invasive species (e.g., refs. 25 and 26). Austin et al.'s 3D niche graphs, with horizontal slices through a temperature x rainfall plot at increasing levels of solar radiation, show not only the niches of each species but (as in Green's study) the available niche space representing conditions in the biotope for the entire multispecies dataset. The niche response surfaces strongly suggested that the niches of some of the species that Austin et al. studied may not be fully expressed in existing environments, another form of partial reciprocity, first formalized by Hutchinson (Fig. 1), with crucial contemporary implications for invasive species and climate change (e.g., refs. 25–28).

Hutchinson's duality lies at the heart of the enterprise in species distribution modeling, in all its forms (26, 29–32). The duality is at work when modelers use the environmental characteristics of the places that a species now occurs to model its niche and project its potential distribution in environmentally similar places, including places that descendants may occur in the future (e.g., refs. 25–27 and 33), under climate change; that ancestors probably occurred in the past (e.g., refs. 34 and 35); or that as-yet-undetected individuals of the species or its close relatives live in the present (e.g., ref. 36). The duality is most obvious in the use of methods such as BIOCLIM (37), in which environmental envelopes in niche space, based on georeferenced presence data, are explicitly reprojected back into geographical space. But even the most sophisticated methods (30, 31) of species distribution modeling assume the duality implicitly. As we discuss in the following sections, however, the application of the duality in species distribution modeling requires us to think clearly about whether distributions fully reveal the ecological potential of the current gene pool [often expressed by assuming that species are “in equilibrium” with environment (e.g., ref. 38)], whether the niche is changing in time, and whether geographic distributions reflect the constraints of dispersal limitation.

Fundamental and Realized Niches and Distributions in the Biotope

It is not likely to have escaped the reader's notice that, so far, we have not once mentioned realized vs. fundamental niches, Hutchinson's (1, 6) daunting distinction between the niche with vs. without taking into account the effects of species interactions.* The reason we postponed this issue is simple: Hutchinson's duality, the reciprocal correspondence between niche space and geographic space, neither requires nor depends on the distinction between realized and fundamental niches for its utility and conceptual power.^† In fact, the distinction between fundamental and realized niches itself depends crucially on the duality between niche and biotope. Competition, predation, and mutualism, as processes that may shape spatial (including geographical) distributions, happen in the physical world, the biotope. But the effect of these processes on the realized niche can be appreciated only when the distribution expressed in the biotope is projected back into niche space, often in a conditional or spatially dependent manner, and compared with some estimate of the fundamental niche.

Living food resources, consumable abiotic resources, competitors, natural enemies, and mutualistic partners are among the factors that Hutchinson (1) called bionomic and that capture information from the biotope that might distinguish fundamental from realized niches in niche space. The algal food size niche axis in Fig. 1 exemplifies such factors, but it also suggests the difficulties in treating bionomic factors as simple niche axes. Because the algae have their own population dynamics, modeling the realized niches of algae-eating species 1 and species 2 requires modeling the algae dynamically as well, as argued persuasively by Chase and Leibold (11). Bionomic resources are characterized by dynamic linkage to species numbers (13). In contrast, climatic, topographic, edaphic, and other environmental conditions that cannot be consumed but “set the scene” are “scenopoetic” niche axes (6, 13).

In species distribution modeling, as it is currently practiced, conditions (scenopoetic factors) are represented spatially in geographical (biotope) space and projected into niche space. But that does not mean that the niche thus defined is a fundamental niche, just because bionomic axes are not included in the model. Any niche defined in niche space based on observed distributions of species in the physical world is, at best, a realized niche, unless demonstrated otherwise. Experimental studies and physiological models have the potential to estimate the limits of fundamental niches (e.g., refs. 41 and 42), but the delineation of niches in niche space based simply on distributions in the biotope cannot be counted on to do so. No geographic distribution can be assumed, without careful study, either to be unaffected by species interactions or to demonstrate their effect (13, 43, 44).

Even if we had divine knowledge that species interactions had no effect in constraining or extending the current geographical distribution of a species, the full extent of its fundamental niche might not be revealed by the environmental conditions that characterize its range, because of the partial reciprocity of the niche–biotope duality (45). On a smaller spatial scale, Hutchinson (6) made this point with his lake example (Fig. 1), which intentionally excludes competitive species interactions. The yellow region of niche space (large food particles below the summer thermocline in Fig. 1) is unavailable in the lake. Because the yellow region in Fig. 1 includes the cross-hatched portion of the fundamental niche of consumer species S₂, there is no way to infer the full fundamental niche of this species from its distribution in the lake. Part of the fundamental niche remains unexpressed, at least, in this particular lake at this particular time.

On a geographical scale, other lakes might offer large food particles in deep water, but species S₂ might or might not have managed to colonize those lakes or did so but became locally extinct. Dispersal limitation among discontinuous habitats can, of course, restrict the geographical range of a species, leaving regions of the biotope unoccupied that correspond to portions the fundamental niche of the species in niche space (e.g., refs. 2, 13, and 46), including appropriate habitats where local extinction has not been reversed by recolonization. The worldwide assault by introduced species makes clear the importance of dispersal limitation (e.g., refs. 25 and 26). Finally, some regions of the fundamental niche may not correspond to any contemporary biotope (42, 47, 48). In the example of Fig. 1, this would mean, for species S₂, that the combination of water temperature and food particle size represented by the cross-hatched area is not available in any contemporary lake. Jackson and Overpeck (45) introduced the useful term “potential niche” for those regions of the fundamental niche that correspond to combinations of environmental variables that actually exist somewhere in the biotope, at a specified time.

When observed geographical distributions fail to reveal the full extent of the fundamental niche in niche space, not because of species interactions, but because of either dispersal limitation or portions of the fundamental niche do not correspond to any region of the contemporary biotope, what do we call the niche that corresponds, in niche space, to the observed distribution? Hutchinson would probably not have approved, but the best option appears be to extend the term “realized niche” to these cases. Jackson and Overpeck (45) take this approach by including dispersal limitation among the possible causes of the difference between the realized and fundamental niche, and by defining the realized niche as the projection in niche space of the observed geographical distribution of a species [a practice dating at least to Austin et al.'s early work (24)].

In summary, the scope of environmental conditions that characterize the observed geographic distribution of a species may fail to reveal the full extent of its fundamental niche for three completely different reasons: (i) portions of a species' fundamental niche may be unexpressed because they do not correspond to any contemporary biotope; (ii) every part of the fundamental niche might have a correspondence somewhere in the current biotope, but dispersal limitation prevents the species from occupying certain environmentally distinct patches; or (iii) the potential geographical range is truncated by constraining species interactions. Only the last of these three mechanisms has anything to do with the orthodox distinction between fundamental and realized niches, but all three have come to be considered as defining the limits of the realized niche in biogeography.

Niche Evolution and Range Dynamics: The Duality in Time

Useful as it is as a way to conceptualize relationships between organisms and the environment at a moment in time, Hutchinson's niche–biotope duality proves its true worth to biogeography in the context of ecological and evolutionary dynamics (e.g., refs. 5, 26, 27, 33–35, 42, and 45–50). The duality separates what happens to the geographical distribution of a species in a changing physical world from the causes and consequences of these changes for the species as whole, as modeled in niche space. The many-to-one link from biotope to niche space unifies information from shifting, fragmented, and dispersal-limited ranges, whereas the niche-to-biotope link identifies which parts of the fundamental niche are unexpressed and which regions of the biotope are appropriate, but unoccupied, as the niche evolves or the biotope changes.^‡

As time passes, population processes, including dispersal, local extinctions, and species interactions, can potentially alter a geographical range without any change in the fundamental niche. Likewise, environmental changes, whether progressive or cyclical (on annual to multimillennial scales), can potentially alter ranges without any change in the fundamental niche. As the idealization of a species' performance under optimal conditions and with optimal resources, the fundamental niche can be altered only through evolutionary change, which may affect its position, shape, size, and fitness contours (5, 12, 51–53). The biotope, the physical world, is of course the setting for all evolutionary changes, but the duality projects these changes into niche space. Over evolutionary time periods, the niche moves through niche hyperspace like a hyperfish in a hypertank, expanding and contracting in response fitness changes owing to mutation, selection, and genetic drift under changing conditions in the biotope. Meanwhile, the corresponding geographical distribution may change dramatically, minimally, or, in principle, not at all [the biogeographical version of the Red Queen, who runs as fast as she can just to stay in place (54)].

Niche Adaptation vs. Niche Conservatism in a Changing Climate.

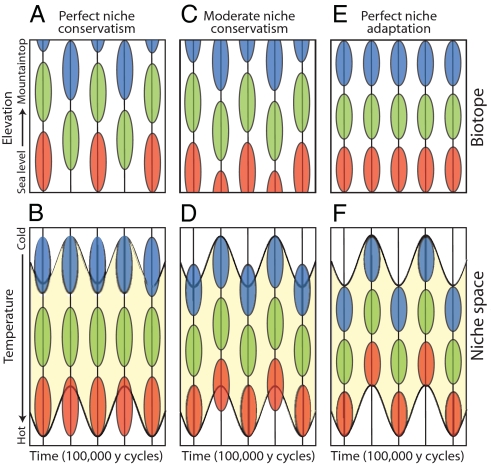
The range of possibilities is captured in Fig. 2, which illustrates the niche–biotope duality for three hypothetical species (red, green, and blue) distributed along an elevational gradient, during a series of 100,000-year glacial cycles. Fig. 2 A, C, and E shows geographical distributions (biotope) from sea level (bottom) to mountaintop (top), and Fig. 2 B, D, and F illustrates the projection of these elevational ranges in a niche space of one dimension: mean annual temperature. (To make the mapping between biotope and niche space easier to visualize, the temperature axis runs from cold at the top to hot at the bottom of the lower graphs, in parallel with temperature on the elevational gradient in the upper graphs.)

Fig. 2.

The effect of niche conservatism on the niche–biotope duality for three hypothetical species (red, green, and blue), distributed along an elevational gradient, during a series of 100,000-year glacial cycles. A, C, and E show geographical distributions (biotope) from sea level (bottom) to mountaintop (top), and B, D, and F illustrate the projection of these elevational ranges on a temperature axis, assuming a linear decline in temperature with elevation (linear lapse rate). Note that the temperature axis in B, D, and F runs from cold at the top to hot at the bottom, and that the vertical lines are half-cycles, 50,000 years apart. The yellow region outlines the “climate space” (45) available in the biotope during sequential warm interglacial and cold glacial episodes. With perfect niche conservatism (A and B), niches are do not evolve, so that ranges move in synchrony with thermal zones on the mountainside. In contrast, with perfect niche adaptation (E and F), ranges are static and niches shuffle back and forth in niche space by evolutionary change. C and D (moderate niche conservatism) show a more realistic balance between adaptation and conservatism.

In Fig. 2 A and B the fundamental niches (temperature tolerances) of the three species are fixed over time [perfect niche conservatism, in the sense of Pearman et al. (5)], and their distributions simply track thermal zones up and down the mountain as the climate warms and cools. In contrast, in Fig. 2 E and F, elevational ranges on the mountainside remain fixed, despite cyclic temperature changes at all elevations, by means of perfect adaptive evolution of the niche to changing temperature (the biogeographical Red Queen). Fig. 2 C and D shows a more realistic mix of conservatism and adaptation, although just where real species lie on this spectrum is a matter of current discussion and research (e.g., refs. 51, 52, and 55).

The biotope and niche patterns in Fig. 2 illustrate an issue of intense current interest (27), as the global climate warms rapidly, already within 1 °C of the warmest it has been in at least the last million years (56). In the biotope panels for perfect or moderate niche conservatism (Fig. 2 A and C), notice the truncated distributions of the blue species, as ranges move up the mountain in warm interglacials, and of the red species, as range move down with cooling climate. Elevational ranges (and thus populations) contract, alternately, at both extremes, illustrating the potential for mountaintop extinctions during warm interglacials (at any latitude; e.g., ref. 50), and for sea-level extinctions during glacial maxima at tropical latitudes, where there is nowhere warmer to go (57).

In the corresponding niche panels for perfect and moderate niche conservatism (Fig. 2 B and D), it is the niches that are truncated (not fully expressed), as extreme environmental conditions (the hottest lowland or coldest mountaintop climates) alternately appear and disappear. How long do species retain adaptations to past climatic conditions, in the absence of stabilizing selection and in the possible presence of opposing directional selection? In the current climate, have lowland tropical species retained upper niche limits for heat tolerance from previous warm Pleistocene interglacials, or even from the Miocene or Eocene, when conditions were last substantially warmer than at present (27, 28)? If not, then biotic attrition in the lowland tropics may be in the works, as some lowland species either move upslope (e.g., ref. 58) or fail to survive or adapt to warmer lowland temperatures in the tropics (59), although many may persist in the lowlands in cooler and wetter refugia (57). Hutchinson's duality allows us to conceptualize these questions more precisely (e.g., ref. 60) than a simple narrative approach.

Invasive Species.

The widely used application of Hutchinson's duality to the study of invasive species (26) echoes many of the same themes discussed above for climate change, which, itself, may be expected to play a role in biological invasions, current and predicted (e.g., refs. 61 and 62). Like range shifts in a changing climate, the spread of an invasive species may advance as a simple demographic response to opportunity, colonizing geographical locations where conditions correspond to regions in niche space that lie within the fundamental niche set by the gene pool in its native range (niche conservatism). However, adaptive evolution in the invasive range may alter the fundamental niche, particularly when the invasive range becomes large enough to cover a wide spectrum of conditions. For example, de novo adaptation is suspected in the case of cane toads [Chaunus (Bufo) marinus] in Australia, introduced in 1935 from tropical America. Urban et al. (63) modeled the recent expansion of the range margin based on historical records and current climatic and landscape factors, demonstrating a rapid increase in the pace of range expansion in the toad's northern range, while the expansion of southern populations slowed. Philips et al. (64) implicated selection for longer limbs at the advancing front for this population.

By modeling the realized niche of spotted knapweed (Centaurea maculosa) in both its native European and invasive North American ranges, Broennimann and colleagues (25, 62) showed that, while conditions in the locality of introduction in North America lie squarely within the niche of the European populations, the full geographical range of the invasive population includes regions of niche space, particularly drier climates, not currently occupied by knapweed in Europe. Whether the fundamental niche of the European population encompasses a latent capacity for life in dryer climates or the North American population has expanded the fundamental niche of the species by adapting to drier climates remains unsettled and will require careful experiments.

Theoretical models often assume that optimal environmental conditions for species correspond to the center of a species' niche (65), and spatially explicit models of species abundance on simple environmental gradients predict that abundance should peak at the center of species' range (e.g., ref. 66). The complexity of the duality between irregular niches and heterogeneous biotopes, as demonstrated by the cane toad and spotted knapweed, however, suggest that reality is likely to be more complex (29), particularly when niche evolution may be playing a role.

Using the Duality to Discover Species

When niche conservatism constrains the ecological divergence of closely related species, or even as a simple consequence of “phylogenetic signal” (55), niche models based on the known geographical distribution of a focal species may help guide the discovery of previously unsuspected locations in the biotope corresponding to conditions that lie within its niche. What may be found there depends on the circumstances: previously undocumented populations of the focal species, potential habitats for introduction and spread of the focal species, or even previously unknown phylogenetic relatives of the focal species. For example, using historical collection data, Raxworthy et al. (36) modeled the niche of 11 species of Madagascan endemic chameleon species, based on 29 remotely sensed map layers (climate, topography, hydrology, and land cover) for Madagascar. The study might have been just another predictive species distribution modeling exercise had Raxworthy et al. not validated their predictions against independent occurrence data from 11 additional, recent inventories. When the modeled niche for each species was projected from niche space back into geographical space, the predicted species distribution closely matched the species distribution that was observed in the more recent, independent inventories. Remarkably, subsequent field surveys in two largely unexplored areas that were predicted by the models to be especially rich in chameleon species discovered seven species of chameleons new to science.

In the sea, water temperatures and landmasses set dispersal barriers for seaweed (macroalgae). Verbruggen et al. (67), using tools from both macroevolution and macroecology, developed a phylogenetic hypothesis for all known species of Halimeda and modeled the global potential distribution of each species. Based on hotspots of suitability, they predicted the probable Eastern Pacific localities of hypothetical, but as-yet-undiscovered sister species of known Caribbean Halimeda, originating from genetic isolation imposed by the Pliocene rise of the Central American Isthmus.

Hutchinson's Duality in Dynamic, Biogeographical Simulations

The niche–biotope duality has proven itself a crucial conceptual tool in building stochastic simulation models to study patterns in species richness that emerge from fundamental evolutionary and biogeographical processes. In simulation models, the effects and interactions of these processes can be isolated in ways that statistical models, based on purely empirical data, do not easily permit.

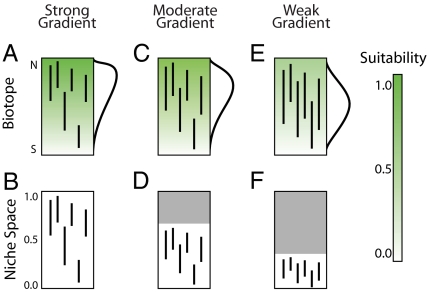
For example, Rangel and Diniz-Filho (68) modeled speciation, range expansion, and extinction in a 1D bounded biotope (called a geographical domain in the stochastic modeling literature) (Fig. 3A, C, and E). In their model, an environmental gradient of “suitability” increases linearly from one end of the domain to the other (the green shading in Fig. 3 A, C, and E). The difference in suitability between the least suitable (whitest) and most suitable (greenest) ends of the gradient determines its strength, which decreases in Fig. 3 from A to C to E. Weaker gradients in the biotope correspond to smaller regions of niche space (the white portions of Fig. 3 B, D, and F). Niches (vertical line segments in niche space) correspond one-to-one with distributions (vertical line segments in the biotope), which sum to species richness curves on the right side of Fig. 3 A, C, and E. In the simulation, stochastic speciation rate and the direction of range expansion are, by design, correlated with environmental suitability.

Fig. 3.

Stochastic simulation of species distributions in three biotopes (A, C, and E) and in the corresponding niche space (B, D, and F). Biotopes are characterized by a south–north linear gradient of environmental suitability (intensity of green). Shaded areas of niche space in D and F represent environmental conditions not present in the corresponding biotopes (C and E). After many cycles of random speciation and extinction (see ref. 67 for details), species richness (curves on the right side of the biotopes) peaks closer to the more suitable end of the gradient when the suitability gradient is stronger (A). With weaker environmental gradients, species increasingly overlap in the middle of the biotope (geometric constraints on range location are stronger), and a clear middomain pattern of richness arises (C and E).

The conventional prediction, that speciation and extinction dynamics should lead to a peak of richness at the “more suitable” end of the domain (e.g., ref. 69), is realized only when the environmental gradient is very strong (Fig. 3A), forcing species to have small ranges, given their environmental tolerances in niche space (Fig. 3B). As the environmental gradient weakens, ranges become larger (65) and are thus increasingly constrained in their location on the gradient. With decreasing gradient strength, the richness peak shifts toward the center of the gradient (compare Fig. 3 A, C, and E) because larger ranges increasingly overlap toward middomain (the middomain effect; refs. 70 and 71), as geometric constraints on range location become stronger than direct effects of the environmental gradient on richness patterns. This simple application of Hutchinson's duality offers a mechanistic hypothesis for widespread empirical patterns on bounded environmental gradients (72).

More realism (and more complexity) can be added by replacing the simple 1D biotope of the previous model, with its static environmental gradient, by a spatially heterogeneous, multifactor, fluctuating environment in two geographic dimensions, together with a corresponding multidimensional niche space. In Rangel et al.'s (53) model (Fig. S1), each map cell is characterized by an array of environmental variables, which collectively define a spatially heterogeneous pattern within the biotope, where ranges expand and fragment under environmental change and where speciation and extinction are played out. As always, with the niche–biotope duality, the same environmental variables serve as the axes of niche space (not shown in Fig. S1), in which niche evolution moves and shape niches according to the parameters of the model.

Rangel et al. (53) used this model to show how niche shifts through evolutionary time affect spatial patterns in species richness. When species cannot readily adapt to local environmental conditions (niche conservatism), they accumulate in regions that are environmentally similar to those occupied by their ancestors. On a spatially autocorrelated map, those regions tend to be geographically closer to the center of origin. In contrast, if species readily evolve through niche space to exploit different environmental conditions, they can colonize regions that were environmentally unsuitable to their ancestors, geographically further from their ancestors' range. Using South American birds as a model system, Rangel et al. (53) showed that niche evolutionary dynamics and the geographical center of origin strongly affect spatial patterns in species richness. Hutchinson's duality plays a continual, explicit role in both the conceptualization and the implementation of this temporally and spatially dynamic biogeographical model.

The Once and Future Niche: Retrospect and Prospect

Some conceptual tools serve a purpose and then die a graceful death of neglect, others prove to be misleading (or worse) and are dismembered by detractors, and yet others undergo metamorphosis or even death and reincarnation as they are put to new uses by new generations. The niche concept, now a century old, has a history that falls somewhere between metamorphosis and reincarnation. After a slow and steady increase in the literature during the first half of the 20th century (11), the niche concept, as a conceptual framework for ecological and evolutionary research, underwent a meteoric rise in usage between 1950 and the mid-1980s, when the number of papers with niche in the title or text peaked.^§

Hutchinson's (1) formalization of the niche was surely a force behind this midcentury success, driven in large part by the linkage between niche and interspecific competition that he championed. Once the evidentiary bar for competition was raised by Simberloff (e.g., ref. 73) and others in the 1980s, however, the sometimes overly facile use the niche concept declined steeply by association with untested presumptions about competition. Although community ecologists (e.g., ref. 74) kept the niche alive and still do (11, 75), accelerating rates of application in biogeography and conservation biology have also given new life to the niche. When Hutchinson (1) sliced the Gordian knot between niche and place, he created a powerful conceptual structure that continues to find new uses in biogeography, quite apart from the degree to which competition does or does not structure communities.

Indeed, a prospective view of further application of the niche concept (and of Hutchinson's duality in particular) in biogeography reveals a number of exciting growing points. Species distribution modeling, which relies fundamentally on the duality, is a rapidly developing enterprise with strong links to conservation biology, invasive species ecology, paleoecology, and climate change biology that offers novel approaches to classical questions in biogeography (e.g., refs. 21, 25, 33–35, 46, 61, 63, and 75). Although considerably more demanding of time and resources than working with distributional data, integrating experimental approaches to defining niche limits with physiologically based, dynamic distribution models, by means of the duality, offers a rigorous path forward (e.g., refs. 41, 42, 76, and 77). Stochastic models in biogeography, built explicitly on the duality, are increasingly mechanistic and increasingly evolutionary, producing simulated phylogenies and simulated biogeographical patterns that can be assessed against empirical benchmarks (53, 78). The integration of macroevolutionary and niche perspectives (e.g., refs. 35 and 66) has begun to bring the niche, and the power of the duality, to new generations of evolutionary biologists. The integration of macroevolutionary and macroecoclogical approaches with stochastic biogeographical models, while still on the horizon, promises a potent synthesis. In all of these endeavors, a explicit recognition and exploitation of the duality, and the partial reciprocity, between niche and biotope that we have emphasized throughout this article promises to both clarify and unite these growing points in biogeography.