Skip to main content
PMC home page
NIHPA Author Manuscripts logoLink to NIHPA Author Manuscripts
. Author manuscript; available in PMC: 2010 Nov 13.
Published in final edited form as: J Biomech. 2009 Aug 7;42(15):2535–2539. doi: 10.1016/j.jbiomech.2009.07.009

An Experimental Study on the Ultimate Strength of the Adventitia and Media of Human Atherosclerotic Carotid Arteries in Circumferential and Axial Directions

Zhongzhao Teng 1, Dalin Tang 1,*, Jie Zheng 2, Pamela K Woodard 2, Allen H Hoffman 3
PMCID: PMC2783388  NIHMSID: NIHMS133222  PMID: 19665126

Abstract

Atherosclerotic plaque may rupture without warning causing heart attack or stroke. Knowledge of the ultimate strength of human atherosclerotic tissues is essential for understanding the rupture mechanism and predicting cardiovascular events. Despite its great importance, experimental data on ultimate strength of human atherosclerotic carotid artery remains very sparse. This study determined the uniaxial tensile strength of human carotid artery sections containing type II and III lesions (AHA classifications). Axial and circumferential oriented adventitia, media and intact specimens (total=73) were prepared from 6 arteries. The ultimate strength in uniaxial tension was taken as the peak stress recorded when the specimen showed the first evidence of failure and the extensibility was taken as the stretch ratio at failure. The mean adventitia strength values calculated using the 1st Piola-Kirchoff stress were 1996±867kPa and 1802±703kPa in the axial and circumferential directions respectively, while the corresponding values for the media sections were 519±270kPa and 1230±533kPa. The intact specimens showed ultimate strengths similar to media in circumferential direction but were twice as strong as the media in the axial direction. Results also indicated that adventitia, media and intact specimens exhibited similar extensibility at failure, in both the axial and circumferential directions (stretch ratio 1.50 +/−0.22). These measurements of the material strength limits for human atherosclerotic carotid arteries could be useful in improving computational models that assess plaque vulnerability.

Keywords: atherosclerosis, carotid artery, plaque, ultimate strength, rupture

1. Introduction

Atherosclerosis is the most common disease of the arterial wall. The abrupt closure of an artery by an occlusive thrombus is the main cause of myocardial infarction and other thrombotic consequences of atherosclerosis. The thrombosis is often associated with rupture of fibrous cap covering a large lipid-rich necrotic core (Rekhter et al., 1998; Cullen et al., 2003). Histology has shown that most rupture sites are also sites of increased mechanical stress (Cullen et al., 2003; Li et al., 2006). It has been widely accepted that atherosclerosis leads to locally increased stresses in the region of lesions. However, validation of this hypothesis has been impeded by a lack of experimental data on ultimate strength (i.e. the maximum value of stress at failure) of atherosclerotic tissues. Knowledge of ultimate strength of human atherosclerotic tissues is essential for understanding plaque rupture mechanisms and assessing the risk of possible ruptures (Tang et al., 2005). It is also of great importance in predicting the outcome of interventional treatments such as balloon angioplasty (Holzapfel et al., 2002).

Available experimental data for the ultimate strength of human atherosclerotic arteries is very limited. Most research efforts have focused on quantifying the stress-strain relationship of atherosclerotic tissues over physiological loading ranges. Lee (1991) and Loree (1994) et al. performed dynamic and static tensile tests on plaque caps from abdominal and thoracic aortas. These results indicated that stiffness of cap increased with loading frequency, calcified cap was stiffest and the circumferential tangential modulus at 25kPa did not depend on the cap type. Lendon et al. (1991, 1993) showed that the weaker plaque caps appear to be associated with increased macrophage activity. Holzapfel et al. (2004) investigated layer- and direction-dependent ultimate tensile stress and stretch ratio of human atherosclerotic iliac arteries. Anisotropic and highly nonlinear tissue properties were observed as well as interspecimen differences. The adventitia demonstrated the highest strength and fibrous cap in circumferential direction showed low fracture stress. Using in vivo medical screening approaches, Nagaraj et al., (2005) observed stiffening elastic modulus with lesion progression in porcine carotid artery. Using noninvasive echotracking system, Paini et al. (2007) found plaque region in human carotid artery was associated with larger bending and stiffer material properties. Walraevens et al. (2008) used uniaxial unconfined compression tests to distinguish the mechanical properties of healthy tissue from calcified tissue in aorta. More recently, Ebenstein et al., (2008) used nanoindentation to measure the mechanical properties of blood clots, fibrous tissue, partially calcified fibrous tissue and bulk calcifications of human atherosclerotic plaque tissue. The results demonstrated that the stiffness of plaque tissue largely depends on the mineral content. Most of these previous studies focused on the fibrous cap and are based on tests in one direction. It is well known that atherosclerotic plaques also have fiber-oriented multi-layered structures. Different layers display different direction-dependent mechanical behaviors (Holzapfel et al., 2000 and 2004). Layer- and direction-dependent experimental data are crucial for realistic computational models and accurate stress-strain predictions (Holzapfel et al., 2002).

Accurate knowledge of ultimate strength of arteries will provide the mechanical loading threshold for plaque vulnerability assessment based on computational stress/strain predictions (Cheng et al., 1993; Li et al., 2006). While image-based computational models are capable of representing the mechanical loading in the plaque (Tang et al., 2005; Yang et al., 2007), it is hard to assess the risk of plaque rupture without knowing the local material strength of the blood vessel. In this paper, we will quantify the ultimate strength of human atherosclerotic carotid arteries by direct mechanical testing.

2. Methods

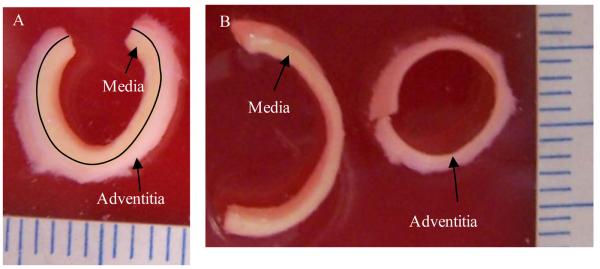
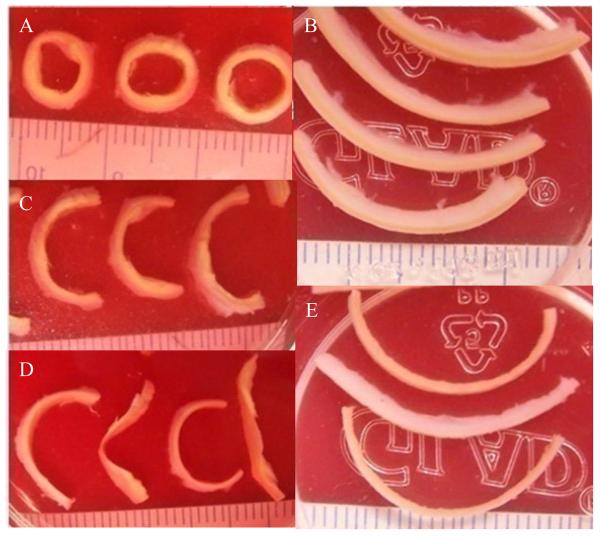
Three pairs of human carotid arteries were tested (69.3±17.7yr; one female and two males). Carotid arteries were obtained from the Department of Pathology, Washington University School of Medicine. Specimens were obtained and de-identified under the auspices of a Washington University Institutional Review Board Protocol. Each pair of arteries was obtained at autopsy and fresh frozen in PBS. On the day of testing, each sample was thawed at room temperature and dissected. In order to investigate the layer- and direction-specific strength behaviors, four circumferential and axial strips were cut from intact atherosclerotic arteries and then the adventitia and media layers were separated. The adventitia and media could be easily identified visually. The media was a darker yellow than the adventitia (Fig.1). It was not difficult to identify and separate the two layers. A cut was made at one end of the strip, and then the two layers were teased apart with fine tweezers. Any specimen that appeared to sustain damage was discarded. The intima resided on the inner surface of the media specimens. A series of circumferential rings (Fig.2A) and axial strips (Fig.2B) were dissected from each artery. Only sections with type II and III lesions (Cai et al., 2002) were selected for testing. That is, only sections with fatty streak or preatheroma with extracellular lipid pools were used and those with large lipid pool and calcification were avoided when creating the test specimens. Radial cuts were made on the arterial rings to create the uniaxial circumferential specimens (Fig.2C). Strips for layer-specific test were further dissected to create media and adventitia specimens (Fig.2D&E). The dimensions, including width and thickness, were measured using vernier caliper (0.1mm in precision) at three equally spaced locations along the specimen. . The average value was used for further calculation of the stress. Circumferential arterial sections were typically 2 mm wide and 9-10 mm in length. Axial specimens were typically 2 mm wide and 15 mm long. The thicknesses of media, adventitia and intact strips were 0.61±0.21mm, 0.60±0.19mm and 1.24±0.30mm, respectively Super glue was used to attach pieces of sandpaper to the specimen. The distance between the closer edges of the two pieces of sandpaper was measured as the initial length of the tissue strip. The tissue specimen was then mounted on a customized uniaxial test frame (Inspec 2200, Instron Corp., Norwood, MA, USA) that had been modified to operate under the control of software developed using LabVIEW (National Instruments Corporation, USA). The sample was carefully positioned so that the edges of clamps and the sandpaper coincided. The current length of the specimen was computed by adding the initial length of the specimen and the displacement of the jaw during the test. A load cell measured the load and clamp to clamp displacement was used to calculate the stretch ratio. Based upon preliminary tests, adventitia specimens were preconditioned by loading twice to a stretch ratio of 1.1, while intact (full thickness) and circumferential specimens were preconditioned by loading twice to a stretch ratio of 1.05. After preconditioning, each specimen was loaded at 0.1mm/s until rupture occurred (Fig. 3). Force and displacement data were recorded at a sampling rate of 10/s. The first Piola-Kirchhoff stress and stretch ratio data were calculated using the initial measured dimensions of the specimen. The tissue surface was maintained in a moist condition by spraying with PBS.

Figure 1.

Figure 1

Open in a new tab

Specimen showing visual distinction between adventitia and media layers. A: the specimen before separation; B: separated adventitia and media strips.

Figure 2.

Figure 2

Open in a new tab

Sample strips for material testing. A: arterial rings with small extracellular lipid pools; B: axial strips with fatty streaks; C: open-up sectors after radial cuts were made in the rings in (A); D: separated layers, adventitia (sectors with relatively round shape) and media (sectors with more irregular shape) of the open-up sectors; E: separated layers, adventitia (thicker strip) and media (thinner strips) of axial strips. Each small grid is 1mm width.

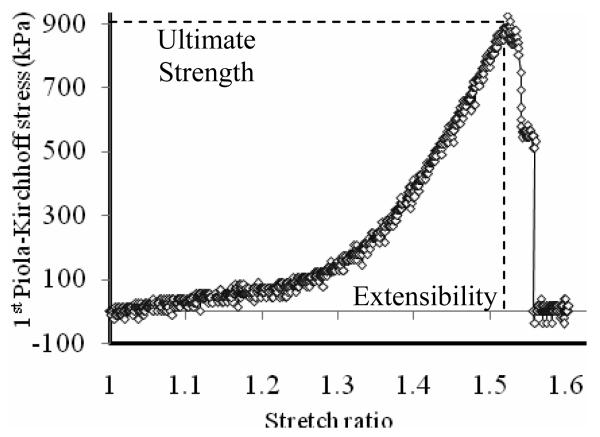
Figure 3.

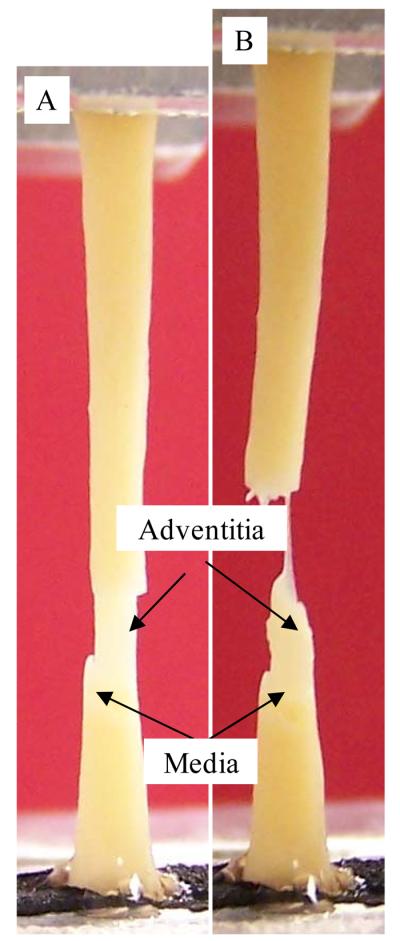
Figure 3

Open in a new tab

Photograph of a full thickness (intact) specimen that initially failed in the media. A: initial failure in the media part; B: final rupture occurred in the adventitia. Figure 6 shows a typical stress-stretch ration plot for this type of behavior.

Each artery yielded 12±1 specimens, 2 in each of the following categories: axial adventitia (AA), axial media (AM), circumferential adventitia (CA), circumferential media (CM), axial intact (AI) and circumferential intact (CI). In total, seventy-three (73) specimens from 6 human carotid arteries were tested. A t-test (P<0.05) was used to determine if there was a significant difference between the strength of the 6 types of specimens.

3. Results

Tissue ultimate strength can be captured by the sudden drop of the stress-stretch curve (Fig. 4). The recorded data set for each specimen consisted of about 1000 stress-stretch data points. To minimize the possible effect of signal noise, the ultimate strength value for each specimen was determined by averaging 5 data points centered at the peak stress value and the corresponding value of stretch ratio was taken as the extensibility.

Figure 4.

Figure 4

Open in a new tab

Stress (the first Piola-Kirchhoff stress)-stretch ratio plot for an axial media (AM) specimen.

3.1 Ultimate Strength

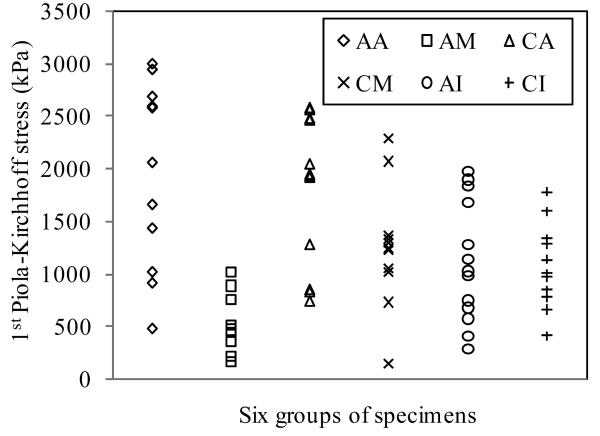
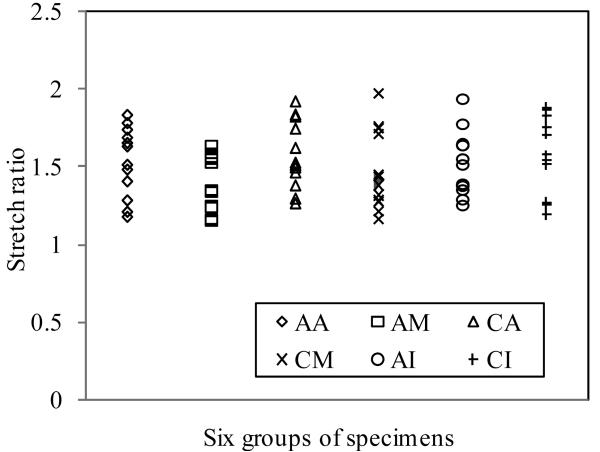
Fig. 5 plots ultimate stress data from all 73 specimens. Mean values for the six groups (AA, CA, AM, CM, AI and CI) are summarized in Table 1. The most significant finding was that mean ultimate strength of media in axial direction had the lowest ultimate strength (520kPa) and was significantly lower than that from the other five groups (P<0.001). Mean ultimate strength values for adventitia were 1996±867kPa for AA (axial direction) and 1802±703kPa for CA (circumferential direction) respectively and were not significantly different (P=0.552). Mean ultimate strength for media in the axial (519±270kPa) or in the circumferential (1230±533kPa) direction was significantly lower than that of adventitia in both directions. The ultimate strength of axial intact specimens (AI) (1047±536kPa) was similar to the strength of the circumferential intact specimens (CI) (1022±427kPa), p=0.899. The intact specimens in both directions showed significantly lower strength than the adventitia and had similar rupture strengths to the media in circumferential direction.

Figure 5.

Figure 5

Open in a new tab

Comparison of direction- and layer-specific ultimate strength of atherosclerotic tissue from human carotid artery. AA: Axial adventitia; AM: Axial media; CA: Circumferential adventitia; CM: Circumferential media; AI: Axial intact; CI: Circumferential intact. Number of specimens for each group is given in Table 1.

Table 1.

Mean values and relative differences of ultimate strength (the first Piola-Kirchhoff stress) and extensibility of the six groups of atherosclerotic carotid artery tissue specimens indicating that axial ultimate strength of media has the lowest value among the 6 groups considered and may provide a reference threshold value for vessel ultimate strength which is useful in atherosclerotic plaque rupture risk assessment. AA: Axial adventitia; AM: Axial media; CA: Circumferential adventitia; CM: Circumferential media; AI: Axial intact; CI: Circumferential intact. The percentage value is relative to AM.

Axial Groups AA AM AI
# of Specimens 12 11 13
Extensibility 1.54±0.23 110% 1.40±0.18 100% 1.50±0.20 107%
Ultimate Stress 1996±867 385% 519±270 100% 1047±536 202%
Circumferential Groups CA CM CI
# of Specimens 12 13 12
Extensibility 1.57±0.22 112% 1.47±0.25 105% 1.53±0.27 109%
Ultimate Stress 1802±703 347% 1230±533 237% 1022±427 197%
Open in a new tab

3.2 Extensibility

Stretch ratios at failure for all 73 specimens are plotted in Fig. 6. Mean values for the six groups are given in Table 1. The mean stretch ratio at failure for all 72 human carotid specimens was 1.50±0.22. Except for the fact that extensibility of AM was significantly lower than CA (P=0.025), no significant difference was found between any other two groups. Adventitia showed relatively larger mean extensibility, which was 1.54±0.23 for axial and 1.57±0.22 for circumferential, respectively. Media specimens had lower extensibility (AM: 1.40±0.18; CM: 1.47±0.25).

Figure 6.

Figure 6

Open in a new tab

The comparison of direction- and layer-specific ultimate extensibility of atherosclerotic tissue from human carotid artery (AA: Axial adventitia; AM: Axial media; CA: Circumferential adventitia; CM: Circumferential media; AI: Axial intact; CI: Circumferential intact. Number of specimens for each group is given in Table 1.

4. Discussion

4.1 Ultimate Strength of Human Atherosclerotic Carotid Arteries

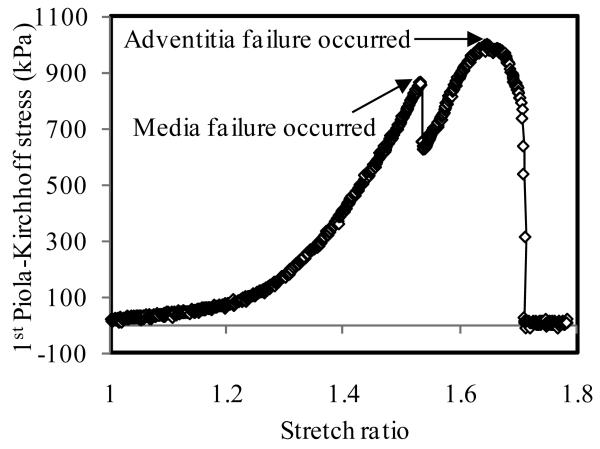
The ultimate strength of atherosclerotic tissues in human carotid artery was layer- and direction-dependent. The adventitia was much stronger than the media. The same phenomenon was observed by Holzapfel et al. (2004) in human atherosclerotic iliac artery. The media in axial direction had the lowest rupture stress. For the extensibility, except for the pair of CA vs. AM, no significant difference was found between any other two groups. The media had relatively lower extensibility (Table 1). This conclusion could be confirmed by the rupture behavior of intact specimens. When the failure test was performed with intact specimens, eight (8) of 25 specimens were observed where the initial rupture occurred in the media (4 in axial tests and 4 in circumferential tests; Fig. 3A&B). The initial rupture was reflected by a small sudden drop in stress as shown in Fig.7. Initial rupture was never observed in the adventitia. After the media broke, the adventitia undertook the further loading until it failed (the final sudden drop in stress in Fig.7). It should be emphasized that in these cases the ultimate strength of intact specimen was taken from the peak value at the first evidence of failure (i.e. the value associated with the failure of the media).

Figure 7.

Figure 7

Open in a new tab

The failure behavior of full thickness specimen with an initial failure in the media. The ultimate strength is taken as the stress at which the media fails.

The experimental data also demonstrated that human atherosclerotic human carotid tissues with Type II and III lesions display nonlinear material properties (Fig.4&7). At the beginning of the stretch, stress increased slowly with stretch. After stretch ratio exceeded 1.3, stress increased more rapidly. At a stretch ratio of approximately 1.5, the stress exceeded material strength limit and disruption occurred.

The ultimate strength and stretch ratio from samples from the 6 arteries displayed considerable variations. These variations result from individual differences between the properties of each of the arteries as well as variations in the measured properties from specimens from the same artery. Our results indicated that minimum ultimate stretch ratio of AA was 1.18 and the maximum was 1.84. Minimum ultimate stress of AA was 482 kPa, while the maximum reached nearly 3000 kPa (2997kPa). The lowest ultimate strength of CM was about 150 kPa. Such low strength limit could easily be exceeded under physiological load conditions.

4.2 Use of Ultimate Strength and Extensibility in Plaque Vulnerability Assessment

Plaque rupture is widely thought as the result of failure of fibrous cap (Fuster et al., 1998; Li et al., 2006; Tang et al., 2009). Ideally, ultimate strength and extensibility of all tissue types in the plaque, especially fibrous caps over large lipid cores, should be tested and quantified to provide a complete reference data base for plaque mechanical analysis and vulnerability assessment. However, direct test of ultimate strength of fibrous cap was beyond the scope of this study due to limitation of our test equipment and availability of plaque samples. From material point of view, failure of any component of arterial tissue including media and adventitia will result in a higher risk of rupture. Thus values of ultimate strength, particularly, of media, can still serve as a useful reference point in plaque vulnerability assessment.

Current plaque vulnerability assessment is mainly based on morphological data obtained medical imaging technologies, such as CT and MRI. However, plaque rupture represents a mechanical failure which occurs when the extra loadings on fibrous cap (and other possible vulnerable sites) due to blood pressure, flow and heart motion exceed the material strength (Yang et al., 2008). Complete plaque mechanical analysis combining material ultimate strength information, stress/strain predictions from computational models and plaque morphology from medical images will lead to better accuracy in assessing plaque vulnerability (Tang et al., 2005 and 2006).

4.3 Limitations of this study

Results from this study showed little difference in stretch ratio at failure for the media, adventitia and intact vessels. However, the difficulty in establishing the initial length at zero load for nonlinear soft tissues is well known and our recorded values for stretch ratios may be affected slightly by this fact for some cases. Although the grips were coated with rubber, several specimens (27of 73) broke at or near one of the grips, most likely due to stress concentrations. In these cases it is likely that the ultimate strength was underestimated.

Dividing the atherosclerotic carotid artery as media and adventitia over-simplified the complexity of the structure of the artery. The intima resided on the inner surface of the media specimens. To provide a more accurate and more general data base for modeling use in plaque assessment, determination of the material properties and ultimate strength of all component tissue types (especially fibrous cap) will be required. The mean ultimate strength of adventitia was about 280% and 50% higher than that of media in axial and circumferential directions, respectively. The mean ultimate strength of media in the axial direction (520kPa) could be considered as a reference threshold value for plaque vulnerability assessment schemes. While uniaxial tests gave insight into the presence of anisotropic material behavior, biaxial testing would be required to fully describe plaque tissue anisotropic behaviors. Testing using more samples and specimens of all plaque component tissues (especially fibrous cap with indication of rupture) is needed to provide more in-depth direct knowledge in understanding the ultimate material strength of human atherosclerotic carotid arteries.

Acknowledgement

This research was supported in part by NIH grant NIH/NIBIB R01 EB004759 and NSF/NIGMS DMS-0540684.

Footnotes

Publisher's Disclaimer: This is a PDF file of an unedited manuscript that has been accepted for publication. As a service to our customers we are providing this early version of the manuscript. The manuscript will undergo copyediting, typesetting, and review of the resulting proof before it is published in its final citable form. Please note that during the production process errors may be discovered which could affect the content, and all legal disclaimers that apply to the journal pertain.

Conflict of Interest

The authors declare that there are no conflicts of interest associated with this research.

REFERENCES

  • 1.AHA (American Heart Association) Heart disease and stroke statistics-2003 update. Dallas, Texas: 2003. [Google Scholar]
  • 2.Cai JM, Hatsukami TS, Ferguson MS, Small R, Polissar NL, Yuan C. Classification of human carotid atherosclerotic lesions with in vivo multicontrast magnetic resonance imaging. Circulation. 2002;106:1368–1373. doi: 10.1161/01.cir.0000028591.44554.f9. [DOI] [PubMed] [Google Scholar]
  • 3.Cheng GC, Loree HM, Kamm RD, Fishbein MC, Lee RT. Distribution of circumferential stress in ruptured and stable atherosclerotic lesions. A structural analysis with histopathological correlation. Circulation. 1993;87:1179–1187. doi: 10.1161/01.cir.87.4.1179. [DOI] [PubMed] [Google Scholar]
  • 4.Cullen P, Baetta R, Bellosta S, Bernini F, Chinetti G, Cignarella A, Eckardstein AV, Exley A, Goddard M, Hofker M, Hurt-Camejo E, Kanters E, Kovanen P, Lorkowski S, McPheat W, Pentikäinen M, Rauterberg J, Ritchie A, Staels B, Weitkamp B, Menno de Winther for the MAFAPS Consortium Rupture of the atherosclerotic plaque: does a good animal model exists? Arteriosclerosis, Thrombosis, and Vascular Biology. 2003;23:535–542. doi: 10.1161/01.ATV.0000060200.73623.F8. [DOI] [PubMed] [Google Scholar]
  • 5.Ebenstein DM, Coughlin D, Chapman J, Li C, Pruitt LA. Nanomechanical properties of calcification, fibrous tissue, and hematoma from atherosclerotic plaques. J. Biomed. Mater. Res. 2008 Dec. 23(Part A) doi: 10.1002/jbm.a.32321. [DOI] [PubMed] [Google Scholar]
  • 6.Fuster V. In: The Vulnerable Atherosclerotic Plaque: Understanding, Identification, and Modification. Fuster V, Cornhill JF, Dinsmore RE, Fallon JT, Insull W, Libby P, Nissen S, Rosenfeld ME, Wagner WD, editors. Futura Publishing; Armonk, NY: 1998. (AHA Monograph Series). [Google Scholar]
  • 7.Holzapfel GA, Gasser TC, Ogden RW. A new constitutive framework for arterial wall mechanics and a comparative study of material models. Journal of Elasticity. 2000;61:1–48. [Google Scholar]
  • 8.Holzapfel GA, Stadler M, Schulze-Bauer CAJ. A layer-specific three-dimensional model for the simulation of balloon angioplasty using magnetic resonance imaging and mechanical testing. Annals of Biomedical Engineering. 2002;30:753–767. doi: 10.1114/1.1492812. [DOI] [PubMed] [Google Scholar]
  • 9.Holzapfel GA, Sommer G, Regitnig P. Anisotropic mechanical properties of tissue components in human atherosclerotic plaques. Journal of Biomechanical Engineering. 2004;126:657–665. doi: 10.1115/1.1800557. [DOI] [PubMed] [Google Scholar]
  • 10.Lee RT, Grodzinsky AJ, Frank EH, Kamm RD, Schoen FJ. Structure dependent dynamic mechanical behavior of fibrous caps from human atherosclerotic plaque. Circulation. 1991;83:1764–1770. doi: 10.1161/01.cir.83.5.1764. [DOI] [PubMed] [Google Scholar]
  • 11.Lendon CL, Davis MJ, Born GVR, Richardson PD. Atherosclerotic plaque caps are locally weakened when macrophage density is increased. Atherosclerosis. 1991;87:87–90. doi: 10.1016/0021-9150(91)90235-u. [DOI] [PubMed] [Google Scholar]
  • 12.Lendon CL, Davies MJ, Richardson PD, Born GV. Testing of small connective tissue specimens for the determination of the mechanical behavior of atherosclerotic plaques. Journal of Biomedical Engineering. 1993;15:27–33. doi: 10.1016/0141-5425(93)90089-h. [DOI] [PubMed] [Google Scholar]
  • 13.Li ZY, Howarth S, Trivedi RA, U-King-Im JM, Graves MJ, Brown A, Wang LQ, Gillard JH. Stress analysis of carotid plaque rupture based on in vivo high resolution MRI. Journal of Biomechanics. 2006;39:2611–2622. doi: 10.1016/j.jbiomech.2005.08.022. [DOI] [PubMed] [Google Scholar]
  • 14.Loree HM, Grodzinsky AJ, Park SY, Gibson LJ, Lee RT. Static circumferential tangential modulus of human atherosclerotic tissue. Journal of Biomechanics. 1994;27:195–204. doi: 10.1016/0021-9290(94)90209-7. [DOI] [PubMed] [Google Scholar]
  • 15.Nagaraj A, Kim H, Hamilton AJ, Mun JH, Smulevitz B, Kane BJ, Yan LL, Roth SI, McPherson DD, Chandran KB. Porcine carotid arterial material property alterations with induced atheroma: an in vivo study. Med. Eng. Physics. 2005;27:147–156. doi: 10.1016/j.medengphy.2004.09.014. [DOI] [PubMed] [Google Scholar]
  • 16.Paini A, Boutouyrie P, Calvet D, Zidi M, Agabiti-Rosei E, Laurent S. Multiaxial mechanical characteristics of carotid plaque: analysis by multiarray echotracking system. Stroke. 2007;38:117–123. doi: 10.1161/01.STR.0000251796.38954.b2. [DOI] [PubMed] [Google Scholar]
  • 17.Rekhter MD, Hicks GW, Brammer DW, Work CW, Kim J, Gordon D, Keiser JA, Ryan MJ. Animal model that mimics atherosclerotic plaque Rupture. Circulation Research. 1998;83:705–713. doi: 10.1161/01.res.83.7.705. [DOI] [PubMed] [Google Scholar]
  • 18.Tang D, Yang C, Zheng J, Woodard PK, Saffitz JE, Sicard GA, Pilgram TK, Yuan C. Qunatifying effects of plaque structure and material properties on stress behaviors in human artherosclerotic plaques using 3D FSI models. Journal Biomechanical Engineering. 2005;127:1185–1194. doi: 10.1115/1.2073668. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 19.Tang D, Yang C, Yuan C. Mechanical image analysis using finite element method. In: Gillard J, Graves M, Hatsukami T, Yuan C, editors. Carotid Disease—The Role of Imaging in Diagnosis and Management. Vol. 2006. Cambridge, U.K.: 2006. pp. 323–339. [Google Scholar]
  • 20.Tang D, Teng ZZ, Canton G, Yang C, Ferguson M, Huang XY, Zheng J, Woodard PK, Yuan C. Sites of Rupture in Human Atherosclerotic Carotid Plaques are Associated with High Structural Stresses: an In Vivo MRI-Based 3D Fluid-Structure Interaction Study, Stroke (Accepted) 2009 doi: 10.1161/STROKEAHA.109.558676. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 21.Walraevens J, Willaert B, Win GD, Ranftl A, Schutter JD, Sloten JV. Correlation between compression, tensile and tearing tests on healthy and calcified aortic tissues. Medical Engineering & Physics. 2008;30:1098–1104. doi: 10.1016/j.medengphy.2008.01.006. [DOI] [PubMed] [Google Scholar]
  • 22.Yang C, Tang D, Kobayashi S, Zheng J, Woodard PK, Teng Z, Bach R, Ku DN. Cyclic bending contributes to high stress in a human coronary atherosclerotic plaque and rupture risk: in vitro experimental modeling and ex vivo MRI-based computational modeling approach. MCB: Molecular & Cellular Biomechanics. 2008;5:259–274. [PMC free article] [PubMed] [Google Scholar]
  • 23.Yang C, Tang D, Yuan C, Hatsukami TS, Zheng J, Woodard PK. In vivo/ex vivo MRI-based 3D non-Newtonian FSI models for human atherosclerotic plaques Compared with fluid/wall-only models. CMES: Computer Modeling in Engineering and Sciences. 2007;19(3):233–245. [PMC free article] [PubMed] [Google Scholar]

RESOURCES