Errors in Multi-Digit Arithmetic and Behavioral Inattention in Children With Math Difficulties

Abstract

Errors in written multi-digit computation were investigated in children with math difficulties. Third-and fourth-grade children (n = 291) with coexisting math and reading difficulties, math difficulties, reading difficulties, or no learning difficulties were compared. A second analysis compared those with severe math learning difficulties, low average achievement in math, and no learning difficulties. Math fact errors were related to the severity of the math difficulties, not to reading status. Contrary to predictions, children with poorer reading, regardless of math achievement, committed more visually based errors. Operation switch errors were not systematically related to group membership. Teacher ratings of behavioral inattention were related to accuracy, math fact errors, and procedural bugs. The findings are discussed with respect to hypotheses about the cognitive origins of arithmetic errors and in relation to current discussions about how to conceptualize math disabilities.

The rate of math disabilities is similar to that of reading disabilities in school-aged children (Kosc, 1974; Shalev, Auerbach, Manor, & Gross-Tsur, 2000), and math disability co-occurs in about 40% of individuals with reading disability (Lewis, Hitch, & Walker, 1994), though it may also occur on its own. Furthermore, difficulties in math and attention often co-occur (Fletcher, 2005; Marshall, Hynd, Handwerk, & Hall, 1997; Zentall, 1990; Zentall, Smith, Lee, & Wieczorek, 1994). It is not surprising, then, that current research concerns the nature of math difficulties in groups of children defined in relation to whether there is a coexisting reading disability and the role of attention in mathematical performance.

Math disabilities (MD) are typically defined by performance on standardized tests of single and multi-digit arithmetic. Recent research has characterized single-digit arithmetic performance in children with MD in relation to whether there is a coexisting reading disability (RD; Geary, Hoard, Byrd-Craven, & DeSoto, 2004; Jordan, Hanich, & Kaplan, 2003), the severity and persistence of math difficulties (Geary, Hoard, Byrd-Craven, Nugent, & Numtee, 2007), and the presence of problems in attention (Cirino, Fletcher, Ewing-Cobbs, Barnes, & Fuchs, 2007). Much less is known about multi-digit arithmetic performance in children with MD, including the types of errors they make and whether those errors vary as a function of reading status, severity of the problems in math, and the presence of problems in attention.

Multi-Digit Arithmetic in Typically Developing Children and in Children With MD

The performance of typically developing children in multi-digit arithmetic suggests that errors may be a valuable source of information about their procedural and conceptual knowledge and such information is relevant for instruction (Resnick, 1984). For example, children who are beginning to learn multi-digit subtraction may erroneously subtract the smaller number from the larger when the number in the minuend is smaller than its corresponding digit in the subtrahend (e.g., 926 – 764 = 242). Given the iterative relationship between procedural and conceptual knowledge in mathematics (Rittle-Johnson, Siegler, & Alibali, 2001), these procedural errors may reflect a lack of conceptual knowledge about the base 10 system (Fuson & Kwon, 1992). van Lehn (1982) distinguished between procedural errors that occur consistently (bugs) and that may reflect a lack of conceptual knowledge and procedural errors that occur occasionally (slips), which may reflect a lack of consolidation of procedures, and math fact errors, which are mistakes due to imperfect arithmetic believed to reflect difficulty with the retrieval of math facts from long-term memory or inaccurate use of backup counting strategies. Other types of errors in multi-digit computation such as those due to columnar misalignment and misreading and writing of numbers, sometimes called visual-spatial or visual-monitoring errors, have been observed in typically developing children but not studied systematically (Russell & Ginsburg, 1984).

There is relatively little information about the types of errors that children with MD make in multi-digit arithmetic (Geary, Hoard, Nugent, & Byrd-Craven, 2007). Yet learning of concepts and procedures in multi-digit arithmetic is an important instructional focus in the primary and junior grades. The most comprehensive study asked fourth graders with MD and fourth-and third-grade control groups to write down multi-digit addition and subtraction problems to dictation, solve those problems, and detect and explain errors in other “solved” problems (Russell & Ginsburg, 1984). Although the error data were not analyzed statistically, there were three main findings of relevance to the current study: younger control children (matched to children with MD on the basis of grade level in math) and children with MD were often indistinguishable from each other in the number and types of procedural errors they made; children with MD had a relatively large percentage of simple arithmetic (math fact) errors; and children with MD had difficulty identifying errors due to misalignment of numbers in columns, which may or may not be related to difficulties in visual monitoring given the particular paradigm used. These findings were used to suggest that procedural errors may represent developmental delays in mathematical processing given similarities between older children with math disabilities and younger typically developing children and that math fact errors and visual monitoring errors may be more characteristic of MD per se.

The idea that math fact errors characterize the multi-digit arithmetic performance of children with MD is consistent with recent findings that children with MD have difficulty with accuracy and fluency in solving single-digit arithmetic problems (Geary, Hamson, & Hoard, 2000; Jordan et al., 2003). More specifically, some studies that have compared children with MD to those with coexisting math and reading disabilities (MD+RD) report that children with MD only are better in strategy use and accuracy but not response time compared to children with MD+RD (Barnes et al., 2006; Geary et al., 2000; Jordan & Montani, 1997). The suggestion that children with MD might be particularly prone to making errors reflecting problems in visual monitoring is consistent with some subtyping studies on the neurocognitive profiles of children with MD (no RD) that reported these children have difficulties in visual-spatial processing and visual-spatial working memory (Ackerman & Dykman, 1995; Fletcher, 1985; McLean & Hitch, 1999; Passolunghi & Siegel, 2004; Rourke, 1993; Siegel & Ryan, 1989; but see lack of findings for visual-spatial deficits in MD in Bull, Johnston, & Roy, 1999; Geary, Hoard, Nugent, & Byrd-Craven, 2008; Temple & Sherwood, 2002; Wu et al., 2008; and meta-analysis of Swanson & Jerman, 2006). Geary (1993, 2004) has suggested that there may be a subtype of MD without RD associated with deficits in the spatial representation of quantitative information similar to those reported in brain-injured adults with spatial dyscalculia (Hartje, 1987). Because subgroups of MD based on reading status were not tested in the Russell and Ginsburg (1984) study, the relation of math fact errors and visual errors to MD+RD and MD, respectively, cannot be evaluated.

Attention and Math Disabilities

Population studies suggest that attention deficits may be more strongly associated with MD than RD (Gross-Tsur, Manor, & Shalev, 1996; Shaywitz, Fletcher, & Shaywitz, 1994), and children with attention deficits are most likely to show diminished classroom performance in arithmetic computations (Zentall, 1990). Fuchs et al. (2005) found that teacher ratings of inattentive behavior uniquely predicted the development of first-grade mathematical skills, including math computation and fact retrieval, as well as a range of math outcomes in third-grade students (Fuchs et al., 2006). The relation between attention and the errors that children with MD make in multi-digit computation has not been examined; nevertheless, it has been suggested that some types of errors could reflect difficulties in attention. Children with MD have been observed to add when subtraction is required on mixed format, written computation tasks (Jordan & Hanich, 2000). Moreover, Rourke (1993) suggested that children with MD without RD experience problems shifting psychological sets such that when two or more operations of one kind (e.g., subtraction) were followed by an operation of another kind (e.g., addition), children with MD only continued to apply the practiced procedure (subtraction) to the new operation (addition). It has been suggested that these operation switch errors may reflect the influence of attention difficulties on written computation tasks, in particular, problems with those aspects of attention that involve inhibitory control and switching. Consistent with this explanation, children with poor mathematical skills, particularly young children, have impaired response inhibition and mental set switching (Bull et al., 1999; Bull & Scerif, 2001), and some of these skills in the preschool years are related to later achievement in arithmetic (Mazzocco & Kover, 2007). What has not been systematically investigated is whether operation switch errors actually occur with greater frequency in children with MD, whether operation switch errors particularly characterize children with MD and no RD (Rourke, 1993), and whether switch errors are related to symptoms of inattention and/or hyperactivity.

Current Issues in Defining Math Disabilities

Although studying math performance in subtypes of MD may be important for understanding and remediating mathematical difficulties, how these groupings should be formed is a matter of current debate—with respect to common coexisting conditions such as reading and attention problems as reviewed above and/or with respect to the severity of the difficulties in math. The literature on MD has been criticized for the variable ways in which MD is defined in research studies, with some including children with scores on standardized math tests as high as the 45th percentile and others paying scant attention to whether these scores represent persistence of difficulties in math over time (reviewed in Mazzocco, 2007). Although the application of cutoff points to define RD lacks validity (Fletcher, Lyon, Fuchs, & Barnes, 2007), it has been suggested that math may differ from reading in important ways making identification strategies for math and reading disabilities not entirely comparable, at least not at this point given the relatively sparser knowledge base on math than reading (Mazzocco, 2007).

A few recent studies (Geary, Hoard, Byrd-Craven, et al., 2007; Mabbott & Bisanz, 2008; Murphy, Mazzocco, Hanich, & Early, 2007) have looked at mathematical performance and supporting cognitive competencies such as working memory in children whose difficulties in math are very low and persistent across grade compared to those whose math performance is in the low average to low end of the average range. These studies suggest that there may be differences between children with more severe and less severe difficulties in math in terms of cognitive characteristics and how those cognitive characteristics mediate mathematical performance. These findings, as well as those from math disability studies, that take reading difficulties into account suggest that mathematical processing and potential underlying cognitive competencies in groups constituted according to different definitions should be studied (Mazzocco, 2007; Murphy et al., 2007) because different subgroups of children with math disabilities may require different instructional approaches, have different neurobiological signatures, and result in different long-term outcomes.

The Present Study

The types of errors that children with MD make in multi-digit arithmetic, whether the nature of those errors varies as a function of reading status or severity of the difficulty in math, and whether and how attention is related to computation errors have not received systematic study. Thus, the first aim of the study was to examine types of errors in third- and fourth-grade children (i.e., math fact, procedural bugs and slips, visual, operation switch) on a multi-digit computation task across subgroups of children with math difficulties constituted according to reading status (MD+RD, MD, RD, and no learning difficulties [No LD]) and, in separate analyses, in groups constituted according to severity of the difficulties in math achievement (very low achievement [MLD], low average achievement [LA], and No LD).

In the analyses according to reading status, children with math difficulties were identified using the more liberal criteria used in several cognitive studies, in this case, math achievement below the 30th percentile. We predicted that (a) to the extent that math difficulties in children with MD+RD reflect underlying deficits in phonological working memory and some reports of severer deficits in simple arithmetic in MD+RD, this group would make more math fact errors in the context of multi-digit arithmetic than all other groups. However, children with MD who have difficulties in some aspects of math fact retrieval might also make more errors than children with No LD; (b) procedural errors would not distinguish MD+RD and MD groups; and (c) to the extent children with MD only may have difficulties in visual-spatial aspects of math and problems in set switching, the rate of visual-spatial and operation switch errors should be highest in this group.

In the second set of analyses, children with low math achievement below the 16th percentile (MLD) were compared to those with low average math achievement between the 17th and 30th percentiles (LA) and a control group with no learning disabilities (No LD). Given the newness of these alternate categorizations of MD, we posed only two specific hypotheses: Given that children with MLD were chosen on the basis of achievement on standardized measures of math calculations, we expected lower accuracy in multi-digit arithmetic for the MLD group than the LA group; and based on reporting of greater retrieval errors in single digit arithmetic for MLD (Geary, Hoard, Byrd-Craven, et al., 2007), we expected the greatest number of math fact errors in this group.

The second aim of this study was to examine the effect of behavioral inattention on general math outcomes and process-related variables, such as types of computation errors. Based on previous findings, we predicted that behavioral ratings of inattention would be related to multi-digit computation accuracy but made no predictions about relations with specific errors—math fact, procedural, or visual-spatial errors. Given the suggestions in the literature on the origin of operation switch errors and the relation of set switching and inhibitory control to arithmetic achievement, it was hypothesized that switch errors would be related to behavioral inattention and/or hyperactivity/impulsivity.

Method

Participants

Two hundred and ninety-one children in the third and fourth grades were recruited from 20 schools within Houston, Texas, and Nashville, Tennessee. These children were participating in the first year of a longitudinal research program investigating math competency in children with MD, with and without coexisting RD. All children were required to have an IQ score of 80 or above on the Wechsler Abbreviated Scales of Intelligence (WASI; Psychological Corporation, 1999), as this criterion was used to screen for intellectual deficiency.

Learning difficulty categories were determined using cutoff scores below the 30th percentile on standardized subtests of reading and arithmetic from the Wide Range Achievement Test–Third Edition (WRAT-3; Wilkinson, 1993). Children with scores below the 30th percentile on both the arithmetic and the reading subscales were considered to have MD+RD, children with scores below the 30th percentile on the arithmetic subscale but above the 40th percentile on the reading subscale were considered to have MD, children with scores below the 30th percentile on the reading subscale but above the 40th percentile on the arithmetic subscale were considered to have RD, and children with scores above the 40th percentile on both the reading and the arithmetic subscales were considered as having No LD.

Table 1 shows demographic information and achievement scores for each group. Comparisons among groups were made using analysis of variance (ANOVA), and significant group effects were investigated using the Tukey post hoc test, controlling alpha at p < .05. As would be expected, the groups differed in reading achievement, F(3, 287) = 235.35, p < .001. The No LD group had higher scores than every other group, the MD group had higher scores than the MD+RD and RD groups, and the RD group had a higher score than the MD+RD group. The groups also differed in math achievement, F(3, 287) = 258.20, p < .001. The No LD group had higher scores than all other groups, and the RD group had higher scores than the MD+RD and MD groups, which did not differ from each other.

Table 1.

Descriptive Information on Math and Reading Difficulty Groups

Variable	Category/Scale	No LD (n = 85)	RD (n = 66)	MD (n = 51)	MD+RD (n = 89)
Age	Years	9.30 (0.7)a	9.65 (0.7)b,c	9.55 (0.7)a,b	9.89 (0.8)c
Sex	Female	49%	50%	35%	45%
Reduced lunch	Yes	55%	59%	63%	64%
Retained	Yes	2%	6%	25%	36%
Grade	3	55%	42%	65%	60%
	4	45%	58%	35%	40%
Ethnicity	African American	50%	59%	57%	53%
	Caucasian	19%	17%	14%	16%
	Hispanic	27%	21%	23%	28%
	Other	4%	3%	6%	3%
WASI FSIQ	Standard score	100.48 (11.3)a	93.72 (11.2)b	92.51 (8.7)b,c	89.18 (8.5)c
WRAT-3 Arithmetic	Standard score	108.29 (8.1)a	104.44 (6.3)b	87.63 (4.6)c	85.93 (4.7)c
WRAT-3 Reading	Standard score	107.59 (7.7)a	87.00 (4.6)c	104.39 (6.5)b	84.11 (7.4)d
SWAN-Inattention	−27 to +27	+3.58 (13.6)a	−3.64 (11.2)b	−7.44 (9.4)b	−7.52 (9.6)b
WAS	x/12	9.15 (2.9)a	8.92 (2.7)a	6.96 (3.3)b	5.88 (3.2)b

Note: Standard deviations are enclosed in parentheses. Values with different superscripts are significantly different from one another. FSIQ = Full Scale Intelligence Quotient; MD = math difficulty; MD+RD = math and reading difficulty; No LD = no learning difficulty; RD = reading difficulty; SWAN = Strengths and Weaknesses of ADHD and Normal Behavior; WAS = Written Addition and Subtraction Task; WASI = Wechsler Abbreviated Scales of Intelligence; WRAT-3 = Wide Range Achievement Test–Third Edition.

For the second set of analyses to test the hypothesis that students who could be considered MLD differ from those with more broadly low math achievement, we reconstituted the MD and MD+RD subgroups described above. Specifically, individuals in those two groups were divided into those whose math achievement was either above or below the 16th percentile leaving reading free to vary (MLD and LA groups, respectively). Our reconfiguration of the groups is not identical to either Geary, Hoard, Byrd-Craven, et al. (2007) or Murphy et al. (2007) because the study was originally designed with respect to co-occurrence of learning difficulties in reading and math rather than to very low versus low average math performance; we wanted to reconfigure the students originally identified as having MD (either alone or in combination with RD) rather than create new subgroups that are overlapping though not synonymous with the first set of analyses, and we needed to balance sample size issues with the ability to detect potential differences between groups. Although we were unable to measure math skills in these groups across the first few grades of school, difficulties in math by the third and fourth grades may signify more long-standing problems in this domain. We also used the No LD group to make comparisons compatible with other studies in the literature.

Table 2 shows demographic information and achievement scores for these reconstituted groups. As for the first method of grouping, group comparisons were made using analysis of variance, and significant group effects were investigated using the Tukey post hoc test, controlling alpha at p < .05. The groups differed in math achievement, with the No LD group having higher scores than the other two groups and the LA group having higher scores than the MLD group. The groups also differed in reading achievement showing the same pattern as for math. With respect to IQ, the No LD group had higher scores than the MLD and LA groups, which did not differ from each other.

Table 2.

Descriptive Information on Math Learning Disability, Low Achievement, and No Learning Disability Groups

Variable	Category/Scale	No LD (n = 85)	LA (n = 104)	MLD (n = 36)
Age	Years	9.30 (0.69)a	9.72 (0.8)b	9.88 (0.8)b
Sex	Female	49%	38%	53%
Reduced lunch	Yes	41%	69%	64%
Retained	Yes	2%	29%	42%
Grade	3	55%	58%	72%
	4	45%	42%	28%
Ethnicity	African American	51%	56%	50%
	Caucasian	19%	14%	19%
	Hispanic	27%	25%	31%
	Other	3%	5%	0%
WASI FSIQ	Standard score	100.48 (11.30)a	90.30 (8.7)b	90.67 (8.7)b
WRAT-3 Arithmetic	Standard score	108.29 (8.09)a	88.75 (2.6)b	80.19 (3.5)c
WRAT-3 Reading	Standard score	107.59 (7.07)a	93.41 (11.3)b	85.97 (12.7)c
SWAN-Inattention	−27 to +27	3.58 (13.57)a	−6.19 (9.6)b	−11.19 (8.3)c
WAS	x/12	9.15 (2.93)a	6.78 (3.17)b	4.81 (3.21)c

Note: Standard deviations are enclosed in parentheses. Values with different superscripts are significantly different from one another. LA = math low achievement; MLD = math learning disability; No LD = no learning difficulty; RD = reading difficulty; SWAN = Strengths and Weaknesses of ADHD and Normal Behavior; WAS = Written Addition and Subtraction Task; WASI = Wechsler Abbreviated Scales of Intelligence; WRAT-3 = Wide Range Achievement Test–Third Edition.

Materials and Procedure

Written multi-digit computation task

Children were tested individually in a quiet classroom by a research assistant. Each child was asked to complete a problem sheet with 12 multi-digit addition and subtraction questions presented in a vertical format. Children were told to complete as many questions as they could, not to skip any questions, and to show all of their work. Children were allowed 7 minutes to complete the problem sheet; however, no one required the full amount of time. The multi-digit computation questions varied in level of difficulty, ranging from easy questions, those requiring minimal conceptual and procedural knowledge (i.e., 36 + 48), to difficult questions, those requiring more sophisticated knowledge (i.e., 2006 – 42). The addition and subtraction questions were arranged such that children were required to switch between operations nine times.

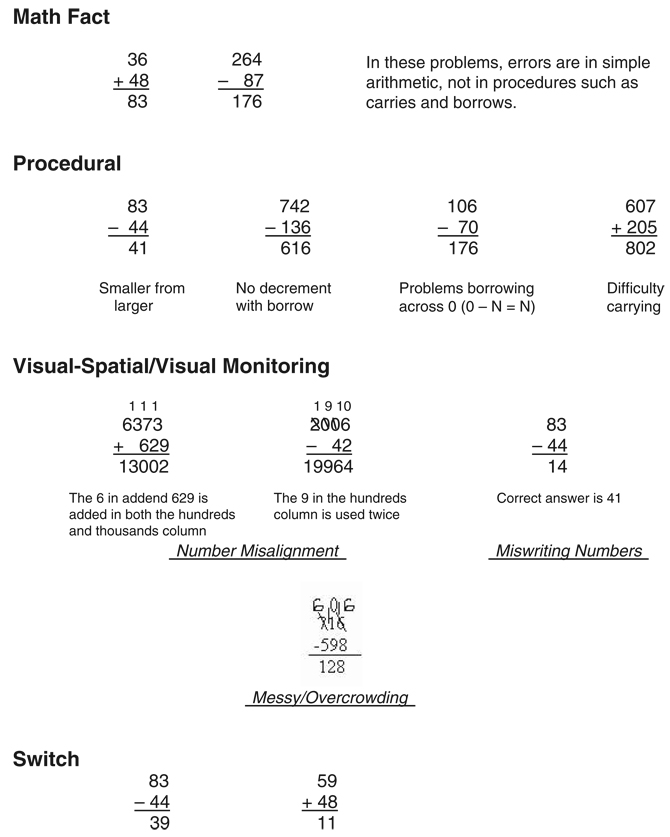
Within this problem set, errors were coded as math fact, procedural, visual-spatial, and/or switch errors. Math fact errors reflect an error on single-digit addition or subtraction within the multi-digit problem. Errors indicating the misapplication of arithmetic procedures or lack of knowledge of procedures, such as problems borrowing from zero or subtracting the smaller number from the larger number instead of borrowing, were coded as procedural errors. Two different types of procedural errors were coded: procedural bugs and procedural slips. A procedural bug is coded when the child makes the same type of procedural error on at least two problems, whereas procedural slips are coded when the child makes only one procedural error despite the opportunity to make at least two of the same type of error. In this problem set, the opportunity to make all of the procedural errors that could be coded actually exceeded two opportunities. Visual-spatial errors suggest problems in visual-spatial processing or visual monitoring, such as misreading numbers, errors due to problems in column alignment either in completing the computation or in writing the answer, or crowding of written work. Errors reflecting difficulty switching from one operation (i.e., addition) to another (i.e., subtraction) were coded as operation switch errors. Examples of errors are in Figure 1.

Figure 1.

Examples of the Four Error Types Examined in This Study

The number of problems solved correctly and the number of errors were recorded. Math fact errors and procedural bugs and slips were coded using van Lehn’s detailed error scoring method, titled the Subtraction Bug Glossary (van Lehn, 1982), which was revised based on Hartje (1987) to include visual-spatial errors and Barnes et al. (2002) to include a comparable addition bug glossary. Two coders scored errors independently. Interrater reliability was sufficient at .85; however, all scoring discrepancies were resolved through discussion and careful consideration of the coding manual, so that the coders reached 100% agreement.

SWAN inattention subscale

Children’s classroom teachers were asked to complete the Strengths and Weaknesses of ADHD–Symptoms and Normal Behavioral Rating Scale (SWAN; Swanson et al., 2005), an 18-item questionnaire based on the attention-deficit/hyperactivity disorder (ADHD) criteria identified in the Diagnostic and Statistical Manual of Mental Disorders–Fourth Edition, Text Revision (American Psychiatric Association, 2000). The SWAN rates children’s attentive, hyperactive, and impulsive behaviors in comparison to same age peers. Scoring of each item ranged on a Likert scale from a low level of problems (3, 2, 1) through average (0) to a high level of problems (−3, −2, −1).

Analyses

Group differences on variables utilized school grade as a covariate. Although we considered using chronological age as a covariate instead of or in addition to grade, we thought that instructional exposure was more relevant than actual age in the development of multistep algorithmic computation, even in cases where a given student was retained, which did occur with some frequency in this sample, particularly among disability groups. Also, within grade level, age was not related significantly to any of the dependent variables of interest (median r = .07). Therefore, grade was the only covariate used.

The distributions of the error dependent variables were nonnormal, with many students making no errors, or many making one to two errors of a particular variety. Because the distributions were generally not improved by variable transformations, we used logistic regression to determine the extent to which grade and/ or group membership was associated with making one or more errors of a given type. Most variables were dichotomized into subgroups that made no errors versus those that made at least one error. Error frequencies for the entire group of 291 participants are in Table 3. In cases where further differentiation could be made (e.g., into no errors, one error, and more than one error), these were also evaluated, though none of these results differed from the “none versus one or more” dichotomy. In each of these models, the interaction of grade and group was tested and, where nonsignificant, was trimmed from the model. This was the case for all dependent variables, so all the models described are with only main effects. The impact of behavioral inattention and hyperactivity/impulsivity was evaluated by adding these variables to the models above. However, across dependent measures, point-biserial correlations for the former variable were higher relative to the latter, and whenever both types of scores were considered in predictive models, impulsivity/hyperactivity was never significant in the context of the inattention variable, but the reverse was never true. Therefore, in the analyses reported below, only inattention was considered, which is consistent with previous studies showing relations between computation and inattention but not hyperactive/impulsive items (Fuchs et al., 2005; Fuchs et al., 2006). As with grade, the interaction of behavioral inattention and group was tested and found to be nonsignificant in all models, so the role of inattention is reported as a main effect only. Follow-up group comparisons were performed in cases of significant group main effects. For overall performance, these comparisons were corrected for multiple comparisons, but for error analyses, these values were left uncorrected given the uniqueness of these data.

Table 3.

Error Frequencies Among Participants

Error Type	Total No. Errors	Frequency of Participants	% of Participants
Math fact	0	163	56.01
	1	74	25.43
	2	37	12.71
	>2	17	5.85
Procedural bugs	0	158	54.30
	1	102	35.05
	2	25	8.59
	>2	6	2.06
Procedural slips	0	147	50.52
	1	69	23.71
	2	51	17.53
	>2	24	8.25
Visual-spatial	0	253	86.94
	1	33	11.34
	2	5	1.72
	>2	0	0
Switch	0	226	77.66
	1	53	18.21
	2	10	3.44
	>2	2	0.69

Results

Analyses for Groups With and Without Reading Difficulties

Overall accuracy

An ANOVA was conducted to examine the effects of grade and group membership on overall accuracy on the multi-digit computation task. Main effects were found both for grade, F(1, 286) = 41.38, p < .0001, and for group, F(3, 286) = 21.25, p < .0001. Post hoc follow-up Tukey corrected group comparisons indicated that students with MD+RD and MD did not differ from one another, but both performed more poorly than RD and No LD students; the latter two subgroups did not differ from one another (means are in Table 1).

Computation errors

The proportion of students from each of the four learning difficulty subgroups and for the two grades committing each type of error is presented in Table 4. As already noted, there were no interactions between grade and group.

Table 4.

Percentage of Participants Committing None (N) or Some (S) Errors by Group or Grade

	Group								Grade
	No LD		RD		MD		MD+RD		3		4
Error Type	N	S	N	S	N	S	N	S	N	S	N	S
Math fact	65	35	62	38	49	51	47	53	51	49	62	38
Procedural bugs	74	26	70	30	37	63	34	66	43	57	68	32
Procedural slips	53	47	56	44	41	59	49	51	41	59	62	38
Visual-spatial	94	6	83	17	90	10	81	19	83	17	92	8
Switch	80	20	82	18	76	24	73	27	76	24	79	21

Note: MD = math difficulty; MD+RD = math and reading difficulty; No LD = no learning difficulty; RD = reading difficulty.

For math fact errors, the overall likelihood ratio was significant, χ²(4, N = 291) = 10.37, p < .04. However, neither grade, Wald χ²(1, N = 291) = 2.89, p > .05, nor group membership, Wald χ²(3, N = 291) = 6.49, p > .05, was a significant predictor of the presence of math fact errors.

For procedural bugs, the overall likelihood ratio was significant, χ²(4, N = 291) = 58.15, p < .0001. Both grade, Wald χ²(1, N = 291) = 15.57, p < .0001, and group membership, Wald χ²(3, N = 291) = 35.75, p < .0001, were significant predictors of the presence of procedural bugs. In terms of grade, students in Grade 3 made more bugs than those in Grade 4. Post hoc group contrasts indicated that the proportion of students with MD+RD and MD who made procedural bugs did not differ from one another (p > .05), but these proportions were higher than RD (MD+RD, Wald χ² = 15.60, p < .0001; MD, Wald χ² = 8.67, p < .003) and higher than No LD (MD+RD, Wald χ² = 28.83, p < .0001; MD, Wald χ² = 15.97, p < .0001) students; RD and No LD subgroups did not differ from one another. It is worth noting that children’s bugs mostly reflected errors made on multiple problems; that is, the bug error was made on most of the problems for which that type of error could be made.

Procedural errors were examined qualitatively to determine whether the groups differed in the types of errors they made. Across all groups, addition bugs were a relatively rare occurrence (5% overall), and neither grade nor group was a significant predictor of these bugs. Subtraction bugs comprised the bulk of procedural bugs in general. The most common subtraction bug was smaller from larger, in which the child does not borrow but subtracts the smaller digit from the larger one. In particular, 31% of the MD+RD group committed the smaller from larger subtraction bug, which was significantly more than proportions of the RD (9%) and No LD groups (9%) but not the MD (25%) group who made this type of bug, F(3, 287) = 6.96, p < .001. Another common subtraction bug was borrow no decrement, in which the child adds 10 correctly but does not change any columns to the left when borrowing. The proportions of the No LD (8%), RD (8%), MD (18%), and MD+RD (12%) groups committing this type of bug were similar, F(3, 287) = 1.31, ns. A final common subtraction bug, borrowing across zero (0 – N = N) occurs when a child doesn’t borrow but records N as the answer when there is a column in the form 0 – N. Like smaller from larger bugs, borrowing across zero bugs were committed by a greater proportion of students with MD+RD (20%), relative to students with RD (6%) and No LD students (7%) but not students with MD (16%), F(3, 287) = 3.48, p < .05.

For procedural slips, the overall likelihood ratio was significant, χ²(4, N = 291) = 14.78, p < .005. Grade was a significant predictor, Wald χ²(1, N = 291) = 11.69, p < .0006, with Grade 3 students making more slip errors than those in Grade 4. Group membership was not a significant predictor of the presence of procedural slips, Wald χ²(3, N = 291) = 1.59, p > .05.

For visual-spatial errors, the overall likelihood ratio was significant, χ²(4, N = 291) = 13.65, p < .009. Both grade, Wald χ²(1, N = 291) = 4.80, p < .03, and group membership, Wald χ²(3, N = 291) = 7.88, p < .05, were significant predictors of the presence of visual-spatial errors. In terms of grade, students in Grade 3 made more errors than those in Grade 4. Post hoc group contrasts indicated that a greater proportion of students with MD+RD and RD made errors relative to No LD students (MD+RD, Wald χ² = 5.99, p < .02; RD, Wald χ² = 5.04, p < .03); other group comparisons were not significant.

For switch errors, the overall likelihood ratio was not significant, χ²(4, N = 291) = 2.23, p > .05, indicating that grade and group membership were not significant predictors of the presence of switch errors.

Attention and computation errors

An ANOVA was conducted to examine the effects of grade and group membership on attention ratings. The results revealed a main effect of grade, F(1, 283) = 6.60, p < .01, and a main effect of group F(3, 283) = 16.91, p < .0001; there was no significant interaction between grade and group (p > .05). Tukey corrected post hoc comparisons indicated that each of the learning difficulty groups exhibited more behavioral inattention relative to students in the No LD group (all p < .0002), although these learning difficulty groups did not differ from one another.

When behavioral inattention was added as a predictor of type of math errors along with grade and group in the above analyses, the overall pattern of results did not change. That is, inattention did not interact with grade and/or group in predicting math errors and did not generally alter the main effects of grade or group membership or the pattern of relationships therein. For visual-spatial errors, however, while the overall model still retained a significant likelihood ratio, χ²(5, N = 291) = 13.27, p < .02, the effects of both grade, Wald χ²(1, N = 291) = 3.79, p < .06, and group membership, Wald χ²(3, N = 291) = 6.07, p < .11, were diminished and no longer significant. Behavioral inattention was a significant effect for overall accuracy, F(1, 282) = 47.81, p < .0001; for math fact errors, Wald χ²(1, N = 291) = 13.71, p < .0002; and for procedural bugs, Wald χ²(1, N = 291) = 19.15, p < .0001. However, for procedural slips, visual-spatial errors, and switch errors, there was no effect for behavioral inattention (p > .05). This general pattern was evident in the zero-order correlations among error types and behavioral inattention (overall accuracy, r = .48, p < .01; math fact errors, r = −.21, p < .01; procedural bug errors, r = −.36, p < .01). Behavioral inattention ratings were not significantly correlated with switch errors and visual-spatial errors.

Analyses for MLD Versus LA and No LD Groups

Overall accuracy

The overall group effect for accuracy on the multi-digit computation task was significant, F(2, 221) = 27.66, p < .0001, as was grade, F(1, 221) = 25.85, p < .0001. Fourth graders were more accurate than third graders, as expected, and follow-up contrasts revealed that the No LD group outperformed both the LA and the MLD groups and that the LA group outperformed the MLD group (means in Table 2).

Computation errors

Error data are in Table 5. For math fact errors, the overall likelihood ratio was significant, χ²(3, N = 225) = 12.62, p < .006. As in the original analyses, grade was not a significant predictor (p < .053), but unlike the original analyses, group membership was significant, Wald χ²(2, N = 225) = 7.44, p < .024. Here, follow-up contrasts revealed that students with MLD differed from No LD (p < .009), but the LA group did not differ from either the MLD or the No LD group (both p > .05).

Table 5.

Percentage of Participants Committing None (N) or Some (S) Errors by Group

	No LD		LA		MLD
Error Type	N	S	N	S	N	S
Math fact	65	35	52	48	36	64
Procedural bugs	74	26	39	61	22	78
Procedural slips	53	47	42	58	58	42
Visual-spatial	94	6	86	14	81	19
Switch	80	20	76	24	69	31

Note: LA = math low achievement; MLD = math learning disability; No LD = no learning difficulty.

For procedural bugs, the overall likelihood ratio was significant, χ²(3, N = 225) = 44.67, p < .001. Both grade, Wald χ²(1, N = 225) = 7.42, p < .006, and group membership, Wald χ²(2, N = 225) = 31.09, p < .0001, were significant predictors of the presence of procedural bugs. Specifically, a higher proportion of Grade 3 students made these types of errors, and although fewer students with No LD made procedural bugs than either LA or MLD students (both p < .0001), the latter two groups did not differ from one another. When addition and subtraction bugs were examined individually, no differences were observed for addition bugs; however, for subtraction bugs, the overall likelihood ratio was significant, χ²(3, N = 225) = 43.46, p < .0001. Both grade, Wald χ²(1, N = 225) = 5.17, p < .023, and group membership, Wald χ²(2, N = 225) = 31.39, p < .0001, were significant predictors. Again, a higher proportion of Grade 3 students made these types of bugs. Also, fewer students with No LD made procedural subtraction bugs than either LA or MLD students (both p < .0001), but a greater proportion of MLD students made this kind of error relative to LA students (p < .046).

For procedural slips, the overall likelihood ratio was significant, χ²(3, N = 225) = 15.52, p < .001. Grade was a significant predictor, Wald χ²(1, N = 225) = 11.46, p < .0007, with errors more common in Grade 3 relative to Grade 4, but group membership was not, Wald χ²(2, N = 225) = 4.70, p > .095.

For visual-spatial errors, the overall likelihood ratio was significant, χ²(3, N = 225) = 14.58, p < .002. Grade was significant, Wald χ²(1, N = 225) = 6.84, p < .009, with errors more common in Grade 3 relative to Grade 4. Unlike the original analyses, group membership, Wald χ²(2, N = 225) = 4.21, p > .05, was not a significant predictor of the presence of visual-spatial errors.

For switch errors, the overall likelihood ratio was not significant, χ²(3, N = 225) = 1.62, p > .05, and neither grade nor group membership was a significant predictor of the presence of switch errors.

When behavioral inattention was added as a predictor of type of math errors along with grade and group as noted above, in general, the pattern of results did not change, as was the case with the original set of analyses. That is, inattention did not interact with grade and/or group in predicting math errors and did not generally alter the main effects of grade or group membership or the pattern of relationships therein. However, two exceptions were noted. For math fact errors, the overall model was significant, Wald χ²(4, N = 225) = 30.55, p < .0001, although in the presence of behavioral inattention, neither grade nor group membership was a significant predictor of math fact errors, whereas a significant effect of group was noted above. For subtraction bugs, the overall model was significant, Wald χ²(4, N = 225) = 56.64, p < .0001; in the presence of behavioral inattention, grade was now not significant, Wald χ²(1, N = 225) = 3.75, p > .053. Furthermore, although group membership remained significant, Wald χ²(2, N = 225) = 15.44, p < .0004, and fewer students with No LD made subtraction bugs relative to students with either MLD (p < .004) or LA (p < .002), now, the MLD and LA subgroups no longer differed from one another (p > .123). In sum, students with LA outperformed those with MLD on their overall accuracy performance but did not differ on any error index in the presence of behavioral inattention. Across models, behavioral inattention was a significant effect for overall accuracy (p < .0001), math fact errors (p < .0001), and all procedural bugs (all p < .005), though it was unrelated to procedural slips, visual-spatial errors, and switch errors (p > .05).

Discussion

The present study provides a systematic analysis of the types of errors that children with math difficulties make in multi-digit arithmetic—an understudied aspect of mathematical competence. In the context of multi-digit arithmetic, the analyses addressed two current issues in the math disabilities literature: (a) the types of errors made by children with math difficulties grouped according to the presence or absence of coexisting reading difficulties or according to overall level of math achievement and (b) the relation of teacher ratings of inattention to errors in multi-digit arithmetic. In general, children with math difficulties grouped according to the presence or absence of co-occurring reading difficulties were more similar in their multi-digit arithmetic performance than they were different, and for math fact errors and procedural slips, the math difficulty groups did not differ from children without learning disabilities. The presence of reading difficulties, even when there were no math difficulties, was related to visual-spatial or visual monitoring errors, inconsistent with predictions from some subtyping models. More differences emerged when comparing MLD and LA children; specifically, math fact errors distinguished MLD children from those with No LD, and MLD children made more subtraction bug errors than LA children. Contrary to predictions, operation switch errors were not more common in disability groups regardless of how they were constituted nor were they related to attention. However, teacher ratings of inattention were related to math fact and procedural errors.

Math Difficulties and Computation Errors: Do Reading Status and Level of Math Achievement Make a Difference?

Children with MD, specific or otherwise, performed worse on the multi-digit computation task than children with no MD. Although children with MD+RD often demonstrate more pervasive problems in math than children with MD alone, performance on the multi-digit computation task did not differentiate the two groups. This finding is consistent with studies showing that written computation tasks may not be sensitive to the differences between children with MD+RD and children with MD alone (e.g., Jordan et al., 2003). However, it is worth noting that neither accuracy nor response times distinguished these same groups on a test of single-digit addition (Cirino et al., 2007). A simpler explanation may be that in this study, the math achievement of the MD+RD group (as measured by the WRAT-3) was almost the same as that of the MD group, whereas in some other studies comparing MD+RD and MD, the MD+RD group sometimes has lower math achievement (e.g., Fuchs & Fuchs, 2002). As would be expected, children in the MLD group, explicitly chosen to have lower math achievement than the LA group, were less accurate on the experimental test of multi-digit arithmetic. The findings that are of more interest in this study have to do with the types of errors that are associated with children with math difficulties defined in different ways.

In contrast to predictions, the MD+RD group in this study did not make more math fact errors than other groups. The only group in the study that made more math fact errors than children with no learning difficulties was the MLD group. Other studies have found more math fact errors in multi-digit arithmetic in children with spina bifida with MD+RD compared to children with no learning disabilities (Barnes et al., 2006) and in children with MD whose reading status is unknown (Russell & Ginsburg, 1984). What do the studies and comparisons showing a higher incidence of math fact errors have in common? The MD+RD group in the Barnes et al. (2006) study had average math achievement below the 10th percentile. This was also the case for the MD group in the Russell and Ginsburg (1984) study (MD group mean at the 2.29 stanine); although the error data in this latter study were not analyzed statistically, the rate of math fact errors in their MD group was twice that of the control group. The average achievement of the MLD group in the current study is also in this range, whereas the average achievement of the MD+RD group, using the more lenient criteria used in many similar studies, is closer to the 20th percentile. In contrast, students with MLD and MD+RD had very similar reading scores. Because MLD and MD+RD groups differed in their math scores but not their reading scores, errors in simple arithmetic in the context of multi-digit written calculation may be less related to co-occurring reading problems than to the actual level of math achievement. This interpretation is consistent with findings that phonological skill is only modestly related to fact retrieval processes (Barnes et al., 2006). Children with math achievement in the low average range may be more able to use backup strategies to ensure accuracy in simple arithmetic where fluency is not critical for success (Geary et al., 2000; Geary, Hoard, Byrd-Craven, et al., 2007), regardless of whether they are good or poor readers.

In the present study, children with MD+RD and MD committed more procedural bugs than children with RD and No LD, which may reflect a lack of conceptual and procedural knowledge. In general, all groups committed similar types of procedural bugs, and there were no differences in the frequency of bugs made by the MD+RD versus MD groups, although more children from the MD+RD group did commit the smaller from larger and borrowing across zero bugs than the RD and No LD groups. The findings for MLD versus LA groups differed in one respect; the MLD group had more bugs in subtraction but not addition than the LA group. It would appear that even children with significant difficulties in math come to learn addition procedures to a level similar to that of their low average achieving peers but may lag behind them in knowledge of the more recently instructed operation (see also Barnes et al., 2002). In sum, the MLD group had the greatest deficits in conceptual and procedural knowledge needed for accurate performance on multi-digit subtraction.

Investigations of mathematical performance among typically achieving children suggest that with age and experience, they develop increasingly sophisticated conceptual and procedural knowledge that facilitates their performance on multi-digit computation tasks, reducing the frequency of procedural errors (van Lehn, 1982). We found that children in Grade 4 committed fewer procedural bugs and slips than children in Grade 3, regardless of learning difficulty status, suggesting that children in Grade 4 demonstrated superior acquisition and implementation of procedural knowledge and greater consolidation of this knowledge than their younger peers. These findings both for children with math difficulties and for typically developing children are consistent with the hypothesis that procedural deficits, even in multi-digit arithmetic, may represent developmental delays as opposed to cognitive deficits that are found in math fact retrieval (Geary, 1993); that is, children with math difficulties do improve in their knowledge and implementation of computational procedures, possibly to a greater extent than they improve in processes related to simple arithmetic retrieval and fluency (Russell & Ginsburg, 1984).

In contrast to procedural bugs, there was no difference among groups in the number of procedural slips committed whether reading status or level of math achievement was used to define the groups, though children in Grade 3 committed more slips than children in Grade 4. These results are consistent with previous research examining procedural errors in children with congenital (Ayr, Yeates, & Enrile, 2005; Barnes et al., 2002) and acquired brain lesions (Ashcraft, Yamashita, & Aram, 1992) associated with deficient computation skills in which bugs but not slips differentiated the brain injured groups from typically developing peers.

In contrast to what might be predicted from early neuropsychological studies in which children with MD only showed deficits in visual-spatial processing (Rourke & Finlayson, 1978) and from studies of brain-injured adults with visual-spatial errors in arithmetic (Ardila & Rosselli, 1994), there was no evidence that the errors made by children with MD only reflected difficulties in visual processing. The fact that groups with poor visual-spatial skills often have a greater incidence of MD is sometimes taken as evidence for a relation between the two. However, more direct tests of this relation in both typically and atypically developing individuals have often yielded null results. For example, Barnes et al. (2002, 2006) found no evidence of visual-spatial dyscalculia in children with spina bifida myelomeningocele and MD alone, a group with known deficits in spatial cognition and math (see also Rovet, Szekely, & Hockenberry, 1994).

Of particular interest, in the present study, it was children with MD+RD and RD who committed more visual errors than children with No LD as well as children with MLD who had lower reading scores, but younger children also made more of these errors than older children. It is unclear why children with reading difficulties and younger children should make more of these types of errors involving number alignment, skipping columns in the middle of a question even when other similar problems were completed correctly, miswriting of numbers (e.g., writing 41 instead of 14), and the like. However, we do note that visual errors were associated with poorer readers in both subgroup analyses (RD, MD+RD, and MLD). It may be that difficulties of poor readers include processing of alphanumeric symbols, not just letters, and that even poor readers with no deficits in mathematical skills per se may, from time to time, make errors that are related to less efficient processing of alphanumeric symbols (see also van Loosbroek, Dirkx, Hulstijn, & Janssen, in press). Russell and Ginsburg (1984) reported some of these types of errors in their MD group, but because reading status was not taken into account and the error analysis was qualitative in nature, it is unclear whether these types of errors might have been more common in those children with co-occurring reading disability. In all, these findings in combination with similar findings that systematically investigated visual-spatial errors in multi-digit calculation and related visual-spatial skills to calculation (Ayr et al., 2005; Barnes et al., 2002, 2006) suggest that visual-spatial skills may be less important for calculation than sometimes proposed, though they are likely involved in certain aspects of early mathematical development (Rasmussen & Bisanz, 2005) and in domains of math other than calculation (Barnes et al., 2002; Geary, 1996; Holmes & Adams, 2006; Kyttala & Lehto, 2008). In any event, in developmental math disabilities whether the groups are defined with respect to reading or level of math achievement, there is little evidence for visual-spatial dyscalculia of the type sometimes seen in adults with acquired brain injury.

Attention and Computation Errors

Some studies suggest that attention may be particularly related to math disabilities (Gross-Tsur et al., 1996; Shaywitz et al., 1994). In the current study, inattention ratings did not distinguish between groups of children with RD, MD, and MD+RD. However, children in the MLD group were rated as being more inattentive than children in the LA group. Similar to other recent studies that found attention, as rated by classroom teachers, to be a robust predictor of mathematical outcome (Fuchs et al., 2005, 2006), we found that children who were rated as being more inattentive answered fewer questions correctly on the multi-digit computation task than their peers who were rated as being more attentive. The interpretation of these findings is not entirely clear. Behavioral ratings of inattention have recently been related to cognitive measures of working memory or inhibitory control in typically developing children suggesting that these rating scales may be sensitive to difficulties with controlled aspects of attention (Liu & Tannock, 2007). Whether teacher ratings of behavioral inattention are tapping difficulties in controlled aspects of attention in children with severe difficulties in math in the present study and in other studies of math disabilities is unknown.

We extended the work of these previous studies by examining not only the effect of behavioral inattention on mathematical outcomes but also the effect of behavioral inattention on process related variables, such as specific computation errors. When the effect of behavioral inattention along with grade and group on visual-spatial errors was examined, there were no longer main effects for grade or group, and the effect of behavioral inattention was also not significant. This finding is of interest because recent research suggests that some of the types of errors that are typically coded as visual-spatial in nature and ascribed to deficits in visual processes may be due to poor monitoring of the sequence of steps of an algorithm and poor skills in detecting and then self-correcting errors (Geary et al., 2004). Difficulties in working through sequences and concurrently monitoring for errors are thought to require attention or concentration and working memory. However, we note that attention ratings were not correlated with visual-spatial errors. Whether cognitive measures of attention and working memory are related to these types of errors would be of interest.

We also found that children who were rated as less attentive committed more math fact errors than children who were rated as more attentive. One view is that children with math difficulties who make errors in simple arithmetic (and perhaps younger children as well) have problems inhibiting irrelevant associations (e.g., retrieve 8 for 3 + 4) because of inefficient inhibitory processes in working memory (Geary, 2004). Studies relating difficulties in cognitive inhibition (rather than teacher-reported symptoms of inattention) and math fact performance in children with attention difficulties have yet to be reported. Higher ratings of inattention were also related to procedural bugs. The execution of mathematical procedures often involves relatively laborious series of steps, and so inattentive behavior may be detrimental to performance on multi-digit problem solving. This interpretation is consistent with dual task studies of multi-digit arithmetic in adults that demonstrate considerable cognitive resources are required to solve multi-digit arithmetic problems (Imbo, Vandierendonck, & Vergauwe, 2008). The lack of relation between inattention and procedural slips and the grade effect for slips suggests that these types of errors may reflect lack of consolidation of procedures rather than lapses of attention during problem solving.

In contrast to predictions, operation switch errors, when studied systematically, were not related to math difficulties or attention. According to anecdotal reports, children with specific MD have difficulty switching from one operation (e.g., addition) to another (e.g., subtraction) when completing a standardized arithmetic task (Rourke, 1993). Switch errors are hypothesized to represent the attentional difficulties experienced by these children when completing mixed format computation tasks. It is possible that anecdotal reports of switch errors among children with specific MD do not stand up to systematic evaluation when making comparisons across groups, particularly when those comparisons include typically developing controls. Or it may be that most children, regardless of their learning difficulty status, evidence greater numbers of switch errors as they are learning a new operation but not once they have more experience with different operations. Although it might be argued that the task employed here may not have allowed children to build a psychological set as children were often required to switch operations after completing only one or at most two questions, it is important to note that operation switch errors did occur with sufficient frequency across all of the groups and grades.

Limitations and Implications for Future Research

It is unclear at present whether and how MDs should be subtyped or classified. In recent studies, including this one, the presence or absence of an RD may not serve to distinguish substantially different patterns of math performance in children with MDs. Although severity of the math difficulty was associated with higher rates of both math fact and certain procedural errors, and such information might be useful for instruction, it is an open question whether severe versus less severe math difficulties differ in their neurobiological and cognitive origins. The same problems in applying cutoff points along what is a normally distributed continuum of skill, as is the case for reading, may also be relevant for MD (Fletcher et al., 2007), and genetic studies of both unselected samples and samples that test at the “extremes” thus far suggest that difficulties in math represent the tail end of a normally distributed skill (Alarcon, DeFries, Light, & Pennington, 1997; Petrill & Plomin, 2007; Thompson, Detterman, & Plomin, 1991). Across studies, samples of children with MD may vary not only in severity of the math difficulties and presence of RD but also in terms of demographic characteristics such as ethnicity, gender, social-economic status, and the like. Whether sample-specific characteristics including ethnicity and socioeconomic disadvantage affected some of the findings in the current study are unknown but potentially important for generalization of the findings.

Although it is important to know what kinds of errors characterize the performance of children with MD in multi-digit arithmetic, it is also important to know what types of errors are not related to MD despite anecdotal reports. In this study, systematic testing of the types of errors variously proposed to be associated with MD more generally or with different subgroups of MD provided no support for the idea that visual errors or switch errors are associated with MD or for the idea that switch errors might be related to attention and inhibitory control. The finding that behavioral ratings of inattention are related to both math fact errors and procedural bugs suggests the need for cognitive research on how attention affects specific mathematical processes and on interventions that aim to effect change in those mathematical processes. A limitation of this study is that it did not include cognitive measures of attention and working memory (Liu & Tannock, 2007) or of switching (e.g., Murphy et al., 2007), which might have helped to narrow the explanations for the current pattern of results. Research that links types of errors in arithmetic with hypothesized cognitive mechanisms would be informative for models of mathematical processing.

Biographies

Kimberly P. Raghubar, MA, is a graduate student in clinical neuropsychology at the University of Houston. Her research interests are in mathematical cognition and math learning disabilities.

Paul T. Cirino, PhD, is a research associate professor in the Department of Psychology and the Texas Institute for Measurement, Evaluation and Statistics at the University of Houston. He is a developmental neuropsychologist whose interests include disorders of math and reading, executive function, and measurement.

Marcia A. Barnes, PhD, is a professor of pediatrics at the University of Texas Health Science Center at Houston, where she holds the Chair in Childhood Reading and Learning in the Children’s Learning Institute. She conducts research on mathematical learning disabilities and in reading comprehension.

Linda Ewing-Cobbs, PhD, is a professor of pediatrics at the University of Texas Health Science Center at Houston and director of the Dan L. Duncan Children’s Neurodevelopmental Clinic. She is a developmental neuropsychologist who conducts research on children with acquired and developmental disabilities.

Jack M. Fletcher, PhD, is a Distinguished University Professor of Psychology at the University of Houston. He is a child neuropsychologist who has completed research on many issues related to reading, learning disabilities, and dyslexia, including definition and classification, neurobiological correlates, and intervention.

Lynn S. Fuchs, PhD, is the Nicholas Hobbs Professor of Special Education and Human Development at Vanderbilt University. She conducts research on classroom-based assessment and reading and mathematics instructional methods.