Exchangeable oxygens in the vicinity of the molybdenum center of the high-pH form of sulfite oxidase and sulfite dehydrogenase

Abstract

The electron spin echo envelope modulation (ESEEM) investigation of the high-pH (hpH) form of sulfite oxidase (SO) and sulfite dehydrogenase (SDH) prepared in buffer enriched with H₂ ¹⁷O reveals the presence of three types of exchangeable oxygen atoms at the molybdenum center. Two of these oxygen atoms belong to the equatorial OH ligand and the axial oxo ligand, and are characterized by ¹⁷O hyperfine interaction (hfi) constants of about 37 MHz and 6 MHz, respectively. The third oxygen has an isotropic hfi constant of 3–4 MHz and likely belongs to a hydroxyl moiety hydrogen-bonded to the equatorial OH ligand. This exchangeable oxygen atom is not observed in the ESEEM spectra of the Y236F mutant of SDH, where the active site tyrosine has been replaced by phenylalanine.

Introduction

Sulfite-oxidizing enzymes (SOEs) occur in vertebrates, plants, and certain bacteria. In vertebrate sulfur metabolism, sulfite oxidase (SO) converts toxic sulfite to sulfate in the final degradation step of the sulfur-containing amino acids cysteine and methionine.¹ Sulfite dehydrogenase (SDH), isolated from the soil bacterium Starkeya novella, oxidizes sulfite during chemolithotrophic growth with thiosulfate serving as its energy source.^2,3 Despite their different metabolic roles, both SO and SDH catalyze the same reaction

$$\begin{array}{c}{{\text{SO}}_3}^{2-}+{\text{H}}_2\text{O}+2{(\text{cyt}c)}_{\text{ox}}\to \\ {{\text{SO}}_4}^{2-}+2{(\text{cyt}c)}_{\text{red}}+2{\text{H}}^{+}\end{array}$$ (1)

and have nearly identical active site geometries, which contain several conserved residues including Y236 (SDH numbering).⁴

The square pyramidal oxomolybdenum active site of either SO or SDH (see Fig. 1) has an axial oxo ligand, one equatorial sulfur from a cysteine amino acid residue, two equatorial sulfurs from the molybdopterin (MPT) cofactor, and one exchangeable equatorial ligand that depends on the stage of the catalytic cycle.^4–6 In the fully oxidized Mo(VI) state the exchangeable equatorial ligand is an oxo group. In the generally accepted catalytic mechanism,⁷ the equatorial oxo ligand undergoes nucleophilic attack by sulfite to form a sulfate-bound Mo(IV) intermediate. Subsequent hydrolysis of the sulfate and one-electron oxidation lead to a Mo(V)–OH species that can be studied by EPR spectroscopy.⁸

Fig. 1.

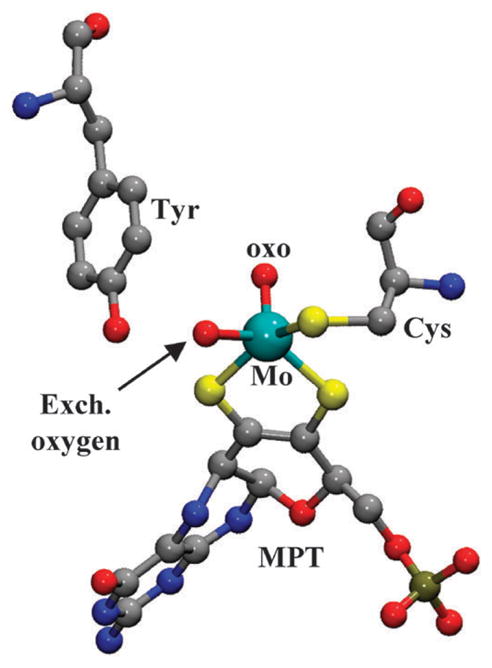
Structural representation of the Mo center of wt SO and SDH enzymes (prepared from the cSO coordinates from pdb 1SOX), including the MPT cofactor, the cysteine (Cys) residue, and the tyrosine (Tyr) residue that is within hydrogen-bonding distance from the exchangeable equatorial oxygen ligand.

Extensive EPR investigations of the Mo(V) state have shown that the spectra depend on pH, anions in the media, the method of generating the Mo(V) state, as well as the source organism and mutations near the active site. In particular, it was found that the signals formed at pH ≤ 7.5 and pH ≥ 9 in chicken SO (cSO) are characterized by different principal g-values,^9–14 and that the low-pH signal exhibits hyperfine splittings from a nearby proton of the equatorial OH ligand. The structurally different forms of SO (i.e., the Mo(V) active center) corresponding to these signals are referred to as the low-pH (lpH) and high-pH (hpH) forms, respectively. Similar lpH and hpH forms have also been identified for wild-type (wt) and mutated SOEs from human (hSO), plant (Arabidopsis thaliana, At–SO), and bacteria (Starkeya novella SDH).^1,15,16 In addition, recent pulsed EPR measurements have detected a blocked form of SOEs where the sulfate ligand remains bound to the Mo(V) center.^17–19

Continuous wave (CW) EPR spectra are helpful for qualitative characterization of the Mo(V) center of SOEs under various conditions, but they provide very little information about the hyperfine and nuclear quadrupole interactions (hfi and nqi, respectively) of neighboring magnetic nuclei. The hfi and nqi reflect the geometric and electronic structure of the Mo center and can provide details about the enzyme function (e.g., electron transfer, substrate binding, product release, etc.). Therefore, their experimental determination represents an active systematic effort that requires using high-resolution pulsed EPR methods, including electron spin echo envelope modulation (ESEEM) and electron-nuclear double resonance (ENDOR). For example, the ¹H hfi for the equatorial OH ligand and the active site protein environment have been estimated using these techniques, supplemented by measurements of the deuterium nqi in ¹H → ²H exchanged samples.^20–24 These experiments have directly established the presence of the equatorial OH ligand in the hpH form of SOEs and have revealed some of the structural differences between the hpH and lpH forms. In the blocked forms of SOEs prepared with ³³S-enriched sulfite, the detection of a nearby ³³S with non-zero isotropic hfi has proven the presence of a sulfate ligand.^17,19 Similarly, the detection of ³¹P has shown the coordination of phosphate in the phosphate-inhibited form of SO.²⁵ Finally, in lpH SOEs, the presence of a chloride near the active center has been definitively revealed,²⁶ and the interpretation of the experimentally determined Cl⁻ parameters by density functional theory (DFT) has shown that it is a second sphere ligand rather than being directly coordinated to the Mo(V).

Attempts to measure the hfi of the oxygen ligands in ¹⁷O-enriched preparations of SOEs have been the focus of several investigations, with the degree of success depending on the location of the ¹⁷O ligand (either equatorial or axial). For equatorial ¹⁷O, hfi splittings on the order of tens of MHz were observed by CW EPR for some of the EPR turning points, although the complete hfi tensor could not be extracted.²⁷ Detecting this oxygen by pulsed EPR was also never completely successful because of the strong hfi. For lpH SO, this ¹⁷O was observed by hyperfine sublevel correlation (HYSCORE) spectroscopy at the intermediate-field (g_Y) and high-field (g_X) EPR turning points,²⁸ while detection at the low-field turning point (g_Z) was not possible. A similar situation was encountered for the blocked form of the R160Q mutant of hSO. In hpH SO, HYSCORE detection was not possible, presumably because of the significantly larger hfi constant.

In addition to the strongly coupled equatorial oxygen, the preliminary ESEEM investigations have also revealed the spectral features of a weakly coupled ¹⁷O.²⁹ This was tentatively assigned to the oxo-ligand, which was later confirmed by comparison with the synthetic model Mo(V) complex, [Mo¹⁷O(SPh)₄]⁻.³⁰

In this work, we performed a detailed ESEEM study of the exchangeable oxygens in samples of hpH cSO and SDH. Since no HYSCORE cross-peaks for the equatorial oxygen could be observed, we applied more traditional one-dimensional (1D) techniques of two-pulse and three-pulse ESEEM, where at least the low-frequency fundamental line of this oxygen nucleus could be detected. This approach allowed the hfi to be measured, although the nqi splittings could not be detected. The results obtained for the axial oxo ligand were more detailed, however, and both the hfi and nqi tensors were evaluated for it. In addition to these two ligand oxygen nuclei, a third ¹⁷O nucleus was detected. This oxygen nucleus has a nearly isotropic hfi, and several possibilities for its origin are discussed.

The reasons for presenting the results obtained from the hpH forms of vertebrate and bacterial SOEs together in this work are explained as follows. First, it was interesting to compare the ¹⁷O spectroscopic parameters of the SOEs from different organisms, as these parameters reflect subtle structural details of their respective Mo centers. Second, with the similarity between wt cSO and SDH established, we studied the Y236F mutant SDH in order to address the structural role of the conserved active site tyrosine residue (Y236 in SDH; see Fig. 1 for the relative location of the tyrosine and the Mo center). The choice of the bacterial enzyme was determined by the fact that for another available mutant of this kind, Y343F hSO, the EPR spectrum shows a mixture of the hpH and lpH forms even at pH ~10.¹⁸ According to X-ray crystallographic studies, the tyrosine is within a hydrogen-bonding distance from the equatorial ligand of the Mo center,⁵ yet the same types of EPR signals are formed (although at somewhat different pH) despite the absence of this bond in the Y236F SDH mutant.

In this work, we report some preliminary structural insights from ¹⁷O hfi and nqi parameters, where DFT calculations have been used to interpret the experimental parameters. A more thorough interpretation of these parameters in terms of the complete active site structure will require a significant separate effort and will be the subject of future work.

Materials and methods

Sample preparation

The hpH forms of cSO and Starkeya novella SDH in H₂ ¹⁷O-enriched water were prepared by first concentrating a 100 μL to 300 μL solution of the enzyme (1 and 3 mgs) in 100 mM bis-tris propane pH 9.0 to reduce the amount of H₂ ¹⁶O. Next, a solution of 100 mM bis-tris propane pH 9.0 buffer was vacuum centrifuged to evaporate the H₂ ¹⁶O, and the pelleted buffer was redissolved in the same volume of H₂ ¹⁷O. The concentrated enzyme sample was then incubated in 30 μL of the buffer prepared with H₂ ¹⁷O for approximately 3 h at 4 °C for long incubation. Finally, the enzyme was reduced with a 20-fold of excess sodium sulfite under argon, and the samples were immediately frozen in liquid nitrogen. For short incubation, the samples were frozen immediately after addition of H₂ ¹⁷O buffer and sulfite.

ESEEM measurements

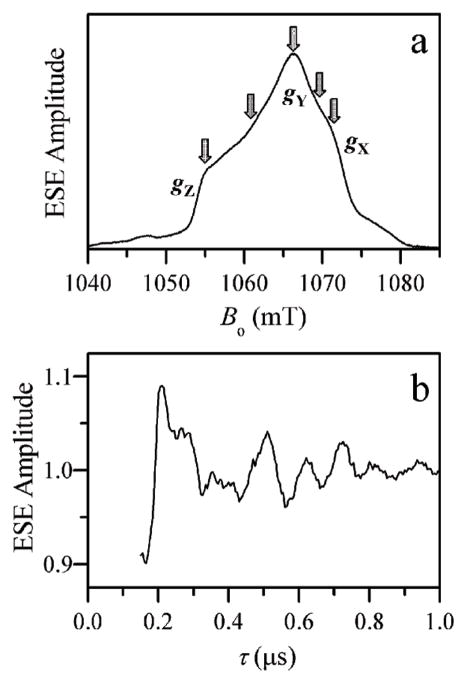
The orientation-selective ESEEM experiments were performed on a home-built K_a-band (26–40 GHz) pulsed EPR spectrometer²⁸ at the microwave (mw) frequency, ν_mw, of about 29 GHz. A representative set of measurement positions is shown in the ESE field-sweep spectrum of Fig. 2a. The average amplitude of the normalized ¹⁷O ESEEM was about 10% (see Fig. 2b). The measurement temperature was about 21 K. Numerical simulations of the ESEEM spectra were performed using the SimBud software (available for a free download from the website of the Department of Chemistry, University of Arizona: see http://quiz2.chem.arizona.edu/epr for details).

Fig. 2.

(a) Two-pulse ESE field sweep spectrum of hpH cSO. Arrows indicate the EPR positions where the two-pulse ESEEM experiments of Fig. 4 were performed. (b) Normalized two-pulse ¹⁷O ESEEM recorded at g_Z. Experimental conditions: mw frequency, 29.34 GHz; mw pulses, 2 × 15 ns; time interval between the mw pulses (for panel a), τ = 200 ns; temperature, 21 K.

Theoretical calculations

The DFT calculations were performed using the ORCA computational package (www.thch.uni-bonn.de/tc/orca). Geometry optimizations were performed using the BP86 functional³¹ in conjunction with the all-electron TZVP basis³² in its scalar relativistic re-contraction³³ and modeling the protein environment through dielectric continuum methods (conductor like screening model, COSMO)³⁴ using a dielectric constant of four.³⁵ Density fitting³⁶ was used to accelerate these calculations. Relativistic effects were treated at the level of the zeroth order regular approximation (ZORA)³⁷ in one-component form using the model potential of van Wüllen (as implemented in ORCA).³⁸ For geometry optimizations, the one-center ZORA scalar relativistic correction was employed.³⁹

Starting coordinates for different structural models describing the possible orientations of the hydroxo ligand (in 30° increments about the Mo(V) hydroxo oxygen bond with respect to the Mo(V) oxo bond) were based on the X-ray crystal structures of wt cSO.^40,41 The models were modified to include the equatorial hydroxo ligand (replacing the equatorial oxo ligand from the cSO crystal structure). In addition, the pterin portion of the molybdopterin cofactor was omitted, the cysteinate residue was replaced by ethanethiolate, and the ethanethiolate dihedral angles were constrained to their crystal structure values relative to the axial Mo(V) oxo bond in order to prevent unrealistic rotation of that group during geometry optimization. The ¹⁷O oxo/hydroxo hfi and nqi parameters and the ¹H hfi parameters were calculated using the B3LYP functional,⁴² the TZVP basis set, the ZORA method, and COSMO (using a dielectric constant of four). Since the scalar relativistic TZVP basis set is less heavily contracted than its nonrelativistic counterpart, further decontraction is not necessary in order to obtain accurate hfi and nqi predictions close to the basis set limit. Since we have previously found that the inclusion of picture change effects in the ZORA-4 formalism⁴³ did not improve the quality of calculated quadrupole couplings,⁴⁴ we did not apply such corrections here. For the correct ZORA calculation of hyperfine couplings,⁴⁵ it is nevertheless necessary to apply the relativistic formalism.

Results and discussion

1 General situation with ¹⁷O nuclei in the vicinity of the Mo(V) centers of hpH SO and SDH

The CW EPR spectra of hpH cSO and SDH prepared in H₂ ¹⁷O-enriched buffer are similar to those reported earlier²⁷ (see the ESI†). The only ¹⁷O nucleus detectable by CW EPR for these samples is that of the equatorial OH ligand (for hpH cSO, splittings at the low-field EPR turning point are, on average, about 1.1 mT²⁷). ESEEM, however, reveals a total of three types of ¹⁷O nuclei. The axial oxo ligand exchanges to ¹⁷O relatively slowly (tens of minutes). When the sample is prepared with a very short incubation time in H₂ ¹⁷O (~2 min) before adding sulfite, the contribution of the ¹⁷O-oxo is significantly diminished, and the other two exchangeable oxygens dominate the ESEEM spectra. As an example, traces 1 and 2 in Fig. 3 (panels a and b corresponding to g_Z and g_X, respectively) show the two-pulse ESEEM spectra of wt hpH cSO prepared with long (~3 h) and short (~2 min) incubation time, respectively. All of the features seen in these spectra originate from ¹⁷O because they were absent in the spectra of the sample prepared in the buffer containing the natural abundance of oxygen isotopes (0.037% ¹⁷O, trace 3 in both panels of Fig. 3). The contributions from the three different types of oxygen nuclei are indicated, and the spectroscopic information obtained for each of these oxygen nuclei is described below.

Fig. 3.

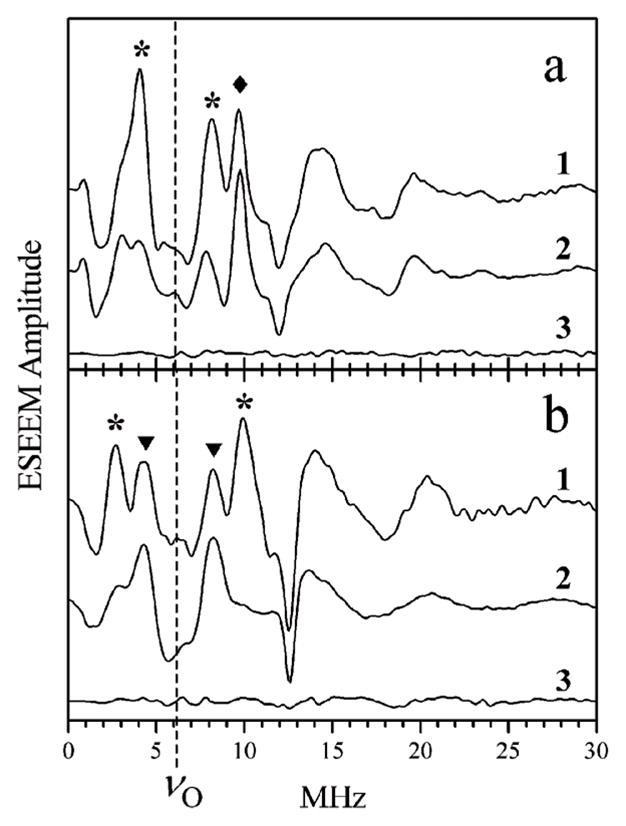
Panels a and b, two-pulse ESEEM spectra (cosine FT) of wt hpH cSO obtained at g_Z (B_o = 1055 mT) and g_X (B_o = 1071.5 mT), respectively. Traces 1 and 2 in each panel, spectra of samples prepared with H₂ ¹⁷O with long and short incubation time, respectively. Trace 3 in each panel, spectra of samples prepared with H₂ ¹⁶O. Experimental conditions: mw frequency, 29.34 GHz; mw pulses, 2 × 15 ns; temperature, 21 K. Asterisks, diamonds and triangles indicate the Δm_I = 1 fundamental lines of axial oxo-¹⁷O, equatorial ¹⁷O (from the OH ligand), and unidentified weakly coupled ¹⁷O, respectively. The vertical dashed line in each panel shows the Zeeman frequency of ¹⁷O, ν_O.

In the following we discuss in detail the data obtained for hpH wt cSO. The data obtained for wt and Y236F SDH were generally similar, with the differences pointed out when necessary. The representative ¹⁷O ESEEM spectra of wt and Y236F SDH are shown in the ESI. †

2 Axial ¹⁷O-oxo ligand

We have reported earlier²⁹ that the axial oxo ligand of the molybdenum center of SO exchanges to ¹⁷O when the enzyme is incubated in the H₂ ¹⁷O-enriched buffer. Later, using a synthetic oxomolybdenum(V) model complex, we refined the ESEEM-based approach to obtain information about the hfi and nqi of the ¹⁷O-oxo.³⁰ A similar approach was then applied to study the ¹⁷O-oxo ligand in various preparations of SO and SDH. While some of the results of those investigations were mentioned briefly elsewhere,⁴⁶ no detailed analysis of the spectra has been presented so far. In this work, we describe the data obtained for hpH cSO in greater detail.

As in our previous studies, it was convenient to use the two-pulse ESEEM technique to obtain the information about the ¹⁷O-oxo hfi. For the nqi, however, a higher spectral resolution was required, and to achieve this resolution the τ-integrated four-pulse ESEEM technique was used.⁴⁷ The spectra for the ¹⁷O-oxo were obtained by subtracting the spectra of short-incubation samples from those of long-incubation samples. Such spectra will be referred to as difference ESEEM spectra.

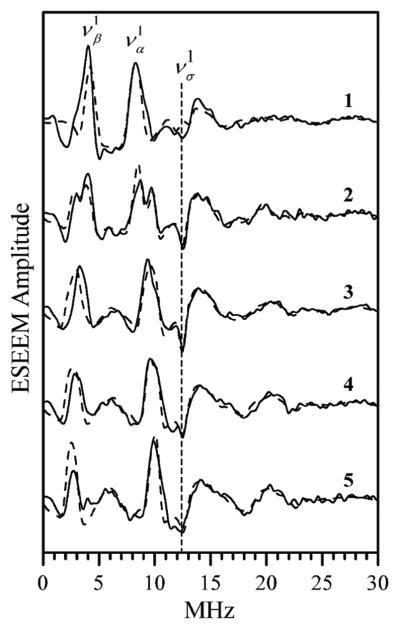
Fig. 4 shows the difference spectra of the two-pulse ESEEM of hpH cSO obtained at different EPR positions. The starting estimates of the hfi constants at each EPR position were obtained as a difference between the frequencies of the fundamental lines of Δm_I = 1 transitions of ¹⁷O (ν_α¹ and ν_β¹ in Fig. 4, where the superscript “1” indicates Δm_I = 1), A = ν_α¹ − ν_β¹. The smallest hfi constant (A ~ 4.2 MHz) was observed at the low-field EPR turning point, g_Z. At higher fields the hfi constant gradually increased and reached the maximum value of ~7.3 MHz at the high-field turning point, g_X. This hfi range is similar to that observed for the synthetic model complex [Mo(V)¹⁷O(SPh)⁴]⁻ (from 3.5 to 7.9 MHz). The starting estimates of the hfi parameters are also similar, a_iso ~ 6.3 MHz and T_⊥ = 1 MHz (where a_iso is the isotropic hfi constant and T_⊥ is the perpendicular component of the anisotropic hfi tensor, which at this point is assumed to be axial for simplicity).

Fig. 4.

Solid traces, primary ESEEM spectra (cosine FT) of wt hpH cSO obtained as a difference between the spectra of samples incubated for 3 h and 2 min in H₂ ¹⁷O buffer. Traces 1 through 5 are obtained at magnetic fields B_o = 1055 mT (g_Z), 1061 mT, 1066.3 mT (g_Y), 1069.5 mT and 1071.5 mT (g_X), respectively. Experimental conditions: mw frequency, 29.34 GHz; mw pulses, 2 × 15 ns; temperature, 21 K. Dashed traces, ¹⁷O primary ESEEM spectra calculated with the following parameters: a_iso = 6.3 MHz; (T₁₁,T₂₂,T₃₃) = (2.4, 1, −3.4) MHz; (φ_h,θ_h,ψ_h) = (55°, 20°, −10°); e²Qq/h = 1.7 MHz; η = 1; (φ_q,θ_q,ψ_q) = (70°, 30°, 0°). The calculated traces were divided by 11 in order to make their amplitude similar to the experimental one.

The nqi parameters can be conveniently estimated from the splittings between the components of the sum combination line ${\nu }_{\sigma }^1={\nu }_{\alpha }^1+{\nu }_{\beta }^1$.³⁰ These splittings, however, are rather small and are not observed in the two-pulse ESEEM spectra of Fig. 4 because the resolution of these spectra is limited by a relatively short transverse relaxation time (T₂ ~ 800 ns). Therefore, to estimate the nqi, the integrated four-pulse ESEEM spectra were used. The attainable resolution in these spectra is determined by the longitudinal relaxation time (T₁ ~ 300 μs at the temperature of ~20 K) and is sufficient for observation of the nqi splittings that are expected to be on the order of several tenths of a MHz.

As an example, Fig. 5 shows the sum combination line region of the spectra obtained at the EPR turning points. The splittings observed at g_Z, g_Y, and g_X are about 0.4, 0, and 0.4 MHz, respectively. The similarity of the splittings at g_Z and g_X shows that the nqi tensor is very rhombic, with an asymmetry parameter, η, of ~1. In contrast, for the axially symmetric model compound [Mo(V)¹⁷O(SPh)⁴]⁻ an axial nqi tensor was obtained (η = 0). From the largest splitting of 0.4 MHz (at g_X and g_Z), using the expressions given in ref. 30, the quadrupole coupling constant can be estimated as e²Qq/h = (40/12)·Δν_σ ~ 1.3 MHz.

Fig. 5.

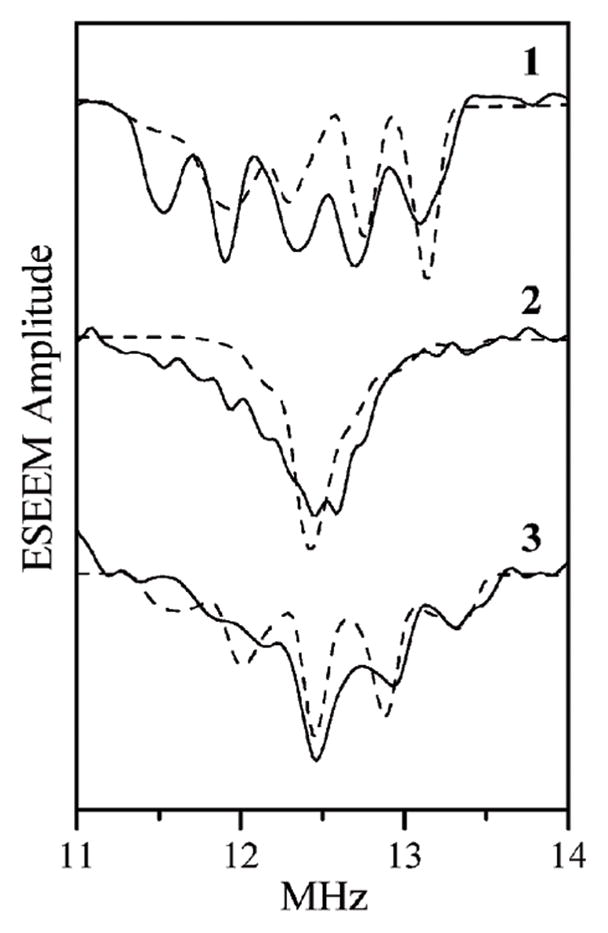
¹⁷O sum combination line regions of τ-integrated four-pulse ESEEM spectra (cosine FT). Solid traces, experimental for wt hpH cSO, obtained as a difference between the spectra of samples incubated for 3 h and 2 min in H₂ ¹⁷O buffer. Magnetic fields for traces 1 through 3, B_o = 1056.5 mT (g_Z), 1067.7 mT (g_Y) and 1073 mT (g_X), respectively. Experimental conditions: mw frequency, 29.38 GHz; mw pulses, 14, 14, 22 and 14 ns; temperature, 21 K. Dashed traces, calculated with the same parameters as in Fig. 4.

Using the above starting estimates of the hfi and nqi parameters, numerical simulations of the ESEEM spectra were performed. While the number of variable parameters involved in such simulations is rather large (a total of eleven), the nqi of the ¹⁷O-oxo is relatively weak and mostly results in minor broadening of the fundamental lines without affecting their positions (which are determined by the hfi). In contrast, the shape and features of the ${\nu }_{\sigma }^1$ quintet are mostly determined by the nqi. The sensitivity of different spectral features to different interactions has effectively uncoupled the variation of the hfi- and nqi-related parameters and has significantly simplified the entire simulation process. The parameters resulting from the simulations are summarized in Table 1.

Table 1.

The hfi and nqi parameters of exchangeable ¹⁷O nuclei in the vicinity of Mo(_V) center of hpH SOEs, as determined by ESEEM measurements in this work. For equatorial ¹⁷O the sign of a_iso is taken negative based on the DFT calculation results shown in Fig. 10. Correspondingly, the signs of the anisotropic hfi components for the equatorial ¹⁷O are also opposite to those in the text

Type of 17O (enzyme)	aiso/MHz	(T11,T22,T33)/MHz	(ψh,θh,ϕh)	e2Qq/h/MHz	η	(ψq,θq,ϕq)
oxo-17O (wt cSO)	6.3 ± 0.1	(2.4, 1, −3.4) ± (0.1, 0.1, 0.2)	(55°, 20°, 310°) ± (10°, 5°, 10°)	1.6 ± 0.1	0.9 ± 0.1	(65°, 25°, −5°) ± (10°, 5°, 10°)
Equatorial 17O (wt cSO)	−37	(−2.5, −2.5, 5)	~ (0°, 0°, 0°)	—	—	—
Equatorial 17O (wt SDH)	−37	(−2.5, −2.5, 5)	~ (0°, 0°, 0°)	—	—	—
Equatorial 17O (Y236F SDH)	−39	(−2.5, −2.5, 5)	~ (0°, 0°, 0°)	—	—	—
Weakly coupled 17O (wt cSO)	4	|Tii| ≤0.5	—	—	—	—
Weakly coupled 17O (wt SDH)	3	|Tii| ≤ 0.5	—	—	—	—

Examples of simulated spectra are shown by dashed lines in Fig. 4 and 5. The assignment of various features seen in the spectra of Fig. 4 is given in the ESI. † The overall quality of the simulations is quite reasonable. However, it is obvious from Fig. 5 that while the dependence of the nqi splittings of the ν_σ feature on the EPR position is qualitatively reproduced, the exact lineshapes are not. Although the variation of the nqi parameters and the orientation of the nqi tensor with respect to the g-frame (within the limits shown in Table 1) improved the agreement in some of the spectra (e.g., at g_Z), it was always achieved at the expense of worsening the agreement in other spectra (e.g., at g_X). A possible reason for the inabilty to obtain “perfect” simultaneous fits for all of the spectra is the fact that the simulations were performed for fixed magnetic resonance parameters. In reality, however, some static distribution of these parameters is always present, mainly caused by the orientational distribution of the equatorial OH ligand. The distributions of different parameters (principal g-values, hfi, and nqi tensors and their orientations) are correlated if they result from common structural variations. Including such correlated distributions into the simulations could arguably improve the quality of the fit. However, we did not attempt such an improvement in this work because doing so would be very time-consuming and largely cosmetic, and since the distribution widths and the corrections to the mean values of the parameters are expected to be small (within the error limits shown in Table 1).

It is interesting to note that the amplitude of the experimental two-pulse ESEEM spectral lines could only be reproduced if the simulated spectrum amplitude was divided by 11 (see Fig. 4). This indicates that even for the long-incubation sample in buffer containing about 60% H₂ ¹⁷O, only about 10% of the Mo(V) centers had their axial oxo ligand exchanged to ¹⁷O.

3 Strongly coupled ¹⁷O of the equatorial OH ligand

The strongly coupled ¹⁷O of the exchangeable equatorial ligand was observed by HYSCORE spectroscopy at K_a band (~30 GHz) for lpH SO^28,48 and the blocked form of R160Q mutant hSO.¹⁹ The hfi constants estimated for these systems were about 26 MHz and 18 MHz, respectively. Based on the CW EPR splittings, the expected hfi constant for the hpH form of SO or SDH is significantly larger.²⁷ We have never been able to observe the HYSCORE correlation peaks for the hpH SOEs, probably because the incomplete excitation results in the amplitude of the high-frequency lines being too small to be detectable. We have found, however, that in 1D ESEEM spectra, the low-frequency line is observable, and from the position of this line, the hfi constants at different EPR positions can be estimated.

The low-frequency line of the ¹⁷OH ligand (indicated by a diamond symbol in spectrum 1 of Fig. 3a) is readily observed in the two-pulse ESEEM spectrum obtained at g_Z. Unfortunately, at higher magnetic fields this line decreases in amplitude and shifts to higher frequencies where it overlaps with the (negative) sum combination line. Therefore, this line becomes effectively undetectable in the two-pulse ESEEM spectra. In order to observe it, three-pulse ESEEM was used since the spectra of the three-pulse ESEEM only contain the fundamental lines and not the homonuclear sum combination lines. As for the heteronuclear combination lines, they are completely negligible because the overall ¹⁷O ESEEM amplitude in K_a band is rather small (~10%).

To minimize the contribution of the axial ¹⁷O-oxo ligand, samples prepared with short incubation time were used. In addition, to eliminate the blind spots that corrupt the spectra at any fixed time interval τ between the first two mw pulses, τ-integrated stimulated ESEEM was used. The idea behind this approach is as follows. The three-pulse ESEEM harmonics can be generally written as:⁴⁹

$$\begin{array}{l}V(\tau ,T)=(A+Bcos({\omega }_{\alpha (\beta )}\tau ))·cos({\omega }_{\beta (\alpha )}(\tau +T))\\ \equiv (A+Bcos({\omega }_{\alpha (\beta )}\tau ))·cos({\omega }_{\beta (\alpha )}t)\end{array}$$ (2)

where A and B are constants, τ was already defined above, T is the time interval between the second and third mw pulses (thus, t = τ + T is the interval between the first and third pulses), and ω_α and ω_β are the frequencies of nuclear transitions within the α and β electron spin manifolds. For a nuclear spin I = 1/2 there is only one ω_α and one ω_β. For arbitrary I, there are I(2I + 1) transitions within each electron spin manifold, and all possible combinations of ω_α and ω_β enter the two time-dependent factors of eqn (2). A two-dimensional (2D) three-pulse ESEEM experiment can be performed with the two time coordinates being τ and t=τ+T. In order to be able to start each of the traces along t at the same initial interval t_o (irrespective of τ), the experiment has to be performed using swapping of the mw pulses.⁵⁰ Summing up all of the traces recorded at different τ-values (effectively, integrating the 2D data set over τ) yields a 1D integrated three-pulse ESEEM with the general term being simply A·cos ω_β(α)t.

The spectra of the τ-integrated stimulated ESEEM of wt hpH cSO are shown in Fig. 6. In order to suppress the baseline rolling caused by non-zero dead time (t_d ~ 150 ns) and make the lines from the equatorial ¹⁷O easily observable, the ESEEM traces before the FT were extrapolated into the dead time using the FT slicing (FTS) algorithm.⁵¹ The spectra of the original ESEEM without extrapolation are shown in ESI.†

Fig. 6.

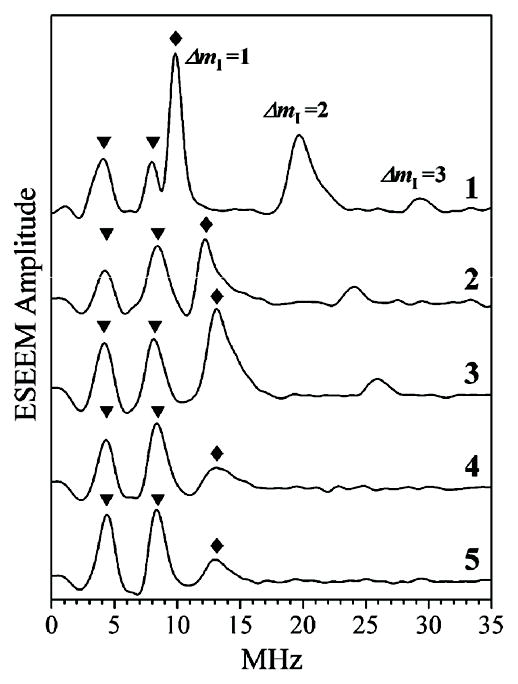
Traces 1 through 5, τ-integrated three-pulse ESEEM spectra (cosine FT) of wt hpH cSO for the samples incubated for 2 min in H₂ ¹⁷O buffer, obtained at B_o=1071.8 mT (g_Z), 1079.5 mT, 1083.4 mT (g_Y), 1086 mT and 1088.6 mT (g_X), respectively. Before the FT, the experimental ESEEM traces were extrapolated into the dead time using FTS algorithm.⁵¹ Experimental conditions: ν_mw = 29.810 GHz; mw pulses, 3 × 13 ns; temperature, 21 K. Diamonds and triangles indicate the Δm_I = 1 fundamental lines of equatorial ¹⁷O (from the OH ligand) and unidentified weakly coupled ¹⁷O, respectively. The higher-frequency lines belong to Δm_I = 2 and Δm_I = 3 fundamental lines of equatorial ¹⁷O.

The low-frequency fundamental line of the equatorial ¹⁷O in each spectrum of Fig. 6 is marked by a diamond. This line belongs to the Δm_I = 1 transitions of ¹⁷O within one of the electron spin manifolds. While the frequency of −1/2 ↔ 1/2 nuclear transition does not depend on the nqi to first order, the frequencies of all other transitions do. However, the largest splitting between the separate lines is only about Δν_max ~ 0.15 e²Qq/h.³⁰ A quadrupole coupling constant of e²Qq/h ~ 7 MHz (similar to that in H₂O^52–54) would give a Δν_max of ~1 MHz. This splitting is smaller than the experimental linewidth (~1.5 MHz), and it is likely to be masked by even a slight statistical distribution of the hfi constant about the mean value of ~30 MHz (see below). As a result, all of the Δm_I = 1 fundamental lines within the same electron spin manifold merge into a single feature observed in the spectra of Fig. 6. The hfi constant at each EPR position can be found from the frequency of this feature as |A| = 2 (|ν_α| + |ν_O|), where ν_α is the assumed assignment of the experimental ESEEM frequency and ν_O is the Zeeman frequency of ¹⁷O.

Fig. 7 shows the dependence of the hfi constant A on the EPR position obtained for wt hpH cSO (black squares). From the full range of the variation of A, one can estimate the anisotropic hfi constant as T_⊥ ≈ 2.5 MHz (assuming the interaction to be axial). The fact that the hfi constant reaches its minimum at g_Z shows that g_Z approximately corresponds to the canonical orientation of the anisotropic hfi. The isotropic constant is estimated as a_iso = 37 MHz.

Fig. 7.

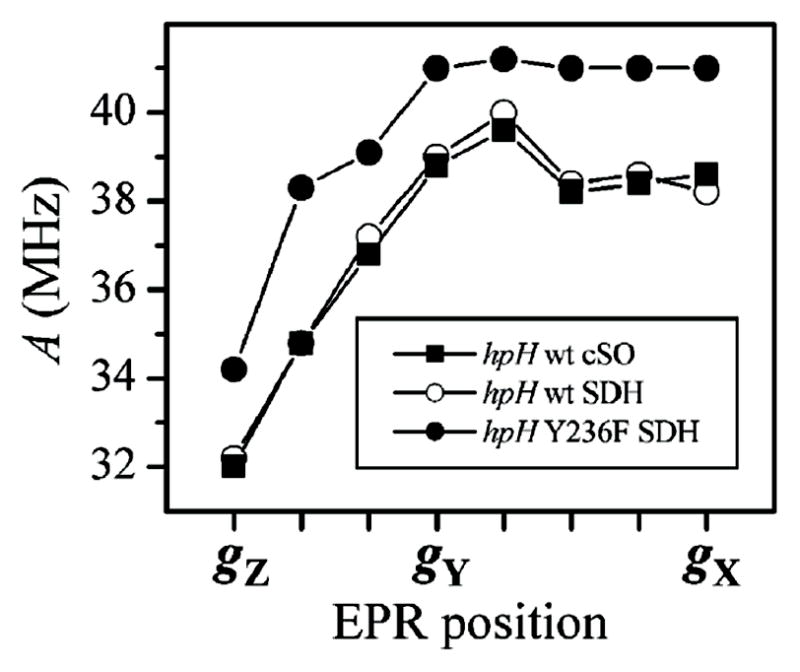
Hyperfine constants of the equatorial ¹⁷O ligand of several different hpH SOEs as a function of the EPR position, calculated from the position of the experimental fundamental line ν_α as A = 2(ν_α + ν_O).

Similar measurements were performed for the samples of hpH wt and Y236F SDH (open and black circles in Fig. 7). For wt SDH, the ¹⁷O hfi parameters of the equatorial OH ligand are similar to those obtained for hpH cSO, while for hpH Y236F SDH the isotropic hfi constant is slightly larger (39 MHz). In all samples of hpH SO and SDH, the hfi constant was considerably larger than for lpH SO (37–39 MHz vs. 26 MHz²⁸).

An interesting feature of the experimental spectra of the equatorial ¹⁷O is that, while they do not show the high-frequency line of Δm_I = 1 transitions, they do show a series of lines with progressively decreasing amplitudes corresponding to Δm_I = 2, 3, etc., located at the frequencies that are integer multiples of the low-frequency line discussed above. These lines are most clearly seen in the spectrum obtained at g_Z, where the amplitude of the Δm_I = 1 line is the largest (see the Δm_I = 2 and Δm_I = 3 features at the frequencies of ~20 and ~30 MHz in trace 1 of Fig. 6).

4 Weakly coupled ¹⁷O

In addition to the ligand ¹⁷O nuclei, we have also observed a weakly coupled ¹⁷O nucleus with hfi parameters that are very different from those of the axial oxo ligand (see Fig. 6, lines marked by triangles). For wt hpH SO, this oxygen nucleus does not show much hf anisotropy, and the splitting between the lines of ~4 MHz gives a good estimate of the isotropic hfi constant, a_iso. The lack of visible orientation dependence of the splitting between the lines indicates that the anisotropic hfi constant does not exceed 1 MHz. No nqi-related splittings of the sum combination line are observed in either two-pulse or integrated four-pulse ESEEM spectra, which makes estimating the nqi impossible. The hfi constant for wt hpH SDH is slightly smaller, about 3 MHz. For hpH Y236F SDH, no additional weakly coupled ¹⁷O could be reliably observed.

5 Structural implications

The lpH and hpH forms are the two distinct forms of the Mo(V) center of SOEs that contain an equatorial OH ligand. The large isotropic hfi constant of the OH ligand proton observed in the lpH form and the nearly zero hfi constant for this proton in the hpH form have been linked to the orientation of the OH ligand with respect to the Mo center.²⁰ Similar explanations were put forward to rationalize the OH ligand proton hfi constant in other molybdoenzymes, namely dimethyl sulfoxide reductase (DMSOR)⁵⁵ and xanthine oxidase.^56,57 Thus, in the lpH form, the OH ligand was assumed to be nearly parallel to the equatorial plane, while in the hpH form it was thought to be oriented out of the equatorial plane (with a dihedral angle between the planes formed by O==Mo–O(H) and (O==)Mo–OH close to 90°). The orientation of the OH ligand has been, in fact, the only obvious and explicitly discussed major structural difference between the lpH and hpH forms.

In order to determine if the OH orientation alone could account for the spectroscopic differences between the lpH and hpH forms, DFT calculations were performed. The orientation of the OH ligand in the calculations was described by the dihedral angle, θ_OH, the Mo–O–H plane forms with the O==Mo–O(H) plane. The angle θ_OH = 0° corresponds to the OH being about parallel to Mo==O bond, while θ_OH = 90° corresponds to OH being about parallel to Mo–S(MPT) bond and θ_OH = 270° corresponds to OH being about parallel to Mo–S(Cys). The structural model used in the calculations and described in the Materials and methods section is shown in Fig. 8. Possible protein interactions that may impose restrictions on the range of θ_OH were not taken into account in this preliminary work.

Fig. 8.

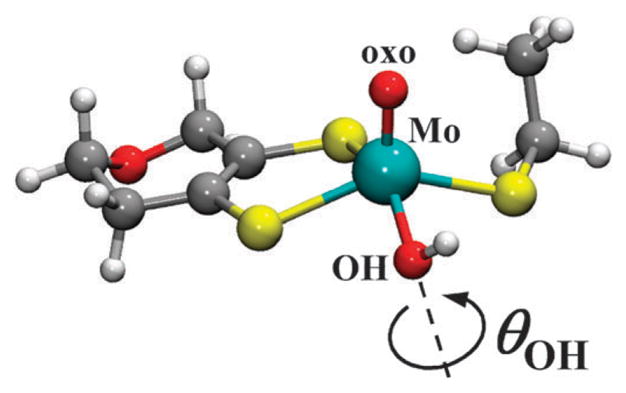
Representative geometry-optimized computational model (where θ_OH = 330°) from which the various spectroscopic parameters were calculated as a function of θ_OH.

a Oxo-ligand

The hfi and nqi of the ¹⁷O-oxo in the enzyme are similar in magnitude to those in the [Mo(V)¹⁷O(SPh)⁴]⁻ model compound. The most notable difference is in the rhombicity of the interactions, which is related to the lack of the axial symmetry in the ligand surroundings of the Mo(V) center of SO. The main effect of this asymmetry is to make the d_xz and d_yz orbitals of Mo(V) electronically inequivalent, which results in different spin polarizations of and electron delocalizations from the p_x and p_y orbitals of the oxo-ligand that are overlapping with these d-orbitals. Since the orbitals of the equatorial OH ligand also overlap with the d_xz orbital, and their overlap is dependent on the OH ligand orientation, it is reasonable to expect that the OH ligand orientation will affect the inequivalence of the d_xz and d_yz orbitals and the hfi and nqi parameters of the ¹⁷O-oxo.

Fig. 9 shows the results of DFT calculations of the hfi and nqi parameters of the ¹⁷O-oxo ligand as a function of θ_OH. The calculated a_iso and e²Qq/h are about 40% smaller than the respective experimental values. The DFT results presented elsewhere³⁰ for the model synthetic complex [Mo¹⁷O(SPh)₄]⁻ and for the Mo center of lpH SO²⁶ were of similar quality. Currently, the origins of the low accuracy of the calculated parameters for the ¹⁷O-oxo ligand are not clear since the above two reports, this work, and earlier CW EPR publications^58,59 represent the only available sources of experimental data that could be used for comparison and refinement of the DFT methodology as applied to oxo-Mo(V) systems.

Fig. 9.

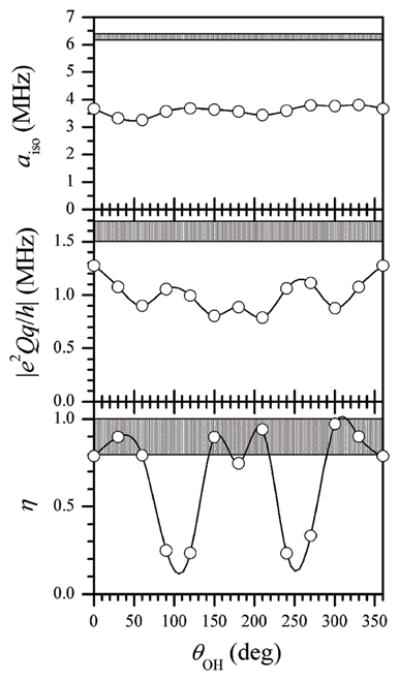
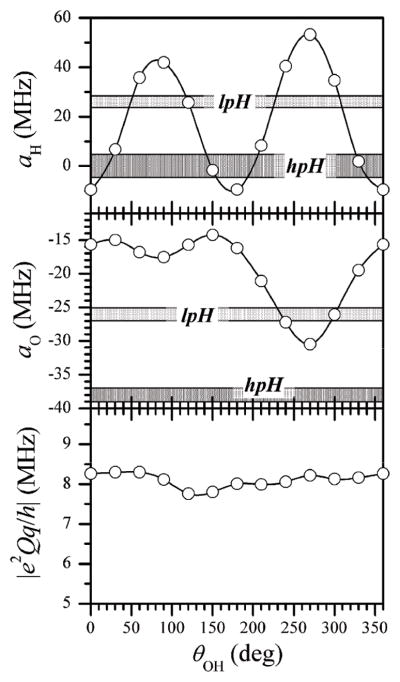
Hyperfine and quadrupole interaction parameters of the oxo-¹⁷O ligand calculated by DFT as a function of the orientation of the OH ligand. Grey strips correspond to the experimental values.

Keeping the accuracy issues in mind, it is still interesting to note that, while the calculated a_iso and e²Qq/h are practically independent of θ_OH, the nqi asymmetry parameter, η, shows a strong dependence on θ_OH. When the OH ligand is near the equatorial plane (θ_OH~90° and 270°), the nqi tensor becomes nearly axial (η ~ 0), while for the OH direction being significantly out of the equatorial plane, the nqi tensor becomes very rhombic. In particular, for θ_OH corresponding to small hfi constants of the OH ligand proton (see below) the calculated η approaches 1, in agreement with our experimental measurements.

b Equatorial OH ligand

Fig. 10 shows the calculated ¹H and ¹⁷O hfi constants for the equatorial OH ligand. The proton hfi constant is mostly determined by the overlap of the OH bond orbital with the Mo(V) d_xy orbital and is rather accurately described by a simple approximate expression: a_H~ −9.5+57·cos²(θ_OH+90°) [MHz]. In contrast, the oxygen hfi constant, a_O, has comparable contributions from the valence orbitals that are not involved in σ-bonding with Mo(V). If all three of these orbitals were strictly equivalent and the geometry of the Mo(V) SOMO were perfectly planar (d_xy orbital), then a_O would not depend on θ_OH. However, the OH bond orbital is not equivalent to the two lone pair orbitals, and this results in the oscillating dependence of a_O.

Fig. 10.

Hyperfine and quadrupole interaction parameters of ¹H (top panel) and ¹⁷O (middle and bottom panels) of the OH ligand calculated by DFT as a function of the orientation of this ligand. Dark and light grey strips indicate the experimental values for hpH and lpH SOEs, respectively. For the ¹⁷O quadrupole coupling constant the experimental value is unavailable.

Throughout the complete range of θ_OH, a_O remains negative, indicating the presence of total positive spin population in the oxygen valence orbital system. The first minimum of a_O (maximum of |a_O|), at θ_OH ~ 90°, is much less pronounced then the second minimum, at θ_OH ~ 270°. A similar effect is also seen for a_H (the two oscillations have somewhat different sizes), although it is much less pronounced than that observed for a_O. The inequivalence of the situations with θ_OH~90° and 270° obviously reflects the slight asymmetry of the Mo(V) SOMO related to the electronic inequivalence of the Mo–S(MPT) and Mo–S(Cys) bonds, as well as the geometrical distortions of the Mo(V) center in SOEs.

The experimental hfi constants for the equatorial hydroxo ¹H and ¹⁷O in lpH SO are (a_H, |a_O|) ≈ (26, 26) [MHz], and they are reasonably reproduced by the DFT calculation at θ_OH ~ 230° and 310° (see Fig. 10). In contrast, the hfi constants (a_H, |a_O|) ≈ (0, 37) [MHz] found for hpH SO are not reproduced simultaneously at any θ_OH. Moreover, the DFT calculations predict a synphase variation of a_H and |a_O| as a function of θ_OH, which seems to be incompatible with the experimental observation that the smaller experimental a_H corresponds to the larger experimental |a_O|. Since even the predicted trend of the variation of a_H and a_O with θ_OH in this case is in disagreement with the experimental results, it is very likely that the discrepancy is not related to a limited accuracy of the DFT calculations, but is rather caused by an overly simplistic structural model that does not include any interactions of the ligands (mostly, the OH ligand) with second sphere molecules. The observation of the weakly coupled ¹⁷O in this work and an earlier observation of two (rather than one) nearby exchangeable protons in hpH SO⁴⁶ each testify in favor of this explanation.

Finally, the bottom panel of Fig. 10 shows the ¹⁷O-hydroxo quadrupole coupling constant estimated by DFT. The calculated constant is virtually independent of θ_OH, always remaining close e²Qq/h ~ −8 MHz. The nqi asymmetry parameter also did not show much variation with θ_OH, always remaining close to 0.6 (η = 0.58 ± 0.05). Both nqi parameters can obviously change if the H-bonding of the hydroxo ligand is incorporated into the calculation.

c Weakly coupled ¹⁷O

The origin of the observed weakly-coupled exchangeable ¹⁷O is not immediately clear. From general considerations it is evident that an isotropic hfi constant of several MHz can only be caused by a relatively efficient through-bond propagation of spin density from the Mo(V) center to the oxygen atom in question. Several conceivable structural models that could allow for this possibility are shown in Fig. 11, including ¹⁷OH (or H₂ ¹⁷O) (a) weakly coordinated at the axial position trans to the oxo ligand; (b) hydrogen-bonded to the oxygen of the equatorial hydroxo ligand; (c) hydrogen-bonded to the hydrogen of the equatorial hydroxo ligand; or (d) SO₄ ²⁻ hydrogen-bonded to the equatorial OH ligand in the active site pocket of the protein in vitro. Model (d) requires that the H-bonded oxygen of the sulfate be ¹⁷O (presumably formed during the reaction of sulfite with the ¹⁷O labeled Mo(VI) center).

Fig. 11.

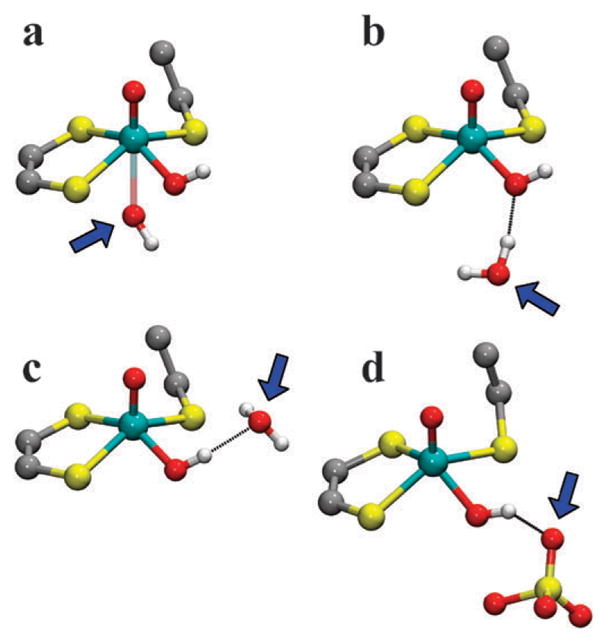
Structural models that may account for the ESEEM observation of the weakly coupled exchangeable ¹⁷O at the Mo(V) center of hpH SOEs (indicated by a blue arrow in each structure): ¹⁷OH (or H₂ ¹⁷O) (a) weakly coordinated at the axial position trans to the oxo ligand; (b) hydrogen-bonded to the oxygen of the equatorial hydroxo ligand; (c) hydrogen-bonded to the hydrogen of the equatorial hydroxo ligand; or (d) ¹⁷OSO₃ ²⁻ hydrogen-bonded to the equatorial OH ligand.

As a preliminary consideration, model (a) may prove unrealistic due to electronic reasons and because the available space between the Mo center and the active site Arg residue would be insufficient to accommodate such an axial ligand.²⁵ Models (c) and (d) are unlikely to result in any measurable hfi constant of the H-bonded remote oxygen because the H-bond is nearly perpendicular to the plane of the Mo(V) d_xy orbital. Only model (b) is expected to result in a measurable a_iso of the remote ¹⁷O. Work is currently underway to investigate each of these possibilities using DFT. However, unlike the simple DFT calculations discussed above, these calculations must include interactions with nearby protein surroundings that would stabilize the position of the second sphere OH or SO₄ ²⁻. This will be the subject of a separate work.

Summarizing the results obtained for the hpH SOEs from vertebrates and bacteria, we may note that similar hfi parameters of the ¹⁷O ligands, taken together with the earlier data for the OH ligand proton^21,22 and the similarity of the g-tensors, suggest that the Mo center structures in the hpH SOEs of these very different organisms are nearly identical.

The Y236F mutation has no substantial effect on the hfi constant of the equatorial ¹⁷O. However, if the tyrosine were hydrogen-bonded to the OH ligand, an appreciable difference would be expected between the wt and mutant enzymes. Instead, the weakly coupled oxygen that was detected for the wt enzymes became unobservable for Y236F SDH. A possible explanation of these observations is that the tyrosine in hpH SOEs does not interact directly with the equatorial hydroxo ligand, but rather forms a hydrogen bond to a second sphere ¹⁷O-containing species (likely, OH or H₂O) detected in the ESEEM spectra. Substitution of the tyrosine by phenylalanine removes the hydrogen bond to this species and results in a change of its position relative to the OH ligand. Such structural rearrangement may lead to a significantly smaller spin density delocalization onto the remote ¹⁷O, rendering it unobservable by ESEEM.

Conclusions

In this work, we have used ¹⁷O ESEEM spectroscopy to study the exchangeable oxygens in samples of hpH cSO and SDH. For the exchangeable oxo ligand of hpH cSO, detailed high resolution data were obtained and analyzed. For the equatorial hydroxo ¹⁷O, the hfi parameters were accurately measured for the first time, although the nqi parameters could not be determined. Because of the very strong hfi of the hydroxo ¹⁷O in hpH SOEs, the reliable observation of ESEEM spectra for this oxygen could only be done using the τ-integrated three-pulse ESEEM. In addition to the two ligand ¹⁷O nuclei, a third ¹⁷O nucleus was detected. This oxygen nucleus has a nearly isotropic hfi, and it is likely to belong to a second-sphere OH hydrogen-bonded to the equatorial hydroxo ligand.

The preliminary DFT calculations were performed using a simplified structural model that did not include any interactions of the Mo(V) center or its ligands with any other group. The θ_OH dependences of ¹H and ¹⁷O hfi constants for the hydroxo ligand, were incompatible with the combined experimental results for the lpH and hpH forms of SOEs. This indicates that θ_OH is not the only structural parameter responsible for spectroscopic differences between the lpH and hpH forms. More advanced computational models that accurately reflect the structure of the active site should clarify other structural differences and establish the origin of the remote weakly coupled ¹⁷O. This work is currently in progress.