Human odometer is gait-symmetry specific

Abstract

In 1709, Berkeley hypothesized of the human that distance is measurable by ‘the motion of his body, which is perceivable by touch’. To be sufficiently general and reliable, Berkeley's hypothesis must imply that distance measured by legged locomotion approximates actual distance, with the measure invariant to gait, speed and number of steps. We studied blindfolded human participants in a task in which they travelled by legged locomotion from a fixed starting point A to a variable terminus B, and then reproduced, by legged locomotion from B, the A–B distance. The outbound (‘measure’) and return (‘report’) gait could be the same or different, with similar or dissimilar step sizes and step frequencies. In five experiments we manipulated bipedal gait according to the primary versus secondary distinction revealed in symmetry group analyses of locomotion patterns. Berkeley's hypothesis held only when the measure and report gaits were of the same symmetry class, indicating that idiothetic distance measurement is gait-symmetry specific. Results suggest that human odometry (and perhaps animal odometry more generally) entails variables that encompass the limbs in coordination, such as global phase, and not variables at the level of the single limb, such as step length and step number, as traditionally assumed.

1. Introduction

For many animals, travel from and to home (e.g. nest or hive) is a seemingly continuous process that can occur without the use of familiar places as landmarks, relying instead on the stimulation made available by locomotor activity (Etienne & Jeffery 2004). The process is referred to as path integration, and the class of information derived strictly from the animal's locomotion is labelled idiothetic (Mittelstaedt & Mittelstaedt 1982). In the outbound journey the incremental integration of direction and distance ensures a constant updating of the home vector. Achieving the update in the absence of vision (i.e. in darkness or blindfolded) relies on idiothetic information about movement with respect to the substrate and with respect to inertial space (the general background of resistance to acceleration). The haptic perceptual system detects the former, whereas the vestibular system detects the latter. When both kinds of information are available, the substratal variant appears to be predominant (Mittelstaedt & Mittelstaedt 2001).

The haptic perceptual system is the perceptual system by which the body and the adjacent environment are perceived by means of the body (Gibson 1966). The variant of haptic sensibility that is tied to the mechanoreceptors of the muscles and related tissues—the haptic sensibility of primary significance to the detection of the substratal variant of idiothetic information—is referred to as dynamic touch (also known as effortful touch, kinaesthetic touch, muscle-based perception). Dynamic touch is at work proprioceptively whenever segments of the body are moved relative to the body and to each other, and exteroceptively whenever an object is wielded, lifted, hefted, pushed or pulled, or a part of the environment is contacted with a handheld implement (Turvey & Carello 1995; Turvey 1996; Carello & Turvey 2004). It is also at work exteroceptively whenever the body is displaced by muscle power (e.g. legged locomotion) relative to the substrate. A mobile organism can come to know about the environment, and its relation to it, by extended episodes of mechanical, tactile contact, as Berkeley highlighted three centuries ago.

In his ‘Essay towards a new theory of vision’, Berkeley (1709, section 45, p. 188) asserted for the human that distance is measurable by ‘the motion of his body, which is perceivable by touch’. The assertion suggests the hypothesis that legged locomotion from a location A to another location B is, in and of itself, specific to the distance from A to B. For this to be so, however, a very special kind of perceptual constancy must hold. A stretch of non-visible ground between A and B can be traversed at different speeds and in different styles of gait. Berkeley's hypothesis requires that legged locomotion without vision must yield an unvarying impression of the distance traversed despite variations in the manner of legged locomotion.

That legged locomotion without vision might yield an invariant measure of distance is suggested by investigations with mammals (e.g. Seguinot et al. 1998), arachnids (e.g. Seyfarth & Barth 1972), arthropods (Walls & Layne 2009) and insects (e.g. Wohlgemuth et al. 2001). It is similarly suggested by an investigation using a simple homing task with blindfolded human participants (Schwartz 1999). On any given trial, a participant went from a fixed starting point A to a variable terminus B—signalled during locomotion by the experimenter—and then attempted to return to A. From A to B (distances from 5 to 50 m) the participants either walked with the aid of a long cane to prevent veering from the path or jogged with the aid of a sighted partner, similarly to prevent veering from the path. Additionally, in both modes of travel between A and B, the participant was distracted by conversation with the sighted partner. From B to A, participants walked alone with the aid of a long cane and without distraction. Schwartz (1999) found that the return trip matched the outgoing trip and did so equally for both modes. Taking the accuracy of the return trip as the index of perceived distance in the outgoing trip, the implication is that distance is perceptible by legged locomotion and is so indifferently to travel duration, number of steps and style of locomotion. This implication is strengthened by the further observation by Schwartz (1999) that perceived distances conform closely to actual distances over combinations of systematic variations in step cadence and step length.

On the issue of the basis of distance perception by legged locomotion, Schwartz recommended (1999, p. 863) examination of the ‘walking activity itself as the possible source of information’ (as opposed to cognitive-like operations such as counting steps or estimating duration) with the focus upon dynamic touch as the means by which it is perceived. The present research follows Schwartz's recommendation.

We manipulated human bipedal locomotion according to a distinction between primary and secondary gaits revealed in symmetry group analyses of locomotion patterns exhibited by networks of coupled identical ‘cells’ (defined by systems of ordinary differential equations and likened to central pattern generators; Golubitsky et al. 1999; Pinto & Golubitsky 2004, 2006). To encompass the gaits exhibited by bipeds, quadrupeds and so on requires a network of twice as many cells as the animal has legs. In primary gaits, the cells send the same signal, up to a phase shift. In secondary gaits, the cells send two different signals, exhibited as two different waveforms. Primary gaits have more symmetry than secondary gaits.

2. Material and methods

(a). Participants

There were 44 participants in total: ten in each of experiments 1, 2 and 4, five in experiment 3, and nine in experiment 5. All were students from the University of Connecticut. Undergraduate participants provided informed consent and received partial course credit. The university's institutional review board approved the experimental protocols.

(b). Material and design

The five experiments were conducted in an indoor basketball court measuring 30.5 × 15.2 m. The starting point A was marked with tape, as were three target distances (7.6 m, 15.2 m, 22.9 m). To accommodate possible straying from a straight line, three semi-circles with radii measured from A of 7.6, 15.2 and 22.9 m were taped on the floor. In experiments 1 and 5, participants, on reaching the target radius, were turned clockwise either 180° (to face A directly) or 145°. Experiments 2, 3 and 4 did not include the 145° condition. In each experiment there were a single return (‘report’) gait and two outbound (‘measure’) gaits, one of which was used as the return gait, that were randomized across trials. There were 36 trials (three at each distance, direction, outbound gait combination) in experiments 1 and 5, and 24 trials (three at each distance, outbound gait combination) in experiments 2–4. A blindfold and noise-reducing headphones were worn continuously throughout the trials. A stopwatch and a tape measure were used to measure the outbound and return durations and distances.

(c). Procedure

In each of experiments 1–5, participants received preparatory training in the two outbound gaits and in straight-line locomotion with their eyes closed. Typically, three training runs, in which participants visually checked their performance, were sufficient. They were then instructed about the experimental task: to travel from the starting point by the assigned outbound gait at a comfortable pace, to stop when an experimenter audibly signalled stop, and to reproduce with the assigned and fixed return gait the outbound distance. Participants were not informed about the number of outbound distances, nor that the distances and number were the same for both outbound gaits. They were instructed to avoid counting steps. Before each trial the outbound gait for the trial was identified. The experimenter giving the ‘stop’ instruction walked or jogged close by, without contacting the participant, ensuring an audible signal and safe and straight-line travel. After stopping at the target or B radius for the trial, another experimenter recorded the duration, number of steps and actual distance of outbound travel (the participant could stop just short or just beyond the target radius). The participant was then turned and told to reproduce the outbound distance using the return gait identified for the experiment, with the experimenter walking or jogging close by. On completion of the trial, return distance, duration and number of steps were recorded with the participant then guided back to location A on a path that varied from trial to trial.

3. Experiments and results

(a). Experiment 1

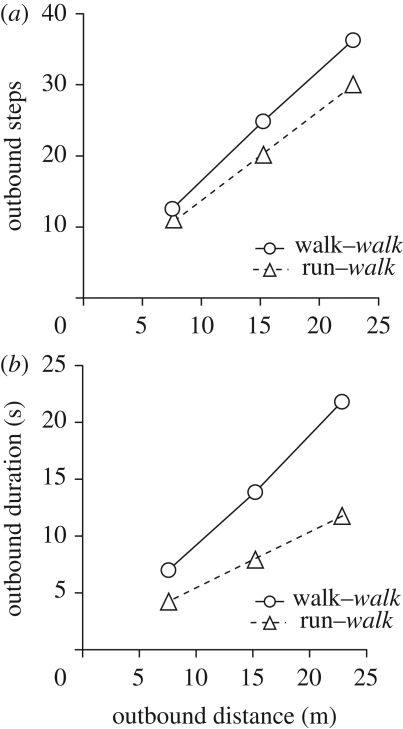
In experiment 1, the gaits by which participants measured the target distances were the primary gaits walk and run (more exactly, jog). The gait by which the participants reported the measured distances was walk. The target distances of 7.6, 15.2 and 22.9 m were reported, on average, to be 8.98 ± 1.24, 15.76 ± 1.67 and 22.25 ± 2.03 m when measured by walk, and 9.20 ± 1.08, 16.27 ± 1.76 and 21.99 ± 2.64 m when measured by run. Even though analysis of variance revealed that walk used more steps (F(1,9) = 29.94, p < 0.0001) and took more time (F(1,9) = 156.71, p < 0.0001) than run (figure 1), the reproduced distances were indifferent to the measuring gait (F(2,18) = 1.36, p = 0.28) and to the angle of return (F(2,18) = 2.65, p = 0.10). In brief, our results (figures 1 and 2a) confirmed those of Schwartz (1999), whose participants measured distances of 10 to 50 m by walk or run and reported them by walk.

Figure 1.

Outbound steps (a) and outbound duration (b) as a function of outbound distance travelled blindfolded by walk and travelled blindfolded by run in experiment 1. Upright text, outbound gait; italic text, return gait.

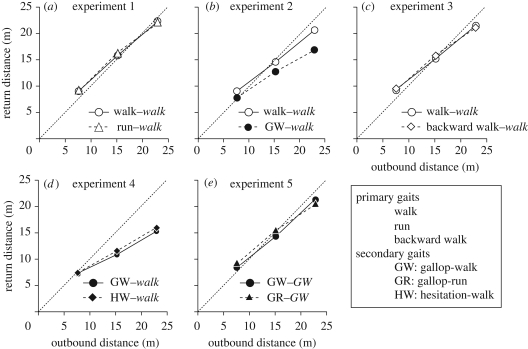
Figure 2.

Return distance as a function of outbound distance. Open symbols indicate that outbound gait was primary; filled symbols indicate that outbound gait was secondary. Upright text, outbound gait; italic text, return gait.

As a hypothesized odometer, human legged locomotion does not seem to measure in units of time (it is not a timer) nor in units of steps (it is not a step counter or pedometer). It might, however, measure in units of stride length (it might be a stride length integrator; Wittlinger et al. 2006, 2007; Walls & Layne 2009). One would expect that for any distance measure made in units definable at the level of the single limb, gait should not be a factor, as figure 2a would seem to affirm. Experiments 2 to 4 suggested otherwise.

(b). Experiment 2

In experiment 1 (figure 2a) distances were measured by primary gaits (walk, run) and reported by a primary gait (walk). In experiment 2, distances were measured by either a primary gait (walk) or a secondary gait (gallop-walk) and reported by a primary gait (walk). Our question was whether distances reported by a primary gait would be independent of the symmetry (primary or secondary) of the measure gait. We implemented gallop-walk as a repetition of the rule ‘Step forward with the right foot, then bring the left foot into alignment with the right foot and pause’. As shown in figure 2b, there was a significant gait–distance interaction (F(2,18) = 4.02, p < 0.05), with the measure gait gallop-walk tending to result in increasingly smaller measures of distance (7.75 ± 1.48, 12.71 ± 1.77 and 16.81 ± 2.91 m) than the measure gait walk (8.99 ± 1.19, 14.52 ± 1.26 and 20.58 ± 3.52 m). Gallop-walk was slower than walk (F(1,9) = 156.71, p < 0.0001). Counting steps used in gallop-walk as the number made with the leading leg yielded fewer steps for gallop-walk (mean 17.3) than walk (mean 25.6; F(1,9) = 232.86, p < 0.0001). Counting steps of gallop-walk in terms of the steps by both the leading and lagging leg yielded more steps for gallop-walk (mean 34.6) than walk (F(1,9) = 137.61, p < 0.0001). In summary, the results of experiment 2 satisfy neither the pedometer hypothesis nor the stride integrator hypothesis. For the latter hypothesis, the sums of individual stride lengths in gallop-walk and walk should have been equal, meaning that the two measure gaits should have resulted in the same reported distance.

(c). Experiment 3

The two measure gaits of experiment 2 differed in familiarity in addition to the primary–secondary classification. Experiment 3 compared the less familiar primary gait backward walk with the highly familiar primary gait (forward) walk. The report gait was walk. Reported distances did not differ (F < 1) for backward walk and walk as measure gaits: 9.61 ± 1.71, 15.83 ± 2.32 and 21.04 ± 4.03 m, and 9.21 ± 1.34, 15.28 ± 2.35 and 21.42 ± 4.08 m, respectively (figure 2c). Number of steps (mean 28.18 versus mean 23.02) and duration (mean 17.19 s versus mean 14.00 s) were larger (all p < 0.0001) for backward walk. It can be assumed that the results of experiment 2 were not due to a familiarity difference.

Returning to experiment 1, although walk and run are primary gaits, and although the symmetry groups for both gaits are dihedral (Pinto & Golubitsky 2004, 2006), it is nonetheless the case that the component symmetries of the two gaits are not exactly the same (reflecting in part the pendulum nature of walking and the pogo stick nature of running). The results of experiment 1, and of Schwartz (1999), suggest that the difference between walk and run in component symmetries is not a difference of relevance to the measuring of distance by legged locomotion. The difference of relevance to distance measurement, as experiments 2 and 3 seem to show, is the primary versus secondary difference.

(d). Experiment 4

In experiment 4, distances were measured by secondary gaits (gallop-walk, hesitation-walk) and reported by a primary gait (walk). The so-called hesitation-walk (commonly used in wedding and graduation marches) is a repetition of the rule ‘Step forward with the right foot, then bring the left foot into alignment with the right foot and pause. Step forward with the left foot, then bring the right foot into alignment with the left foot and pause’. These secondary gaits are characterized by different symmetry groups (C. M. A. Pinto, personal communication, 8 January 2009) that translate in the gallop-walk having a lead leg and in the hesitation-walk having both legs doing the same set of movements, but a half period out of phase. It was expected that this difference would not manifest as a difference in reported distance. As shown in figure 2d, the two secondary gaits of experiment 4 measured distance equally (F < 1), like the two primary gaits of experiment 1. They did not, however, measure as accurately as the two primary gaits, especially at the two longer distances (gallop-walk, 7.37 ± 1.73, 11.00 ± 1.83 and 15.47 ± 2.69; hesitation-walk, 7.51 ± 1.78, 11.71 ± 2.69 and 16.18 ± 2.69). With steps in the gallop-walk counted as the number of lead leg steps and steps in the hesitation walk counted as the number of left and right steps, the gallop-walk exceeded the hesitation-walk (F(1,9) = 34.12, p < 0.0001); durations of travel, however, were not significantly different (F < 1).

(e). Experiment 5

The observed differences between primary and secondary gaits suggest that the measure of distance by legged locomotion, at least for the human, is not specific to the dynamics of the individual limb, but rather to the dynamics of the limbs as coordinated—that is, the dynamics determined by gait symmetry. Raising the issue of distance measure as specific to gait symmetry invites a reconsideration of our four experiments. In all four experiments, the gait used to reproduce or report perceived distance was walk, a primary gait. In experiments 1 and 3, both measure gaits were primary; in experiment 2 one measure gait was primary and one was secondary; and in experiment 4 both measure gaits were secondary. Experiment 5 replicated the design of experiment 1, with two secondary gaits as measure gaits, gallop-walk and gallop-run, and a secondary gait, gallop-walk, as the report gait. The protocol for gallop-run was that of the gallop-walk without the pause. Our expectation was that under the circumstances of the same gait category for measure and report, measurement of distances by secondary gaits would match measurement of distance by primary gaits.

This expectation derives from recognition of parallels between (i) experiments with humans (Schwartz 1999; Mittelstaedt & Mittelstaedt 2001) and ants (Wittlinger et al. 2006, 2007) of relevance to Berkeley's hypothesis, and (ii) experiments (with humans) on implicit memory, a category of memory that includes memory for actions (so-called procedural memory) as a subcategory (Schacter et al. 2000). In experiments of similar basic design to those presented here, Mittelstaedt & Mittelstaedt (2001) found that when humans walked at assigned speeds, accuracy of the report walk depended on whether the speed was the same or different for the report and measure walks, and Wittlinger et al. (2006, 2007) found that when ants walked with legs of an assigned length (elongated, shortened or normal), accuracy of the report walk depended on whether leg length was the same or different for the report and measure walks. Of particular significance is Schwartz's experiment 3, in which report walk was held constant (normal cadence, normal step length) for measure walks that varied in cadence (normal, 20 steps min⁻¹ lower than normal, or 20 steps min⁻¹ higher than normal) and step length (normal, or longer than normal). There were no main effects of measure cadence and step length on signed report distance, but overshoot at shorter distances (<30 m) and undershoot at longer distances (>30 m) were least when cadence and step length were invariant over report and measure.

In both the human and ant experiments, report accuracy was best when report locomotion and measure locomotion were of the same kind. Turning to (ii), one major perspective on implicit memory is that it depends on the kinds of processes and conditions shared between the original experiencing of an event (call it ‘study’) and the subsequent testing of the memory for that event (Neath & Surprenant 2003). That is, whether a form of study leads to good or poor performance on a memory test depends on the test's similarity to study. Translating for the present context: whether use of a particular style of legged locomotion to measure distance leads to a good or poor report of distance depends on the similarity of the report and measure styles of legged locomotion.

The expectation for experiment 5 was confirmed (figure 2e). Experiment 5 with secondary gaits for both measure and report reproduced the results of experiment 1 with primary gaits for both measure and report (figure 2a). Gallop-walk (8.38 ± 0.67, 14.22 ± 1.00, 21.33 ± 1.19) and gallop-run (9.28 ± 0.92, 15.51 ± 1.02, 20.59 ± 1.62) as measure gaits approximated actual distances in equal degree (p < 0.05), indifferent to the angle of return (F < 1), and despite gallop-walk using more steps (F(1,8) = 71.80, p < 0.0001) and taking more time (F(1,8) = 154.15, p < 0.0001) than gallop-run.

4. Discussion

In summary, we have found that the human odometer is not a pedometer or a stride integrator (although there are conditions in which it might appear to be either) and that its mode of measuring distance, however construed, is sensitive to the primary–secondary symmetry distinction between gaits. These two findings are situated within a third finding of perhaps greater significance. The conditions for satisfying Berkeley's hypothesis with respect to a distance d are not at the level of the act of legged traversal of d. That is, they are not at the level of locomotion as ‘measure’. Rather, they seem to be defined, minimally, at the level comprising locomotion as ‘measure’ and locomotion as ‘report’. The domain of Berkeley's hypothesis is a measure–report system (or instrument).

We can gain an appreciation of the measure–report system by further consideration of the theory that motivated experiment 5, the theory that ‘forgetting is temporary’ (McGeoch 1932; Neath & Surprenant 2003). Two notions that help ground this claim are transfer-appropriate processing (TAP) and encoding specificity (ES). If these notions were being discussed with respect to experiments in human memory, they would be discussed as follows. TAP emphasizes internal context: recall is maximized when the to-be-responded-to stimuli at study and test engage identical mental operations. ES emphasizes external context: recall is maximized when the same environmental conditions embed the stimuli and the encoding of the stimuli at both study and test. Together, the complementary principles of TAP and ES (Franks et al. 2000) yield the following claim: memory performance (the match of items reported at test to those presented at study) is maximal when the mental operations and environmental circumstances are invariant over the study-to-test transformation. The results summarized in figure 2 can be interpreted in similar terms: the match of reported distance to measured distance is maximal when the symmetry class of the gait is invariant over the measure-to-report transformation.

The question of what the human odometer registers if it does not register step variables has to be raised eventually in the context of the measure–report system. For immediate purposes we can note that variables defined over gait patterns that would serve odometry are far from obvious. Discovering them may require emerging methods to assay (i) the variants and invariants of locomotion's global phase dynamics in response to everyday environmental perturbations (bumps, slopes, brinks and so on; Revzen et al. 2009), and (ii) the relative phasing of whole-body eigenmodes of coordination revealed by principle components analysis (Lamoth et al. 2009). It may also require a willingness to think more abstractly about nature's instruments. The polar planimeter is often used to make such a case (Runeson 1977). This mechanical organization of a wheel and two rods, one fixed and one mobile, achieves an area measure of any irregular planar form by integrating the line integral of a vector field with constant curl. Are measures of similar abstraction, detectable by the dynamic touch subsystem of haptic perception, at work in animal odometry? The answer from the fiddler crab seems to be ‘yes’. The crab's stride or step is equated with one complete cycle of all eight legs. But neither the specific kinematic and kinetic details of the individual legs, nor the availability of the full complement of legs (as when two or more are involved in carrying mud), appear to be relevant to the measure of stride length. In fiddler crab locomotion there is, apparently, ‘a more abstract indication of step size’ (Walls & Layne 2009, p. 27).