Do release-site biases reflect response to the Earth's magnetic field during position determination by homing pigeons?

Abstract

How homing pigeons (Columba livia) return to their loft from distant, unfamiliar sites has long been a mystery. At many release sites, untreated birds consistently vanish from view in a direction different from the home direction, a phenomenon called the release-site bias. These deviations in flight direction have been implicated in the position determination (or map) step of navigation because they may reflect local distortions in information about location that the birds obtain from the geophysical environment at the release site. Here, we performed a post hoc analysis of the relationship between vanishing bearings and local variations in magnetic intensity using previously published datasets for pigeons homing to lofts in Germany. Vanishing bearings of both experienced and naïve birds were strongly associated with magnetic intensity variations at release sites, with 90 per cent of bearings lying within ±29° of the magnetic intensity slope or contour direction. Our results (i) demonstrate that pigeons respond in an orderly manner to the local structure of the magnetic field at release sites, (ii) provide a mechanism for the occurrence of release-site biases and (iii) suggest that pigeons may derive spatial information from the magnetic field at the release site that could be used to estimate their current position relative to their loft.

1. Introduction

The remarkable ability of homing pigeons to navigate directly home after being released at unfamiliar locations distant from their loft has long defied scientific explanation. Although many different types of environmental information have been suggested as the source of spatial information during pigeon homing (e.g. Gould 1982; Hagstrum 2000; Biro et al. 2004; Lipp et al. 2004; Gagliardo et al. 2006, 2008), it has been extremely difficult to determine which information the birds actually use to determine position. It is clear, however, that information available at release sites is sufficient on its own for the birds to be able to determine position because experienced pigeons transported to release sites under general anaesthesia showed no differences in initial orientation and homing performance from control birds (Walcott & Schmidt-Koenig 1973; Kiepenheuer 1978; Wiltschko & Wiltschko 1978, 1985). Both magnetic and olfactory cues are available at the release site, and experimental studies of position determination by pigeons have focused on these two potential sources of spatial information for some decades (e.g. Wiltschko & Wiltschko 1995; Wallraff 2005).

It has been suggested that the phenomenon of the release-site bias is related to the map step of the map-and-compass hypothesis for the navigation system of pigeons (Kramer 1953, 1961) and, more specifically, that such biases may arise from local irregularities in the pigeons’ navigational map (Keeton 1973, 1974). Release-site biases occur when experimentally untreated pigeons are released individually at a site and repeatedly vanish (typically at a distance of 2 km) from the observers’ view in a direction different from the homeward direction (Keeton 1973). Release sites may have a bias to the left or right associated with them, but at other sites, pigeons vanish close to or in the true home direction (HD).

The Earth's magnetic field has attracted interest as a source of information for position determination in pigeon navigation (Gould 1980; Moore 1980) because it is omnipresent, stable over evolutionary time and varies systematically over the Earth (Skiles 1985). The intensity of the Earth's magnetic field increases systematically from the equator to the poles (Skiles 1985) and could be used to determine latitude (Gould 1982). Considerable indirect evidence of both temporal and spatial variations in the Earth's magnetic field affecting the initial orientation of homing pigeons at the release site are consistent with this idea (reviewed in Wiltschko & Wiltschko 1995). Proposals for a second coordinate equivalent to longitude have been challenged on a variety of grounds (Lohmann & Lohmann 1996a,b; Courtillot et al. 1997; Åkesson & Alerstam 1998; Walker 1998, 1999; Wallraff 1999; Reilly 2002) and the mechanism by which homing pigeons determine position remains unresolved.

A recent model for magnetic navigation in pigeons (Walker 1998, 1999) suggested that the direction of the steepest slope of the total magnetic field intensity theoretically permits localization along the east–west axis (longitude). The model predicted that responses to magnetic anomalies should ‘dominate’ the behaviour of homing pigeons at release sites (Walker 1998). The first detailed evidence for the importance of magnetic intensity contours and slope during position determination came from pigeons carrying global positioning devices (GPDs; Dennis et al. 2007). The pigeons' tracks showed a strong association of the birds' flight paths with the local contour lines of total magnetic intensity as the pigeons repeatedly flew parallel and/or perpendicular to the direction of the steepest slope in the vicinity of the release site. This led to the suggestion that local distortions in the Earth's magnetic field at the release site caused by magnetic anomalies (Skiles 1985) could cause the birds to make errors in position determination that result in release-site biases (Walker 1998; Dennis et al. 2007; Mora & Walker 2008). Paradoxically, however, very few studies have successfully demonstrated any association between magnetic anomalies and vanishing bearings (the directions flown by pigeons as they leave release sites; Frei & Wagner 1976; Wagner 1976; Walcott 1978; Frei 1982; Wiltschko & Wiltschko 2003).

Here, we attempt to resolve these contradictions between theory and experimental results through a post hoc analysis of first- and second-order mean vanishing bearings in relation to (i) HD and (ii) the direction of the magnetic intensity slope at the time of vanishing for a large number of release sites around three lofts in Germany. To test the hypothesis that release-site biases are indeed errors in position determination caused by local distortions in the Earth's magnetic field, we first demonstrated the dependence of vanishing bearings at release sites on local variations in magnetic intensity. We then drew a connection between release-site biases, magnetic contour patterns and use by pigeons of the systematic variation in intensity of the Earth's magnetic field for position determination.

2. Material and methods

(a). Second-order mean vanishing bearing data

The second-order mean vanishing bearings analysed in this study were previously published as maps with each of the four datasets containing a large number of release sites distributed around one of the three lofts in Germany. Multiple releases of untreated homing pigeons were conducted at each site and second-order mean vanishing bearings calculated by averaging the mean vanishing direction of each group of control pigeons released from a given site. Release sites for two datasets (fig. 1 in Grüter et al. 1982; fig. 8 in Wiltschko 1992) were located around a loft in Frankfurt, Germany (50°07.6′ N and 8°39.6′ E) at distances of 8–200 km. The datasets included releases of experienced pigeons at 75 sites from 1976 to 1981 (Frankfurt I) and at 60 sites from 1976 to 1992 (Frankfurt II). An estimated 54 sites of the Frankfurt II study were the same sites used in the Frankfurt I study. However, owing to slight differences in the aspect ratios of the maps published by the original authors, it was not possible to determine with certainty which sites were identical in both publications and which sites were added, removed or replaced by new sites in close proximity to previously used sites between 1981 and 1992. In the cases where sites were clearly identical in both studies, it was also not known whether further release data were added between 1981 and 1992 when mean bearings were calculated. We therefore treated both studies as separate ‘snapshots’ in time of vanishing bearings for sites surrounding the same loft. In the two Frankfurt datasets, any site for which the mean vanishing bearing was classified by the original studies as not significantly oriented and/or no magnetic data were available (usually owing to the location of such sites near the border with the former East Germany) was excluded from our analysis (10 and 6 sites for Frankfurt I and Frankfurt II, respectively). Also excluded were sites that did not fulfil the minimum requirements of replication set by the original authors as having more than one release (16 sites for the Frankfurt I study) or 50 or more bearings from five or more releases (9 sites for the Frankfurt II study) for a given site. Thus, the sites included for the Frankfurt loft totalled 49 (Frankfurt I) and 45 (Frankfurt II). The third (fig. 1 in Ganzhorn & Schmidt-Koenig 1988) and fourth (fig. 3.4 in Wallraff 2005) datasets cover, respectively, releases of experienced pigeons from 29 sites surrounding a loft in Hohenentringen near Tübingen (48°33′ N, 8°59′ E) at distances of 6–116 km and of naïve birds, displaced away from the loft for the first time, from 35 sites surrounding a loft near Würzburg (49°48′ N, 9°56′ E) at distances of 14–138 km.

(b). Location of release sites

The location of each release site in relation to its loft (direction and distance) was determined from the original figures using graphical software tools (CorelDRAW v. 9.0). Next, a site's position was marked on an aeromagnetic map (scale of 1 : 500 000; ILH GeoCenter 1976). The map's magnetic measurements had been collected by an aeroplane flying at an altitude of 300–800 m, from which the IGRF (International Geomagnetic Reference Field) was then subtracted. The IGRF is a mathematical model of the magnetic field generated in the core of the Earth (the main field; Skiles 1985) and varies very slowly (up to several hundred nanoTeslas (nT)) over the distances from which pigeons typically home in experimental releases. The map thus showed the magnetic field intensity deviations (the residual field), which are due to iron-rich deposits in crustal rocks (Skiles 1985), from the main field plotted at contour spacings of 5 nT.

A 2 km vanishing circle, drawn to scale, was centred on the release site, representing the distance at which pigeons typically vanish from view, and their vanishing directions were recorded. The mean vanishing direction and HD for each release site, as plotted in the original figures, was then marked on the map as two lines from the release site's location to the vanishing circle.

(c). ΔH values

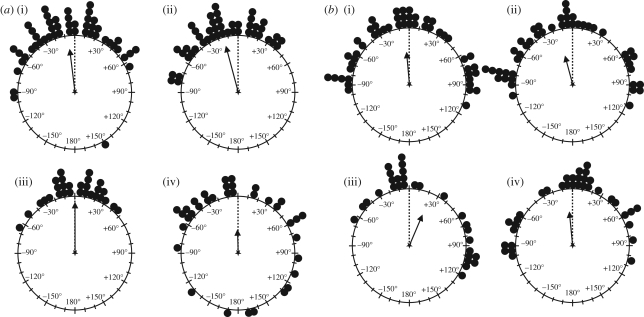
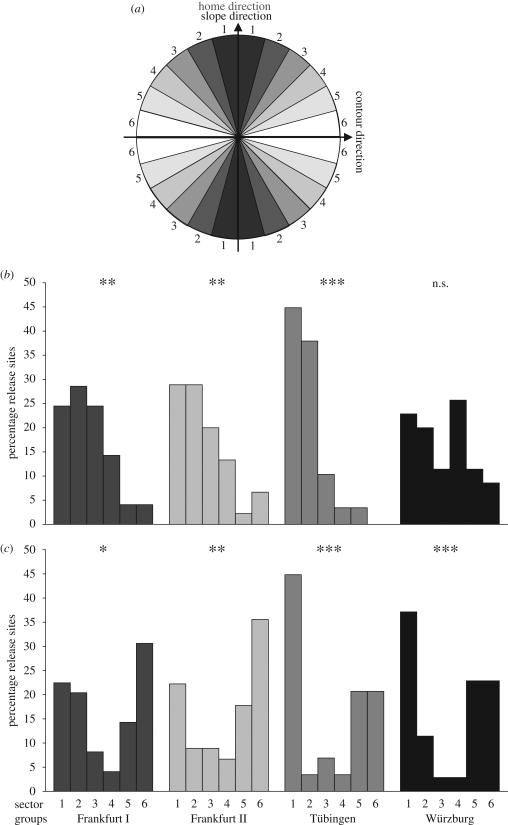
For each site, the difference between the second-order mean vanishing direction and the true HD (ΔH) was calculated, plotted as a circular distribution (figure 1a) and tested for non-randomness using the Rayleigh test (Batschelet 1981). The ΔH values were also divided according to their absolute size into six sector groups, each group encompassing a total of 60° on a circular diagram with the HD defined as 0° (figure 2a), and plotted as a histogram (figure 2b). A χ² goodness-of-fit test (Sokal & Rohlf 1981) tested the null hypothesis that there was no association between the distribution of vanishing bearings and the HD for each loft.

Figure 1.

Circular distribution of second-order mean vanishing bearings of experienced (Frankfurt I, Frankfurt II, Tübingen) and inexperienced (Würzburg) homing pigeons relative to (a) the HD (ΔH) and (b) the direction of the steepest magnetic intensity slope at the point of vanishing (ΔM). Vanishing data were derived from four previously published figures for three lofts in Germany. (i) 49 release sites, Frankfurt loft I (fig. 1 in Grüter et al. 1982). (ii) 45 release sites, Frankfurt loft II (fig. 8 in Wiltschko 1992). (iii) 29 release sites, Tübingen loft (fig. 1 in Ganzhorn & Schmidt-Koenig 1988). (iv) 35 release sites, Würzburg loft (fig. 3.4 in Wallraff 2005). The direction of (a) home and (b) the steepest magnetic intensity slope is indicated by a dashed line. The overall mean vanishing vector for each dataset is indicated by a black arrow. Each distribution was tested for non-randomness with the Rayleigh test.

Figure 2.

Sector analyses of ΔH and ΔM values. (a) Division of a circular diagram into six sector groups with each group encompassing 60°. For analysis of ΔH (mean vanishing direction in relation to HD), HD was standardized as 0° for each release site. For analysis of ΔM (mean vanishing direction in relation to the direction of the steepest magnetic field intensity slope at the point of vanishing), slope and contour directions were standardized as y- and x-axes, respectively. Percentage of (b) ΔH and (c) ΔM values that fell in each of the six sector groups defined in figure 2a for the four datasets. Significance levels for χ² goodness-of-fit test testing the null hypothesis of equal distribution of ΔH and ΔM values across all six sectors: *p < 0.05, **p < 0.01, ***p < 0.001, n.s., not significant.

(d). ΔM values

At the point of intersection of the second-order mean vanishing direction and the 2 km vanishing circle, the direction of the nearest 5-nT-intensity contour line was measured and the direction of the steepest magnetic intensity slope (contour line direction plus 90°) was calculated. For each site, the difference between the mean vanishing direction and the direction of the steepest magnetic intensity slope (ΔM) was calculated, plotted as a circular distribution (figure 1b) and tested for non-randomness using the Rayleigh test (Batschelet 1981). As for ΔH, ΔM values were divided according to their absolute size among the six sector groups (figure 2a) and plotted as a histogram (figure 2c). However, for this sector analysis, the y-axis in the circle corresponded to the direction of the steepest magnetic intensity slope and, accordingly, the x-axis to the direction of the magnetic intensity contour lines at the release site at the time of vanishing. Thus, Groups 1 and 2 represented deviations of the vanishing direction from the slope direction (SD) by ±0–14° and ±15–29°, respectively, and Groups 5 and 6 a deviation from the contour lines by ±0–14° and ±15–29°, respectively, while Groups 3 and 4 were intermediate sectors. A χ² goodness-of-fit test (Sokal & Rohlf 1981) tested the null hypothesis that the ΔM values were uniformly distributed among the six sector groups for each loft.

(e). Mean vanishing directions of individual release from nine sites

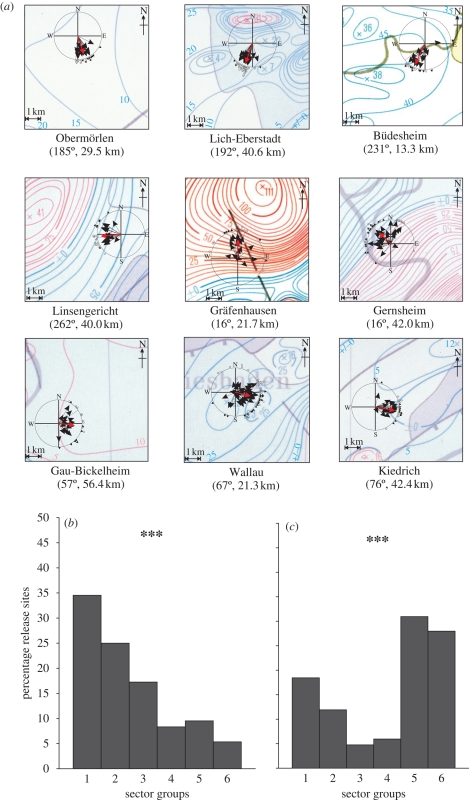
For nine sites of the Frankfurt II dataset, mean vanishing vectors of individual releases conducted at these sites from 1976 to 1992 (fig. 7 in Wiltschko 1992), as well as the distance and direction of each site from the loft (table 4 in Wiltschko 1992), were available. Each site's position was marked on the aeromagnetic map as described above and the circular diagrams shown in fig. 7 in Wiltschko (1992) were transposed onto the map with the centre of the circle at the release site's location (top of circle = geographical North; figure 3a). The size of the circle was scaled to represent the typical 2 km vanishing distance. For each site, the direction of each individual vanishing vector was measured. Values of ΔH and ΔM for all nine sites combined were calculated as described above, divided into the six sector groups (figure 2a), plotted as histograms (figure 3b,c) and their distribution among the groups tested for non-randomness with a χ² goodness-of-fit test (Sokal & Rohlf 1981). Any non-significant bearings were excluded from this analysis.

Figure 3.

(a) Circular distribution of mean vanishing bearings of individual releases for nine release sites (fig. 7 in Wiltschko 1992) transposed onto an aeromagnetic map showing magnetic field intensity contour lines at 5 nT intervals (pink and blue lines indicating deviations above and below the Earth's background field, respectively; all other lines not relevant to the current analysis). HD and distance from loft are listed below the panel for each site. Circles scaled to represent typical 2 km vanishing distance. Mean vanishing vectors for individual releases from each site: black arrows inside circle. Vanishing directions: symbols on circle's circumference (solid triangle, mean vanishing bearing showing a statistically significant bias; open triangle, mean vanishing bearing homeward orientated; open circle, mean vanishing bearing not significant according to Rayleigh test). HD, dashed line; median second-order vanishing vector (table 4 in Wiltschko 1992), bold red arrow. Sector analyses of (b) ΔH and (c) ΔM values combined for all individual releases from nine sites (fig. 7 in Wiltschko 1992). Only significantly oriented mean vanishing vectors (Rayleigh test) were included in this analysis. Percentage of (b) ΔH and (c) ΔM values that fell in each of the six sector groups defined in figure 2a. Significance levels for χ² goodness-of-fit test testing the null hypothesis of equal distribution of ΔH and ΔM values across all six sectors: *p < 0.05, **p < 0.01, ***p < 0.001, n.s., not significant.

3. Results

(a). Second-order mean vanishing bearing data

The ΔH values were significantly concentrated around the HD in all four circular distributions (Rayleigh test, p < 0.01–0.001; figure 1a), with virtually all values being located within the homeward semicircle for experienced birds and only eight (23%) ΔH values for the naïve birds being located in the semicircle away from home. The ΔH values in all the circular distributions, however, were not as narrowly focused around the homeward direction (ΔH = 0) as expected (figure 1a). When the ΔH values were plotted by 15° sectors (figure 2a), just over a third of the bearings for experienced birds were within ±14° of the true HD, around two-thirds were between ±15° and ±59°, and slightly over 10 per cent were ±60° or more away from the true HD. Consistent with the circular distributions, ΔH was non-uniformly distributed across the six sectors for the experienced birds (χ² goodness-of-fit test, p < 0.01 to p < 0.001; figure 2b), whereas almost 60 per cent of ΔH values for the naïve birds were greater than ±29°, resulting in a distribution relative to home that was not different from random (χ² goodness-of-fit test, p > 0.05; figure 2b). For the Würzburg dataset, eight ΔH values were greater than ±90° and were relatively uniformly distributed across the semicircle facing away from the HD. Their positions therefore did not affect the outcome of the sector analysis in which equivalent sectors in the homeward and non-homeward semicircles are combined into the six sector groups (figure 2a).

The circular distributions for ΔM values were non-uniform for all four datasets showing a trimodality with concentrations of mean bearings around the directions of the magnetic intensity slope (0°) and the contours of equal total magnetic intensity (±90°; Rayleigh test, p < 0.001; figure 1b). That is, pigeons predominantly vanished from a release site in directions aligned either parallel or perpendicular to SD. The only exception was the Tübingen dataset, where only two of the three modes seen in the other three datasets were observed. For both experienced and naïve pigeons, the sector analysis (figure 2a) revealed a strong association between ΔM and either the slope of magnetic intensity or the intensity contours at the release sites. The great majority (84–94%, mean ± s.e. 89 ± 1.8%) of bearings fell within ±29° of either the slope or contour direction (χ² goodness-of-fit test, p < 0.05 to p < 0.01; figure 2c). Each dataset contained a wide range of slope (and conversely contour) directions and a site's SD was found to be independent of its HD (sector analysis of HD–SD, χ² goodness-of-fit test, p > 0.05).

(b). Mean vanishing directions of individual releases from nine sites

The nine release sites of the Frankfurt II dataset were not distributed evenly around the loft, being concentrated to the northeast and southwest. The local patterns of magnetic intensity contour lines for these sites showed considerable variation, however, with each site's pattern being unique in its combination of steepness of the local intensity slope(s), curvature of the contour lines and overall complexity of the pattern (figure 3a). Nevertheless, combined analysis of ΔH and ΔM values for mean vanishing bearings from individual releases at these sites produced results very similar to the analysis of second-order means.

Values of ΔH were non-uniformly distributed across the six sectors, with only a third of the bearings for experienced birds falling within ±14° of the true HD, around 50 per cent between ±15° and ±59°, and 15 per cent ±60° or more away from the true HD (χ² goodness-of-fit test, p < 0.001; figure 3b). The distribution of the ΔM values was also non-uniform across the six sectors (χ² goodness-of-fit test, p < 0.001; figure 3c). There was a strong association between ΔM and either the slope of magnetic intensity or the intensity contours for the individual releases, with 89 per cent of the ΔM values for a combined sector analysis of bearings and up to 96 per cent (mean±s.e. 89±2.8%) of first-order means for individual sites falling within ±29° of either the slope or contour direction. That is, even at the level of first-order means, pigeons predominantly vanished from a release site in directions aligned either parallel or perpendicular to SD.

4. Discussion

We suggest the present study provides a substantial body of evidence that: (i) identifies the mechanism underlying the occurrence, and so the ability to predict release-site biases; (ii) elucidates the relationship between the release-site bias and the HD; and (iii) demonstrates that the above results are consistent with predictions made by the vector subtraction model of magnetic position determination (Walker 1998, 1999).

(a). Origin of release-site biases

Our results permit explanation of the puzzling and unpredictable occurrence of release-site biases in terms of the structure of the Earth's magnetic field at release sites. Our analysis shows the release-site bias reflects responses by the birds to the Earth's magnetic field that can be detected for releases at individual sites, in data pooled over large numbers of sites around a loft and across multiple datasets and lofts. Because pigeons vanished from release sites aligned either with the magnetic slope or contour line direction, left or right biases consequently occurred at sites where slope or contour directions lay to the left or right of home, respectively. Conversely, biases were absent at sites where either the slope or contour direction coincided with the HD. We therefore suggest that the release-site bias is not really a ‘bias’ after all because at sites without a bias (where the 95% confidence interval of the mean vanishing direction includes the HD), pigeons are simply following local slope or contour lines that by chance coincide with the homeward course. It follows that the vanishing bearings of homing pigeons can, in theory, be predicted from the magnetic intensity contours and slope at release sites.

The combination of (i) greater concentration of ΔH values in the homeward semicircle observed for experienced than for naïve birds and (ii) association of vanishing bearings of naïve birds with magnetic field patterns implies that the response by the birds to magnetic intensity slope and contours is innate. Furthermore, the fact that almost a quarter of the second-order means for naïve birds fell in the semicircle away from home implies that the birds respond first to the magnetic intensity slope and contours at release sites and do so independent of HD. Prior homing will then permit experienced birds to identify the HD more rapidly after release than naïve birds and result in higher proportions of vanishing bearings in the homeward semicircle.

An analysis such as this must also consider the possibility of confounding with other variables at the release site. Thus, magnetic anomalies may be associated with topographic features such as volcanoes, while the behaviour of pigeons at release sites can be influenced by both natural and man-made geographical features such as forest edges, bodies of water and villages. The lofts where the data used in this analysis were collected are all located in areas of low topographic relief, with two lofts being in river valleys (Tübingen, Würzburg) and the third (Frankfurt) in a tectonic graben (M. Winklhofer 2009, personal communication). Furthermore, release sites for studies using vanishing bearings are generally very carefully chosen to avoid any visual obstructions (e.g. hills, forests, buildings) that could result in the observers prematurely losing the pigeons from view. The pigeons, typically flying at a height of fifty to several hundred metres, were therefore unlikely to have needed to fly over or around geographical features as they departed from the release sites around the three lofts analysed here. Other geographical features (e.g. forest edges, nearby villages), to which pigeons may respond, have no known association with magnetic intensity contour patterns and thus vary independently of both the HD and the direction of magnetic intensity slope at the release site. They should therefore have had no net impact on vanishing bearings over the large number of release sites contained in each dataset analysed here.

(b). Implications for the vector subtraction model of magnetic position determination

The domination of the behaviour of pigeons at release sites by localized variations in the Earth's magnetic field is predicted by the vector subtraction model of magnetic position determination (Walker 1998, 1999) and has been shown by our analysis here to result from responses by the birds to magnetic intensity and intensity slope. Similar behaviour was observed in pigeons released near weak anomalies in the Swiss Alps. The vanishing bearings and flight tracks of pigeons observed by helicopter were strongly correlated with the directions of the steepest magnetic intensity slope at the anomalies, but independent of surface topography (Frei & Wagner 1976; Wagner 1976; Frei 1982).

It remains to be established, however, whether release-site biases occur because pigeons are attempting to determine position using the systematic variation in the Earth's magnetic field. At release sites, pigeons only have access to total magnetic intensity, which is the sum of the systematically varying field generated in the core of the Earth (typically well over 90% of the observed field) and residual fields generated by iron-rich deposits within rocks in the Earth's crust (magnetic anomalies; Skiles 1985). These magnetic anomalies cause variations in magnetic intensity and intensity SD over short distances. If pigeons are to use systematic regional variations of these two field parameters to determine position, as described in the vector subtraction model (Walker 1998), then any stimulus noise owing to local variations must be filtered out for the pigeon to determine its position relative to the loft.

Repeated sampling of variations in space of the intensity and slope of the Earth's magnetic field will theoretically permit the birds to reduce the variance in their estimate of position at release sites. Recent GPD-tracking experiments showed multiple segments of flight aligned parallel with and perpendicular to contours and slope of total magnetic intensity over distances up to 4 km from the release site (Dennis et al. 2007). Such behaviour is consistent with the hypothesis that pigeons sample these variables independently and repeatedly over the area around release sites. The vector subtraction model (Walker 1998) predicts that repeated sampling will permit the birds to: (i) estimate the intensity and SD of the systematically varying field produced in the Earth's core over the areas around release sites; (ii) determine their approximate position relative to the loft; and (iii) set a course for home. It follows that high variance in either the intensity or the SD (owing, for example, to curvature and/or steepness of intensity contours and slope, respectively, such as those seen in figure 3a) will require that the birds sample over larger areas and longer times before they can determine their position and set a course for home. Such conditions at release sites can be expected to result in increased scatter of vanishing bearings and randomly distributed vanishing bearings in extreme cases (Walcott 1978).

5. Conclusion

We conclude that pigeons respond to the Earth's magnetic field at the release site when deciding on a flight direction and that vanishing bearings may directly reflect the interaction between response to local and large-scale variations in magnetic intensity as the birds seek to estimate their current location relative to the loft. We suggest that the analysis of differences in accuracy of position determination for displacements along the intensity contour (the latitude) and the SD (the longitude) through the loft of the systematic component of the Earth's magnetic field is a further prediction of this model that is relatively easily evaluated (Walker 1998). We invite experimental studies that test this and further predictions of this model, and look forward to their results.