Abstract
Social discounting in economics involves applying a diminishing weight to community-wide benefits or costs into the future. It impacts on public policy decisions involving future positive or negative effects, but there is no consensus on the correct basis for determining the social discount rate. This study presents an evolutionary biological framework for social discounting. How an organism should value future benefits to its local community is governed by the extent to which members of the community in the future are likely to be its kin. Trade-offs between immediate and delayed benefits to an individual or to its community are analysed for a modelled patch-structured iteroparous population with limited dispersal. It is shown that the social discount rate is generally lower than the individual (private) discount rate. The difference in the two rates is most pronounced, in ratio terms, when the dispersal level is low and the hazard rate for patch destruction is much smaller than the individual mortality rate. When decisions involve enforced collective action rather than individuals acting independently, social investment increases but the social discount rate remains the same.
Keywords: discounting, discount rate, social discounting, altruism, kin selection, dispersal
1. Introduction
Individual and collective decisions taken today may result in future community-wide costs or benefits, and in extreme cases may affect community survival (Diamond 2005). How should such future effects be valued against costs or benefits today? A social investment today may yield a stream of future benefits. Choosing between such an investment and an alternative action that yields only immediate benefits is a difficult problem. It is normal in such public policy dilemmas to discount future social benefits (Goulder & Stavins 2002), with the effect that a potentially indefinite social benefit stream is given a finite present value. The choice of the social discount rate has a bearing on public policy decisions involving costs and benefits at different times (Dasgupta & Heal 1974; Gupta et al. 1996; Atherton & French 1998; Pearce et al. 2003). A lower social discount rate tends to favour more environmental protection and restoration (Gupta et al. 1996). Conversely, more rapid discounting implies more rapid consumption of both non-renewable and renewable resources and can lead to extinction of the latter (Clark 1973; Dasgupta & Heal 1974).
What is the rationale for allocating progressively lower weights to benefits from social investments further into the future? One possible reason is the risk of a future event that will bring to an end the social benefit stream arising from the investment. This could take the form of a catastrophe that wipes out the community in which the investment is located (Dasgupta & Heal 1979), or simply a development that renders a social investment redundant or causes it to stop working (Rambaud & Torrecillas 2005). In addition to such risk, however, there is another consideration: how should a risk-free welfare gain or loss by members of the community at some point in the future be valued against a risk-free welfare gain or loss by members of the community today? Some authors regard it as axiomatic, for moral reasons, that the welfare of all people, present and future, should be accorded the same weight in public policy decisions (Broome 1994). However, decisions can be expected to take account of the welfare of future people only to the extent that decision makers care about future people (Marglin 1963). A simple population analysis indicates that, as long as present-day decision makers give some positive weight to the welfare of future members of the community, the social discount rate will be lower than the private discount rate for individual benefits (Sumaila & Walters 2005). But this leaves open the question of exactly what weighting should be used.
The present study considers social discounting as a general problem in evolutionary biology, applicable to organisms that live in viscous populations with limited dispersal. It is concerned with the following question: what social discount rate would result from natural selection? This raises a more basic question: why should an organism, governed by its selfish genes, care about the future welfare of its community? A possible answer comes from the biological theory of kin selection: if welfare gains translate to improved survival or reproductive success, then, other things being equal, selection will tend to favour behaviours that result in welfare gains to relatives. If, additionally, geographical proximity is associated with a positive level of relatedness (Hamilton 1975; Manica et al. 2005), and there is some degree of long-term persistence to this association, evolution may select for an individual to act in a way that benefits members of its community or locality in future as there will be a statistical tendency for the recipients of such benefits to be her/his kin. This can be understood by reference to the principle that altruism can evolve where there is some form of assortment within the population so that altruists are more likely to be recipients of altruistic benefits than are non-altruists (Fletcher & Doebeli 2009). Lehmann (2007) has shown that, in a patch-structured semelparous population with discrete generations, this effect can lead to selection for altruistic acts whose effects last for several generations. The present study takes this approach further by deriving explicit time preferences for both individual and social benefits, for an iteroparous patch-structured population facing trade-offs in continuous time. It establishes that natural selection will favour two distinct discount rates, with the individual discount rate generally being higher than the social discount rate.
This analysis of social discounting arising from the inclusive fitness value to an organism of delayed benefits to its community contrasts with the idea that individual discounting is mediated by the capacity to make transfers to specific relatives (Barro 1974; Rogers 1994). General transfers within a kin group have been modelled for situations in which any conflict of interest between donor and recipient is ignored (Hansson & Stuart 1990; Kaplan & Robson 2002; Lee 2003; Robson & Kalpan 2003) which is equivalent to a donor treating recipients as full kin with a relatedness of one (Rogers 2003), and for situations in which the level and pattern of transfers is exogenously determined (Lee 2008). The present study, however, is concerned with trade-offs involving immediate or delayed benefits to self or local community; the decay over time of expected relatedness to an organism of members of its local community is critical to the analysis.
2. Population model
Consider an infinite population occupying discrete patches, each patch containing a fixed number N of individuals. The population is asexual (a sexual population is considered in the electronic supplementary material). It is governed by a Moran-type dynamic process with individuals and entire patches subject to random replacement events, whereby an individual or patch is duplicated and replaces (i.e. kills) another individual or patch, as follows:
Individual reproduction and mortality event: an individual is duplicated; with probability v, the duplicate disperses to a different patch, chosen at random, and kills and replaces a random member of that patch, and with probability (1−v), the duplicate kills and replaces a member of its own patch, chosen at random from the N members including its parent.
Patch colonization and destruction event: an entire patch is duplicated and replaces one of the other patches, chosen at random.
At equilibrium, each member of the population has an individual reproductive rate (probability per unit time for individual reproduction) of k. The individual mortality rate from the risk of being replaced in such an event is also k. Each patch has a colonization rate (probability per unit time for being duplicated and taking over another patch) of ω. The rate for a patch to be wiped out in a destruction event, i.e. colonized by another patch, is also ω.
(a). Survival functions
An organism's probability si(τ) of surviving a delay τ without being killed through either individual mortality or destruction of its patch is
| 2.1 |
The probability sp(τ) of a patch surviving a delay τ without suffering a patch-level destruction event is
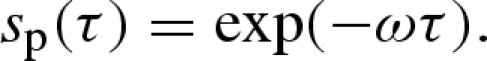 |
2.2 |
(b). Relatedness
For this asexual population, the relatedness of two individuals is the probability that they are identical. R is the relatedness at equilibrium of an individual to all members of its patch including itself. ROTH is the relatedness of an individual to an individual in its patch other than itself. The two measures are related by
or
| 2.3 |
Consider a newborn in an equilibrium population. Its relatedness to a randomly chosen comparison member of its patch is ROTH. But this relatedness can also be calculated as follows: with probability v, the newborn's parent is from another patch and its relatedness to the comparison member is zero. With probability (1−v), the newborn's parent is from the same patch; if so, then with probability 1/N the comparison member is the parent and the newborn's relatedness to the comparison member is 1, and with probability (1−1/N), the comparison member is not the parent and the newborn's relatedness to the comparison member is ROTH. This yields the recurrence relationship
| 2.4 |
Substituting into equation (2.4) from equation (2.3) and simplifying gives
| 2.5 |
3. Preferences
I assume that each organism sporadically faces choices over options involving small immediate or delayed benefits to itself or to its patch. The analysis below determines the preferences that are favoured by natural selection, i.e. the preferences that maximize inclusive fitness. As the total population does not grow, this should be understood as maximizing relative inclusive fitness.
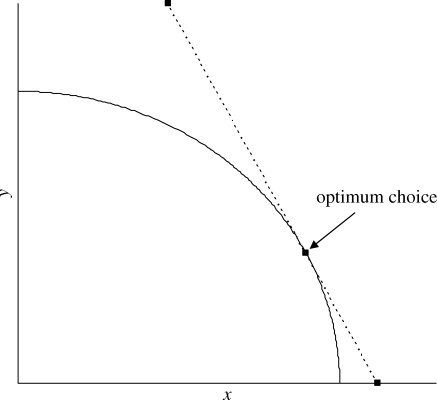
Consider preferences from the point of view of a single organism, denoted as the focal organism. I assume that the focal organism's inclusive fitness gain from a small benefit is proportional to the benefit level, and that multiple benefits have an additive effect on inclusive fitness. The problem faced by the focal organism can be conceptualized as a (multidimensional) trade-off over the various possible benefits. Consider two such benefits, keeping other benefits constant (figure 1). The levels of the two benefits are denoted by x and y. At a given value of x the focal organism would prefer a larger value of y, and at a given value of y it would prefer a larger value of x. Its choices, however, are constrained to lie along the trade-off curve, so that it cannot get more of one benefit without less of the other.
Figure 1.
A hypothetical trade-off between two benefits. For a given level of any one of the two benefits, the focal organism would prefer more of the other. However, its choices are constrained to lie along the trade-off curve so that it cannot gain more of one without getting less of the other. If a unit increase in x has the same effect on fitness as an increase of two units in y, then fitness is maximized by maximizing (x + 2y). At the optimum choice, the tangent to the trade-off curve has a slope of −2.
Preferences, defined by rates of substitution between alternative benefits, are determined by the ratio of marginal increments to inclusive fitness for the focal organism from each benefit. Figure 1 illustrates a situation in which a unit increase in x has the same effect on inclusive fitness as an increase of two units in y. Inclusive fitness is maximized by maximizing the value of (x + 2y). At the optimum choice, the tangent to the trade-off curve has a slope of −2.
(a). Discounting
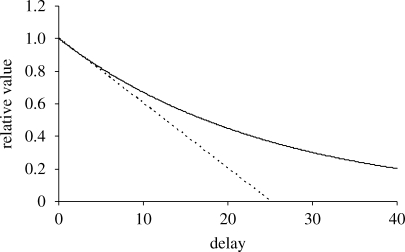
Discounting depends on how the gain to inclusive fitness from some benefit due after a delay declines as a function of delay (Sozou 1998), with the instantaneous time-preference rate (Rogers 1994; Sozou & Seymour 2003) θ given by the proportional rate of decline of inclusive fitness value with delay (figure 2). For what follows, θIND is the individual discount rate, i.e. the time-preference rate for a benefit to the focal organism itself. Immediate and delayed benefits to a patch can arise from patch members acting either independently or through enforced joint action arising from the focal organism's independent action. The social discount rate θSOC is the time-preference rate for benefits to the focal organism's patch. The group social discount rate θSOC,GROUP is the time-preference rate for a benefit to the focal organism's patch arising from a collective action that is enforced among all members of the patch.
Figure 2.
The curve shows relative value against delay for a given benefit. An immediate benefit is taken to have a relative value of 1. The instantaneous time-preference rate θ is determined by the steepness of the curve at a delay of zero. The dotted line is a tangent to the curve at a delay of zero and has a gradient of −θ.
4. Analysis
To derive inclusive fitness-maximizing preferences, including individual and social discount rates, it is necessary to calculate the inclusive fitness consequences of different possible benefits, either to the focal organism or to its patch, for immediate or delayed benefits. The effect of a particular benefit on the inclusive fitness of the focal organism is given by the expected increase in the number of copies of the focal organism in the population arising from the benefit, as described in Taylor (1992). A given benefit is described by three parameters: the type of benefit, its magnitude, and the delay before the benefit is due. The expected inclusive fitness gain for the focal organism is written as w(type of benefit, size of benefit, delay). Three types of benefit are considered: a reproductive benefit to the focal organism itself, denoted by repSELF; a reproductive benefit to a random member of the focal organism's patch, denoted by repPATCH; and a colonization benefit to the focal organism's patch, denoted by colPATCH.
(a). Immediate reproductive benefit to self
Consider a small amount a of immediate reproduction realized immediately by the focal organism. This can be constructed as a temporary increase of magnitude Δk and duration dt in its reproduction rate, with Δk dt = a. It results in a probability a that the organism will produce an additional offspring; if this additional offspring is produced, then with probability v this offspring will disperse to another patch, and with probability (1−v) it will stay on the home patch, displacing another individual of expected relatedness R. The expected inclusive fitness gain is
| 4.1 |
(b). Immediate reproductive benefit to patch (random beneficiary)
Now consider a small amount b of additional reproduction, realized immediately by a member of the focal organism's patch chosen randomly from all members including itself. Thus, a recipient of expected relatedness R to the focal organism has a probability b of producing an extra offspring. With probability v, this offspring disperses to another patch, giving an inclusive fitness gain R to the focal organism; with probability (1−v), this offspring remains on the patch, displacing another individual of relatedness R to the focal organism and therefore giving an inclusive fitness gain of zero. The expected marginal effect on inclusive fitness is
| 4.2 |
(c). Preference with respect to immediate benefits
The focal organism's inclusive fitness benefit from a gain b to reproduction in its patch will be greater than from a gain a to its own reproduction if w(repPATCH, b, 0) > w(repSELF, a, 0). Substituting from equations (2.5), (4.1) and (4.2), this simplifies to
| 4.3 |
Note that b/N is the focal organism's expected increase in reproduction arising from a 1/N share of a benefit b to its patch. Thus, the net effect on the focal's inclusive fitness is fully captured by the change to its own net reproductive rate; the net inclusive fitness effect of changes in the reproductive output of other members of its patch is zero. It follows that, in this model, the focal organism should not be willing to sacrifice some of its own reproduction to either increase or reduce the reproductive output rate of other members of its patch, as has been shown for a semelparous infinite patch-structured population (Taylor 1992) and for semelparous (Rousset 2004) and iteroparous (Taylor et al. 2007) populations on finite networks (and it has been shown (Lehmann et al. 2007) that a number of evolutionary graph theory models can be understood through classic inclusive fitness theory). This is a consequence of local competition counteracting local kinship (Grafen 1984; Wilson et al. 1992).
(d). Immediate colonization benefit to patch
Consider a small amount c of additional colonization realized immediately by the focal organism's patch. This gives a probability c that the patch will enjoy an additional colonization event resulting in a fitness gain NR to the focal organism. Hence the expected fitness gain is
| 4.4 |
(e). Delayed reproductive benefit to self
Consider a small amount a of additional reproduction by the focal organism, to be realized after a delay τ conditional on the focal organism still being alive after the delay (Sozou & Seymour 2003). As long as the focal organism remains alive, its relatedness to other members of the patch remains R. From the reference frame of the present, the reward results in a probability asi(τ) that the focal organism will produce an additional offspring, which will disperse with probability v, and with probability (1−v) will stay on the patch and displace another individual of expected relatedness R. The expected fitness gain is
| 4.5 |
(f). The individual discount rate
Equations (4.1) and (4.5) give
| 4.6 |
The individual discount rate θIND is given by
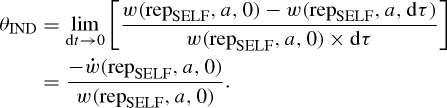 |
Substituting from equations (2.1) and (4.6) yields
| 4.7 |
Hence, the individual discount rate is given by the sum of the patch destruction rate and the individual mortality and so equals the total death rate experienced by the organism.
(g). Decay of relatedness into the future
As long as the focal organism remains alive, its mean relatedness to members of its patch remains at the value R given in equation (2.5). A new member in its patch could be: its own offspring, which will increase its mean relatedness to members of its patch; or the offspring of another member of its patch, which will leave expected relatedness unchanged; or an arrival from another patch, which will decrease its mean relatedness to members of its patch. The tendency for the first process to increase relatedness is balanced by the tendency for the third to decrease it. But if the focal organism dies, the first process ends and so relatedness to it of members of the patch will tend to decrease over time. It follows that if relatedness is calculated from a perspective that is not conditional on the focal organism remaining alive, it will decline over time.
Let RE(τ) be the expected relatedness of other members of the patch to the focal organism as a function of delay, conditional on the patch not having suffered catastrophic destruction during the delay but not conditional on the focal organism remaining alive. The individual mortality rate is k. A mortality event causes a patch member of expected relatedness RE to the focal organism to be replaced by an immigrant of relatedness 0 with probability v, and by an existing patch member of expected relatedness RE with probability (1−v). Hence
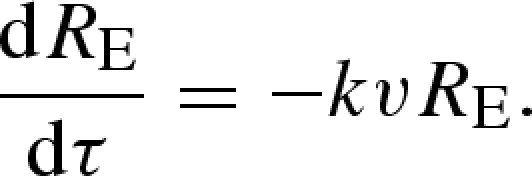 |
And RE(0) = R. This gives
| 4.8 |
Now consider a measure of relatedness of the focal organism to a random member of its patch in the future that allows for both the possibility that the focal organism will have died during the delay and the possibility that the patch will have suffered a colonization event during the delay. This is the unconditional relatedness denoted by RU(τ). With probability sp(τ), the patch will not suffer destruction during the delay and the relatedness at delay τ will be RE(τ). With probability (1−sp(τ)), the patch will suffer destruction and the focal organism's relatedness to a random member at delay τ will be zero. RU(τ) is therefore given by
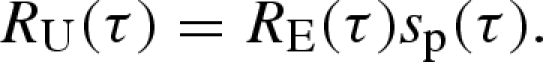 |
4.9 |
Substituting from equations (2.2) and (4.8) gives
| 4.10 |
(h). Delayed reproductive benefit to patch (random beneficiary)
Consider an amount b of additional reproduction, to be realized by a member of the focal organism's patch chosen randomly after a delay τ. It will be nominally assumed that realization of the benefit is conditional on the patch not having suffered a destruction event during the delay, but this assumption is not necessary for the analysis. This delayed benefit gives a probability bsp(τ) that a patch member of expected relatedness RE(τ) to the focal organism produces an additional offspring; with probability v this offspring disperses, giving a fitness gain of RE(τ) to the focal organism, and with probability (1−v) this offspring remains on the patch giving a fitness gain of zero. The expected fitness gain is
From equation (4.9), this may be expressed as
| 4.11 |
(i). Delayed colonization benefit to patch
Consider a small amount c of additional colonization by the focal organism's patch, realized after a delay τ, conditional on the patch not having suffered a destruction event during the delay. This gives a probability csp(τ) that the patch will enjoy an additional colonization event resulting in a fitness gain NRE(τ) to the focal organism. The expected fitness gain is
| 4.12 |
(j). The social discount rate
The social discount rate θSOC is the proportional rate of decline with delay of the fitness value to the focal organism of a benefit to its patch. There are two possible forms of benefit to the patch, delayed reproduction and delayed colonization. From equations (4.10), (4.11) and (4.12), they yield the same social discount rate:
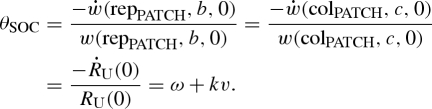 |
4.13 |
The social discount rate is thus given by the patch destruction rate ω plus the product of the individual mortality rate k and the dispersal level v. It is equal to the rate constant in RU(τ), governing the rate of decline of unconditional expected relatedness of the focal organism to a random member of the patch. If v = 1, i.e. all newborns disperse to a new patch, then θSOC = θIND (see equation (4.7)). If v = 0, i.e. there is no dispersal, then θSOC = ω. For the general case of 0 < v < 1, ω < θSOC < θIND. If v ≪ 1 and ω ≪ k, i.e. the dispersal level is low and the patch destruction rate is much lower than the individual mortality rate, then θSOC ≪ θIND.
(k). The effect of enforced collective social action
The analysis so far has considered an organism's choices when these are independent of the actions of other members of its patch. However, social behaviour may instead be enforced (Frank 1995); in a modern human society, for example, this may take the form of prohibition of anti-social activities such as improperly dumping waste.
Let wGROUP be the fitness gain to the focal organism from an action that is adopted by all members of the patch and enforced. I assume that the focal organism has, probabilistically at least, some influence over the choice of action, so that natural selection acts on its preferences for this action. Consider first a collective action resulting in each member of the patch enjoying an immediate individual reproductive benefit a. The fitness gain for the focal organism arises from the proportion v of this increased reproduction, from N patch members of relatedness R to the focal organism, which disperses:
| 4.14 |
Consider now a collective action such that each member of the patch generates immediate additional reproduction b by a random member of the patch. Again, the net fitness gain for the focal organism arises from the proportion of increased reproduction that disperses and is given by
| 4.15 |
Comparing equations (4.14) and (4.15), the focal organism's fitness gain from a collective and enforced decision is greater if each patch member's actions contribute b to a random member than if each member's actions contribute a to its own reproduction if b > a. This is more easily satisfied than equation (4.3), i.e. behaviour will tend to shift in the direction of acts that benefit the patch rather than the individual.
Now consider a collective and enforced decision such that each patch member's action contributes a reproductive benefit b to a random member of the patch after a delay τ, realization of this being conditional on the patch not suffering destruction during the delay. This gives a probability sp(τ) of a total reproductive benefit Nb to recipients of expected relatedness RE(τ) to the focal organism. The expected fitness gain for the focal organism arises from the proportion v of this additional reproduction that disperses outside the patch. This is equal to Nb RE(τ)sp(τ). From equation (4.9), this may be written as
| 4.16 |
Now consider a joint decision in which the action of each member of the patch contributes a colonization benefit c to the patch after a delay τ, realization of the benefit being conditional on the patch not suffering destruction during the delay. This gives a probability Ncsp(τ) of an additional colonization event; if such an event occurs, it has fitness value NRE(τ) to the focal organism. The focal organism's expected fitness gain from the delayed additional colonization benefit enforced among all members of the patch is therefore cN2RE(τ)sp(τ). From equation (4.9), this may be expressed as
| 4.17 |
The social discount rate for a patch benefit arising from an enforced common decision, affecting delayed reproduction or delayed colonization, is from equations (4.10), (4.16) and (4.17) given by
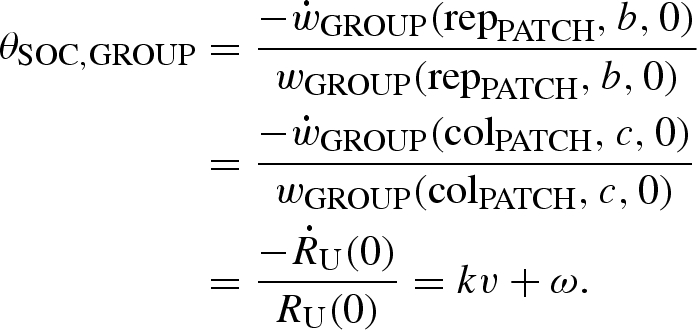 |
4.18 |
Comparing equation (4.18) to equation (4.13), the social discount rate under joint decision making with enforced social investment is the same as under voluntary action.
5. Discussion
Lehmann (2007) showed that it may be adaptive for an organism to act in a way that produces future benefits to its community, if there is a statistical tendency for future members of its community to be its kin. The present study develops this insight further by evaluating how an organism should value future benefits to itself or to its community. This leads to the determination of two separate discount rates. The individual (private) discount rate applies to delayed benefits to the organism itself; the concept of an individual discount rate has been applied in evolutionary biology at least as far back as Fisher (1930). The social discount rate applies to delayed benefits to the organism's community; this is a familiar concept in economics and philosophy (in relation to human communities) (e.g. Marglin 1963; Broome 1994), but has not hitherto been considered explicitly in evolutionary biology. This study shows how the social discount rate is relevant to understanding how an organism should choose between actions resulting in different costs or benefits to its community at different times.
Individual and social discount rates are calculated for a simple modelled asexual population of organisms in discrete patches with limited dispersal, subject to both individual mortality and patch destruction random events. The individual discount rate (expression 4.7) is equal to the sum of an organism's hazard rate for its patch to be destroyed and the hazard rate for it to suffer an individual mortality event, and is therefore equal to its overall death rate. The social discount rate is governed by the decay of expected relatedness to the focal organism of a member of its community chosen at random at some future time (expression 4.10), and is equal to the organism's hazard rate for its patch to be destroyed plus the product of the hazard rate for it to suffer an individual mortality event and the dispersal level (expression 4.13). The social discount rate is lower than the individual discount rate, except in the case of no population viscosity—i.e. a dispersal level of 100 per cent—when the two discount rates are the same. If patches are long-lived relative to individual life expectancy (Sherwood 2007), and the dispersal level is low, the social discount rate will be much lower than the individual discount rate in ratio terms.
The electronic supplementary material shows that the derived results apply exactly to a sexual population, as long as there are no sex differences in birth and death rates and the sex ratio is maintained at exactly 1 : 1 in each patch. If the sex ratio in a patch can drift away from 1 : 1, the results no longer apply exactly, but they will be an increasingly good approximation to optimal behaviour as patch size increases.
There is no direct dependence of the social discount rate on the number of individuals N in each patch. This may seem counterintuitive. For the present model, the dispersal level v and patch destruction rate ω are taken as given, i.e. they are treated as exogenously specified parameters of the model. It would not be unreasonable to assume, however, that a larger patch size may be associated with a lower level of dispersal or a lower patch destruction rate; explicitly putting such a relationship into the model would lead to a lower social discount rate with increasing patch size. This consideration would be important in a model that considered a population comprising patches of different sizes, but is beyond the scope of the present model.
A change from independent individual action to enforced collective action does not change the social discount rate (compare expressions 4.13 and 4.18). This may again seem counterintuitive: enforced collective action will tend to result in a shift towards more socially beneficial action (Hardin 1968). The social discount rate, however, is determined by the relative gain from delayed social benefits as compared with immediate social benefits. Enforced collective action will tend to increase social investment for both immediate and delayed community-wide gains, but the relative gain from a delayed benefit as compared with an immediate benefit remains the same.
A key assumption in this framework is that the delayed social benefits being considered are benefits to a local community. The meaning of ‘local’ is somewhat flexible with respect to general application of the theory. What matters is that there is some relevant sense in which a delayed benefit helps locals in preference to non-locals: evolution is ultimately driven by competition.
Preferences that imply positive valuation of future social benefits, as modelled here, are likely to be found in biological systems comprising long-lived groups within which there is a non-negligible level of relatedness. For example, a pathogen's success in spreading through a population of host organisms may depend in part on how the pathogen influences the future condition of a host it has infected (Frank 1992; Nowak & May 1994; Gandon et al. 2002). If the importance to the pathogen's success of this influence diminishes with delay, and this is reflected in the pathogen's strategy, then the pathogen may be said to exhibit social discounting.
To what extent is this analysis relevant to understanding social discounting in people? There are several considerations that may limit the direct application of these results to humans. In particular, the model assumes a simple population structure; the population is assumed to be homogeneous with respect to both individuals and patches; there is no scope for local population growth or decline; and individuals do not age. But insofar as humans have tended to live in long-lived groups or localities in which local kinship endures over time scales longer than the human lifespan, it seems plausible that some sort of future-valuing social preferences of the form considered here may have been selected in humans.
What of a social discount rate for decisions that impact on the whole population, rather than a locality or specific group? This question arises, for example, in the problem of global climate change. In the absence of competition between planets, there is no basis for behaviours that benefit the planet as a whole to be directly adaptive, and therefore no evolutionary basis for directly determining a social discount rate for global welfare. This seems to lead to a puzzle: why do people care at all about the long-term welfare of humanity as a whole? People may have evolved preferences for positive valuation of long-term general social welfare in ancestral environments in which such preferences would have mainly or always influenced actions with only local effects, and that therefore would have helped kin. But in the modern, global environment, such preferences may cause people to care about global problems such as climate change.
6. Conclusions
This study has derived analytical results for the individual and social discount rates that would arise through natural selection in a patch-structured iteroparous population with limited dispersal. The modelled population is not intended to be a realistic representation of any specific organism. Rather, it provides a simple framework allowing analytical results for the fitness consequences of different behaviours to be derived. These results yield insights into how individual mortality, community destruction and dispersal influence individual and social discount rates. In real organisms, individual and social discounting behaviour is likely to depend on a number of other factors, such as local ecological conditions and environmental variability; an organism's age; whether or not the population of the local community is growing; social structure within a community; and the spatial and network structure of communities. The last of these factors raises the possibility of several social discount rates corresponding to different levels of community organization within certain forms of population structure. Detailed representation of these considerations will require more complex models. However, it is likely that the insights contained in the simple model presented here will carry over to models of greater complexity.
Acknowledgements
This work was supported by the London School of Economics. I thank three anonymous reviewers for comments and suggestions.
References
- Atherton E., French S.1998Valuing the future: a MADA example involving nuclear waste storage. J. Multi-Criteria Decis. Anal. 7, 304–321 [Google Scholar]
- Barro R. J.1974Are government bonds net wealth? J. Polit. Econ. 82, 1095–1117 (doi:10.1086/260266) [Google Scholar]
- Broome J.1994Discounting the future. Philos. Public Aff. 23, 128–156 (doi:10.1111/j.1088-4963.1994.tb00008.x) [Google Scholar]
- Clark C. W.1973The economics of overexploitation. Science 181, 630–634 (doi:10.1126/science.181.4100.630) [DOI] [PubMed] [Google Scholar]
- Dasgupta P., Heal G.1974The optimal depletion of exhaustible resources. Rev. Econ. Stud. 41, 3–28 [Google Scholar]
- Dasgupta P. S., Heal G. M.1979Economic theory and exhaustible resources. Cambridge, UK: Cambridge University Press [Google Scholar]
- Diamond J. M.2005Collapse: how societies choose to fail or survive. London, UK: Allen Lane [Google Scholar]
- Fisher R. A.1930The genetical theory of natural selection Oxford, UK: Clarendon Press [Google Scholar]
- Fletcher J. A., Doebeli M.2009A simple and general explanation for the evolution of altruism. Proc. R. Soc. B 276, 13–19 (doi:10.1098/rspb.2008.0829) [DOI] [PMC free article] [PubMed] [Google Scholar]
- Frank S. A.1992A kin selection model for the evolution of virulence. Proc. R. Soc. Lond. B 250, 195–197 (doi:10.1098/rspb.1992.0149) [DOI] [PubMed] [Google Scholar]
- Frank S. A.1995Mutual policing and repression of competition in the evolution of cooperative groups. Nature 377, 520–522 (doi:10.1038/377520a0) [DOI] [PubMed] [Google Scholar]
- Gandon S., van Baalen M., Jansen V. A. A.2002The evolution of parasite virulence, superinfection and host resistance. Am. Nat. 159, 658–669 (doi:10.1086/339993) [DOI] [PubMed] [Google Scholar]
- Goulder L. H., Stavins R. N.2002Discounting—an eye on the future. Nature 419, 673–674 (doi:10.1038/419673a) [DOI] [PubMed] [Google Scholar]
- Grafen A.1984Natural selection, kin selection and group selection. In Behavioural ecology (eds Krebs J. R., Davies N. B.), pp. 62–84, 2nd edn. Oxford, UK: Blackwell Scientific Publications [Google Scholar]
- Gupta S., Van Houtven G., Cropper M.1996Paying for permanence: an economic analysis of EPA's cleanup decisions of superfund sites. RAND J. Econ. 27, 563–582 (doi:10.2307/2555844) [Google Scholar]
- Hamilton W. D.1975Innate social aptitudes of man: an approach from evolutionary genetics. In Biosocial anthropology (ed. Fox R.), pp. 133–155 New York, NY: John Wiley & Sons [Google Scholar]
- Hansson I., Stuart C.1990Malthusian selection of preferences. Am. Econ. Rev. 80, 529–544 [Google Scholar]
- Hardin G.1968The tragedy of the commons. Science 162, 1243–1248 [PubMed] [Google Scholar]
- Kaplan H. S., Robson A. J.2002The emergence of humans: the coevolution of intelligence and longevity with intergenerational transfers. Proc. Natl Acad. Sci. USA 99, 10 221–10 226 (doi:10.1073/pnas.152502899) [DOI] [PMC free article] [PubMed] [Google Scholar]
- Lee R. D.2003Rethinking the evolutionary theory of aging: transfers, not births, shape senescence in social species. Proc. Natl Acad. Sci. USA 100, 9637–9642 (doi:10.1073/pnas.1530303100) [DOI] [PMC free article] [PubMed] [Google Scholar]
- Lee R.2008Sociality, selection and survival: simulated evolution of mortality with intergenerational transfers and food sharing. Proc. Natl Acad. Sci. USA 105, 7124–7128 (doi:10.1073/pnas.0710234105) [DOI] [PMC free article] [PubMed] [Google Scholar]
- Lehmann L.2007The evolution of trans-generational altruism: kin selection meets niche construction. J. Evol. Biol. 20, 181–189 (doi:10.1111/j.1420-9101.2006.01202.x) [DOI] [PubMed] [Google Scholar]
- Lehmann L., Keller L., Sumpter D. J. T.2007The evolution of helping and harming on graphs: the return of the inclusive fitness effect. J. Evol. Biol. 20, 2284–2295 (doi:10.1111/j.1420-9101.2007.01414.x) [DOI] [PubMed] [Google Scholar]
- Manica A., Prugnolle F., Balloux F.2005Geography is a better determinant of human genetic differentiation than ethnicity. Hum. Genet. 118, 366–371 (doi:10.1007/s00439-005-0039-3) [DOI] [PMC free article] [PubMed] [Google Scholar]
- Marglin S. A.1963The social rate of discount and the optimal rate of investment. Q. J. Econ. 77, 95–111 (doi:10.2307/1879374) [Google Scholar]
- Nowak M. A., May R. M.1994Superinfection and the evolution of parasite virulence. Proc. R. Soc. Lond. B 255, 81–89 (doi:10.1098/rspb.1994.0012) [DOI] [PubMed] [Google Scholar]
- Pearce D., Groom B., Hepburn C., Koundouri P.2003Valuing the future: recent advances in social discounting. World Econ. 4, 121–141 [Google Scholar]
- Rambaud S. C., Torrecillas M. J. M.2005Some considerations on the social discount rate. Environ. Sci. Policy 8, 343–355 (doi:10.1016/j.envsci.2005.04.003) [Google Scholar]
- Robson A. J., Kaplan H. S.2003The evolution of human life expectancy and intelligence in hunter-gatherer economies. Am. Econ. Rev. 93, 150–169 [DOI] [PubMed] [Google Scholar]
- Rogers A. R.1994Evolution of time-preference by natural selection. Am. Econ. Rev. 84, 460–481 [Google Scholar]
- Rogers A. R.2003Economics and the evolution of life histories. Proc. Natl Acad. Sci. USA 100, 9114–9115 (doi:10.1073/pnas.1733942100) [DOI] [PMC free article] [PubMed] [Google Scholar]
- Rousset F.2004Genetic structure and selection in subdivided populations Princeton, NJ: Princeton University Press [Google Scholar]
- Sherwood S.2007Discounting and uncertainty: a non-economist's view. Clim. Change 80, 205–212 (doi:10.1007/s10584-006-9164-9) [Google Scholar]
- Sozou P. D.1998On hyperbolic discounting and uncertain hazard rates. Proc. R. Soc. Lond. B 265, 2015–2020 (doi:10.1098/rspb.1998.0534) [Google Scholar]
- Sozou P. D., Seymour R. M.2003Augmented discounting: interaction between ageing and time-preference behaviour. Proc. R. Soc. Lond. B 270, 1047–1053 (doi:10.1098/rspb.2003.2344) [DOI] [PMC free article] [PubMed] [Google Scholar]
- Sumaila U. R., Walters C.2005Intergenerational discounting: a new intuitive approach. Ecol. Econ. 52, 135–142 (doi:10.1016/j.ecolecon.2003.11.012) [Google Scholar]
- Taylor P. D.1992Altruism in viscous populations—an inclusive fitness model. Evol. Ecol. 6, 352–356 (doi:10.1007/BF02270971) [Google Scholar]
- Taylor P. D., Day T., Wild G.2007Evolution of cooperation in a finite homogeneous graph. Nature 447, 469–472 (doi:10.1038/nature05784) [DOI] [PubMed] [Google Scholar]
- Wilson D. S., Pollock G. B., Dugatkin L. A.1992Can altruism evolve in a purely viscous population? Evol. Ecol. 6, 311–341 [Google Scholar]