Prehension Synergies

Abstract

The precision grip requires the control of the normal and tangential forces exerted by the fingers as well as the control of the rotational equilibrium of the grasped object. Prehension synergies involve the conjoint changes in finger forces and moments during multifinger gripping tasks. Some of these adjustments are dictated by mechanics, whereas others are the result of a choice by the performer.

INTRODUCTION

People can manipulate hand-held objects, such as a glass filled with liquid or a tool, with amazing dexterity. Mechanically, the task of manipulation is complex. To perform a task successfully, the CNS should take into account object orientation in space, its weight, its inertia matrix, its center of gravity location, and the friction at the digit–object interfaces. Each of the five digits exerts three force components and three moment components on the object, resulting in 30 variables.

This paper is limited to a prismatic precision grip, that is, a grip by the tips of the digits in which the thumb and the fingers oppose each other. The analysis is restricted to a planar case. In such a task, three constraints (see below) are imposed on 15 elemental variables (five normal force components, five tangential finger force components, and five coordinates of the points of force application in the vertical direction). Therefore, prehension is an example of a biomechanically redundant task (see the Bernstein problem (3)). The grasping hand is a convenient object to study the Bernstein problem because all the involved forces can be measured directly and the relations between them investigated.

THE CONCEPT OF PREHENSION SYNERGY

The term prehension synergy is used to characterize a conjoint change of finger forces and moments during multifinger prehension tasks. A prehension synergy is manifested via adjustments to changes in task parameters (11), compensation for external or self-inflicted disturbances (e.g., when a force exerted by a finger is voluntarily changed, other fingers compensate for these changes without a time delay), and error compensation reflected in a negative covariation among elemental variables recorded in different trials (10) or in single trials of a long duration (9).

BASIC MECHANICS OF PRISMATIC GRIPS

Mechanically, an individual finger–sensor interaction is a so-called soft contact. In soft finger contacts, sticking of the fingertip to the sensor is not allowed, finger forces are unidirectional (the fingers press but do not pull on the object), and the moment of finger force is in the plane of sensor surface. In addition, fingers can roll on the sensor surface. Hence, the point of force application on the sensor can be displaced.

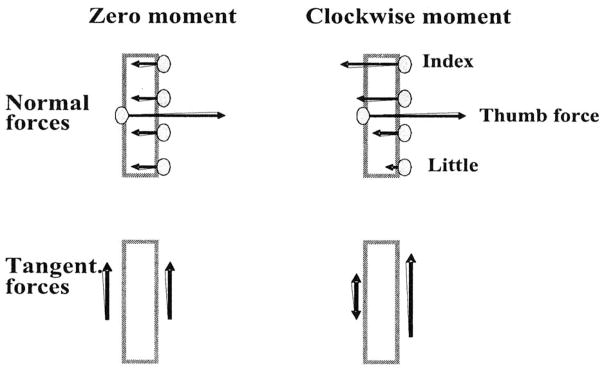
Consider a planar static task, when a vertically oriented hand-held object is grasped by a prismatic precision grip. We assume that the friction at the digit–object interface is sufficiently large to prevent the object from slipping at any exerted digit forces. For the system to be at rest, the sum of all forces and moments acting on the handle should be equal to zero. Hence, the following three requirements must be satisfied: (a) the sum of the normal forces of the four fingers must equal the normal force of the thumb; (b) the sum of the digit tangential forces must equal the weight of the hand-held object; and (c) the total moment produced by the digit forces must be equal and opposite to the external torque exerted on the object. Both normal and tangential finger forces can contribute to the moment production (Fig. 1).

Figure 1.

Schematic of digit forces at two different torques: (left) zero moment, (right) moment in a clockwise direction, (upper) normal forces, (bottom) tangential forces.

Because the torque constraint (c, above) includes all normal and tangential digit forces as well as the corresponding moment arms, whereas constraints (a) and (b) deal with the normal and tangential force components separately, the torque constraint (c) is core: any change of the finger forces must satisfy the condition of rotational equilibrium (11).

HIERARCHICAL (“VERTICAL”) ORGANIZATION OF PREHENSION, VIRTUAL FINGER

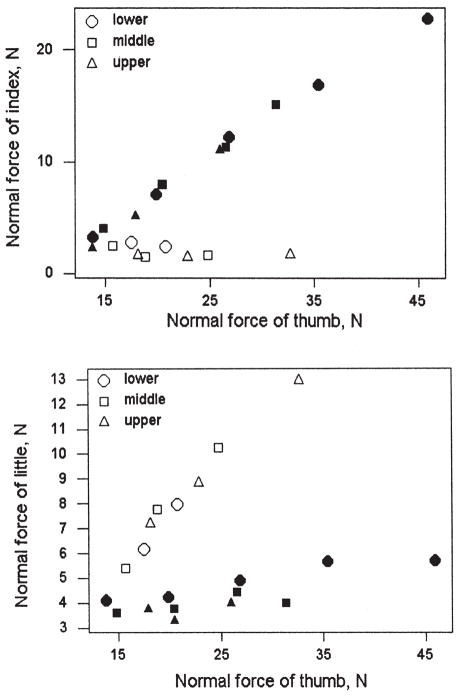
The forces of the fingers opposing the thumb can be reduced to a resultant force and a moment of force. This is equivalent to replacing a set of fingers with a virtual finger (VF) (6). A VF generates the same mechanical effect as a set of actual fingers. There is considerable evidence that the prehension synergy is organized in a hierarchical fashion and includes at least two levels of control. At the higher level (the VF level), the forces exerted by the thumb and the VF are defined. At the lower level, the forces exerted by the individual fingers (IF) are determined (2,11). When task parameters vary, the VF and IF forces can show qualitatively different patterns. In particular, the magnitudes of the normal forces of the thumb and of the VF show a strong positive correlation defined by constraint (a) over a range of loads and external torques (11). In contrast, at the level of IF, the relations of the individual finger forces to the external torque resemble rotated and distorted ‘V’ letters, with the branches corresponding to pronation and supination torques, respectively (Fig. 2). Prehension synergies can be studied both at the VF and IF levels.

Figure 2.

Relations between normal thumb forces and the fingers at various torque levels: (upper) index finger, (bottom) little finger. Filled symbols represent supination torques, and unfilled symbols represent pronation. Lower, middle, and upper refer to the different thumb positions. (Reprinted from Zatsiorsky, V. M., F. Gao, and M. L. Latash. Prehension synergies: effects of object geometry and prescribed torques. Exp. Brain Res. 148:77–87, 2003. Copyright © 2003 Springer-Verlag. Used with permission.)

“HORIZONTAL” ORGANIZATION OF PREHENSION SYNERGIES

At each of the above-mentioned levels, VF and IF, the prehension synergy may be composed of a number of multi-digit synergies (subsynergies) that function relatively independently. Among them, the grasping synergy has been most thoroughly studied.

Grasping Synergy

A grasping synergy is defined as a conjoint change of the normal digit forces. The normal forces of the thumb and the VF change concurrently (4,9). They are modulated by the weight of the object, gravity changes during parabolic flights, abrupt vertical load perturbations, tangential pulling forces, friction conditions, and inertial forces that occur during fast movements (7). Concurrent changes in the grasping and load forces during fast movements have led to the conclusion that these forces are controlled by a feed-forward mechanism; the central controller adjusts the grasping force in advance of the actual change in the load force.

Local skin anesthesia makes the coordination of the grip and load forces less precise. However, the general pattern of coordination is still preserved; an increase in the load force is associated with an increase in the grip force. This suggests that the grasping synergy is contributed to from both feed-forward control and feedback mechanisms triggered by cutaneous sensation (8).

Interaction of the Normal and Tangential Forces

Finger force vectors (Fig. 3) can be resolved into the normal and tangential forces. The contribution of the tangential forces to the total torque depends on the grasp geometry (the width of the grasp and the spreading of the fingers) and can exceed 50% (12,13). Hence, tangential forces cannot be neglected when studying prehension.

Figure 3.

Force vectors at the digit tips, group average (N = 8). The pronation torque efforts are positive, and the supination torque efforts are negative. The digit force direction depends on the torque. Note that in some tasks, not all fingers support the load, for example, during the negative torque production (supination efforts), the index and middle fingers generate forces that are directed downward. The weight is supported only by the thumb and the little finger. In all cases, however, the finger forces contribute to the torque production. [Adapted from Zatsiorsky, V. M., F. Gao, and M. L. Latash. Finger force vectors in multi-finger prehension. J. Biomechanics 36:1745–1749, 2003. Copyright © 2003 Elsevier Ltd. Used with permission.]

Correlations Among the Digit Forces and the Principle of Superposition at the Virtual Finger Level

The correlations among the elemental variables, that is, normal and tangential finger forces and the coordinates of force application, have been studied over multiple repetitions of a standard task (10) and across tasks that differed in the external load and moment as well as in the handle geometry and the thumb location (11,13,14).

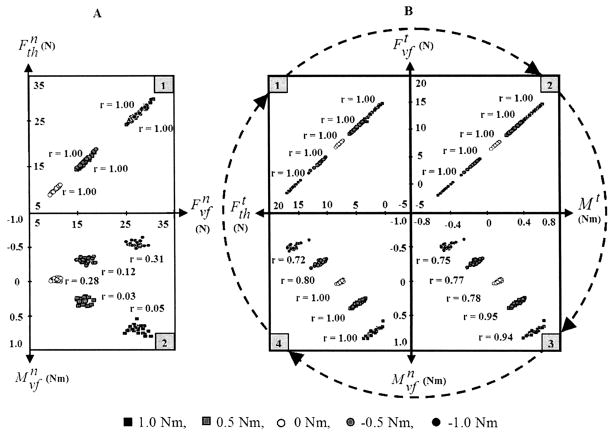
Over multiple repetitions of a standard task, all elemental variables belong to one of the two subsets (Fig. 4). The variables within each subset are highly correlated with each other over repetitions of a task, whereas variables from different subsets are not correlated significantly. The first subset (Fig. 4A) includes normal forces of the thumb and VF. The second subset (Fig. 4B) includes tangential forces of the thumb and VF, the moments produced by the tangential and normal forces, and the moment arm of the VF normal force $D_{vf}^n$ (the moment arm is not shown in the figure). As an illustration of the independence of the two subsets, trial-to-trial changes of the VF normal force $F_{vf}^n$ did not correlate with the variations of the moment of the normal force $M_{vf}^n$, (Fig. 4A-2). Because $M_{vf}^n$ is simply the product of $F_{vf}^n$ and its moment arm, this lack of correlation is counter intuitive. In contrast, a high correlation between $M_{vf}^n$ and the tangential force $F_{th}^t$ was discovered (Fig. 4B-4). The high correlation between $F_{th}^t$ and $D_{vf}^n$ also was found (not shown in the figure).

Figure 4.

Interrelations among the experimental variables. Representative examples. F and M designate the force and moment; superscripts n and t refer to the normal and tangential force components; subscripts th and vf refer to the thumb and virtual finger, respectively. In the right panels, the arrows signify the sequence of events resulting in the high correlation between $F_{th}^t$ and $M_{vf}^n$ (chain effects). Starting from the upper left panel (B-1) and moving consecutively along the arrows to B-2, B-3, and B-4, the reader can trace the chain effects explaining the correlation between $F_{th}^t$ and $M_{vf}^n$. (A-1) $F_{th}^n$ are strongly correlated with $F_{vf}^n$. (A-2) $F_{vf}^n$ are weakly correlated with $M_{vf}^n$. (B-1) $F_{th}^t$ versus $F_{vh}^t$. The values of $F_{th}^t$ and $F_{vf}^t$ lie o0n a straight line because $F_{th}^t+F_{vh}^t=\text{Constant}(\text{weight}\text{of}\text{handle})$. The different location of $F_{vf}^n$ and $F_{vf}^t$ values along the straight line signifies the different magnitude of M^t. (B-2) $F_{vf}^n$ versus $M^t([M^t=0.5(F_{vf}^t-F_{th}^v)],\text{where}d=68\text{mm})$. Because the sums $F_{th}^t$ and $F_{vf}^t$ are constant, a change in one of these forces determines the difference between their values and, hence, the moment that these force produce. (B-3) M^t versus $M_{vf}^n$. The relations are negative because the sum of the moment of the tangential forces M^t and the moment of the normal forces $M_{vf}^n$ must equal the resisted torque. (B-4) $M_{vf}^n$ versus $F_{th}^t$ . As a consequence of the chain effects, the moments of the normal forces $M_{vf}^n$ are highly correlated with the thumb tangential forces $F_{th}^t$. (Reprinted from Zatsiorsky, V. M., M. L. Latash, F. Gao, and J. K. Shim. The principle of superposition in human prehension. Robotica, 2004 (In press). Copyright © 2004 Cambridge University Press. Used with permission.)

Functionally, fine-tuning the variables from the first subset prevents the object from slipping out of the hand and from moving in the horizontal direction. Conjoint adjustments of the variables of the second subset maintained both the torque and the vertical orientation of the handle constant (they also prevent the object from moving in the vertical direction).

In another experiment, the resisted load and torque varied in a systematic manner. Highly significant effects were observed for both the normal and tangential finger forces (P < 0.001). However, the effects of the LOAD × TORQUE interaction were not significant (P > 0.6). These findings suggest an additive action of LOAD and TORQUE commands (14).

The findings in both experiments conform to the principle of superposition used in robotics, which states that some actions of a robot can be decomposed into several elemental actions that are independently controlled (1). The effects of the commands are additive. Such a control strategy decreases the computation time. The data described in this report agree with the principle of superposition and suggest that forces and moments of individual digits in humans are defined by two independent commands: “Grasp the object to prevent slipping” and “Maintain the rotational equilibrium of the object.” The effects of the two commands are summed up. In mathematical parlance, a completely compensated variability manifests a null space of variables. Hence, the CNS specifies at least two null spaces of the elemental variables during prehension.

Null Spaces Versus Optimization: Fine-Tuning Versus Gross Motor Control

The data on null spaces provoke a question on the applicability of numerous optimization models that have been used to solve the problem of motor redundancy. It is evident from Figure 4 that the CNS does not use a single optimal solution for a given task; despite all the efforts to perform the task in the same way, the variability of the elemental variables was substantial. Hence, if the CNS uses some kind of optimization to solve the motor redundancy problem in multifinger prehension (as can be concluded, for instance, from (12)), the optimization does not specify a single solution. It identifies only a subspace of solutions (a null space), whereas other mechanisms are responsible for fine motor adjustments of the motor variables to the task requirements.

Chain Effects

Bernstein (3) suggested that a synergy never reacted to a small local change with another small local change but with changes in all its elemental variables. These coordinated changes are manifested as a chain effect during prehension, when a local change in the output of an elemental variable leads to a sequence of changes in other elemental variables, some of which can be necessitated mechanically, whereas others may involve choice by the controller.

Figure 4B is an example of such an effect. Any change in $F_{th}^t$ requires a correlated change in $F_{vf}^t$. → A change in $F_{vf}^t$ induces a concomitant change in M^t. → Because the sum of $M_v^t$ and $M_{vf}^n$ is constant, an increase in M^t must be accompanied by a decrease in $M_{vf}^n$ and vice versa. → Consequently, $M_{vf}^n$ is highly correlated with $F_{th}^t$ (i.e., the moment of the normal forces correlates with the tangential force), whereas it is not correlated with the normal force.

Chain effects also have been found in experiments with changes in the handle width (12). An increase of the handle width induced the following effects: the tangential forces remained unchanged, the same tangential forces produced a larger moment M^t, the increased M^t was compensated by a smaller moment of the normal forces Mⁿ, and the individual normal finger forces were rearranged to generate a smaller moment. Specifically, the normal forces of the little and index fingers changed systematically with the width of the handle. These fingers have the largest moment arms and their forces were adjusted to decrease the moment of the normal forces when the handle width increased. Ultimately, the normal forces were modified in response to an alteration of the moment arms of the tangential forces.

Relations Necessitated and Not Necessitated by the Task Mechanics

Some of the observed relations are mechanically necessitated; the task cannot be performed in a different way. For instance, high correlations (approaching r = 1.0) between the normal forces of the thumb and the VF (Fig. 4A-1), which form the basis of a grasping synergy, can be expected from the task mechanics; for an object to be at rest, the normal forces of the thumb and VF must cancel each other. However, not all the observed relations are dictated by the task mechanics. Some relations represent a choice made by the CNS. For instance, the aforementioned lack of correlation between the total normal force and the moment that the force generates cannot be predicted from pure mechanics. Another example is the different contribution of the normal and tangential forces to the total torque production in various subjects and tasks (10,12,13). People have preferred patterns of sharing the total moment between the tangential and normal forces.

Individual Finger Control

In static tasks, fingers can act as force agonists and torque antagonists (see Fig. 1, upper). To prevent the object from slipping, the fingers act as agonists; each of them contributes to the total grip force. In contrast, the index and middle fingers and the ring and little fingers exert moments of force in opposite directions about the pivot point created by the thumb. Hence, when acting against an external torque, some fingers are torque antagonists. To minimize the total finger force, the antagonist fingers should not produce any force. At the same time, to prevent slipping of the object, they should contribute to grip force. These two requirements are contradictory.

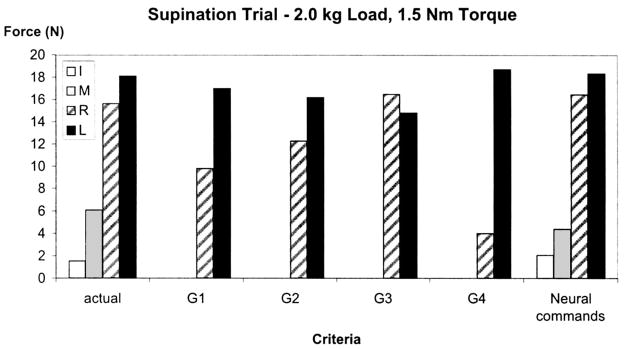
At any magnitude of the resisted load and torque, antagonist torques are always observed, even though the activation of the antagonist fingers is mechanically unnecessary (13). Attempts have been made to predict the activation of antagonist fingers by optimizing various cost functions that were based on the finger forces. None of the cost functions was able to predict the activation of the antagonist fingers in all force-torque production tasks (see Fig. 6). These attempts failed because finger interdependence (enslaving) was not taken into account.

Figure 6.

Comparison of actual force data with force patterns predicted by different optimization criteria. The norms of the following vectors were used as cost functions. (G1) Finger forces, N. (G2) Finger forces expressed in percent with respect to the maximal forces measured in single-finger tasks. (G3) Finger forces expressed in percent with respect to the maximal forces measured in four-finger tasks. (G4) Finger forces normalized with respect to the maximal moments that can be generated by the fingers while grasping an object with four fingers. Criteria G1, G2, G3, and G4 did not predict successfully the activation of the antagonist fingers, whereas optimization of the neural commands did. Hence, the objective function that accounted for the enslaving effects yielded better results. (Reprinted from Zatsiorsky, V. M., R. W. Gregory, and M. L. Latash. Force and torque production in static multi-finger prehension: biomechanics and control. II. Control. Biol. Cybern. 87:40 –49, 2002. Copyright © 2002 Cambridge University Press. Used with permission.)

Fingers of a hand are not completely independent. Turn your palm up and wiggle the ring finger. You will see that other fingers also move. Suppressing this inadvertent movement is a difficult task; not all people can do it. The involuntary finger activation—termed enslaving—is the result of both peripheral morphologic connections and neural mechanisms.

The interfinger connections have been quantitatively estimated using an interfinger connection matrix (IFM). The elements of an IFM depend on the number of fingers involved in the task; an increase in the number of fingers engaged in the task is associated with a reduction of the maximal force generated by each finger (force deficit). The IFMs can be computed by artificial neural networks (15) or they can be estimated by simple algebraic procedures (5). The latter approach led to the concept of finger modes that are combinations of finger forces induced by a central command to one of the fingers. The command intensity ranges from zero (no voluntary activation) to one (maximal voluntary contraction).

Optimization of the Central Commands

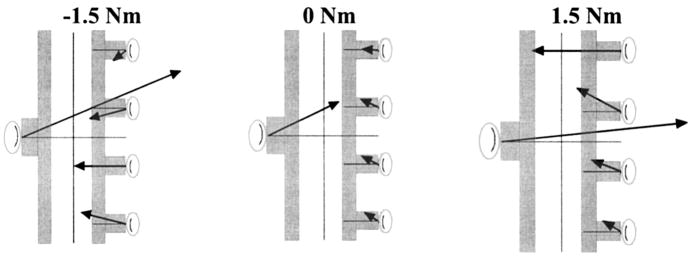
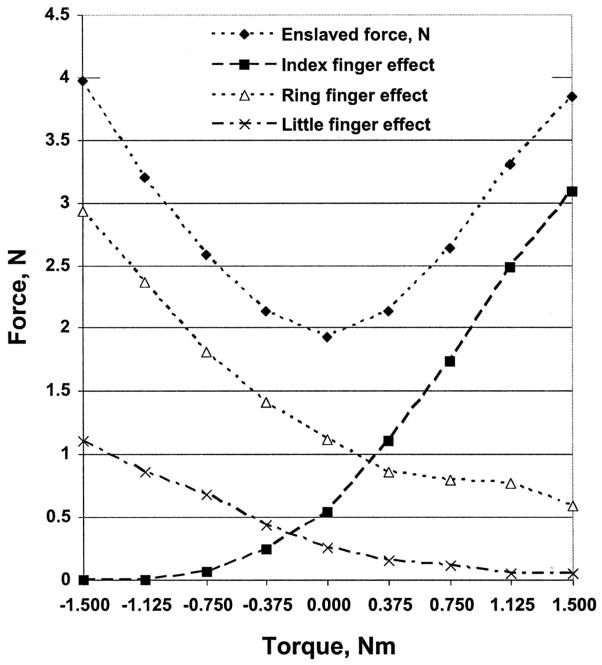
Knowledge of the IFM and the actual finger forces produced in a task allows the intensity of the central commands sent to the fingers to be determined (reconstructed). When the command intensity is reconstructed, the individual finger forces can be represented as a result of summation of the commands sent to a given finger (direct finger force) and the force induced by the commands sent to other fingers (enslaved force). The effects induced by commands to the individual fingers can be computed, for example, force exerted by the middle finger because of a command sent to the little finger can be determined (Fig. 5).

Figure 5.

Decomposition of the enslaved force produced by the middle finger at the different magnitudes of the generated torque, from −1.5 Nm to 1.5 Nm (the load was 2.0 kg). A representative example. An enslaved force is the force generated by a finger resulting from the commands sent to other fingers. The total enslaved force of the middle finger is the sum of the enslaving effects from the commands to the index, ring, and little fingers. The enslaved force increased with the increase of the torque magnitude. [Adapted from Zatsiorsky, V. M., R. W. Gregory, and M. L. Latash. Force and torque production in static multi-finger prehension: biomechanics and control. II. Control. Biol. Cybern. 87:40 –49, 2002. Copyright © 2002 Cambridge University Press. Used with permission.]

The patterns of finger forces of different people performing the same prehension task are very similar (11). It is natural to assume that people exert finger forces that are optimal with respect to some criteria, that is, the patterns of finger forces are selected to minimize a certain objective function. Among the possible objective functions are those that are based on the finger forces and those that are based on the central commands. The difference between the two is in enslaving effects; optimization of finger forces does not take into account the enslaving effects, whereas objective functions based on the central commands do. Command optimization has resulted in a better correspondence between actual and predicted finger forces (13) (Fig. 6). In particular, the activation of the antagonist fingers has been always predicted. The antagonist moments are—at least in part—the result of enslaving effects; strong commands to agonist fingers also activate antagonist fingers.

SUMMARY

Control of prehension is organized in a hierarchical fashion. Tangential digit forces can contribute more than 50% to the total torque and, hence, cannot be neglected. The forces and moments at the digit tips are defined by a linear superposition of at least two commands, one command to assure stable grasping and the second one to regulate the orientation of the object. A local change in the output of an elemental variable leads to chain effects, that is, a sequence of changes in other elemental variables. Some of the observed relations among the elemental variables are mechanically necessitated, whereas others involve choice made by the controller. Finger forces during prehension are affected by finger interdependence (enslaving). In particular, the antagonist moments in part are the result of enslaving effects; strong commands to agonist fingers also activate antagonist fingers.