Deterioration, death and the evolution of reproductive restraint in late life

Abstract

Explaining why organisms schedule reproduction over their lifetimes in the various ways that they do is an enduring challenge in biology. An influential theoretical prediction states that organisms should increasingly invest in reproduction as they approach the end of their life. An apparent mismatch of empirical data with this prediction has been attributed to age-related constraints on the ability to reproduce. Here we present a general framework for the evolution of age-related reproductive trajectories. Instead of characterizing an organism by its age, we characterize it by its physiological condition. We develop a common currency that if maximized at each time guarantees the whole life history is optimal. This currency integrates reproduction, mortality and changes in condition. We predict that under broad conditions it will be optimal for organisms to invest less in reproduction as they age, thus challenging traditional interpretations of age-related traits and renewing debate about the extent to which observed life histories are shaped by constraint versus adaptation. Our analysis gives a striking illustration of the differences between an age-based and a condition-based approach to life-history theory. It also provides a unified account of not only standard life-history models but of related models involving the allocation of limited resources.

A counted number of pulses only is given to us of a variegated, dramatic life.

Walter Pater

1. Introduction

Life-history theory is concerned with how organisms schedule reproduction over their life times (Roff 1992; Stearns 1992). Fundamental questions include whether animals deteriorate as they get older and how reproductive effort depends on age (Clutton-Brock 1984; Baudisch 2008; Monaghan et al. 2008). An influential result is the prediction of a ‘terminal investment’, which holds that, in the words of Isaac & Johnson (2005), ‘as organisms approach the end of their life, they should increase their reproductive effort’. Whereas the inconclusiveness of empirical data on this topic has drawn attention to difficulties of measuring reproductive effort (Clutton-Brock 1984), here we argue that the conditions favouring a terminal investment may be much more restrictive than hitherto thought, and in fact that the opposite pattern (i.e. reproductive restraint in late life) will often be optimal.

Models in which a terminal investment is optimal typically assume that organisms have a fixed maximum lifespan (Gadgil & Bossert 1970). Because any unused resources at the time of death are wasted, this leads to the prediction that organisms should raise their reproductive effort when running out of time towards the end of life. As a corollary, with the additional assumption that instantaneous mortality is positively associated with reproductive effort, this type of model predicts that mortality should increase with age.

Our starting point is the insight that an organism's lifespan may not be limited by time per se, but rather by the body's physical deterioration with time, i.e. the build up of damage. According to this view, an organism's state, rather than age, plays the central role in limiting its future performance (McNamara & Houston 1996; Kirkwood & Austad 2000; Mangel & Bonsall 2004; Munch & Mangel 2006; Baudisch 2008). Changes of state are likely to depend on an organism's activity. In particular, if damage accumulation increases with reproduction, then by reproducing fast an organism in effect brings forward its own death. From this perspective, it appears plausible that an organism might benefit from reproducing more slowly towards the end of its life, so as to defer death and hence gain more time for reproducing.

To analyse this problem formally, we need to account for all relevant aspects of an organism's life in a single currency that combines the value of reproducing, the cost of incurring damage, and the cost of dying.

2. The model

We envisage damage as a combined measure of physical (e.g. wear and tear) and physiological (e.g. DNA and cellular protein) deterioration that may culminate in an organism's death (e.g. Beckman & Ames 1998; Sohal et al. 2002; Monaghan et al. 2009). Let x be an organism's current level of damage (0 ≤ x ≤1) that increases with time t according to the positive-valued function D(u, x) = dx/dt. Here, u is a vector containing variables that specify the organism's strategy in terms of behaviour (e.g. how much time to spend feeding, reproducing, etc.) and in terms of resource allocation to different physiological needs (e.g. gamete production, repair of damage). Similarly, we define reproductive rate r(u, x) and instantaneous mortality rate m(u, x) as functions of the organism's strategy and damage level. If the organism has not succumbed to this mortality, then it dies once its damage level has reached the critical value of x = 1. For simplicity, we limit our attention to organisms that show little growth after reproductive maturity (e.g. birds, mammals), making it unnecessary to include body size as an additional state variable.

Integrating reproduction, damage and mortality in a single currency, we show (appendix A) that an optimal strategy must satisfy

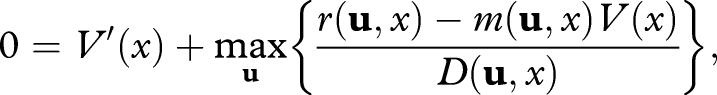2.1

where V(x) is the organism's reproductive value (defined as its expected future reproductive success if it behaves optimally in the future, McNamara & Houston 1996; Houston & McNamara 1999). The optimal strategy at any given damage level is given by the vector u maximizing the expression in curly brackets. If m is identically equal to zero, the optimal strategy maximizes the amount of reproduction per unit of damage accumulated (the Gilliam criterion, Werner & Gilliam 1984).

We can simplify the multi-dimensional strategy space by focusing on the rate of reproduction, r, achieved by a given strategy. Momentarily disregarding dependence on damage level x, this allows us to express damage accumulation D(r) and mortality m(r) as functions of r, such that activities other than reproduction are implicit in the shape of these functions. For example, if an organism has a fixed resource income and allocates it between reproduction and repair, then the greater the allocation to reproduction the faster damage builds up since less is available for repair; i.e. the resultant damage accumulation D(r) will be an increasing function. Although damage repair makes it logically possible for an organism to maintain indefinitely some given level of damage (Yearsley et al. 2005), in what follows we are concerned with organisms whose body eventually deteriorates, i.e. whose level of damage increases during their lifetime.

3. Results

Expressed in terms of our model, the classic assumption of a fixed maximum lifespan amounts to taking D(r) to be constant in r, so that damage builds up at a constant rate until, after a fixed time, x = 1 is reached and the animal dies. From equation (2.1), in this case, the optimal strategy maximizes

3.1

which is simply the difference between the rate r of fitness gain from reproduction and the rate m(r)V(x) of fitness loss due to mortality (cf. Houston & McNamara 1989, 1999). Because V(x) will eventually approach zero, expression (3.1) implies that an organism should maximally reproduce towards the end of its life, i.e. it should make a terminal investment.

We now assume, by contrast, that damage accumulation D(r) increases with reproduction r in an accelerating manner. Taking m(r) ≡ m to be constant for simplicity, from equation (2.1), the optimal strategy now maximizes

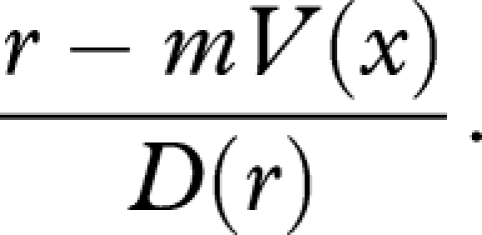3.2

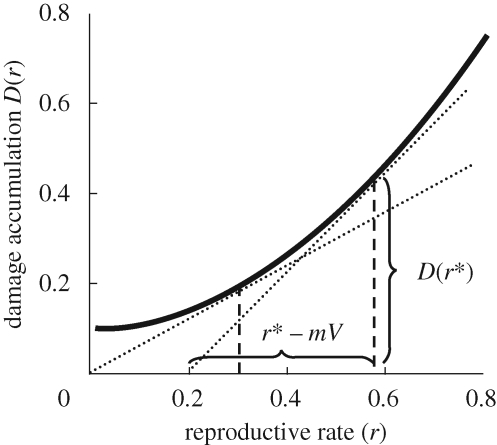
By the graphical argument shown in figure 1, this implies that the organism should reproduce less towards the end of its life, i.e. it should not make a terminal investment.

Figure 1.

A graphical determination of the optimal reproductive rate r* when instantaneous mortality m is constant. According to expression (3.2), we seek to maximize [r − mV(x)]/D(r). This is maximized by the r value of the point at which the tangent to the curve D(r) from the point (m V(x),0) and the curve touch. Note that at this point there is the highest net reproductive gain rate (r* − mV; horizontal curly bracket) per damage accumulation (vertical curly bracket). Two cases are shown: mV(x) = 0 and mV(x) = 0.2. Curly brackets refer to the latter case.

More generally, because reproduction may often involve activities (e.g. courting, parental care) associated with some risk (e.g. predation risk, Magnhagen 1991), we now consider that not only damage x but also instantaneous mortality increases with reproduction. In other words, we let both D(r) and m(r) be increasing functions of r. We can then distinguish three cases that differ in how the relative magnitude of two types of reproductive cost (in terms of damage accumulation versus instantaneous mortality) changes as the rate of reproduction increases. For each of these cases, we can show how optimal reproductive rate r* changes with x, and hence with age (appendix B).

Case 1. m(r)/D(r) increases as r increases, i.e. instantaneous mortality becomes relatively more relevant towards high rates of reproduction. In this case, r* increases with x.

Case 2. m(r)/D(r) decreases as r increases, i.e. damage accumulation becomes relatively more relevant towards high rates of reproduction. In this case, r* decreases with x.

Case 3. m(r)/D(r) is constant. In this case, r* is also constant.

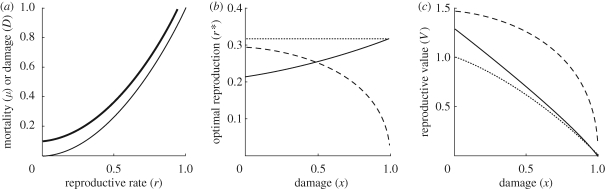
Examples of these cases are shown in figure 2. Note that, if m(r) is an increasing function, then in case 2, low reproduction in late life implies low instantaneous mortality in late life. This pattern of mortality has been termed negative senescence (Vaupel et al. 2004).

Figure 2.

The effect of different combinations of (a) mortality and damage functions on (b) optimal reproduction and (c) reproductive value, in a situation where instantaneous mortality depends on reproductive rate r but not on damage level x. Functions f(r) = r² (a; thin line) and g(r) = 0.1 + r² (a; bold line) are used as mortality and damage functions in three combinations to produce the results of case 1 (solid line) case 2 (dashed) and case 3 (dotted) in (b) and (c). Case 1: m(r) = f(r), D(r) = g(r); case 2: m(r) = g(r), D(r) = f(r); case 3: m(r) = D(r) = f(r).

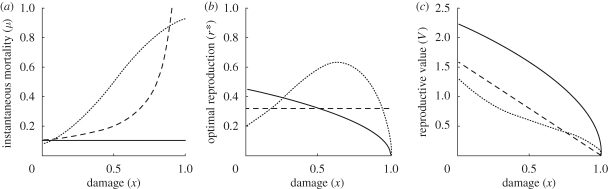
As made explicit in equation (2.1), instantaneous mortality m may depend not only on reproduction but also on an organism's current damage level x. Specifically, because damage may compromise an individual's ability to master or avoid dangerous situations, we may expect mortality to increase with damage level x. This makes it more difficult to predict patterns of reproduction. As stated above (see expression (3.2)), reproductive restraint in late life is predicted if mV approaches zero as x → 1. However, given that V will decrease with x, whereas m may increase, it is not clear a priori whether mV will generally increase or decrease with x. In fact, by numerically computing the optimal strategy by dynamic programming (Houston & McNamara 1999) using equation (2.1), we can find examples where r* first increases and then decreases with x, peaking at an intermediate damage level (figure 3b).

Figure 3.

Effect of different (a) mortality functions on (b) optimal reproduction and (c) reproductive value, in a situation where instantaneous mortality depends on damage level x but not on reproductive rate r. Mortality functions are: m(x) = 0.1 (solid line); m(x) = 0.1 + r² (dashed); m(x) = e^5x−2.5/(1 + e^5x−2.5) (stippled). Damage function: D(r) = r².

4. Discussion

Our model can account for the evolution of diverse reproductive trajectories. Specifically, consistent with common patterns of age-specific reproduction (e.g. in pipefish (Syngnathus typhle; Billing et al. 2007); reindeer (Rangifer tarandus; Weladji et al. 2002); horses (Equus caballus; Cameron et al. 2000)), we outline broad conditions that favour reproductive restraint in late life, thus putting into perspective the classical (but rarely empirically verified, e.g. Velando et al. 2006) prediction of a terminal investment (Williams 1966).

An earlier model has suggested that a reproductive decline with age, where it occurs, may reflect a constraint on older organisms’ ability to acquire resources. According to this view, older organisms are so limited in their resource acquisition that, despite making a relatively greater investment in reproduction, they reproduce less in absolute terms (Cichon 2001). This differs profoundly from our present suggestion, previously derived computationally for a special case (Yearsley et al. 2005), that ageing individuals may benefit by reducing reproduction in both absolute and relative terms.

To appreciate the intuition behind this result, consider an organism that faces an extrinsic mortality risk. The organism should reproduce quickly before mortality strikes, although time pressure will be less severe if the extrinsic mortality rate is low. An organism that has accumulated substantial damage can expect to die soon for intrinsic reasons, and hence faces a low probability of being struck by extrinsic mortality in its remaining lifetime. This reduces time pressure in a way analogous to a low extrinsic mortality rate, allowing the organism to reproduce more slowly if other advantages (e.g. greater cost efficiency) can thus be gained.

In contrast to earlier attempts to find exceptions to the prediction of a terminal investment, our model does not require us to assume arguably implausible functions (Roff 1992) or a rapidly growing population (Charlesworth & Leon 1976), nor do we need to invoke a state variable (such as body size) that has an effect on mortality and the ability to reproduce (Baudisch 2008).

Our results apply to any case in which an organism is subject to a mortality risk and reproduction ceases once a state variable falls to zero. Parker & Courtney (1984) investigate models in which females can lay a fixed total number of eggs during their lifetime. Thus, in these models, the proportion of eggs already laid can be interpreted as a measure of damage, and their finding that the optimal clutch size decreases with age is given a broader context by our general analysis. Similarly, in models of sperm allocation, amount of sperm is analogous to damage and when future matings are uncertain, the optimal sperm allocation decreases as number of copulations increases (Reinhold et al. 2002).

Because life-history strategies are likely to affect multiple aspects of an organism's biology, our model may have implications beyond the interpretation of reproductive patterns. For example, it is tempting to speculate that the decreasing metabolic rate in ageing vertebrates (e.g. Moe et al. 2008) may not (or not wholly) be a side effect of ageing, but may instead reflect a strategy aimed at slowing down damage accumulation. Devising empirical tests for these ideas may turn out to be challenging. Detailed studies on the mechanistic basis of ageing, including a characterization of any trade-offs involving damage, reproduction and mortality, may prove crucial in this endeavour. We hope that our study will motivate empiricists in pursuing this line of research.

Appendix A. Derivation of equation (2.1)

At any time, an organism is characterized by its current level of damage x, in the range 0 ≤ x ≤1. Once the damage builds up to x = 1, the animal dies (if it has not previously done so).

An organism's strategy specifies, for each level of damage, the actions u taken at that level of damage. Here u is a vector containing any number of variables that specify, e.g. how hard to work to obtain food, how much energy to allocate to reproduction and how much to invest in repair.

A strategy determines m(u, x), the instantaneous rate of mortality at level of damage x, r(u, x), the instantaneous rate of reproduction at level of damage x, and D(u, x), the instantaneous rate of build up of damage at the level of damage x.

Note that these are all rates per time. In particular, m is a mortality rate, not a probability.

These quantities determine x(t), the level of damage at age t (it is assumed that x(0) = 0), S(x), the probability of survival to the level of damage x, and V(x) the reproductive value at level of damage x (equals future lifetime reproductive success).

Damage builds up as

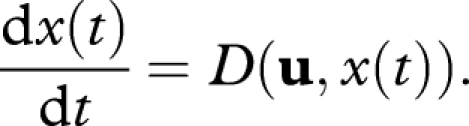A 1

Since m(u,x(t)) is the instantaneous mortality rate at age t, we have

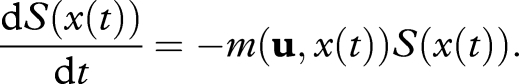A 2

However, rather than using m, it is more convenient to work with

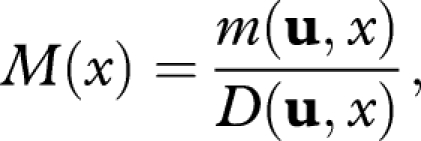A 3

which is the rate of mortality per damage accumulated. By equations (A 1) to (A 3),

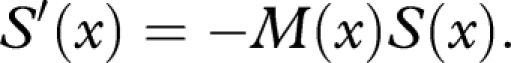A 4

Note that the probability that an individual with damage x is still alive at damage level y is S(y)/S(x).

Again, rather than using r it is more convenient to work with

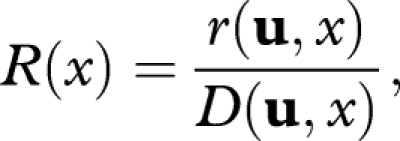A 5

which is the rate of reproduction per unit of damage. Then

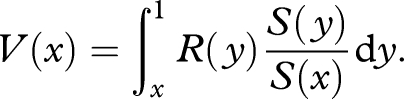A 6

Differentiating both sides of equation (A 6) gives

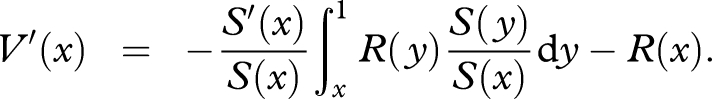Thus, by equations (A 4) and (A 6),

A 7

(a). Optimality equations

An optimal strategy maximizes V(0). Let the function 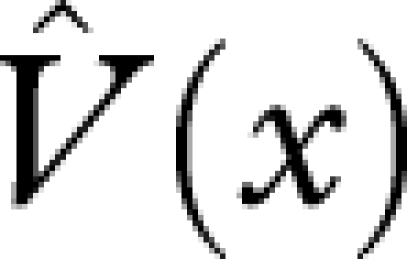satisfy the terminal condition 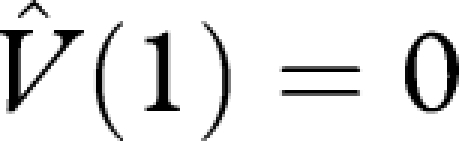and satisfy

A 8

Here, at each x, the maximization is over all actions available at that x. Consider the strategy that achieves the maximum for each x. We refer to this as the currency maximization strategy. Let 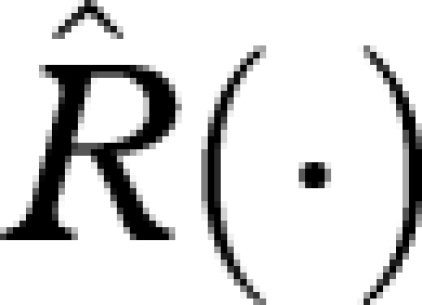and 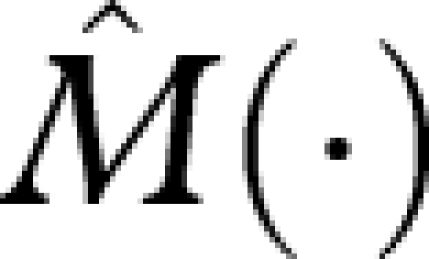denote the R and M functions under this strategy, so that

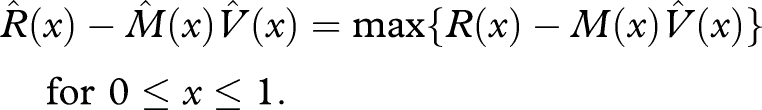A 9

Then by equation (A 8),

A 10

Comparing this equation with equation (A 7), and noting that 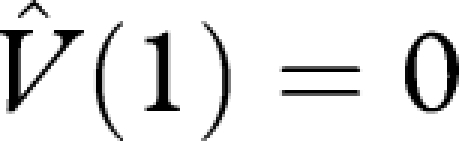, we see that 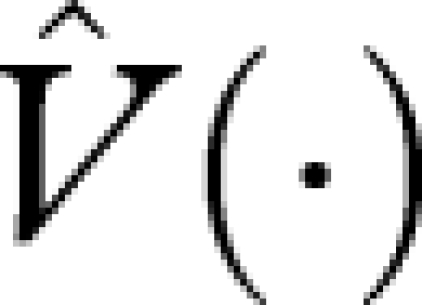is the reproductive value function under this strategy.

We now compare the currency maximization strategy we have just defined with an alternative strategy with functions 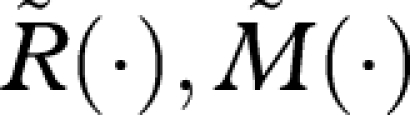and 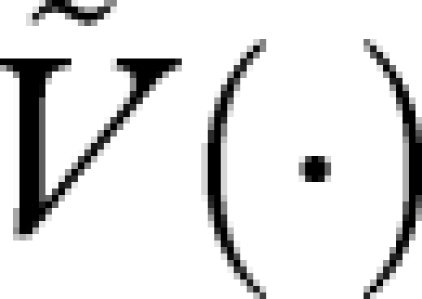. By equations (A 7) and (A 8),

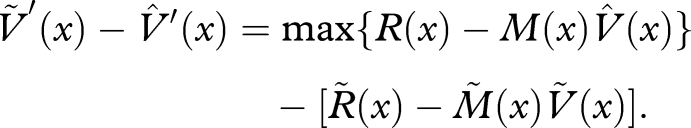Thus

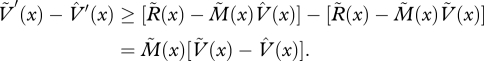Setting 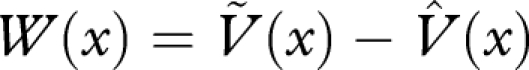, we then have 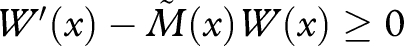. Setting

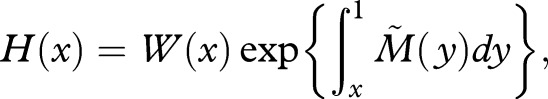we obtain H′(x) ≥ 0. Since this holds for all x in the range 0 ≤ x ≤ 1, and since 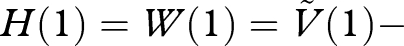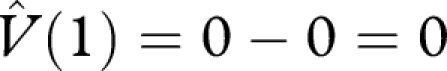, we deduce that H(0) ≤ 0. Thus, W(0) ≤ 0, and hence 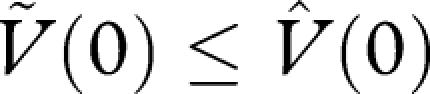. This shows that the currency maximization strategy achieves a lifetime reproductive success that is at least as great as the alternative strategy. As the alternative strategy was arbitrary, we deduce that the currency maximization strategy maximizes lifetime reproductive success, i.e. is an optimal strategy.

Equation (2.1) is then obtained from equation (A 8) by re-expressing R and M in terms of r, m and D.

Appendix B. Change in reproductive rate when mortality is independent of x

In this appendix, we consider the special case in which the mortality rate can depend on r, but does not depend on x, i.e. μ ≡ μ (r). In this case, the optimal rate of reproduction at level of damage x maximizes

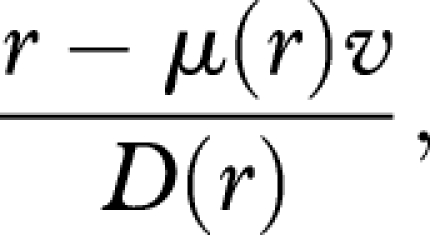B 1

where we have abbreviated V(x) to v. This notation makes clear that we can regard r* as a function of v, and its dependence on x is through the dependence of v on x. It will be convenient to abuse notation and regard r* as a function of v in the analysis below.

Differentiating expression (B 1) with respect to r and setting the derivative equal to zero at r = r* gives

B 2

Note that since expression (B 1) is maximized at r* the second derivative of expression (B 1) must be negative at r*. This condition reduces to

B 3

Treating r* in equation (B 2) as a function of v, and differentiating with respect to v, we have

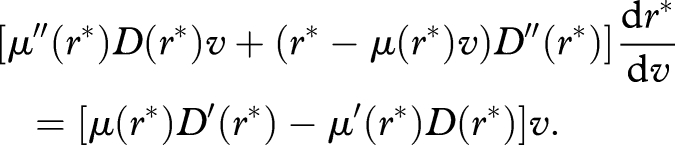Thus, by inequality (B 3),

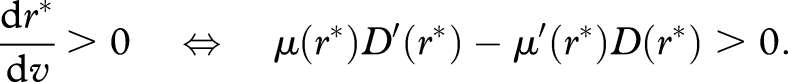We now distinguish three cases.

Case 1. μ(r)/D(r) increases as r increases. In this case, μ(r)D′(r) − μ′(r)D(r) < 0. Thus, r* decreases with increasing v. As v = V(x) decreases as x increases, r* increases as x increases. Thus, r* increases with age.

Case 2. μ(r)/D(r) decreases as r increases. In this case, μ(r)D′(r) − μ′(r)D(r) > 0. Thus, r* decreases as x increases, so that r* decreases with age.

Case 3. μ(r)/D(r) is constant in r. In this case, μ(r)D′(r) − μ′(r)D(r) = 0. Thus, r* remains constant as x increases.