Preliminary Analyses of Tympanic-Membrane Motion from Holographic Measurements

Abstract

Computer-aided, personal computer (PC) based, optoelectronic holography (OEH) was used to obtain preliminary measurements of the sound-induced displacement of the tympanic membrane (TM) of cadaver cats and chinchillas. Real-time time-averaged holograms, processed at video rates, were used to characterise the frequency dependence of TM displacements as tone frequency was swept from 400 Hz to 20 kHz. Stroboscopic holography was used at selected frequencies to measure, in full-field-of-view, displacements of the TM surface with nanometer resolution. These measurements enable the determination and the characterisation of inward and outward displacements of the TM. The time-averaged holographic data suggest standing wave patterns on the cat’s TM surface, which move from simple uni-modal or bi-modal patterns at low frequencies, through complicated multimodal patterns above 3 kHz, to highly ordered arrangements of displacement waves with tone frequencies above 15 kHz. The frequency boundaries of the different wave patterns are lower in chinchilla (simple patterns below 600 Hz, ordered patterns above 4 kHz) than cat. The stroboscopic holography measurements indicate wave-like motion patterns on the TM surface, where the number of wavelengths captured along sections of the TM increased with stimulus frequency with as many as 11 wavelengths visible on the chinchilla TM at 16 kHz. Counts of the visible number of wavelengths on TM sections with different sound stimulus frequency provided estimates of wave velocity along the TM surface that ranged from 5 m s⁻¹ at frequencies below 8 kHz and increased to 25 m s⁻¹ by 20 kHz.

Introduction

The tympanic membrane (TM) is the initial structure involved in the middle-ear’s acousto-mechanical transformation of environmental sounds to sound pressure and velocity within the inner ear. While some basic facts are known about the mechanics of the healthy TM at low frequencies, there are still many unresolved issues: e.g. the effect of TM shape and pathology on function, the effect of large differences in TM mechanical properties across species, the nature of TM function at higher frequencies, and the hypothesised presence of standing or travelling surface waves on the surface of the TM [1].

Many of these issues can be addressed by measuring the motion of the entire TM surface in response to sound. Most of the few extant measurements of TM motion were obtained with time-averaged laser holography using photographic plates, which require lengthy and elaborated photographic processing procedures [2–12]. With this approach, displacements were estimated by counting fringes in the recorded interference patterns. New opto-electronic techniques [13–23] that take advantage of high-speed, high-resolution, digital cameras and advanced signal processing techniques can generate holographic images in real-time at rates up to 500 frames s⁻¹ [21].

In this work, we measured sound-induced motion of the TM surface in cat and chinchilla using two new opto-electronic techniques: real-time time-averaged holography, which provides displacement amplitude and resolution data comparable to the earlier technique but is much faster, as processing of holographic data is performed digitally; and stroboscopic holography, which rapidly determines the magnitude and phase of displacements over the entire TM surface with nanometer resolution [19–21]. We (i) demonstrate the utility of the new techniques; (ii) validate them by comparing with other measurements; (iii) present new data that suggest fundamental differences in the mechanics of the chinchilla and cat TM; and (iv) show new evidence of standing waves in TM motion in cat and chinchilla.

Methods

Personal computer (PC) based opto-electronic holography system

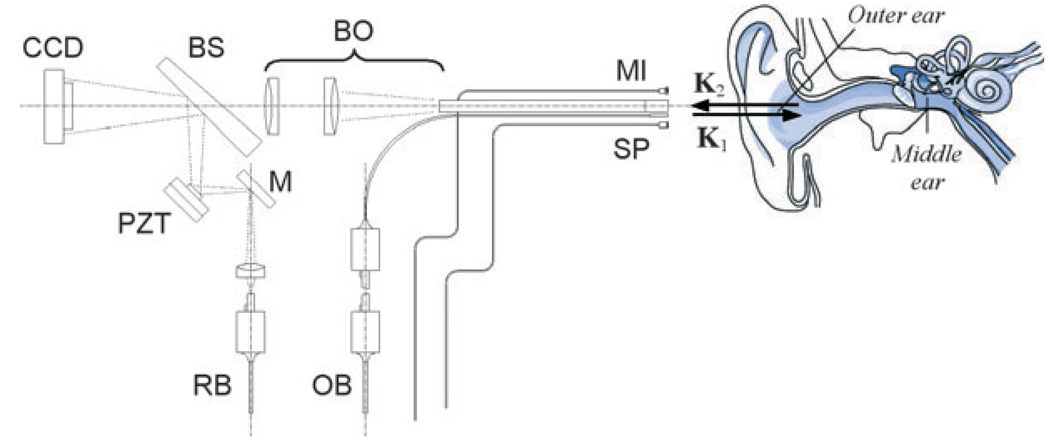
Opto-electronic holography system (OEH) has successfully been applied to different fields of non-destructive testing of mechanical components subjected to static and dynamic loading conditions [14–21]. Being noninvasive and providing qualitative and quantitative full-field information are some of the main advantages of OEH over other experimental techniques. In addition, it requires much less mechanical stability than does required in conventional holographic interferometry, which makes it very suitable for in-vivo investigations. OEH, implemented in a PC, is based on a combined use of speckle interferometry, phase stepping, and image acquisition and processing techniques. Figure 1 shows a diagram of the optical probe (OP) being developed for characterising shape and deformation of objects of interest in confined spaces by OEH.

Figure 1.

Optical probe (OP) being developed for characterising shape and deformation of samples in confined spaces by optoelectronic holography (OEH). CCD, the high speed camera; BS, the beam splitter; BO, the borescope assembly; PZT, the piezoelectric mirror; M, the steering mirror; RB and OB, the reference and object beam fibres; MI, the microphone; SP, the sound source; K₁ and K₂, the illumination and observation direction vectors

Optical probe is constructed with a borescope assembly (BO) consisting of relay and imaging lenses. Coherent light from a 532-nm laser source is coupled into single mode optical fibres to define the object illumination fibre (OB) and the reference beam fibre (RB). Output of the OB is attached to the BO to illuminate the sample of interest whereas the output of the RB is directed to the steering mirror (M), to the piezoelectric mirror (PZT) for modulation of the laser source, and to the beam splitter (BS). Light collected by the BO is combined with the RB at the CCD camera. Acoustic excitation of the sample and the monitoring of the sound pressure levels (SPL) are achieved by the use of the sound source (SP) and the microphone (MI) respectively. In the configuration shown in Figure 1, K₁ and K₂ represent the illumination and observation direction vectors defining the sensitivity vector K [5, 15]. K₁ and K₂ are parallel to each other in the nominal configuration, which enable the measurement of the inward and outward components of deformation of the samples of interest.

DOUBLE-EXPOSURE INVESTIGATIONS

In OEH, information is extracted from the interference pattern of object and reference beams having complex light fields F_o and F_r. After the beam splitter, and considering phase stepping, irradiance I_n of the combined wavefronts as recorded by the n-th video frame can be described by

$$\begin{array}{cc}{I}_n\hfill & =(F_{\mathrm{o}}+F_{\mathrm{r}}){(F_{\mathrm{o}}+F_{\mathrm{r}})}^{*}\hfill \\ \hfill & =|A_{\mathrm{o}}{|}^2+|A_{\mathrm{r}}{|}^2+2A_{\mathrm{o}}{A}_{\mathrm{r}}\text{cos}[({\varphi }_{\mathrm{o}}-{\varphi }_{\mathrm{r}})+\mathrm{\Delta }{\theta }_n],\hfill \end{array}$$ (1)

where A_o and A_r are the amplitudes of the object and reference beams, ϕ_o the randomly varying phase of the object beam, ϕ_r the phase of the reference beam and Δθ the known phase step introduced between the frames [14–21]. To facilitate double-exposure investigations, the argument of the periodic term of Equation (1) is modified to include the phase change because of deformations of the object of interest subjected to specific loading and boundary conditions. This phase change is characterised by the fringe-locus function Ω, whose constant values define fringe loci on the surface of the object as

$$\begin{array}{cc}\mathrm{\Omega }(x,y)=2\pi n(x,y)\hfill & =[{\mathbf{K}}_2(x,y)-{\mathbf{K}}_1(x,y)]·\mathbf{L}(x,y)\hfill \\ \hfill & =\mathbf{K}(x,y)·\mathbf{L}(x,y),\hfill \end{array}$$ (2)

where n(x,y) is the interferometric fringe order at the (x,y) location in the image space, K the sensitivity vector, and L the displacement vector [5, 15]. Therefore, the irradiance from a deformed object is described by

$$I_n^{\prime }=I_{\mathrm{o}}+I_{\mathrm{r}}+2A_{\mathrm{o}}{A}_{\mathrm{r}}\text{cos}(\mathrm{\Delta }\varphi +\mathrm{\Omega }+\mathrm{\Delta }{\theta }_n),$$ (3)

where Δϕ = ϕ_o − ϕ_r, and I_o and I_r represent the intensities of the object and reference beams respectively. In Equation (3), I_o is assumed to remain constant and the (x,y) arguments were omitted for clarity. As it is Ω, which carries information pertaining to mechanical displacements and/or deformations, the OEH’s video processing algorithm eliminates Δϕ from the argument of the periodic function of the irradiance distributions, given in Equations (1) and (3), yielding an image which is intensity modulated by a periodic function with Ω as the argument [14–21].

The OEH operates in either display or data mode. In display mode, interference patterns are observed at video rate speed and are modulated by a cosinusoidal function of the form

$$8A_{\mathrm{o}}{A}_{\mathrm{r}}\text{cos}\left(\frac{\mathrm{\Omega }}{2}\right),$$ (4)

which is obtained by performing specific mathematical operations between frames acquired at the undeformed and deformed states, described by Equations (1) and (3) respectively [14–21]. This mode is used for adjusting the OEH system and for qualitative investigations. Data mode is used for performing quantitative investigations. In the data mode, two images are generated: a cosinusoidal image

$$D=64A_{\mathrm{o}}^2{A}_{\mathrm{r}}^2\text{cos}(\mathrm{\Omega }),$$ (5)

and a sinusoidal image

$$N=64A_{\mathrm{o}}^2{A}_{\mathrm{r}}^2\text{sin}(\mathrm{\Omega }),$$ (6)

which are processed simultaneously to produce quantitative results by computing and displaying at video rates [21]

$$\mathrm{\Omega }={\text{tan}}^{-1}\left(\frac{N}{D}\right).$$ (7)

Because of the discontinuous nature of Equation (7), recovery of the continuous spatial phase distributions Ω(x,y) requires the application of phase unwrapping algorithms [17, 18, 21]. In the measurements presented in this article, the double-exposure mode of the OEH was used for quantitative stroboscopic measurements [20, 21].

TIME-AVERAGED INVESTIGATIONS

For performing time-averaged investigations, or modal analysis of objects of interest, using the OEH, it is necessary to take into consideration a time varying fringe-locus function Ω_t (x,y,t), which is related to periodic motion of the object under investigation [5, 14–21]. Using Equation (3), it is possible to write

$$\begin{array}{cc}{I}_t(x,y,t)=\hfill & I_{\mathrm{o}}(x,y)+I_{\mathrm{r}}(x,y)\hfill \\ \hfill & +2A_{\mathrm{o}}(x,y)A_{\mathrm{r}}(x,y)\text{cos}[\mathrm{\Delta }\varphi (x,y)\hfill \\ \hfill & +{\mathrm{\Omega }}_t(x,y,t)+\mathrm{\Delta }{\theta }_n].\hfill \end{array}$$ (8)

As the CCD camera registers average intensity at the video rate characterised by the period Δt, the intensity that is observed is

$$I(x,y)=\frac{1}{\mathrm{\Delta }t}{\displaystyle \underset{t}{\overset{t+\mathrm{\Delta }t}{\int }}{I}_t(x,y,t)\mathrm{d}t,}$$ (9)

and using phase stepping, the resultant intensity distribution for the nth frame is of the form

$$\begin{array}{cc}{I}_{t_n}(x,y)=\hfill & I_{\mathrm{o}}(x,y)+I_{\mathrm{r}}(x,y)\hfill \\ \hfill & +2A_{\mathrm{o}}(x,y)A_{\mathrm{r}}(x,y)\text{cos}[\mathrm{\Delta }\varphi (x,y)\hfill \\ \hfill & +\mathrm{\Delta }{\theta }_n]M[{\mathrm{\Omega }}_t(x,y)],\hfill \end{array}$$ (10)

where M [Ω_t (x, y)] is known as the characteristic function determined by the temporal motion of the object. For the case of sinusoidal vibrations with the period much shorter than the video framing time

$$M[{\mathrm{\Omega }}_t(x,y)]=J_{\mathrm{o}}[{\mathrm{\Omega }}_t(x,y)],$$ (11)

where J_o [Ω_t (x, y)] is the zero order Bessel function of the first kind [5, 15]. Equation (10) contains four unknowns: irradiances I_o and I_r, phase difference Δϕ, and the fringe-locus function Ω_t (x,y). The OEH’s video frame processing algorithm eliminates Δϕ from the argument of the irradiance function given by Equation (10), using a set of equations obtained at a specific step size value of Δθ [14–21].

For time-averaged investigations, the OEH can work in either display or data modes. In the display mode, interference patterns are observed at video rate and are modulated by a function of the form

$$4A_{\mathrm{o}}{A}_{\mathrm{r}}|M({\mathrm{\Omega }}_t)|.$$ (12)

This mode is used for adjusting the OEH system and for qualitative investigations. The data mode [15–17] is used for performing quantitative investigations. In the data mode, additional images of the form

$$16I_{\mathrm{o}}{I}_{\mathrm{r}}[M^2({\mathrm{\Omega }}_t)]$$ (13)

are generated for quantitative processing and extraction of Ω_t [15, 20].

Preparation of tympanic membrane

The heads of a cat and two chinchillas used in other physiological experiments were harvested from dead animals. The bilateral bullae were exposed and the bullar walls partially removed to expose the tympanic cavity. The cartilaginous ear canals were resected, and the bony external auditory canals were drilled away until at least 90% of the TM surface was visible. To enhance diffuse reflection, the TM was painted with a thin layer of white-ink (minimum effects on the dynamics of the TM were observed, as verified with OEH an laser Doppler vibrometry [24, 25]). The head was oriented in the object beam of the OEH system with the surface of the TM perpendicular to the beam. The reflected object beam was focused with the optical probe (OP) of the OEH and a glass-backed sound coupler was placed over the TM. Acoustic excitation of the TM was provided by the Beyer DT 48 sound source (SP) and the probe-tube microphone (MI) was integrated into the coupler.

Measurement of the Dynamics of TM

To characterise the dynamics and deformations of the TM, the following procedures were used.

1. Time-averaged holography was applied first in order to identify fundamental natural frequencies of vibration and corresponding mode shapes. This was achieved by ramping the excitation frequency and amplitude through ranges of interest with the OEH system providing holographic imaging data at video rates >30 Hz.

2. After identification of fundamental frequencies and excitation levels, double-exposure holographic measurements were performed. For a selected fundamental frequency and an excitation level of interest, stroboscopic illumination to measure optical phase between two stimulus phases was applied. Measured optical phase, described by Equation (7), was unwrapped and nanometer scaled deformations determined by the application of Equation (2) while taking into consideration parallel illumination–observation conditions, as obtained by the geometry of the OEH setup and defined by the sensitivity vector K.

Time-averaged holography: iso-displacement contours in cat and chinchilla, 400 Hz to 19 kHz

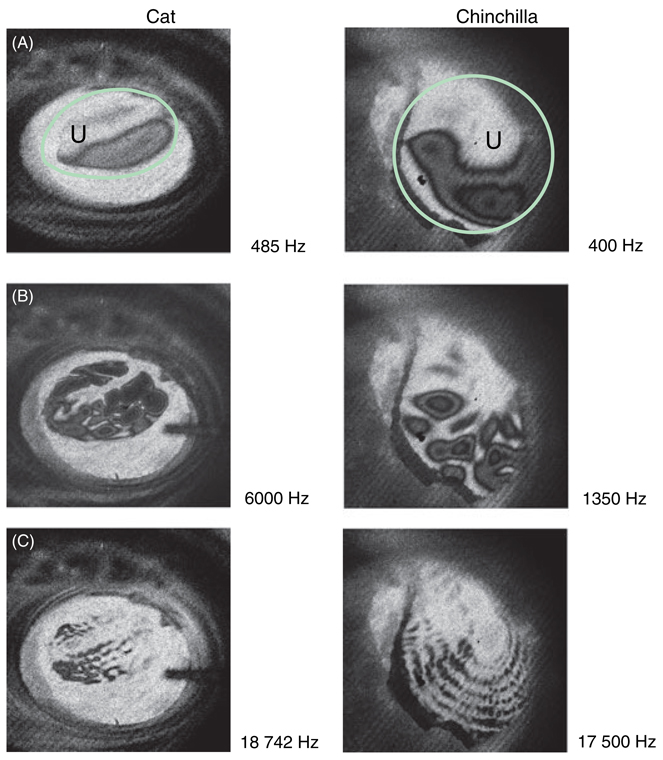
Time-averaged holograms that describe the sound-induced displacements of the TM of cat and chinchilla are shown in Figure 2 at selected frequencies and sound levels. Increasing displacements are indicated by the corresponding fringe densities. At low frequencies, the TM displacement patterns in cat and chinchilla are simple, as shown in Figure 2A. These displacements are characterised by one or two regions of maximal displacement.

Figure 2.

Time-averaged holograms in tympanic membrane (TM) of cat and chinchilla at selected frequencies showing: (A) simple, (B) complex and (C) ordered displacement patterns. The TM is outlined in (A) and the umbo is marked by ‘U’

At intermediate frequencies, the TM displacement patterns are complex, as shown in Figure 2B. These displacements are characterised by multiple regions of maximal displacement separated by areas of small displacement. In cat, the transition from simple to complex occurs near 3000 Hz; in chinchilla, at a much lower frequency, near 600 Hz. The simple and complex displacement patterns in cat are similar to those reported previously [2, 8].

At high frequencies, the TM displacement patterns show an ordered arrangement, as shown in Figure 2C. These displacements are characterised by many regions of maximal displacement, which appear arranged in concentric rings surrounding the manubrium. Radial patterns of rays can also be seen, especially in chinchilla. In cat, the transition from complex to ordered occurs near 15 000 Hz; in chinchilla, at a much lower frequency, near 4000 Hz. The ordered patterns of rings and rays in these time-averaged holograms are indicative of standing waves on the TM, see Evidence for standing waves on the TM.

Stroboscopic holography: subfringe displacements in cat and chinchilla – complex and ordered displacement patterns

Double-exposure holography with stroboscopic illumination was used to measure displacements of TM with nanometer resolution. This method enables distinction between inward motions and outward motions. Figure 3 and Figure 4 show the difference in TM displacement between the condensation and rare-faction phases for selected sound stimuli.

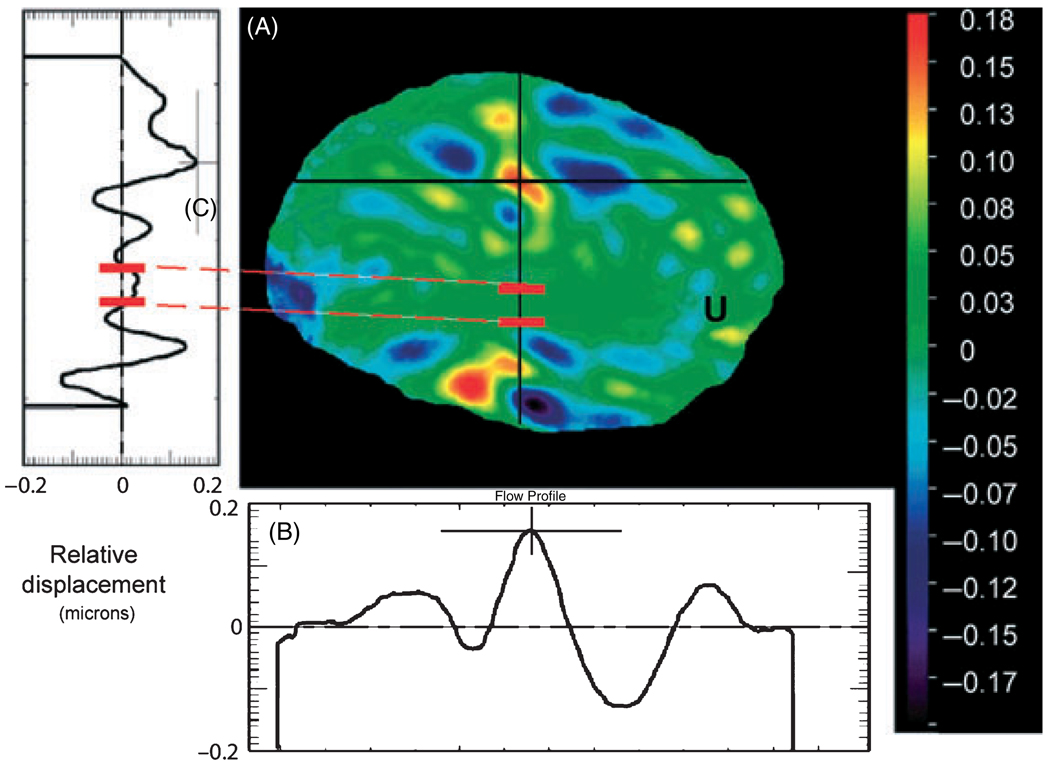
Figure 3.

Full-field-of-view stroboscopic holography measurements in cat at 8776 Hz, 110 dB sound pressure level (SPL), showing a maximum displacement on the order of 350 nm. The vertical contour crosses the manubrium and the umbo is marked by ‘U’

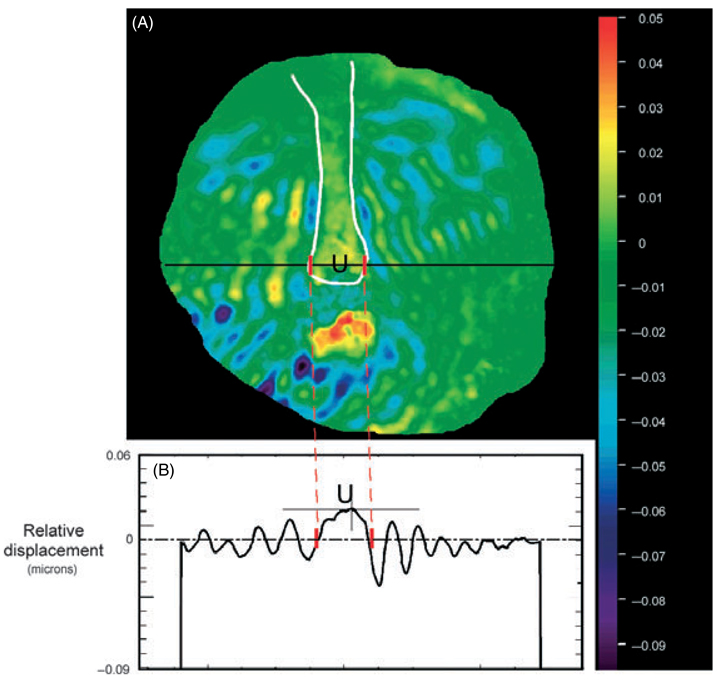
Figure 4.

Full-field-of-view stroboscopic holography measurements in chinchilla at 15 700 Hz, 107 dB sound pressure level (SPL), showing a maximum displacement on the order of 150 nm. The horizontal contour crosses the umbo is marked by ‘U’

The difference in cat TM displacement between the two stimulus phases at 8776 Hz is shown in Figure 3. Multiple positive and negative maxima are apparent across the TM – a complex displacement pattern. Figure 3 demonstrates that regions on the TM undergo motions with directions that are opposite to the direction of motion of the manubrium (shown around bar markers). The peak-to-peak surface displacement is on the order of 350 nm. The biphasic spatial variations shown in Figure 3 are similar to those reported by others [22, 23, 26]. The significance of these observations is yet to be understood.

The difference in chinchilla TM displacement between two stimulus phases at 15 700 Hz is shown in Figure 4 – an ordered displacement pattern. The circumferential arrangement of displacement maxima rings is clear in this example, while the rays are less clear. The circumferential maxima are arranged such that rings of peak positive displacements alternate with rings of peak negative displacements. The patterns are somewhat blurred ventral to the umbo, where a significant positive displacement maximum is surrounded by an arc of negative displacement maxima. Along the horizontal line (8 mm distance) there are >11 cycles of displacement, as seen in Figure 4. The peak-to-peak surface displacement along this horizontal line is on the order of 50 nm.

Automatic mask generation

An important issue in the quantitative analysis of holographic data is to recognise regions of a holographic image that correspond to the moving surface of the TM, where that surface is limited by anatomical constraints (e.g. the size of the TM), and by methodological constraints (e.g. the view of the membrane through the OP as well as the degree of removal of surrounding bone).

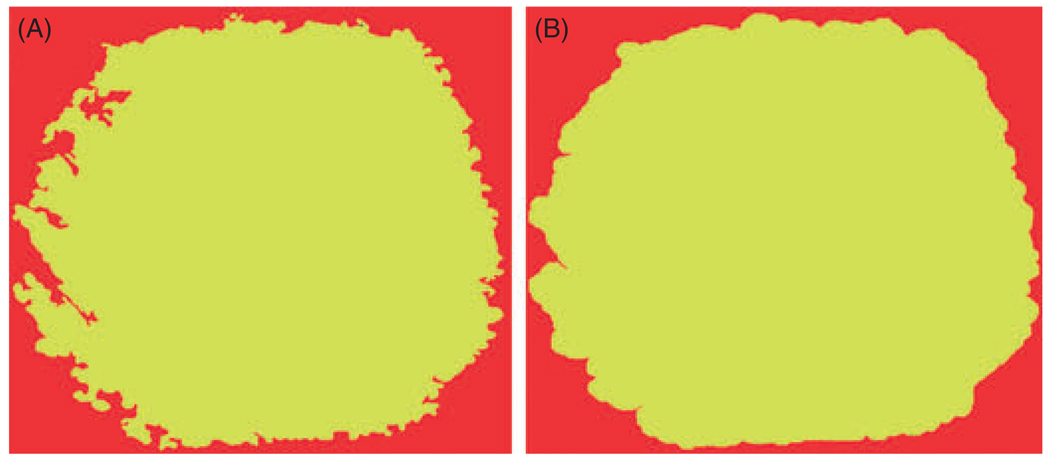
Algorithms have been developed to quantify: (i) the amount of displacement above background noise in an image; (ii) regularities in the gradient of measured displacement; and (iii) local correlations in the coherence of the reflected signal (i.e. intensity modulation). A weighted sum of these extracted features was tested to automatically generate a mask of the moving surface of the chinchilla TM produced by a 2800 Hz, 94 dB SPL stimulus, shown in Figure 5A. Due to the irregular TM boundary, island-filling and spatial smoothing algorithms were applied to produce the mask shown in Figure 5B [27].

Figure 5.

Automatic mask generation applied to data measured in a chinchilla tympanic membrane (TM) at 2800 Hz, 94 dB sound pressure level (SPL) stimulus: (A) mask without edge smoothing; and (B) mask after application of island-filling and spatial smoothing algorithms

The effects of variation in the estimate of the masked area on the determination of volume displacement were quantified by obtaining the volume displacement of the masked area before and after increasing and decreasing the masked area. Small increases or reductions in the masked area produced proportional changes in the estimated volume displacement, such that a change in the masked area of 10% produced a similar percentage change in the estimate of TM volume displacement.

Discussion

Comparison of TM displacements in cat and chinchilla

Differences in TM motion were observed between cat and chinchilla, perhaps related to the fact that the TM in chinchilla is anatomically larger and rounder than in cat. Chinchilla TM displacement patterns change from simple to complex to ordered at lower frequencies than in cat, as shown in Figure 2. The chinchilla TM and umbo deform more than cat for a given sound level, indicating a greater compliance and a greater response to sound. Umbo displacements computed from cat and chinchilla holograms are consistent with previous single-point measurements [22, 24, 25, 28].

Evidence for standing waves on the TM

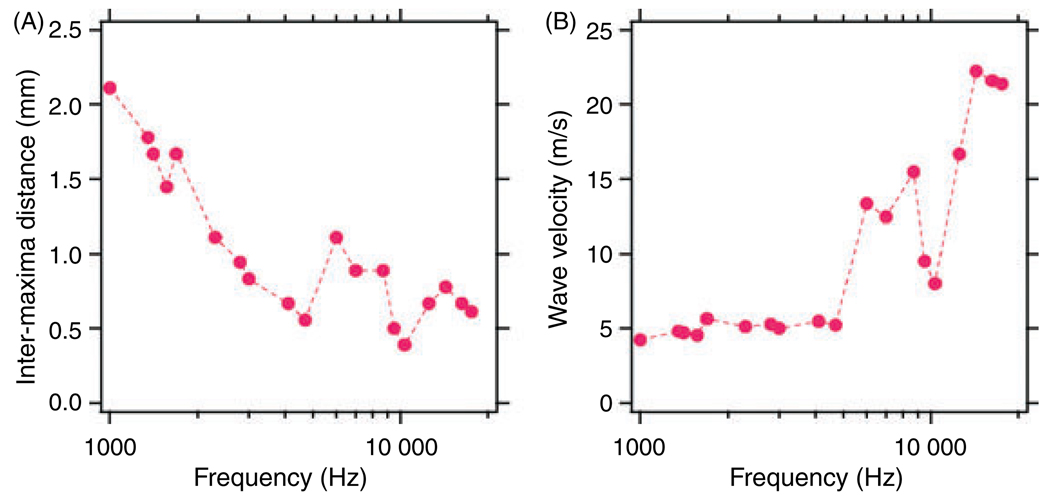
The circumferential ring pattern of displacement maxima in Figure 2 and Figure 4 is consistent with the presence of standing waves on the TM surface. (Any travelling wave-like displacement is averaged out of Figure 2.) Standing waves may result from the interaction of surface waves moving inward from the tympanic ring toward the umbo and outward toward the tympanic ring (possibly reflected at the umbo). The wave speed c_w can be computed from the stimulus frequency f and the spatial separation of the displacement maxima (the distance between + and − magnitude maxima in the stroboscopic measurements) x with

$$c_{\mathrm{W}}=2xf.$$ (14)

Figure 6A shows displacement maxima separation in a chinchilla ear determined from holograms at various frequencies; Figure 6B shows the wave velocity computed from Figure 6A. The computed wave speeds are 5–25 m s⁻¹, within the range computed by Fay et al. [26]. The corresponding delay associated with the 4 mm distance between tympanic ring and umbo varies from 800 µs at low frequencies to 160 µs at high frequencies. These delays are 4–20 times larger than the 0.040 ms middle-ear transmission delays observed in gerbil [29, 30] or predicted for wave motion along the TM of cat [1].

Figure 6.

Separation between displacement maxima in stroboscopic holograms from a chinchilla ear at various frequencies (A); and (B) wave speed computed from data in (A) by using Equation (14)

Summary and Future Works

We are developing a PC based OEH system to obtain measurements of dynamically induced, nanometer scale deformations in the TM. The OEH system, operated in time-averaged and double-exposure modes, has been used to obtain preliminary measurements of TM behaviour in two species in-vitro. Namely, time-averaged holography has been used to determine modal characteristics of the TM in cat and chinchilla, while double-exposure stroboscopic holography has been applied to obtain quantitative full-field-of-view measurements of deformations with nanometer resolution. The results obtained are consistent with measurements reported previously [2, 8, 23] and have been extended, for the first time, to cover higher frequencies and amplitudes of excitation.

Preliminary measurements, to be expanded and validated by future investigations, indicate that TM displacements progress from simple to complex to ordered patterns as excitation frequency increases. The difference in TM displacement pattern transition frequencies between cat and chinchilla is consistent with differences in TM mechanical properties. In addition, the time-averaged and double-exposure stroboscopic measurements in both cat and chinchilla suggest standing waves on the TM at frequencies above 1–4 kHz.

It is stressed that the preliminary measurements presented in this paper were performed to validate and demonstrate the applicability of our, currently under development, OEH system. Measurements, as presented, have already shown behaviour of the TM that had not been observed and quantified previously, specially at high audible frequencies.

Our developments will continue with the miniaturisation of the OEH system and its optical probe. These developments will follow by the application of this new system to test, characterise, and diagnose human TM. Challenges that are expected range from investigations on the fundamentals of laser-to-tissue interaction (to determine optimal characteristics of the laser to use in TM), to improve and develop high-speed digital holographic algorithms (to acquire sufficient holographic data with a minimum number of images in order to account for variations in boundary conditions imposed by physiological changes in live samples), to incorporation of new MEMS and MOEMS systems (to enable the realisation of a miniaturised optical probe that is suitable for use in a medical environment.) Furthermore, the OEH system and its optical probe will be used to attempt answering fundamental questions related to middle-ear mechanics; for instance, the effects of shape and function of the TM across different species, the effects of middle-ear modifications in hearing capabilities, the effects of perforations in the TM, the determination of mechanical properties of the TM and the definition and validation of physical and mechanical models of the TM. Further investigations are in progress and will be reported in future publications.