Evidence of a general 2/3-power law of scaling leaf nitrogen to phosphorus among major plant groups and biomes

Abstract

Scaling relations among plant traits are both cause and consequence of processes at organ-to-ecosystem scales. The relationship between leaf nitrogen and phosphorus is of particular interest, as both elements are essential for plant metabolism; their limited availabilities often constrain plant growth, and general relations between the two have been documented. Herein, we use a comprehensive dataset of more than 9300 observations of approximately 2500 species from 70 countries to examine the scaling of leaf nitrogen to phosphorus within and across taxonomical groups and biomes. Power law exponents derived from log–log scaling relations were near 2/3 for all observations pooled, for angiosperms and gymnosperms globally, and for angiosperms grouped by biomes, major functional groups, orders or families. The uniform 2/3 scaling of leaf nitrogen to leaf phosphorus exists along a parallel continuum of rising nitrogen, phosphorus, specific leaf area, photosynthesis and growth, as predicted by stoichiometric theory which posits that plants with high growth rates require both high allocation of phosphorus-rich RNA and a high metabolic rate to support the energy demands of macromolecular synthesis. The generality of this finding supports the view that this stoichiometric scaling relationship and the mechanisms that underpin it are foundational components of the living world. Additionally, although abundant variance exists within broad constraints, these results also support the idea that surprisingly simple rules regulate leaf form and function in terrestrial ecosystems.

1. Introduction

Scaling relations among plant traits result from and impact on a broad range of ecological and evolutionary processes (Reich et al. 1997; Sterner & Elser 2002; McGroddy et al. 2004; Wright et al. 2004; Kerkhoff et al. 2005; Ågren 2008). Metabolic, chemical and physical leaf traits are often quantitatively related, generating scaling functions between pairs of traits that can be defined by characteristic slopes and intercepts on a log–log scale (Reich et al. 1997; Sterner & Elser 2002; McGroddy et al. 2004; Wright et al. 2004; Kerkhoff et al. 2005), based on the general scaling relationship Y = β X^α, where X and Y represent two functional traits of leaves and β and α are, respectively, the elevation and slope of the log-transformed Y versus X regression curve. The observation that such trait-based relationships sometimes are conserved (i.e. consistent or invariant) across ecosystems and biomes that differ dramatically (Reich et al. 1997; Sterner & Elser 2002; Wright et al. 2004) implies the existence of fundamental axes of differentiation in plant strategies and/or convergent scaling owing to biophysical constraints and evolutionary selection (Reich et al. 1997; Sterner & Elser 2002; McGroddy et al. 2004; Wright et al. 2004; Kerkhoff et al. 2005). The leaf nitrogen (N_L) to phosphorus (P_L) relationship is of particular interest, as both elements are essential for metabolic reactions involved in light capture, photosynthetic capacity and growth, and their restricted availabilities often act to limit plant carbon acquisition and growth (Ericsson & Ingestad 1988; Reich et al. 1997; Sterner & Elser 2002; Ågren 2004, 2008; McGroddy et al. 2004; Wright et al. 2004; Kerkhoff et al. 2005; Niklas & Cobb 2005; Elser et al. 2007). Plant investment in N_L relative to P_L varies with differences in both physiological growth strategies among species and relative N versus P limitation across local to global scale soil environments (Walker & Syers 1976; Vitousek 1984; Ericsson & Ingestad 1988; Chadwick et al. 1999; Westoby et al. 2002; Güsewell 2004; Reich & Oleksyn 2004; Kerkhoff et al. 2005; Niklas 2006; Lovelock et al. 2007; Townsend et al. 2007; Ågren 2008; Lambers et al. 2008).

While it has been suggested that the strategic allocation of N_L and P_L in plant tissue may follow fundamental stoichiometric rules (Sterner & Elser 2002; Kerkhoff et al. 2005, 2006; Niklas et al. 2005; Niklas 2006; Ågren 2008), our quantitative understanding of how these two nutrients are coupled in leaf tissue across biomes and taxonomic composition variation remains limited despite recent advances (McGroddy et al. 2004; Reich & Oleksyn 2004; Han et al. 2005; Kerkhoff et al. 2005, 2006; Lovelock et al. 2007; Townsend et al. 2007; Watanabe et al. 2007). Prior work (Sterner & Elser 2002; McGroddy et al. 2004; Wright et al. 2004) finding generally similar N_L versus P_L scaling relations in independently evolved lineages and different biomes has led to suggestions that evolutionary history and degree of environmental convergence have led to a set of rules that generally modulate the stoichiometry of nutrients in plant organs. However, prior studies have shown some statistically significant differences in the N_L versus P_L scaling slopes between woody and herbaceous taxa, as well as differences in scaling slopes rooted in phylogenetic history (Kerkhoff et al. 2006). Moreover, N_L versus P_L scaling relations with slopes of 0.75, 0.67, 0.67, 0.72 and 0.70 have been reported in studies with 131 (Niklas et al. 2005), 745 (Han et al. 2005), 1176 (Wright et al. 2004), 1287 (Kerkhoff et al. 2006) and 3873 (Reich & Oleksyn 2004) observations, respectively, with significant differences sometimes noted across taxonomic groups or geographical locations. Here, we use a larger and more comprehensive dataset (more than 9300 observations) to ask whether these previously reported differences are systematic among major taxonomic groups and/or biomes or whether a single N_L versus P_L scaling fit is common, and moreover whether results generally can be reconciled with theories about N versus P scaling.

Given the important role of N in proteins, most particularly Rubisco that drives photosynthesis, and of P in rRNA needed to generate and maintain protein levels vital to cell growth and metabolism, these two elements have been linked in several stoichiometric growth models (Elser et al. 2000, 2003; Ågren 2004; Niklas et al. 2005). These models encompass what hereafter we will call the growth rate hypothesis (GRH) (Elser et al. 2000, 2003), stating that plants with greater metabolic and growth rates are disproportionately more P-rich than N-rich because of allocational shifts in favour of P-rich rRNA required to support the elevated protein synthesis demands of rapid metabolism and growth. Such models have been used to predict growth rate differences when stoichiometric scaling between N and P is assumed to follow a 3/4-power function (Niklas et al. 2005; Niklas 2006).

While such efforts provide a stoichiometric framework linking the subcellular ‘machinery’ of protein/ribosomal metabolism to the observed growth patterns of multicellular organisms (Elser et al. 2000, 2003; Ågren 2004; Niklas et al. 2005), they have not yet fully resolved strategic differences in N_L–P_L scaling in plants as a function of local environments, growth strategies, climate variation or taxonomic grouping. Kerkhoff et al. (2005) suggested that the GRH could explain the declining N : P ratios observed from equatorial to high latitude regions based on abbreviated polar growing seasons. However, alternative (and possibly complementary) hypotheses involving patterns of soil substrate age and how these vary at continental and biome scales (Walker & Syers 1976; Vitousek 1984; Chadwick et al. 1999; Reich & Oleksyn 2004) are also consistent with empirical evidence. Moreover, none of these previous studies comprehensively tested whether N_L versus P_L scaling varies among biomes, as would be predicted by the soil substrate age hypothesis but not by the GRH.

Herein, we address two important remaining questions. First is the N_L–P_L scaling relationship common across major plant groups, taxa or biomes? That is, despite localized and idiosyncratic sources of environmental variation (Ågren 2008), are N_L versus P_L scaling slopes conserved across major sources of variation? Second, what is the slope of that relationship and how does it relate to theoretical predictions?

2. Material and methods

In this report, we use a leaf trait dataset (540 sources; table 1 in the electronic supplementary material) compiled from published studies that include 9356 paired observations of N_L and P_L from a total of approximately 2500 species from 70 countries through six continents, with associated specific leaf area (SLA) and mass-based net photosynthetic capacity (A_max) data available for many of these. The dataset has been contributed as part of a new international ecological compendium of databases (TRY) and is open to all users (http://www.try-db.org/). These observations are an expanded version of an earlier dataset (Reich & Oleksyn 2004).

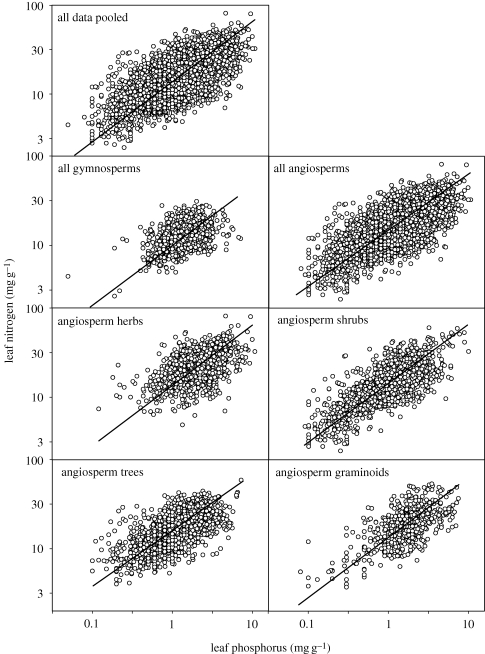
We compared data for plants grouped by phylogeny (angiosperm, gymnosperm), for four functional groups within the angiosperms, for orders and families within the angiosperms, and for three different biomes within the angiosperms. These choices largely reflect practical considerations. The contrasts of angiosperm versus gymnosperm, or of the four functional groups of different life forms (graminoid, herb, shrub and tree) within the angiosperms, were selected a priori because, among the studied taxa, they represented the simplest divisions based on important aspects such as phylogeny and life form that also resulted in sufficient sample sizes. The dataset included information for 115 gymnosperm species and 2441 angiosperm species. As is common in cross-species analyses, we used log₁₀ data to normalize the distributions and minimize patterns in residuals (Reich et al. 1997; Reich & Oleksyn 2004).

Given 6466 observations from 2441 species, the angiosperm database is largely dominated by among-species variation, with only an average of two to three replicates per species. Given this, it is not surprising that relationships based on species averages for angiosperms were similar to those resulting from analyses of all observations, which are those we report in this paper. For instance, using average values for all 2441 angiosperm species, the relationship of log N to log P had r² = 0.54, slope of 0.64, intercept of 1.17 and the range of 95% CI from 0.62 to 0.66—all very similar to relationships for all angiosperm observations (table 1). By contrast, the gymnosperm observations (n = 2890) are from 115 species, with nine species making up approximately equal to 2200 of those observations. Hence, for gymnosperms, the data largely reflect within-species variation, and we did not further subdivide the data as we did for angiosperms. The biomes were defined according to temperature- and precipitation-based biome classifications and on the descriptions provided by the authors of the original publications. Not all observations had sufficient information to be placed into a biome category. Biomes included moist tropical (including subtropical), Mediterranean, temperate (including boreal), desert and dry tropical. Sufficient data were available only to evaluate relationships for the first three. Moist tropical, Mediterranean and temperate data were obtained from 26, 10 and 43 countries, respectively. We did not use data for fertilized plants, planted, urban or polluted sites.

Table 1.

Scaling of leaf nitrogen concentration N_L in relation to leaf phosphorus concentration P_L for all data pooled, for plants grouped by phylogeny (angiosperm, gymnosperm), by four functional groups within the angiosperms and by three different biomes within the angiosperms. (All relations were significant (p < 0.0001). All equations were fit using the log–log version of the equation: Y = β X^α. Reduced major axis intercepts and slopes (exponents) are shown, as well as the lower and upper 95% CI of the exponent, and r². n, the number of observations, i.e. unique species-site combinations with data for N_L and P_L obtained from same individuals. Significant differences (p < 0.05) in exponents among groups (for appropriate contrasts separated by blank lines) are shown by the lack of shared letters. Intercepts were not standardized to a common slope, and thus are not contrasted among groups. Biomes were broadly defined, such that temperate includes temperate and boreal; and moist tropical is both wet and moist tropical and subtropical.)

plant group	n	intercept	exponent	low CI	high CI	r2
all	9356	1.113	0.676	0.658	0.694	0.37
divisions
angiosperm	6466	1.166	0.637 a	0.621	0.653	0.48
gymnosperm	2890	1.002	0.696 a	0.650	0.746	0.22
angiosperm functional groups
graminoid	699	1.105	0.688 a	0.631	0.751	0.42
forb	1072	1.127	0.664 a	0.595	0.742	0.23
shrub	1518	1.155	0.652 a	0.624	0.682	0.56
trees	2878	1.195	0.633 a	0.610	0.658	0.48
biomes
temperate	3147	1.134	0.686 a	0.641	0.734	0.21
Mediterranean	714	1.143	0.655 a	0.623	0.689	0.68
moist tropical	1866	1.203	0.651 a	0.614	0.690	0.38

A subset of these observations also included data on other leaf traits such as SLA and net photosynthetic capacity. Photosynthetic capacity is defined as the maximum photosynthetic rate per unit leaf mass measured under ambient CO₂ concentrations and saturating irradiance (Reich et al. 1997), and operationally measured in the field in mid-morning under optimal moisture and temperature conditions. Here, SLA is defined as the one-sided projected area of foliage per unit dry mass (Reich et al. 1997).

We used standardized major axis regression (Falster et al. 2003; Warton et al. 2006) to compare scaling exponents within and among plant groups and biomes. We also used ‘funnel’ graph analyses to evaluate the dependence of the observed scaling slopes on sample size (Palmer 1999; Wright et al. 2005). The strength of bivariate trait relationships was quantified with standard correlation and ordinary least-squares regression statistics in conjunction with standardized major axis slopes (also known as reduced major axis, or reduced major axis (RMA) slopes). A standardized major axis fit is the line minimizing sums of squares in X and Y dimensions simultaneously; and these routines were run using the SMTR computer package (Falster et al. 2003). In this program, heterogeneity between RMA slopes is tested via a permutation test. Where deemed non-heterogeneous, a common RMA slope is estimated using a likelihood-ratio method and differences in elevations (i.e. the intercepts) are then tested.

3. Results and discussion

Among all observations, N_L varied 32-fold and P_L by 200-fold, with the mean (10th, 90th percentiles) of N_L and P_L being 17.1 mg g⁻¹ (9.3, 27.0) and 1.57 mg g⁻¹ (0.6, 2.7). For all species and sites pooled (n = 9356), N_L increased with P_L (p < 0.001, r² = 0.37; figure 1 and table 1), with the scaling exponent = 0.676 (95% CI, 0.658–0.694). We found strong support for the hypothesis that the N_L versus P_L scaling slope is conserved across the two major taxonomic plant groups, despite the relationship being of modest strength. For both angiosperms and gymnosperms, the scaling exponents were close to 2/3 (table 1). Given the greater sampling of angiosperms across a wider range of species, functional groups and biomes than for gymnosperms, and the dominance (75% of all observations) of nine species within the gymnosperm data, we focus on angiosperms hereafter. Among the four major functional groups within the angiosperms, the N_L versus P_L scaling exponents were again close to 2/3, with a narrow range of scaling exponents—from 0.63 in trees to 0.70 in graminoids. The 95% CI of slopes included the 2/3 value for all data pooled, within divisions, or within angiosperm functional groups. There was also similar N_L versus P_L scaling across biomes (table 1), with the scaling exponent very near to 2/3 in temperate, Mediterranean and tropical biomes.

Figure 1.

Relationships of leaf N (N_L) to leaf P (P_L) for all data pooled, and for plants grouped by both phylogeny (angiosperm, gymnosperm) and life form within the angiosperm group. The details of these relations using reduced major axis (RMA) regressions are presented in table 1.

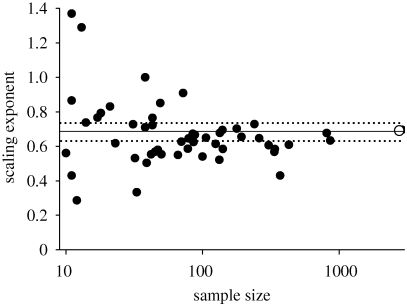
To assess whether N_L versus P_L scaling is similar at narrower phylogenetic scales, we focussed at the order and family levels. There are 75 angiosperm orders represented in the dataset. The mean of the N_L versus P_L RMA slope for the 50 orders with a sample size greater than 10 was again close to 2/3 (figure 2). The distribution of the RMA slopes among orders was broad (similar to a prior report of phylogenetic variation in this slope; Kerkhoff et al. 2006); however, a ‘funnel plot’ assessment of the slope in relation to the sample size indicates that much of the variation in the RMA slope results from low sample size (figure 2). As the number of observations per order increases, the slopes converge around the mean (approx. equal to 2/3).

Figure 2.

Relationship between the scaling exponent (the RMA slope) of the N_L–P_L relationship and the sample size, for 50 angiosperm orders (closed circles). The mean (0.667) and 95% CI for the scaling exponent are shown in the solid and dashed lines. The mean for the gymnosperm order ‘Pinales’ is shown in the open circle for comparison.

Although it is hypothetically possible that changes in the heterogeneity of slope with sample size represent increasingly greater convergence in N_L versus P_L scaling in orders with larger numbers of species represented in the dataset (as if, for example, more species occurred in more recently diverged lineages, and N_L versus P_L scaling also converged over time), we argue that a statistical explanation is the most parsimonious. The data support three straightforward predictions regarding how analyses of unbiased data should vary with sample size (Palmer 1999). As sample size decreases, the variation about the ‘true’ effect (in this case, the scaling slope) should increase owing to increased sampling error; figure 2 shows this to be true. Second, the average scaling slope should be independent of sample size: this was also true (data not shown); and third, regardless of sample size, individual slopes should exhibit a normal distribution about the true mean slope, which was true (p < 0.0001).

Hence, the average of RMA slopes of N_L versus P_L relations within individual angiosperm orders is similar to the slope of all taxa pooled or among all angiosperms within any biome or life form, and these values are quite close once sample sizes become sufficient. Similar results were found in examining frequency distribution and funnel plots of N_L versus P_L scaling relations among the 62 angiosperm families in the dataset (data not shown). Given the strong convergence in scaling slopes across biomes, major taxonomic and functional groups, and among orders and families when sampling is robust, it is likely that some fraction of reported variance in slopes among biomes, studies or plant groups in earlier publications probably arose from relatively small sample sizes.

Now, we evaluate whether the GRH prediction of consistent allometric (rather than isometric) scaling of N_L–P_L is consistent with greater scaling exponents for P_L than N_L in relation to growth rate surrogates SLA and A_max (Lambers & Poorter 1992; Nielsen et al. 1996; Reich & Oleksyn 2004). This prediction is based on the reasoning that plants with high growth rates will require both an increased allocation of P-rich RNA (Elser et al. 2003) and an increased metabolic rate to support the energy demands of macromolecular (protein, rRNA) synthesis. SLA and A_max are appropriate surrogates for growth rate as both have been shown to correlate with total plant growth rate (Lambers & Poorter 1992; Nielsen et al. 1996; Reich et al. 1997) and by definition are positively related to energy capture (light interception and CO₂ fixation, respectively) per unit leaf mass.

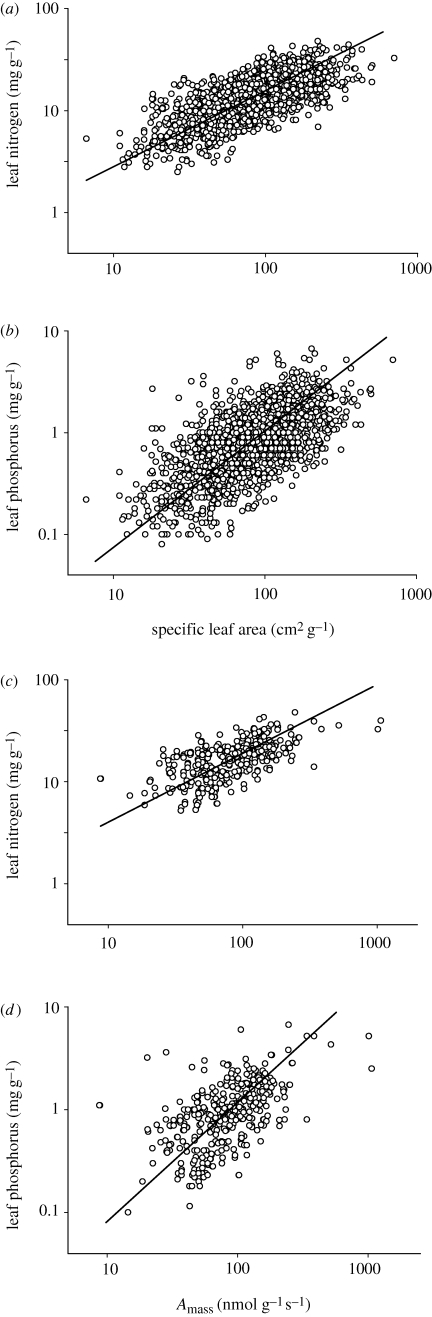
The relationships are consistent with the line of reasoning derived from the GRH, as for all angiosperms (figure 3), or for divisions of the data sorted by functional groups or by biomes (table 2), the scaling of P_L to SLA always had a greater slope than the scaling of N_L to SLA, usually by approximately equal to 0.4 units. Similarly, higher slopes of P_L to photosynthetic capacity, than N_L to photosynthetic capacity, were also observed (figure 3). These slope differences (for N_L and P_L in relation to the growth rate surrogates) translate to the 2/3 scaling of N_L–P_L observed within and among taxa and biomes (table 1). It is notable though that this dataset includes differences in N_L, P_L and growth rate that result both from species differences (e.g. intrinsically fast versus slow-growing taxa) and from site differences (e.g. soils relatively poorer in P or N), but cannot differentiate among these.

Figure 3.

Relationship of leaf N (N_L) and leaf P (P_L) to specific leaf area and net photosynthetic capacity (A_mass) for all angiosperm data pooled. (a) For leaf N (N_L) and (b) leaf P (P_L), the relationships to specific leaf area (SLA) were log N_L = −0.326 + 0.759 (log SLA); n = 1819, r² = 0.54, p < 0.0001; and log P_L = −2.28 + 1.141 (log SLA); n = 1819, r² = 0.45; p < 0.0001. Statistics for specific leaf area relations for subgroups and biomes are listed in table 2. (c) For leaf N (N_L) and (d) leaf P (P_L), the relationships to net photosynthetic capacity were log N_L = −0.090 + 0.681 (log A_mass); n = 391, r² = 0.37, p < 0.0001; and log P_L = −2.21 + 1.131 (log A_mass); n = 391, r² = 0.30; p < 0.0001.

Table 2.

Scaling relationships for angiosperms of leaf nitrogen concentration N_L and leaf phosphorus concentration P_L in relation to SLA for all data pooled, for plants grouped by life form and for three biomes. (All relations were significant (p < 0.0001). All equations were fit using the log–log version of the equation: Y = β X^α. Reduced major axis slopes (exponents) are shown, as well as the lower and upper 95% CI of the exponent, and r². n, number of observations. Significant differences (p < 0.05) in exponents among groups (for appropriate contrasts separated by blank lines) are shown by the lack of shared letters.)

		NL			PL
plant group	n	exponent	low CI, high CI	r2	exponent	low CI, high CI	r2
all	1819	0.76	0.73, 0.79	0.54	1.14	1.08, 1.12	0.45
angiosperm functional groups
graminoid	34	0.59 a	0.41, 0.85	0.52	1.17 a	0.81, 1.69	0.51
forb	22	0.72 a	0.33, 1.56	0.34	1.01		ns
shrub	535	0.83 a	0.76, 0.89	0.55	1.19 a	1.08, 1.31	0.45
trees	1112	0.75 a	0.70, 0.80	0.45	1.17 a	1.09, 1.26	0.39
biomes
temperate	449	0.67 a	0.59, 0.76	0.35	0.95 a	0.80, 1.12	0.24
Mediterranean	321	0.93 b	0.83, 1.03	0.54	1.41 b	1.26, 1.58	0.49
moist tropical	950	0.78 a	0.71, 0.85	0.34	1.21 ab	1.08, 1.35	0.24

These analyses suggest that the scaling of N_L–P_L is best described as a 2/3-power law function, long ago proposed for metabolic scaling as a result of area-to-volume allometry. The N_L–P_L scaling is closely associated with different relations of mass-based nutrient concentrations (i.e. N_L, P_L) to SLA, which by definition therefore involve contrasting area–mass relations. Light harvesting and gas fluxes can be considered area-based phenomena that impact the economics of investments of elements (C, N and P) quantified per unit mass. Given that P-rich rRNA is critical to the maintenance of protein pools (econometrically quantified on a mass basis) that in turn influence the rate at which harvested light (for which SLA is a useful surrogate) is used to fix diffused CO₂, the coupling of area-to-mass processes may play important roles in controlling the scaling slope, consistent with the recent scaling theory regarding hydraulic and mechanical constraints on leaf architecture (Niklas et al. 2009).

4. Summary

Our results show that N_L versus P_L power law scaling averages approximately equal to 2/3 in angiosperms and gymnosperms, and also averages approximately equal to 2/3 for angiosperms whether examined for closely related (families or orders) or distantly related (all angiosperms) taxa, among four different life forms, or in differing biomes. Moreover, scaling slopes converged around the 2/3 value whenever sample sizes were large. The generality of this finding supports the view that this stoichiometric relationship and the mechanisms that underpin it are foundational components of the living world (Elser et al. 2000, 2003; Ågren 2004; Kerkhoff et al. 2006). Additionally, although abundant variance exists within the broad constraints, these results also support the idea that surprisingly simple rules govern leaf form and function in all corners of the terrestrial world.