Sensitivity of Hemoglobin Concentration on Optical Probe Positioning in Image-guided Near Infrared Spectroscopy

Abstract

Multi-modality image-guided near infrared spectroscopy provides volume-based quantification of total hemoglobin, oxygen saturation, water and scatter in various tissue types in-vivo. The accuracy of these parameters depends on the location of the imaging probe and its distance from the tumor. In a numerical study, we have performed simulation to analyze this effect in a breast-specific imaging domain. Results show that the accuracy of total hemoglobin decreases by 25% for every centimeter away from the tumor center, for a typical size of 35mm cancer in the breast. Image guidance is necessary for accurate positioning, and multi-plane acquisition can improve this accuracy.

I. Introduction

Image-guided optical systems combining conventional techniques such as MRI, CT and ultrasound with near-infra red (NIR) optical techniques make high-resolution functional imaging possible with intrinsic contrast mechanisms. In this new generation of multi-modality optical-based systems[1-4], the anatomical structure from traditional imaging is incorporated into the ill-posed optical image reconstruction. This inclusion of priors makes the recovery of optical estimates better posed, more robust and accurate. When the tissue boundaries are enforced strictly (termed ‘hard priors’[5]) with assumption of piece-wise constant homogeneous tissue types, the method is akin to region-based volume spectroscopy. This type of image-guided spectroscopy provides the bulk estimates of various tissue types than can be delineated from the traditional imaging exams. For example, in a typical breast, an MR scan can provide a spatial template of adipose and fibro-glandular tissues. In an abnormal breast, this may be extended to cystic and/or malignant tumor tissues. Image-guided NIR spectroscopy measured through multiple source-detector geometry allows deep tissue penetration and provides total hemoglobin concentration, oxygen saturation, water and scatter estimates of each of the tissues. This has been used to study breast tissue in-vivo geared towards cancer diagnosis as well as monitoring neoadjuvant chemotherapy[5, 6]. Recent experience in this multi-modality imaging has indicated that the positioning of the optical probe is likely to influence the recovered chromophore concentrations and hence, estimates of total hemoglobin (HbT). This is due to the non-linear inverse problem as well as exponential attenuation of light signal. In this paper, we endeavor to study the effect of the measurement probe position on the reconstructed hemoglobin of the tumor. A numerical study was performed on a typical breast shape obtained from patient MRI, by varying the imaging plane as a function of distance from the center of the tumor. The recovered estimates indicate that guidance for alignment of NIR probe or multi-plane acquisition will be key for accurate spectroscopy.

II. Methods

A. Patient-Specific Geometry

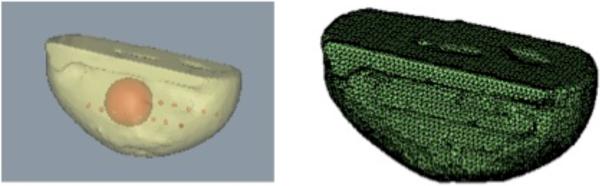
T1-weighted MRI obtained from a subject diagnosed with infiltrating ductal carcinoma and undergoing neoadjuvant chemotherapy was used for this study. The MRI volume was segmented to obtain the outer breast shape as shown in Figure 1(a) using a commercial software package (Mimics™). Using the breast shape as background, a spherical inclusion 35mm in diameter was created in the center to mimic a tumor. This patient-specific geometry was used for the simulation study using a boundary element method (BEM) for light propagation in tissue. The optical fiber probe in the clinical setting consists of 16 sources with 15 detectors per source in a slab geometry [1]. This measurement setup was used in the simulations.

Fig. 1.

(a) Imaging domain used in the study is shown. The outer shape was segmented from T1-weighted MR volume, with a spherical inclusion of size 35 mm simulated in the center of the domain. Red markers show the locations of the source-detector fibers. (b) Surface mesh for the outer shape used in the forward model is shown. The forward mesh contained surfaces for the outer as well as inner inclusion with a total of 7136 nodes and 14264 triangles. The reconstruction mesh contained a total of 4260 nodes and 8512 triangles.

B. BEM Multi-spectral Spectroscopic Recovery

A boundary element method (BEM) was used to numerically model the diffusion equation for light propagation in tissue [7]. This method is specific to multi-modality imaging and allows for direct incorporation of the tissue boundaries from MRI into the optical recovery. The strength of the method is that BEM requires discretization of the domain surface only. This results in more accurate and reliably discretized domains and a smaller system of equations. Details of the implementation of this method can be found elsewhere [8, 9]. Briefly, this consists of using Green's identities and fundamental solutions to describe the diffusion equation in 3-D using boundary formulation. The forward model solves for the light fluence in the domain and intensity and phase measurements at the boundary given optical properties for different homogeneous tissue layers. The corresponding inverse problem reconstructs for the optical properties concentrations in each of the tissue layers given boundary measurements. Multi-spectral constraints allow for direct recovery of chromophore concentrations and scatter estimates with a substantial reduction in cross talk [10, 11].

The surface mesh for the forward model used in the simulation study is shown in Figure 1(b). The red markers in Figure 1(a) show the locations of the source-detector fibers. The plane represented by the fibers was successively moved from the plane containing the center of the tumor, towards the nipple in spacing of 2.5 mm. Two different levels of contrast for the tumor versus background were simulated based on typical values found in the breast. 5% random Gaussian Noise was added to simulated boundary measurements to mimic experimental setting. A modified Newton's method [12] was used to iteratively reconstruct for estimates of oxyhemoglobin, deoxyhemoglobin, water and scatter in the background and tumor tissues. The convergence criterion for the iterative procedure was when the change in least squares norm of difference between measured and model data was less than 2% between successive iterations.

III. Results

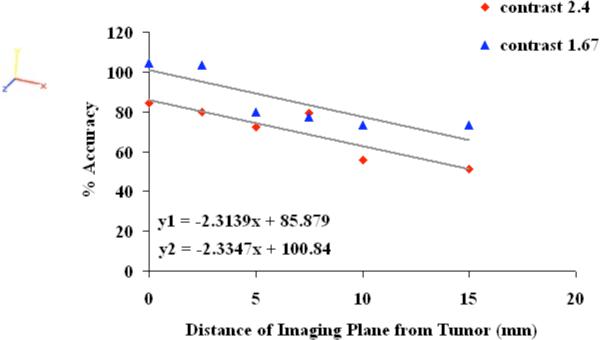
The percent accuracy in total hemoglobin (HbT) recovered in the tumor is plotted as a function of distance in Figure 2 for the two levels of contrast explored in this study. One set of simulations with distance was carried out for contrast of 2.4 between tumor HbT and background; and the other had contrast of 1.67. The percent accuracy was defined as recovered HbT in tumor divided by true HbT in tumor. All simulations had an increase of 30% in water content of tumor versus background as well as contrast in scatter. The focus of this study is to explore errors in HbT, hence the error in recovered water and scattering are not analyzed here. Oxygen saturation showed an error of up to 5% with distance. The results show that the accuracy of recovered HbT decreases linearly with distance; the slope of which may be independent of contrast. The value of the slope (2.3) shows that for every 4 mm distance away from the center of the tumor, the accuracy drops by ~10%. The accuracy was lower for the higher contrast of 2.4, as compared to the 1.67 contrast study. This indicates that there may be a threshold in recoverable contrast between tumor and background. The background HbT showed a variation of 20% with change in distance with higher error further away from the tumor.

Fig. 2.

The percent accuracy of recovered total hemoglobin in the tumor is shown as a function of distance of the optical measurement probe from the plane containing the center of the tumor; for two different levels of contrast. The accuracy decreases linearly with distance at a rate of 2.3% per millimeter.

When two planes of measurements (at 5 mm and 10 mm from the center of the tumor) were used for the study with higher contrast, the accuracy increased to 64%. This is higher than using plane at 10 mm alone (55.5%). Similarly, use of 2 planes at 0mm and 5mm increased the accuracy to 83%, higher than use of 5 mm alone (72.3%). This illustrates the improvement in recovered HbT quantification with multi-plane acquisition.

IV. Conclusions

The numerical simulation study allowed us to model the effect of the imaging plane position on the recovered HbT quantification. Results on a breast domain containing a 35 mm inclusion for two different levels of contrast showed that the accuracy of the HbT decreased by ~25% for every centimeter error in positioning of the optical fibers. This illustrates the need for accurate positioning of the optical probe. The study also showed that multiple plane acquisition reduces the error in the quantification. Efforts are underway to build a probe that can acquire measurements at different planes from the breast.