An evaluation of the Bausch & Lomb Zywave aberrometer

Abstract

Purpose

The Bausch & Lomb Zywave uses Shack-Hartmann aberrometry to determine wavefront aberrations of the human eye and provide an estimate of refractive error. We investigated the effect of pupil size on the repeatability and validity of refractive errors estimated by the Zywave and the repeatability of higher-order aberrations.

Methods

Twenty-three subjects were measured with the Zywave under natural and cycloplegic conditions on two occasions separated by at least one week. Refractive error was also measured using a Nidek ARK-700A autorefractor. At one visit, a cycloplegic subjective refraction was performed. Measured ocular wavefront aberrations were expressed as the polynomial coefficients from a least-squares fitted fifth-order Zernike polynomial expansion over three, five and seven millimetre diameters. Repeatability and validity were evaluated by calculating the difference between pairs of refractive estimates or Zernike terms, determining the mean and standard deviation of these differences and calculating the 95% limits of agreement (LoA = mean ± 1.96 × SD).

Results

The repeatability of refractive error estimated by the Zywave was better than that of the Nidek autorefractor for both manifest and cycloplegic conditions. Manipulating the pupil size on the Zywave from three to seven millimetres changed the mean cycloplegic spherical equivalent from −1.91 D to −2.60 D, a shift that was negatively correlated with spherical aberration. As expected, the magnitude of the Zernike coefficients increased with increasing pupil diameter, as did their corresponding 95% LoA. The 95% LoA decreased for higher-order terms but the magnitude of the terms and the variation between subjects also decreased with increasing order. To compensate for these factors, the ratio of the SD between sessions to the SD across subjects was calculated. The ratios were lowest for second-order terms (less than 0.08 for 7.0mm pupil), intermediate for the C4,0 spherical aberration term (0.14) and third-order terms (~0.25) but approached and exceeded 1.0 for many fourth-and fifth-order terms.

Conclusions

The Zywave provides valid and repeatable estimates of refractive error. We attribute the myopic shift for larger pupils to the eye’s spherical aberration. The repeatability of the Zernike terms measured with the Zywave was acceptable for the second-order and spherical aberration terms but for other higher-order terms, the variation between sessions may exceed the variation between subjects indicating unacceptable repeatability. This may have important ramifications for wavefront-guided LASIK.

Liang and colleagues¹ were the first to use a Shack-Hartmann aberrometer on human eyes and mathematically explain the wavefront maps to the fourth-order Zernike terms from the local derivatives of the centroid patterns.² The laboratory Shack-Hartmann aberrometer of Liang and colleagues¹ provided adequate measurements of higher-order aberrations described by Zernike terms and conventional refractive error measurements. In the subsequent decade, Shack-Hartmann wavefront measurement has been used extensively in the clinical setting to measure ocular aberrations in keratoconus, dry eye, before and after refractive surgery and cataract.^3,4

Bausch & Lomb Zywave

The Zywave (Bausch & Lomb Zywave, Rochester, NY) is a Shack-Hartmann aberrometer used to measure ocular aberrations.² The Zywave focuses an infrared laser beam of 785nm on the retina, which serves as a point source for light propagated out of the eye. The Zywave provides an adjustable optical system to compensate for patient’s refractive errors and adjusts for the subject’s far point by fogging the image to control for accommodation. As a wavefront is propagated back out of the eye, a lenslet array in the Zywave that is conjugate with the pupil plane focuses the wavefront into a 76-point centroid pattern on a CCD detector. The spatial displacement of each centroid from its ideal location is used to determine the slope of the aberrated wavefront. The shape of the wavefront is determined by integration of the slopes for each location in the pupil plane. The Zywave uses Zernike terms to fit the slope data and the coefficients can be used to mathematically reconstruct the wavefront. The Zywave measures total wavefront aberrations and mathematically represents them up to the fifth-order Zernike terms. Each Zernike term represents a specific aberration with a specific mathematical definition and predetermined shape.⁵ Each Zernike term has a corresponding coefficient that represents the magnitude of the wavefront shape in microns and is a quantitative measure that represents how much of that aberration is present.

The Zywave can give an estimate of refractive error, representing the second-order aberrations of the eye. The Zywave was designed to measure refractive errors over a range of +6.00 to −12.00 DS and up to 5.00 D of cylinder. The Zywave is part of the Zyoptix Diagnostic Workstation (along with the Orbscan IIz corneal topographer) for wavefront-guided refractive surgery.

Previous studies of the Zywave have paid little attention to the effect of pupil size and control of accommodation on the repeatability and validity of estimates of refractive error and Zernike terms. The goals of this study were:

• To determine the repeatability and validity of the refractive error measured by the Zywave under manifest and cycloplegic conditions for a range of pupil sizes (three, five and seven millimetres plus the instrument’s default) and in comparison to a conventional autorefractor

• To determine the repeatability of higher-order aberrations under cycloplegic conditions, specified as Zernike coefficients, measured by the Zywave for a range of pupil sizes (three, five and seven millimetres) and compared with the range of values found across subjects.

METHODS

The tenets of the Declaration of Helsinki were followed and all procedures were approved by the Office of Research Risks and Protection at The Ohio State University. Written informed consent was obtained from each subject prior to any procedures being performed. Subjects were recruited from The Ohio State University College of Optometry. Subjects were required to be at least 18years of age and have correctable visual acuity of at least 6/6 in the right eye. Contact lens wearers and patients with any ocular disease or pathology that could affect vision, for example, keratoconus or cataract, were excluded.

Patients attended for two visits separated by at least one week. On each occasion, three refractive error measurements were taken of the right eye with the Zywave (Bausch & Lomb, Rochester, NY) and a Nidek ARK–700A autorefractor (Nidek, Fremont, CA). One drop of 1% tropicamide was instilled into the subject’s right eye and 30 minutes later, the Zywave and autorefractor measurements were repeated. Zywave measurements were taken immediately after a blink to limit tear film disruption. On one of the two visits, the subject’s refractive error was determined using standard subjective techniques under cycloplegia by one of two authors (MDT or MAB). No artificial pupil was used. All measurements were referenced to the spectacle plane.

The Zywave Version 3.21 software also allows manipulation of the pupil diameter by limiting the area of the wavefront used. Among the data provided is an estimate of the subject’s refractive error. Refractive error was determined for the default pupil size of 3.5mm for both manifest and cycloplegic measurements along with pupil diameters of three, five and seven millimetres under cycloplegia. The Zywave software also calculates complete wavefront aberration data in Zernike terms. These data were determined and exported for pupil diameters of three, five and seven millimetres under cycloplegia. The exported data are normalised Zernike polynomial coefficients but do not strictly conform to OSA, ANSI, and ISO standards. Specifically, the Zywave uses the opposite reference for the direction of wavefront propagation, where positive propagation is toward the eye. As a consequence, the sign of the coefficients was reversed and the preferred notation used (Z_n^m for the polynomial and C_n^m for the corresponding coefficient).⁶

Data analysis

Refractive error data from the Zywave and autorefractor were converted to the Fourier components M, J₀, and J₄₅ for further analysis.⁷ Repeatability of refractive error data was assessed by comparing the mean values for each session and for each subject. The mean and standard deviation of the differences were determined along with the 95% limits of agreement (LoA) consistent with the recommendations of Bland and Altman.⁸ The validity of Zywave and autorefractor measurements was assessed by comparing values to the subjective refraction using the same methods.

The repeatability of the higher-order Zernike terms was assessed using the same methods. The mean, SD and95% LoA were calculated for each Zernike term and for each pupil size. To further assess the impact of these values, the calculated between-session standard deviation for each Zernike term was compared to the between-subject standard deviation. In this way, the repeatability of each Zernike term could be placed in the appropriate context by comparing the value to the variation across subjects.

RESULTS

Twenty-three subjects met the eligibility criteria and completed the study. Ten were male and 13 (57 per cent) were female. Subjects ranged in age from 20 to 48years (mean: 27.6 ± 6.8years). The mean spherical equivalent for subjective refraction under cycloplegia was −2.15 ± 2.87 D (range −9.25 to +1.25 D.

Repeatability and validity of refractive error measurements

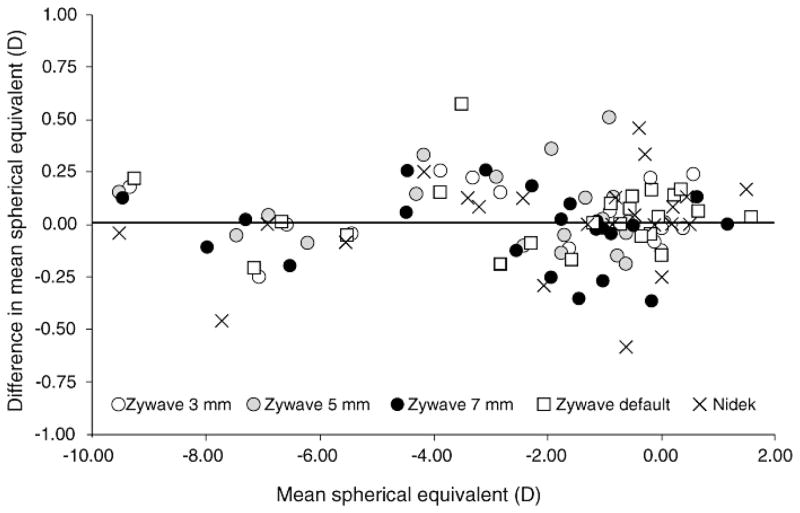
The distribution of between-session differences in spherical equivalent are shown in Figure 1 and the repeatability of refractive error measurements is summarised in Table 1. As expected, none of the mean differences was significantly different from zero. For the spherical equivalent (M), the repeatability of the Zywave was better than that of the autorefractor under both manifest and cycloplegic conditions. The 95% LoA were narrowest for the default and three millimetre pupils under cycloplegia. The repeatability of astigmatism showed similar trends.

Figure 1.

Summary mean versus difference plot comparing the Zywave at all pupil sizes and the Nidek autorefractor under cycloplegic conditions

Table 1.

The repeatability of refractive error measurement for the Nidek ARK–700A autorefractor and the Bausch & Lomb Zywave. All values are in dioptres.

Measurement	M			J0			J45
	Mean	SD	95% LoA	Mean	SD	95% LoA	Mean	SD	95% LoA
Nidek ARK–700A— Manifest	−0.03	0.28	−0.57 to +0.52	+0.01	0.10	−0.19 to +0.22	−0.02	0.12	−0.26 to +0.22
Nidek ARK–700A— Cycloplegic	+0.01	0.23	−0.45 to +0.47	+0.01	0.12	−0.22 to +0.24	+0.01	0.08	−0.15 to +0.17
Zywave— Manifest (default)	+0.04	0.22	−0.39 to +0.48	+0.01	0.08	−0.15 to +0.18	−0.00	0.06	−0.13 to +0.12
Zywave— Cycloplegic (default)	+0.04	0.17	−0.29 to +0.37	+0.00	0.07	−0.14 to +0.14	+0.00	0.07	−0.13 to +0.13
Zywave— Cycloplegic (3mm)	+0.04	0.14	−0.23 to +0.32	−0.01	0.11	−0.23 to +0.21	−0.01	0.09	−0.18 to +0.16
Zywave— Cycloplegic (5mm)	+0.03	0.21	−0.37 to +0.43	−0.03	0.16	−0.33 to +0.28	−0.03	0.11	−0.25 to +0.19
Zywave— Cycloplegic (7mm)	−0.02	0.16	−0.34 to +0.30	+0.01	0.09	−0.16 to +0.19	+0.00	0.06	−0.11 to +0.12

The validity of refractive error measurements is summarised in Table 2. All values are in comparison with the cycloplegic subjective refraction. For the spherical equivalent (M), the mean values for the autorefractor are closest to zero. The 95% LoA are narrowest for the autorefractor under cycloplegia, closely followed by the Zywave under cycloplegia for the default and three millimetre pupil. For astigmatism, the validity is similar for all measurements.

Table 2.

The validity of refractive error measurements compared to the subjective refractions. All values are in dioptres.

Measurement	M			J0			J45
	Mean	SD	95% LoA	Mean	SD	95% LoA	Mean	SD	95% LoA
Nidek ARK–700A—Manifest	+0.06	0.40	−0.73 to +0.84	+0.06	0.16	−0.25 to +0.36	+0.00	0.12	−0.24 to +0.23
Nidek ARK–700A— Cycloplegic	−0.06	0.28	−0.62 to +0.49	−0.07	0.15	−0.36 to +0.22	−0.03	0.11	−0.23 to +0.18
Zywave— Manifest (default)	+0.11	0.46	−0.80 to +1.02	+0.10	0.13	−0.16 to +0.37	+0.02	0.13	−0.23 to +0.28
Zywave— Cycloplegic (default)	+0.21	0.35	−0.47 to +0.90	+0.11	0.13	−0.15 to +0.37	+0.01	0.10	−0.19 to +0.20
Zywave— Cycloplegic (3mm)	+0.23	0.35	−0.46 to +0.92	+0.10	0.14	−0.16 to +0.37	+0.00	0.09	−0.18 to +0.18
Zywave— Cycloplegic (5mm)	−0.26	0.51	−1.26 to +0.75	−0.26	0.13	−0.50 to +0.01	−0.04	0.10	−0.24 to +0.16
Zywave— Cycloplegic (7mm)	−0.45	0.54	−1.51 to +0.61	+0.11	0.15	−0.19 to +0.41	−0.08	0.11	−0.29 to +0.14

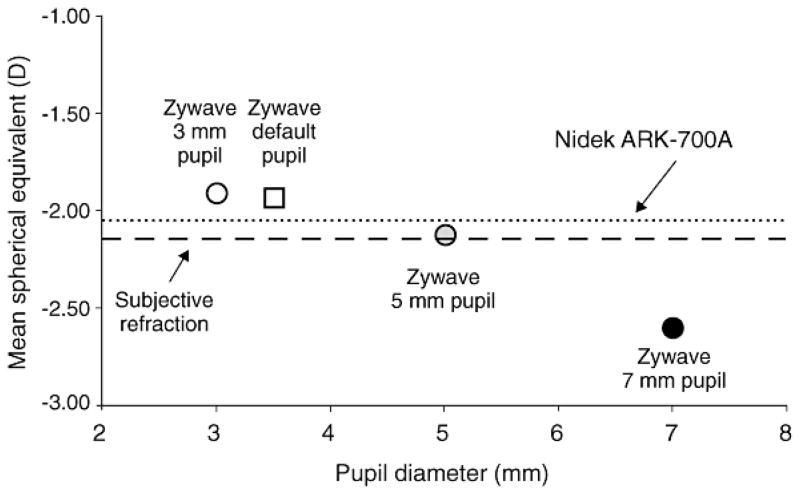
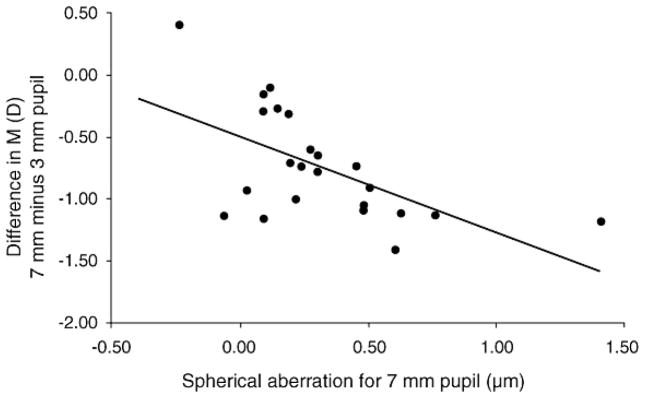
For the Zywave, the spherical equivalent showed a systematic shift in the myopic direction as the pupil size is increased from three to seven millimetres. This trend is shown in Figure 2, which illustrates that the mean is −1.91 D for the three millimetre pupil but −2.60 D for the seven millimetre pupil. It was hypothesised that this shift was due to spherical aberration so the myopic shift was plotted as a function of the C₄⁰ coefficient obtained for a seven millimetre pupil (Figure 3). As can be seen, those eyes with higher levels of spherical aberration show the greatest myopic shift associated with increasing pupil size (r ²= 0.35).

Figure 2.

Mean spherical equivalent determined by the Zywave as a function of pupil size. The values for subjective refraction and the Nidek autorefractor are plotted as lines for comparison.

Figure 3.

Change in spherical equivalent refraction when pupil size is increased from 3mm to 7mm as a function of spherical aberration (C₄⁰) for a 7mm pupil

Repeatability of higher-order aberrations

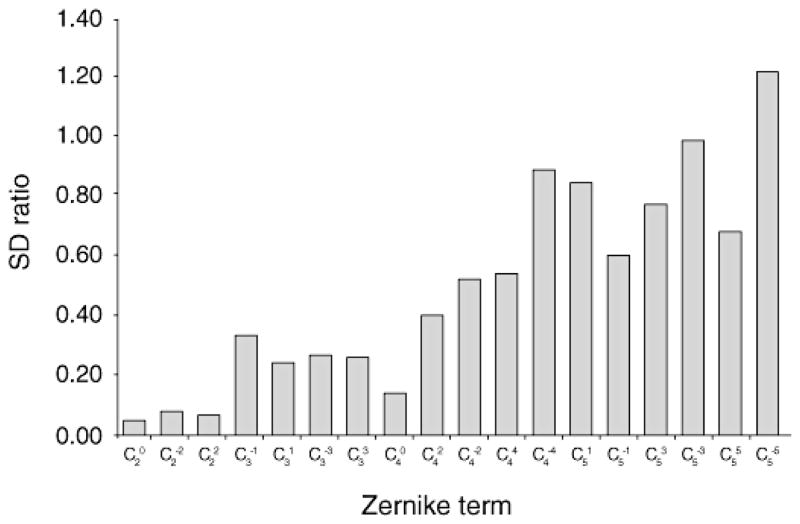
Table 3 shows the repeatability of the Zernike terms for the three, five and seven millimetre pupils. For the three millimetre pupil, the Zywave only generates Zernike terms through the third order. Inspection of Table 3 suggests that repeatability, as indicated by the size of the 95% LoA, is best for the higher-order terms and poorest for the lower-order aberrations. For example, the largest standard deviation and 95% LoA belongs to the C₂⁰ coefficient, which corresponds to the spherical defocus. This is misleading because for a given subject, the low order terms are relatively large and vary considerably across the subjects. In contrast, the higher-order terms tend to be very small in magnitude and vary little between subjects. To compensate for this, the within-subject, between-session standard deviations for the seven millimetre pupil from Table 3 are shown again in Table 4 along with the between-subject, within-session standard deviations. The latter values represent the distribution of Zernike terms across our subject population. The ratios of these two standard deviations were calculated and are shown in Table 4 and plotted in Figure 4. A low ratio indicates good repeatability relative to the variation within the subject population. As would be expected, the second order Zernike terms representing sphere and cylinder have very low ratios indicating that the between-session variability within a subject is very low compared to the variability across subjects. In contrast, some of the higher-order aberrations have ratios of around one, indicating that a repeated measure on a single subject will differ by a similar amount to a new measure on a completely different subject. Of the higher-order aberrations, the coma coefficient (C ₃⁺¹ and C₃⁻¹) and spherical aberration coefficient (C₄⁰) have the lowest ratios. Analysis of the five millimetre pupil data shows very similar trends but neither the data nor the analysis are shown in the interest of space.

Table 3.

Repeatability of higher-order aberrations measured with the Zywave. All values are in μm.

Zernike coeff.	3mm pupil Mean	SD	95% LoA	5mm pupil Mean	SD	95% LoA	7mm pupil Mean	SD	95% LoA
C20	+0.075	0.282	−0.478 to +0.628	+0.033	0.127	−0.216 to+0.282	+0.024	0.233	−0.433 to +0.481
C2−2	+0.011	0.037	−0.062 to +0.084	+0.009	0.050	−0.089 to+0.108	+0.016	0.064	−0.106 to+0.141
C2+2	+0.006	0.047	−0.086 to +0.098	+0.010	0.071	−0.130 to+0.150	+0.006	0.102	−0.194 to+0.206
C3−1	−0.006	0.024	−0.053 to +0.042	−0.022	0.042	−0.104 to +0.061	−0.017	0.113	−0.237 to +0.204
C3+1	+0.003	0.018	−0.031 to +0.037	+0.009	0.045	−0.080 to +0.098	+0.013	0.059	−0.103 to +0.129
C3−3	−0.005	0.023	−0.050 to +0.040	+0.008	0.035	−0.061 to +0.076	+0.005	0.053	−0.099 to +0.108
C3+3	−0.004	0.015	−0.034 to +0.025	+0.006	0.026	−0.044 to +0.056	+0.018	0.051	−0.081 to +0.117
C40				−0.004	0.015	−0.035 to +0.026	−0.010	0.043	−0.095 to +0.075
C4+2				+0.001	0.013	−0.024 to +0.027	+0.008	0.046	−0.083 to +0.098
C4−2				−0.002	0.014	−0.030 to +0.026	−0.001	0.034	−0.067 to +0.066
C4+4				+0.001	0.014	−0.026 to +0.028	−0.002	0.051	−0.102 to +0.097
C4−4				−0.002	0.022	−0.044 to +0.041	−0.005	0.054	−0.111 to +0.100
C5+1				−0.000	0.010	−0.021 to +0.020	−0.003	0.025	−0.051 to +0.046
C5−1				+0.002	0.013	−0.023 to +0.027	+0.009	0.029	−0.048 to +0.065
C5+3				+0.001	0.007	−0.013 to +0.016	−0.011	0.028	−0.065 to +0.043
C5−3				−0.001	0.012	−0.025 to +0.023	−0.003	0.031	−0.064 to +0.057
C5+5				−0.002	0.010	−0.021 to +0.017	−0.009	0.034	−0.077 to +0.058
C5−5				−0.002	0.009	−0.020 to +0.016	+0.005	0.038	−0.070 to +0.080

Table 4.

Comparison of the repeatability (within subject SD, in μm) with the between-subject variability (SD, in μm) for higher-order aberrations measured with the Zywave for 7mm pupil. The final column shows the ratio of these values, a smaller value representing better repeatability.

Zernike coeff.	Within-subject SD (from Table 3)	Between-subject SD	Ratio
C20	0.233	4.985	0.047
C2−2	0.064	0.787	0.081
C2+2	0.102	1.467	0.070
C3−1	0.113	0.333	0.338
C3+1	0.059	0.240	0.246
C3−3	0.053	0.197	0.268
C3+3	0.051	0.193	0.263
C40	0.043	0.304	0.143
C4+2	0.046	0.114	0.402
C4−2	0.034	0.065	0.524
C4+4	0.051	0.093	0.546
C4−4	0.054	0.060	0.893
C5+1	0.025	0.029	0.850
C5−1	0.029	0.047	0.606
C5+3	0.028	0.036	0.774
C5−3	0.031	0.031	0.988
C5+5	0.034	0.050	0.684
C5−5	0.038	0.031	1.221

Figure 4.

The ratio of within-subject SD to between-subject SD for all Zernike aberration terms measured with the Zywave for 7mm pupil. A smaller ratio represents better repeatability.

DISCUSSION

Repeatability and validity of refractive error measurements

The Zywave gives valid and repeatable estimates of refractive error as its measurements compare favourably with the Nidek ARK-700 autorefractor and the 95% LoA are consistent with those found in previous studies of the repeatability of autorefractors.^9,10 Estimates of repeatability and validity can depend on the number of measurements taken. Previous studies have averaged between three and 10 measurements. The Zywave also gives valid estimates of a subject’s manifest refraction and is comparable to other Shack-Hartmann aberrometers.^11–15

Two other studies have compared the repeatability and validity of estimates of refractive error using the Zywave. Hament, Nabar and Nuijts¹¹ compared Zywave measurements to subjective refraction and autorefraction in 20 myopic eyes. They found that with a dilated pupil the Zywave gave substantially more myopia than subjective refraction (mean difference −1.10 ± 0.46 D). For a 3.5mm pupil, the difference was smaller but still significant (mean difference −0.55 ± 0.48 D). They also report a repeatability coefficient of − −0.25 to +0.25 D based on three repeat measurements during a short period. Mirshahi and co-workers¹⁶ determined repeatability (SD of within-session measures) to be −0.15 to +0.15 D using a similar analytical approach in 20 patients. They reported negligible mean differences between the spherical equivalent values from the Zywave and for the studies from subjective refraction but a large range (mean 0.09 D, range −0.81 to +1.83 D). In summary, the Zywave-derived estimates of refractive error data in the current study agree more closely with subjective refraction than in the studies of Hament, Nabar and NJuijts¹¹ and Mirshahi and co-workers.¹⁶

The exact method of determining the Zywave’s or any clinical aberrometer’s autorefraction may not be fully known. In general, clinical aberrometers have autorefractive repeatability that is similar or better than those of clinical autorefractors. Although higher-order aberrations are measured with clinical aberrometers, their fluctuating higher-order aberrations may have minimal effects on the refractive error data. Indeed, clinical aberrometers have been shown to provide consistent refractive error measurements despite fluctuations in high-order aberrations.^16,17

Consistent with the findings of Hament, Nabar and Nuijts,¹¹ as pupil size is manipulated from three, five and seven millimetres on the Zywave, the refractive error becomes more myopic (Table 2, Figure 2). The values of C₂⁰ change in a corresponding manner (data not shown). This reflects the spherical aberration ₀ of the eye and is confirmed by the correlation between the myopic shift in refraction and the C₄ coefficient shown in Figure 3. The higher the magnitude of the spherical aberration term, a higher myopic shift is seen in spherical equivalent. This relationship among spherical aberration, pupil size and refractive error has been described and discussed previously.^18,19 The influence of pupil size on the estimate of spherical equivalent refractive error will depend ultimately on the algorithm used by the instrument and some aberrometers adjust for the eye’s spherical aberration to give an estimate of refractive error approaching that obtained with a smaller pupil.¹⁹

Repeatability of higher-order Zernike terms

Many of the higher-order Zernike terms measured by the Zywave in this study are small in quantity, as many are only one-thousandth of a micron. Because of their small magnitude, this can give the impression that these measurements are very consistent. In contrast, measures of second-order aberrations—corresponding to sphere and cylinder—appear less repeatable, having larger between-session standard deviations, however, these lower-order aberrations vary considerably among subjects. We attempted to take a balanced approach by referencing the between-session standard deviations to the corresponding between-subject standard deviations. We assert that the calculated ratio of these standard deviations gives a truer indication of the repeatability of the Zywave’s ability to estimate each Zernike term. Figure 4 emphasises that the repeatability of the second-order terms is good with ratios less than 0.1. The repeatability of third-order aberrations and spherical aberration coefficients (C₄⁰) may be considered acceptable with ratios of around 0.2. In other words, the variation between measurements on the same subject are small compared to those between subjects. In contrast, the repeatability of other higher-order Zernike terms should be considered poor, with ratios closer to 1.

A similar conclusion was drawn by Mirshahi and co-workers.¹⁶ Their approach was slightly different—they calculated the coefficient of variation for each term (SD/mean)—but the outcomes show trends similar to our data, with unsatisfactory repeatability for most fourth-and fifth-order Zernike terms. Inspection of their Figure 2 shows that it has an appearance very similar to our Figure 4.

With regards to higher-order aberrations, there are many factors one must examine as sources for differences in repeatability data. For example, instrument alignment,²⁰ time between measurements,^{20, 21} and biological variables (ocular optics, tears, accommodation, pupil size)²² among others must be considered.

Aberrometry measurements and wavefront-guided refractive surgery

It is estimated that the higher-order aberrations of a normal and healthy eye account for 0.25 D or less of dioptric blur^4,23,24 and yet, wavefront guided treatments have increasingly become the standard of care in corneal refractive surgery.²⁵ In part, this is because the visual results of wavefront guided treatments are somewhat better.^26–28 Although higher-order wavefront errors are only a small fraction of the total optical aberrations of the normal eye, when combined with the additional aberrations induced by refractive surgical treatment, they can become visually significant.^29,30 Applegate, Sarver and Khemsara³¹ have demonstrated that not all aberrations have an equal influence on vision. Several third-and fourth-order terms significantly degrade vision in the postoperative eye, suggesting that accurate measurements to at least this level are needed.³¹

When accurate preoperative measurements of aberrations are combined with information about the magnitude of treatment-induced aberrations, it is possible to minimise the treatment-induced aberrations and optimise vision. The ability to achieve this goal depends on several factors. These include:

1. The ability to correctly measure ocular wavefront errors

2. Translation of the measured error to an appropriate treatment plan

3. Successful execution of the treatment plan

4. A predictable biological response to the treatment.

Here we report on only the first of these factors that can influence visual results after wavefront customised corneal refractive surgery.

Wavefront sensing has developed rapidly in the field of refractive surgery due to the understanding of not only how refractive surgery may induce higher-order aberrations³² but also analysis of higher-order aberrations pre-operatively to design a customised correction that can achieve excellent visual performance.³³ Analysing the inconsistency of some higher-order aberrations, potentially one may relate the importance of specific higher-order aberrations in complete wavefront error, despite the overall sum being less than 0.25 D or less of dioptric blur. Cheng and colleagues²¹ also proposed that certain higher-order aberrations are more worthy of correction than others. Trying to correct a small and fluctuating higher-order aberration may lead to the generation of a further higher-order aberration. Therefore, it is important to identify the most relevant Zernike terms, their role in determining the overall wavefront and, ultimately, their effect on vision when developing algorithms for custom refractive surgery.

Caveats and limitations

Here we report on the performance of a single commercially-available clinical aberrometer. The Zywave produces repeatable and valid estimates of refractive error. Given its level of sophistication compared to traditional autorefractors, its superior repeatability might have been anticipated. The data were taken on the first generation of the Zywave and it is possible that subsequent hardware and software upgrades may further improve the repeatability of this instrument. We did not evaluate other clinical aberrometers, which are believed to produce similar values,^15,34 although differences may exist.²⁰ One important difference between the Zywave and other clinical aberrometers is the relatively low spatial resolution of its Shack-Hartmann grid. The Zywave has 76 spots on its centroid pattern while others such as the LADARWave (Alcon Labs, Inc, Fort Worth, TX) has 204 to 213, the Wavescan (VISX, Santa Clara, CA) has 240 and the COAS (AMO-Wavefront Sciences, Albuquerque, NM) has 1,452 sampling points.³⁵ Work may be done to analyse benefits/limitations of the centroid patterns and sampling points. It appears that the Zywave will have a dynamic range that is limited to calculating fewer Zernike polynomial terms of lower frequency.¹⁵ Although this study was not specifically designed to quantify the impact of Shack-Hartmann spatial resolution on measurement precision, we expect that this Zywave design limitation explains some of the poorer repeatability that we observed with higher-order terms.

SUMMARY

The Zywave provides repeatable measures of refractive error at the adjusted pupil diameters under both cycloplegic and manifest conditions over a range of refractive errors for normal eyes. The Zywave refractive error estimates are comparable to subjective refraction and another autorefractor for small pupils but more myopic when set to five or seven millimetres. The second-order Zernike terms along with the spherical aberration and coma terms derived from the Zywave show good repeatability. Some of the higher-order Zernike terms are small in magnitude and are variable between sessions.