Q-space and Conventional Diffusion Imaging of Axon and Myelin Damage in the Rat Spinal Cord after Axotomy

Abstract

Parallel and perpendicular diffusion properties of water in the rat spinal cord were investigated 3 and 30 days after dorsal root axotomy, a specific insult resulting in early axonal degeneration followed by later myelin damage in the dorsal column white matter (WM). Results from q-space analysis (i.e. the diffusion probability density function, PDF) obtained with strong diffusion weighting were compared to conventional anisotropy and diffusivity measurements at low b-values, as well as to histology for axon and myelin damage. Q-space contrasts included the height (PZERO), full width at half maximum (FWHM), root mean square displacement (RMSD), and kurtosis excess (KE) of the PDF, which quantifies the deviation from Gaussian diffusion. Following axotomy, a significant increase in perpendicular diffusion (with decreased KE) and decrease in parallel diffusion (with increased KE) were found in lesions relative to uninjured WM. Notably, a significant change in abnormal parallel diffusion was detected from 3 to 30 days with FWHM, but not with conventional diffusivity. Also, directional FWHM and RMSD measurements exhibited different sensitivities to WM damage. When compared to histology, the increase in perpendicular diffusion was not specific to demyelination, whereas combined reduced parallel diffusion and increased perpendicular diffusion was associated with axon damage.

INTRODUCTION

Recent Diffusion Tensor Imaging (DTI) studies have suggested that parallel (also termed axial or longitudinal) diffusivity may be sensitive to axonal injury, while perpendicular (also termed radial or transverse) diffusivity may be sensitive to myelin injury (1–4). The prospect that such individual water diffusion properties could potentially report specifically on different types of white matter (WM) injury is exciting. However, the conclusive assignment of changes in diffusion properties to axon and/or myelin damage is not straightforward because axon and myelin damage are often histopathologically linked, and vary in time and space according to the specifics of each disease model. In addition, the biophysics of diffusion is not fully understood and the choice of the diffusion tensor model and the assumptions made for its analysis can have significant ramifications on the quantification of diffusion properties and the potential correlation with WM damage. For instance, DTI approaches commonly work under the assumption of free diffusion (monoexponential diffusion signal attenuation), i.e. corresponding to a Gaussian distribution for the probability density function (PDF). The PDF is the probability that a spin diffuses a given distance from its initial position during the allowed diffusion time. The shape of the PDF contains information about the diffusion environment. A tall, narrow PDF suggests a low diffusion constant, whereas a low, broad PDF suggests a high diffusion constant.

An alternative diffusion weighted MRI (DWI) analysis method is the q-space approach (5–8), which unlike conventional analyses does not assume a pre-defined shape for the PDF. This q-space technique has been applied to study several animal models of spinal cord injury, including myelin development (9,10), WM damage due to crush injury (11), myelin deficiency (12,13), hypertension induced neurodegeneration (14) and experimental allergic encephalomyelitis (EAE) (15). More recently, it has been used to study multiple sclerosis in the human brain (16–18) and spinal cord (19). Q-space contrasts include the height (i.e., the return to zero displacement probability, PZERO), full width at half maximum (FWHM), root mean square displacement (RMSD), and kurtosis excess (KE) (20,21) of the PDF. This later parameter can quantify whether diffusion is free or restricted. A Gaussian PDF has KE = 0, while a PDF that is more peaked and has larger tails than a Gaussian PDF of equal variance has a positive KE. Similar to diffusivity, KE can be reported as the mean value over all orientations ($\stackrel{‒}{\mathrm{KE}}$) or alternatively, measured perpendicular (KE_⊥), or parallel (KE_∥) to the WM fiber orientation. Previous q-space DWI studies have shown that water diffusion in WM is non-Gaussian and have reported the maximum KE over a number of directions in myelin deficient rat brain (22), together with KE_⊥ in the healthy rat spinal cord (23), mouse spinal cord (24), and human brain (25). Recently, a q-space DWI study of the human brain reported that $\stackrel{‒}{\mathrm{KE}}$ is sensitive to WM damage due to multiple sclerosis (18).

The spinal cord, with densely packed myelinated axons oriented in the rostral-caudal direction, is an ideal model system in which to investigate the effects of restricted diffusion and changes in the DWI signal due to WM damage. This a priori directional information allows diffusion weighting gradients to be oriented parallel and perpendicular to the WM fiber bundles, which is difficult to achieve in the brain due to its complex fiber architecture. The current study used high resolution ex vivo DWI to examine Wallerian degeneration (26) in the rat spinal cord after dorsal root axotomy (27). The time course of WM damage in this model has been well characterized, with dorsal root axotomy resulting in rapid axonal degeneration of the ascending fibers in the dorsal column, followed by delayed demyelination over the course of several weeks, with a distinct lack of an inflammatory response (27–30). The main objective was to compare q-space contrasts to conventional anisotropy and diffusivity measurements and to histology for axon and myelin damage, under the hypothesis that q-space contrasts are more sensitive to WM damage than conventional anisotropy and diffusivity measurements. We also investigated whether changes in diffusion contrasts are specific to axon or myelin damage identified with histology. We demonstrate that q-space analysis provides a more comprehensive assessment of WM damage and offers several advantages over conventional DWI analysis, including the measurement of the deviation from Gaussian diffusion behavior and sensitivity to the fast and slow diffusion components (under conditions of non-monoexponential decay when using high b-values). This affords improved detection of abnormal diffusion due to WM damage. To our knowledge, this is the first q-space DWI study to report $\stackrel{‒}{\mathrm{KE}}$ and KE_∥ in the spinal cord, and the first to report changes in $\stackrel{‒}{\mathrm{KE}}$, KE_⊥, and KE_∥ due to WM damage.

MATERIALS AND METHODS

Theory

The relationship between the measured echo attenuation E_tdif (q), the PDF ${\stackrel{‒}{\mathrm{P}}}_{\mathrm{s}}(\mathbf{R},{\mathrm{t}}_{\mathrm{dif}})$ at a given diffusion time t_dif and position R, and the loss of phase coherence due to spin displacement is

$${\mathrm{E}}_{\mathrm{tdif}}\left(\mathbf{q}\right)=\int {\stackrel{‒}{\mathrm{P}}}_{\mathrm{s}}(\mathbf{R},{\mathrm{t}}_{\mathrm{dif}})\exp (\mathrm{i}2\pi \mathbf{q}\cdot \mathbf{R})\mathrm{d}\mathbf{R}$$ [1]

Where q (a measure of the amount of diffusion weighting) is defined as:

$$\mathbf{q}=\gamma \delta \mathbf{G}∕\left(2\pi \right)$$ [2]

The key principle in q-space analysis is that a Fourier transform (FT) of the signal attenuation with respect to q provides the PDF for diffusion:

$${\stackrel{‒}{P}}_s(\mathbf{R},t_{dif})=FT\left\{E_{tdif}\left(\mathbf{q}\right)\right\}$$ [3]

The shape of the PDF can be characterized by the maximum height (i.e., the return to zero displacement probability, PZERO) and full width at half maximum (FWHM) (29). In the specific case of free diffusion (i.e., Gaussian PDF), the diffusion constant D and the root mean square displacement (RMSD) for one-dimensional diffusion can be computed from the FWHM (8):

$$\mathit{RMSD}=\frac{\mathit{FWHM}}{2\sqrt{2\ln \left(2\right)}}=\sqrt{2D{t}_{dif}}$$ [4]

Several previous q-space DWI studies have reported the RMSD of the PDF by scaling the FWHM (i.e., RMSD = 0.425 × FWHM) (10,12–16,19,31–33). However, when diffusion is not characterized by a Gaussian PDF (e.g., in the case of non-monoexponential signal attenuation for water diffusion in WM in the central nervous system), the RMSD and FWHM of the PDF may contain unique information and are not related in such a direct way. In this study, the FWHM is computed by linearly interpolating between points on the PDF and the RMSD is computed as:

$$\mathrm{RMSD}=\sqrt{(\int {\stackrel{‒}{\mathrm{P}}}_{\mathrm{s}}\cdot {\mathbf{R}}^2\mathrm{d}\mathbf{R})}$$ [5]

The kurtosis excess (KE) (20,21) of a distribution offers additional information regarding the shape of the PDF and is defined as:

$$\mathrm{KE}=({\mu }_4∕{{\mu }_2}^2)-3$$ [6]

Where μ₂ (the variance of the PDF) and μ₄ are computed about the mean, μ, according to:

$${\mu }_{\mathrm{N}}=\int {(\mathrm{R}-\mu )}^{\mathrm{N}}{\stackrel{‒}{\mathrm{P}}}_{\mathrm{s}}\left(\mathbf{R}\right)\mathrm{d}\mathbf{R}$$ [7]

Animal Preparation

All procedures were approved by the Animal Research Committee of the Johns Hopkins University School of Medicine. Twelve female Lewis rats (160–200g, Charles River Laboratories Inc., Wilmington, MA, USA) were used. Rats were anesthetized with isoflurane and a hemilaminectomy was performed at the L₄ to L₆ vertebral levels to expose the cauda equina on the left side. The left L₂ to L₄ dorsal roots were transected just proximal to the dorsal root ganglion, thereby affecting ascending fibers on the left side of the dorsal column. The wound was closed with wound clips and the animals were allowed to recover. Of the 12 animals, six were permitted to recovery for 3 days, and the remaining six for 30 days. Before ex vivo imaging, the rats were deeply anaesthetized and perfused through the heart with cold phosphate buffered saline (PBS) followed by freshly prepared 4% paraformaldehyde. The spinal cords were excised and fixed in 4% paraformaldehyde overnight at 4 °C before transferring to PBS and stored at 4 °C.

Diffusion Weighted MRI

Imaging was performed on an 11.7 Tesla spectrometer equipped with a gradient system capable of a maximum gradient strength of 300 Gauss/cm (Bruker Biospin, Billerica, MA, USA). For the duration of the experiment, spinal cord specimens were kept in a 5 mm NMR tube filled with fomblin (Fomblin Perfluoropolyether, Solvay Solexis, Inc., West Deptford, NJ, USA) to prevent dehydration, and the temperature was maintained at 30 °C via the spectrometer's temperature control system. A 5-mm diameter saddle coil was used as the radio frequency transmitter and signal receiver. Diffusion weighted images (DWIs) of the spinal cord (5 slices centered on L₁, spanning from approximately L₂ to T₁₀) were acquired with a stimulated echo sequence (TR/TE of 1600ms/18.5 ms, acquired resolution of 0.1 × 0.1 × 1.5 mm), with 8 signal averages. DWIs were acquired at 12 q-values from 102 to 1533 cm⁻¹ (b_max= 74202 s/mm²) for diffusion weighting perpendicular to the spinal cord (two orientations, G = [1, 0, 0] and [0, 1, 0]), and 8 q-values from 102 to 575 cm⁻¹ (b_max= 10435 s/mm²) for diffusion weighting parallel to the spinal cord (G = [0, 0, 1]). The duration (δ = 3ms) and leading edge spacing (Δ = 81 ms) of the diffusion gradients were held constant for all experiments, yielding a diffusion time (t_dif = Δ − δ/3) of 80ms. The b-values and corresponding q-values are shown in table 1.

Table 1.

b-values and corresponding q-values for images with diffusion weighting perpendicular (⊥) and parallel (∥) the long axis of the spinal cord at t_dif = 80ms.

Perpendicular (⊥)		Parallel (∥)
b-value [s/mm2]	q-value [1/cm]	b-value [s/mm2]	q-value [1/cm]
330	102	330	102
1010	179	1159	192
2061	255	2061	255
4638	383	3221	319
8245	511	4638	383
12882	639	6312	447
18550	766	8245	511
25249	894	10435	575
32979	1022
41739	1150
51529	1277
74202	1533

Data Processing

Data processing was done offline in Matlab (Mathworks, Natick, MA) running on a Sun Fire V880 server (Sun Microsystems, Santa Clara, CA). For signal to noise ratio (SNR) calculations, mean images and difference images were computed from DWIs with diffusion weighting along [1,0,0] and [0,1,0]. The SNR was computed as the mean value in a region of interest (ROI) in the contralateral dorsal column WM in the mean image divided by the standard deviation over the voxels in the same ROI in the difference image. At the level of L₁, the SNR was 30±10 and 7±2 at b-values of 330 and 74202 s/mm², respectively (averaged over the 12 rats). An artificial noise floor was defined as 1.5 times the standard deviation over all voxels in the difference image at b = 74202 s/mm². Signal falling below this noise floor in magnitude images was set to zero and these voxels were excluded from analysis.

For the quantification of diffusion perpendicular to the spinal cord, DWIs with diffusion weighting along [1, 0, 0] and [0, 1, 0] were averaged. Conventional analysis was performed by fitting the Stejskal-Tanner equation to the first three points of the signal decay curves at low b-values (≤ 2061 s/mm² for both perpendicular and parallel diffusion weighting) where the signal decay appeared mono-exponential. Parallel diffusivity (D_∥), perpendicular diffusivity (D_⊥), and mean diffusivity (MD) as well as fractional anisotropy (FA) were calculated on a voxel by voxel basis under the assumption of an oriented, cylindrically symmetric tensor.

For q-space analysis, a bi-exponential was fit to the signal decay as a function of b-value. The PDFs for diffusion in the perpendicular and parallel orientations, respectively, were computed from the analytical Fourier transform of each exponential component.

$${\stackrel{‒}{P}}_s(\mathbf{R},t_{dif})=\sum _{i=1}^N{f}_i\frac{1}{\sqrt{4\pi D_i{t}_{dif}}}\exp \left(\frac{-R^2}{4D_i{t}_{dif}}\right)$$ [8]

Where N = 2, f_i and D_i are the volume fractions and diffusion constants of each component, and ${\sum }_{\mathrm{i}=1}^{\mathrm{N}}{\mathrm{f}}_{\mathrm{i}}=1$. The KE of a PDF composed of N Gaussian components is given by (34):

$$\mathrm{KE}=3\frac{\mathrm{V}\left(\mathrm{D}\right)}{{\left(\stackrel{‒}{\mathrm{D}}\right)}^2}$$ [9]

Where $\stackrel{‒}{\mathrm{D}}={\sum }_{\mathrm{i}=1}^{\mathrm{N}}{\mathrm{f}}_{\mathrm{i}}{\mathrm{D}}_{\mathrm{i}}$ and $\mathrm{V}\left(\mathrm{D}\right){\sum }_{\mathrm{i}=1}^{\mathrm{N}}{\mathrm{f}}_{\mathrm{i}}{({\mathrm{D}}_{\mathrm{i}}-\stackrel{‒}{\mathrm{D}})}^2$. Q-space contrast maps were computed on a voxel-by-voxel basis for perpendicular (PZERO_⊥, FWHM_⊥, RMSD_⊥, KE_⊥) and parallel (PZERO_∥, FWHM_∥, RMSD_∥, KE_∥) diffusion, and the mean kurtosis, $\stackrel{‒}{\mathrm{KE}}$, was computed as

$$\stackrel{‒}{\mathrm{KE}}=({\mathrm{KE}}_{\parallel }+2{\mathrm{KE}}_{\perp })∕3$$ [10]

A ROI analysis was performed on a single slice at the L₁ level in each animal. ROIs were delineated on DWIs with diffusion weighting parallel to the spinal cord (b = 2061 s/mm²) as the lesion was hyperintense on these images. Three ROIs were selected in each animal to include lesioned WM, uninjured contralateral WM, and gray matter (GM), respectively. The ROIs contained approximately 40 voxels. The mean and standard deviation of the ROI-based measurements over the 6 animals in each group are reported.

Histology

Tissues were dissected out of the bone and cut into 11 cross-sectional pieces 5–7mm long. These were pre-embedded together in agar-gelatin (35) and then processed for paraffin embedding by routine procedures. Seven micron thick sections were cut and stained for myelin and axon markers following detailed methods described in (36). Myelin staining was performed with Luxol Fast Blue (LFB), while axon damage was assessed with monoclonal antibody SMI-31 (staining for hyper-phosphorylated neurofilaments indicative of healthy axons), monoclonal antibody SMI-32 (staining for hypo-phosphorylated neurofilament indicative of damaged axons), and the accumulation of amyloid pre-cursor protein (APP) which indicates an interruption of fast axonal transport. Axon and myelin morphology was assessed by toluidine blue staining (1μm thick cross-sections) following detailed methods described in (36) and examined by light microscopy with a 100× oil-immersion objective lens.

Statistical Analysis

Two-tailed Student's t-Tests, assuming unequal variance, were performed to test the null hypothesis that ROI-based measurements in lesion, and contralateral uninjured WM had equal means (at a significance level of p = 0.01). Pair-wise comparisons were made between the lesion at 3 days, the lesion at 30 days, and contralateral uninjured WM. Separate tests were performed for each q-space and conventional DWI contrast. To determine the contrasts that showed the greatest sensitivity to abnormal diffusion, the percent change of each contrast was computed between: (1) the lesion at 3 days and contralateral WM, (2) the lesion at 30 days and contralateral WM, and (3) the lesion at 3 days and lesion at 30 days.

RESULTS

Diffusion Weighted Imaging

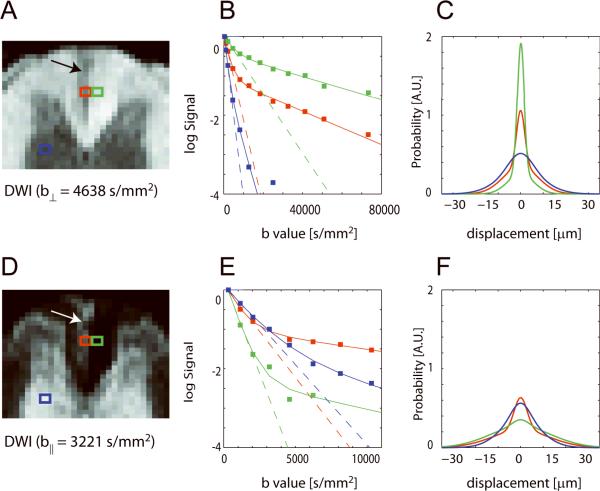
The lesion is approximately wedge shaped at the level of L₁ and appears as a hypointensity and hyperintensity in DWIs with perpendicular and parallel diffusion weighting, respectively (Figure 1A, D). The lesion is approximately 0.1 to 0.4 mm wide, and 0.6 mm long and extends from the dorsal surface of the dorsal column to the border with the corticospinal tract at the ventral portion of the dorsal column in agreement with the known course of ascending fibers affected by L₂–L₄ axotomy (37). Signal attenuation curves from representative single voxels in lesion, uninjured contralateral WM, and GM show that for diffusion weighting perpendicular to the long axis of the spinal cord, signal in contralateral WM is preserved even in the high b-value range, whereas signal in the lesion drops off more rapidly with b-value (Figure 1B). The amount of signal attenuation in GM exceeds that found in lesion or healthy WM due to more free diffusion. In the case of diffusion weighting parallel to the long axis of the spinal cord, the signal in contralateral WM falls off rapidly while the signal in the lesion is preserved at higher b-values (Figure 1E). For parallel diffusion, the signal attenuation in healthy GM lies between that found in lesion and healthy WM. Notably, the monoexponential fits from which D_⊥ and D_∥ are calculated describe the data well only at low b-values (< 2061 s/mm²), while the biexponential function fits the full signal attenuation curve (Figure 1B, E). The PDF for perpendicular diffusion in the lesion is wider and shorter than the PDF in contralateral uninjured WM, and is distinct from the PDF in GM (Figure 1C). For parallel diffusion, the PDF in the lesion is narrower and taller than the PDF in contralateral WM and is distinct from the PDF in GM (Figure 1F).

Figure 1.

Diffusion weighted images of the dorsal column at the level of L₁, signal attenuation curves, and computed PDFs for diffusion perpendicular (⊥) (A–C) and parallel (∥) (D–F) to the long axis of the rat spinal cord 30 days after axotomy. The location of the lesion in the left dorsal column is indicated (arrows). Results for single voxels in lesion (red), uninjured contralateral WM (green), and GM (blue) are shown. The monoexponential fit to the signal attenuation at low b-values and the bi-exponential fit over all b-values are indicated by dotted and solid lines, respectively.

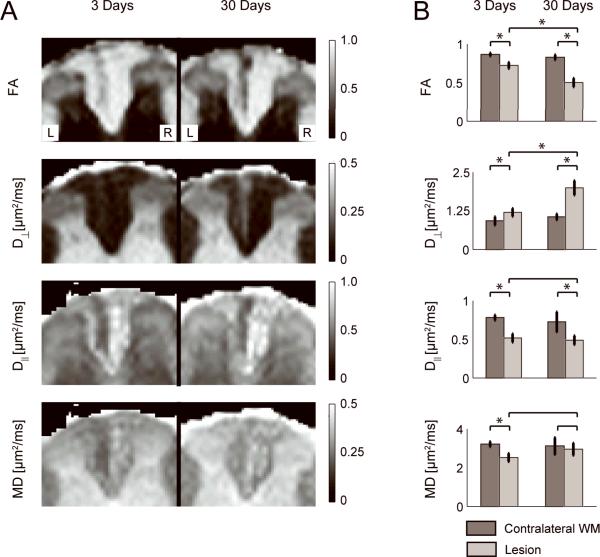
Figure 2A shows diffusion contrast maps from conventional DWI analysis for two representative animals. At 3 days post axotomy the lesion can be identified as a hyperintensity on the D_⊥ map and a hypointensity on the D_∥, FA, and MD maps with respect to the surrounding WM and uninjured contralateral WM. The lesion is markedly more conspicuous at 30 days post axotomy in the D_⊥ and FA maps. In the case of D_∥, the images at 3 days and 30 days show little difference in lesion conspicuity. The increase of D_⊥ and stabilization of D_∥ between 3 and 30 days results in a pseudo-normalization of MD, with the lesion appearing less conspicuous at 30 days. This is confirmed by the quantitative ROI-based analysis in Figure 2B, showing negligible difference, while measurements of D_⊥ and FA were all significantly different (p < 0.01) for comparisons of the lesion at 3 days and contralateral WM, the lesion at 30 days and contralateral WM, and the lesion at 3 days and 30 days.

Figure 2.

A) Fractional anisotropy (FA), perpendicular diffusivity (D_⊥), parallel diffusivity (D_∥), and mean diffusivity (MD) maps for an animal at 3 days (left image) and another animal at 30 days (right image) post-axotomy. B) ROI analysis of the conventional DWI contrasts in the contralateral WM (dark bars) and lesion (light bars) over the 6 animals in each group. * denotes significant difference (p < 0.01).

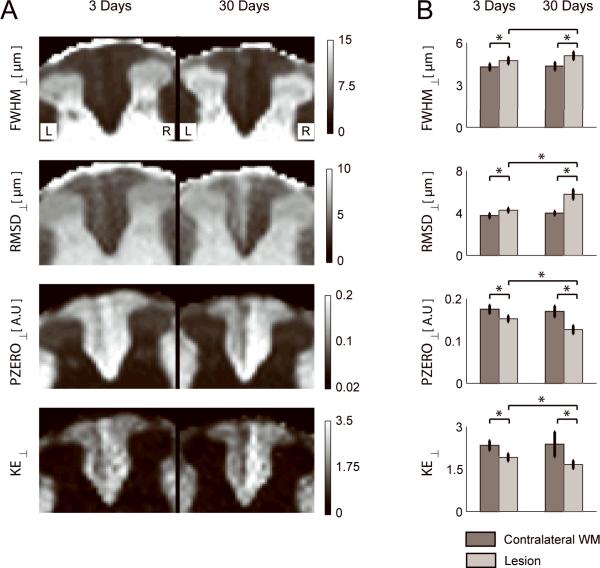
Figure 3A shows q-space contrast maps for diffusion perpendicular to the long axis of the spinal cord. At 3 days post axotomy the lesion can be identified as hyperintense on FWHM_⊥ and RMSD_⊥ maps, and hypointense on PZERO_⊥ and KE_⊥ maps, with respect to the uninjured contralateral WM. Q-space contrasts for perpendicular diffusion were all abnormal at 3 and 30 days after axotomy compared to uninjured contralateral WM (p < 0.01, Figure 3B). The lesion is more conspicuous at 30 days for RMSD_⊥, PZERO_⊥ and KE_⊥ and measurements in lesions at 3 days and 30 days were significantly different (p < 0.01) for these three parameters.

Figure 3.

A) Maps of the full width at half maximum (FWHM_⊥), root mean square displacement (RMSD_⊥, height (PZERO_⊥), and kurtosis excess (KE_⊥) of the PDF for perpendicular diffusion for an animal at 3 days (left image) and another animal at 30 days (right image) post-axotomy. B) ROI analysis of the q-space contrasts in the contralateral WM (dark bars) and lesion (light bars) over the 6 animals in each group. * denotes significant difference (p < 0.01).

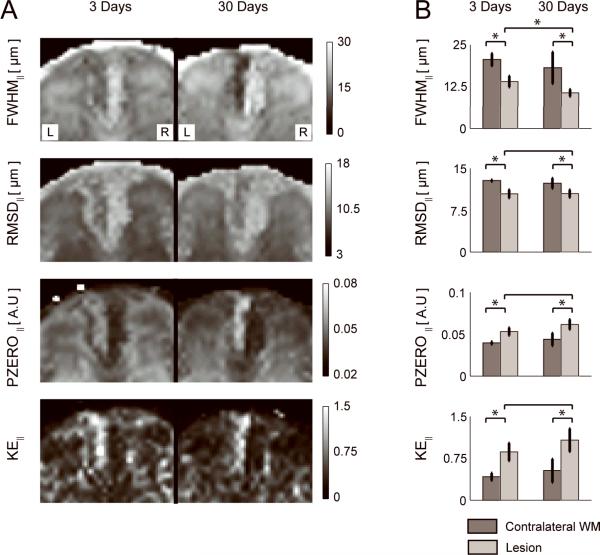
Figure 4A shows q-space contrast maps for diffusion parallel to the long axis of the spinal cord. At 3 days post axotomy, the lesion is hypointense on FWHM_∥ and RMSD_∥ maps, and hyperintense on PZERO_∥ and KE_∥ maps. Q-space contrasts for parallel diffusion were all abnormal at 3 and 30 days after axotomy compared to uninjured contralateral WM (p < 0.01, Figure 4B). The lesion is more conspicuous at 30 days for FWHM_∥ and PZERO_∥, which is reflected in measurements of FWHM_∥ at 3 days and 30 days being significantly different (p < 0.01).

Figure 4.

A) Maps of the full width at half maximum (FWHM_∥), root mean square displacement (RMSD_∥), height (PZERO_∥), and kurtosis excess (KE_∥) of the PDF for parallel diffusion in an animal at 3 days (left image) and another animal at 30 days (right image) post-axotomy. B) ROI analysis of the q-space contrasts in the contralateral WM (dark bars) and lesion (light bars) over the 6 animals in each group. * denotes significant difference (p < 0.01).

The relative changes for each diffusion parameter both with respect to contralateral normal WM and as a function of time are reported in Table 2. For perpendicular diffusion, D_⊥, PZERO_⊥, FWHM_⊥, RMSD_⊥, and KE_⊥ exhibited comparable sensitivity to abnormal diffusion at 3 days, with D_⊥ exhibiting the largest change, followed by KE_⊥. D_⊥ also exhibited the largest abnormality at 30 days (89 % increase), and largest change in contrast for the lesion at 3 and 30 days (66% increase), in both cases followed by RMSD_⊥. For parallel diffusion, D_∥, FWHM_∥ and PZERO_∥ showed comparable sensitivity to abnormal diffusion at 3 days post axotomy. KE_∥ exhibited the largest lesion contrast (>100%) both at 3 days and 30 days post axotomy. In assessing the lesion contrast change in parallel diffusion from 3 to 30 days, the change in D_∥ was not statistically significant (p=0.4), while FWHM_∥ was (p=0.002), demonstrating an improved detection of abnormal diffusion along the length of the WM fibers with q-space DWI. Mean kurtosis measurements ($\stackrel{‒}{\mathrm{KE}}$) were less sensitive than direction-specific KE (i.e., KE_⊥ and KE_∥) and were not significantly different in lesions at 3 days compared to contralateral WM ($\stackrel{‒}{\mathrm{KE}}=1.56\pm 0.08$, $\stackrel{‒}{\mathrm{KE}}=1.69\pm 0.10$, p=0.03), nor in lesions at 30 days compared to contralateral WM ($\stackrel{‒}{\mathrm{KE}}=1.76\pm 0.10$, $\stackrel{‒}{\mathrm{KE}}=1.46\pm 0.14$, p=0.02).

Table 2.

The relative change of diffusion contrasts between lesions at 3 days, 30 days, and uninjured contralateral white matter.

Contrast	Lesion at 3 Days		Lesion at 30 Days		Lesion at 30 Days
	Vs. Uninjured WM		Vs. Uninjured WM		vs. Lesion at 3 Days
	Change (%)	p-value	Change (%)	p-value	Change (%)	p-value
FA	−16 ± 5	* < 0.0001	−39 ± 8	* < 0.0001	−31 ± 10	* < 0.0001
D⊥	30 ± 21	* 0.005	89 ± 26	* < 0.0001	66 ± 23	* < 0.0001
D∥	−34 ± 9	* < 0.0001	−33 ± 21	* 0.002	−5 ± 16	0.4
MD	−22 ± 8	* < 0.0001	−5 ± 18	0.5	17 ± 15	0.02
FWHM⊥	10 ± 8	* 0.009	17 ± 9	* 0.001	7 ± 8	0.05
RMSD⊥	14 ± 9	* 0.002	45 ± 13	* < 0.0001	35 ± 12	* < 0.0001
PZERO⊥	−13 ± 7	* 0.001	−25 ± 10	* < 0.0001	−17 ± 8	* 0.0004
KE⊥	−18 ± 9	* 0.0007	−30 ± 20	* 0.004	−13 ± 10	* 0.0091
FWHM∥	−32 ± 13	* < 0.0001	−41 ± 29	* 0.004	−24 ± 14	* 0.002
RMSD∥	−18 ± 6	* < 0.0001	−15 ± 9	* 0.003	0 ± 10	0.9
PZERO∥	35 ± 13	* < 0.0001	41 ± 23	* 0.001	16 ± 14	0.02
KE∥	106 ± 45	* < 0.0001	103 ± 69	* 0.001	24 ± 31	0.08
$\stackrel{‒}{\mathrm{KE}}$	−8 ± 8	0.03	−17 ± 16	0.02	−6 ± 10	0.2

Positive (negative) values denote an increase (decrease) in the diffusion measure relative to the uninjured contralateral WM (columns 1 and 2) or relative to the lesion 3 days after axotomy (column 3).

^*denotes significant difference (p < 0.01) for the ROI analysis presented in Figures 2B, 3B and 4B.

Histology of White Matter Damage

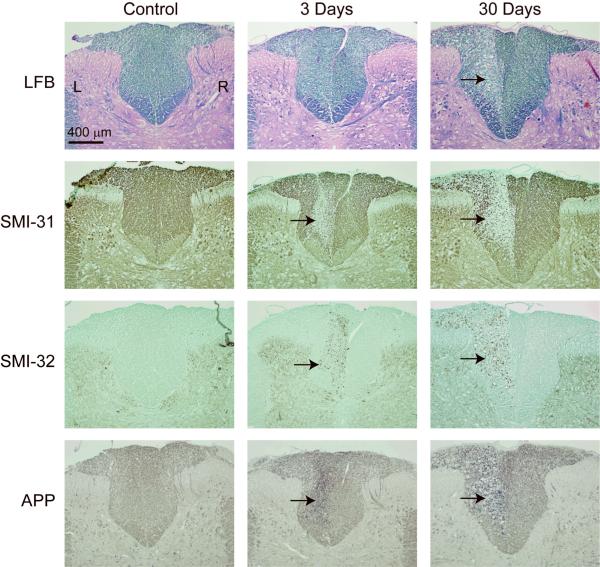
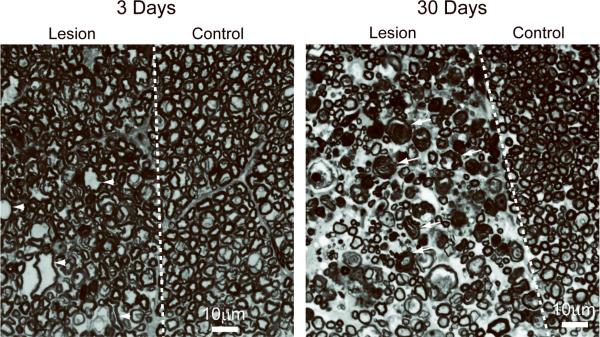
Histological assessment of axon and myelin damage in the dorsal column of a control animal, and two separate animals 3 and 30 days after axotomy is shown in Figure 5. At the level of L₁, LFB staining shows negligible change in myelin pallor three days after axotomy, which suggests no demyelination, while LFB shows a visible loss of myelin staining 30 days after axotomy. Staining for axonal markers showed a decrease in SMI-31 and an increase in SMI-32 immunoreactive neurofilament in the lesion three days after axotomy, which suggest significant axon damage. Furthermore, the accumulation of APP three days after axotomy is indicative of an interruption of fast axonal transport. Notably, the decreased detection of SMI-31, increased detection of SMI-32 and accumulation of APP persisted 30 days after axotomy. Figure 6 shows toluidine blue staining of injured and uninjured contralateral WM at 3 and 30 days post-axotomy. At 3 days post-axotomy, the lesion shows the clear presence of several irregularly shaped and enlarged axons. Both healthy and affected axons appear to be surrounded by intact, normal appearing myelin similar to contralateral uninjured WM. At 30 days post-axotomy the effects of WM degeneration are very prominent and the lesion can be clearly distinguished from the uninjured contralateral WM. Notably, several collapsed and degenerating axons show unraveling myelin and have the characteristic appearance of myelin ovoids.

Figure 5.

Histological images of the dorsal column WM of the rat spinal cord. Images are shown for a control animal, and animals 3 and 30 days after axotomy. Stains include luxol fast blue (LFB) for myelin, SMI-31 for phosphorylated neurofilament, SMI-32 for hypo-phosphorylated neurofilament, and APP for amyloid pre-cursor protein. The location of the lesion in the left dorsal column is indicated by black arrows.

Figure 6.

Grayscale images of toluidine blue staining of the dorsal column WM of the rat spinal cord at 3 and 30 days after axotomy. Examples of irregularly shaped and enlarged axons (arrowheads), and collapsed, degenerating axons surrounded by unraveling myelin (arrows) are indicated. The scale bar indicates 10 μm.

DISCUSSION

Axon and myelin membranes present barriers to water diffusion, and diffusion properties measured perpendicular and parallel to WM fibers can provide information about the microstructural environment. Using conventional DWI analysis, this study found that D_∥ and FA are decreased and D_⊥ is increased in damaged spinal cord WM after dorsal root axotomy. These findings are in agreement with previous DTI studies of axonal injury, including dorsal root axotomy (36), traumatic injury to the mouse brain (38), spinal cord injury to the mouse spinal cord (39–41), and dorsal column transection in the rat spinal cord (42). DTI studies of EAE (39,43) and WM damage after retinal ischemic injury (3,44,45) have also reported a decrease of D_∥ and an increase in D_⊥.

This study found a change in several measures of perpendicular diffusion (increased D_⊥, FWHM_⊥ and RMSD_⊥ together with decreased PZERO_⊥ and KE_⊥) at 3 days post axotomy without a concomitant loss of LFB staining. This is in agreement with a recent DTI study (36), which included time points spanning from 16 hours to 30 days post axotomy and reported a significant increase in D_⊥ at 3 days post-axotomy while no significant decrease in LFB staining (quantified with optical-density measurements) was detected at day 3. As confirmation, Zhang et al. (36) also reported no change in antibody reactivity to CNPase (2'–3' cyclic nucleotide 3' phosphodiesterase), an alternative stain for myelin, at 3 days post axotomy. The observation of increased perpendicular diffusion early after injury with no noticeable change in LFB staining is in line with findings in previous DTI studies of Wallerian degeneration in the spinal cord. In a model of contusion spinal cord injury, D_⊥ was increased 7 days post-injury in both LFB-defined spared and demyelinated regions at the site of impact (39). Similarly, in a study of spinal cord injury in mice, D_⊥ was elevated at 3, 7 and 14 days post-injury in ventral and lateral WM both at and caudal to the site of injury (41). Notably, at 14 days post-injury LFB demonstrated demyelination at the injury impact site (with elevated D_⊥), however LFB staining caudal to the injury site was qualitatively similar to controls (in contrast to the elevated D_⊥ in this region) (41). One factor to consider, that may account for elevated perpendicular diffusion at 3 and 30 days post axotomy, is that irregularities in the myelin sheath (as shown in Figure 6) have been noted early after the onset of granular degeneration of the axon cytoskeleton and include: irregular folding and splitting of the myelin lamellae to form concentric rings around the degenerating axon, and contraction of the sheath concurrent with collapse and fragmentation of the axon (27,29,46). These morphological changes may not result in changes in LFB staining. Taken together, our findings of an elevated D_⊥ at 3 days post-axotomy suggest that perpendicular diffusion may be sensitive to a host of factors (e.g. changes in membrane permeability, axon and myelin morphology, and intra- and extra-cellular water populations) in addition to the prominent demyelination that occurs in late stages following dorsal root axotomy. In the future, studies that combine DTI, q-space imaging, and quantitative histology at multiple closely spaced time points may further clarify the causal relationship between myelin damage, myelin loss, and the increase in diffusion perpendicular to WM tracts.

The reduction in parallel diffusion (reflected in decreased D_∥, FWHM_∥, and RMSD_∥, together with increased PZERO_∥ and KE_∥) in lesions at 3 and 30 days post axotomy is likely due to additional barriers to parallel diffusion. These barriers may be imposed by axonal beading (formation of bulges along the length of the axon (47)), the fragmentation of the axon into short sections (myelin ovoids) encompassed by myelin and the accumulation and slow removal of axon and myelin debris (which can persist up to 90 days) in the dorsal root axotomy model (27–30). This interpretation is consistent with the increased staining for SMI-32 and APP (Figure 5), which indicates degeneration of the cytoskeleton and breakdown of fast-axon transport, respectively, and the abnormal axon and myelin morphology consistent with the formation of myelin ovoids (Figure 6). Previous DTI studies have also noted a decrease of D_∥ with increased APP and SMI-32 staining after axon injury (36,38,43,45). The emerging relationship between the breakdown of axon transport, accumulation of proteins (such as APP and degraded cytoskeleton components) and axonal organelles at localized sites, axonal bead formation, and Wallerian degeneration in several central nervous system disorders is covered in a recent compressive review (28). In related work, Monte-Carlo simulations of water diffusion showed that axon damage (modeled as periodic bulges along the length of impermeable cylinders) can cause a reduction in D_∥ and FA, and an increase in D_⊥ and KE_∥ (48,49). This suggests that axonal beading may play a prominent role in reducing parallel diffusion. In the future, more comprehensive and realistic models of tissue damage could be developed by incorporating both intra- and extra-axonal water, molecular exchange, relaxation, and biologically relevant geometries (49). Additionally, the interpretation of changes in parallel diffusion may benefit significantly from the recent elucidation of the spatiotemporal evolution of axon pathology by fluorescent microscopy studies (30,50).

Our study found a decrease of D_∥ at 3 days, which was maintained at 30 days post injury. This is in agreement with previous DTI studies of Wallerian degeneration that have reported that D_∥ is decreased after axon injury, including transient retinal ischemia in mice (3,44,45), dorsal root axotomy in the rat spinal cord (36), and spinal cord transection (42). While the initial decrease in D_∥ may be sensitive to axonal degeneration, a consistent level of reduced D_∥ during an extended period of axon and myelin debris removal is somewhat surprising. Notably, the temporal evolution of D_∥ in animal models which exhibit a breakdown of the blood-brain, or blood-spinal cord barrier after injury is quite different, owing perhaps to the effects of edema and macrophage infiltration which accelerate debris clearance. Compared to our findings of a maintained level of decreased D_∥, an initial decrease in D_∥ followed by a pseudo-normalization that remained below, or reached control levels has been reported after traumatic spinal cord injury (41) and traumatic brain injury (38), respectively. A decrease in D_∥, however, is not ubiquitous across different models of WM damage and changes in parallel diffusion are likely closely related to the specific pathology and the time after injury at which diffusion is measured for each disease process or injury model. Evidence of this is that a DTI study of myelin deficient rat spinal cords (51) has reported that both D_⊥ and D_∥ are increased relative to normal WM. Notably, an increase in D_∥ can also occur during tissue recovery as noted by Sun et al. (4), who reported a transient decrease of D_∥ in the corpus callosum of mice during cuprizone administration, followed by a normalization of D_∥ which persisted during the recovery phase. The need to reconcile these differences, find contrasts that are more sensitive to tissue damage, and understand how changes in diffusion properties correlate with microstructural changes motivates the performance of DWI experiments over a wide range of b-values and the utilization of analysis techniques, such as q-space analysis, that can quantify non-Gaussian diffusion behavior.

In this study, a prominent departure from mono-exponential signal attenuation was observed at high b-values (b > 2000 s/mm²) for perpendicular diffusion in both lesioned WM and uninjured WM (Figure 1B). The non-Gaussian diffusion in damaged WM 30 days post axotomy is notable and suggests that many barriers to perpendicular diffusion persist despite the effects of demyelination. This makes sense in view of the fact that myelin is not the sole contributor to restricted diffusion (52) and the vacuolation and astrogliosis (53) that occurs during the later stages of Wallerian degeneration may provide sufficient barriers to diffusion. Furthermore, non-Gaussian diffusion was also observed for parallel diffusion in GM, lesioned WM, and uninjured WM (Figure 1E). In principle, the signal attenuation for water diffusion along the length of healthy axons is expected to be mono-exponential as a function of the b-value due to the lack of structural barriers to water displacement in this orientation (52). However, the structural heterogeneity of WM fiber bundles over the size of an MRI voxel may provide sufficient barriers to parallel diffusion to result in non-Gaussian diffusion in healthy WM. This finding is consistent with previous reports of KE_∥ > 0 in healthy WM (Table 3).

Table 3.

Comparison of mean ($\stackrel{‒}{\mathrm{KE}}$) and directional kurtosis excess (KE_⊥ and KE_∥) values from DKI and q-space DWI studies of water diffusion in healthy gray matter and white matter.

Kurtosis Measure		Organism/Organ	Tissue Type	Study	Reference
Gray Matter
$\stackrel{‒}{\mathrm{KE}}$	0.30 ± 0.05	Human Brain	GM	Q-space	(25)
	0.51 ± 0.12	″	put	Q-space	(18)
	0.38 ± 0.05	″	put	DKI	(18)
	0.82 ± 0.03	″	Cortical GM	DKI	(34)
	0.74 ± 0.03	″	GM	DKI	(54)
	0.87 ± 0.03	Rat Brain	cpt	DKI	(56)
	0.93 ± 0.03	″	Ex vivo, cpt	DKI	(56)
	0.39 ± 0.08	Rat Spinal Cord	Ex vivo, GM	Q-space	This study
White Matter
$\stackrel{‒}{\mathrm{KE}}$	0.26 ± 0.04	Human Brain	cc	Q-space	(25)
	0.81 ± 0.12	″	deep WM	Q-space	(18)
	0.84 ± 0.03	″	deep WM	DKI	(18)
	0.65 ± 0.17	″	cc	Q-space	(18)
	0.72 ± 0.07	″	cc	DKI	(18)
	1.41 ± 0.11	″	frontal WM	DKI	(34)
	1.09 ± 0.01	″	Brain	DKI	(54)
	1.02 ± 0.03	Rat Brain	cc	DKI	(56)
	1.60 ± 0.10	″	Ex vivo, cc	DKI	(56)
	1.1 ± 0.1+	Rat Spinal Cord	Ex vivo, WM	DKI	(57)
	1.7 ± 0.1	″	Ex vivo, gracilis tract	Q-space	This study
KE⊥	0.59 ± 0.15	Human Brain	Optic radiation	Q-space	(25)
	1.55 ± 0.10	Rat Brain	cc	DKI	(56)
	1.94 ± 0.15	″	Ex vivo, cc	DKI	(56)
	1.5 ± 0.1+	″	cc	DKI	(55)
	11.4 ± 0.2	″	cc	DKI	(22)
	17 ± 1 and 55 ± 5*	Mouse Spinal Cord	Ex vivo, gracilis tract	Q-space	(24)
	9.51**	Rat Spinal Cord	Ex vivo, gracilis tract	Q-space	(23)
	2.1 ± 0.2+	″	Ex vivo, WM	DKI	(57)
	2.3 ± 0.2	″	Ex vivo, gracilis tract	Q-space	This study
KE∥	0.29 ± 0.09	Human Brain	Optic radiation	Q-space	(25)
	0.74 ± 0.03	Rat Brain	cc	DKI	(56)
	1.30 ± 0.06	″	Ex vivo, cc	DKI	(56)
	0.75 ± 0.1+	″	cc	DKI	(55)
	0.6 ± 0.1+	Rat Spinal Cord	Ex vivo, WM	DKI	(57)
	0.42 ± 0.07	″	Ex vivo, gracilis tract	Q-space	This study

Putamen (put), caudate and putatmen (cpt), corpus callosum (cc). KE values for cortical gray matter in the human brain (18), (34), and thalamus (54) are not listed. For this study, the average value across 6 rats (3 days post axotomy) is reported for ROIs in uninjured dorsal column WM and GM.

^*estimated from graph for cases satisfying narrow gradient pulse with and without truncation.

^**error estimate not provided.

⁺Estimated from graph.

In agreement with previous q-space DWI studies of rat spinal cord injury (11–14), this study found a significant increase in perpendicular diffusion in lesions compared to contralateral WM. Parallel q-space diffusion properties have been less well explored, possibly due to SNR considerations when using very high b-values. However, the findings that parallel diffusion is decreased (decreased FWHM_∥, RMSD_∥, increased PZERO_∥) agrees with a study of crush injury in the rat spinal cord (11) and EAE in the swine spinal cord (15). Our values for $\stackrel{‒}{\mathrm{KE}}$ in healthy GM (0.39 ± 0.08), together with $\stackrel{‒}{\mathrm{KE}}$ (1.7 ± 0.1), KE_⊥ (2.3 ± 0.2), and KE_∥ (0.42 ± 0.07) in healthy WM are in good agreement with previous q-space studies of the human brain in vivo (18,25), together with diffusion kurtosis imaging (DKI) studies of the human brain in vivo (18,25,34,54), the rat brain ex vivo (55,56), and the rat spinal cord ex vivo (57) (Table 3). DKI (34,54) is methodologically distinct from q-space DWI and can also be used to quantify the deviation from Gaussian diffusion, though is limited to intermediate b-values (< 2500 s/mm²). Notably, however, two recent q-space DWI studies in the rat (23) and mouse spinal cords (24) have reported significantly larger KE_⊥ values in spinal cord WM. This difference could, in part, be due to acquisition parameters and methodological considerations which are discussed in more detail in the technical considerations section of the current work.

Our findings of a significant decrease of KE_⊥ and increase of KE_∥ in response to WM damage in the dorsal root axotomy model can probably be explained by the loss of axon and/or myelin barriers to diffusion in the perpendicular direction and the introduction of additional barriers to diffusion in the parallel direction, respectively. One important finding of this q-space DWI study is the demonstration that directional KE measurements (i.e., KE_⊥ and KE_∥) are more sensitive to abnormal diffusion in damaged WM than $\stackrel{‒}{\mathrm{KE}}$ measurements, in line with results from a recent DKI study of EAE in the rat spinal cord (57). Though no significant change in $\stackrel{‒}{\mathrm{KE}}$ was measured in the current study, a decrease of $\stackrel{‒}{\mathrm{KE}}$ has been reported for multiple sclerosis lesions in the human brain (18).

One of the specific goals of this study was to compare the sensitivity of conventional DWI contrasts (computed at low b-values) and q-space contrasts to abnormal diffusion due to WM damage at the early and later stages of Wallerian degeneration (Table 2). An important finding of the current study was that, in the case of parallel diffusion, the only contrast to exhibit a statistically significant difference from 3 to 30 days was FWHM_∥ (decreased by 24%, p = 0.002). By comparison, D_∥ decreased at 3 days and remained unchanged at 30 days, in agreement with the findings from a recent DTI study (36). This suggests that high b-value q-space DWI may be more sensitive to the evolving morphological changes that occur during the time course of Wallerian degeneration and their effect on diffusion parallel to the WM fiber orientation. The decrease of FWHM_∥ suggests a more restricted diffusion environment at 30 days compared to 3 days, which may be due to the formation of degenerating tissue characterized by vacuolation and astrogliosis (53). In the future, q-space and DTI experiments that include later time points (perhaps up to 90 days in the case of dorsal root axotomy (27,29)) may further elucidate the sensitivity of parallel diffusion properties to the clearance of myelin and axon debris. Additionally, while the PZERO, FWHM and RMSD can be expressed in terms of each other for a Gaussian PDF, this is not the case, in general, for non-Gaussian PDFs. In this study, PZERO, FWHM and RMSD exhibited significantly different sensitivities to WM damage in the perpendicular and parallel orientations. This is well illustrated by the fact that a large increase of RMSD_⊥ was measured from 3 to 30 days post axotomy, while no significant change in FWHM_⊥ was noted. Interestingly, FWHM_∥ was sensitive to the change in abnormal diffusion from 3 to 30 days, while no significant change in RMSD_∥ was noted. This observation may be due to different sensitivities of FWHM and RMSD to the fast and slow diffusion components. It is therefore a recommendation of this study that both RMSD and FWHM measurements are reported in q-space DWI studies as the information provided by these contrasts could be different.

Technical Considerations

Q-space analysis offers two key advantages compared to conventional DWI analysis: sensitivity to both the fast and slow diffusion components, and the ability to quantify non-Gaussian diffusion. Though this new information comes with the cost of additional scan time and SNR constraints at high b-values, which may prohibit some in vivo applications, it is encouraging to note that q-space DWI of multiple sclerosis is feasible in the human brain (16–18) and spinal cord (19). An additional benefit of q-space analysis is that measurements of restricted diffusion can be combined with tissue models to extract morphological properties such as axon diameter, together with intra and extra-cellular water fractions (23,24,32). Though careful histological validation is needed, this approach may have the potential to elucidate tissue microstructure at the sub-voxel level.

A necessary consideration is that acquisition parameters (which are also relevant for diffusion metrics obtained with DTI) can affect the size and shape of the PDF. Important factors include the duration of the diffusion weighting gradients (δ), the prescribed diffusion time (t_dif), and the spatial resolution of the PDF (Δx) which is determined by:

$${\Delta }_x=1∕\left(N\Delta q\right)=1∕q_{\max }$$ [11]

where N is the number of q-values acquired in steps of Δq up to q_max. Simulation-based (58,59) and experimental studies of restricted diffusion in microcapillary tubes (60,61), together with imaging studies of the rat sciatic nerve (16) and spinal cord (62) have shown that, at a fixed q_max, long diffusion gradient pulses (i.e. violation of the short gradient pulse (SGP) criteria, δ << Δ) produce PDFs that are narrower (and correspondingly taller) than would be expected based on the size of the confining geometry. Despite this limitation, the magnitude of the bias is small (FWHM decreased by a factor of two when δ was increased from 4.5 to 72ms in the sciatic nerve (16), and decreased by 10% when δ was increased from 2 to 20ms in a study of the rat spinal cord (62)). The time that molecules have to explore their local environment also has a strong effect on measured diffusion properties and longer diffusion times have recently been shown to enhance the contrast between GM and WM on q-space contrast maps of perpendicular diffusion (62). Based on the results presented by Bar-Shir et al. (61) who investigated the effect of δ and Δ/δ, the parameters used in the current study (δ =3ms, Δ =80ms) are sufficient to ensure accurate displacement measurements and good contrast between GM and WM.

Despite this improved understanding, it is not clear how violation of the SGP criteria will affect KE measurements. One possibility is that the overemphasis of the slow diffusion component may produce elevated KE values that suggest a larger degree of non-Gaussian diffusion. A separate issue is that KE is expected to be particularly sensitive to the overall shape of the PDF, and may be prone to bias when the calculated PDF fails to adequately represent the true diffusion process. This is relevant when Δx is too large and the signal attenuation fails to decay to approximately 1 to 4% of the original value. In this instance the PDF contains few points and erroneously high KE values can be obtained, as shown by a recent simulation study (63). While linear interpolation of PDFs with large Δx may provide reasonable displacement values, this approach may not be suitable for the accurate quantification of KE. To combat this, the signal attenuation can be zero-filled or extrapolated prior to the FT in order to decrease Δx. However, zero-filling can introduce ringing artifacts into the PDF which may further complicate the calculation of KE. To address this, Latt et al. (64) have suggested the use of a cut-off value whereby the outer portions of the PDF (<1% of PZERO) are set to zero to prevent erroneously high and noisy KE values. Notably, the current study assumed a bi-exponential fit to the signal attenuation and computed KE from the analytical Fourier transform of the fit components to avoid the effects of Δx and ringing artifacts. Overall, the inter-study comparison of KE values and interpretation of KE in healthy and diseased neural tissue would both benefit from a detailed assessment of the effect of acquisition parameters and signal processing procedures. Research on this topic, building on the preliminary findings presented in (63), is currently under way.

CONCLUSION

Q-space analysis provides a more comprehensive assessment of WM damage and offers several key advantages over conventional DWI analysis including the measurement of the kurtosis excess of diffusion and the quantification of both the fast and slow diffusion components. While the initial increase in perpendicular diffusion was not specific to demyelination, the decrease in parallel diffusion may be a specific marker for initial axon damage. Finally, for non-Gaussian behavior, the relationship between RMSD and FWHM is not straightforward and it is recommended that these PDF-based contrasts are reported separately.