A new one-dimensional radiative equilibrium model for investigating atmospheric radiation entropy flux

Abstract

A new one-dimensional radiative equilibrium model is built to analytically evaluate the vertical profile of the Earth's atmospheric radiation entropy flux under the assumption that atmospheric longwave radiation emission behaves as a greybody and shortwave radiation as a diluted blackbody. Results show that both the atmospheric shortwave and net longwave radiation entropy fluxes increase with altitude, and the latter is about one order in magnitude greater than the former. The vertical profile of the atmospheric net radiation entropy flux follows approximately that of the atmospheric net longwave radiation entropy flux. Sensitivity study further reveals that a ‘darker’ atmosphere with a larger overall atmospheric longwave optical depth exhibits a smaller net radiation entropy flux at all altitudes, suggesting an intrinsic connection between the atmospheric net radiation entropy flux and the overall atmospheric longwave optical depth. These results indicate that the overall strength of the atmospheric irreversible processes at all altitudes as determined by the corresponding atmospheric net entropy flux is closely related to the amount of greenhouse gases in the atmosphere.

1. Introduction

Climate models built on the principles of energy, momentum and mass balances have been extensively used to study the Earth's climate and climate change, ranging from simple energy balance models to state-of-the-science global circulation models. Although these mainstream models have made great contributions to develop climate theories and to improve our understanding of the Earth's climate system, much remains elusive, for example, the large uncertainties in climate sensitivity and feedbacks (e.g. IPCC 2007; Schwartz et al. 2007; a comment by Kerr 2007; Kiehl 2007; Roe & Baker 2007; Knutti 2008; Sanderson et al. 2008; Schwartz 2008). To enhance the ability of climate models in quantifying and projecting the Earth's climate change, integration of additional constraint(s) into the building blocks of climate models seems necessary.

The Earth system as a whole is virtually driven and maintained by the radiation exchange between the Earth system and space (e.g. Lesins 1990; Stephens & O'Brien 1993; Wu & Liu in press). The Earth system absorbs solar (shortwave, SW hereafter) radiation energy after reflecting about 30 per cent incident solar radiation back to space, converts it into other energy forms through various irreversible processes, and re-emits terrestrial (i.e. longwave or infrared; LW hereafter) radiation back to space. Under a steady state, the absorbed SW radiation energy is balanced with the emitted LW radiation energy. However, the emitted LW radiation has much greater entropy than the absorbed SW counterpart because of the conversion of the high-energy SW photons from a small solid angle into the low-energy LW photons nearly isotropically (e.g. Lesins 1990; Stephens & O'Brien 1993; Wu & Liu in press). The resulting negative net entropy flux from the radiation exchange between the Earth system and space constrains the internal entropy production rate of the Earth system. Thus, it appears natural and necessary to consider the second law of thermodynamics (e.g. Planck 1922; Prigogine 1980; Rubi 2008) as an additional constraint to the Earth system processes (e.g. Kleidon & Lorenz 2005; Whitfield 2005).

Application of the second law of thermodynamics, especially of entropy-related extremal principles, to the Earth's climate study has been explored since the 1970s (e.g. Paltridge 1975, 1978; Golitsyn & Mokhov 1978; Nicolis & Nicolis 1980; Grassl 1981; Mobbs 1982; Essex 1984; Peixoto et al. 1991; Stephens & O'Brien 1993; Goody 2000; Ozawa et al. 2003; Pujol 2003; Paltridge et al. 2007; Pauluis et al. 2008). However, theoretical development along this line is still in an infant stage. One central question lies in the role of radiation entropy in determining the Earth's climate and how to accurately calculate the Earth's internal entropy production (e.g. Essex 1984; Lesins 1990; Peixoto et al. 1991; Stephens & O'Brien 1993; Pelkowski 1994; Goody & Abdou 1996; Goody 2000; Ozawa et al. 2003). We have recently reviewed the major existing expressions for calculating radiation entropy flux scattered in different disciplines and developed for different purposes, examined their applicabilities for calculating the Earth's radiation entropy flux, and identified the most accurate expressions under specific conditions as applied to the Earth system as a whole (Wu & Liu in press).

Furthermore, the previous studies have been mainly concerned about the internal entropy production rate of the Earth system quantified by the Earth's net radiation entropy flux when the Earth system is treated as a whole (e.g. Stephens & O'Brien 1993; Wu & Liu in press). The detailed vertical profile of the Earth's atmospheric radiation entropy flux and its variation with atmospheric conditions such as atmospheric opacity have rarely been investigated, with only two studies to the best of the authors' knowledge. Li and co-workers (1994) used a Canadian Climate Center one-dimensional radiative-convective model to investigate the vertical profile of the atmospheric radiation entropy flux. But, the atmospheric LW radiation entropy transfer equation (eqn (17) in Li et al. 1994) were derived under the assumption that the atmospheric emission source is a blackbody, which is obviously an over-simplification. Moreover, the atmospheric LW radiation entropy transfer equation is linearly parallel to the atmospheric LW radiation energy transfer equation (eqn (15) in Li et al. 1994). In deriving their atmospheric LW radiation entropy transfer equation, the atmospheric LW radiation energy flux was assumed to be equal to the energy flux of the atmospheric blackbody emission source (eqn (16) in Li et al. 1994). Although Li and co-workers (1994) applied this assumption only for simplifying radiation entropy calculation, this assumption suggests a zero divergence of the atmospheric LW radiation energy flux, or a constant atmospheric LW radiation energy flux at all altitudes when applying the assumption to the atmospheric LW radiation energy transfer equation (eqn (15) in Li et al. 1994). Thus, the energy flux of the atmospheric blackbody emission is a constant at all altitudes, which does not seem consistent with the vertical profile of decreasing atmospheric temperatures with altitudes. Pelkowski (1994) investigated the atmospheric LW radiation entropy flux at the top and bottom of the atmosphere for different atmospheric LW optical depths, wherein the Earth's surface is assumed to be a blackbody that absorbs all the incident solar radiation entering the Earth system (i.e. atmospheric SW absorption and scattering are completely neglected within the atmosphere, so that the divergence of atmospheric radiative flux involves only LW radiation, SW scattering occurring only at the top of the atmosphere). He used the assumption of the atmospheric LW radiation emission source being a blackbody as in Li and co-workers (1994) in calculating the atmospheric LW radiation energy flux. Substitution of the definition of monochromatic radiation temperature, that is, a ratio of the corresponding spectral radiation energy flux to spectral radiation entropy flux, into the radiation energy transfer equation leads to a complicated radiation entropy transfer equation. By further ignoring some complicated terms (he called the terms as ‘the anisotropic part’) in the radiation entropy transfer equation, he arrived at a radiation entropy transfer equation (eqn (35) in Pelkowski 1994) for calculating the atmospheric LW radiation entropy flux, exactly in the same form as eqn (17) in Li et al. (1994). Moreover, the vertical profile of the atmospheric temperature needs to be given beforehand in his calculation, and four different kinds of vertical atmospheric temperature profiles were examined, including one from a radiative equilibrium model. However, no discussion was given on the detailed vertical profile of the atmospheric radiation entropy flux within the atmosphere.

In this paper, we further investigate the vertical profile of the Earth's atmospheric radiation entropy flux by considering the atmospheric emission source as a greybody, instead of a blackbody, and using the most accurate approximate expressions available for calculating the Earth's radiation entropy flux as identified in Wu & Liu (in press). A new radiative equilibrium model is formulated that permits analytical evaluation of the vertical profile of the atmospheric radiation entropy flux in addition to the vertical profiles of the atmospheric temperature and radiation energy flux. Especially, the introduction of the effective atmospheric LW emissivity and the application of a diluted blackbody to SW radiation allows us to examine the relationship between the vertical profile of the Earth's atmospheric radiation entropy flux and atmospheric opacity. The theoretical framework of the new model is described in §2. The results derived from the model are analysed in detail in §3. Concluding remarks are summarized in §4.

2. Theoretical framework

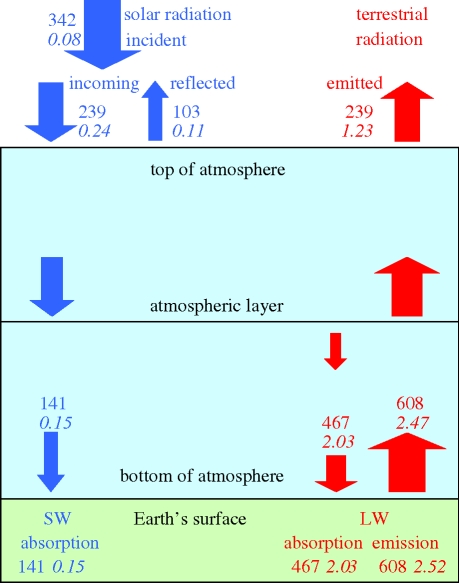
We consider a one-dimensional steady-state Earth system that comprises a grey atmosphere with a constant effective atmospheric LW emissivity and a Lambertian surface at each altitude for atmospheric SW radiation processes (i.e. the atmospheric SW energy flux at a Lambertian surface is the same in all directions). The Earth's surface is assumed to be a blackbody. The system is in radiative equilibrium, i.e. no vertical or horizontal heat transfer by conduction, air motions or latent heat release is involved. Thus, the net radiation energy flux is equal to zero at all altitudes. Moreover, for the sake of simplicity, SW radiation is treated as a diluted blackbody with the reflection of incident solar radiation at the top of the atmosphere (TOA) equal to TOA SW albedo; SW scattering processes occurring in the atmosphere are not treated explicitly. This assumption commonly used in previous vertical one-dimensional models (e.g. Ozawa & Ohmura 1997; Pujol & Fort 2002; Pujol 2003) allows us to concern only about the net atmospheric SW radiation in this model. The philosophy for choosing such an idealized Earth system is that it retains enough physics and can still be described by a simple one-dimensional vertical climate model to allow analytical evaluation of the vertical profiles of temperature, radiation energy and entropy fluxes. Figure 1 illustrates this simple model schematically with the radiation energy and entropy fluxes at the top and bottom of the atmosphere and at the Earth's surface, which will be discussed in §3.

Figure 1.

An illustration of the one-dimensional grey-atmosphere radiative equilibrium model. Blue arrows represent solar radiation; red arrows represent atmospheric LW radiation. The line thickness reflects the corresponding magnitude qualitatively. Note that the numbers denote energy fluxes (regular) in W m⁻² and entropy fluxes (italics) in W m⁻² K⁻¹, obtained from this study as the overall atmospheric LW optical depth τ_* is 3.0 except that the entropy flux 0.08 W m⁻² K⁻¹ of incident solar radiation is from Wu & Liu (in press). Note also that TOA SW ‘incoming’ means ‘incoming the Earth system’.

(a). Equations for radiation energy fluxes and temperature profile

Radiative transfer equation has been extensively studied for solving the problems of the atmospheric radiative energy transfer (e.g. Goody & Yung 1989; Lenoble 1993; Liou 2002). For the atmospheric LW radiation, we derive the equations of governing the atmospheric LW radiation energy flux by applying Eddington's approximation (e.g. eqn (6.5.21) in Liou (2002)) into the radiative transfer equation (e.g. eqn (7.4.1) in Liou (2002)) (note that here the atmospheric LW emission uses greybody emission instead of blackbody emission), and then performing integration over the range of zenith angles from 0 to π after multiplying by sine or cosine of zenith angle, namely,

2.1

and

2.2

where ɛ represents the effective atmospheric LW emissivity; τ represents the atmospheric LW optical depth at any altitude; ɛB(τ) represents the atmospheric LW emission [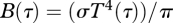, σ = 5.670 × 10⁻⁸ W m⁻² K⁻⁴ is the Stefan-Boltzmann constant and T(τ) is the atmospheric temperature]; I₀(τ) and I₁(τ) are two basic components that determine the upward and downward atmospheric LW radiation energy fluxes coming from Eddington's approximation. Similar equations can also be found elsewhere (e.g. eqns (6a) and (6b) in Pujol (2003)). It should be emphasized that, as will be shown later, the introduction of ɛ eliminates the problem of surface thermal discontinuity that has long bothered similar one-dimensional vertical climate models (see relevant discussions in Pujol & Fort 2002), and allows for examination of the effect of greenhouse gases on the vertical atmospheric structures (temperature, radiation energy and entropy fluxes) by combining with the dilution application for SW radiation. These unique features separate our model from the existing ones.

The expressions of the upward [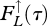] and downward [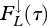] atmospheric LW radiation energy fluxes can be directly obtained by performing integrations over the corresponding solid angles to Eddington's approximation after multiplying by cosine of zenith angle,

2.3

and

2.4

Note that the upward integration spans the azimuth angle from 0 to 2π and the zenith angle from 0 to 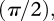while the azimuth angle is from 0 to 2π and the zenith angle is from π to 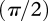for downward integration. Similar equations can be found in some references (e.g. eqns (3a) and (3b) in Pujol (2003)).

The atmospheric SW radiation energy flux obeys Beer's law of absorption such that (Goody & Yung 1989; Ozawa & Ohmura 1997),

2.5

where τ^S represents the atmospheric SW optical depth at any altitude; F_S(0) and 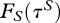represent the atmospheric SW radiation energy flux at TOA and at any altitude, respectively. Note that τ^S in general represents an effective atmospheric SW optical depth that implicitly contains the overall result of all the atmospheric SW absorption and scattering processes within the atmosphere.

Moreover, the atmospheric SW optical depth τ^S is assumed to be linearly related to the atmospheric LW optical depth τ, i.e.

2.6

where a₀ is an empirical constant. The physical justification for equation (2.6) is that both the atmospheric SW and LW optical depths are heavily affected by the amount of water vapour. Based on equation (2.6), equation (2.5) can be re-written as

2.7

The conservation of energy dictates that the atmospheric net SW radiation energy flux is balanced with the atmospheric net LW radiation energy flux at all altitudes, i.e.

2.8

Substitution of equations (2.3), (2.4) and (2.7) into equation (2.8) yields

2.9

At TOA, the incoming SW radiation energy flux has to be balanced with the outgoing LW radiation energy flux (i.e. no TOA incoming LW radiation energy flux). Mathematically, this TOA boundary condition can be written as

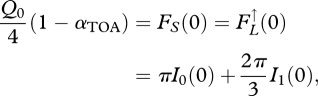2.10

where Q₀ is the solar constant and α_TOA is TOA SW albedo.

The analytical solutions of the set of equations (2.1), (2.2) and (2.9) with the TOA boundary condition (2.10) are

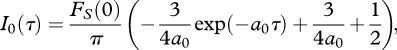2.11

2.12

and

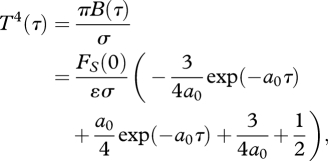2.13

The effective atmospheric LW emissivity ɛ in the above solution can be further related to the boundary condition at the Earth's surface, which forces the surface air temperature T(τ_*) equal to the Earth's surface temperature T_srf (τ_* is the overall atmospheric LW optical depth). Mathematically, this surface boundary condition is described by

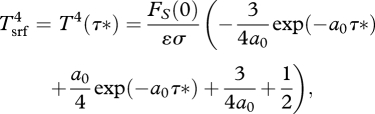2.14a

or

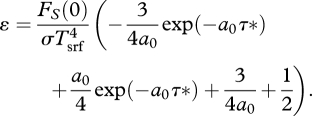2.14b

The Earth's surface temperature T_srf can be further determined according to the energy balance at the Earth's surface, i.e. the emissive energy flux 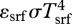is balanced with the absorbed SW radiation energy flux F_S(τ_*) and LW radiation energy flux 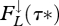emitted by surface air,

2.15a

or

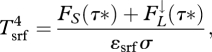2.15b

where ɛ_srf is the Earth's surface emissivity (ɛ_srf = 1.0 if the Earth's surface is assumed to be a blackbody as in this study). The absorbed SW radiation energy flux F_S(τ_*) at the Earth's surface can be directly calculated according to equation (2.7). The absorbed LW radiation energy flux 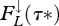at the Earth's surface can be calculated by substituting the expressions of I₀(τ) (equation (2.11)) and I₁(τ) (equation (2.12)) into equation (2.4).

As will be shown in §3, one advantage of this new model, compared with other one-dimensional vertical climate models, is that it eliminates the surface thermal discontinuity problem. In addition, for any given overall atmospheric LW optical depth τ_* as well as the solar constant Q₀, TOA SW albedo α_TOA and the constant a₀ in equation (2.6), the atmospheric temperature, radiation energy flux and the corresponding effective atmospheric LW emissivity can be readily obtained in simple analytical forms. The analytical formulation in turn provides clear physical insight into the issues in question.

(b). Equations for radiation entropy fluxes

Atmospheric LW and SW radiation processes both do not follow the well-established laws for blackbody radiation. Thus, the calculations of the atmospheric LW and SW radiation entropy fluxes are much more complicated than that of blackbody radiation entropy flux. Methodology in calculation of non-blackbody radiation entropy flux has attracted much attention of many different fields (such as Engineering or the Earth Sciences) for several decades and various expressions have been developed (e.g. Petela 1964, 2003; Landsberg & Tonge 1979; Stephens & O'Brien 1993; Wright et al. 2001; Zhang & Basu 2007). We have recently examined the performance of major analytical expressions for calculating radiation entropy flux and the associated approximations (Wu & Liu in press), finding that the expression proposed by Wright et al. (2001) exhibits the best overall performance among all the approximate expressions in the calculation of the Earth's LW radiation entropy flux under the assumption that the Earth's LW radiation emission behaves as a greybody. The approximate expression developed by Stephens & O'Brien (1993) was found to be one of the most accurate approximate expressions in the calculation of the Earth's SW radiation entropy flux under the assumption that the Earth's reflected SW radiation behaves as a diluted blackbody. Detailed discussions on the similarity and difference between greybody and diluted blackbody are referred to Wu & Liu (in press). Here we apply the approximate expressions by Wright et al. (2001) and by Stephens & O'Brien (1993) to the one-dimensional Earth system to derive the expressions for evaluating the vertical profiles of the atmospheric LW or SW radiation entropy fluxes, respectively.

For the atmospheric LW radiation entropy flux, we know that the atmospheric net LW radiation entropy flux at each altitude is equal to the summation of the corresponding upward and downward atmospheric LW radiation entropy fluxes, namely,

2.16

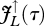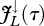where and represent the magnitudes of the upward and downward atmospheric LW radiation entropy fluxes, respectively.

According to Planck's radiation theory (Planck 1913), if one knows the upward [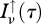] and downward [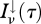] atmospheric LW spectral radiation energy fluxes, the upward 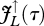and downward 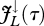atmospheric LW radiation entropy fluxes can be calculated by using the Planck's expression of the spectral radiation entropy flux (as a function of the corresponding spectral radiation energy flux, e.g. Planck (1913) or Wu & Liu (in press)) and then conducting the integration over the effective solid angle and over all frequencies (discussion and demonstration about generalizing the Planck's expression to non-blackbody radiation can be found in Wu & Liu (in press)),

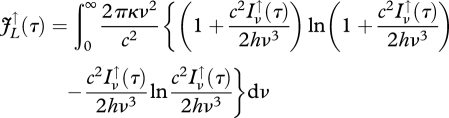2.17

and

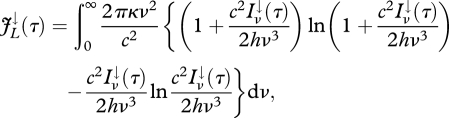2.18

where h, c, κ and ν are Planck's constant 6.626 × 10⁻³⁴ J s, speed of light in vacuum 2.9979 × 10⁸ m s⁻¹, Boltzmann constant 1.381 × 10⁻²³ J K⁻¹ and frequency, respectively. The integration involved in these two equations is generally too complicated to render analytical solutions, and adequate approximations are desirable. One such approximation was proposed by Wright and co-workers (2001) for greybody radiation (see also Wu & Liu in press). Application of this approximation simplifies equations (2.17) and (2.18) to

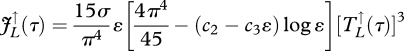2.19

and

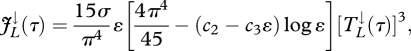2.20

where parameters c₂ = 2.336 and c₃=0.260; 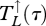and 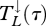denote the equivalent emissive temperatures of the upward and downward atmospheric LW radiation energy fluxes, respectively. The two equivalent emissive temperatures 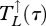and 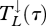can be determined from the corresponding radiation energy fluxes using the following equations:

2.21

and

2.22

These equations physically mean that the upward [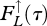] and downward [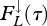] atmospheric LW radiation energy fluxes are equivalent to the same energy fluxes from grey atmospheric emission. Thus, 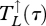and 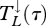are explicitly determined based on equations (2.21) and (2.22) combined with the analytical solutions of the upward [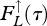] and downward [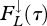] atmospheric LW radiation energy fluxes and the effective atmospheric LW emissivity [ɛ] from §2(a). Note that, Ω^↑ and Ω^↓ represent the ranges of upward and downward solid angles (upward Ω^↑: azimuth angle from 0 to 2π and zenith angle from 0 to 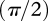; downward Ω^↓: azimuth angle from 0 to 2π and zenith angle from π to 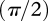). Substitution of equations (2.19) and (2.20) into equation (2.16) leads to the equation that describes the vertical profile of the atmospheric net LW radiation entropy flux. Also, it is readily shown that the atmospheric temperature given by equation (2.13) is related to 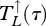and 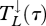by using equations (2.7), (2.8), (2.21) and (2.22)

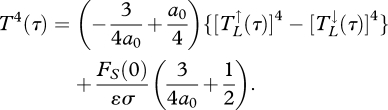2.23

The equation for calculating the atmospheric SW radiation entropy flux can be formulated by applying the SW radiation assumption of a diluted blackbody to each altitude or optical depth τ as follows. First, the atmospheric SW radiation entropy flux J_S(τ) at any altitude can be written as an integration of the atmospheric SW spectral radiation entropy flux, which is a function of the corresponding spectral energy flux, 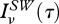, based on the generalized Planck's expression of the spectral radiation entropy flux for a diluted blackbody (e.g. Wu & Liu in press)

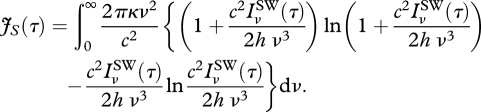2.24

Then, the corresponding atmospheric SW spectral radiation energy flux 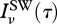under the Lambertian assumption is further calculated by

2.25

where 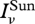is the spectral radiation energy flux of incident solar radiation per unit solid angle per unit frequency (W m⁻² sr⁻¹ s), and δ(τ) is the dilution factor for the atmospheric SW at each altitude or optical depth τ. By substituting equation (2.25) into equation (2.24) and then applying the approximate expression derived by Stephens & O'Brien (1993) (see also Wu & Liu in press), equation (2.24) can be simplified as

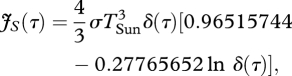2.26

where T_Sun is the Sun's effective emissive temperature. It is evident from this equation that the key to calculating the atmospheric SW radiation entropy flux J_S(τ) at each altitude is to determine the corresponding atmospheric dilution factor δ(τ).

Under the assumption of a Lambertian surface at each altitude, the net solar energy flowing into the atmospheric layer at optical depth τ can be written as

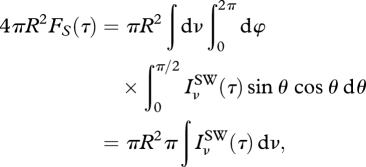2.27

where R is the corresponding spherical radius, and F_S(τ) is the spherical average atmospheric SW radiation energy flux. Substitution of equation (2.25) into equation (2.27) leads to

2.28

Moreover, the incident solar radiation energy flux at TOA can be expressed as

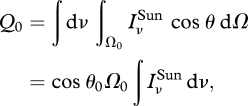2.29

where cos θ₀ = 0.25 is the average cosine solar zenith angle and Ω₀ = 67.7 × 10⁻⁶ sr is the solar solid angle to the Earth. Comparison of equations (2.28) and (2.29) leads to

2.30

Accordingly, the dilution factor δ(τ) is given by

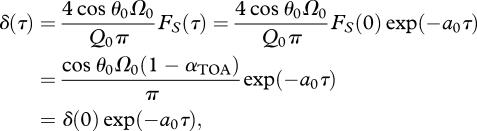2.31a

with

2.31b

where δ(0) denotes the dilution factor at TOA (i.e. τ = 0). Note that, the TOA atmospheric SW radiation energy flux F_S(0) = (1 − α_TOA)Q₀/4 is used in the derivation of the third equality of equation (2.31a). At the Earth's surface (i.e. τ = τ_*), we have (by using equation (2.31a))

2.31c

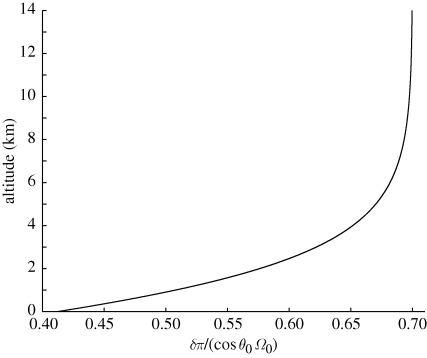
Equation (2.31a) indicates that the dilution factor decreases from TOA [δ(0)] to the Earth's surface [δ(τ_*)] (see also figure 2). The decrease of the dilution factor from TOA to the Earth's surface reflects the continuous dilution of TOA incoming solar radiation at every altitude as described by Beer's law of absorption. Substitution of equation (2.31a) into equation (2.26) yields the final expression for describing the vertical profile of the atmospheric SW radiation entropy flux,

2.32

Figure 2.

Vertical profile of the atmospheric dilution function δ after multiplying by the scaling factor π/(cos θ₀ Ω₀). The average cosine solar zenith angle cos θ₀ = 0.25 and the solar solid angle to the Earth Ω₀ = 67.7 × 10⁻⁶ sr.

It is worth mentioning that the atmospheric LW optical depth τ can be related to the atmospheric height z by

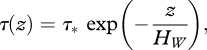2.33

where H_W represents a typical scale height of the atmosphere, and is approximately 2000 m for atmospheric water vapour. Note that this expression, which assumes that τ is mainly affected by the atmospheric water vapour, has been widely used for the Earth's climate modelling (e.g. Goody & Yung 1989; Pelkowski 1994; Ozawa & Ohmura 1997; Pujol & Fort 2002).

3. Results

According to the derivation presented in §2, the vertical profiles of atmospheric structures (temperature, radiation energy and entropy fluxes) can be evaluated analytically when the following inputs are known: the solar constant Q₀, TOA SW albedo α_TOA, Sun's temperature T_Sun, the empirical constant a₀ and the overall LW optical depth τ_*. Note that the empirical constant a₀ has been often determined by the overall atmospheric SW () and LW (τ_*) optical depths according to equation (2.6): 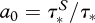, with 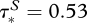based on the analysis of the measurements of the atmospheric SW radiation energy fluxes at the Earth's surface and at TOA by using Beer's law of absorption (Ozawa & Ohmura 1997). In the following examples, we take Q₀ = 1367 W m⁻², T_Sun = 5779 K, 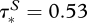, and α_TOA = 0.30 as constants, focusing on the effect of varying τ_*.

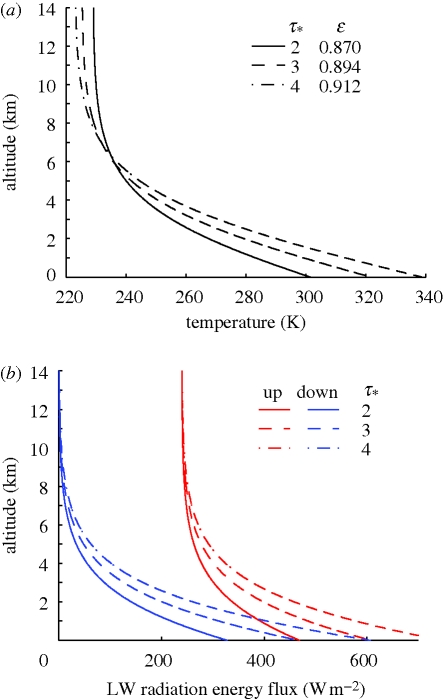
The fidelity of the new model is first examined from the obtained vertical structures of the atmospheric temperature and radiation energy flux. Figure 3a shows the vertical profile of the atmospheric temperature as the overall atmospheric LW optical depth τ_* equals 2.0, 3.0 or 4.0, respectively. Evidently, the atmospheric temperature decreases with altitude (faster in the lower troposphere and much slower in the upper troposphere). Furthermore, when the overall atmospheric LW optical depth τ_* increases, the atmospheric temperature increases fast in the lower troposphere but decreases slowly in the upper troposphere. The obtained effective atmospheric LW emissivity is 0.870, 0.894 or 0.912, when τ_* is 2.0, 3.0 or 4.0, respectively. It implies that a larger overall atmospheric LW optical depth τ_* corresponds to a larger effective atmospheric LW emissivity, i.e. a darker atmosphere in terms of atmospheric LW radiation emission. As a consequence, a darker atmosphere traps more heat (leading to warmer temperature) in the lower troposphere (this characteristic was also captured by Ozawa & Ohmura (1997)), but traps less heat (leading to cooler temperature) in the upper troposphere. However, both upward and downward atmospheric LW radiation energy fluxes increase at all altitudes (especially in the lower troposphere), when the overall atmospheric darkness increases (figure 3b). These results indicate that despite its simplicity, the new model yields the basic vertical atmospheric structures reasonably well, and more importantly, removes the problem of the surface thermal discontinuity that has long bothered other similar models.

Figure 3.

Vertical profiles of the atmospheric temperature (a) and LW radiation energy fluxes (b) when the overall atmospheric LW optical depth τ_* is 2.0, 3.0 or 4.0. The Greek symbol ɛ represents the effective atmospheric LW emissivity, being a constant for the whole atmospheric column.

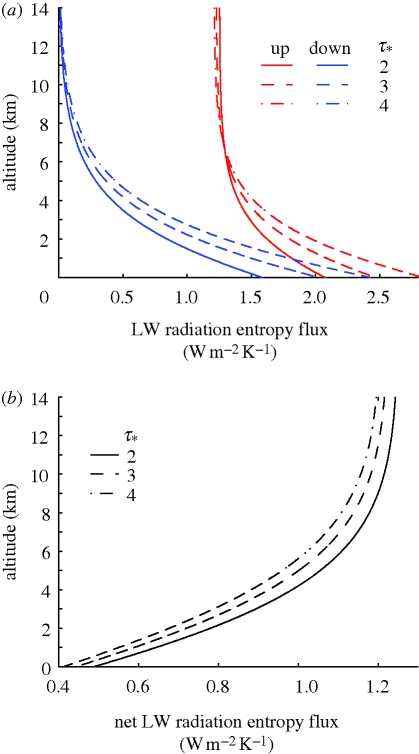
Figure 4a shows the upward and downward atmospheric LW radiation entropy fluxes. Like the upward and downward atmospheric LW radiation energy fluxes (figure 3b), both upward and downward atmospheric LW radiation entropy fluxes decrease with altitude. The basic structures are qualitatively similar to those reported by Li and co-workers (1994, fig. 1). The upward atmospheric LW radiation entropy flux at the Earth's surface encloses the result obtained by Li and co-workers (1994), with the result by Li and co-workers (1994) falling within the present results of τ_* = 2.0 and 3.0. However, the upward atmospheric LW radiation entropy flux at TOA (being 1.25, 1.23 or 1.21 W m⁻² K⁻¹ as τ_* is 2.0, 3.0 or 4.0, respectively) is much smaller than approximately 1.75 W m⁻² K⁻¹ obtained by Li and co-workers (1994). The downward atmospheric LW radiation entropy flux when τ_* = 3.0 presents almost the same pattern as that obtained by Li and co-workers (1994). Notice that, although the magnitudes of the upward atmospheric LW radiation entropy flux at TOA are much smaller than that obtained by Li and co-workers (1994), they are close to 1.22 W m⁻² K⁻¹ reported in Pelkowski (1994, table 2) when a vertical profile of the atmospheric temperature from a radiative equilibrium model was applied.

Figure 4.

Vertical profiles of the upward or downward (a), and net (b) atmospheric LW radiation entropy fluxes, when the overall atmospheric LW optical depth τ_* is 2.0, 3.0 or 4.0.

Moreover, like the energy flux, a darker atmosphere yields greater upward and downward atmospheric LW radiation entropy fluxes at almost all altitudes, especially in the lower troposphere (figure 4a). The only exception is that there is a tiny decrease of the upward atmospheric LW radiation entropy flux near TOA. A similar tendency was also found in Pelkowski (1994, table 2). Clearly, the magnitude of the increase of the downward atmospheric LW radiation entropy flux at any altitude is greater than that of the upward counterpart, corresponding to the increase of the overall atmosphere LW darkness (i.e. LW optical depth τ_*). As a consequence, the atmospheric net LW radiation entropy flux decreases at all altitudes as the overall atmosphere LW optical depth increases. This characteristic at TOA and at the Earth's surface was also captured by Pelkowski (1994, table 2) although the trend shown in his table 2 is much less. The result indicates that the atmospheric net LW radiation entropy flux is intrinsically connected with the overall atmospheric LW optical depth τ_*.

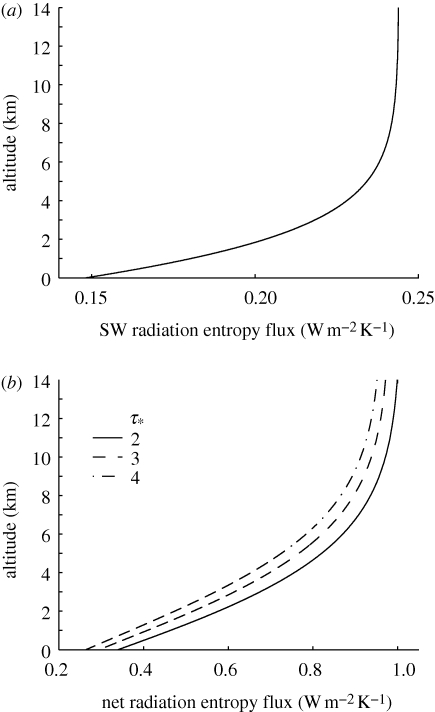
Figure 5a shows the vertical profile of the atmospheric SW radiation entropy flux. It can be seen that the atmospheric SW radiation entropy flux increases with altitude as a direct result of the increasing atmospheric SW radiation energy flux with altitude, according to Planck's radiation theory (see equation (2.24) or (2.26)). The atmospheric SW radiation entropy flux is one order in magnitude smaller than the corresponding atmospheric net LW radiation entropy flux. The atmospheric SW radiation entropy flux at TOA is equal to 0.24 W m⁻² K⁻¹.

Figure 5.

Vertical profiles of the atmospheric SW (a) and net (b) radiation entropy fluxes when the overall atmospheric LW optical depth τ_* is 2.0, 3.0 or 4.0.

Finally, the atmospheric net radiation entropy flux at each altitude can be obtained by combining the corresponding atmospheric net LW and SW radiation entropy fluxes. Figure 5b shows the vertical profile of the atmospheric net radiation entropy flux when the overall atmospheric LW optical depth is equal to 2.0, 3.0 or 4.0. Obviously, the atmospheric net radiation entropy flux increases with altitude. A darker atmosphere (i.e. with larger overall atmospheric LW optical depth) corresponds to a smaller atmospheric net radiation entropy flux at all altitudes. The atmospheric net radiation entropy flux at TOA is equal to 1.01, 0.99 or 0.97 W m⁻² K⁻¹, respectively, corresponding to the overall atmospheric LW optical depth 2.0, 3.0 or 4.0.

By subtracting the corresponding atmospheric net radiation entropy flux at the bottom of the atmosphere (i.e. 0.34, 0.29 or 0.26 W m⁻² K⁻¹, respectively), we obtain the total entropy production rate within the Earth's atmosphere, being 0.67, 0.70 or 0.71 W m⁻² K⁻¹ corresponding to the overall atmospheric LW optical depth 2.0, 3.0 or 4.0. If we further add the Earth's reflected TOA SW radiation entropy flux 0.11 W m⁻² K⁻¹ (i.e. the entropy production rate from the overall 30 per cent Earth's reflection of incident solar radiation according to Wu & Liu (in press)), the total entropy production rate of the Earth's atmosphere is, respectively, 0.78, 0.81 and 0.82 W m⁻² K⁻¹ when the overall atmospheric LW optical depth is 2.0, 3.0 and 4.0, respectively. Furthermore, the entropy production from the Earth's surface radiative transfer processes (i.e. SW and LW radiation absorptions and the Earth's surface (blackbody) LW radiation emission) must be included in order to obtain the total entropy production rate from the Earth system. The entropy production rate from the Earth's surface radiative transfer processes can be calculated by 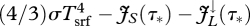being, respectively, 0.34, 0.34 or 0.37 W m⁻² K⁻¹ corresponding to the overall atmospheric LW optical depth 2.0, 3.0 or 4.0. Thus, the total entropy production rate from the Earth system contributing to the entropy increase of the universe from this simple model is equal to 1.12, 1.15 or 1.19 W m⁻² K⁻¹, respectively, corresponding to the overall atmospheric LW optical depth 2.0, 3.0 or 4.0. Notice especially that the entropy flux from the Earth's surface (blackbody) radiation emission is slightly larger than that from surface air (greybody) upward radiation emission (see figure 1 for an example).

4. Conclusions

A new one-dimensional radiative equilibrium model is built that allows analytical evaluation of the vertical profile of the atmospheric radiation entropy flux in addition to the atmospheric temperature and radiation energy flux, by introducing an effective LW emissivity for the whole atmosphere and applying the best approximate expressions for calculating non-blackbody radiation entropy flux. Further analysis of the results from this model shows that both atmospheric SW and net LW radiation entropy fluxes increase with altitude and the latter is one order in magnitude greater than the former, which are consistent with the results from previous study (Li et al. 1994). It is striking that in this simple radiative equilibrium model, even with the same atmospheric SW radiation energy deposited (absorbed) at each altitude z (see equation (2.7) combined with equations (2.33) and (2.6)), a darker atmosphere with a larger overall atmospheric LW optical depth τ_* leads to a smaller atmospheric net radiation entropy flux at all altitudes.

It should be emphasized that, to the best of the authors' knowledge, it is the first time that the effective atmospheric LW emissivity is introduced into a radiative equilibrium model and a diluted blackbody is applied to SW radiation for calculating the vertical profile of the atmospheric SW radiation entropy flux. The former emissivity introduction not only eliminates the surface thermal discontinuity problem that has long bothered similar one-dimensional vertical climate models, but also provides a natural link between atmospheric radiation entropy flux and atmospheric opacity. The latter dilution application yields a simple analytical evaluation of the vertical atmospheric SW radiation entropy flux. As discussed in Wu & Liu (in press), a diluted blackbody is often applied to treat processes like ‘radiation dilution’, such as scattering and absorption, and the dilution factors could embody reflectivity, absorptivity or their combination. Of course, a dilution process causes entropy increase (e.g. Wu & Liu in press). A simple check for the model's general ability shows that this new model is capable of simulating the basic vertical profiles of the atmospheric temperature and radiation energy flux reasonably well. In addition, the analytical solution from this new radiative equilibrium model about the vertical profiles of the atmospheric temperature and radiation energy flux as well as the effective atmospheric LW emissivity can be easily obtained as a function of the atmospheric altitude (or LW optical depth). Furthermore, the analytical solution is in simple form and thus easy to be used for other applications.

It is noteworthy that the results obtained from this study reveal that the atmospheric net radiation entropy flux at all altitudes is intrinsically connected with the overall atmospheric LW optical depth, which further implies the sensitivity of the atmospheric net entropy flux (or production rate) to greenhouse gases (i.e. increased overall atmospheric LW optical depth). Application of this new model to the study of climate change is underway. Also noted is that processes such as SW scattering and clouds have not been explicitly accounted for in this simple radiative equilibrium model. Future effort will be to generalize this model to consider the atmospheric SW scattering processes within the atmosphere and cloud-related processes such as convection, and examine their roles in determining atmospheric energy and entropy profiles.